Interior Dynamics of Neutral and Charged Black Holes in f(R) Gravity
Abstract
:1. Introduction
1.1. Mass Inflation
- (1)
- Neutral collapse: neutral scalar collapse toward a black hole formation.
- (2)
- Neutral scattering: neutral scalar collapse in a (neutral) Schwarzschild geometry.
- (3)
- Charge scattering: neutral scalar collapse in a (charged) Reissner-Nordström geometry. In this process, the scalar field is scattered by the inner horizon of a Reissner-Nordström black hole.
1.2. New Results
- (1)
- Type I: spacelike scattering. When the scalar field is very strong, the inner horizon can contract to zero volume rapidly, and the central singularity becomes spacelike. The dynamics near the spacelike singularity is similar to that in neutral collapse.
- (2)
- Type II: null scattering. When the scalar field is intermediate, the inner horizon can contract to a place close to the center or reach the center. For each variable (the metric elements and physical scalar field), the spatial and temporal derivatives are almost equal. In the case of the center being reached, the central singularity is null. This type has two stages: early/slow and late/fast. In the early stage, the inner horizon contracts slowly, and the scalar field also varies slowly. In the late stage, the inner horizon contracts quickly, and the dynamics is similar to that in the spacelike case.
- (3)
- Type III: critical scattering. This case is on the edge between the above two cases. The central singularity becomes null.
- (4)
- Type IV: weak scattering. When the scalar field is very weak, the inner horizon contracts but not much. Then the central singularity remains timelike.
- (5)
- Type V: tiny scattering. When the scalar field is very tiny, the influence of the scalar field on the internal geometry is negligible.
2. Framework
2.1. Action
2.2. Theory
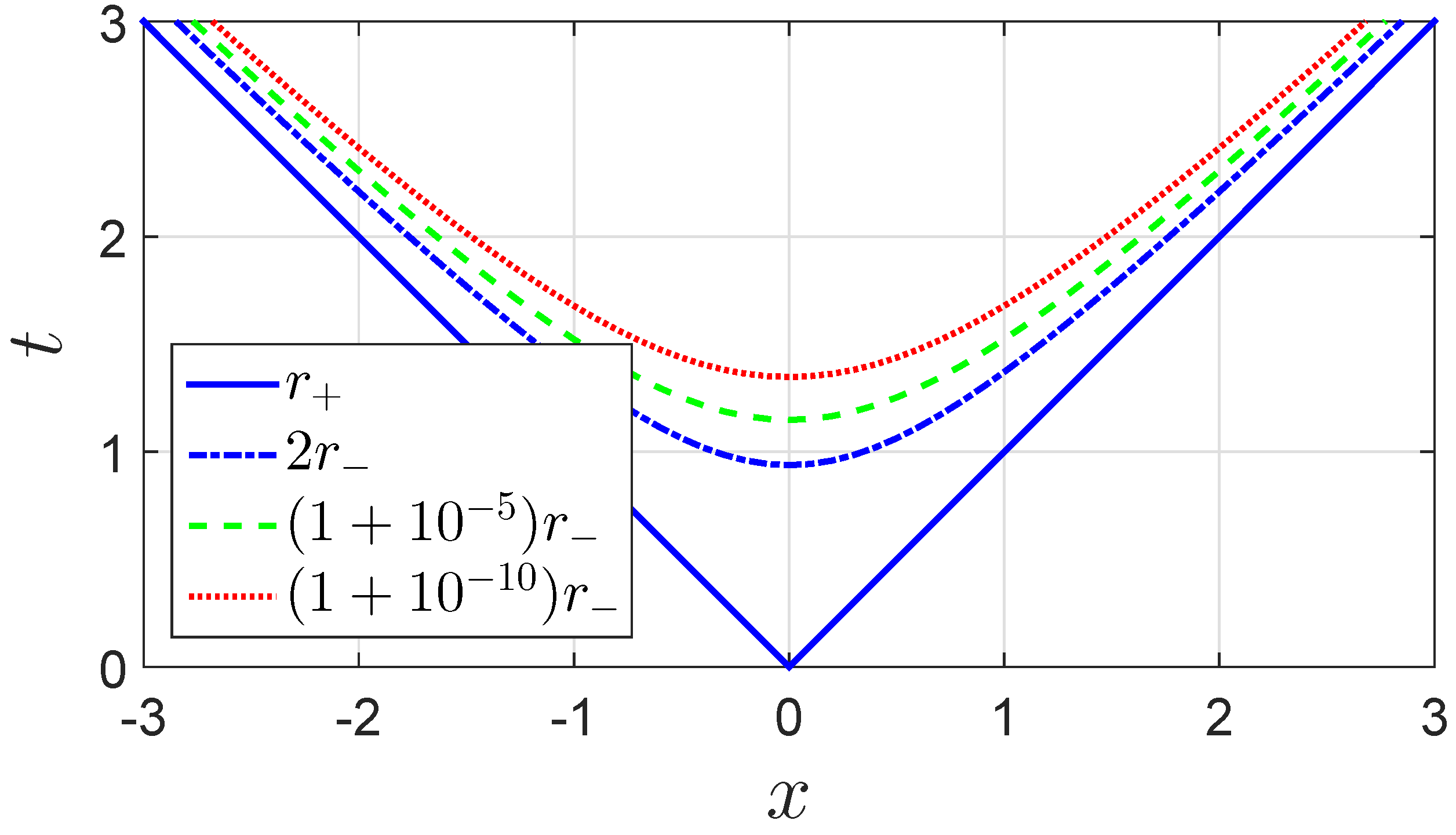
2.3. Coordinate System

- (1)
- In the line element Equation (26), one coordinate is timelike and the rest are spacelike. This is a conventional setup. It is more convenient and more intuitive to use this set of coordinates. For the set of coordinates described by Equation (25), in the equations of motion, many terms are mixed derivatives of u and v; while for the set of coordinates described by Equation (26), in the equations of motion, spatial and temporal derivatives are usually separated.
- (2)
- We set initial conditions close to those in the Reissner-Nordström metric. Consequently, with the terms related to the scalar fields being removed, we can test our code by comparing the numerical results to the analytic ones in the Reissner-Nordström case conveniently. Moreover, by comparing dynamics for scalar collapse to that in the Reissner-Nordström case, we can obtain intuitions on how the scalar fields affect the geometry.
- (3)
- The interactions between scalar fields and the geometry are local effects. In References [29,30], the space between the inner and outer horizons are compactified into finite space. This overcompactification, at least to us, makes it a bit hard to understand the dynamics. In the configuration that we choose, the space is partially compactified, and the picture of charge scattering turns out to be simpler.
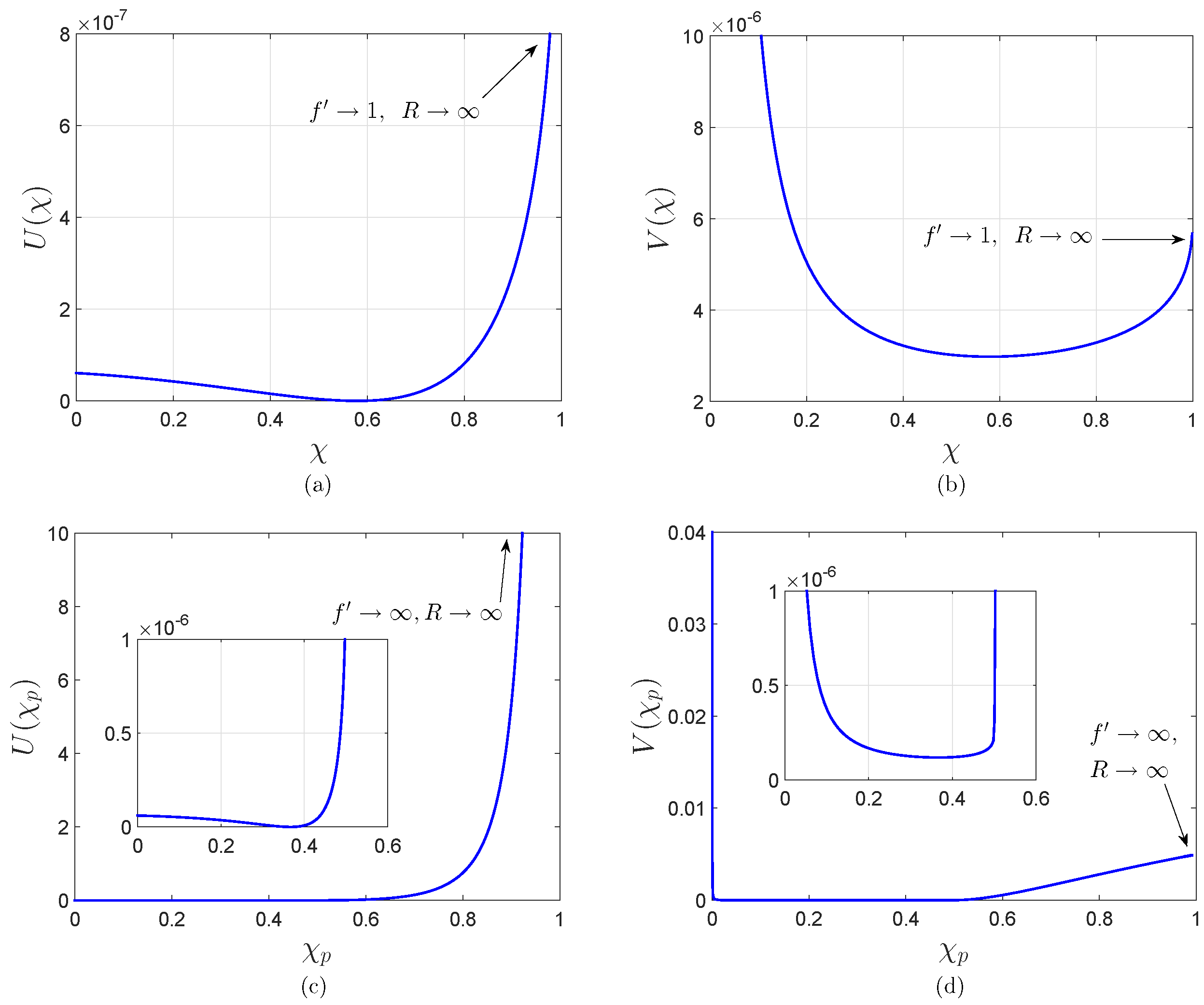
2.4. Model
3. Numerical Setup for Charge Scattering
3.1. Field Equations
3.2. Initial Conditions
3.3. Boundary Conditions
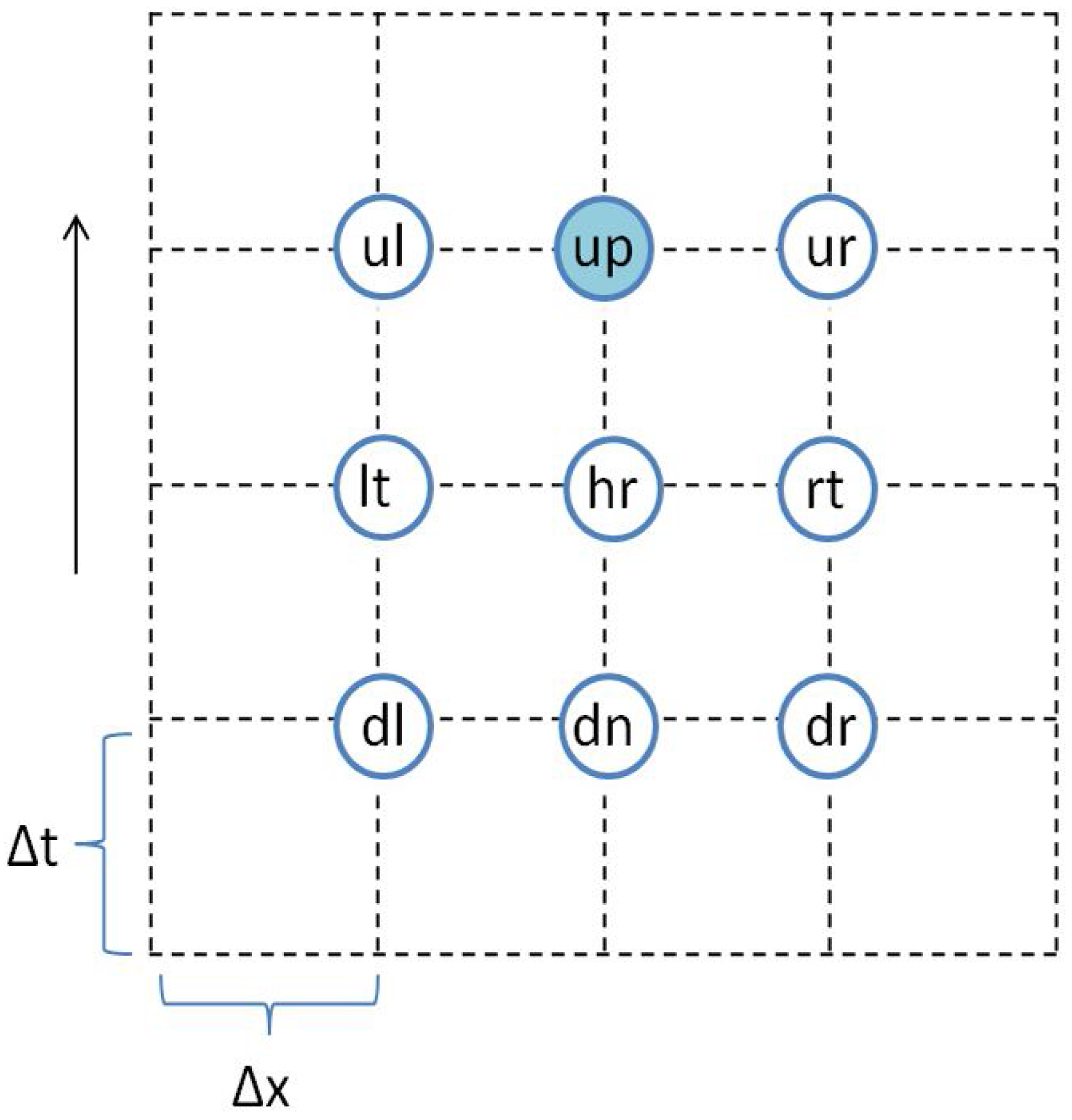
3.4. Discretization Scheme
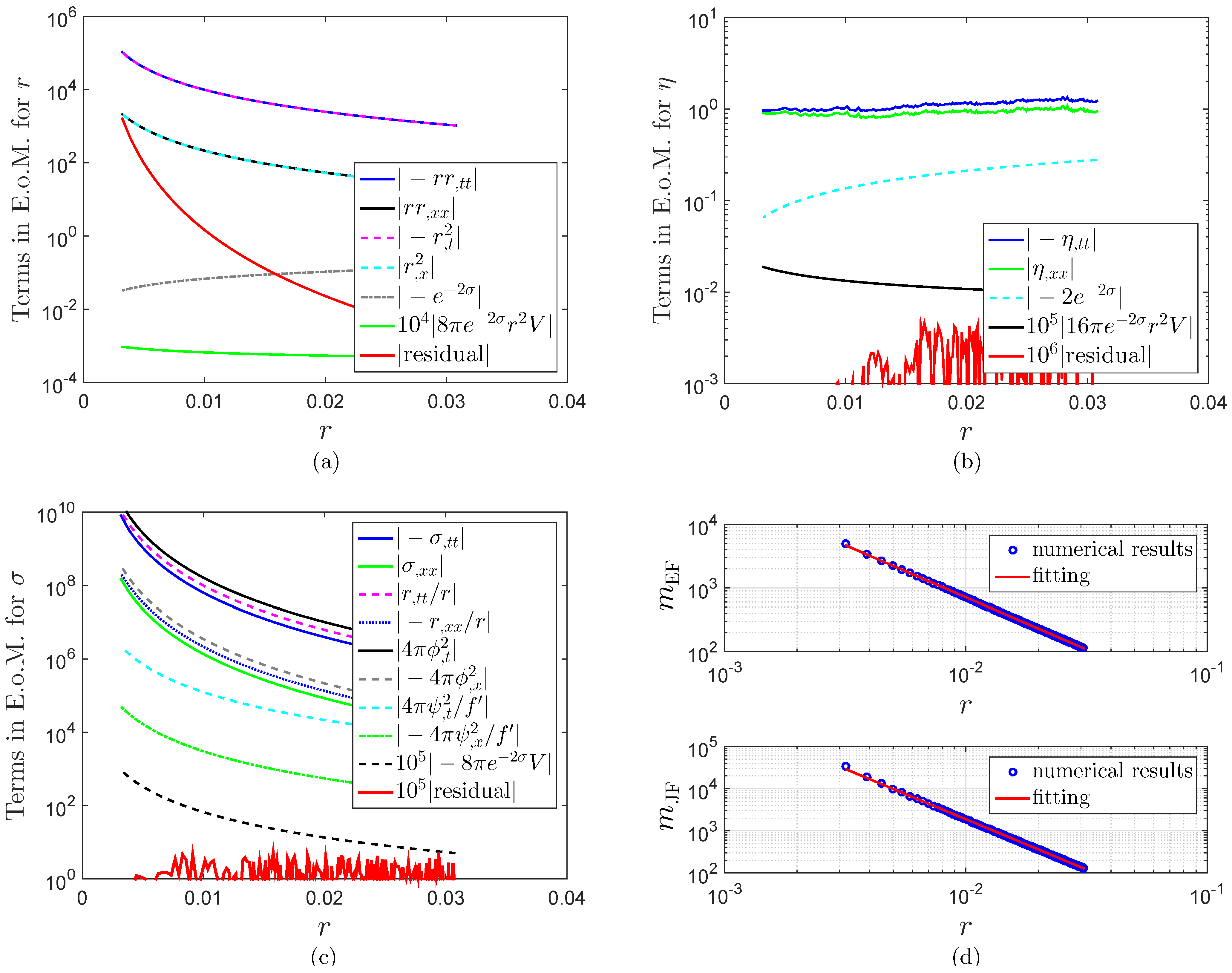
3.5. Tests of Numerical Code

4. Neutral Scalar Collapse
4.1. Numerical Setup
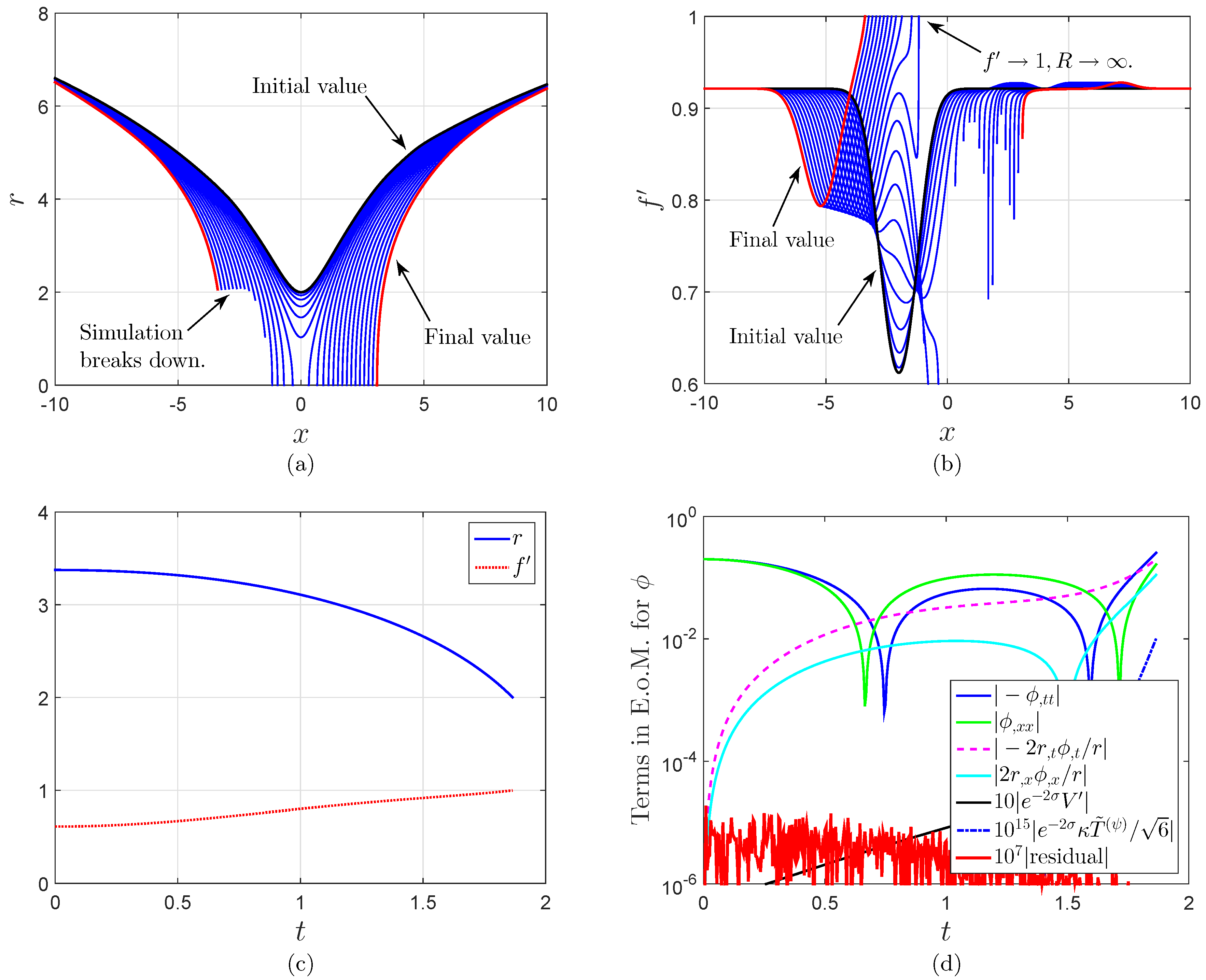
4.2. Black Hole Formation
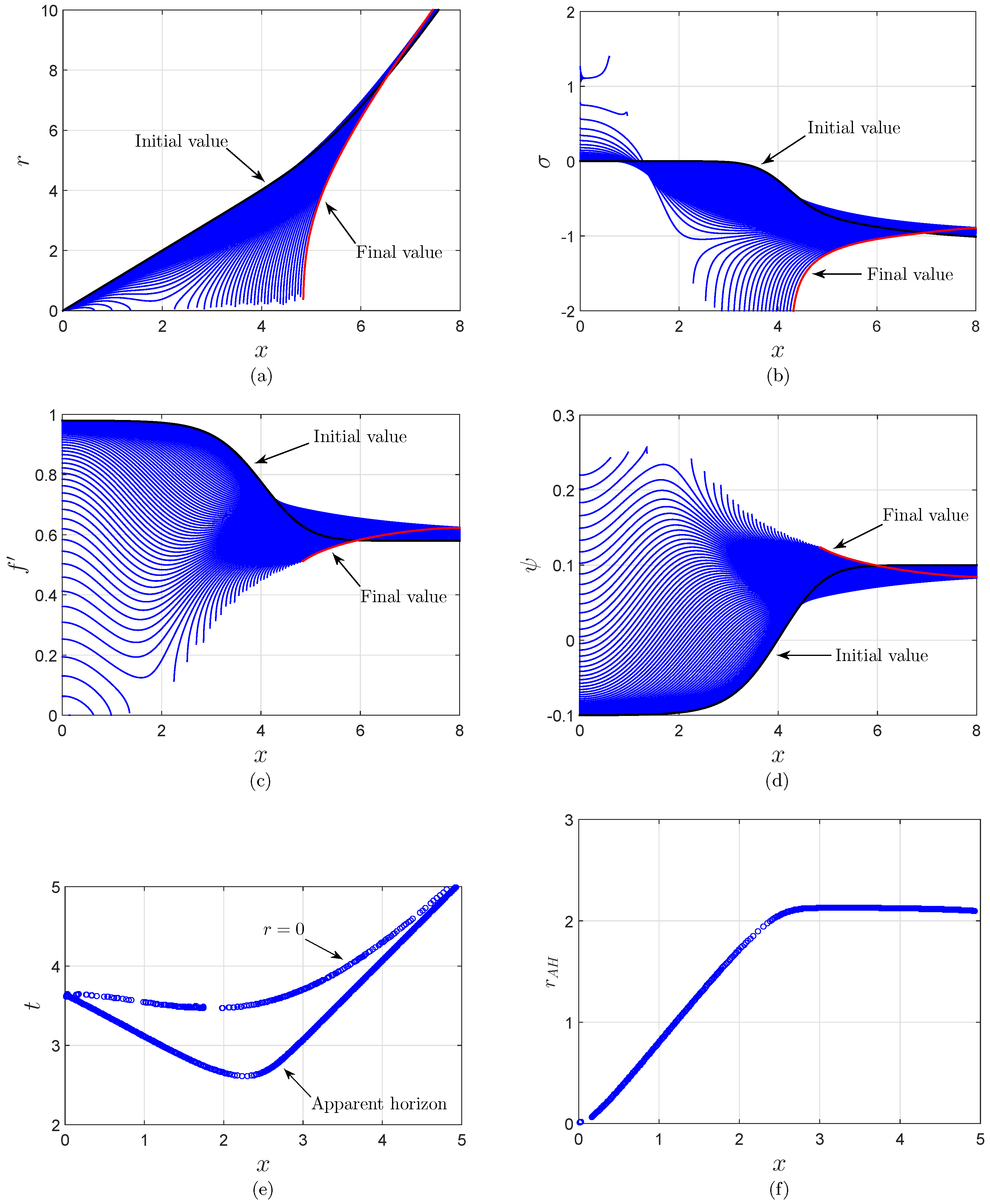
4.3. Asymptotic Dynamics in the Vicinity of the Central Singularity of the Formed Black Hole
4.4. Mass Inflation
- (1)
- Values of σ. Due to the backreaction of the scalar fields on the geometry, σ in small-x regions is greater than in large-x regions. In fact, σ is positive in small-x regions, while negative in large-x regions. (See Figure 6b). Our numerical results of the parameter C in are at and at . Then we have and at , while and at . (See Equation (72)).
- (2)
- (3)

5. Neutral Scalar Scattering
5.1. A Dark Energy Singularity Problem
- (1)
- Schwarzschild geometry: .
- (2)
- Physical scalar field: , , , and .
- (3)
- model: , , and .
- (4)
- Scalar degree of freedom: , , , , and .
- (5)
- Grid. Spatial range: . Grid spacings: .
5.2. Avoidance of the Singularity Problem

6. Results for Charge Scattering
- (1)
- Reissner-Nordström geometry: , and .
- (2)
- Physical scalar field: , , , and .
- (3)
- model: , , and .
- (4)
- Scalar degree of freedom: , with .
- (5)
- Grid. Spatial range: . Grid spacings: for Section 6.1 and Section 6.2, and for Section 6.3 and Section 6.4.
6.1. Evolutions
6.1.1. Outline
- (1)
- Metric components: r and σ. They contribute as gravity.
- (2)
- Scalar fields: ϕ and ψ. They contribute as self-gravitating fields.
- (3)
- Electric field and . As implied in Equation (33), they are repulsive forces. However, the numerical results show that in charge scattering, compared to the contributions from other quantities, the contribution from is negligible.

- (1)
- Type I: spacelike scattering. When the scalar field is very strong, the inner horizon can contract to zero volume rapidly, and the central singularity becomes spacelike. Sample slice: in Figure 11. See Section 6.2.
- (2)
- Type II: null scattering. When the scalar field is intermediate, the inner horizon can contract to a place close to the center or reach the center. For each variable, the spatial and temporal derivatives are almost equal. In the case of the center being reached, the central singularity becomes null. This type has two stages: early/slow and late/fast. In the early stage, the inner horizon contracts slowly, and the scalar field also varies slowly. In the late stage, the inner horizon contracts quickly, and the dynamics is similar to that in the spacelike case. Sample slice: in Figure 11. See Section 6.3 and Section 6.4.
- (3)
- Type III: critical scattering. This case is on the edge between the above two cases. When the central singularity is reached, it becomes null. Sample slice: in Figure 11. Due to the similarity to that in general relativity discussed in Reference [41], details on this type of scattering in gravity are skipped in this paper.
- (4)
- (5)
- Type V: tiny scattering. When the scalar field is very tiny, the influence of the scalar field on the geometry is negligible. Sample slice: in Figure 11.
6.1.2. Causes of Mass Inflation and Evolutions
6.1.3. Locations of Horizons
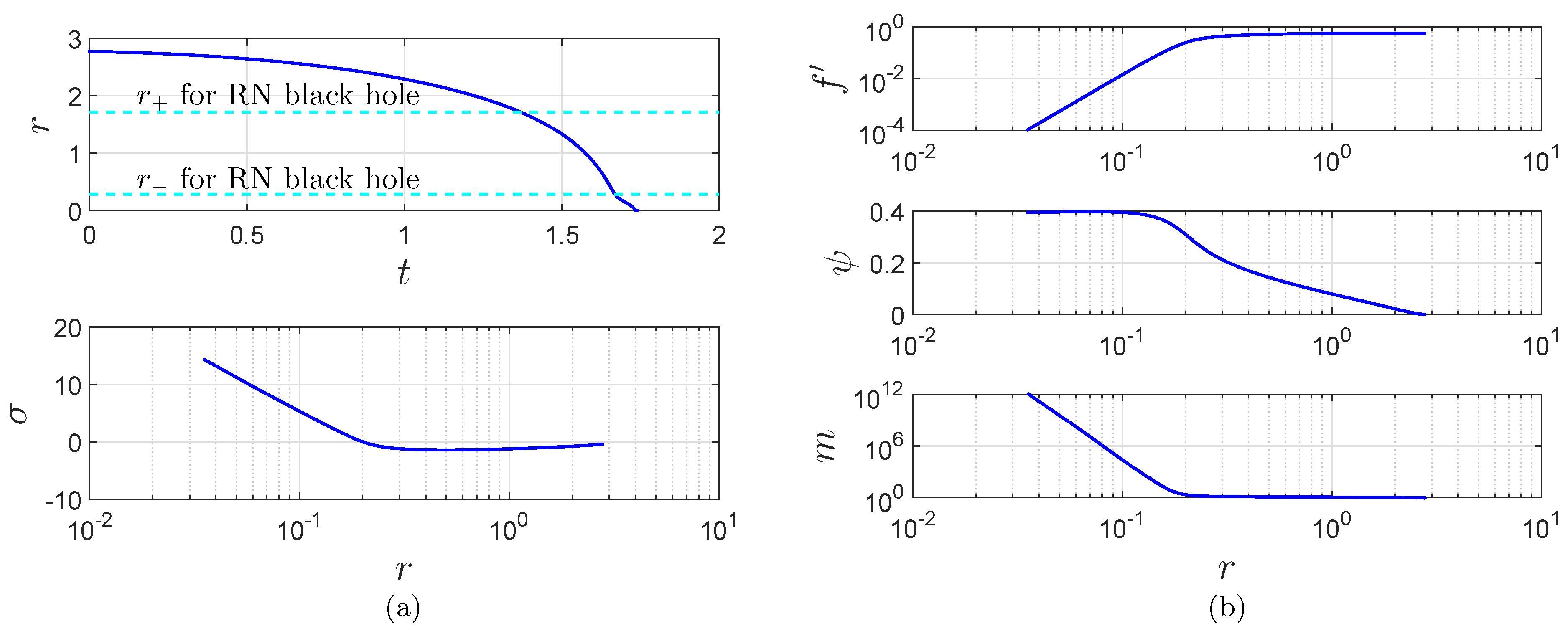
6.2. Spacelike Scattering
- (1)
- Motion of r. The quantity r does not decelerate much when it crosses the inner horizon of the given Reissner-Nordström black hole, and it can approach the center. (See Figure 12).
- (2)
- Nature of the central singularity. The central singularity converts from timelike into spacelike.
- (3)
- The dynamics in spacelike scattering is similar to that in strong, neutral scalar collapse. The quantity σ takes large positive values, such that in the vicinity of the central singularity, compared to other terms, the term in the equation for r Equation (33) and the term in the equation for σ Equation (35) are negligible. As a result, in the vicinity of the central singularity, the dynamics is similar to that in strong, neutral scalar collapse as expressed by Equations (67)–(70). (See Figure 7 and Figure 13). Then the quantities r, σ, ϕ, and m take similar forms as those in neutral collapse. (See Figure 14).In both strong, neutral scalar collapse and spacelike scattering, the equation of motion for η is reduced to . (See Figure 7b and Figure 13b).Since is negative, the term in Equation (37) functions as a friction force for ψ. Consequently, compared to ϕ, ψ grows slowly. As the singularity is approached, ψ even approaches constant values. (See Figure 14c,d). As shown in Figure 13e, near the singularity, two major sets of terms can be expressed asAlternatively,
- (4)
- Growth of mass function.In spacelike scattering, the equation of motion for ϕ can be simplified asConsequently, with the results obtained in Section 4.3, σ has the following asymptotic solution:with . Then similar to the mass function Equation (76) in neutral collapse, with Equaution Equation (81), the mass function in spacelike scattering can be written asNumerical results show that, for the sample slice , near the central singularity, the slope of the singularity curve K is about . As shown in Figure 14d, we linearly fit the numerical results of m viaobtainingFitting numerical results for σ according to Equation (87) and combining Equations (88) and (89), we obtainSimilarly, fitting numerical results for ϕ according to , we obtainOne can see that the above three sets of results match well.
6.3. The Late/fast Stage of Null Scattering
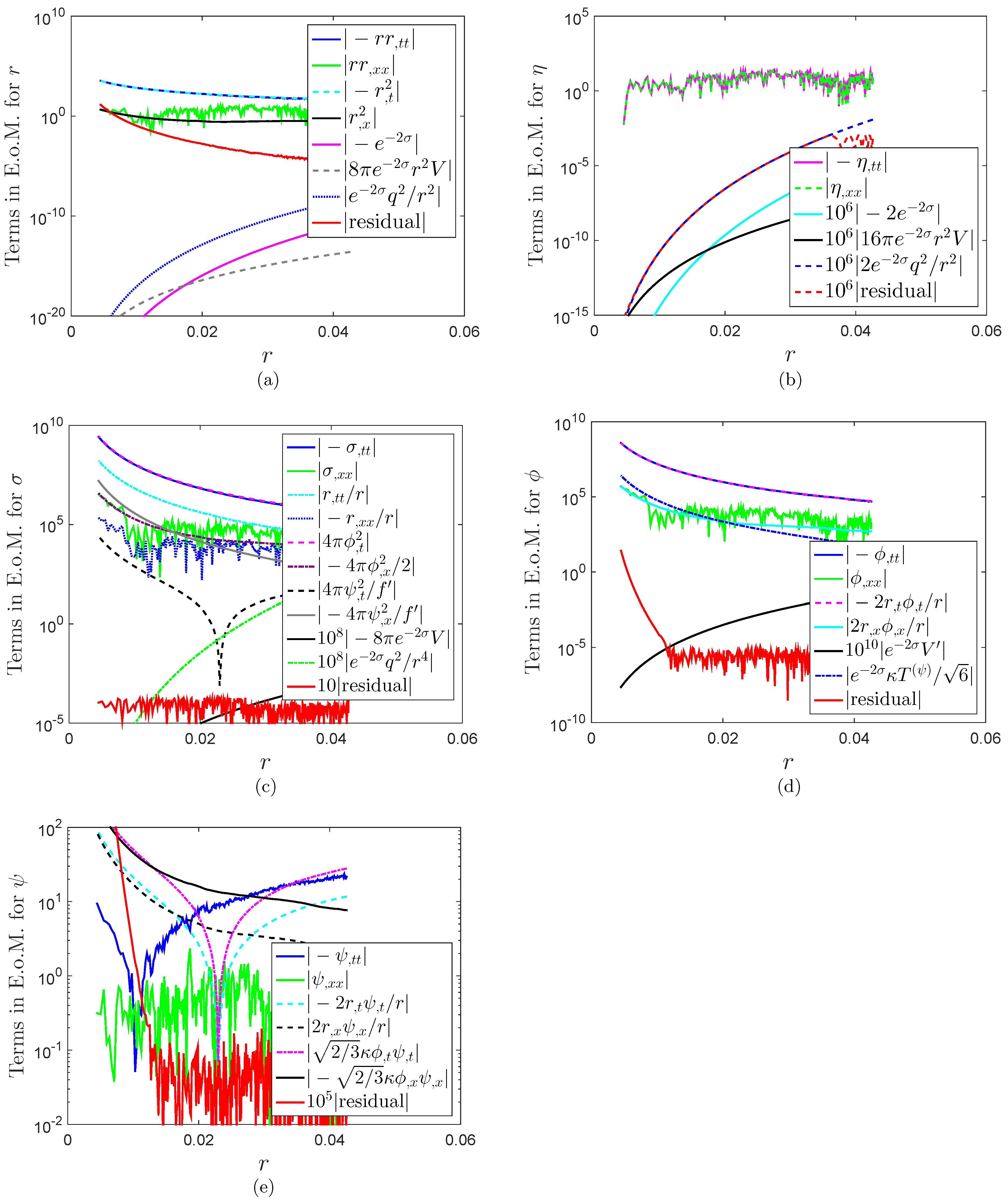



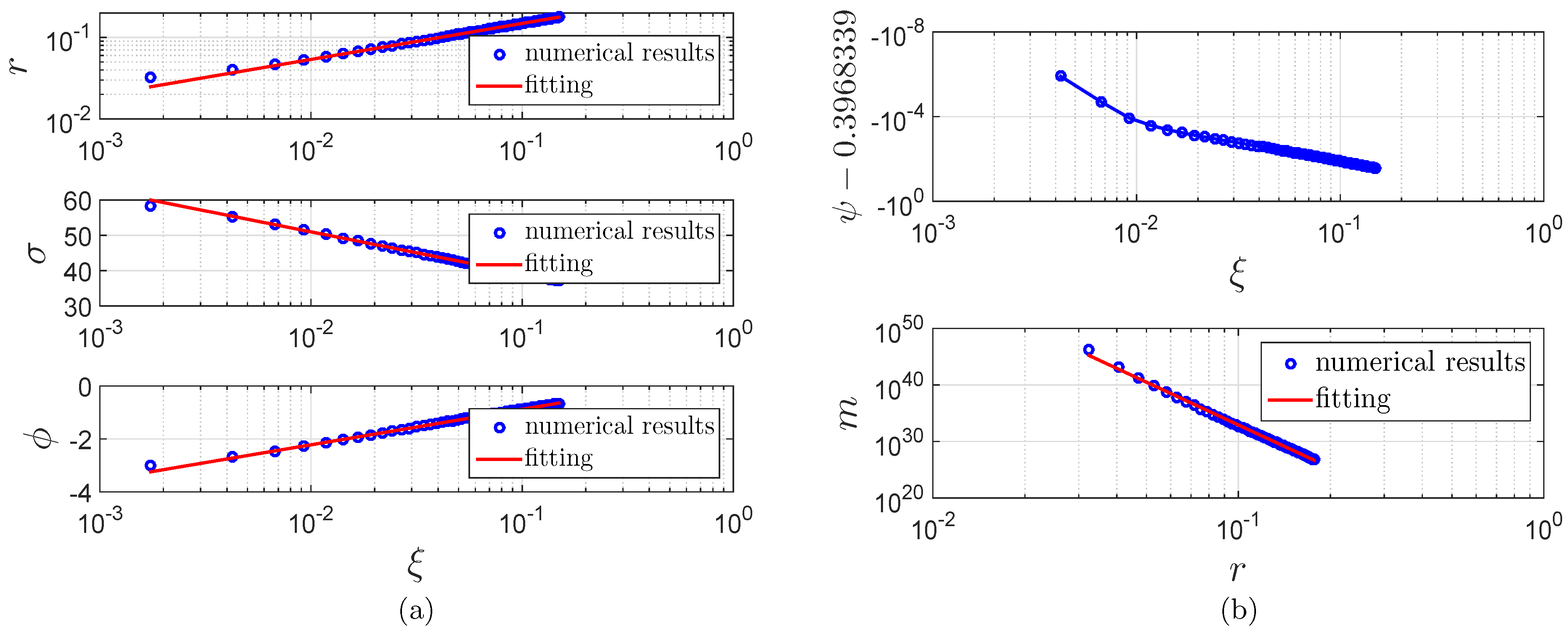
6.4. The Early/slow Stage of Null Scattering

- (1)
- , , , , .
- (2)
- , , , .
- (3)
- , , , , .
- (4)
- , , , , .
- (5)
- , , .
- (6)
- , , , .
7. Weak Scalar Charge Scattering
- (1)
- Physical scalar field: , , , and .
- (2)
- Grid. Spatial range: . Grid spacings: .


8. Dark Energy Singularity Problem in Charge Scattering
- (1)
- Grid spacings: .
- (2)
- Physical scalar field ψ: , , , and .
- (3)
- Scalar degree of freedom ϕ: , with , , and .
- (4)
- model Equation (109): , , and .


9. Summary
9.1. Computational Issues
- (1)
- The Jordan frame vs the Einstein frame. The field equations for gravity in the Jordan frame are more complex than those in general relativity. Therefore, for ease of computation, we transform gravity from the Jordan frame into the Einstein frame, in which the formalism can be formally treated as Einstein gravity coupled to a scalar field.
- (2)
- vs. in double-null coordinates. In the studies of mass inflation, the format of the Kruskal-like coordinates, , is usually used. In the field equations, many terms are mixed derivatives of u and v, e.g., . In this paper, we used the format instead, , with and . In the line element, one coordinate is timelike, and the rest are spacelike. We are used to this setup. It is more convenient and more intuitive to use this set of coordinates. Moreover, for the choice, spatial and temporal derivatives are usually separated, e.g., .We set the initial conditions close to those in a Reissner-Nordström geometry. With this setup, it is convenient to test the code. Removing the terms related to the scalar fields, we can test our code by comparing the numerical results to the analytic ones in a Reissner-Nordström geometry. Moreover, by comparing numerical results for charge scattering to the dynamics in the Reissner-Nordström geometry, we can obtain intuitions as to how the scalar fields affect the geometry.
- (3)
- Cauchy horizon: infinite or local regions? As implied by Equation (28), the exact inner horizon is at the regions where and are infinite. However, r still can be very close to the inner horizon even when and take moderate values. Consequently, at regions where and take some moderate values, the scalar fields and the inner horizon still can have strong interactions, resulting in mass inflation.
9.2. Physical Issues
- (1)
- Scalar collapse in gravity vs scalar collapse in general relativity. In scalar collapse, the scalar degree of freedom plays a similar role as a physical scalar field in general relativity. Regarding the physical scalar field in the case, when is negative (positive), the physical scalar field is suppressed (magnified) by ϕ.
- (2)
- The inner horizon in a Reissner-Nordström black hole vs the central singularity in a Schwarzschild black hole. These two share some similarities.For Reissner-Nordström and Schwarzschild black holes, throughout the whole spacetime, the Misner-Sharp mass function is constant. When a scalar field impacts the inner horizon of a Reissner-Nordström black hole, the scalar field can modify the geometry in the vicinity of the inner horizon significantly, especially on . The inner horizon contracts and mass inflation takes place. In neutral scalar collapse toward a Schwarzschild black hole formation, the scalar field can also modify the geometry in the vicinity of the central singularity dramatically, especially on the metric component σ [20]. Then mass inflation also happens.The Belinskii, Khalatnikov, and Lifshitz (BKL) conjecture is an important result on dynamics in the vicinity of a spacelike singularity [76,77,78,79]. The first statement of this conjecture is that as the singularity is approached, the dynamical terms dominate the spatial terms in the field equations. In other words, the way gravity changes over time is more important than the variation of the gravitational field from one location to the next [79]. We would like to say that, to a large extent, later evolutions in a strong gravitational field largely erase away the initial information on the connections between neighboring points. As discussed in Reference [20] and also in this paper, in double-null coordinates, using the above argument, one can interpret the following behaviors displayed in numerical simulations: near the central singularity of a Schwarzschild black hole and also near the inner horizon of a Reissner-Nordström black hole, there areIn this paper, it was shown that Equation (110) can explain the causes of mass inflation, while Equation (111) can explain the dark energy singularity problem in collapse.The second and third statements of the BKL conjecture are that i) the metric terms will dominate the matter field terms, while the matter field may not be negligible if it is a scalar field; ii) the dynamics of the metric components and the matter fields is described by the Kasner solution. These two statements were confirmed in simulations of neutral scalar collapse in gravity in Reference [20] and in general relativity in Reference [41]. The second statement was also verified in charge scattering in this paper. However, the third statement on Kasner solution may not apply to the dynamics near the inner horizon in charge scattering.
- (3)
- Compact stars vs black holes in gravity. The internal structure of compact stars is usually in an equilibrium state and is static. In gravity, inside compact stars, the scalar degree of freedom, , can be coupled to the energy density of the stars and then is not very free to move. However, due to strong gravity, the internal structure of black holes is dynamical. and the matter fields are decoupled. As a result, is more free to move than in the compact stars case. It can keep increasing or decreasing until singularities are met.
- (4)
- Dark energy singularity problem: cosmology (or static compact objects) vs black hole physics. In dark energy gravity, the Ricci scalar R can be singular in both cosmology and black hole physics. We consider a homogeneous cosmological model. Using the flat Friedmann-Robertson-Walker metric,the equation of motion for iswhere H is the Hubble parameter. Due to the finiteness of the potential barrier, the force from a perturbation of may push to 1. Correspondingly, the Ricci scalar goes to singularity [72]. In the black hole case that we discussed in this paper, the equation of motion for has more complex structure Equation (36),As the central singularity of a Schwarzschild black hole or the inner horizon of a Reissner-Nordström black hole is approached, the above equation can be simplified as Equation (111), and gravity from the black hole, , can cause a similar singularity problem as in cosmology or static compact stars.
- (5)
- The combined model and the model: singular or non-singular? At the center of a Schwarzschild black hole and in the very early Universe, the tidal forces are singular, and general relativity fails. Taking into account quantum-gravitational effects, Starobinsky obtained an model, . This model has a non-singular de Sitter solution, which is unstable both to the past and to the future [42,43]. In Section 5, scalar collapse in a Schwarzschild geometry for the combined model (a combination of dark energy model and model) was explored. A new Schwarzschild black hole, including a new central singularity, can be formed. Moreover, under certain initial conditions, and R can be pushed to infinity as the central singularity is approached. In Reference [73], scalar collapse in flat geometry for the model was simulated. Similar results were obtained. Namely, the classical singularity problem, which is present in general relativity, remains in collapse in these models.
- (6)
- Inside vs. outside black holes: local vs global. Throughout the whole spacetime of stationary Schwarzschild and Reissner-Nordström black holes, the Misner-Sharp mass function is equal to the black hole mass. For a gravitational collapsing system, at asymptotic flat regions, the mass function describes the total mass of the dynamical system. However, in this system, near the central singularity of a Schwarzschild black hole or near the inner horizon of a Reissner-Nordström black hole, the dynamics is local. Then the mass function does not provide global information on the mass of the collapsing system.
Acknowledgments
Author Contributions
Conflicts of Interest
A. Equations of Motion for a Physical Scalar Field and in the Einstein Frame
B. Reissner-Nordström Metric in Kruskal-Like Coordinates
B.1. Patch I:
B.2. Patch II:
C. Einstein Tensor and Energy-Momentum Tensor for a Massive Scalar Field
References
- Burko, L.M.; Ori, A. (Eds.) Internal Structure of Black Holes and Spacetime Singularities; Institute of Physics Publishing: Bristol, UK, 1998.
- Brady, P.R. The Internal Structure of Black holes. Prog. Theor. Phys. Suppl. 1999, 136, 29–44. [Google Scholar] [CrossRef]
- Berger, B.K. Numerical Approaches to Spacetime Singularities. Living Rev. Relativ. 2002, 5, 1. [Google Scholar] [CrossRef]
- Joshi, P.S. Gravitational Collapse and Spacetime Singularities; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Henneaux, M.; Persson, D.; Spindel, P. Spacelike Singularities and Hidden Symmetries of Gravity. Living Rev. Relativ. 2008, 11, 1. [Google Scholar] [CrossRef]
- Price, R.H. Nonspherical Perturbations of Relativistic Gravitational Collapse. I. Scalar and Gravitational Perturbations. Phys. Rev. D 1972, 5, 2419–2438. [Google Scholar] [CrossRef]
- Clifton, T.; Ferreira, P.G.; Padilla, A.; Skordis, C. Modified gravity and cosmology. Phys. Rep. 2012, 513, 1–189. [Google Scholar] [CrossRef]
- Sotiriou, T.P.; Faraoni, V. f(R) Theories Of Gravity. Rev. Mod. Phys. 2010, 82, 451–497. [Google Scholar] [CrossRef]
- Felice, A.D.; Tsujikawa, S. f(R) Theories. Living Rev. Relativ. 2010. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S. D. Unified cosmic history in modified gravity: From F(R) theory to Lorentz non-invariant models. Phys. Rept. 2011, 505, 59–144. [Google Scholar] [CrossRef]
- Capozziello, S.; de Laurentis, M. Extended Theories of Gravity. Phys. Rept. 2011, 509, 167–321. [Google Scholar] [CrossRef]
- Cruz-Dombriz, A.; de la Dobado, A.; Maroto, A.L. Black Holes in f(R) theories. Phys. Rev. D 2009, 80, 124011. [Google Scholar] [CrossRef]
- Olmo, G.J.; Rubiera-Garcia, D. Palatini f(R) Black Holes in Nonlinear Electrodynamics. Phys. Rev. D 2011, 84, 124059. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Anti-Evaporation of Schwarzschild-de Sitter Black Holes in F(R) gravity. Class. Quantum Gravity 2013, 30, 125003. [Google Scholar] [CrossRef]
- Sebastiani, L.; Momeni, D.; Myrzakulov, R.; Odintsov, S.D. The instabilities and (anti)-evaporation of Schwarzschild-de Sitter black holes in modified gravity. Phys. Rev. D 2013, 88, 104022. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Instabilities and Anti-Evaporation of Reissner-Nordström Black Holes in modified F(R) gravity. Phys. Lett. B 2014, 735, 376–382. [Google Scholar] [CrossRef]
- Avelino, P.P.; Hamilton, A.J.S.; Herdeiro, C.A.R. Mass Inflation in Brans-Dicke gravity. Phys. Rev. D 2009, 79, 124045. [Google Scholar] [CrossRef]
- Borkowska, A.; Rogatko, M.; Moderski, R. Collapse of Charged Scalar Field in Dilaton Gravity. Phys. Rev. D 2011, 83, 084007. [Google Scholar] [CrossRef]
- Hwang, D.-I.; Lee, B.-H.; Yeom, D.-H. Mass inflation in f(R) gravity: A conjecture on the resolution of the mass inflation singularity. J. Cosmol. Astropart. Phys. 2011, 2011, 006. [Google Scholar] [CrossRef]
- Guo, J.-Q.; Wang, D.; Frolov, A.V. Spherical collapse in f(R) gravity and the Belinskii-Khalatnikov-Lifshitz conjecture. Phys. Rev. D 2014, 90, 024017. [Google Scholar] [CrossRef]
- Simpson, M.; Penrose, R. Internal instability in a Reissner-Nordström black hole. Int. J. Theor. Phys. 1973, 7, 183–197. [Google Scholar] [CrossRef]
- Dafermos, M. Stability and instability of the Cauchy horizon for the spherically symmetric Einstein-Maxwell-scalar field equations. Ann. Math. (N. Y.) 2003, 158, 875–928. [Google Scholar] [CrossRef]
- Dafermos, M. Black holes without spacelike singularities. Commun. Math. Phys. 2014, 332, 729–757. [Google Scholar] [CrossRef]
- Ringström, H. Origins and development of the Cauchy problem in general relativity. Class. Quantum Gravity 2015, 32, 124003. [Google Scholar] [CrossRef]
- Isenberg, J. On Strong Cosmic Censorship. 2015; arXiv:1505.06390[gr-qc]. [Google Scholar]
- Poisson, E.; Israel, W. Inner-horizon instability and mass inflation in black holes. Phys. Rev. Lett. 1989, 63, 1663–1666. [Google Scholar] [CrossRef] [PubMed]
- Poisson, E.; Israel, W. Internal structure of black holes. Phys. Rev. D 1990, 41, 1796–1809. [Google Scholar] [CrossRef]
- Barrabes, C.; Israel, W.; Poisson, E. Collision of light-like shells and mass inflation in rotating black holes. Class. Quantum Gravity 1990, 7, L273–L278. [Google Scholar] [CrossRef]
- Gnedin, N.Y.; Gnedina, M.L. Instability of the internal structure of a Reissner-Nordström black hole. Sov. Astron. 1992, 36, 296, (Astron. Zh. 1992, 69, 584–592.). [Google Scholar]
- Gnedin, M.L.; Gnedin, N.Y. Destruction of the Cauchy horizon in the Reissner-Nordstrom black hole. Class. Quantum Gravity 1993, 10, 1083–1102. [Google Scholar] [CrossRef]
- Brady, P.R.; Smith, J. Black hole singularities: A numerical approach. Phys. Rev. Lett. 1995, 75, 1256–1259. [Google Scholar] [CrossRef] [PubMed]
- Burko, L.M. Structure of the black hole’s Cauchy horizon singularity. Phys. Rev. Lett. 1997, 79, 4958–4961. [Google Scholar] [CrossRef]
- Burko, L.M.; Ori, A. Late-time evolution of nonlinear gravitational collapse. Phys. Rev. D 1997, 56, 7820–7832. [Google Scholar] [CrossRef]
- Hansen, J.; Khokhlov, A.; Novikov, I. Physics of the interior of a spherical, charged black hole with a scalar field. Phys. Rev. D 2005, 71, 064013. [Google Scholar] [CrossRef]
- Hod, S.; Piran, T. Mass Inflation in Dynamical Gravitational Collapse of a Charged Scalar Field. Phys. Rev. Lett. 1998, 81, 1554–1557. [Google Scholar] [CrossRef]
- Oren, Y.; Piran, T. Collapse of charged scalar fields. Phys. Rev. D 2003, 68, 044013. [Google Scholar] [CrossRef]
- Burko, L.M.; Ori, A. Analytic study of the null singularity inside spherical charged black holes. Phys. Rev. D 1998, 57, R7084–R7088. [Google Scholar] [CrossRef]
- Burko, L.M. Strength of the null singularity inside black holes. Phys. Rev. D 1999, 60, 104033. [Google Scholar] [CrossRef]
- Hu, W.; Sawicki, I. Models of f(R) Cosmic Acceleration that Evade Solar-System Tests. Phys. Rev. D 2007, 76, 064004. [Google Scholar] [CrossRef]
- Cai, R.G.; Cao, L.M.; Hu, Y.P.; Ohta, N. Generalized Misner-Sharp Energy in f(R) Gravity. Phys. Rev. D 2009, 80, 104016. [Google Scholar] [CrossRef]
- Guo, J.-Q.; Joshi, P.S. Interior dynamics of neutral and charged black holes. 2015; arXiv:1507.01806[gr-qc]. [Google Scholar]
- Starobinsky, A.A. A new type of isotropic cosmological models without singularity. Phys. Lett. B 1980, 91, 99–102. [Google Scholar] [CrossRef]
- Vilenkin, A. Classical and quantum cosmology of the Starobinsky inflationary model. Phys. Rev. D 1985, 32, 2511–2521. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. The future evolution and finite-time singularities in F(R)-gravity unifying the inflation and cosmic acceleration. Phys. Rev. D 2008, 78, 046006. [Google Scholar] [CrossRef]
- Bamba, K.; Nojiri, S.; Odintsov, S.D. Future of the universe in modified gravitational theories: Approaching to the finite-time future singularity. J. Cosmol. Astropart. Phys. 2008, 10, 045. [Google Scholar] [CrossRef]
- Capozziello, S.; de Laurentis, M.; Nojiri, S.; Odintsov, S.D. Classifying and avoiding singularities in the alternative gravity dark energy models. Phys. Rev. D 2009, 79, 124007. [Google Scholar] [CrossRef]
- Appleby, S.A.; Battye, R.A.; Starobinsky, A.A. Curing singularities in cosmological evolution of F(R) gravity. J. Cosmol. Astropart. Phys. 2010, 06, 005. [Google Scholar] [CrossRef]
- Elizalde, E.; Nojiri, S.; Odintsov, S.D.; Sebastiani, L.; Zerbini, S. Non-singular exponential gravity: A simple theory for early- and late-time accelerated expansion. Phys. Rev. D 2011, 83, 086006. [Google Scholar] [CrossRef]
- Bamba, K.; Nojiri, S.; Odintsov, S.D. Time-dependent matter instability and star singularity in F(R) gravity. Phys. Lett. B 2011, 698, 451–456. [Google Scholar] [CrossRef] [Green Version]
- Poisson, E. A Relativist’s Toolkit: The Mathematics of Black-Hole Mechanics; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Frolov, A.V. Is It Really Naked? On Cosmic Censorship in String Theory. Phys. Rev. D 2004, 70, 104023. [Google Scholar] [CrossRef]
- Graves, J.C.; Brill, D.R. Oscillatory Character of Reissner-Nordström Metric for an Ideal Charged Wormhole. Phys. Rev. 1960, 120, 1507–1513. [Google Scholar] [CrossRef]
- Reall, H. Lecture Notes on Black Holes. Available online: http://www.damtp.cam.ac.uk/user/hsr1000/black_holes_lectures_2015.pdf (accessed on 29 August 2015).
- Nunez, A.; Solganik, S. The content of f(R) gravity. 2004; arXiv:hep-th/0403159. [Google Scholar]
- Dolgov, A.D.; Kawasaki, M. Can modified gravity explain accelerated cosmic expansion? Phys. Lett. B 2003, 573, 1–4. [Google Scholar] [CrossRef]
- Amendola, L.; Gannouji, R.; Polarski, D.; Tsujikawa, S. Conditions for the cosmological viability of f(R) dark energy models. Phys. Rev. D 2007, 75, 083504. [Google Scholar] [CrossRef]
- Guo, J.-Q.; Frolov, A.V. Cosmological dynamics in f(R) gravity. Phys. Rev. D 2013, 88, 124036. [Google Scholar] [CrossRef]
- Khoury, J.; Weltman, A. Chameleon Fields: Awaiting Surprises for Tests of Gravity in Space. Phys. Rev. Lett. 2004, 93, 171104. [Google Scholar] [CrossRef] [PubMed]
- Khoury, J.; Weltman, A. Chameleon Cosmology. Phys. Rev. D 2004, 69, 044026. [Google Scholar] [CrossRef]
- Chiba, T.; Smith, T.L.; Erickcek, A.L. Solar System constraints to general f(R) gravity. Phys. Rev. D 2007, 75, 124014. [Google Scholar] [CrossRef]
- Tamaki, T.; Tsujikawa, S. Revisiting chameleon gravity - thin-shells and no-shells with appropriate boundary conditions. Phys. Rev. D 2008, 78, 084028. [Google Scholar] [CrossRef]
- Tsujikawa, S.; Tamaki, T.; Tavakol, R. Chameleon scalar fields in relativistic gravitational backgrounds. J. Cosmol. Astropart. Phys. 2009, 05, 020. [Google Scholar] [CrossRef]
- Guo, J.-Q. Solar system tests of f(R) gravity. Int. J. Mod. Phys. D 2014, 23, 1450036. [Google Scholar] [CrossRef]
- Pretorius, F. Numerical Relativity Using a Generalized Harmonic Decomposition. Class. Quantum Gravity 2005, 22, 425–452. [Google Scholar] [CrossRef]
- Sorkin, E.; Piran, T. Effects of Pair Creation on Charged Gravitational Collapse. Phys. Rev. D 2001, 63, 084006. [Google Scholar] [CrossRef]
- Golod, S.; Piran, T. Choptuik’s Critical Phenomenon in Einstein-Gauss-Bonnet Gravity. Phys. Rev. D 2012, 85, 104015. [Google Scholar] [CrossRef]
- Misner, C.W.; Sharp, D.H. Relativistic Equations for Adiabatic, Spherically Symmetric Gravitational Collapse. Phys. Rev. 1964, 136, B571–B576. [Google Scholar] [CrossRef]
- Hayward, S.A. Gravitational energy in spherical symmetry. Phys. Rev. D 1996, 53, 1938–1949. [Google Scholar] [CrossRef]
- Baumgarte, T.W.; Shapiro, S.L. Numerical Relativity: Solving Einstein’s Equations on the Computer; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Csizmadia, P.; Racz, I. Gravitational collapse and topology change in spherically symmetric dynamical systems. Class. Quantum Gravity 2010, 27, 015001. [Google Scholar] [CrossRef]
- Garfinkle, D. Choptuik scaling in null coordinates. Phys. Rev. D 1995, 51, 5558–5561. [Google Scholar] [CrossRef]
- Frolov, A.V. A Singularity Problem with f(R) Dark Energy. Phys. Rev. Lett. 2008, 101, 061103. [Google Scholar] [CrossRef] [PubMed]
- Guo, J.-Q.; Joshi, P.S. Spherical collapse for the Starobinsky R2 model. 2015; in preparation. [Google Scholar]
- Hong, S.E.; Hwang, D.-I.; Stewart, E.D.; Yeom, D.-H. The causal structure of dynamical charged black holes. Class. Quantum Gravity 2010, 27, 045014. [Google Scholar] [CrossRef]
- Kawai, H.; Yokokura, Y. Phenomenological Description of the Interior of the Schwarzschild Black Hole. Int. J. Mod. Phys. A 2015, 30, 1550091. [Google Scholar] [CrossRef]
- Belinskii, V.A.; Kalathnikov, I.M.; Lifshitz, E.M. Oscillatory Approach to a Singular Point in the Relativistic Cosmology. Adv. Phys. 1970, 19, 525–573. [Google Scholar] [CrossRef]
- Belinskii, V.A.; Khalatnikov, I.M. Effect of scalar and vector fields on the nature of the cosmological singularity. Zh. Eksp. Teor. Fiz. 1972, 63, 1121–1134. [Google Scholar]
- Belinskii, V.A. On the cosmological singularity. Int. J. Mod. Phys. D 2014, 23, 1430016. [Google Scholar] [CrossRef]
- BKL conjecture. Available online: http://www.einstein-online.info/dictionary/bkl-conjecture (accessed on 29 August 2015).
- Carroll, S. Spacetime and Geometry: An Introduction to General Relativity; Addison-Wesley: San Francisco, U.S.A., 2003. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. The Classical Theory of Fields; Pergamon Press: Oxford, UK, 1971; Volume 2. [Google Scholar]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, J.-Q.; Joshi, P.S. Interior Dynamics of Neutral and Charged Black Holes in f(R) Gravity. Universe 2015, 1, 239-291. https://doi.org/10.3390/universe1020239
Guo J-Q, Joshi PS. Interior Dynamics of Neutral and Charged Black Holes in f(R) Gravity. Universe. 2015; 1(2):239-291. https://doi.org/10.3390/universe1020239
Chicago/Turabian StyleGuo, Jun-Qi, and Pankaj S. Joshi. 2015. "Interior Dynamics of Neutral and Charged Black Holes in f(R) Gravity" Universe 1, no. 2: 239-291. https://doi.org/10.3390/universe1020239
APA StyleGuo, J.-Q., & Joshi, P. S. (2015). Interior Dynamics of Neutral and Charged Black Holes in f(R) Gravity. Universe, 1(2), 239-291. https://doi.org/10.3390/universe1020239




