A Loop Quantum-Corrected Family of Chiral Cosmology Models
Abstract
:1. Introduction
2. Effective LQC Dynamics
- ;
- ;
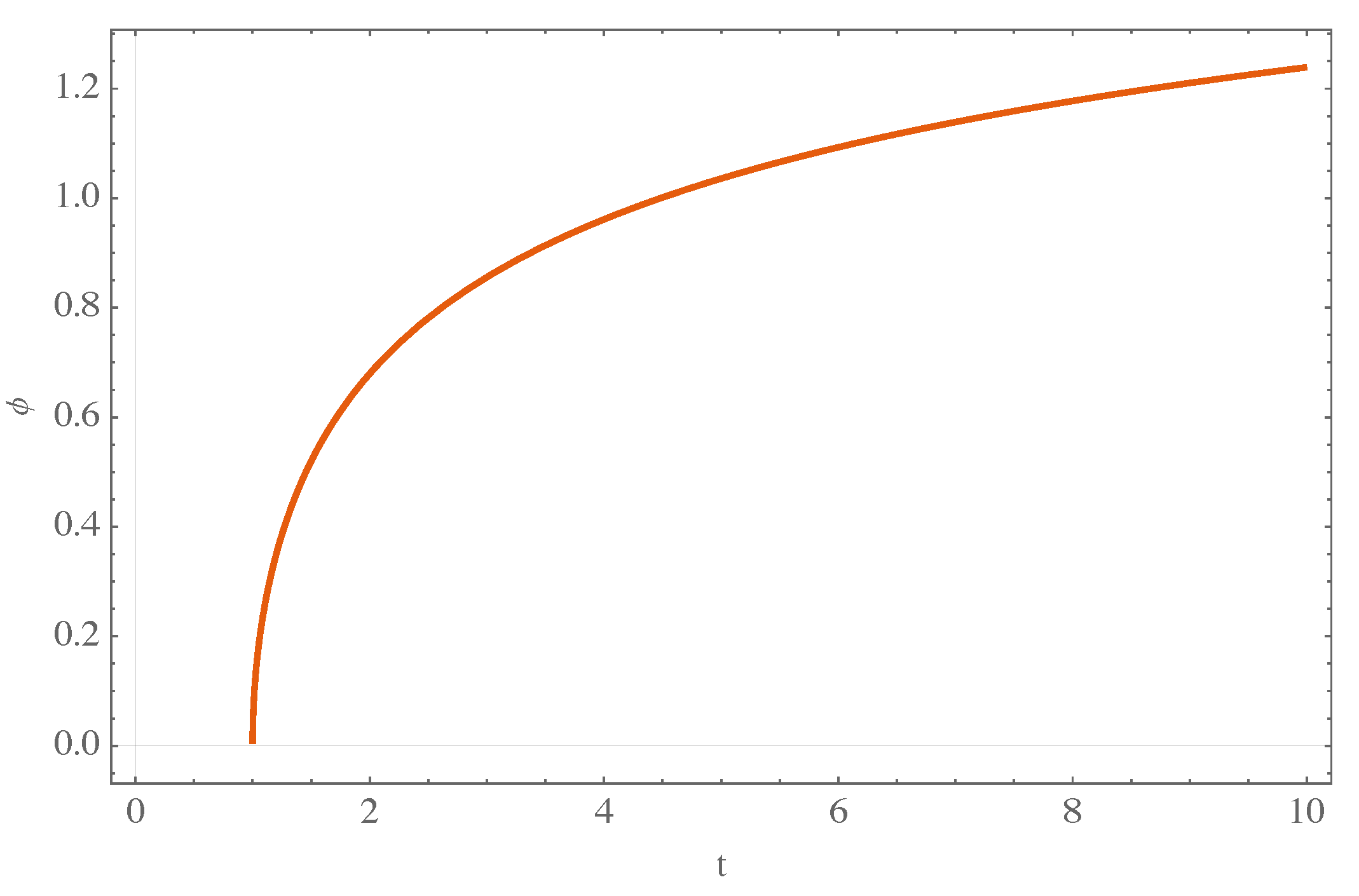
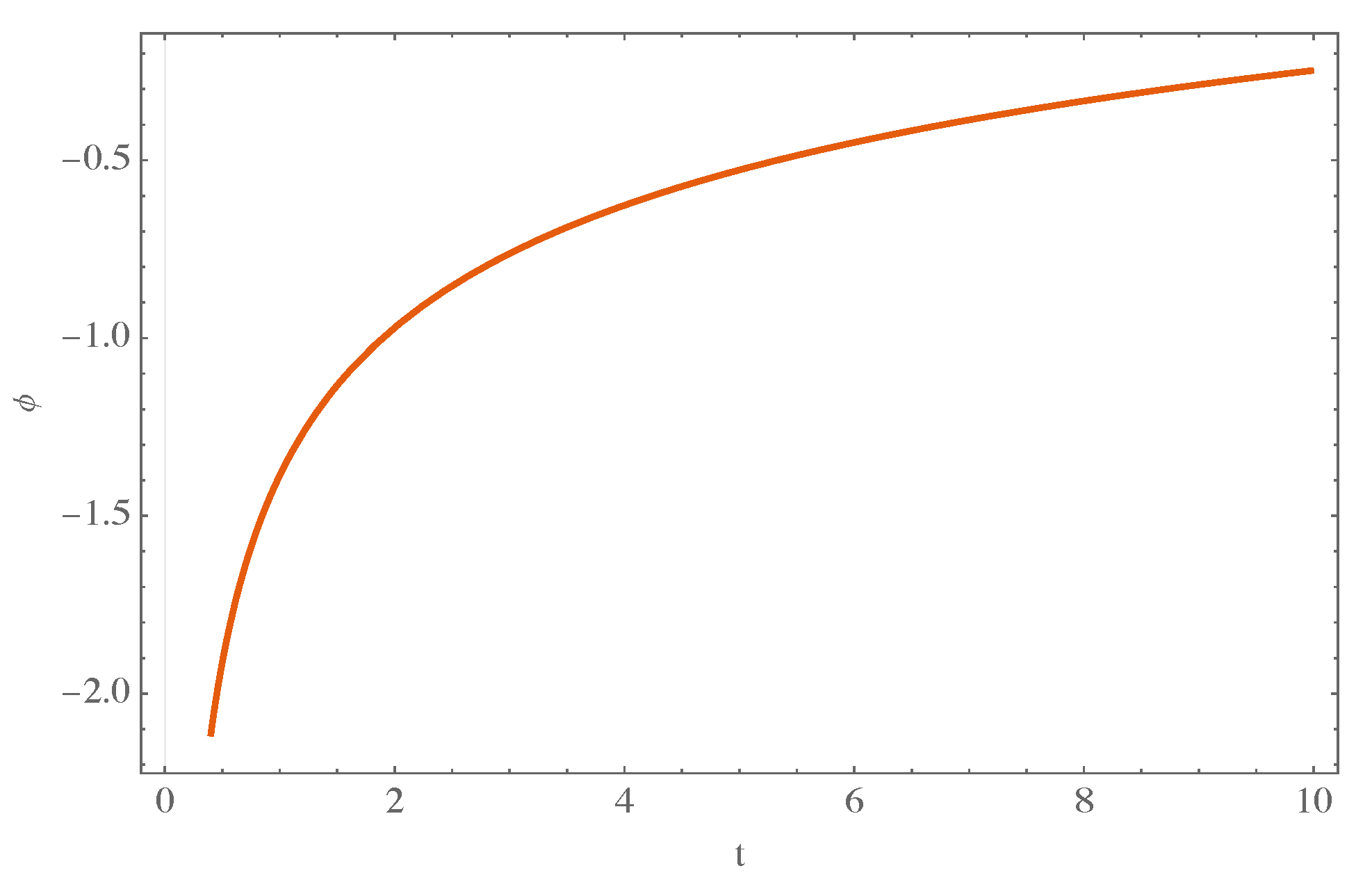
- The volume, V, reaches a minimum at ;
- is monotonic (in particular, decreasing).
3. Effective Dynamics with a Simple -Essence Field
3.1. A Further Geometrical Interpretation of the (k-Essence) Chiral Cosmology Scenario
3.2. Classical Solutions in Connection Variables
3.2.1. (Sáez–Ballester)
3.2.2.
3.3. Holonomized Hamiltonian
3.3.1. (Sáez–Ballester)
3.3.2.
4. Chiral Fields in Effective LQC
4.1. Standard (Semi-)Classical and Quantum Treatments
4.2. Holonomized Chiral Cosmology
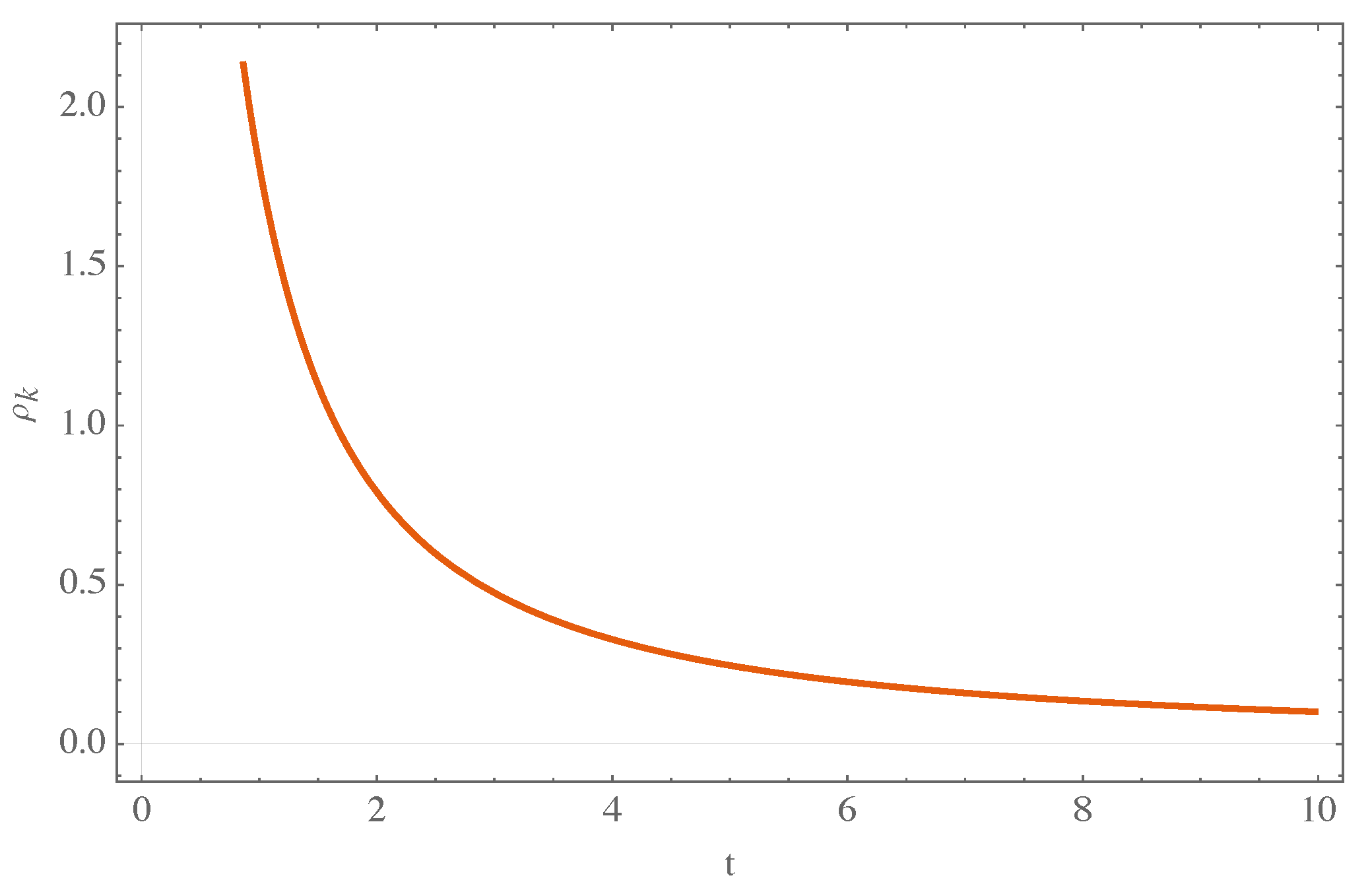
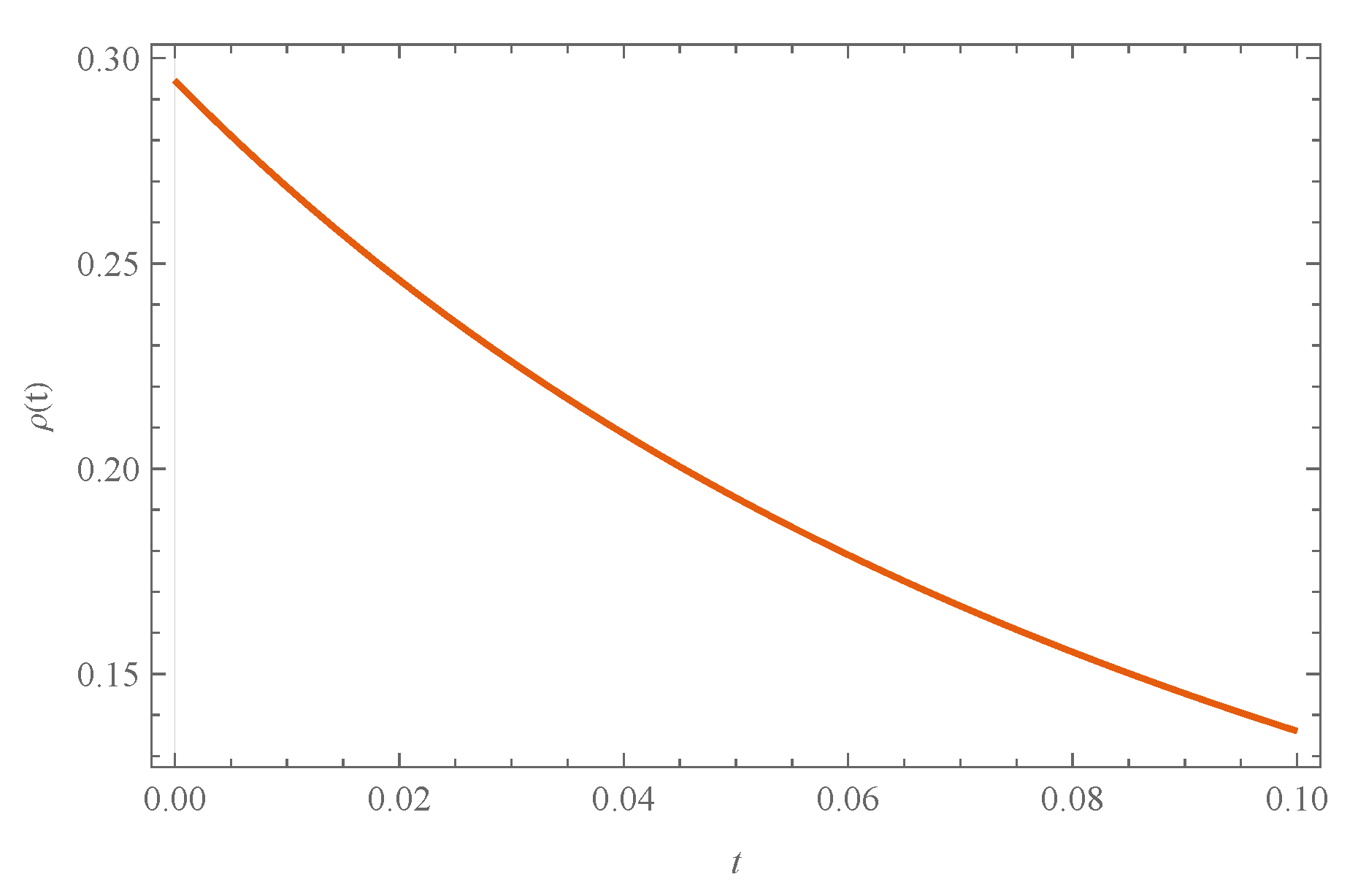
- The energy density function,is only bounded from below, and it is bounded from above provided is itself bounded (here, is not restricted to take values in the interval since no holonomization has been implemented);
- is not forbidden; in fact, it is observed that at , (this is, of course, a cosmological singularity);
- corresponds to either a maximum of V or a minimum of V, according to whether the full chiral kinetic term is positive or negative at , respectively;
- If the potential function, , is negative at , then the volume function reaches a maximum at .
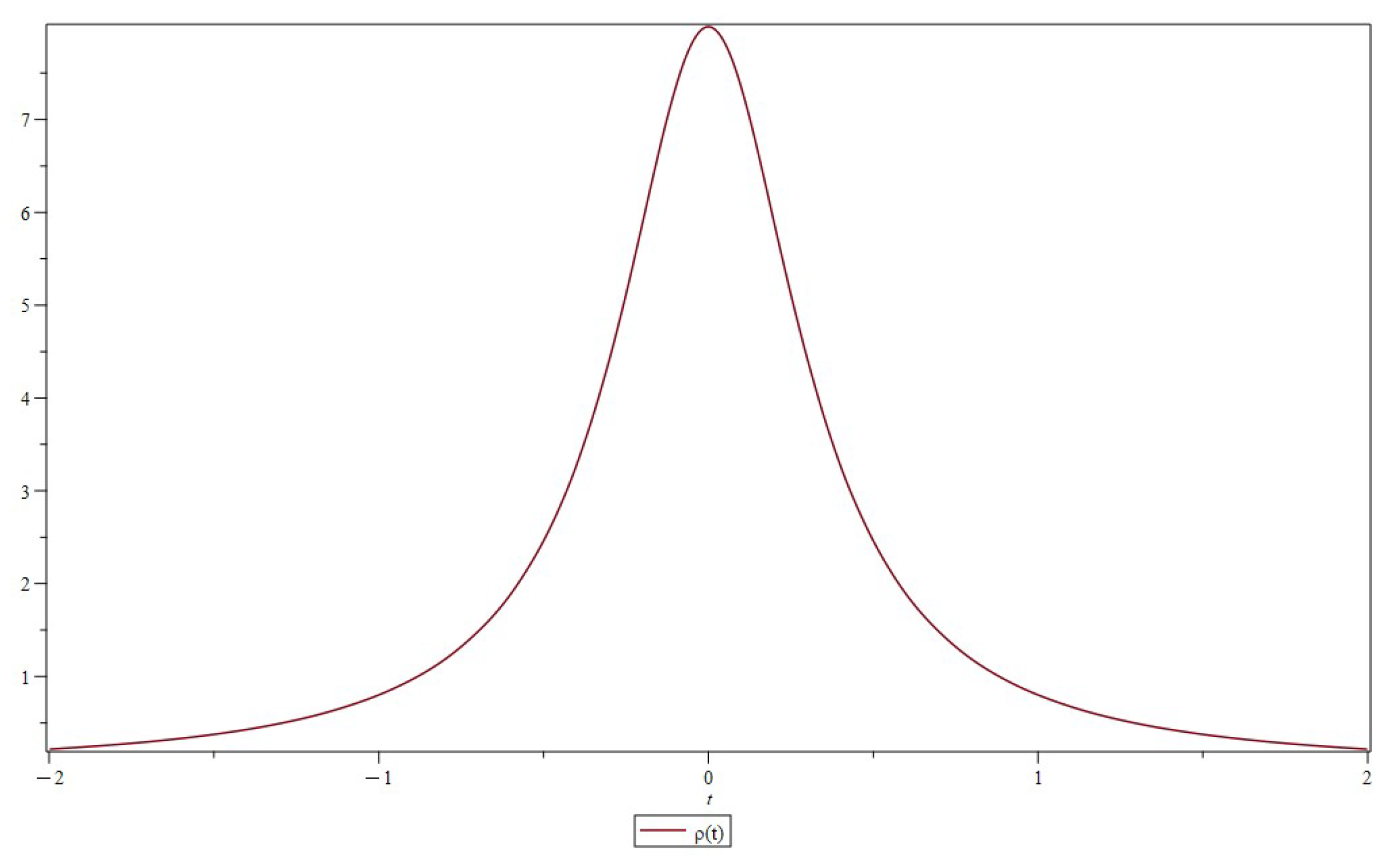
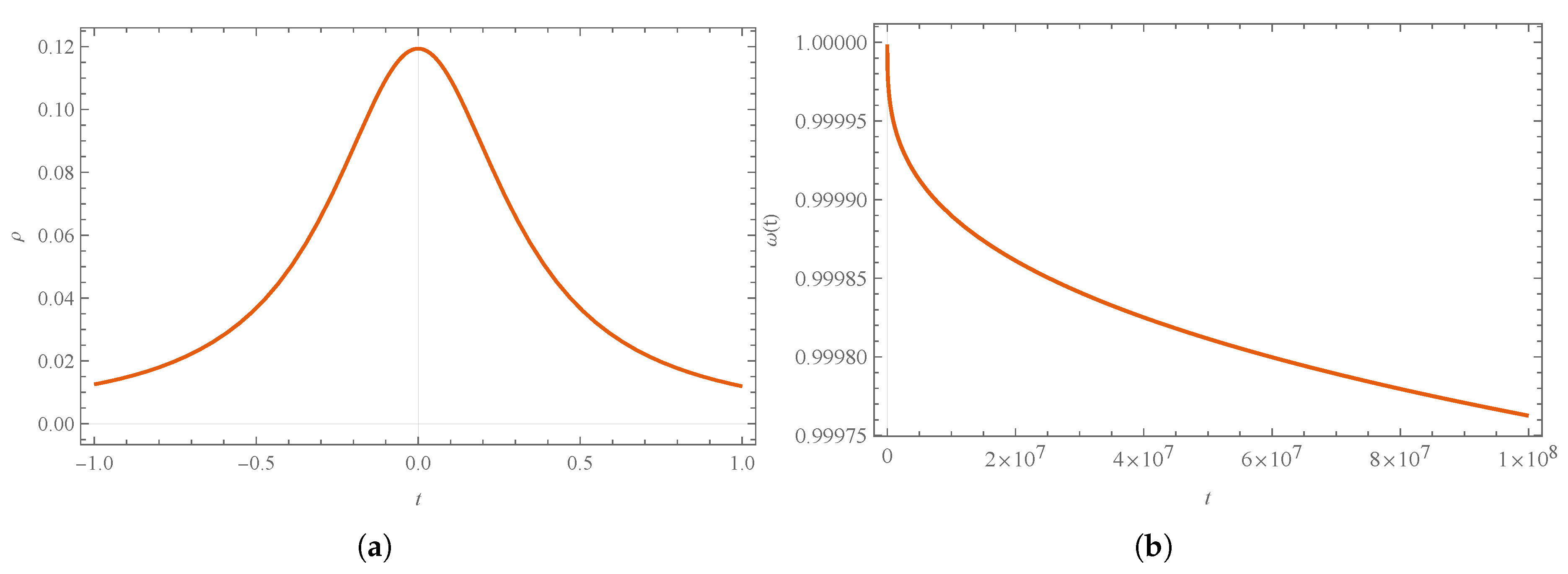
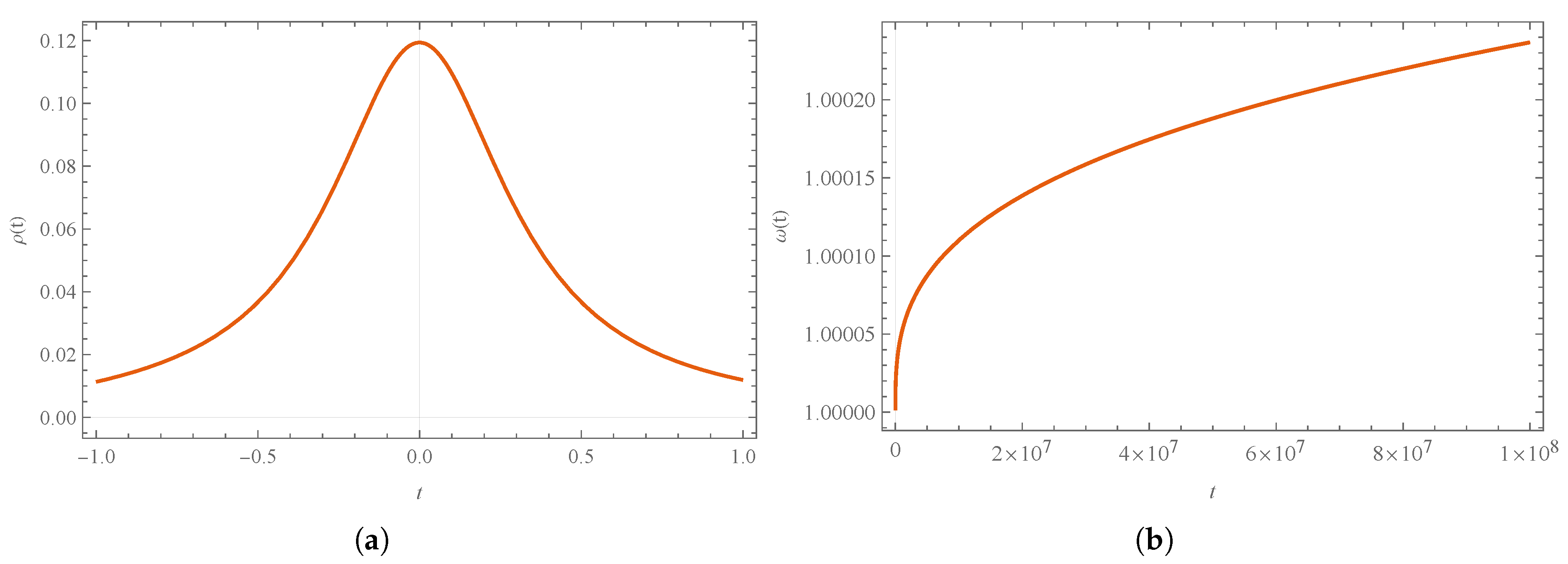
- The energy density function,takes values in the range , with (the same critical density featured in (22) in the standard case);
- The function is a monotonic function of time provided thatis fulfilled, in which case, the maximum is attained only once for solution curves lying at the surface of phase space defined by a specific condition;
- In the case at hand, a simple sufficient condition for the first relation in (122) to be satisfied is ;
- Provided (122) is fulfilled during evolution, the volume function reaches a minimum at —which corresponds to —and it is attained only once, given that the image of is in the interval .
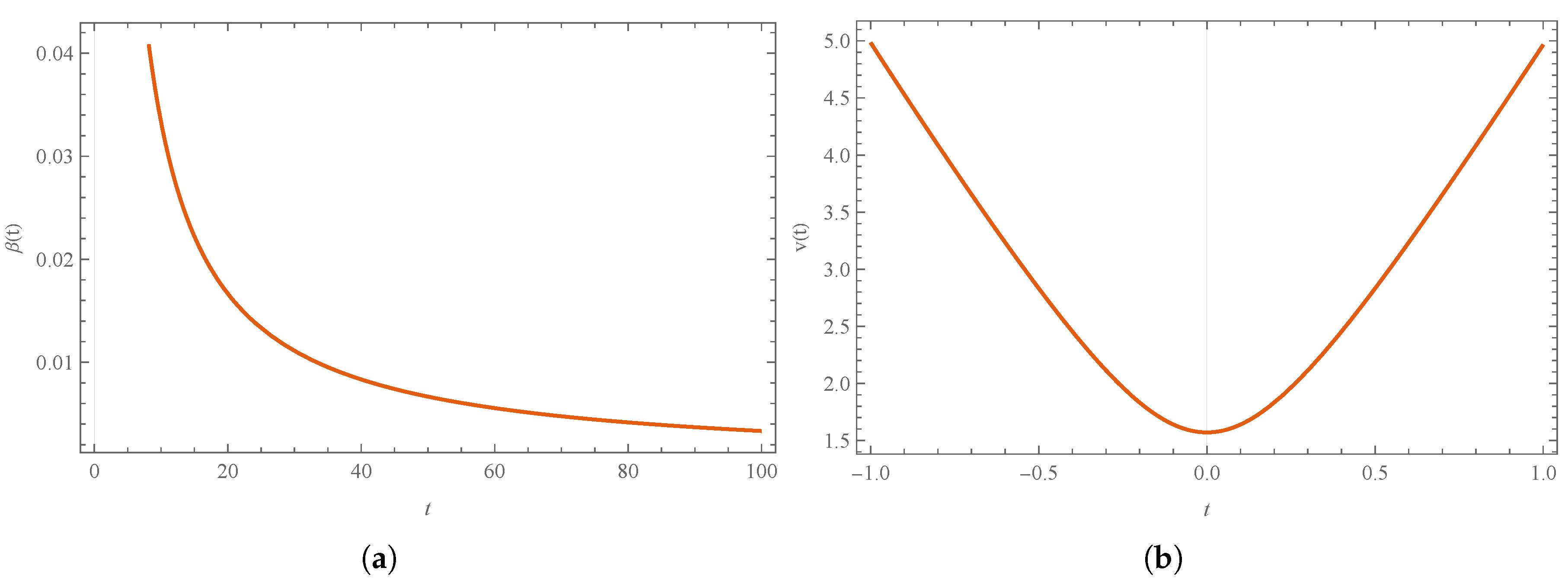
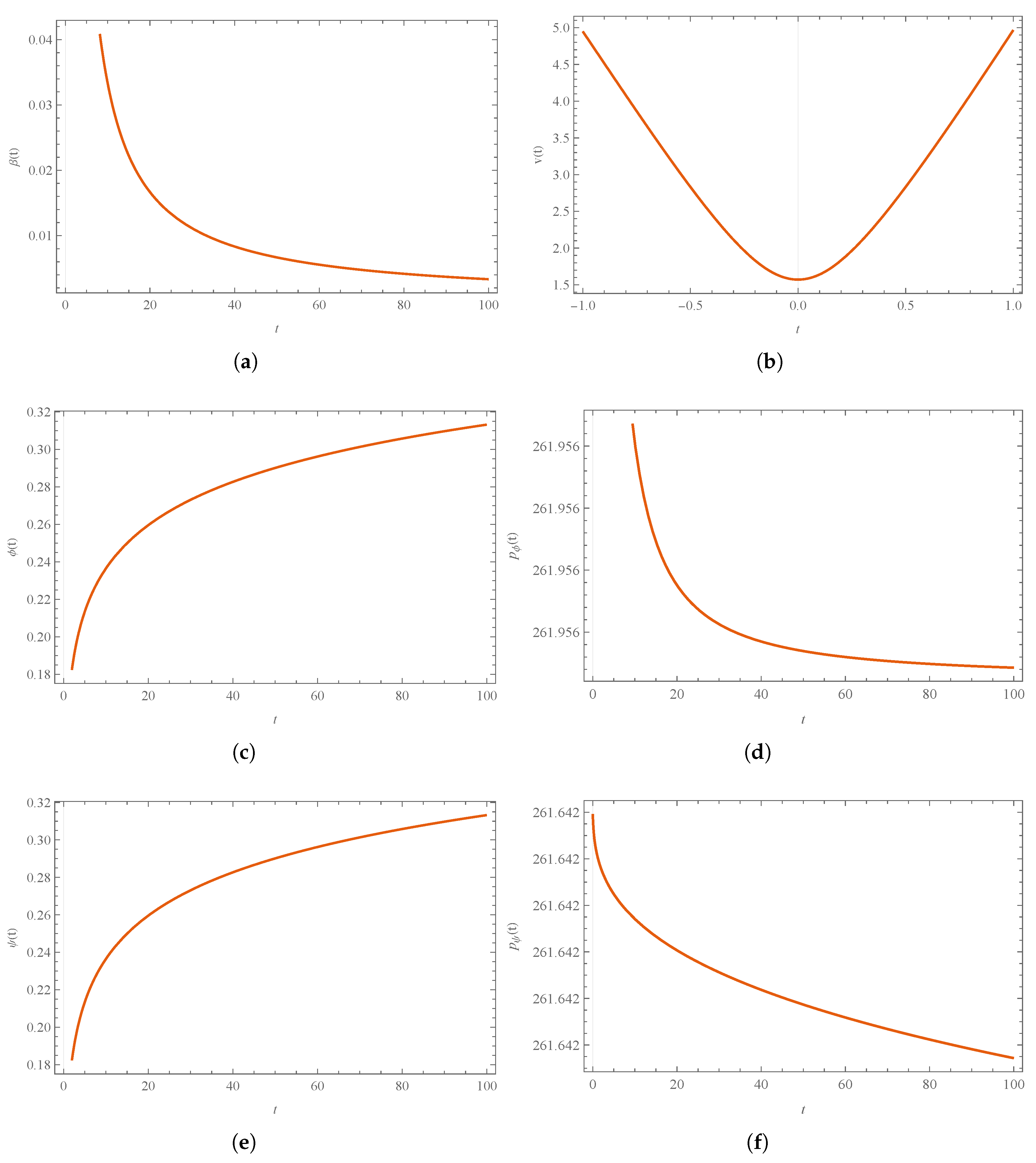
4.2.1. The First Case
4.2.2. The Second Case
5. Summary and Discussion
- In preparation for the main parts of the manuscript, in Section 3, a holonomization of a FLRW background with a particularly simple k-essence field was performed, and some exact solutions were found (which were shown to reduce to the standard classical ones in the limit of a vanishing area gap).
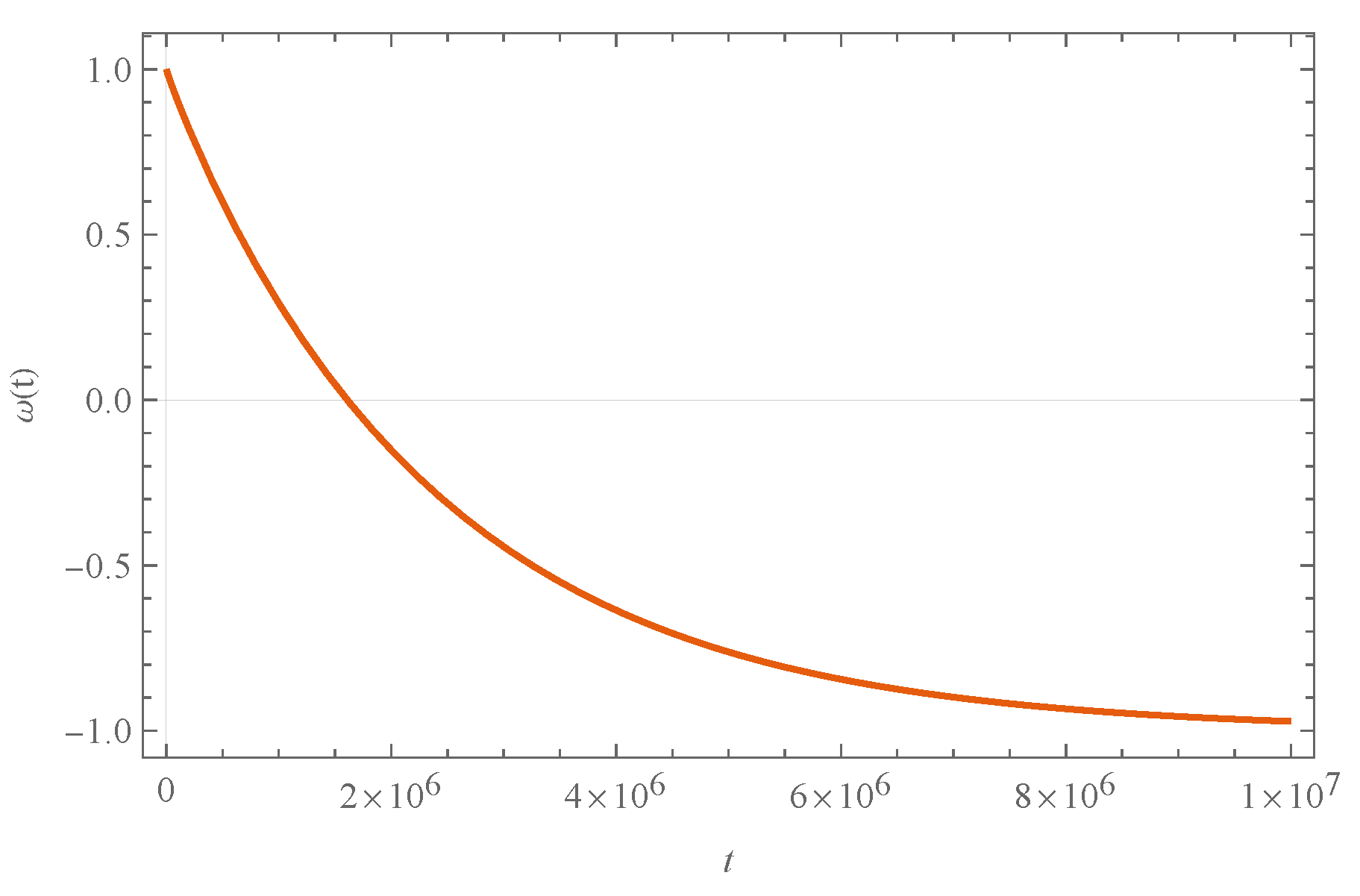
- In Section 4.2, we constructed a family of holonomy-corrected chiral quintom cosmology models. We established that the key single big bounce in the standard LQC paradigm is achieved provided the full chiral kinetic energy does note change sign during its evolution (which is ensured, in particular, by taking parameters ). We also analyzed the corresponding equation-of-state parameter.
- The main objective of Section 4.2.1 and Section 4.2.2 was to exemplify the general remarks given for the holonomy-corrected Hamiltonian system in Section 4.2 via particular numerical solutions.
A Larger Family of Loop Quantum-Corrected Chiral Models
- A negative definite K together with an identically zero U is not consistent with (131), and such a situation is therefore forbidden.
- If U is identically zero, must be satisfied during evolution, and hence the single big bounce takes place.
- The more restrictive situation in which K is positive and definite is, of course, a particular case of the result established above.
- If is satisfied during evolution, then the big bounce occurs. This includes the more restrictive situation in which U is negative and definite. Indeed, if , then from (131), it follows that during evolution.
- The more restrictive situation in which K is negative and definite (in which case it is necessary that during evolution) is, of course, a particular case of the result established above.
- Standard models with holonomy corrections:
- −
- A standard quintom scenario in effective LQC is obtained by considering two scalar fields, and , with (see, e.g., [53]);
- −
- Inflationary scenarios within effective LQC are obtained by considering one scalar field, , with and suitable forms for (see, e.g., [54]);
- −
- The original effective scheme of LQC is achieved by considering one free scalar field, , with .
- Standard models without holonomy corrections: The replacement is to be performed in the Hamiltonian (130), and is no longer restricted to take values only in the range .
- −
- The standard quintom scenario is obtained by considering only two scalar fields, and , and taking with suitable potentials, and . Relevant potentials are reported in [1].
- −
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | In cosmology, one is usually restricted to homogenous (metric and scalar) fields. |
| 2 | The spatial integration featured in the action, when carried over the whole spacelike slice, diverges in the flat case. Due to homogeneity, we can restrict the action to a compact region of the spacelike slice. |
References
- Copeland, E.J.; Sami, M.; Tsujikawa, S. Dynamics of dark energy. Int. J. Mod. Phys. D 2006, 15, 1753–1936. [Google Scholar] [CrossRef]
- Cai, Y.F.; Saridakis, E.N.; Setare, M.R.; Xia, J.Q. Quintom Cosmology: Theoretical implications and observations. Phys. Rep. 2010, 493, 1–60. [Google Scholar] [CrossRef]
- Vikman, A. Can dark energy evolve to the phantom? Phys. Rev. D 2005, 71, 023515. [Google Scholar] [CrossRef]
- Chervon, S.V. On the chiral model of cosmological inflation. Russ. Phys. J. 1995, 38, 539–543. [Google Scholar] [CrossRef]
- Chervon, S.V. Chiral Cosmological Models: Dark Sector Fields Description. Quantum Matter 2013, 2, 71–82. [Google Scholar] [CrossRef]
- Socorro, J.; Pérez-Payán, S.; Hernández-Jiménez, R.; Espinoza-García, A.; Díaz-Barrón, L.R. Quintom Fields from Chiral K-essence Cosmology. Universe 2022, 8, 548. [Google Scholar] [CrossRef]
- Armendariz-Picon, C.; Damour, T.; Mukhanov, V.F. k—Inflation. Phys. Lett. B 1999, 458, 209–218. [Google Scholar] [CrossRef]
- Armendariz-Picon, C.; Mukhanov, V.; Steinbardt, P.J. Dynamical solution to the problem of a small cosmological constant and late-time cosmic acceleration. Phys. Rev. Lett. 2000, 85, 4438. [Google Scholar] [CrossRef]
- Armendariz-Picon, C.; Mukhanov, V.; Steinbardt, P.J. Essentials of k-essence. Phys. Rev. D 2001, 63, 103510. [Google Scholar] [CrossRef]
- De Felice, A.; Tsujikawa, S. f(R) theories. Living Rev. Relativ. 2010, 13, 3. [Google Scholar] [CrossRef]
- Kaiser, D.I. Conformal transformations with multiple scalar fields. Phys. Rev. D 2010, 81, 084044. [Google Scholar] [CrossRef]
- Birrell, N.D.; Davies, P.C.W. Quantum Fields in Curved Space; Cambridge University Press: New York, NY, USA, 1982. [Google Scholar]
- Paliathanasis, A.; Leon, G.; Pan, S. Exact Solutions in Chiral Cosmology. Gen. Relativ. Gravit. 2019, 51, 106. [Google Scholar] [CrossRef]
- Socorro, J.; Pérez-Payán, S.; Hernández-Jiménez, R.; Espinoza-García, A.; Díaz-Barrón, L.R. Classical and quantum exact solutions for a FRW in chiral like cosmology. Class. Quant. Grav. 2021, 38, 135027. [Google Scholar] [CrossRef]
- Paliathanasis, A. Bianchi I Spacetimes in Chiral–Quintom Theory. Universe 2022, 8, 503. [Google Scholar] [CrossRef]
- Socorro, J.; Pérez-Payán, S.; Hernández-Jiménez, R.; Espinoza-García, A.; Díaz-Barrón, L.R. Quintom fields from chiral anisotropic cosmology. Gen. Relativ. Gravit. 2023, 55, 75. [Google Scholar] [CrossRef]
- Ashtekar, A.; Lewandowski, J. Background independent quantum gravity: A status report. Class. Quant. Grav. 2004, 21, R53–R152. [Google Scholar] [CrossRef]
- Thiemann, T. Introduction to Modern Canonical Quantum General Relativity; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Arnowitt, R.; Deser, S.; Misner, C.W. Republication of: The dynamics of general relativity. Gen. Relativ. Gravit. 2008, 40, 1997–2027. [Google Scholar] [CrossRef]
- Rovelli, C. Quantum Gravity; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Ashtekar, A.; Singh, P. Loop quantum cosmology: A status report. Class. Quant. Grav. 2011, 28, 213001. [Google Scholar] [CrossRef]
- Bojowald, M. Loop quantum cosmology. Living Rev. Relativ. 2005, 8, 11. [Google Scholar] [CrossRef]
- Bojowald, M. Absence of singularity in loop quantum cosmology. Phys. Rev. Lett. 2001, 86, 5227–5230. [Google Scholar] [CrossRef]
- Diener, P.; Gupt, B.; Megevand, M.; Singh, P. Numerical evolution of squeezed and non-Gaussian states in loop quantum cosmology. Class. Quant. Grav. 2014, 31, 165006. [Google Scholar] [CrossRef]
- Ashtekar, A.; Bojowald, M.; Lewandowski, J. Mathematical structure of loop quantum cosmology. Adv. Theor. Math. Phys. 2003, 7, 233–268. [Google Scholar] [CrossRef]
- Bojowald, M. Canonical Gravity and Applications; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Ashtekar, A.; Corichi, A.; Singh, P. Robustness of key features of loop quantum cosmology. Phys. Rev. D 2008, 77, 024046. [Google Scholar] [CrossRef]
- Sotiriou, T.P. Covariant effective action for loop quantum cosmology from order reduction. Phys. Rev. D 2009, 79, 044035. [Google Scholar] [CrossRef]
- Ribeiro, A.R.; Vernieri, D.; Lobo, F.S.N. Effective f(R) Actions for Modified Loop Quantum Cosmologies via Order Reduction. Universe 2023, 9, 181. [Google Scholar] [CrossRef]
- Socorro, J.; Pimentel, L.O.; Espinoza-García, A. Classical Bianchi type I cosmology in K-essence theory. Adv. High Energy Phys. 2014, 2014, 805164. [Google Scholar] [CrossRef]
- Corben, H.C.; Stehle, P. Classical Mechanics; Dover Publications: Mineola, NY, USA, 1994. [Google Scholar]
- Goldstein, H.; Poole, C.; Safko, J. Classical Mechanics; Addison-Wesley: Reading, MA, USA, 2002. [Google Scholar]
- Dirac, P.A.M. Lectures on Quantum Mechanics; Dover Publications: Mineola, NY, USA, 2001. [Google Scholar]
- Wipf, A. Hamilton’s Formalism for Systems with Constraints. In Canonical Gravity: From Classical to Quantum; Ehlers, J., Friedright, H., Eds.; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar]
- Christodoulakis, T.; Dimakis, N.; Terzis, P.A. Lie point and variational symmetries in minisuperspace Einstein gravity. J. Phys. A Math. Theor. 2014, 47, 095202. [Google Scholar] [CrossRef]
- Saez, D.; Ballester, V.J. A simple coupling with cosmological implications. Phys. Lett. A 1986, 113, 467–470. [Google Scholar] [CrossRef]
- Shi, J.; Wu, J. Dynamics of k-essence in loop quantum cosmology. Chin. Phys. C 2021, 45, 045104. [Google Scholar] [CrossRef]
- Abraham, R.; Marsden, J.E. Foundations of Mechanics; American Mathematical Society: Providence, RI, USA, 2008. [Google Scholar]
- De Witt, B.S. Quantum Theory of Gravity. I. The Canonical Theory. Phys. Rev. 1967, 160, 1113. [Google Scholar] [CrossRef]
- Ryan, M.P., Jr.; Turbiner, A.V. The conformally invariant Laplace–Beltrami operator and factor ordering. Phys. Lett. A 2004, 333, 30–34. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Course of Theoreitcal Physics. In Quantum Mechanics (Non-Relativistic Theory); Elsevier: Amsterdam, The Netherlands, 1977; Volume 3. [Google Scholar]
- Kaminski, W.; Kolanowski, M.; Lewandowski, J. Dressed metric predictions revisited. Class. Quant. Grav. 2020, 37, 095001. [Google Scholar] [CrossRef]
- Kim, S.A.; Liddle, A.R. Nflation: Multi-field inflationary dynamics and perturbations. Phys. Rev. D 2006, 74, 023513. [Google Scholar] [CrossRef]
- Lazkoz, R.; Leon, G.; Quiros, I. Quintom cosmologies with arbitrary potentials. Phys. Lett. B 2007, 649, 103–110. [Google Scholar] [CrossRef]
- Leon, G.; Paliathanasis, A.; Morales-Martínez, J.L. The past and future dynamics of quintom dark energy models. Eur. Phys. J. C 2018, 78, 753. [Google Scholar] [CrossRef]
- Dimakis, N.; Paliathanasis, A. Crossing the phantom divide line as an effect of quantum transitions. Class. Quant. Grav. 2021, 38, 075016. [Google Scholar] [CrossRef]
- Elizalde, E.; Nojiri, S.; Odintsov, S.D.; Saez-Gomez, D.; Faraoni, V. Reconstructing the universe history, from inflation to acceleration, with phantom and canonical scalar fields. Phys. Rev. D 2008, 77, 106005. [Google Scholar] [CrossRef]
- Christodoulidis, P.; Roest, D.; Sfakianakis, E.I. Scaling attractors in multi-field inflation. J. Cosmol. Astropart. Phys. 2019, 12, 59. [Google Scholar] [CrossRef]
- Beesham, A.; Chervon, S.V.; Maharaj, S.D.; Kubasov, A.S. An Emergent Universe with Dark Sector Fields in a Chiral Cosmological Model. Quantum Matter 2013, 2, 388–395. [Google Scholar] [CrossRef]
- Chervon, S.V.; Abbyazov, R.R.; Kryukov, S.V. Dynamics of Chiral Cosmological Fields in the Phantom-Canonical Model. Russ. Phys. J. 2015, 58, 597–605. [Google Scholar] [CrossRef]
- Socorro, J.; Núñez, O.E. Scalar potentials with Multi-scalar fields from quantum cosmology and supersymmetric quantum mechanics. Eur. Phys. J. Plus 2017, 132, 168. [Google Scholar] [CrossRef]
- Socorro, J.; Núñez, O.E.; Hernández-Jiménez, R. Classical and Quantum Exact Solutions for a FRW Multiscalar Field Cosmology with an Exponential Potential Driven Inflation. Adv. Math. Phys. 2018, 2018, 3468381. [Google Scholar] [CrossRef]
- Wei, H.; Zhang, S.N. Dynamics of quintom and hessence energies in loop quantum cosmology. Phys. Rev. D 2007, 76, 063005. [Google Scholar] [CrossRef]
- Barboza, L.N.; Graef, L.L.; Ramos, R.O. Warm bounce in loop quantum cosmology and the prediction for the duration of inflation. Phys. Rev. D 2020, 102, 103521. [Google Scholar] [CrossRef]
- Tsujikawa, S. Quintessence: A review. Class. Quant. Grav. 2013, 30, 214003. [Google Scholar] [CrossRef]
- Belinskii, V.A.; Khalatnikov, I.M.; Lifshitz, E.M. Oscillatory approach to a singular point in relativistic cosmology. Adv. Phys. 1970, 19, 525. [Google Scholar] [CrossRef]
- Chiou, D.W. Loop quantum cosmology in Bianchi Type I Models: Analytical Investigation. Phys. Rev. D 2007, 75, 024029. [Google Scholar] [CrossRef]
- Chiou, D.W. Effective dynamics, big bounces and scaling symmetry in Bianchi I loop quantum cosmology. Phys. Rev. D 2007, 76, 124037. [Google Scholar] [CrossRef]
- Ashtekar, A.; Wilson-Ewing, E. Loop quantum cosmology of Bianchi type I models. Phys. Rev. D 2009, 79, 083535. [Google Scholar] [CrossRef]
- Wilson-Ewing, E. Loop quantum cosmology of Bianchi type IX models. Phys. Rev. D 2010, 82, 043508. [Google Scholar] [CrossRef]



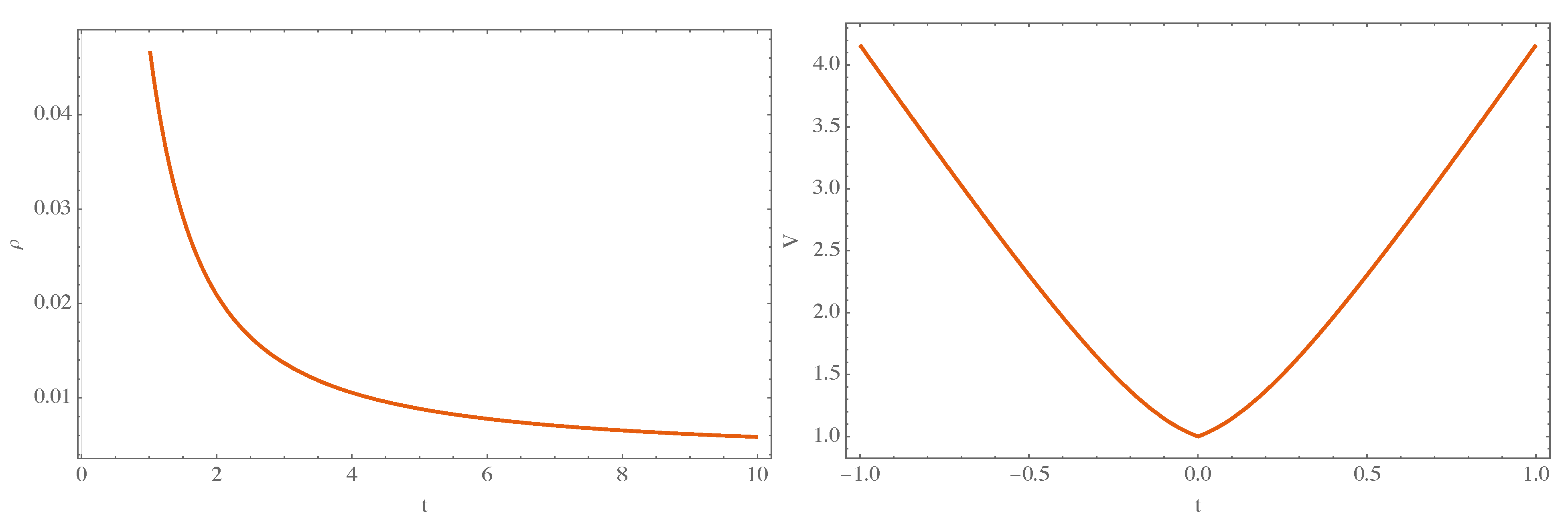




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Díaz-Barrón, L.R.; Espinoza-García, A.; Pérez-Payán, S.A.; Socorro, J. A Loop Quantum-Corrected Family of Chiral Cosmology Models. Universe 2024, 10, 88. https://doi.org/10.3390/universe10020088
Díaz-Barrón LR, Espinoza-García A, Pérez-Payán SA, Socorro J. A Loop Quantum-Corrected Family of Chiral Cosmology Models. Universe. 2024; 10(2):88. https://doi.org/10.3390/universe10020088
Chicago/Turabian StyleDíaz-Barrón, Luis Rey, Abraham Espinoza-García, Sinuhé Alejandro Pérez-Payán, and J. Socorro. 2024. "A Loop Quantum-Corrected Family of Chiral Cosmology Models" Universe 10, no. 2: 88. https://doi.org/10.3390/universe10020088
APA StyleDíaz-Barrón, L. R., Espinoza-García, A., Pérez-Payán, S. A., & Socorro, J. (2024). A Loop Quantum-Corrected Family of Chiral Cosmology Models. Universe, 10(2), 88. https://doi.org/10.3390/universe10020088





