Variation in XCO Factor in N55 Region
Abstract
:1. Introduction
2. ALMA Archive Data
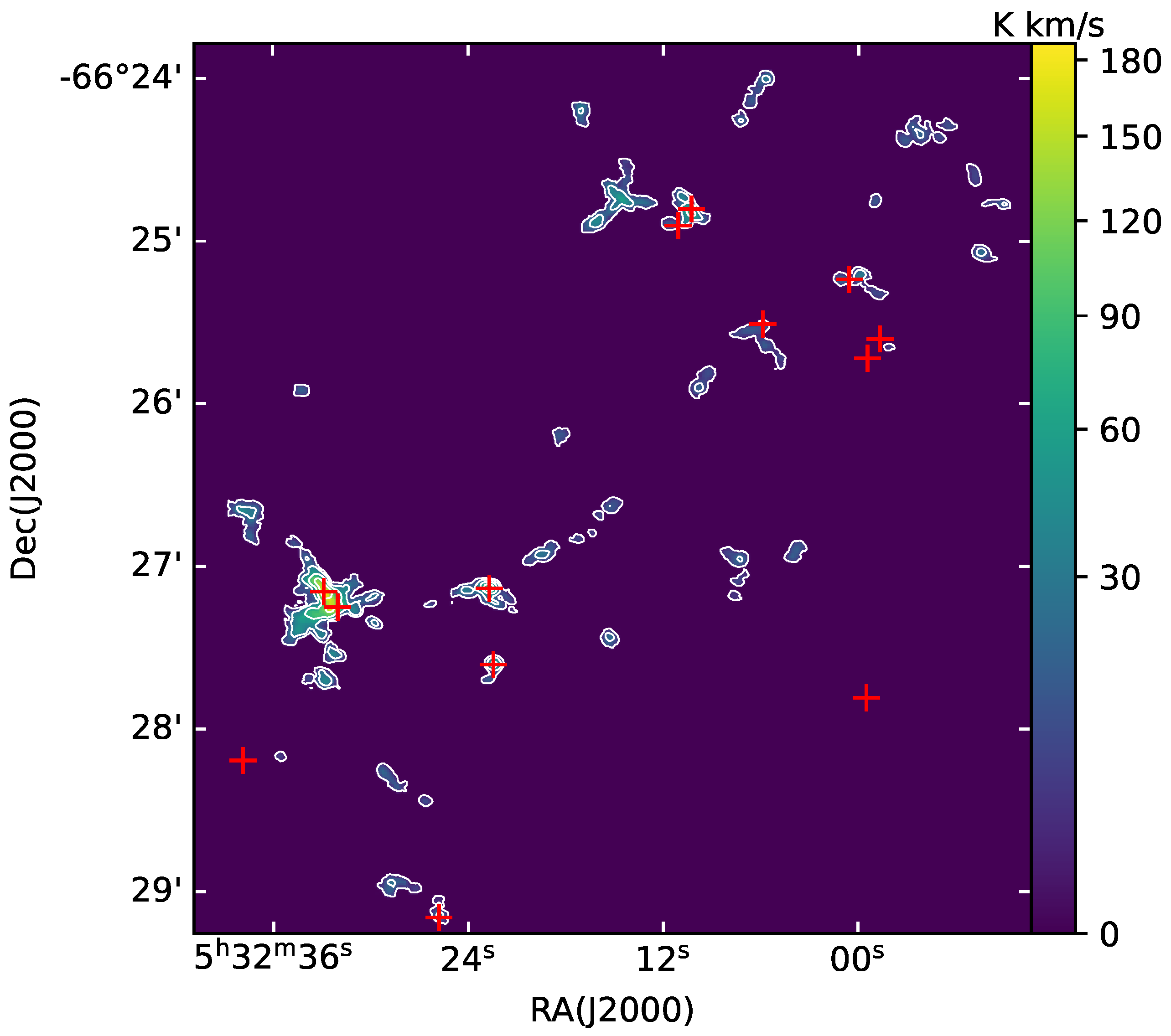
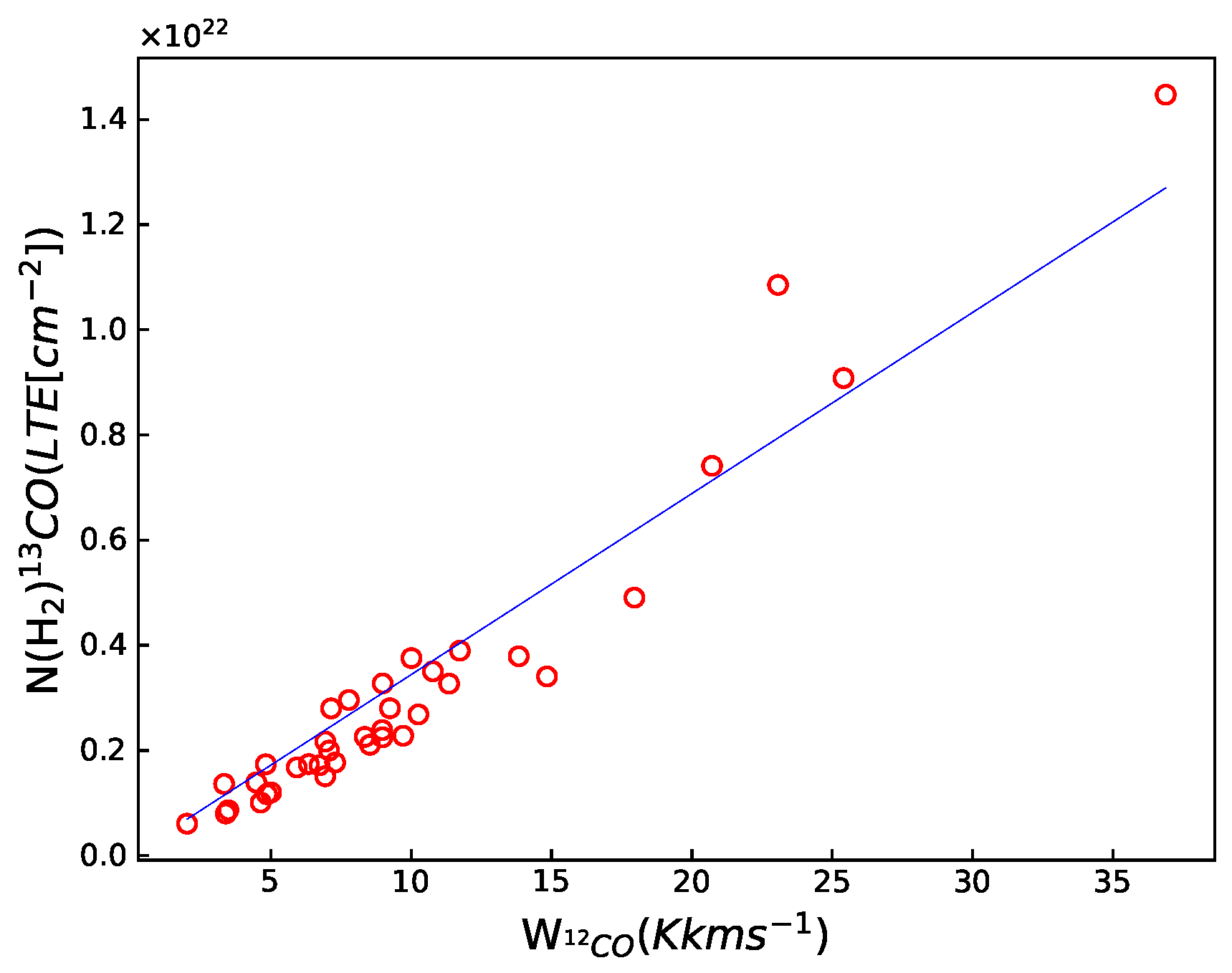
3. Data Analysis
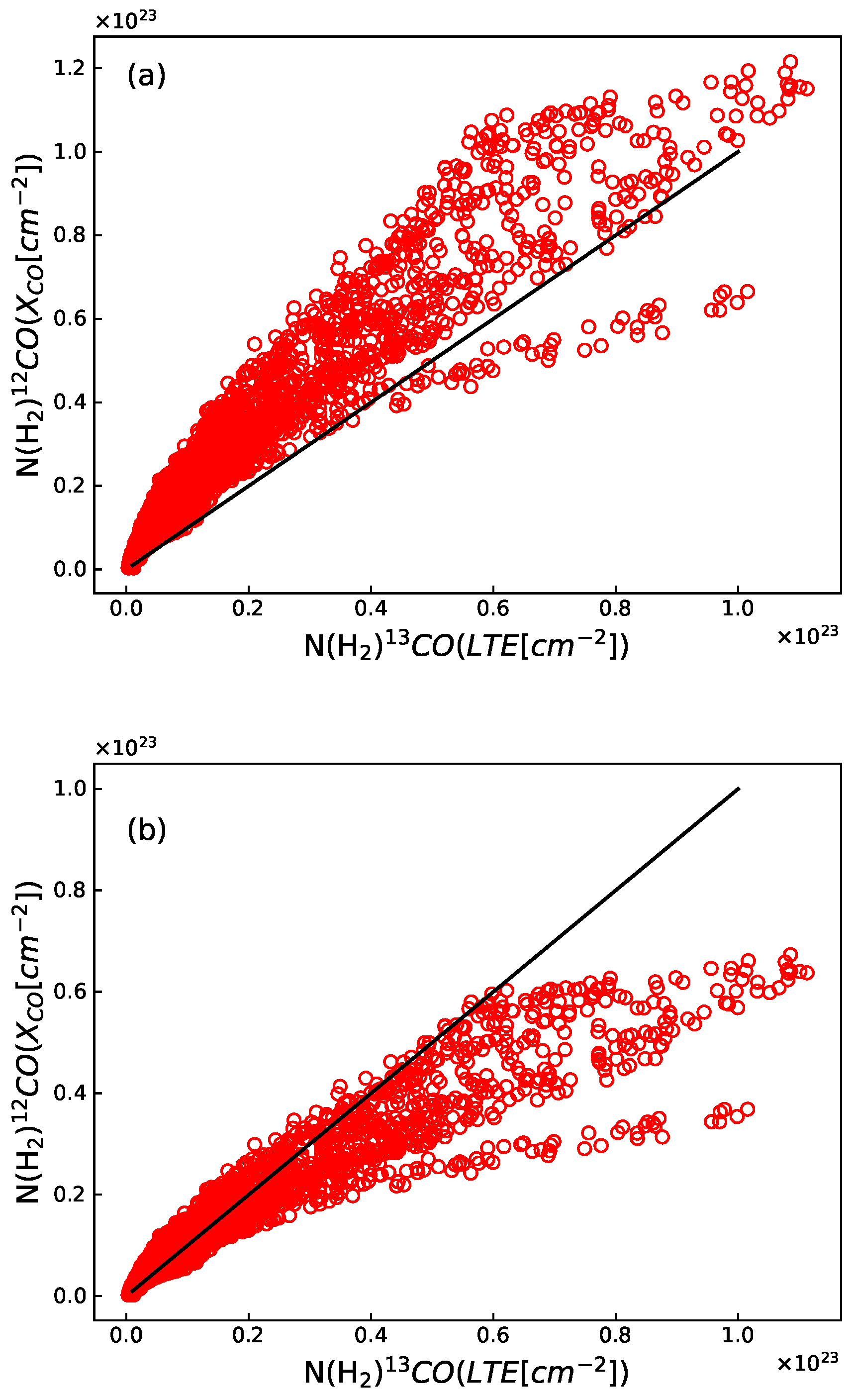
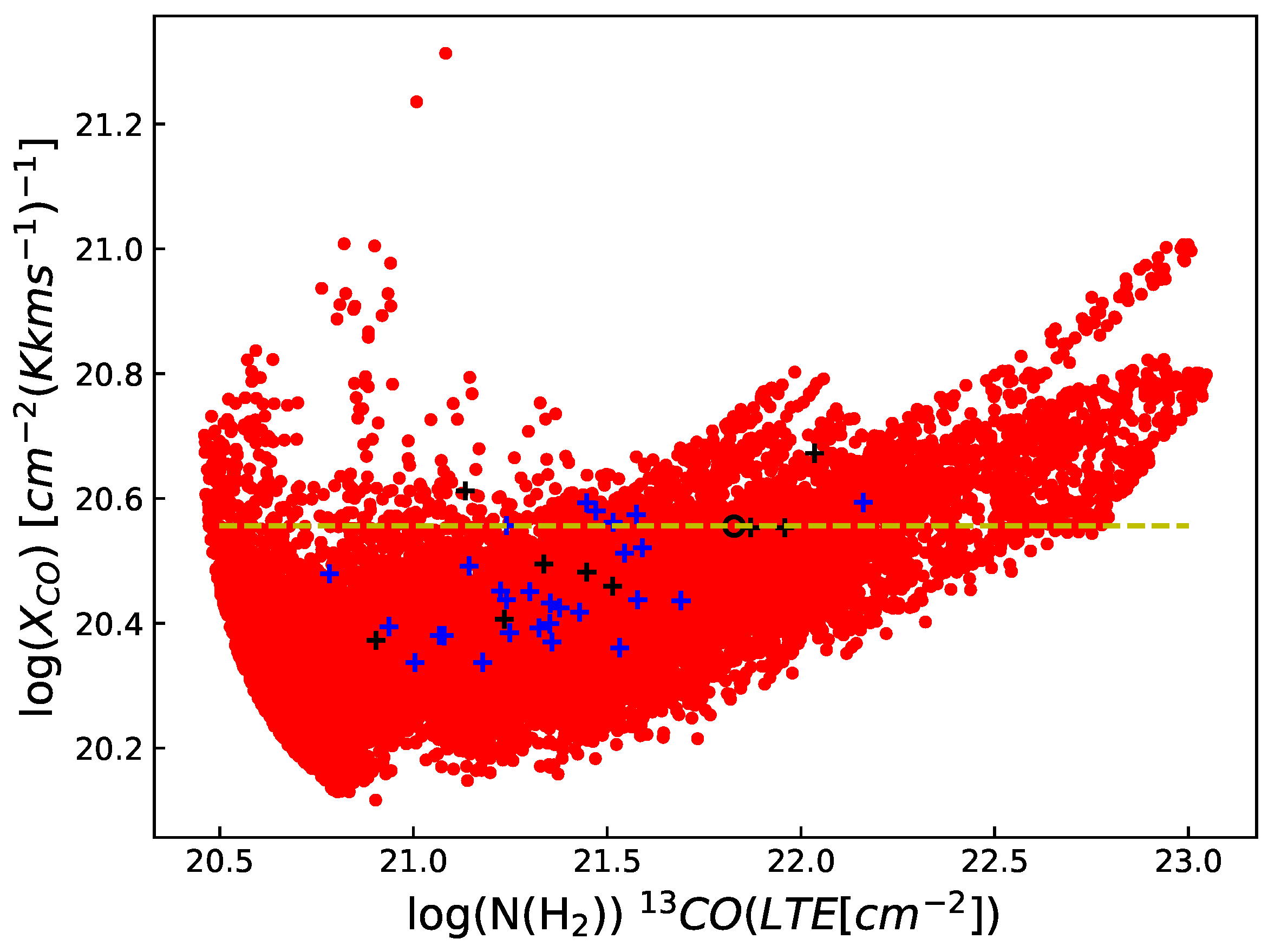
4. Results and Discussion
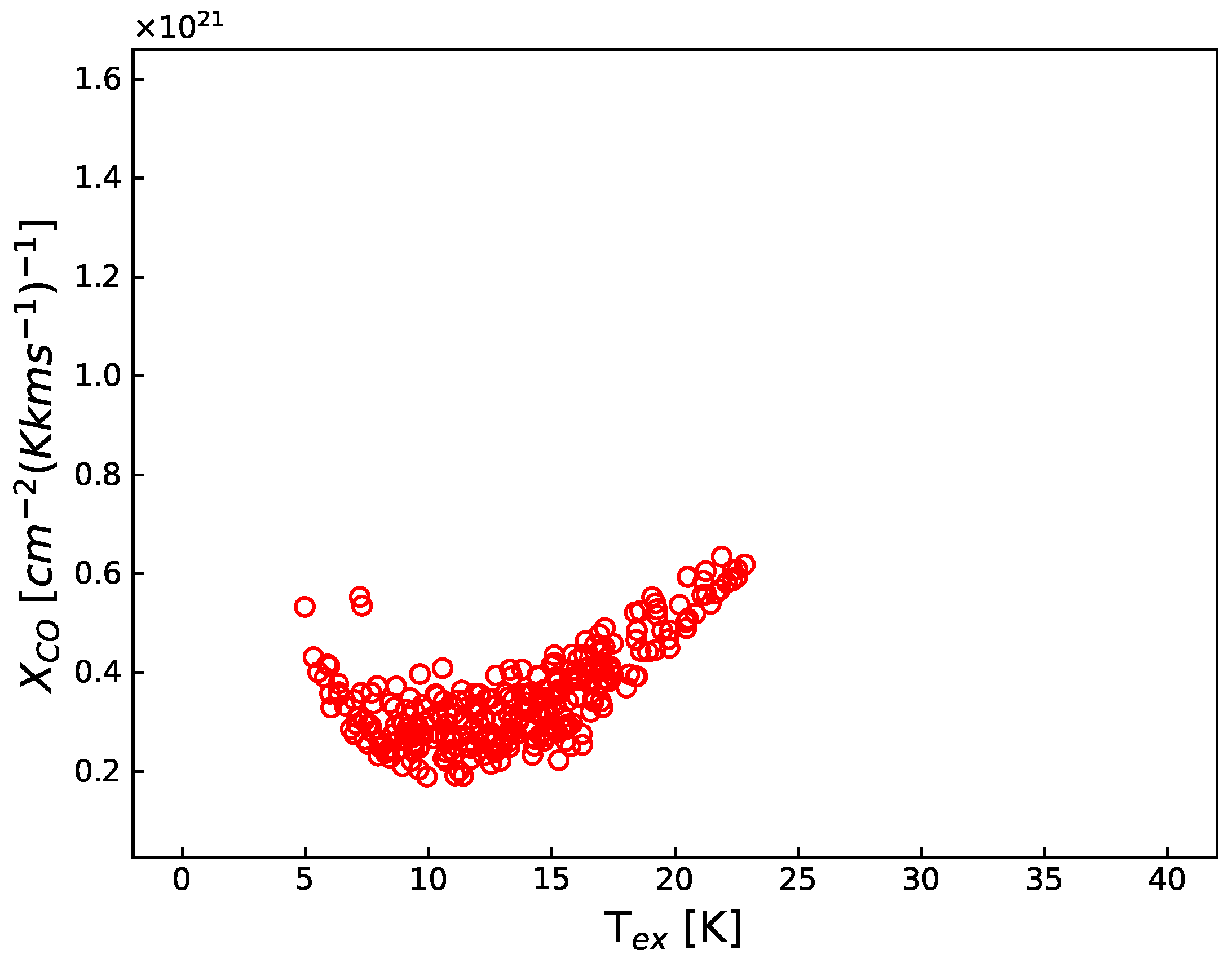
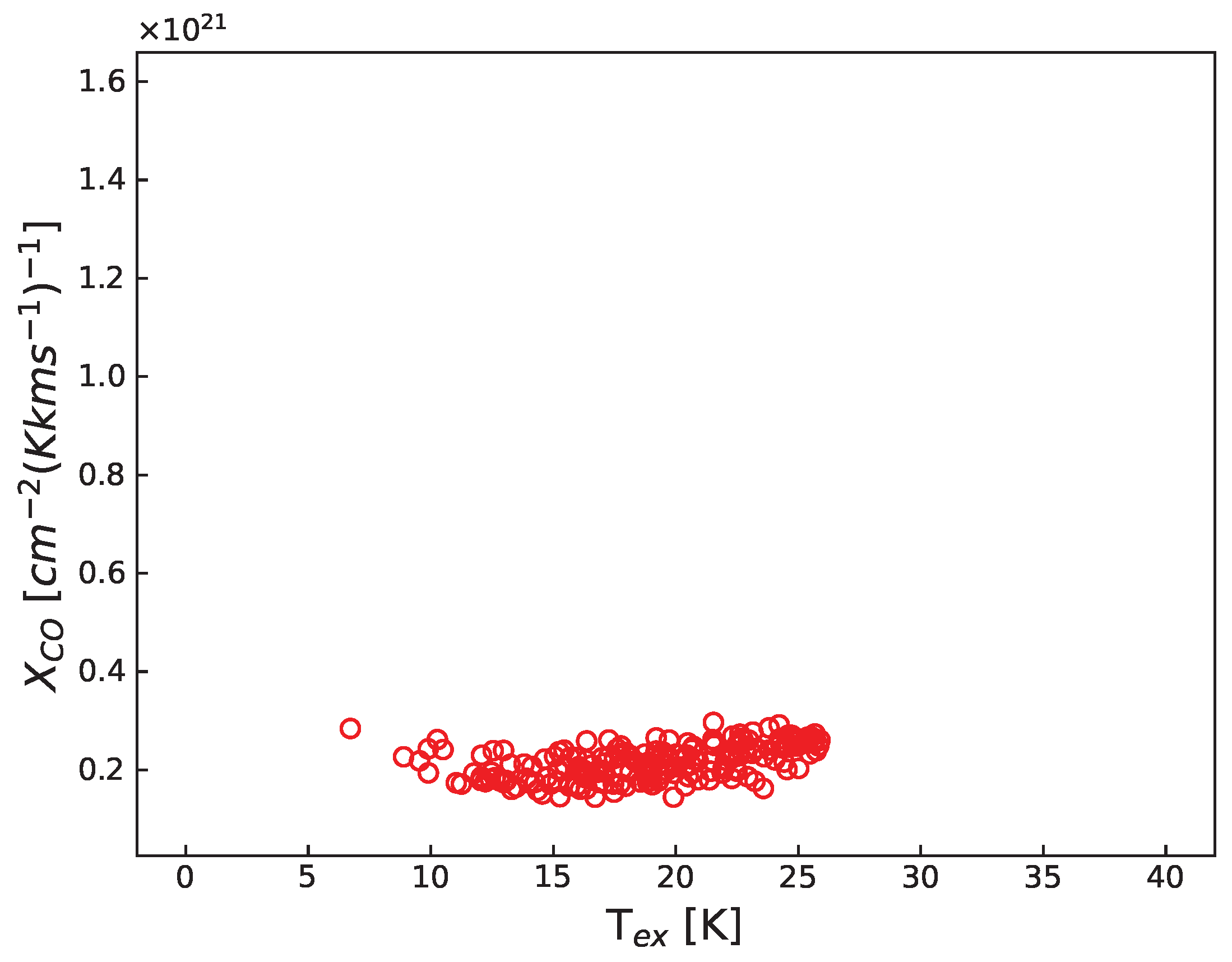
4.1. Factor
4.2. Variability in Factor
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| PDR | Photodissociation region |
| GMC | Giant molecular cloud |
| ALMA | Atacama Large Millimeter Array |
| LMC | Large Magellanic Cloud |
| LTE | Local thermodynamic equilibrium |
| ART−L | Additional Representative Image for Legacy |
| YSO | Young stellar object |
References
- Fukui, Y.; Kawamura, A. Molecular Clouds in Nearby Galaxies. Annu. Rev. Astron. Astrophys. 2010, 48, 547–580. [Google Scholar] [CrossRef]
- Naslim, N.; Tokuda, K.; Onishi, T.; Kemper, F.; Wong, T.; Morata, O.; Takada, S.; Harada, R.; Kawamura, A.; Saigo, K.; et al. ALMA Reveals Molecular Cloud N55 in the Large Magellanic Cloud as a Site of Massive Star Formation. Astrophys. J. 2018, 853, 175. [Google Scholar]
- Kennicutt, R.C.; Evans, N.J. Star Formation in the Milky Way and Nearby Galaxies. Annu. Rev. Astron. Astrophys. 2012, 50, 531. [Google Scholar] [CrossRef]
- Solomon, P.M.; Rivolo, A.R.; Barrett, J.; Yahil, A. Mass, Luminosity, and Line Width Relations of Galactic Molecular Clouds. Astrophys. J. 1987, 319, 730. [Google Scholar] [CrossRef]
- Bolatto, A.D.; Wolfire, M.; Leroy, A.K. The CO-to-H2 Conversion Factor. Annu. Rev. Astron. Astrophys. 2013, 51, 207–268. [Google Scholar] [CrossRef]
- Teng, Y.H.; Sandstrom, K.M.; Sun, J.; Leroy, A.K.; Johnson, L.C.; Bolatto, A.D.; Kruijssen, J.M.D.; Schruba, A.; Usero, A.; Barnes, A.T.; et al. Molecular Gas Properties and CO-to-H2 Conversion Factors in the Central Kiloparsec of NGC 3351. Astron. Astrophys. 2022, 925, 72. [Google Scholar] [CrossRef]
- Bolatto, A.D.; Leroy, A.K.; Rosolowsky, E.; Walter, F.; Blitz, L. The Resolved Properties of Extragalactic Giant Molecular Clouds. Astrophys. J. 2008, 686, 948–965. [Google Scholar] [CrossRef]
- Donovan, M.J.; Koda, J.; Momose, R.; Mooney, T.; Egusa, F.; Carty, M.; Kennicutt, R.; Kuno, N.; Rebolledo, D.; Sawada, T.; et al. Resolved Giant Molecular Clouds in Nearby Spiral Galaxies: Insights from the CANON CO (1-0) Survey. Astrophys. J. 2013, 772, 107. [Google Scholar] [CrossRef]
- Imara, N. Rethinking a Mysterious Molecular Cloud. Astrophys. J. 2015, 803, 38. [Google Scholar] [CrossRef]
- Leroy, A.K.; Bolatto, A.; Gordon, K.; Sandstrom, K.; Gratier, P.; Rosolowsky, E.; Engelbracht, C.W.; Mizuno, N.; Corbelli, E.; Fukui, Y.; et al. The CO-to-H2 Conversion Factor from Infrared Dust Emission across the Local Group. Astrophys. J. 2011, 737, 12. [Google Scholar] [CrossRef]
- Planck Collaboration; Ade, P.A.R.; Aghanim, N.; Arnaud, M.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Balbi, A.; Banday, A.J.; Barreiro, R.B.; et al. Planck early results. XIX. All-sky temperature and dust optical depth from Planck and IRAS. Constraints on the “dark gas” in our Galaxy. Astron. Astrophys. 2011, 536, 19. [Google Scholar]
- Abdo, A.A.; Ackermann, M.; Ajello, M.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; Baughman, B.M.; Bechtol, K.; Bellazzini, R.; et al. Fermi Observations of Cassiopeia and Cepheus: Diffuse Gamma-ray Emission in the Outer Galaxy. Astrophys. J. 2010, 710, 133–149. [Google Scholar] [CrossRef]
- Ackermann, M.; Ajello, M.; Allafort, A.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; Belfiore, A.; Bellazzini, R.; Berenji, B.; et al. The cosmic-ray and gas content of the Cygnus region as measured in γ-rays by the Fermi Large Area Telescope. Astron. Astrophys. 2012, 538, 71. [Google Scholar] [CrossRef]
- Remy, Q.; Grenier, I.A.; Marshall, D.J. Cosmic rays, gas and dust in nearby anticentre clouds. I. CO-to-H2 conversion factors and dust opacities. Astron. Astrophys. 2017, 601, 78. [Google Scholar] [CrossRef]
- Cormier, D.; Bigiel, F.; Jimenez-Donaire, M.J.; Leroy, A.K.; Gallagher, M.; Usero, A.; Sandstrom, K.; Bolatto, A.; Hughes, A.; Kramer, C.; et al. Full-disc 13CO(1-0) mapping across nearby galaxies of the EMPIRE survey and the CO-to-H2 conversion factor. Mon. Not. R. Astron. Soc. 2018, 475, 3909–3933. [Google Scholar] [CrossRef]
- Accurso, G.; Saintonge, A.; Catinella, B.; Cortese, L.; Dave, R.; Dunsheath, S.H.; Genzel, R.; Gracia-Carpio, J.; Heckman, T.M.; Kramer, C.; et al. Deriving a multivariate αCO conversion function using the [C II]/CO (1-0) ratio and its application to molecular gas scaling relations. Mon. Not. R. Astron. Soc. 2017, 470, 4750–4766. [Google Scholar]
- Bigiel, F.; de Looze, I.; Krabbe, A.; Cormier, D.; Barnes, A.T.; Fischer, C.; Bolatto, A.D.; Bryant, A.; Colditz, S.; Geis, N.; et al. SOFIA/FIFI-LS Full-disk [C II] Mapping and CO-dark Molecular Gas across the Nearby Spiral Galaxy NGC 6946. Astrophys. J. 2020, 903, 30. [Google Scholar] [CrossRef]
- Madden, S.C.; Cormier, D.; Hony, S.; Lebouteiller, V.; Abel, N.; Galametz, M.; De Looze, I.; Chevance, M.; Polles, F.L.; Lee, M.-Y.; et al. Tracing the total molecular gas in galaxies: [C II] and the CO-dark gas. Astron. Astrophys. 2020, 643, 141. [Google Scholar] [CrossRef]
- Luo, G.; Li, D.; Tang, N.; Dawson, J.R.; Dickey, J.M.; Bronfman, L.; Qin, S.-L.; Gibson, S.J.; Plambeck, R.; Finger, R.; et al. Revealing the CO X-factor in Dark Molecular Gas through Sensitive ALMA Absorption Observations. Astron. J. 2020, 889, 4. [Google Scholar] [CrossRef]
- Feldmann, R.; Gnedin, N.Y.; Kravtsov, A.V. The X-factor in Galaxies. I. Dependence on Environment and Scale. Astrophys. J. 2012, 747, 124. [Google Scholar] [CrossRef]
- Maloney, P.; Black, J.H. I CO/N(H2) Conversions and Molecular Gas Abundances in Spiral and Irregular Galaxies. Astrophys. J. 1988, 325, 389. [Google Scholar] [CrossRef]
- Narayanan, D.; Krumholz, M.R.; Ostriker, E.C.; Hernquist, L. A general model for the CO-H2 conversion factor in galaxies with applications to the star formation law. Mon. Not. R. Astron. Soc. 2012, 421, 3127–3146. [Google Scholar] [CrossRef]
- O’Neill, T.J.; Indebetouw, R.; Sandstrom, K.; Bolatto, A.D.; Jameson, K.E.; Carlson, L.R.; Finn, M.K.; Meixner, M.; Sabbi, E.; Sewilo, M. Sequential Star Formation in the Young SMC Region NGC 602: Insights from ALMA. Astrophys. J. 2022, 938, 82. [Google Scholar] [CrossRef]
- Shetty, R.; Glover, S.C.; Dullemond, C.P.; Ostriker, E.C.; Harris, A.I.; Klessen, R.S. Modelling CO emission-II. The physical characteristics that determine the X factor in Galactic molecular clouds. Mon. Not. R. Astron. Soc. 2011, 415, 3253–3274. [Google Scholar] [CrossRef]
- Pineda, J.L.; Goldsmith, P.F.; Chapman, N.; Snell, R.L.; Li, D.; Cambresy, L.; Brunt, C. The Relation Between Gas and Dust in the Taurus Molecular Cloud. Astrophys. J. 2010, 721, 686–708. [Google Scholar] [CrossRef]
- Goldsmith, P.F.; Heyer, M.; Narayanan, G.; Snell, R.; Li, D.; Brunt, C. Large-Scale Structure of the Molecular Gas in Taurus Revealed by High Linear Dynamic Range Spectral Line Mapping. Astrophys. J. 2008, 680, 428–445. [Google Scholar] [CrossRef]
- Papadopoulos, P.P.; Padelis, P.; Bisbas, T.G.; Zhang, Z.-Y. New places and phases of CO-poor/C I-rich molecular gas in the Universe. Mon. Not. R. Astron. Soc. 2018, 478, 1716–1725. [Google Scholar] [CrossRef]
- Gong, M.; Ostriker, E.C.; Kim, C.-G.; Kim, J.-G. The Environmental Dependence of the XCO Conversion Factor. Astrophys. J. 2020, 903, 142. [Google Scholar] [CrossRef]
- Kohno, M.; Sofue, Y. The CO-to-H2 conversion factor of Galactic giant molecular clouds using CO isotopologues: High-resolution XCO maps. Mon. Not. R. Astron. Soc. 2024, 527, 9290–9302. [Google Scholar] [CrossRef]
- Pineda, J.E.; Caselli, P.; Goodman, A.A. CO Isotopologues in the Perseus Molecular Cloud Complex: The X-factor and Regional Variations. Astrophys. J. 2008, 679, 481–496. [Google Scholar] [CrossRef]
- Sofue, Y.; Kohno, M. CO-to-H2 conversion and spectral column density in molecular clouds: The variability of the XCO factor. Mon. Not. R. Astron. Soc. 2020, 497, 1851–1861. [Google Scholar] [CrossRef]
- Massardi, M.; Stoehr, F.; Bendo, G.J.; Bonato, M.; Brand, J.; Galluzzi, V.; Guglielmetti, F.; Liuzzo, E.; Marchili, N.; Richards, A.M.S.; et al. The Additional Representative Images for Legacy (ARI-L) Project for the ALMA Science Archive. Publ. Astron. Soc. Pac. 2021, 133, 1026. [Google Scholar] [CrossRef]
- Gruendl, R.A.; Chu, Y.-H. High- and Intermediate-Mass Young Stellar Objects in the Large Magellanic Cloud. Astrophys. J. Suppl. 2009, 184, 172–197. [Google Scholar] [CrossRef]
- Seale, J.P.; Meixner, M.; Sewilo, M.; Babler, B.; Engelbracht, C.W.; Gordon, K.; Hony, S.; Misselt, K.; Montiel, E.; Okumura, K.; et al. Herschel Key Program Heritage: A Far-Infrared Source Catalog for the Magellanic Clouds. Astron. J. 2014, 148, 124. [Google Scholar] [CrossRef]
- Mizuno, Y.; Kawamura, A.; Onishi, T.; Minamidani, T.; Muller, E.; Yamamoto, H.; Hayakawa, T.; Mizuno, N.; Mizuno, A.; Stutzki, J.; et al. Warm and Dense Molecular Gas in the N 159 Region: 12COJ = 4-3 and 13COJ = 3-2 Observations with NANTEN2 and ASTE. Astron. Astrophys. 2010, 62, 51–67. [Google Scholar] [CrossRef]
- Fukui, Y.; Kawamura, A.; Minamidani, T.; Mizuno, Y.; Kanai, Y.; Mizuno, N.; Onishi, T.; Yonekura, Y.; Mizuno, A.; Ogawa, H.; et al. Mass, The Second Survey of the Molecular Clouds in the Large Magellanic Cloud by NANTEN. I. Catalog of Molecular Clouds. Astrophys. J. Suppl. Ser. 2008, 178, 56–70. [Google Scholar] [CrossRef]
- Hughes, A.; Wong, T.; Ott, J.; Muller, E.; Pineda, J.L.; Mizuno, Y.; Bernard, J.-P.; Paradis, D.; Maddison, S.; Reach, W.T.; et al. Physical properties of giant molecular clouds in the Large Magellanic Cloud. Mon. Not. R. Astron. Soc. 2010, 406, 2065–2086. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Q.; Li, M.; Zhang, L.; Pei, S. Variation in XCO Factor in N55 Region. Universe 2024, 10, 200. https://doi.org/10.3390/universe10050200
Li Q, Li M, Zhang L, Pei S. Variation in XCO Factor in N55 Region. Universe. 2024; 10(5):200. https://doi.org/10.3390/universe10050200
Chicago/Turabian StyleLi, Qiang, Mingyue Li, Li Zhang, and Songpeng Pei. 2024. "Variation in XCO Factor in N55 Region" Universe 10, no. 5: 200. https://doi.org/10.3390/universe10050200
APA StyleLi, Q., Li, M., Zhang, L., & Pei, S. (2024). Variation in XCO Factor in N55 Region. Universe, 10(5), 200. https://doi.org/10.3390/universe10050200





