A Zeroth Law Compatible Model to Kerr Black Hole Thermodynamics
Abstract
:1. Introduction
- The zeroth law of black hole mechanics states that the surface gravity κ of a stationary black hole is constant over the horizon, which is essentially the requirement of transitivity of the equilibrium state.
- The first law manifests a relation between variations in the mass M, horizon area A, and angular momentum J if the black hole is perturbed,where is the angular velocity of the horizon.
- The second law of black hole mechanics is Hawking’s area theorem, which states that the surface area of the event horizon never decreases with time,
- The third law is formulated by stating that it is impossible to achieve in a finite series of physical processes.
1.1. Equilibrium Compatibility
1.2. Zeroth Law Compatibility
1.3. A Parametric Approach
1.4. A Nonparametric Approach
2. Results
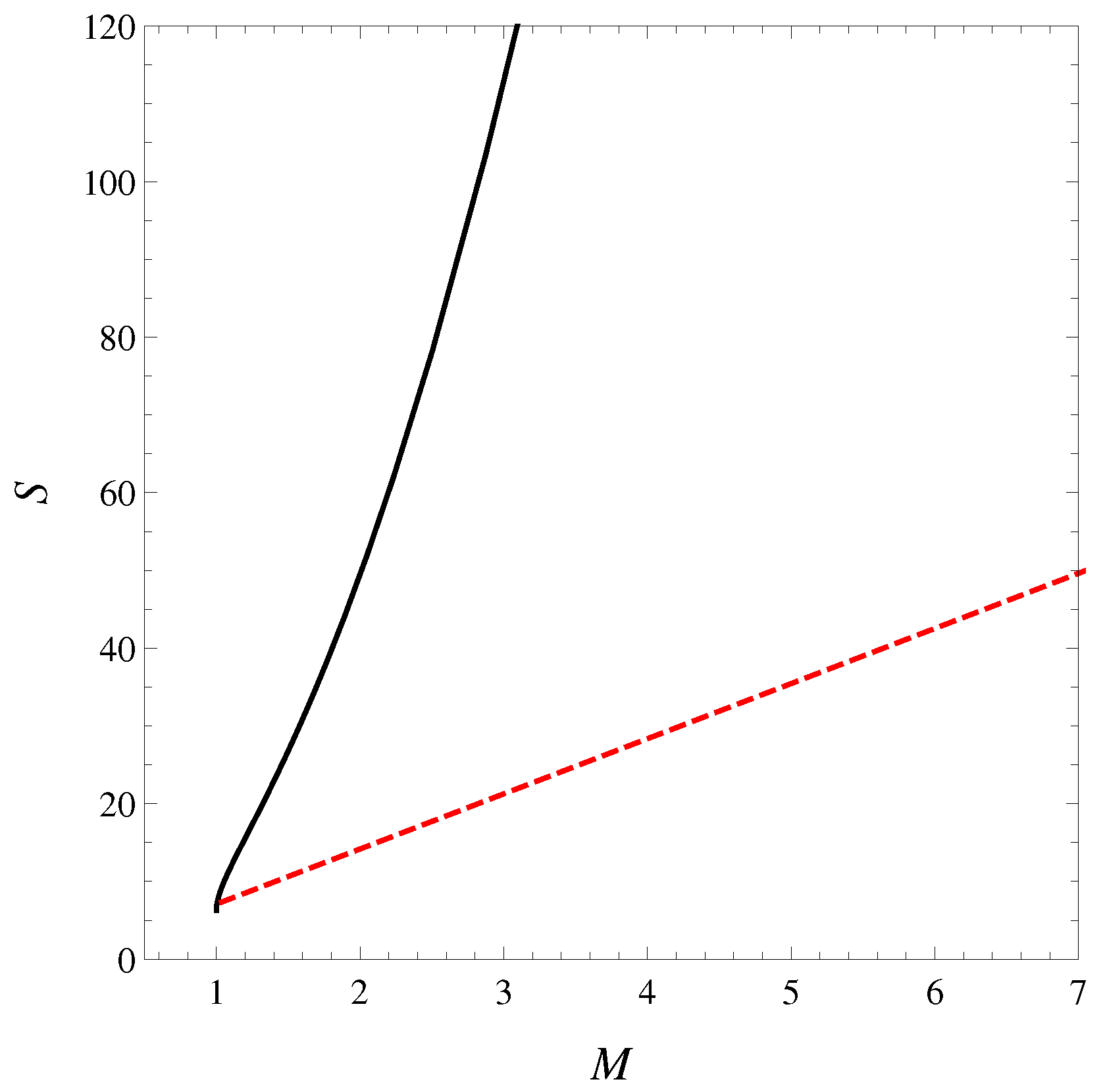
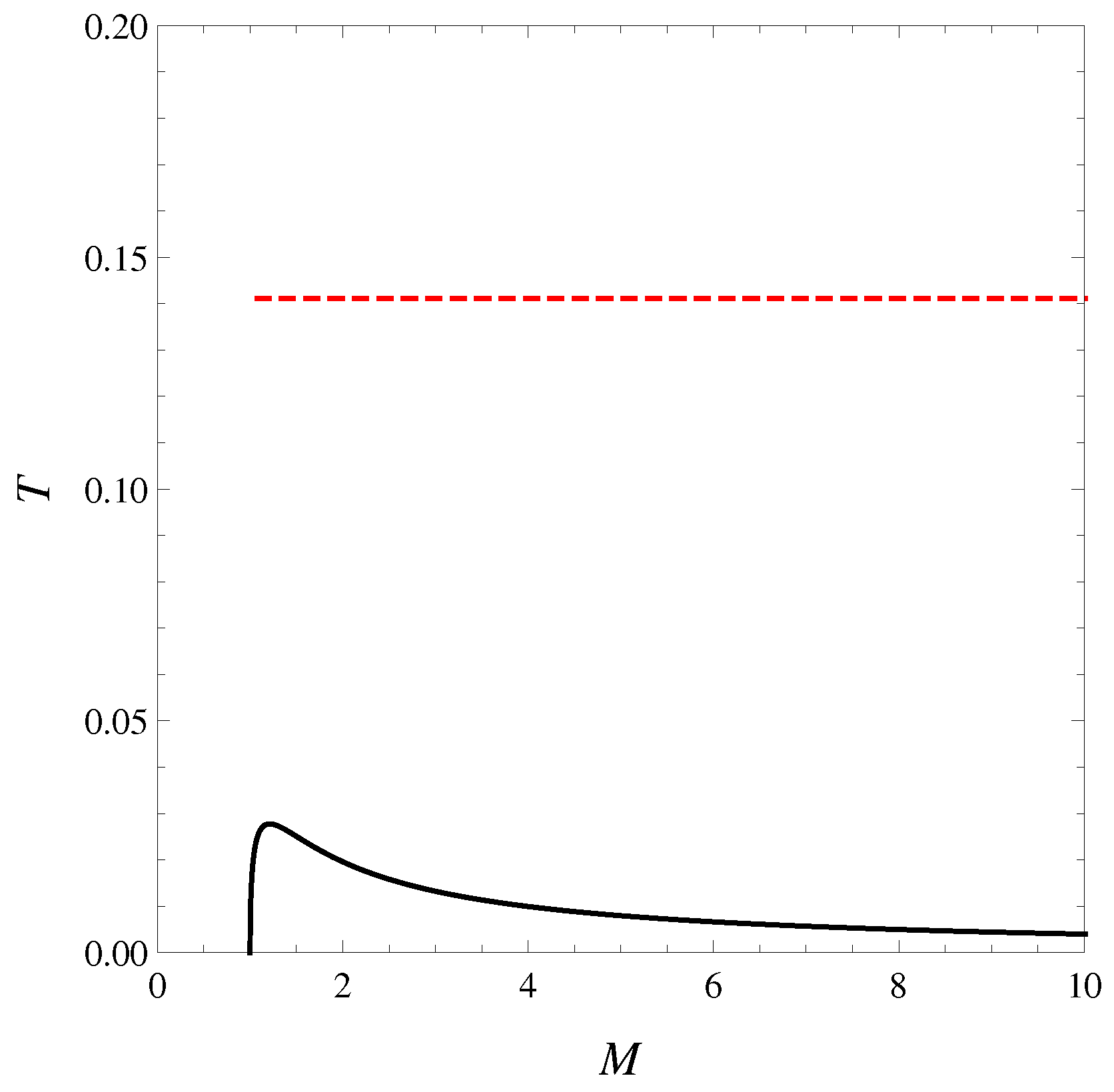
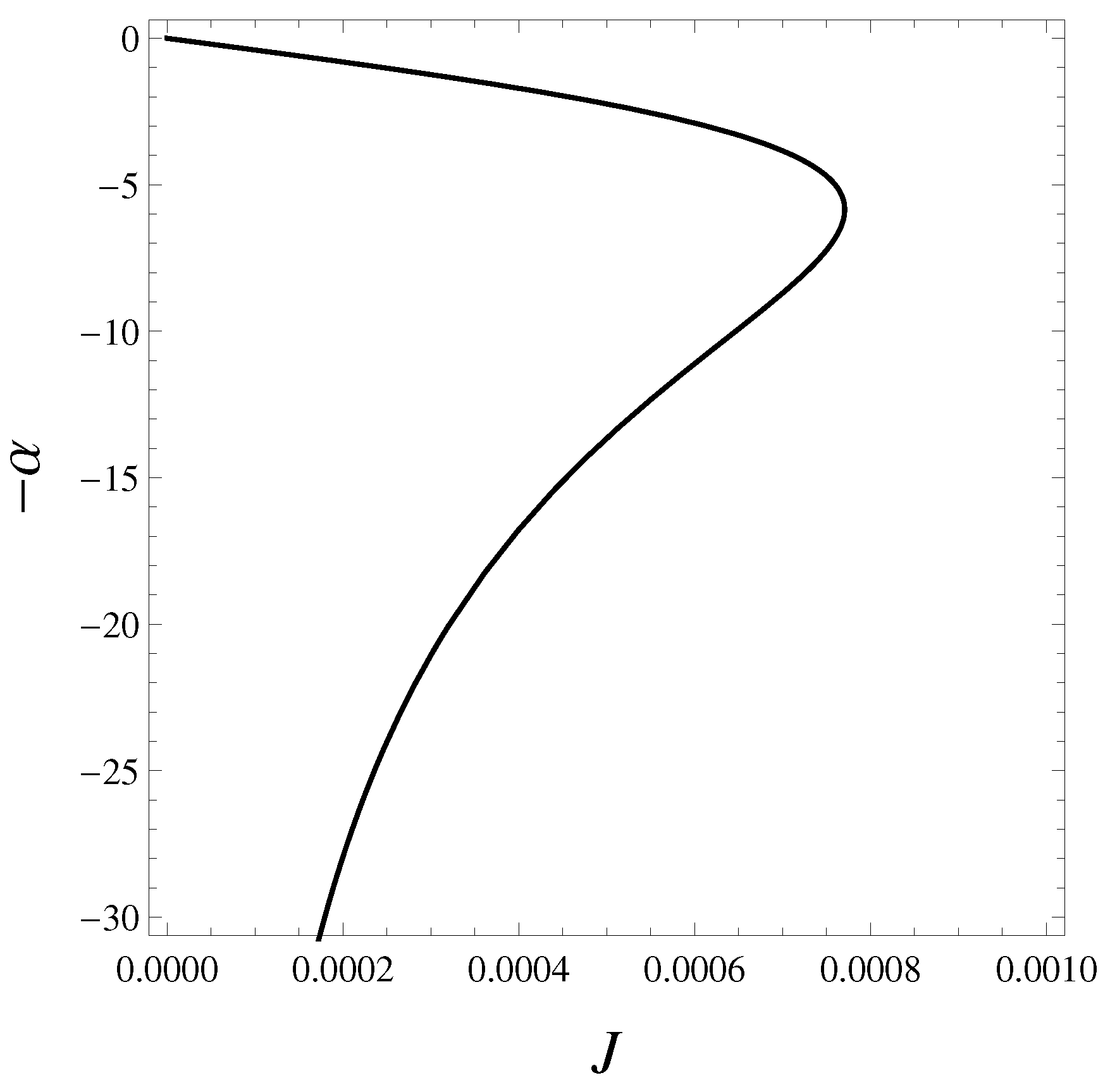
2.1. Kerr Black Holes
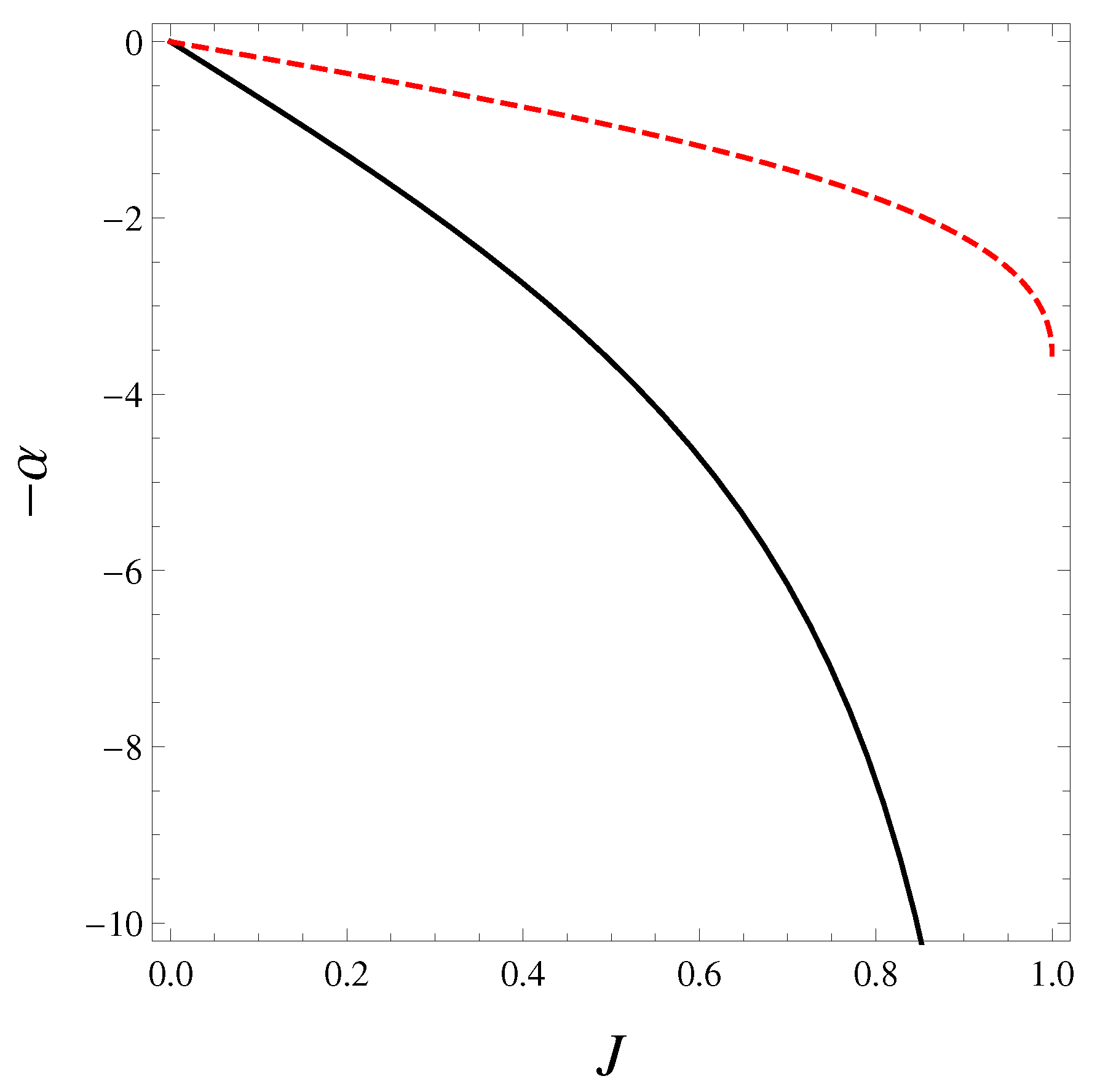
2.2. The Formal Logarithm Approach
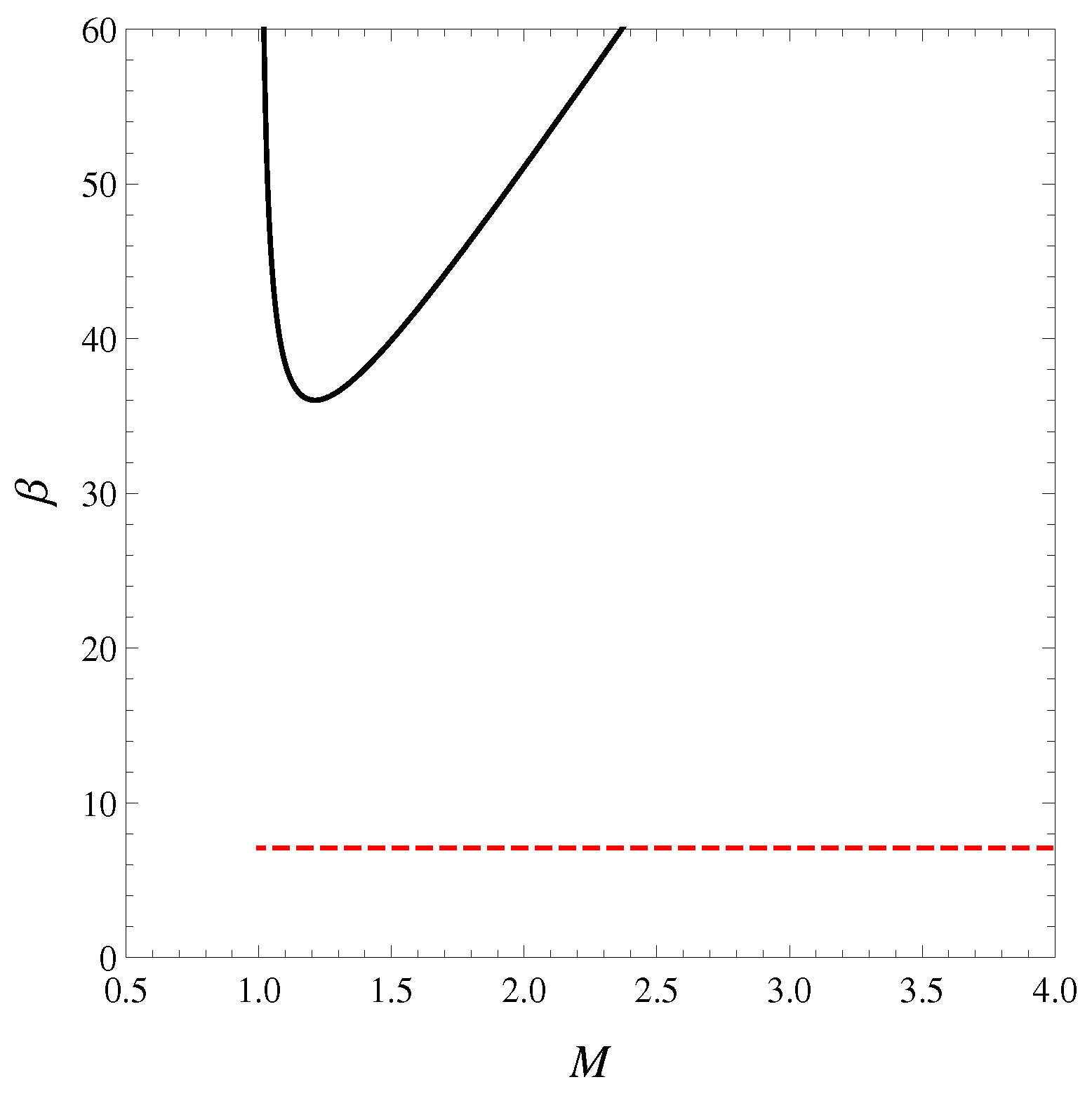
2.3. Stability Analysis
3. Discussion
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Kerr, R.P. Gravitational field of a spinning mass as an example of algebraically special metrics. Phys. Rev. Lett. 1963, 11, 237–238. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Carter, B.; Hawking, S.W. The Four laws of black hole mechanics. Commun. Math. Phys. 1973, 31, 161–170. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Black holes and entropy. Phys. Rev. D 1973, 7, 2333–2346. [Google Scholar] [CrossRef]
- Hawking, S.W. Particle Creation by Black Holes. Commun. Math. Phys. 1975, 43, 199–220. [Google Scholar] [CrossRef]
- Padmanabhan, T. Statistical Mechanics of Gravitating Systems. Phys. Rep. 1990, 188, 285–362. [Google Scholar] [CrossRef]
- Ong, Y.C. Never judge a black hole by its area. J. Cosmol. Astropart. Phys. 2015, 2015, 3. [Google Scholar] [CrossRef] [PubMed]
- Landsberg, P.T.; Tranah, D. Entropies need not to be concave. Phys. Lett. A 1980, 78, 219–220. [Google Scholar] [CrossRef]
- Bishop, N.; Landsberg, P. The thermodynamics of a system containing two black holes and black-body radiation. Gen. Relat. Gravit. 1987, 19, 1083–1090. [Google Scholar] [CrossRef]
- Landsberg, P. Is equilibrium always an entropy maximum? J. Stat. Phys. 1984, 35, 159–169. [Google Scholar] [CrossRef]
- Pavón, D.; Rubí, J. On some properties of the entropy of a system containing a black hole. Gen. Relat. Gravit. 1986, 18, 1245–1250. [Google Scholar] [CrossRef]
- Maddox, J. When entropy does not seem extensive. Nature 1993, 365, 103. [Google Scholar]
- Gour, G. Entropy bounds for charged and rotating systems. Class. Quantum Gravity 2003, 20, 3403–3412. [Google Scholar] [CrossRef]
- Oppenheim, J. Thermodynamics with long-range interactions: From Ising models to black holes. Phys. Rev. E 2003, 68, 016108. [Google Scholar] [CrossRef] [PubMed]
- Pesci, A. Entropy of gravitating systems: Scaling laws versus radial profiles. Class. Quantum Gravity 2007, 24, 2283–2300. [Google Scholar] [CrossRef]
- Aranha, R.F.; de Oliveira, H.P.; Damiao Soares, I.; Tonini, E.V. The Efficiency of Gravitational Bremsstrahlung Production in the Collision of Two Schwarzschild Black Holes. Int. J. Mod. Phys. D 2008, 17, 2049–2064. [Google Scholar] [CrossRef]
- Tsallis, C.; Cirto, L.J.L. Black hole thermodynamical entropy. Eur. Phys. J. C 2013, 73, 2487. [Google Scholar] [CrossRef]
- Czinner, V.G. Black hole entropy and the zeroth law of thermodynamics. Int. J. Mod. Phys. D 2015, 24, 1542015. [Google Scholar] [CrossRef]
- Abe, S. General pseudoadditivity of composable entropy prescribed by the existence of equilibrium. Phys. Rev. E 2001, 63, 061105. [Google Scholar] [CrossRef] [PubMed]
- Tsallis, C. Possible Generalization of Boltzmann-Gibbs Statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Tsallis, C. Introduction to Non-Extensive Statistical Mechanics: Approaching a Complex World; Springer: Berlin, Germany, 2009. [Google Scholar]
- Biró, T.S.; Ván, P. Zeroth law compatibility of nonadditive thermodynamics. Phys. Rev. E 2011, 83, 061147. [Google Scholar] [CrossRef] [PubMed]
- Rényi, A. On the dimension and entropy of probability distributions. Acta Math. Acad. Sci. Hung. 1959, 10, 193–215. [Google Scholar] [CrossRef]
- Renyi, A. Probability Theory; Elsevier: Amsterdam, The Netherlands, 1970. [Google Scholar]
- Biró, T.S.; Czinner, V.G. A q-parameter bound for particle spectra based on black hole thermodynamics with Rényi entropy. Phys. Lett. B 2013, 726, 861–865. [Google Scholar] [CrossRef]
- Czinner, V.G.; Iguchi, H. Rényi entropy and the thermodynamic stability of black holes. Phys. Lett. B 2016, 752, 306–310. [Google Scholar] [CrossRef] [Green Version]
- Arcioni, G.; Lozano-Tellechea, E. Stability and critical phenomena of black holes and black rings. Phys. Rev. D 2005, 72, 104021. [Google Scholar] [CrossRef]
- Kaburaki, O.; Okamoto, I.; Katz, J. Thermodynamic Stability of Kerr Black holes. Phys. Rev. D 1993, 47, 2234–2241. [Google Scholar] [CrossRef]
- Poincaré, H. Sur l’équilibre d’une masse fluide animée d’un mouvement de rotation. Acta Math. 1885, 7, 259–380. [Google Scholar] [CrossRef]
- Katz, J.; Okamoto, I.; Kaburaki, O. Thermodynamic stability of pure black holes. Class. Quantum Gravity 1993, 10, 1323–1339. [Google Scholar] [CrossRef]
- Kaburaki, O. Critical behavior of extremal Kerr-Newman black holes. Gen. Relat. Gravit. 1996, 28, 843–854. [Google Scholar] [CrossRef]
- Azreg-Aïnou, M.; Rodrigues, M.E. Thermodynamical, geometrical and Poincaré methods for charged black holes in presence of quintessence. J. High Energy Phys. 2013, 2013, 146. [Google Scholar] [CrossRef]
- Hawking, S.W.; Page, D.N. Thermodynamics of Black Holes in anti-De Sitter Space. Commun. Math. Phys. 1983, 87, 577–588. [Google Scholar] [CrossRef]
- Czinner, V.G.; Iguchi, H. Thermodynamics, stability and Hawking–Page transition of Kerr black holes from Rényi statistics. 2017; in preparation. [Google Scholar]
- Caldarelli, M.M.; Cognola, G.; Klemm, D. Thermodynamics of Kerr-Newman-AdS black holes and conformal field theories. Class. Quantum Gravity 2000, 17, 399–420. [Google Scholar] [CrossRef]
- Tsai, Y.D.; Wu, X.N.; Yang, Y. Phase Structure of Kerr-AdS Black Hole. Phys. Rev. D 2012, 85, 044005. [Google Scholar] [CrossRef]
- Altamirano, N.; Kubiznak, D.; Mann, R.B.; Sherkatghanad, Z. Thermodynamics of rotating black holes and black rings: Phase transitions and thermodynamic volume. Galaxies 2014, 2, 89–159. [Google Scholar] [CrossRef]
- Biró, T.S. Ideal gas provides q-entropy. Physica A 2013, 392, 3132–3139. [Google Scholar] [CrossRef]
- Biró, T.S.; Barnaföldi, G.G.; Ván, P. Quark-gluon plasma connected to finite heat bath. Eur. Phys. J. A 2013, 49, 110. [Google Scholar] [CrossRef]
- Carlip, S. Black Hole Thermodynamics. Int. J. Mod. Phys. D 2014, 23, 1430023. [Google Scholar] [CrossRef]
- Bekenstein, J.D. A Universal Upper Bound on the Entropy to Energy Ratio for Bounded Systems. Phys. Rev. D 1981, 23, 287–298. [Google Scholar] [CrossRef]

© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Czinner, V.G.; Iguchi, H. A Zeroth Law Compatible Model to Kerr Black Hole Thermodynamics. Universe 2017, 3, 14. https://doi.org/10.3390/universe3010014
Czinner VG, Iguchi H. A Zeroth Law Compatible Model to Kerr Black Hole Thermodynamics. Universe. 2017; 3(1):14. https://doi.org/10.3390/universe3010014
Chicago/Turabian StyleCzinner, Viktor G., and Hideo Iguchi. 2017. "A Zeroth Law Compatible Model to Kerr Black Hole Thermodynamics" Universe 3, no. 1: 14. https://doi.org/10.3390/universe3010014
APA StyleCzinner, V. G., & Iguchi, H. (2017). A Zeroth Law Compatible Model to Kerr Black Hole Thermodynamics. Universe, 3(1), 14. https://doi.org/10.3390/universe3010014




