Spinning Test Particle in Four-Dimensional Einstein–Gauss–Bonnet Black Holes
Abstract
:1. Introduction
2. Motion of a Spinning Test Particle in a Four-Dimensional EGB Black Hole
2.1. Four-Momentum and Four-Velocity of the Spinning Test Particle
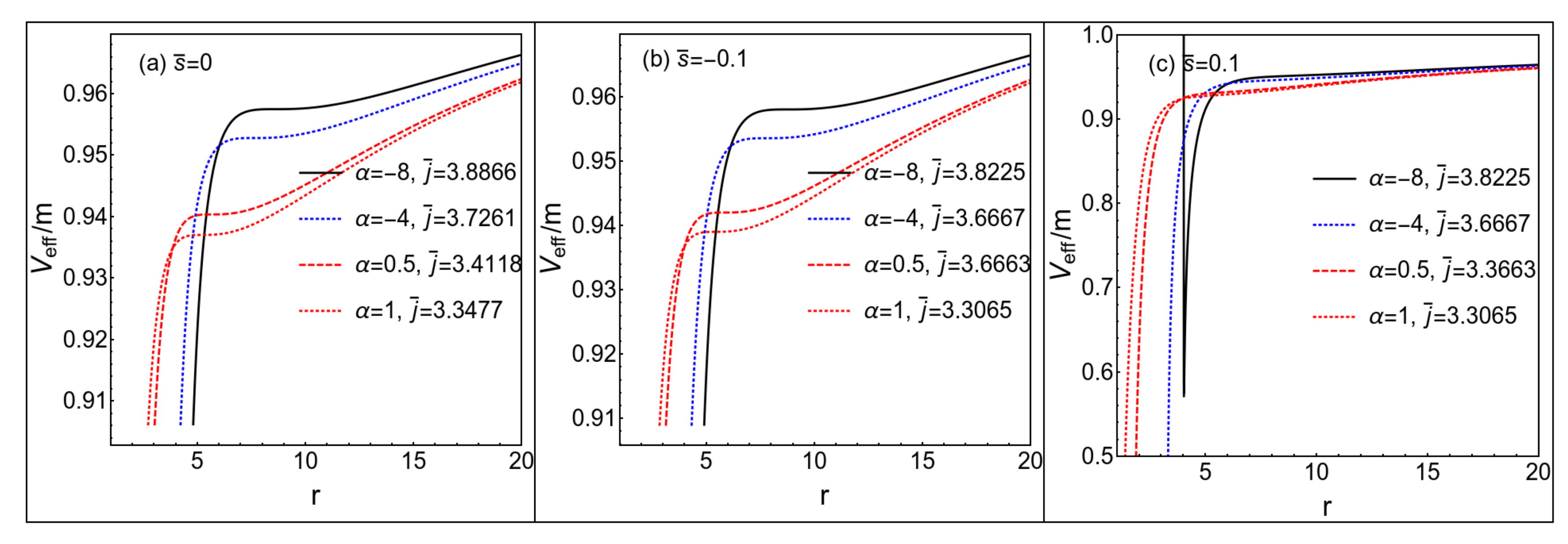
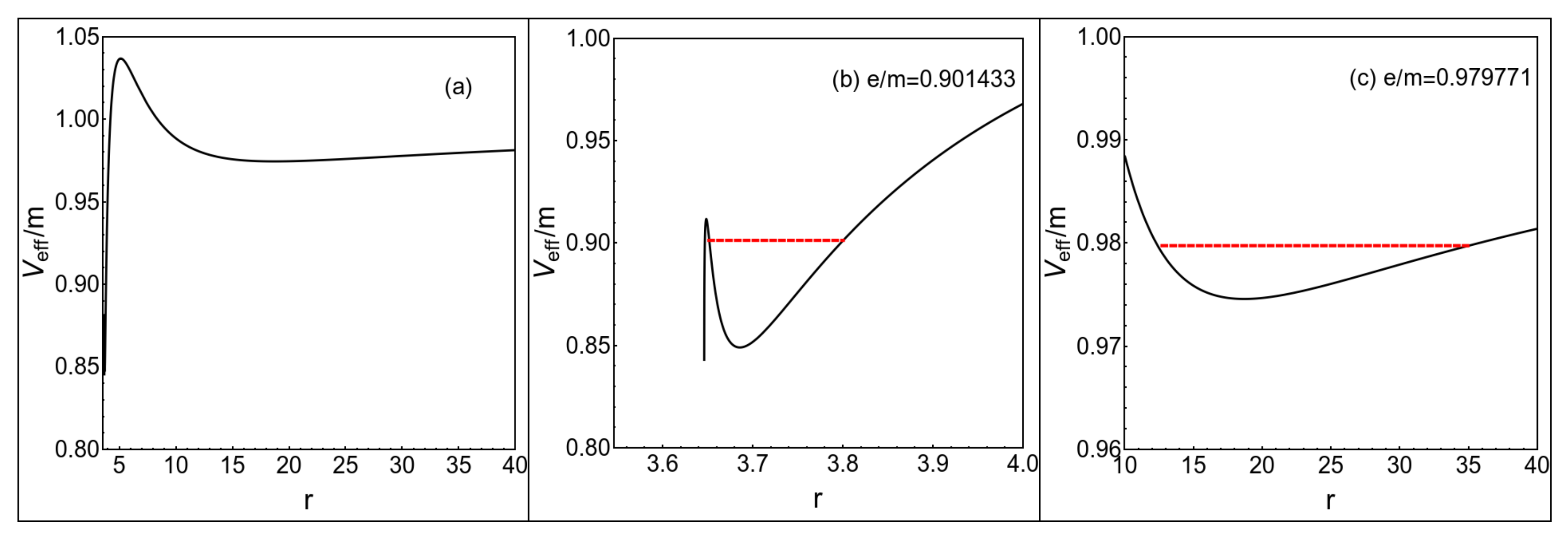
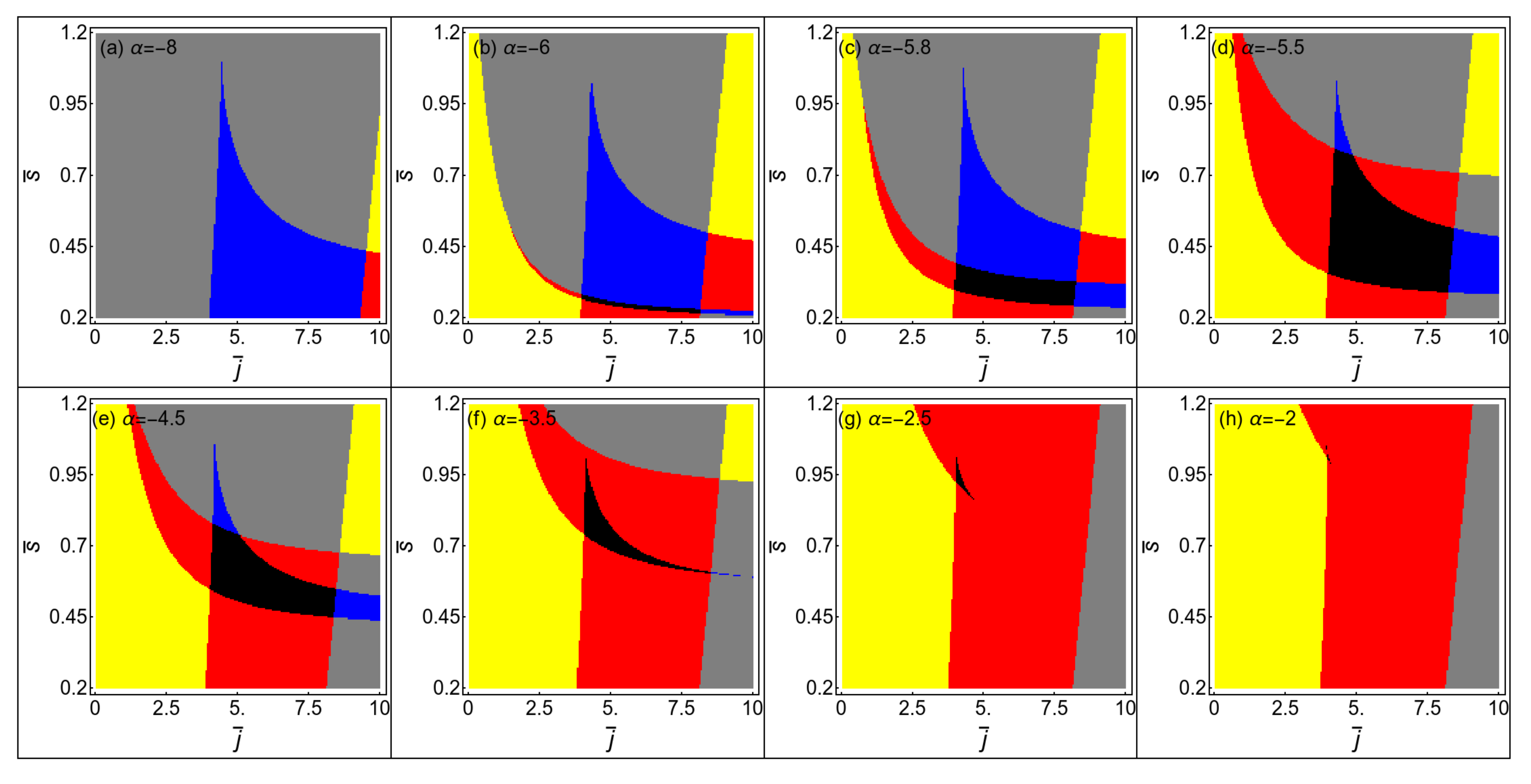
2.2. Circular Orbits of Spinning Test Particle
- For the ISCO of the spinning test particle in four-dimensional EGB black hole, the corresponding radius and angular momentum decrease with the spin when the GB coupling parameter is fixed. When the effect from the GB term is considered, the radius of the ISCO will be smaller than the case of the Schwarzchild black hole in GR, and the Gauss–Bonnet term does not change the laws of the ISCO with spin.
- When the spin of the test particle is fixed, the radius and angular momentum of the ISCO decrease with the GB coupling parameter and this behavior is almost the same as the results of the spinless case in Ref. [38].
3. Summary
Author Contributions
Funding
Conflicts of Interest
References
- Abbott, B.; Jawahar, S.; Lockerbie, N.; Tokmakov, K. [LIGO Scientific Collaboration and Virgo Collaboration]. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.; Jawahar, S.; Lockerbie, N.; Tokmakov, K. [LIGO Scientific Collaboration and Virgo Collaboration]. GW151226: Observation of Gravitational Waves from a 22-Solar-Mass Binary Black Hole Coalescence. Phys. Rev. Lett. 2016, 116, 241103. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B. [LIGO Scientific Collaboration and Virgo Collaboration]. GW170104: Observation of a 50-Solar-Mass Binary Black Hole Coalescence at Redshift 0.2. Phys. Rev. Lett. 2017, 118, 221101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. [LIGO Scientific Collaboration and Virgo Collaboration]. GW170814: A Three-Detector Observation of Gravitational Waves from a Binary Black Hole Coalescence. Phys. Rev. Lett. 2017, 119, 141101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abbott, B.; Jawahar, S.; Lockerbie, N.; Tokmakov, K. [LIGO Scientific Collaboration and Virgo Collaboration]. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef] [Green Version]
- Banados, M.; Silk, J.; West, S.M. Kerr Black Holes as Particle Accelerators to Arbitrarily High Energy. Phys. Rev. Lett. 2009, 103, 111102. [Google Scholar] [CrossRef] [Green Version]
- Wei, S.-W.; Liu, Y.-X.; Guo, H.; Fu, C.-E. Charged spinning black holes as Particle Accelerators. Phys. Rev. D 2010, 82, 103005. [Google Scholar] [CrossRef] [Green Version]
- Penrose, R. Gravitational collapse and space-time singularities. Phys. Rev. Lett. 1965, 14, 57. [Google Scholar] [CrossRef] [Green Version]
- Hawking, S.W.; Penrose, R. The Singularities of gravitational collapse and cosmology. Proc. R. Soc. Lond. A 1970, 314, 529–548. [Google Scholar]
- Gross, D.J.; Witten, E. Superstring Modifications of Einstein’s Equations. Nucl. Phys. B 1986, 277, 1–10. [Google Scholar] [CrossRef]
- Gross, D.J.; Sloan, J.H. The Quartic Effective Action for the Heterotic String. Nucl. Phys. B 1987, 291, 41–89. [Google Scholar] [CrossRef]
- Bento, M.C.; Bertolami, O. Maximally Symmetric Cosmological Solutions of higher curvature string effective theories with dilatons. Phys. Lett. B 1996, 368, 198–201. [Google Scholar] [CrossRef] [Green Version]
- Zwiebach, B. Curvature Squared Terms and String Theories. Phys. Lett. B 1985, 156, 315–317. [Google Scholar] [CrossRef]
- Boulware, D.G.; Deser, S. String-generated gravity models. Phys. Rev. Lett. 1985, 55, 2656–2660. [Google Scholar] [CrossRef] [Green Version]
- Wiltshire, D.L. Spherically symmetric solutions of Einstein-Maxwell theory with a Gauss-Bonnet term. Phys. Lett. B 1986, 169, 36–40. [Google Scholar] [CrossRef]
- Wiltshire, D.L. Black holes in string-generated gravity models. Phys. Rev. D 1988, 38, 2445. [Google Scholar] [CrossRef] [PubMed]
- Cai, R.-G. Gauss-Bonnet black holes in AdS spaces. Phys. Rev. D 2002, 65, 084014. [Google Scholar] [CrossRef] [Green Version]
- Glavan, D.; Lin, C. Einstein-Gauss-Bonnet Gravity in Four-Dimensional Spacetime. Phys. Rev. Lett. 2020, 124, 081301. [Google Scholar] [CrossRef] [Green Version]
- Cognola, G.; Myrzakulov, R.; Sebastiani, L.; Zerbini, S. Einstein gravity with Gauss-Bonnet entropic corrections. Phys. Rev. D 2013, 88, 024006. [Google Scholar] [CrossRef] [Green Version]
- Konoplya, R.A.; Zinhailo, A.F. Quasinormal modes, stability and shadows of a black hole in the novel 4D Einstein-Gauss-Bonnet gravity. arXiv 2020, arXiv:2003.01188. [Google Scholar]
- Fernandes, P.G.S. Charged Black Holes in AdS Spaces in 4D Einstein Gauss-Bonnet Gravity. Phys. Lett. B 2020, 805, 135468. [Google Scholar] [CrossRef]
- Wei, S.-W.; Liu, Y.-X. Testing the nature of Gauss-Bonnet gravity by four-dimensional rotating black hole shadow. arXiv 2020, arXiv:2003.07769. [Google Scholar]
- Konoplya, R.A.; Zhidenko, A. Black holes in the four-dimensional Einstein-Lovelock gravity. arXiv 2020, arXiv:2003.07788. [Google Scholar] [CrossRef] [Green Version]
- Casalino, A.; Colleaux, A.; Rinaldi, M.; Vicentini, S. Regularized Lovelock gravity. arXiv 2020, arXiv:2003.07068. [Google Scholar]
- Ai, W.-Y. A note on the novel 4D Einstein-Gauss-Bonnet gravity. arXiv 2020, arXiv:2004.02858. [Google Scholar]
- Bonifacio, J.; Hinterbichler, K.; Johnson, L.A. Amplitudes and 4D Gauss-Bonnet Theory. Phys. Rev. D 2020, 102, 024029. [Google Scholar] [CrossRef]
- Metin, G.; Sisman, T.C.; Tekin, B. Is there a novel Einstein-Gauss-Bonnet theory in four dimensions? arXiv 2020, arXiv:2004.03390. [Google Scholar]
- Hennigar, R.A.; Kubiznak, D.; Mann, R.B.; Pollack, C. On Taking the D→4 limit of Gauss-Bonnet Gravity: Theory and Solutions. J. High Energy Phys. 2020, 2020, 27. [Google Scholar] [CrossRef]
- Aoki, K.; Gorji, M.A.; Mukohyama, S. A consistent theory of D→4 Einstein-Gauss-Bonnet gravity. arXiv 2020, arXiv:2005.03859. [Google Scholar]
- Shu, F.-W. Vacua in novel 4D Einstein-Gauss-Bonnet Gravity: Pathology and instability? arXiv 2020, arXiv:2004.09339. [Google Scholar]
- Mahapatra, S. A note on the total action of 4D Gauss-Bonnet theory. arXiv 2020, arXiv:2004.09214. [Google Scholar]
- Tian, S.-X.; Zhu, Z.-H. Comment on “Einstein-Gauss-Bonnet Gravity in Four-Dimensional Spacetime”. arXiv 2020, arXiv:2004.09954. [Google Scholar]
- Arrechea, J.; Delhom, A.; Jiménez-Cano, A. Yet another comment on four-dimensional Einstein-Gauss-Bonnet gravity. arXiv 2020, arXiv:2004.12998. [Google Scholar]
- Lu, H.; Pang, Y. Horndeski Gravity as D→4 Limit of Gauss-Bonnet. arXiv 2020, arXiv:2003.11552. [Google Scholar]
- Kobayashi, T. Effective scalar-tensor description of regularized Lovelock gravity in four dimensions. J. Cosmol. Astropart. Phys. 2020, 2020, 013. [Google Scholar] [CrossRef]
- Fernandes, P.G.S.; Carrilho, P.; Clifton, T.; Mulryne, D.J. Derivation of Regularized Field Equations for the Einstein-Gauss-Bonnet Theory in Four Dimensions. Phys. Rev. D 2020, 102, 024025. [Google Scholar] [CrossRef]
- Page, D.N.; Thorne, K.S. Disk-accretion onto a black hole. Time-averaged structure of accretion disk. Astrophys. J. 1974, 191, 499–506. [Google Scholar] [CrossRef]
- Guo, M.-Y.; Li, P.-C. The innermost stable circular orbit and shadow in the novel 4D Einstein-Gauss-Bonnet gravity. Eur. Phys. J. C 2020, 80, 588. [Google Scholar] [CrossRef]
- Suzuki, S.; Maeda, K. Innermost stable circular orbit of a spinning particle in Kerr space-time. Phys. Rev. D 1998, 58, 023005. [Google Scholar] [CrossRef] [Green Version]
- Cai, R.-G.; Cao, L.-M.; Ohta, N. Black Holes in Gravity with Conformal Anomaly and Logarithmic Term in Black Hole Entropy. J. High Energy Phys. 2010, 2010, 082. [Google Scholar] [CrossRef] [Green Version]
- Cai, R.-G. Thermodynamics of Conformal Anomaly Corrected Black Holes in AdS Space. Phys. Lett. B 2014, 733, 183–189. [Google Scholar] [CrossRef] [Green Version]
- Mathisson, M. New mechanics of material systems. Acta Phys. Pol. 1937, 6, 163. [Google Scholar]
- Papapetrou, A. Spinning test-particles in general relativity. I. Proc. R. Soc. Lond. A 1951, 209, 248–258. [Google Scholar]
- Corinaldesi, E.; Papapetrou, A. Spinning test-particles in general relativity. II. Proc. R. Soc. Lond. A 1951, 209, 259–268. [Google Scholar]
- Dixon, W.G. Dynamics of extended bodies in general relativity II. Moments of the charge-current vector. Proc. R. Soc. Lond. A 1970, 319, 509–547. [Google Scholar]
- Hojman, S.A. Electromagnetic and Gravitational Interactions of a Spherical Relativistic Top. Unpublished Ph.D. Thesis, Princeton University, Princeton, NJ, USA, 1975. [Google Scholar]
- Hojman, R.; Hojman, S. Spinning Charged Test Particles in a Kerr-Newman Background. Phys. Rev. D 1977, 15, 2724. [Google Scholar] [CrossRef]
- Mashhoon, B.; Singh, D. Dynamics of Extended Spinning Masses in a Gravitational Field. Phys. Rev. D 2006, 74, 124006. [Google Scholar] [CrossRef] [Green Version]
- Zalaquett, N.; Hojman, S.A.; Asenjo, F.A. Spinning massive test particles in cosmological and general static spherically symmetric spacetimes. Class. Quant. Grav. 2014, 31, 085011. [Google Scholar] [CrossRef] [Green Version]
- Uchupol, R.; Sarah, J.V.; Scott, A.H. Gyroscopes orbiting black holes: A frequency-domain approach to precession and spin-curvature coupling for spinning bodies on generic Kerr orbits. Phys. Rev. D 2016, 94, 044008. [Google Scholar]
- Armaza, C.; Banados, M.; Koch, B. Collisions of spinning massive particles in a Schwarzschild background. Class. Quantum Gravity 2016, 33, 105014. [Google Scholar] [CrossRef]
- Wald, R.M. Gravitational spin interaction. Phys. Rev. D 1972, 6, 406–413. [Google Scholar] [CrossRef]
- Lukes-Gerakopoulos, G.; Seyrich, J.; Kunst, D. Investigating spinning test particles: Spin supplementary conditions and the Hamiltonian formalism. Phys. Rev. D 2014, 90, 104019. [Google Scholar] [CrossRef] [Green Version]
- Filipe, L.; Costa, O.; Lukes-Gerakopoulos, G.; Semerák, O. On spinning particles in general relativity: Momentum-velocity relation for the Mathisson-Pirani spin condition. Phys. Rev. D 2018, 97, 084023. [Google Scholar]
- Lukes-Gerakopoulos, G.; Harms, E.; Bernuzzi, S.; Nagar, A. Spinning test-body orbiting around a Kerr black hole: Circular dynamics and gravitational-wave fluxes. Phys. Rev. D 2017, 96, 064051. [Google Scholar] [CrossRef] [Green Version]
- Lukes-Gerakopoulos, G. Time parameterizations and spin supplementary conditions of the Mathisson- Papapetrou-Dixon equations. Phys. Rev. D 2017, 96, 104023. [Google Scholar] [CrossRef] [Green Version]
- Tulczyjew, W. Motion of multipole particles in general relativity theory. Acta Phys. Pol. 1959, 18, 393. [Google Scholar]
- Deriglazov, A.A.; Ramírez, W.G. Mathisson-Papapetrou-Tulczyjew-Dixon (MPTD) equations in ultra-relativistic regime and gravimagnetic moment. Int. J. Mod. Phys. D 2016, 26, 1750047. [Google Scholar] [CrossRef] [Green Version]
- Deriglazov, A.A.; Ramírez, W.G. Ultrarelativistic Spinning Particle and a Rotating Body in External Fields. Adv. High Energy Phys. 2016, 2016, 1376016. [Google Scholar] [CrossRef]
- Ramírez, W.G.; Deriglazov, A.A. Relativistic effects due to gravimagnetic moment of a rotating body. Phys. Rev. D 2017, 96, 124013. [Google Scholar] [CrossRef] [Green Version]
- Deriglazov, A.A.; Ramírez, W.G. Recent progress on the description of relativistic spin: Vector model of spinning particle and rotating body with gravimagnetic moment in General Relativity. Adv. Math. Phys. 2017, 2017, 7397159. [Google Scholar] [CrossRef] [Green Version]
- Steinhoff, J.; Puetzfeld, D. Multipolar equations of motion for extended test bodies in general relativity. Phys. Rev. D 2010, 81, 044019. [Google Scholar] [CrossRef] [Green Version]
- Han, W.-B. Gravitational Radiations from a Spinning Compact Object around a supermassive Kerr black hole in circular orbit. Phys. Rev. D 2010, 82, 084013. [Google Scholar] [CrossRef] [Green Version]
- Harms, E.; Lukes-Gerakopoulos, G.; Bernuzzi, S.; Nagar, A. Spinning test body orbiting around a Schwarzschild black hole: Circular dynamics and gravitational-wave fluxes. Phys. Rev. D 2016, 94, 104010. [Google Scholar] [CrossRef] [Green Version]
- Mukherjee, S.; Nayak, K.R. Off-equatorial stable circular orbits for spinning particles. Phys. Rev. D 2018, 98, 084023. [Google Scholar] [CrossRef] [Green Version]
- Zhang, M.; Liu, W.-B. Innermost stable circular orbits of charged spinning test particles. Phys. Lett. B 2019, 789, 393. [Google Scholar] [CrossRef]
- Pugliese, D.; Quevado, H.; Ruffini, R. Equatorial circular orbits of neutral test particles in the Kerr Newman spacetime. Phys. Rev. D 2013, 88, 024042. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.-P.; Wei, S.-W.; Guo, W.-D.; Sui, T.-T.; Liu, Y.-X. Innermost stable circular orbit of spinning particle in charged spinning black hole background. Phys. Rev. D 2018, 97, 084056. [Google Scholar] [CrossRef] [Green Version]
- Stuchlík, Z. Equilibrium of spinning test particles in the Schwarzschild-de Sitter spacetimes. Acta Phys. Slov. 1999, 49, 319. [Google Scholar]
- Stuchlík, Z.; Ková, J. Equilibrium conditions of spinning test particles in Kerr-de Sitter spacetimes. Class. Quant. Grav. 2006, 23, 3935. [Google Scholar] [CrossRef]
- Plyatsko, R.; Fenyk, M.; Panat, V. Highly relativistic spin-gravity-Λ coupling. Phys. Rev. D 2017, 96, 064038. [Google Scholar] [CrossRef] [Green Version]
- Plyatsko, R.; Panat, V.; Fenyk, M. Nonequatorial circular orbits of spinning particles in the Schwarzschild-de Sitter background. Gen. Relat. Grav. 2018, 50, 150. [Google Scholar] [CrossRef] [Green Version]
- Han, W.-B. Dynamics of extended bodies with spin-induced quadrupole in Kerr spacetime: Generic orbits. Gen. Relat. Grav. 2017, 49, 48. [Google Scholar] [CrossRef] [Green Version]
- Warburton, N.; Osburn, T.; Evans, C.R. Evolution of small-mass-ratio binaries with a spinning secondary. Phys. Rev. D 2017, 96, 084057. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Liu, W.-B. Energy extraction of a spinning particle via the super Penrose process from an extremal Kerr black hole. Phys. Rev. D 2018, 97, 064024. [Google Scholar] [CrossRef]
- Mukherjee, S. Collisional Penrose process with spinning particles. Phys. Lett. B 2018, 778, 54–59. [Google Scholar] [CrossRef]
- Faye, G.; Blanchet, L.; Buonanno, A. Higher-order spin effects in the dynamics of compact binaries. I. Equations of motion. Phys. Rev. D 2006, 74, 104033. [Google Scholar] [CrossRef] [Green Version]
- Witzany, V.; Steinhoff, J.; Lukes-Gerakopoulos, G. Hamiltonians and canonical coordinates for spinning particles in curved space-time. Class. Quantum Gravity 2019, 36, 075003. [Google Scholar] [CrossRef] [Green Version]
- Jefremov, P.I.; Tsupko, O.Y.; Bisnovatyi-Kogan, G.S. Innermost stable circular orbits of spinning test particles in Schwarzschild and Kerr space-times. Phys. Rev. D 2015, 91, 124030. [Google Scholar] [CrossRef] [Green Version]
- Toshmatov, B.; Malafarina, D. Spinning test particle in the γ space-times. Phys. Rev. D 2019, 100, 104052. [Google Scholar] [CrossRef] [Green Version]
- Nucamendi, U.; Becerril, R.; Sheoran, P. Bounds on spinning particles in their innermost stable circular orbits around rotating braneworld black hole. Eur. Phys. J. C 2020, 80, 35. [Google Scholar] [CrossRef]
- Zhang, Y.-P.; Gu, B.-M.; Wei, S.-W.; Yang, J.; Liu, Y.-X. Charged spinning black holes as accelerators of spinning particles. Phys. Rev. D 2016, 94, 124017. [Google Scholar] [CrossRef] [Green Version]
- Conde, C.; Galvis, C. Properties of the Innermost Stable Circular Orbit of a spinning particle moving in a rotating Maxwell-dilaton black hole background. Phys. Rev. D 2019, 99, 104059. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.-L.; Zhang, X.-D. Maximal efficiency of the collisional Penrose process with spinning particles in Kerr-Sen black hole. Eur. Phys. J. C 2020, 80, 31. [Google Scholar] [CrossRef] [Green Version]
- Hojman, S.A.; Asenjo, F.A. Can gravitation accelerate neutrinos? Class. Quantum Gravity 2013, 30, 025008. [Google Scholar] [CrossRef] [Green Version]
- Kaplan, S.A. On crcular orbits in Einstein’s Gravitation Theory. J. Exp. Theor. Phys. 1949, 19, 951. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. The Classical Theory of Fields; Pergamon: Oxford, UK, 1993. [Google Scholar]
- Zhang, Y.-P.; Wei, S.-W.; Amaro-Seoane, P.; Yang, J.; Liu, Y.-X. Motion deviation of test body induced by spin and cosmological constant in extreme mass ratio inspiral binary system. Eur. Phys. J. C 2019, 79, 856. [Google Scholar] [CrossRef]
- Misner, C.W.; Thorne, K.S.; Wheeler, J.A. Gravitation; Freeman: New York, NY, USA, 1973; p. 911. [Google Scholar]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.-P.; Wei, S.-W.; Liu, Y.-X. Spinning Test Particle in Four-Dimensional Einstein–Gauss–Bonnet Black Holes. Universe 2020, 6, 103. https://doi.org/10.3390/universe6080103
Zhang Y-P, Wei S-W, Liu Y-X. Spinning Test Particle in Four-Dimensional Einstein–Gauss–Bonnet Black Holes. Universe. 2020; 6(8):103. https://doi.org/10.3390/universe6080103
Chicago/Turabian StyleZhang, Yu-Peng, Shao-Wen Wei, and Yu-Xiao Liu. 2020. "Spinning Test Particle in Four-Dimensional Einstein–Gauss–Bonnet Black Holes" Universe 6, no. 8: 103. https://doi.org/10.3390/universe6080103
APA StyleZhang, Y.-P., Wei, S.-W., & Liu, Y.-X. (2020). Spinning Test Particle in Four-Dimensional Einstein–Gauss–Bonnet Black Holes. Universe, 6(8), 103. https://doi.org/10.3390/universe6080103





