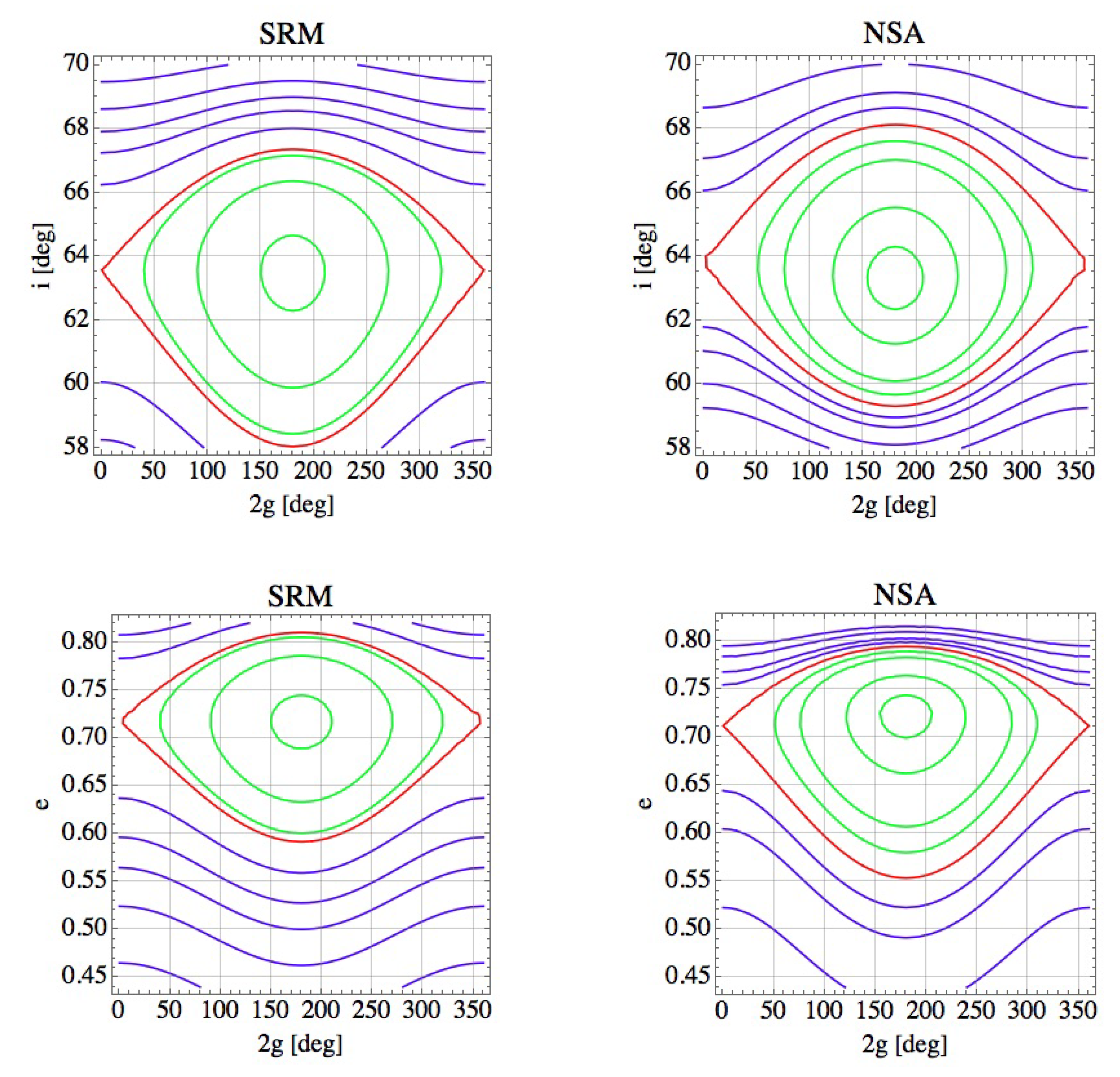
Important results will be translated in terms of Keplerian elements in order to be more understandable.
3.1. The Dominant Terms in the Long-Term Dynamics
According to the theoretical considerations exposed in
Section 2.2 and taking as a reference system (11), we evaluate the
amplitudes of the harmonic coefficients (see
Table 1 and
Table 2), their partial derivatives with respect to the actions (see
Table 3) and the
periods of the harmonic arguments (see Tables 5 and 6) at the Molniya parameters. Since the functions involved are sufficiently regular, the results provide an accurate estimate of the perturbing terms affecting a satellite in a Molniya regime.
Table 1 shows all the amplitudes of the second-order solar harmonics. The number of the third-order solar harmonics computed is 28, but the corresponding coefficients are too small to be displayed: the largest values are of the order of
km
2/s
2. In the lunar case, the number of the second-order harmonics evaluated is 38, ranging from values of approximately
km
2/s
2 to
km
2/s
2. Instead, the third-order contribution consists of 196 harmonics, ranging from approximately
km
2/s
2 to
km
2/s
2. Of all these estimates, the largest amplitudes of both the second- and the third-order lunar potential are listed in
Table 2.
We notice that the largest lunar amplitudes in
Table 2 are only one order of magnitude lower than the
contribution in Equation (7), which is of order
km
2/s
2 when evaluated at the Molniya parameter. This is due to the fact that a Molniya satellite attains high altitudes.
Moreover, from the table, it is clear that, although considering the geocentric orbits of the Moon and of the Sun with their actual eccentricity (, ), the third-order contribution in the Hamiltonian given by a third body is quite small, even if Molniya are highly eccentric and high-altitude orbits.
In
Table 3, we give the estimates of the largest values of the partial derivatives of the harmonic coefficients with respect to the actions, computed in the Molniya region. This analysis would indicate the dominant terms in the angular dynamics defined by the last two equations in Equation (11).
Table 3 on the left shows the lunisolar contribution to
, while, on the right, we report the terms determining the dynamics of
h. Only the second-order lunisolar contribution was taken into account.
The precession caused by the lunar and solar mean terms (
Table 3 on the left) is certainly larger than the secular drift of the argument of perigee due to the Earth’s oblateness, which cancels out, by definition, at the critical inclination.
Moreover, the amplitudes of the oscillations seem to be quite large. These facts imply that the third-body effects on the dynamics of the argument of perigee are small but not negligible if compared with the oblateness effect. If we change slightly the inclination—for example,
—the oblateness drift becomes only one order of magnitude larger than the ones produced by the third-body mean terms. For practical applications, because of the unavoidable errors in spacecraft maneuvering, the conclusion is that the gravitational attraction exerted by the Moon and the Sun is essential to study in depth the periods and the perigee resonance that characterize Molniya-like orbits (
Section 4.1 and
Section 4).
On the contrary, the partial derivatives of both the lunar and the solar mean terms in
Table 3 on the right ensure that the oblateness effect is still the dominant perturbation for
, as it usually happens in the case of a no frozen condition. Indeed,
Hence, to better capture how the lunisolar perturbation might affect the dynamics of the angular terms, the
periods of the arguments involved in the doubly averaged model are computed assuming
and different approximations of
taking into account also the the lunisolar terms listed in
Table 3.
To handle the periodic effects, we need to set initial conditions also for the argument of pericenter and for the longitude of the ascending node of the satellite. An initial argument of perigee at
is the best initial condition to allow the largest coverage of the Russian territory by a Molniya satellite [
3,
4]; thus, we focus only on how lunisolar effects on the angles vary with respect to the initial ascending node of the satellite. This choice is dictated by the fact highlighted, for instance, by Anselmo and Pardini in [
18]: the initial ascending node is crucial for the satellite’s lifetime. Moreover, we can always fix
. The notation adopted to represent the different approximations of
and the details are arranged in
Table 4.
In
Table 5, we list the largest second-order periods obtained, while
Table 6 shows the third-order ones. We remark that the arguments that do not depend on
are also shown for the sake of completeness. As we will see in the second part of the work, to each resonant term including
g, one can associate an integral of motion that comprises the value of the semi-major axis, eccentricity and inclination. Thus, the terms in the disturbing potential that explicitly cause the inclination to evolve implicitly change the long-term picture when combined with the eccentricity resonances.
In both tables, the arguments are grouped with respect to the associated periods to make the reading easier. In
Table 5, macro periods of the order 40.08 years, 18.61 years, 12.71 years, 9.30 years and 7.55 years are highlighted, consistent with the frequency analysis [
2]. The argument
represents the
main resonant angle, because of the critical inclination, and thus we compute a very large period, for any assumptions we take for
. Moreover, we find the well-known value 18.61 years in correspondence with the period of the lunar ascending node. Small periods are related to high frequencies that are not very sensitive to the value of
. On the contrary, the largest periods strongly depend on the approximation chosen. The same feature also emerges from
Table 6, where the groups are of four arguments in the lunar case and of two arguments in the solar case. The solar third-order critical arguments
and
(top of
Table 6) behave as the main resonant angle. Conversely, the third-order lunar arguments
and
behave in the opposite way: by increasing the lunisolar perturbation, the arguments may even become critical.
In
Table 7, all the harmonics whose
km
2/s are shown, from the highest to the lowest, taking as reference the assumption of the fourth column. At the top of the table, we find clearly the main resonant argument
, which is the lunisolar term dominating the dynamics of the eccentricity for Molniya orbits, as is well known.
Subsequently, the harmonics corresponding to
and
h show
km
2/s; thus, they produce a significant contribution to the dynamics; precisely,
affects both
e and
i, while
h only perturbs
i. These arguments are far from being critical; the periods are around 7.55 years, but their amplitudes are quite large (see
Table 1 and
Table 2).
In [
5], the role played by
was analyzed by means of a double resonance model, even though the resonance
does not produce any resonances overlapping. Moreover, in [
2], the authors proved that the terms corresponding to
,
and
h capture the main features of the long-term eccentricity variation, by comparing the pseudo-observation data given by the Two-Line Element (TLE) with ad hoc numerical propagations. Following the results in [
2], in this work, the
dominant terms are chosen as the ones associated with
km
2/s. Indeed, as long as the hypothesis of a constant semi-major axis holds true, a model including the lunar harmonics with
,
and
in Equation (2) and the solar harmonics with
,
in Equation (1) seems to be the “poorest” model capable of representing the third-body effect in the actual mean eccentricity of many Molniya spacecraft. Such terms of the quadrupolar expansion occupy the first 14 lines of
Table 7, having only 2 of them
km
2/s significantly.