Two Applications of the Analytic Conformal Bootstrap: A Quick Tour Guide
Abstract
:1. Introduction
2. Basics of Conformal Field Theory
- Translation:
- Rotation:
- Dilatation:
- Special conformal transformation (SCT):
| Transformation | Generator |
| Translation: | |
| Rotation: | |
| Dilatation: | |
| SCT: | . |
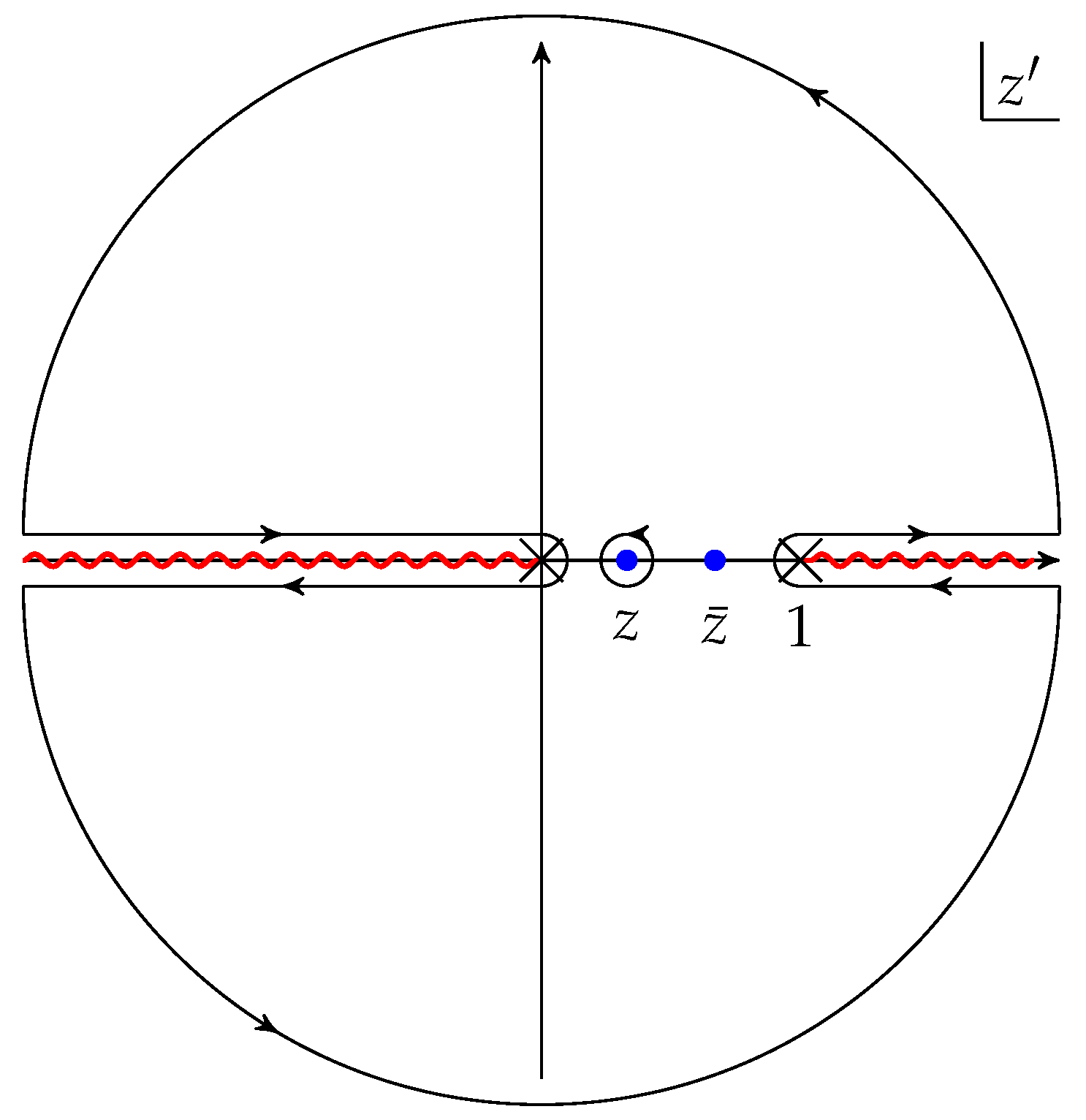
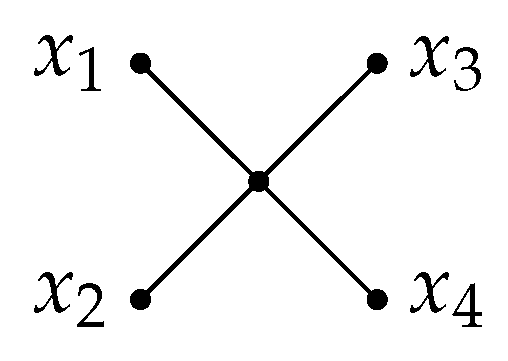
3. Dispersion Relation in CFT
3.1. Analytic Structure of Conformal Blocks
3.2. Crossing Symmetry and Dispersion Relation
3.3. Computing Wilson–Fisher Correlator Using Dispersion Relation
4. Basics of Superconformal Field Theory
- Superprimaries with which give rise to long multiplets. In general, a long multiplet contains states;
- Operators at the unitarity bound and operators with , but still allowed for specific spins and R-charges. These form short multiplets, so called because they obey some “shortening conditions” that are concretely realized in the fact that they are annihilated by a certain amount of Q’s and ’s, and hence, the multiplet can only contain a reduced number of states. These operators are often called BPS and their dimension, being determined by Lorentz and R-symmetry quantum numbers, is protected against quantum corrections5.
5. = 4 Super Yang–Mills
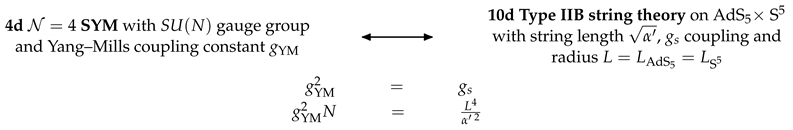
5.1. Operators and Spectrum
- Identity operator, which is a singlet of R symmetry and it has ;
- -BPS operators, scalars annihilated by half of the supercharges. They can either be single trace operators:symmetric traceless tensors transforming in the or multi-trace operators:where stands for projection into the corresponding representation. Their dimension is protected from quantum corrections and supersymmetry completely fixes their three-point functions [26,29,30,31,32,33,34].
- -BPS operators , with Dynkin labels and protected dimension and -BPS operators, multi-trace operators in the having fixed dimension . Both these types are genuinely BPS only in the free theory and mix with descendants of non-BPS operators when interactions are turned on.
- Long operators can transform into a generic R-symmetry representation, as their dimension is not protected but nonetheless subject to the unitarity bound:
5.2. Stress Tensor Multiplet Correlators

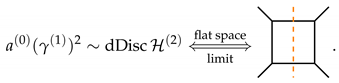

Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1. | In this note, we will mostly deal with dimensional conformal field theories. |
| 2. | In this context, the meaning of low lying refers to the dimension of the operators in the OPE. |
| 3. | Note that this does not work for correlators where there is no gap in the spectrum. |
| 4. | |
| 5. | The precise relation can be inferred by simple reasoning. Let us assume that the operator we want to consider is a superprimary, then in particular it holds . In addition, it has to be annihilated by at least one supercharge, namely . This implies:
|
| 6. | If we restrict to quantum field theories containing at most spin 1 particles. |
| 7. | Instantons corrections are believed to be UV finite as well. |
| 8. | In the literature, this expansion is also called conformal partial wave expansion or conformal partial wave amplitude. |
| 9. | With respect to these expressions, we suppressed the superscript in the definition of the blocks since it is assumed that we are working in four dimensions. |
| 10. | All operators in a superconformal multiplet must have the same anomalous dimension. |
| 11. | maps to the disconnected part of the amplitude. |
| 12. | The other multi-trace operators get corrections at order and higher. |
References
- Polyakov, A.M. Nonhamiltonian approach to conformal quantum field theory. Zh. Eksp. Teor. Fiz. 1974, 66, 23–42. [Google Scholar]
- Ferrara, S.; Grillo, A.F.; Gatto, R. Tensor representations of conformal algebra and conformally covariant operator product expansion. Ann. Phys. 1973, 76, 161–188. [Google Scholar] [CrossRef]
- Rattazzi, R.; Rychkov, V.S.; Tonni, E.; Vichi, A. Bounding scalar operator dimensions in 4D CFT. J. High Energy Phys. 2008, 12, 031. [Google Scholar] [CrossRef] [Green Version]
- Pol, D.; Rychkov, S.; Vichi, A. The Conformal Bootstrap: Theory, Numerical Techniques, and Applications. Rev. Mod. Phys. 2019, 91, 015002. [Google Scholar]
- Alday, L.F. Large Spin Perturbation Theory for Conformal Field Theories. Phys. Rev. Lett. 2017, 119, 111601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Alday, L.F.; Bissi, A.; Lukowski, T. Large spin systematics in CFT. J. High Energy Phys. 2015, 11, 101. [Google Scholar] [CrossRef] [Green Version]
- Heemskerk, I.; Penedones, J.; Polchinski, J.; Sully, J. Holography from Conformal Field Theory. J. High Energy Phys. 2009, 10, 079. [Google Scholar] [CrossRef]
- Aharony, O.; Alday, L.F.; Bissi, A.; Perlmutter, E. Loops in AdS from Conformal Field Theory. J. High Energy Phys. 2017, 2017, 36. [Google Scholar] [CrossRef]
- Caron-Huot, S. Analyticity in Spin in Conformal Theories. J. High Energy Phys. 2017, 2017, 78. [Google Scholar] [CrossRef]
- Dolan, F.A.; Osborn, H. Conformal partial waves and the operator product expansion. Nucl. Phys. B 2004, 678, 491–507. [Google Scholar] [CrossRef] [Green Version]
- Dolan, F.A.; Osborn, H. Conformal Partial Waves: Further Mathematical Results. arXiv 2011, arXiv:1108.6194. [Google Scholar]
- Bissi, A.; Dey, P.; Hansen, T. Dispersion Relation for CFT Four-Point Functions. J. High Energy Phys. 2020, 2020, 92. [Google Scholar] [CrossRef] [Green Version]
- Dolan, F.A.; Osborn, H. Conformal four point functions and the operator product expansion. Nucl. Phys. B 2001, 599, 459–496. [Google Scholar] [CrossRef] [Green Version]
- Pappadopulo, D.; Rychkov, S.; Espin, J.; Rattazzi, R. OPE Convergence in Conformal Field Theory. Phys. Rev. D 2012, 86, 105043. [Google Scholar] [CrossRef] [Green Version]
- Kravchuk, P.; Qiao, J.; Rychkov, S. Distributions in CFT. Part I. Cross-ratio space. J. High Energy Phys. 2020, 2020, 137. [Google Scholar] [CrossRef]
- Fitzpatrick, A.L.; Kaplan, J. Unitarity and the Holographic S-Matrix. J. High Energy Phys. 2012, 2012, 32. [Google Scholar] [CrossRef] [Green Version]
- Gopakumar, R.; Kaviraj, A.; Sen, K.; Sinha, A. A Mellin space approach to the conformal bootstrap. J. High Energy Phys. 2017, 2017, 27. [Google Scholar] [CrossRef] [Green Version]
- Alday, L.F.; Henriksson, J.; van Loon, M. Taming the ϵ-expansion with large spin perturbation theory. J. High Energy Phys. 2018, 2018, 131. [Google Scholar] [CrossRef] [Green Version]
- Nahm, W. Supersymmetries and their Representations. Nucl. Phys. B 1978, 135, 149. [Google Scholar] [CrossRef] [Green Version]
- Minwalla, S. Restrictions imposed by superconformal invariance on quantum field theories. Adv. Theor. Math. Phys. 1998, 2, 783–851. [Google Scholar] [CrossRef] [Green Version]
- Córdova, C.; Dumitrescu, T.T.; Intriligator, K. Multiplets of Superconformal Symmetry in Diverse Dimensions. J. High Energy Phys. 2019, 2019, 163. [Google Scholar] [CrossRef] [Green Version]
- Maldacena, J.M. The Large N limit of superconformal field theories and supergravity. Adv. Theor. Math. Phys. 2018, 2, 231–252. [Google Scholar] [CrossRef]
- Gubser, S.S.; Klebanov, I.R.; Alexander, M.P. Gauge theory correlators from noncritical string theory. Phys. Lett. B 1998, 428, 105–114. [Google Scholar] [CrossRef] [Green Version]
- Witten, E. Anti-de Sitter space and holography. Adv. Theor. Math. Phys. 1998, 2, 253–291. [Google Scholar] [CrossRef]
- Aharony, O.; Gubser, S.S.; Maldacena, J.; Ooguri, H.; Oz, Y. Large N field theories, string theory and gravity. Phys. Rep. 2000, 323, 183–386. [Google Scholar] [CrossRef] [Green Version]
- D’Hoker, E.; Freedman, D.Z. Supersymmetric gauge theories and the AdS / CFT correspondence. In Theoretical Advanced Study Institute in Elementary Particle Physics (TASI 2001): Strings, Branes and EXTRA Dimensions; World Scientific: Singapore, 2002. [Google Scholar]
- ’t Hooft, G. A Planar Diagram Theory for Strong Interactions. Nucl. Phys. B 1974, 72, 461. [Google Scholar] [CrossRef] [Green Version]
- Grimm, R.; Sohnius, M.; Wess, J. Extended Supersymmetry and Gauge Theories. Nucl. Phys. B 1978, 133, 275–284. [Google Scholar] [CrossRef]
- Belitsky, A.V.; Derkachov, S.E.; Korchemsky, G.P.; Manashov, A.N. Superconformal operators in N = 4 superYang–Mills theory. Phys. Rev. D 2004, 70, 045021. [Google Scholar] [CrossRef] [Green Version]
- Howe, P.S.; Sokatchev, E.; West, P.C. Three point functions in N = 4 Yang–Mills. Phys. Lett. B 2004, 444, 341–351. [Google Scholar] [CrossRef] [Green Version]
- Dolan, F.A.; Osborn, H. On short and semi-short representations for four-dimensional superconformal symmetry. Ann. Phys. 2003, 307, 41–89. [Google Scholar] [CrossRef] [Green Version]
- Freedman, D.Z.; Mathur, S.D.; Matusis, A.; Rastelli, L. Correlation functions in the CFT(d) / AdS(d+1) correspondence. Nucl. Phys. B 1999, 546, 96–118. [Google Scholar] [CrossRef] [Green Version]
- Lee, S.; Minwalla, S.; Rangamani, M.; Seiberg, N. Three point functions of chiral operators in D = 4, N = 4 SYM at large N. Adv. Theor. Math. Phys. 1998, 2, 697–718. [Google Scholar] [CrossRef] [Green Version]
- D’Hoker, E.; Freedman, D.Z.; Skiba, W. Field theory tests for correlators in the AdS / CFT correspondence. Phys. Rev. D 1999, 59, 045008. [Google Scholar] [CrossRef] [Green Version]
- Andrianopoli, L.; Ferrara, S. K-K excitations on AdS(5) x S**5 as N = 4 ’primary’ superfields. Phys. Lett. B 1998, 430, 248–253. [Google Scholar] [CrossRef] [Green Version]
- Intriligator, K.A. Bonus symmetries of N = 4 superYang–Mills correlation functions via AdS duality. Nucl. Phys. B 1999, 551, 575–600. [Google Scholar] [CrossRef] [Green Version]
- Intriligator, K.A.; Skiba, W. Bonus symmetry and the operator product expansion of N = 4 SuperYang–Mills. Nucl. Phys. B 1999, 559, 165–183. [Google Scholar] [CrossRef] [Green Version]
- Eden, B.; Howe, P.S.; West, P.C. Nilpotent invariants in N = 4 SYM. Phys. Lett. B 1999, 463, 19–26. [Google Scholar] [CrossRef] [Green Version]
- Petkou, A.; Skenderis, K. A Nonrenormalization theorem for conformal anomalies. Nucl. Phys. B 1999, 561, 100–116. [Google Scholar] [CrossRef] [Green Version]
- Howe, P.S.; Schubert, C.; Sokatchev, E.; West, P.C. Explicit construction of nilpotent covariants in N = 4 SYM. Nucl. Phys. B 2000, 571, 71–90. [Google Scholar] [CrossRef] [Green Version]
- Heslop, P.J.; Howe, P.S. OPEs and three-point correlators of protected operators in N = 4 SYM. Nucl. Phys. B 2002, 626, 265–286. [Google Scholar] [CrossRef] [Green Version]
- Bissi, A.; Lukowski, T. Revisiting N=4 superconformal blocks. J. High Energy Phys. 2016, 2016, 115. [Google Scholar] [CrossRef] [Green Version]
- Korchemsky, G.P.; Sokatchev, E. Four-point correlation function of stress-energy tensors in N=4 superconformal theories. J. High Energy Phys. 2015, 2015, 1–33. [Google Scholar] [CrossRef] [Green Version]
- Belitsky, A.V.; Hohenegger, S.; Korchemsky, G.P.; Sokatchev, E. N = 4 superconformal Ward identities for correlation functions. Nucl. Phys. B 2016, 904, 176–215. [Google Scholar] [CrossRef]
- Beem, C.; Rastelli, L.; van Rees, B.C. The N=4 Superconformal Bootstrap. Phys. Rev. Lett. 2013, 111, 071601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Beem, C.; Rastelli, L.; van Rees, B.C. More N=4 superconformal bootstrap. Phys. Rev. D 2017, 96, 046014. [Google Scholar] [CrossRef] [Green Version]
- Dolan, F.A.; Osborn, H. Superconformal symmetry, correlation functions and the operator product expansion. Nucl. Phys. B 2002, 629, 3–73. [Google Scholar] [CrossRef] [Green Version]
- Nirschl, M.; Osborn, H. Superconformal Ward identities and their solution. Nucl. Phys. B 2005, 711, 409–479. [Google Scholar] [CrossRef] [Green Version]
- Dolan, F.A.; Osborn, H. Conformal partial wave expansions for N = 4 chiral four point functions. Ann. Phys. 2006, 321, 581–626. [Google Scholar] [CrossRef] [Green Version]
- Beem, C.; Lemos, M.; Liendo, P.; Peelaers, W.; Rastelli, L.; Van Rees, B.C. Infinite Chiral Symmetry in Four Dimensions. Commun. Math. Phys. 2015, 336, 1359–1433. [Google Scholar] [CrossRef] [Green Version]
- Alday, L.F.; Bissi, A. The superconformal bootstrap for structure constants. J. High Energy Phys. 2014, 2014, 144. [Google Scholar] [CrossRef] [Green Version]
- Alday, L.F.; Bissi, A. Generalized bootstrap equations for N=4 SCFT. J. High Energy Phys. 2015, 2015, 101. [Google Scholar]
- Bissi, A.; Manenti, A.; Alessandro, V. Bootstrapping mixed correlators in N = 4 super Yang–Mills. J. High Energy Phys. 2021, 2021, 111. [Google Scholar] [CrossRef]
- Aprile, F.; Drummond, J.M.; Heslop, P.; Paul, H. Unmixing Supergravity. J. High Energy Phys. 2018, 2018, 133. [Google Scholar] [CrossRef] [Green Version]
- Aprile, F.; Drummond, J.M.; Heslop, P.; Paul, H. Quantum Gravity from Conformal Field Theory. J. High Energy Phys. 2018, 2018, 35. [Google Scholar] [CrossRef] [Green Version]
- Alday, L.F.; Bissi, A. Loop Corrections to Supergravity on AdS5×S5. Phys. Rev. Lett. 2017, 119, 171601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Alday, L.F.; Caron-Huot, S. Gravitational S-matrix from CFT dispersion relations. J. High Energy Phys. 2018, 2018, 17. [Google Scholar] [CrossRef] [Green Version]
- Aprile, F.; Drummond, J.; Heslop, P.; Paul, H. Double-trace spectrum of N=4 supersymmetric Yang–Mills theory at strong coupling. Phys. Rev. D 2018, 98, 126008. [Google Scholar] [CrossRef] [Green Version]
- Alday, L.F.; Bissi, A.; Perlmutter, E. Genus-One String Amplitudes from Conformal Field Theory. J. High Energy Phys. 2019, 2019, 10. [Google Scholar] [CrossRef] [Green Version]
- Alday, L.F. On Genus-one String Amplitudes on AdS5×S5. arXiv 2018, arXiv:1812.11783. [Google Scholar]
- Rastelli, L.; Zhou, X. Mellin amplitudes for AdS5×S5. Phys. Rev. Lett. 2017, 118, 091602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rastelli, L.; Zhou, X. How to Succeed at Holographic Correlators Without Really Trying. J. High Energy Phys. 2018, 2018, 14. [Google Scholar] [CrossRef] [Green Version]
- Alday, L.F.; Zhou, X. Simplicity of AdS Supergravity at One Loop. J. High Energy Phys. 2020, 2020, 8. [Google Scholar] [CrossRef]
- Caron-Huot, S.; Trinh, A.-K. All tree-level correlators in AdS5×S5 supergravity: Hidden ten-dimensional conformal symmetry. J. High Energy Phys. 2019, 2019, 196. [Google Scholar] [CrossRef] [Green Version]
- Bissi, A.; Fardelli, G.; Georgoudis, A. Towards All Loop Supergravity Amplitudes on AdS5×S5. arXiv 2020, arXiv:2002.04604. [Google Scholar]
- Bissi, A.; Fardelli, G.; Georgoudis, A. All loop structures in Supergravity Amplitudes on AdS5×S5 from CFT. arXiv 2020, arXiv:2010.12557. [Google Scholar]
- Simmons-Duffin, D.; Stanford, D.; Witten, E. A spacetime derivation of the Lorentzian OPE inversion formula. J. High Energy Phys. 2018, 2018, 85. [Google Scholar] [CrossRef] [Green Version]
- Kravchuk, P.; Simmons-Duffin, D. Light-ray operators in conformal field theory. J. High Energy Phys. 2018, 2018, 102. [Google Scholar] [CrossRef] [Green Version]
- Meltzer, D.; Perlmutter, E.; Sivaramakrishnan, A. Unitarity Methods in AdS/CFT. J. High Energy Phys. 2020, 2020, 61. [Google Scholar] [CrossRef] [Green Version]
- D’Hoker, E.; Freedman, D.Z.; Mathur, S.D.; Matusis, A.; Rastelli, L. Graviton exchange and complete four point functions in the AdS / CFT correspondence. Nucl. Phys. B 1999, 562, 353–394. [Google Scholar] [CrossRef] [Green Version]
- Okuda, T.; Penedones, J. String scattering in flat space and a scaling limit of Yang–Mills correlators. Phys. Rev. D 2011, 83, 086001. [Google Scholar] [CrossRef] [Green Version]
- Maldacena, J.; Simmons-Duffin, D.; Zhiboedov, A. Looking for a bulk point. J. High Energy Phys. 2017, 2017, 13. [Google Scholar] [CrossRef] [Green Version]
- Gary, M.; Giddings, S.B.; Penedones, J. Local bulk S-matrix elements and CFT singularities. Phys. Rev. D 2009, 80, 085005. [Google Scholar] [CrossRef] [Green Version]
- Susskind, L. Holography in the flat space limit. AIP Conf. Proc. 1999, 493, 98–112. [Google Scholar]
- Polchinski, J. S matrices from AdS space-time. arXiv 1999, arXiv:hep-th/9901076. [Google Scholar]
- Cutkosky, R.E. Singularities and discontinuities of Feynman amplitudes. J. Math. Phys. 1960, 1, 429–433. [Google Scholar] [CrossRef]
- Meltzer, D.; Sivaramakrishnan, A. CFT Unitarity and the AdS Cutkosky Rules. J. High Energy Phys. 2020, 2020, 73. [Google Scholar] [CrossRef]
- Liam, F.A.; Kaplan, J.; Joao, P.; Suvrat, R.; van Rees Balt, C. A Natural Language for AdS/CFT Correlators. J. High Energy Phys. 2011, 2011, 95. [Google Scholar]
- Arutyunov, G.; Dolan, F.A.; Osborn, H.; Sokatchev, E. Correlation functions and massive Kaluza-Klein modes in the AdS / CFT correspondence. Nucl. Phys. B 2003, 665, 273–324. [Google Scholar] [CrossRef] [Green Version]
- Arutyunov, G.; Sokatchev, E. On a large N degeneracy in N = 4 SYM and the AdS / CFT correspondence. Nucl. Phys. B 2003, 663, 163–196. [Google Scholar] [CrossRef] [Green Version]
- Berdichevsky, L.; Naaijkens, P. Four-point functions of different-weight operators in the AdS/CFT correspondence. J. High Energy Phys. 2008, 2008, 71. [Google Scholar] [CrossRef] [Green Version]
- Uruchurtu, L.I. Next-next-to-extremal Four Point Functions of N = 4 1/2 BPS Operators in the AdS/CFT Correspondence. J. High Energy Phys. 2011, 2011, 133. [Google Scholar] [CrossRef] [Green Version]
- Drummond, J.M.; Paul, H. One-loop string corrections to AdS amplitudes from CFT. arXiv 2019, arXiv:1912.07632. [Google Scholar]
- Drummond, J.M.; Paul, H.; Santagata, M. Bootstrapping string theory on AdS5×S5. arXiv 2020, arXiv:2004.07282. [Google Scholar]
- Aprile, F.; Vieira, P. Large p explorations. From SUGRA to big STRINGS in Mellin space. J. High Energy Phys. 2020, 2020, 206. [Google Scholar] [CrossRef]



| Input | Output (Correlator) | OPE Data |
|---|---|---|
| Identity: | ||
| ⋯ | ⋯ | ⋯ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bissi, A.; Dey, P.; Fardelli, G. Two Applications of the Analytic Conformal Bootstrap: A Quick Tour Guide. Universe 2021, 7, 247. https://doi.org/10.3390/universe7070247
Bissi A, Dey P, Fardelli G. Two Applications of the Analytic Conformal Bootstrap: A Quick Tour Guide. Universe. 2021; 7(7):247. https://doi.org/10.3390/universe7070247
Chicago/Turabian StyleBissi, Agnese, Parijat Dey, and Giulia Fardelli. 2021. "Two Applications of the Analytic Conformal Bootstrap: A Quick Tour Guide" Universe 7, no. 7: 247. https://doi.org/10.3390/universe7070247
APA StyleBissi, A., Dey, P., & Fardelli, G. (2021). Two Applications of the Analytic Conformal Bootstrap: A Quick Tour Guide. Universe, 7(7), 247. https://doi.org/10.3390/universe7070247






