Chiral Magnetic Josephson Junction as a Base for Low-Noise Superconducting Qubits
Abstract
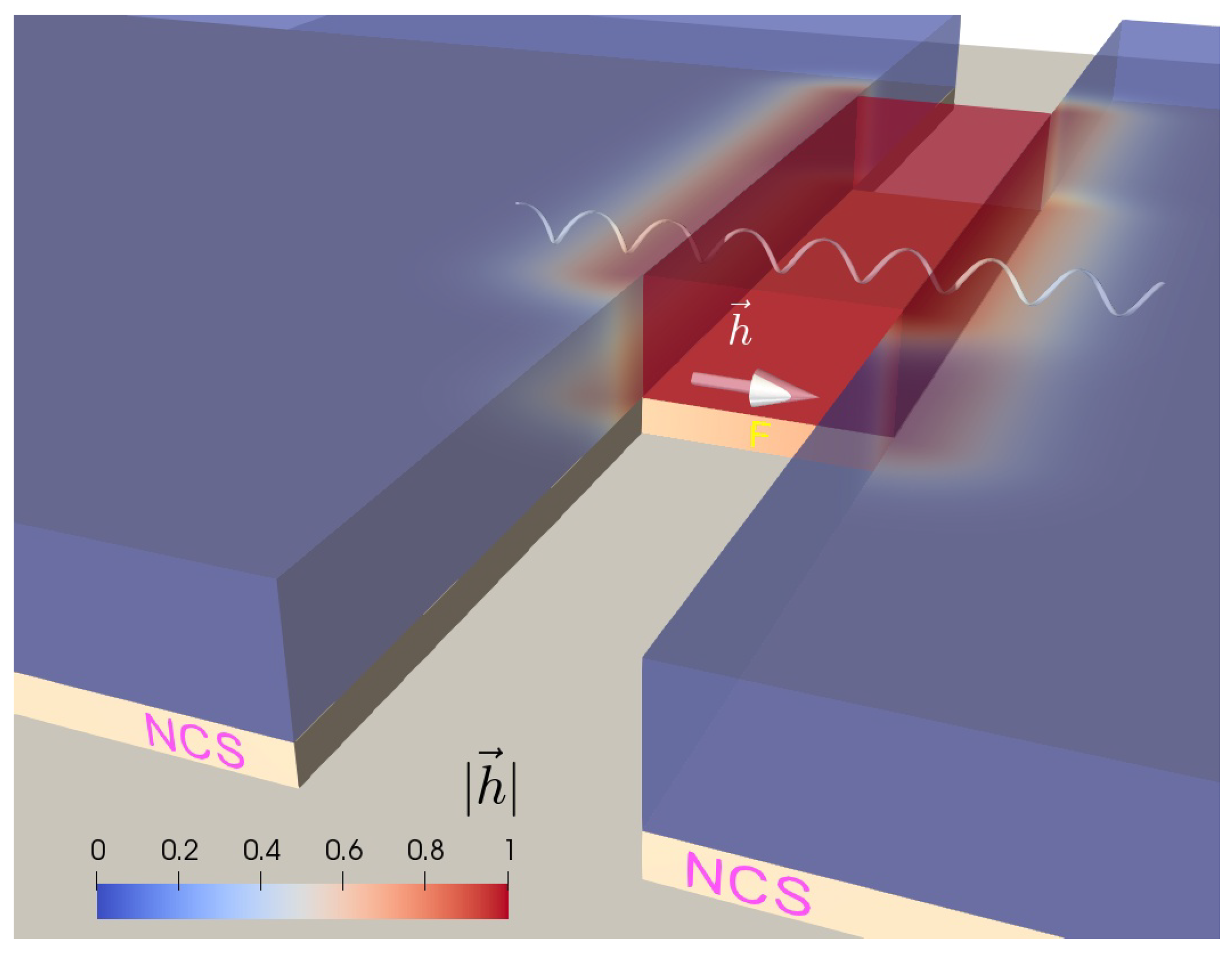
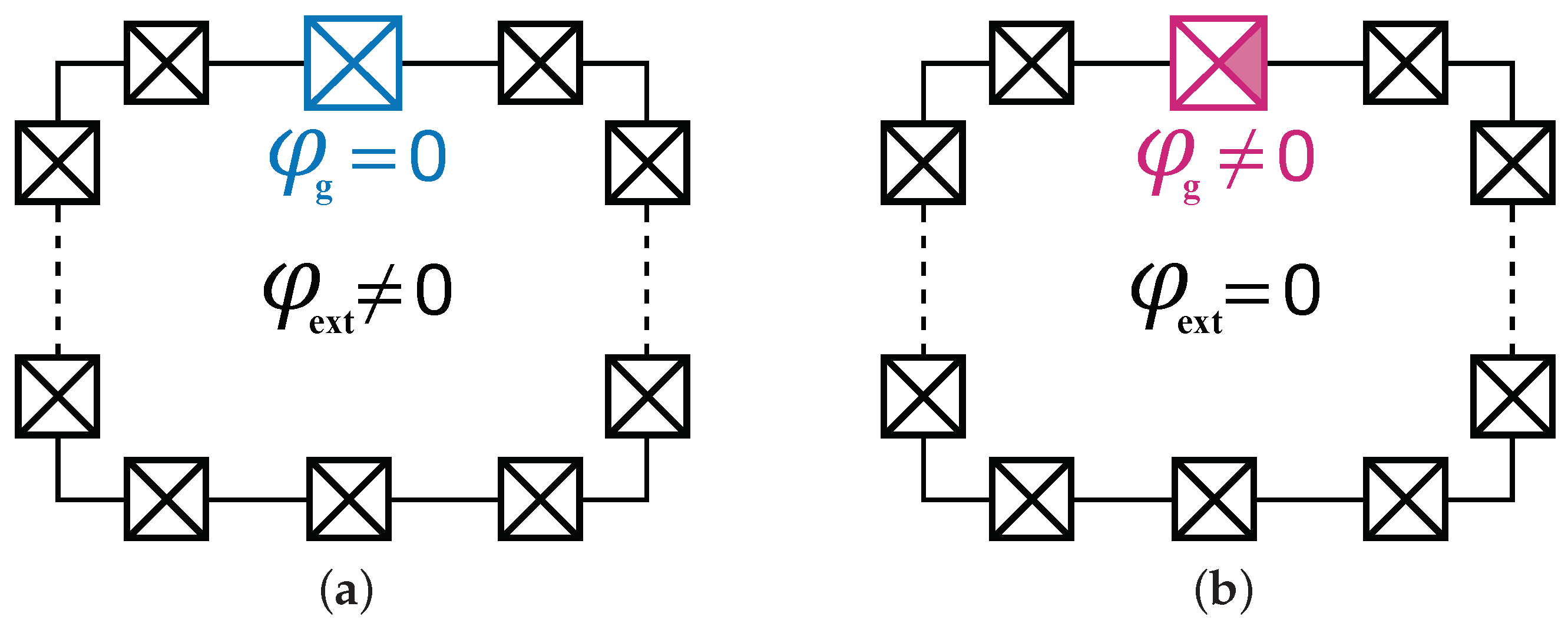
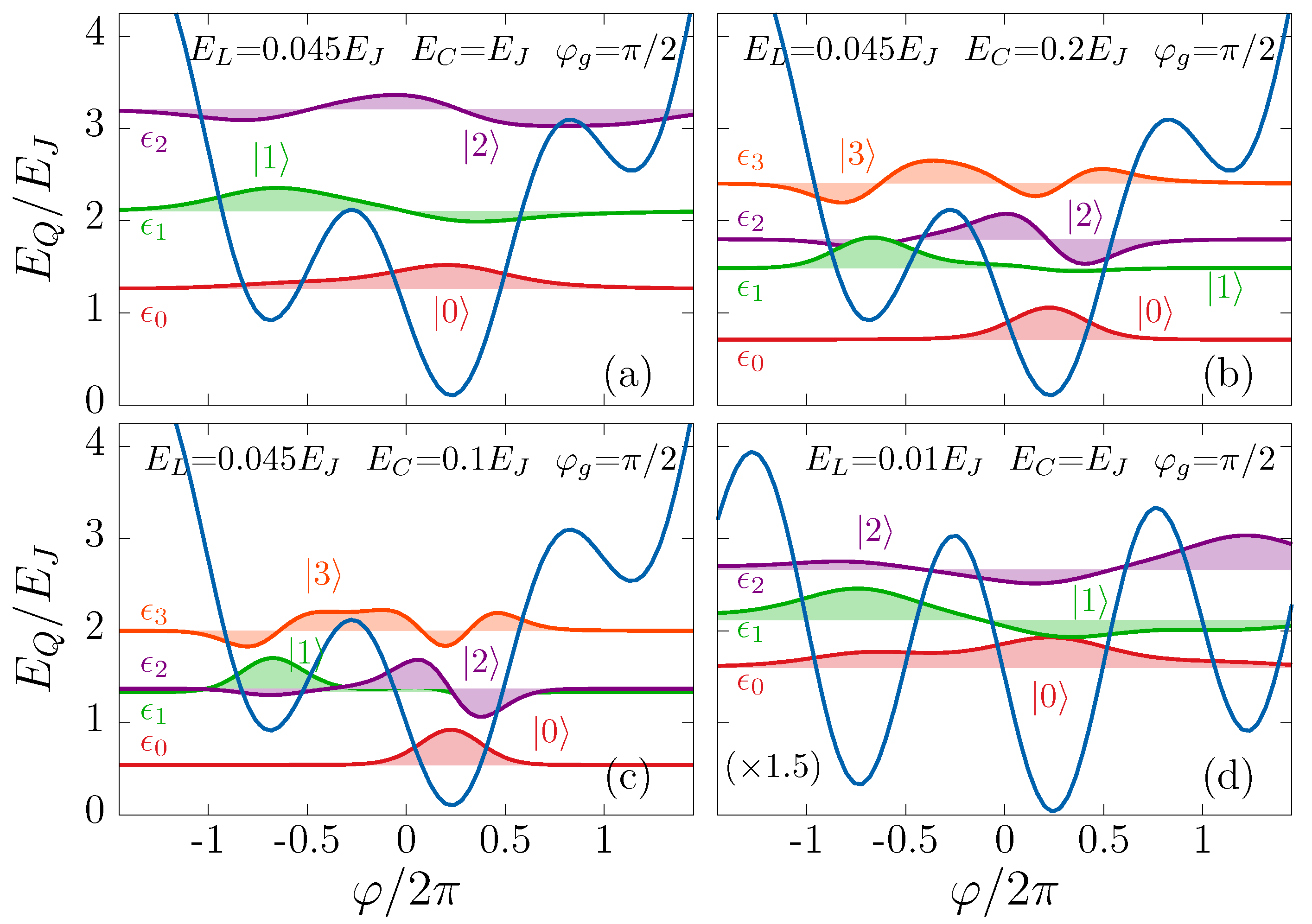
:1. Introduction
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| CMJ | Chiral Magnetic Josephson |
| CME | Chiral Magnetic Effect |
| 1 | Possible candidates for the NCS superconductors should thus have a crystalline structure with either the point group O (Li2Pt3B, Mo3Al2C), T point group (e.g. LaRhSi, LaIrSi), or (La5B2C6), (UIr), etc. On the other hand, the point groups with n = 2, 3, 4, 6 (possessed, for example, by the compounds MoS2, MoN, GaN, CePt3Si, CeRhSi3, amd CeIrSi3 [7]) correspond to the Lifshitz invariants of the type that do not fit our proposal. |
References
- Bulaevskii, L.N.; Guseinov, A.A.; Rusinov, A.I. Superconductivity in crystals without symmetry centers. Sov. J. Exp. Theor. Phys. 1976, 44, 1243. [Google Scholar]
- Levitov, L.S.; Nazarov, Y.V.; Éliashberg, G.M. Magnetostatics of superconductors without an inversion center. Sov. J. Exp. Theor. Phys. Lett. 1985, 41, 445. [Google Scholar]
- Bauer, E.; Hilscher, G.; Michor, H.; Paul, C.; Scheidt, E.W.; Gribanov, A.; Seropegin, Y.; Noël, H.; Sigrist, M.; Rogl, P. Heavy Fermion Superconductivity and Magnetic Order in Noncentrosymmetric CePt3Si. Phys. Rev. Lett. 2004, 92, 027003. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Samokhin, K.V.; Zijlstra, E.S.; Bose, S.K. CePt3Si: An unconventional superconductor without inversion center. Phys. Rev. B 2004, 69, 094514. [Google Scholar] [CrossRef] [Green Version]
- Yuan, H.Q.; Agterberg, D.F.; Hayashi, N.; Badica, P.; Vandervelde, D.; Togano, K.; Sigrist, M.; Salamon, M.B. S-Wave Spin-Triplet Order in Superconductors without Inversion Symmetry: Li2Pd3B and Li2Pt3B. Phys. Rev. Lett. 2006, 97, 017006. [Google Scholar] [CrossRef] [Green Version]
- Bauer, E.; Sigrist, M. (Eds.) Non-Centrosymmetric Superconductors: Introduction and Overview; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef] [Green Version]
- Smidman, M.; Salamon, M.B.; Yuan, H.Q.; Agterberg, D.F. Superconductivity and spin-orbit coupling in non-centrosymmetric materials: A review. Rep. Progr. Phys. 2017, 80, 036501. [Google Scholar] [CrossRef] [Green Version]
- Garaud, J.; Chernodub, M.N.; Kharzeev, D.E. Vortices with magnetic field inversion in noncentrosymmetric superconductors. Phys. Rev. B 2020, 102, 184516. [Google Scholar] [CrossRef]
- Samoilenka, A.; Babaev, E. Spiral magnetic field and bound states of vortices in noncentrosymmetric superconductors. Phys. Rev. B 2020, 102, 184517. [Google Scholar] [CrossRef]
- Garaud, J.; Korneev, A.; Samoilenka, A.; Molochkov, A.; Babaev, E.; Chernodub, M. Toroflux: A counterpart of the Chandrasekhar-Kendall state in noncentrosymmetric superconductors. arXiv 2022, arXiv:2208.08180. [Google Scholar]
- Buzdin, A. Direct Coupling Between Magnetism and Superconducting Current in the Josephson φ0 Junction. Phys. Rev. Lett. 2008, 101, 107005. [Google Scholar] [CrossRef] [Green Version]
- Konschelle, F.; Buzdin, A. Magnetic Moment Manipulation by a Josephson Current. Phys. Rev. Lett. 2009, 102, 017001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Atanasova, P.K.; Panayotova, S.A.; Rahmonov, I.R.; Shukrinov, Y.M.; Zemlyanaya, E.V.; Bashashin, M.V. Periodicity of magnetization reversals in φ0 Josephson junction. arXiv 2019, arXiv:1905.03895. [Google Scholar]
- Shukrinov, Y.M.; Mazanik, A.; Rahmonov, I.R.; Botha, A.E.; Buzdin, A. Re-orientation of the easy axis in φ0-junction. EPL (Europhys. Lett.) 2018, 122, 37001. [Google Scholar] [CrossRef]
- Liu, J.F.; Chan, K.S. Anomalous Josephson current through a ferromagnetic trilayer junction. Phys. Rev. B 2010, 82, 184533. [Google Scholar] [CrossRef] [Green Version]
- Tanaka, Y.; Yokoyama, T.; Nagaosa, N. Manipulation of the Majorana Fermion, Andreev Reflection, and Josephson Current on Topological Insulators. Phys. Rev. Lett. 2009, 103, 107002. [Google Scholar] [CrossRef] [Green Version]
- Dolcini, F.; Houzet, M.; Meyer, J.S. Topological Josephson ϕ0 junctions. Phys. Rev. B 2015, 92, 035428. [Google Scholar] [CrossRef] [Green Version]
- Yokoyama, T.; Eto, M.; Nazarov, Y.V. Anomalous Josephson effect induced by spin-orbit interaction and Zeeman effect in semiconductor nanowires. Phys. Rev. B 2014, 89, 195407. [Google Scholar] [CrossRef] [Green Version]
- Campagnano, G.; Lucignano, P.; Giuliano, D.; Tagliacozzo, A. Spin–orbit coupling and anomalous Josephson effect in nanowires. J. Phys. Condens. Matter 2015, 27, 205301. [Google Scholar] [CrossRef] [Green Version]
- Reynoso, A.A.; Usaj, G.; Balseiro, C.A.; Feinberg, D.; Avignon, M. Anomalous Josephson Current in Junctions with Spin Polarizing Quantum Point Contacts. Phys. Rev. Lett. 2008, 101, 107001. [Google Scholar] [CrossRef] [Green Version]
- Zazunov, A.; Egger, R.; Jonckheere, T.; Martin, T. Anomalous Josephson Current through a Spin-Orbit Coupled Quantum Dot. Phys. Rev. Lett. 2009, 103, 147004. [Google Scholar] [CrossRef] [Green Version]
- Dell’Anna, L.; Zazunov, A.; Egger, R.; Martin, T. Josephson current through a quantum dot with spin-orbit coupling. Phys. Rev. B 2007, 75, 085305. [Google Scholar] [CrossRef] [Green Version]
- Brunetti, A.; Zazunov, A.; Kundu, A.; Egger, R. Anomalous Josephson current, incipient time-reversal symmetry breaking, and Majorana bound states in interacting multilevel dots. Phys. Rev. B 2013, 88, 144515. [Google Scholar] [CrossRef] [Green Version]
- Alidoust, M.; Halterman, K. Evolution of pair correlation symmetries and supercurrent reversal in tilted Weyl semimetals. Phys. Rev. B 2020, 101, 035120. [Google Scholar] [CrossRef]
- Alidoust, M. Self-biased current, magnetic interference response, and superconducting vortices in tilted Weyl semimetals with disorder. Phys. Rev. B 2018, 98, 245418. [Google Scholar] [CrossRef] [Green Version]
- Szombati, D.B.; Nadj-Perge, S.; Car, D.; Plissard, S.R.; Bakkers, E.P.A.M.; Kouwenhoven, L.P. Josephson Φ0-junction in nanowire quantum dots. Nat. Phys. 2016, 12, 568. [Google Scholar] [CrossRef] [Green Version]
- Zhang, H.; Wang, J.; Liu, J.F. Anomalous Josephson effect in noncentrosymmetric superconductors. Appl. Phys. Lett. 2016, 108, 102601. [Google Scholar] [CrossRef]
- Rahnavard, Y.; Manske, D.; Annunziata, G. Magnetic Josephson junctions with noncentrosymmetric superconductors. Phys. Rev. B 2014, 89, 214501. [Google Scholar] [CrossRef]
- Fukushima, K.; Kharzeev, D.E.; Warringa, H.J. Chiral magnetic effect. Phys. Rev. D 2008, 78, 074033. [Google Scholar] [CrossRef]
- Kharzeev, D.E.; Warringa, H.J. Chiral magnetic conductivity. Phys. Rev. D 2009, 80, 034028. [Google Scholar] [CrossRef] [Green Version]
- Li, Q.; Kharzeev, D.E.; Zhang, C.; Huang, Y.; Pletikosić, I.; Fedorov, A.; Zhong, R.; Schneeloch, J.; Gu, G.; Valla, T. Chiral magnetic effect in ZrTe5. Nat. Phys. 2016, 12, 550. [Google Scholar] [CrossRef] [Green Version]
- Xiong, J.; Kushwaha, S.K.; Liang, T.; Krizan, J.W.; Hirschberger, M.; Wang, W.; Cava, R.J.; Ong, N.P. Evidence for the chiral anomaly in the Dirac semimetal Na3Bi. Science 2015, 350, 413–416. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huang, X.; Zhao, L.; Long, Y.; Wang, P.; Chen, D.; Yang, Z.; Liang, H.; Xue, M.; Weng, H.; Fang, Z.; et al. Observation of the Chiral-Anomaly-Induced Negative Magnetoresistance in 3D Weyl Semimetal TaAs. Phys. Rev. X 2015, 5, 031023. [Google Scholar] [CrossRef] [Green Version]
- Girvin, S.M. Chapter 3—Circuit QED: Superconducting qubits coupled to microwave photons. In Quantum Machines: Measurement and Control of Engineered Quantum Systems: Lecture Notes of the Les Houches Summer School: Volume 96, July 2011; Oxford Scholarship Online: Les Houches, France, 2014; Volume 96, pp. 113–256. [Google Scholar] [CrossRef]
- Clerk, A.A.; Devoret, M.H.; Girvin, S.M.; Marquardt, F.; Schoelkopf, R.J. Introduction to quantum noise, measurement, and amplification. Rev. Mod. Phys. 2010, 82, 1155–1208. [Google Scholar] [CrossRef]
- Devoret, M.H.; Wallraff, A.; Martinis, J.M. Superconducting Qubits: A Short Review. arXiv 2004, arXiv:0411174. [Google Scholar]
- Gor’kov, L.P.; Rashba, E.I. Superconducting 2D System with Lifted Spin Degeneracy: Mixed Singlet-Triplet State. Phys. Rev. Lett. 2001, 87, 037004. [Google Scholar] [CrossRef] [Green Version]
- Agterberg, D.F. Magnetoelectric Effects, Helical Phases, and FFLO Phases. In Non-Centrosymmetric Superconductors: Introduction and Overview; Bauer, E., Sigrist, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 155–170. [Google Scholar] [CrossRef]
- Badica, P.; Kondo, T.; Togano, K. Superconductivity in a New Pseudo-Binary Li2B(Pd1−xPtx)3 (x=0–1) Boride System. J. Phys. Soc. Jpn. 2005, 74, 1014–1019. [Google Scholar] [CrossRef]
- Karki, A.B.; Xiong, Y.M.; Vekhter, I.; Browne, D.; Adams, P.W.; Young, D.P.; Thomas, K.R.; Chan, J.Y.; Kim, H.; Prozorov, R. Structure and physical properties of the noncentrosymmetric superconductor Mo3Al2C. Phys. Rev. B 2010, 82, 064512. [Google Scholar] [CrossRef] [Green Version]
- Bauer, E.; Rogl, G.; Chen, X.Q.; Khan, R.T.; Michor, H.; Hilscher, G.; Royanian, E.; Kumagai, K.; Li, D.Z.; Li, Y.Y.; et al. Unconventional superconducting phase in the weakly correlated noncentrosymmetric Mo3Al2C compound. Phys. Rev. B 2010, 82, 064511. [Google Scholar] [CrossRef] [Green Version]
- Golubov, A.A.; Kupriyanov, M.Y.; Il’ichev, E. The current-phase relation in Josephson junctions. Rev. Mod. Phys. 2004, 76, 411–469. [Google Scholar] [CrossRef] [Green Version]
- Asano, Y.; Yamano, S. Josephson effect in noncentrosymmetric superconductor junctions. Phys. Rev. B 2011, 84, 064526. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.B.; Erdmenger, J.; Trauzettel, B. Chirality Josephson Current Due to a Novel Quantum Anomaly in Inversion-Asymmetric Weyl Semimetals. Phys. Rev. Lett. 2018, 121, 226604. [Google Scholar] [CrossRef] [Green Version]
- Shapiro, S. Josephson Currents in Superconducting Tunneling: The Effect of Microwaves and Other Observations. Phys. Rev. Lett. 1963, 11, 80–82. [Google Scholar] [CrossRef]
- Nogueira, F.S.; Nussinov, Z.; van den Brink, J. Josephson Currents Induced by the Witten Effect. Phys. Rev. Lett. 2016, 117, 167002. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ryazanov, V.V.; Oboznov, V.A.; Rusanov, A.Y.; Veretennikov, A.V.; Golubov, A.A.; Aarts, J. Coupling of Two Superconductors through a Ferromagnet: Evidence for a π Junction. Phys. Rev. Lett. 2001, 86, 2427–2430. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Buzdin, A.I. Proximity effects in superconductor-ferromagnet heterostructures. Rev. Mod. Phys. 2005, 77, 935–976. [Google Scholar] [CrossRef] [Green Version]
- Lu, C.K.; Yip, S. Transverse Magnetic Field Distribution in the Vortex State of Noncentrosymmetric Superconductor with O Symmetry. J. Low Temp. Phys. 2009, 155, 160–168. [Google Scholar] [CrossRef] [Green Version]
- Kashyap, M.K.; Agterberg, D.F. Vortices in cubic noncentrosymmetric superconductors. Phys. Rev. B 2013, 88, 104515. [Google Scholar] [CrossRef]
- Hayashi, N.; Iniotakis, C.; Machida, M.; Sigrist, M. Josephson effect between conventional and non-centrosymmetric superconductors. J. Phys. Chem. Solids 2008, 69, 3225–3227. [Google Scholar] [CrossRef]
- Manucharyan, V.E.; Koch, J.; Glazman, L.I.; Devoret, M.H. Fluxonium: Single Cooper-Pair Circuit Free of Charge Offsets. Science 2009, 326, 113–116. [Google Scholar] [CrossRef] [Green Version]
- Pop, I.M.; Geerlings, K.; Catelani, G.; Schoelkopf, R.J.; Glazman, L.I.; Devoret, M.H. Coherent suppression of electromagnetic dissipation due to superconducting quasiparticles. Nature 2014, 508, 369. [Google Scholar] [CrossRef]
- Wendin, G. Quantum information processing with superconducting circuits: A review. Rep. Progr. Phys. 2017, 80, 106001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lin, Y.H.; Nguyen, L.B.; Grabon, N.; San Miguel, J.; Pankratova, N.; Manucharyan, V.E. Demonstration of Protection of a Superconducting Qubit from Energy Decay. Phys. Rev. Lett. 2018, 120, 150503. [Google Scholar] [CrossRef] [PubMed]
- Koch, J.; Yu, T.M.; Gambetta, J.; Houck, A.A.; Schuster, D.I.; Majer, J.; Blais, A.; Devoret, M.H.; Girvin, S.M.; Schoelkopf, R.J. Charge-insensitive qubit design derived from the Cooper pair box. Phys. Rev. A 2007, 76, 042319. [Google Scholar] [CrossRef] [Green Version]
- Earnest, N.; Chakram, S.; Lu, Y.; Irons, N.; Naik, R.K.; Leung, N.; Ocola, L.; Czaplewski, D.A.; Baker, B.; Lawrence, J.; et al. Realization of a Λ System with Metastable States of a Capacitively Shunted Fluxonium. Phys. Rev. Lett. 2018, 120, 150504. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Garanin, D.A.; Ishchenko, V.V.; Panina, L.V. Dynamics of an ensemble of single-domain magnetic particles. Theor. Math. Phys. 1990, 82, 169–179. [Google Scholar] [CrossRef]
- Chubykalo-Fesenko, O.; Nowak, U.; Chantrell, R.W.; Garanin, D. Dynamic approach for micromagnetics close to the Curie temperature. Phys. Rev. B 2006, 74, 094436. [Google Scholar] [CrossRef] [Green Version]
- Kaczér, J. On the domain structure of uniaxial ferromagnets. Sov. J. Exp. Theor. Phys. 1964, 19, 1204. [Google Scholar] [CrossRef]
- Bulaevskii, L.N.; Chudnovsky, E.M. Ferromagnetic film on a superconducting substrate. Phys. Rev. B 2000, 63, 012502. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chernodub, M.N.; Garaud, J.; Kharzeev, D.E. Chiral Magnetic Josephson Junction as a Base for Low-Noise Superconducting Qubits. Universe 2022, 8, 657. https://doi.org/10.3390/universe8120657
Chernodub MN, Garaud J, Kharzeev DE. Chiral Magnetic Josephson Junction as a Base for Low-Noise Superconducting Qubits. Universe. 2022; 8(12):657. https://doi.org/10.3390/universe8120657
Chicago/Turabian StyleChernodub, Maxim N., Julien Garaud, and Dmitri E. Kharzeev. 2022. "Chiral Magnetic Josephson Junction as a Base for Low-Noise Superconducting Qubits" Universe 8, no. 12: 657. https://doi.org/10.3390/universe8120657
APA StyleChernodub, M. N., Garaud, J., & Kharzeev, D. E. (2022). Chiral Magnetic Josephson Junction as a Base for Low-Noise Superconducting Qubits. Universe, 8(12), 657. https://doi.org/10.3390/universe8120657





