4D Einstein–Gauss–Bonnet Gravity Coupled to Modified Logarithmic Nonlinear Electrodynamics
Abstract
:1. Introduction
2. 4D EGB Model
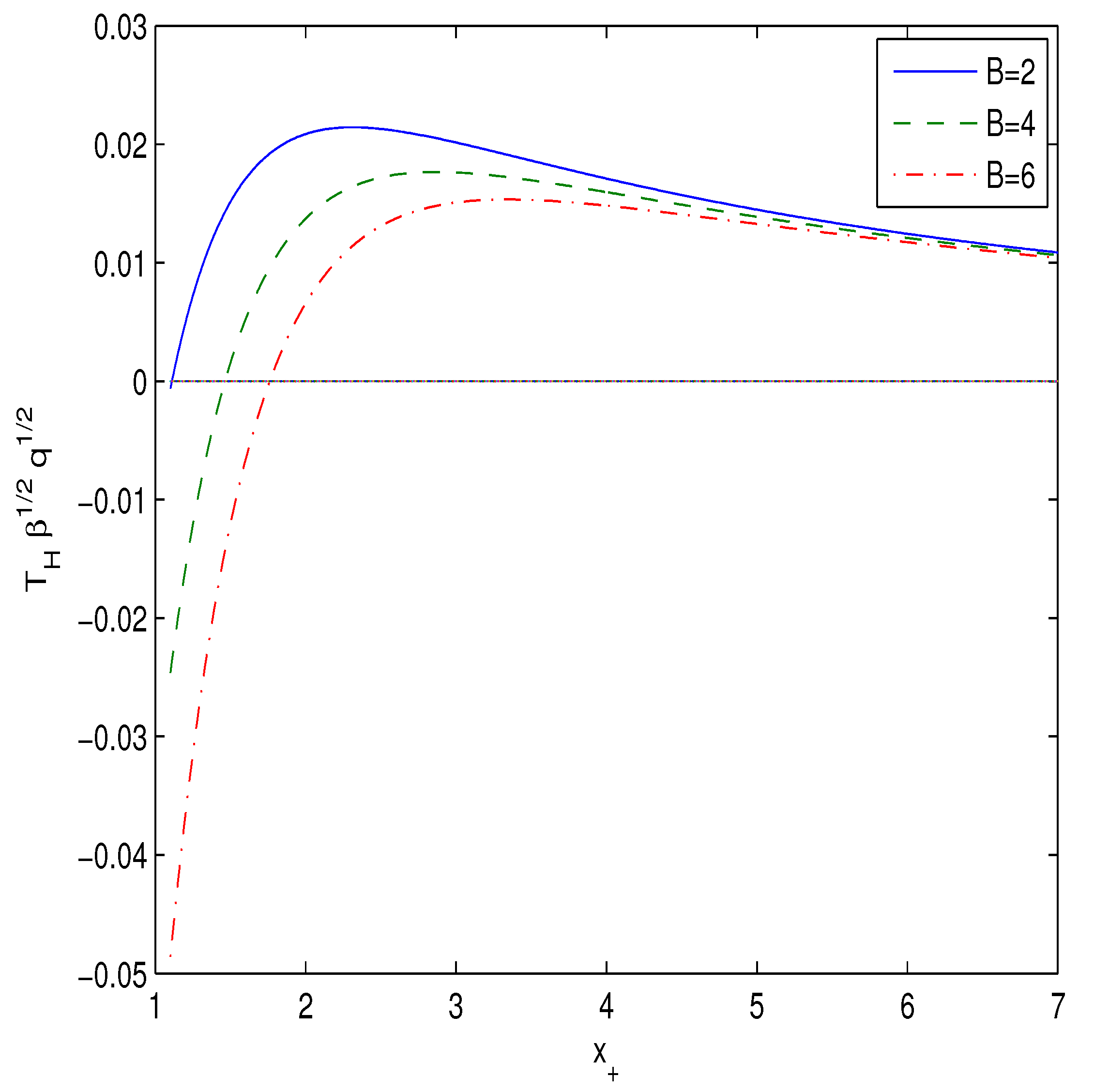
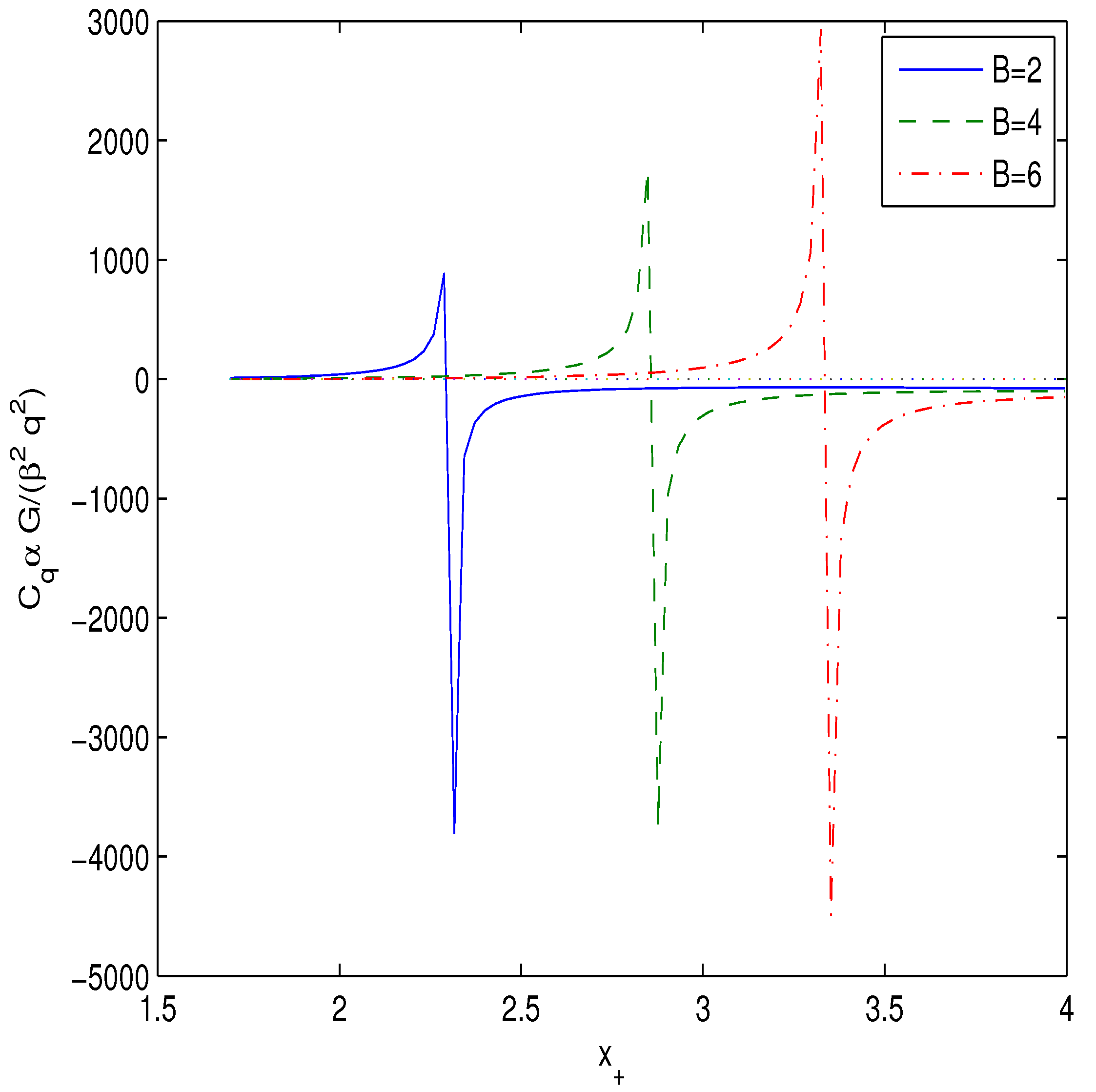
3. The Black Hole Thermodynamics
4. Black Holes Shadows
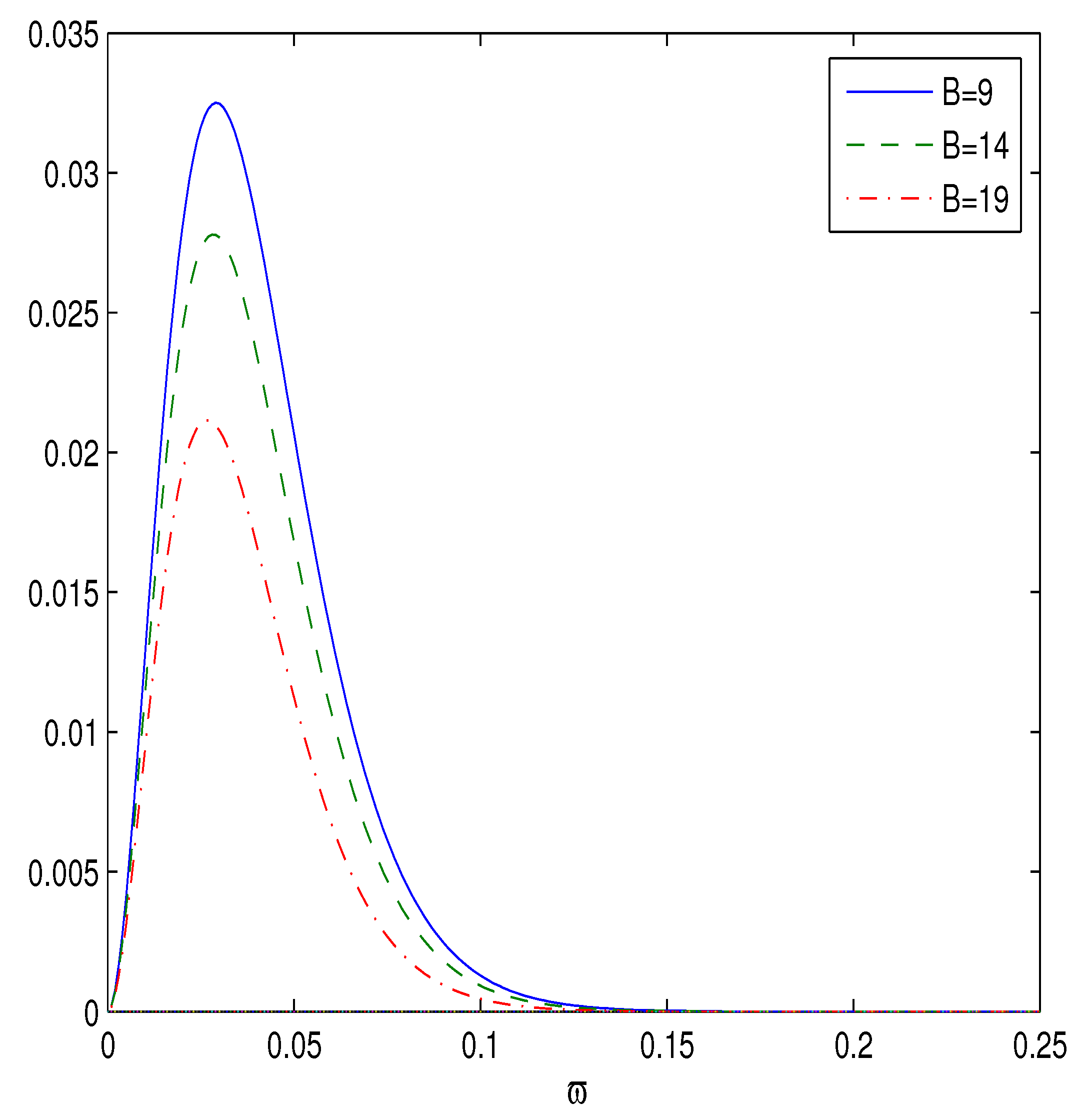
5. Black Holes Energy Emission Rate
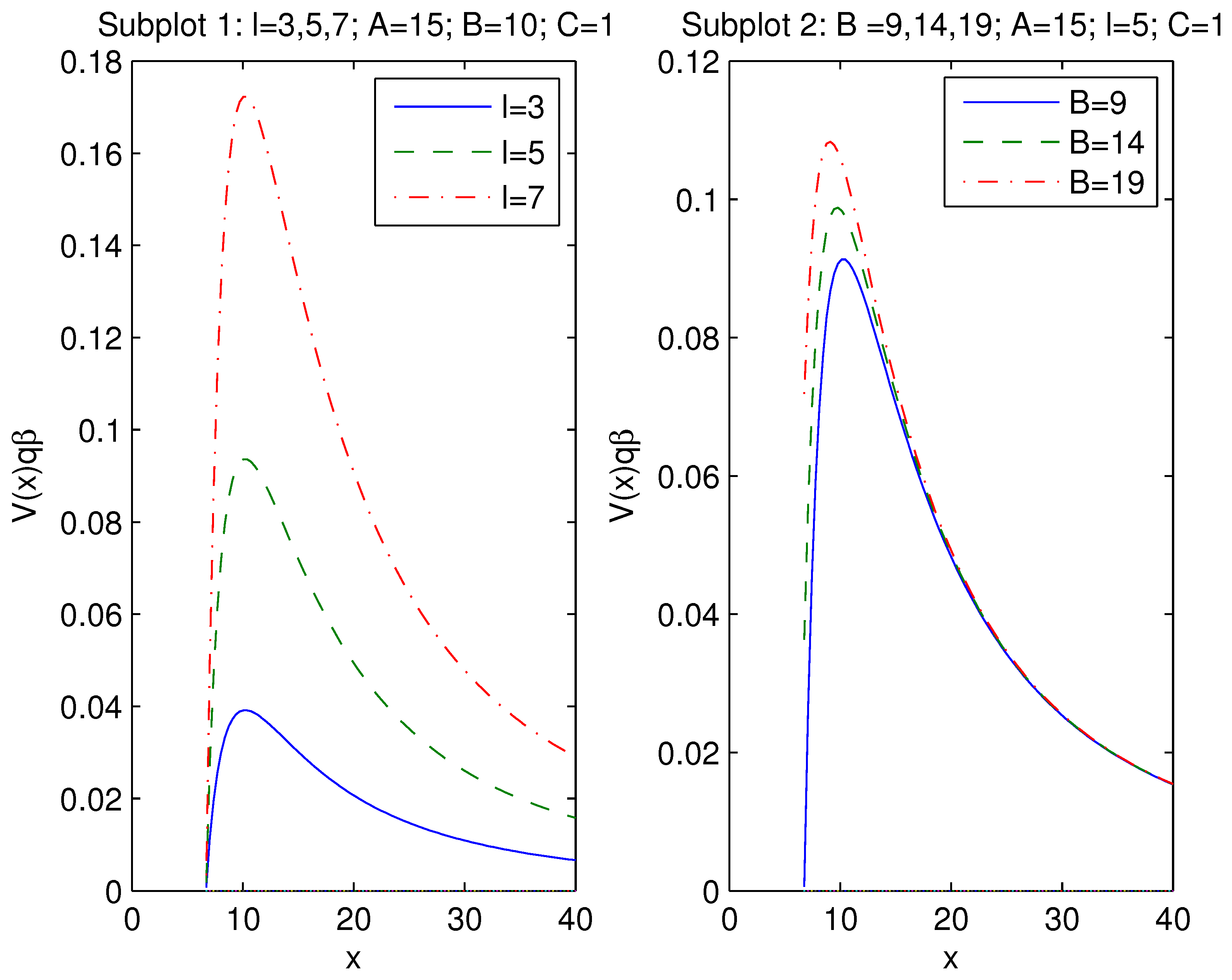
6. Quasinormal Modes
7. Summary
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Clifton, T.; Ferreira, P.G.; Padilla, A.; Skordis, C. Modified Gravity and Cosmology. Phys. Rep. 2012, 513, 1–189. [Google Scholar] [CrossRef] [Green Version]
- Will, C.M. The Confrontation between General Relativity and Experiment. Living Rev. Relativ. 2014, 17, 4. [Google Scholar] [CrossRef] [Green Version]
- Lanczos, C. Elektromagnetismus als natürliche eigenschaft der riemannschen geometrie. Z. Phys. 1932, 73, 147–168. [Google Scholar] [CrossRef]
- Lanczos, C. A remarkable property of the riemann-christoffel tensor in four dimensions. Ann. Math. 1938, 39, 842–850. [Google Scholar] [CrossRef] [Green Version]
- Lovelock, D. Divergence-free tensorial concomitants. Aequationes Math. 1970, 4, 127–138. [Google Scholar] [CrossRef]
- Lovelock, D. The Einstein tensor and its generalizations. J. Math. Phys. 1971, 12, 498–501. [Google Scholar] [CrossRef]
- Ostrogradsky, M. Mémoires sur leséquations différentielles, relatives au problème des isopérimètres. Mem. Acad. St. Petersbourg 1850, 6, 385. [Google Scholar]
- Gross, D.J.; Witten, E. Superstring modifications of Einstein’s equations. Nucl. Phys. B 1986, 277, 1–10. [Google Scholar] [CrossRef]
- Gross, D.J.; Sloan, J.H. The quartic effective action for the heterotic string. Nucl. Phys. B 1987, 291, 41–89. [Google Scholar] [CrossRef]
- Metsaev, R.R.; Tseytlin, A.A. Two-loop β-function for the generalized bosonic sigma model. Phys. Lett. B 1987, 191, 354–362. [Google Scholar] [CrossRef]
- Metsaev, R.R.; Tseytlin, A.A. Order α’ (two-loop) equivalence of the string equations of motion and the σ-model Weyl invariance conditions: Dependence on the dilaton and the antisymmetric tensor. Nucl. Phys. B 1987, 293, 385–419. [Google Scholar] [CrossRef]
- Zwiebach, B. Curvature squared terms and string theories. Phys. Lett. B 1985, 156, 315–317. [Google Scholar] [CrossRef]
- Glavan, D.; Lin, C. Einstein–Gauss–Bonnet gravity in four-dimensional spacetime. Phys. Rev. Lett. 2020, 124, 081301. [Google Scholar] [CrossRef] [Green Version]
- Aoki, K.; Gorji, M.A.; Mukohyama, S. A consistent theory of D→4 Einstein–Gauss–Bonnet gravity. Phys. Lett. B 2020, 810, 135843. [Google Scholar] [CrossRef]
- Aoki, K.; Gorji, M.A.; Mukohyama, S. Cosmology and gravitational waves in consistent D→4 Einstein–Gauss–Bonnet gravity. arXiv 2021, arXiv:2010.03973. [Google Scholar]
- Aoki, K.; Gorji, M.A.; Mizuno, S.; Mukohyama, S. Inflationary gravitational waves in consistent D→4 Einstein–Gauss–Bonnet gravity. J. Cosmol. Astropart. Phys. 2021, 1, 54. [Google Scholar] [CrossRef]
- Jafarzade, K.; Zangeneh, M.K.; Lobo, F.S.N. Shadow, deflection angle and quasinormal modes of Born–Infeld charged black holes. J. Cosmol. Astropart. Phys. 2021, 4, 8. [Google Scholar] [CrossRef]
- Fernandes, P.G.; Carrilho, P.; Clifton, T.; Mulryne, D.J. The 4D Einstein–Gauss–Bonnet theory of gravity: A review. Class. Quant. Grav. 2022, 39, 063001. [Google Scholar] [CrossRef]
- Alexeyev, S.; Sendyuk, M. Black Holes and Wormholes in Extended Gravity. Universe 2020, 6, 25. [Google Scholar] [CrossRef] [Green Version]
- Kruglov, S.I. Magnetic black holes in AdS space with nonlinear electrodynamics, extended phase space thermodynamics and Joule–Thomson expansion. Int. J. Geom. Meth. Mod. Phys. 2023, 20, 2350008. [Google Scholar] [CrossRef]
- Soleng, H.H. Charged black points in General Relativity coupled to the logarithmic U(1) gauge theory. Phys. Rev. D 1995, 52, 6178. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kruglov, S.I. On Generalized Logarithmic Electrodynamics. Eur. Phys. J. C 2015, 75, 88. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zinhailo, A.F. Quasinormal modes, stability and shadows of a black hole in the 4D Einstein–Gauss–Bonnet gravity. Eur. Phys. J. C 2020, 80, 1049. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zinhailo, A.F. 4D Einstein–Lovelock black holes: Hierarchy of orders in curvature. Phys. Lett. B 2020, 807, 135607. [Google Scholar] [CrossRef]
- Belhaj, A.; Benali, M.; Balali, A.E.; Moumni, H.E.; Ennadifi, S.E. Deflection Angle and Shadow Behaviors of Quintessential Black Holes in arbitrary Dimensions. Class. Quant. Grav. 2020, 37, 215004. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Stuchlik, Z. Are eikonal quasinormal modes linked to the unstable circular null geodesics. Phys. Lett. B 2017, 771, 597–602. [Google Scholar] [CrossRef]
- Stefanov, I.Z.; Yazadjiev, S.S.; Gyulchev, G.G. Connection between black-hole quasinormal modes and lensing in the strong deflection limit. Phys. Rev. Lett. 2010, 104, 251103. [Google Scholar] [CrossRef] [Green Version]
- Guo, Y.; Miao, Y.G. Null geodesics, quasinormal modes and the correspondence with shadows in high-dimensional Einstein–Yang–Mills spacetimes. Phys. Rev. D 2020, 102, 084057. [Google Scholar] [CrossRef]
- Wei, S.W.; Liu, Y.X. Null geodesics, quasinormal modes, and thermodynamic phase transition for charged black holes in asymptotically flat and dS spacetimes. Chin. Phys. C 2020, 44, 115103. [Google Scholar] [CrossRef]
- Birkhoff, G.D. Relativity and Modern Physics; Harvard University Press: Cambrige, MA, USA, 1923; p. 253. [Google Scholar]
- Mignemi, S.; Stewart, N.R. Charged black holes in effective string theory. Phys. Rev. D 1993, 47, 5259. [Google Scholar] [CrossRef] [Green Version]
- Medved, A.J.M.; Vagenas, E.C. When conceptual worlds collide: The GUP and the BH entropy. Phys. Rev. D 2004, 70, 124021. [Google Scholar] [CrossRef] [Green Version]
- Kruglov, S.I. Einstein–Gauss–Bonnet gravity with nonlinear electrodynamics. Ann. Phys. 2021, 428, 168449. [Google Scholar] [CrossRef]
- Kruglov, S.I. Einstein–Gauss–Bonnet Gravity with Nonlinear Electrodynamics: Entropy, Energy Emission, Quasinormal Modes and Deflection Angle. Symmetry 2021, 13, 944. [Google Scholar] [CrossRef]
- Kruglov, S.I. Einstein–Gauss–Bonnet gravity with rational nonlinear electrodynamics. EPL 2021, 133, 6. [Google Scholar] [CrossRef]
- Akiyama, K.; Alberdi, A.; Azulay, R.; Baczko, A.-K.; Baloković, M.; Barrett, J.; Blackburn, L.; Bouman, K.L.; Bower, G.C.; Brinkerink, C.D.; et al. First M87 Event Horizon Telescope Results.IV. Imaging the Central Supermassive Black Hole. Astrophys. J. 2019, 875, L1. [Google Scholar]
- Synge, J.L. The escape of photons from gravitationally intense stars. Mon. Not. R. Astron. Soc. 1966, 131, 463–466. [Google Scholar] [CrossRef]
- Kruglov, S.I. 4D Einstein–Gauss–Bonnet Gravity Coupled with Nonlinear Electrodynamics. Symmetry 2021, 13, 204. [Google Scholar] [CrossRef]
- Wei, S.W.; Liu, Y.X. Observing the shadow of Einstein–Maxwell-Dilaton-Axion black hole. J. Cosmol. Astropart. Phys. 2013, 11, 63. [Google Scholar] [CrossRef] [Green Version]
- Jusufi, K. Quasinormal Modes of Black Holes Surrounded by Dark Matter and Their Connection with the Shadow Radius. Phys. Rev. D 2020, 101, 084055. [Google Scholar] [CrossRef] [Green Version]
- Jusufi, K. Connection Between the Shadow Radius and Quasinormal Modes in Rotating Spacetimes. Phys. Rev. D 2020, 101, 124063. [Google Scholar] [CrossRef]
- Dymnikova, I. Regular electrically charged vacuum structures with de Sitter centre in nonlinear electrodynamics coupled to general relativity. Class. Quant. Grav. 2004, 21, 4417. [Google Scholar] [CrossRef]
- Hawking, S.W.; Ellis, G.F.R. The Large Scale Structure of Space-Time; Cambridge University Press: Cambridge, UK, 1973. [Google Scholar]


| B | 9 | 13.5 | 14 | 15 | 16.5 | 17.5 | 18 | 19 |
|---|---|---|---|---|---|---|---|---|
| 6.763 | 6.365 | 6.317 | 6.219 | 6.063 | 5.953 | 5.896 | 5.777 | |
| 10.313 | 9.806 | 9.746 | 9.623 | 9.431 | 9.298 | 9.229 | 9.088 | |
| 18.311 | 17.677 | 17.603 | 17.451 | 17.216 | 17.054 | 16.971 | 16.802 |
| B | 14 | 15 | 16.5 | 17.5 | 18 | 19 |
|---|---|---|---|---|---|---|
| 0.568 | 0.573 | 0.581 | 0.586 | 0.589 | 0.595 | |
| 0.2853 | 0.2852 | 0.2849 | 0.2845 | 0.2842 | 0.2835 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kruglov, S.I. 4D Einstein–Gauss–Bonnet Gravity Coupled to Modified Logarithmic Nonlinear Electrodynamics. Universe 2023, 9, 24. https://doi.org/10.3390/universe9010024
Kruglov SI. 4D Einstein–Gauss–Bonnet Gravity Coupled to Modified Logarithmic Nonlinear Electrodynamics. Universe. 2023; 9(1):24. https://doi.org/10.3390/universe9010024
Chicago/Turabian StyleKruglov, Sergey Il’ich. 2023. "4D Einstein–Gauss–Bonnet Gravity Coupled to Modified Logarithmic Nonlinear Electrodynamics" Universe 9, no. 1: 24. https://doi.org/10.3390/universe9010024






