Gauge Couplings Evolution from the Standard Model, through Pati–Salam Theory, into E8 Unification of Families and Forces
Abstract
:1. Introduction
2. Orbifolding
3. The Orbifold with
4. Anomaly-Cancelling Sector
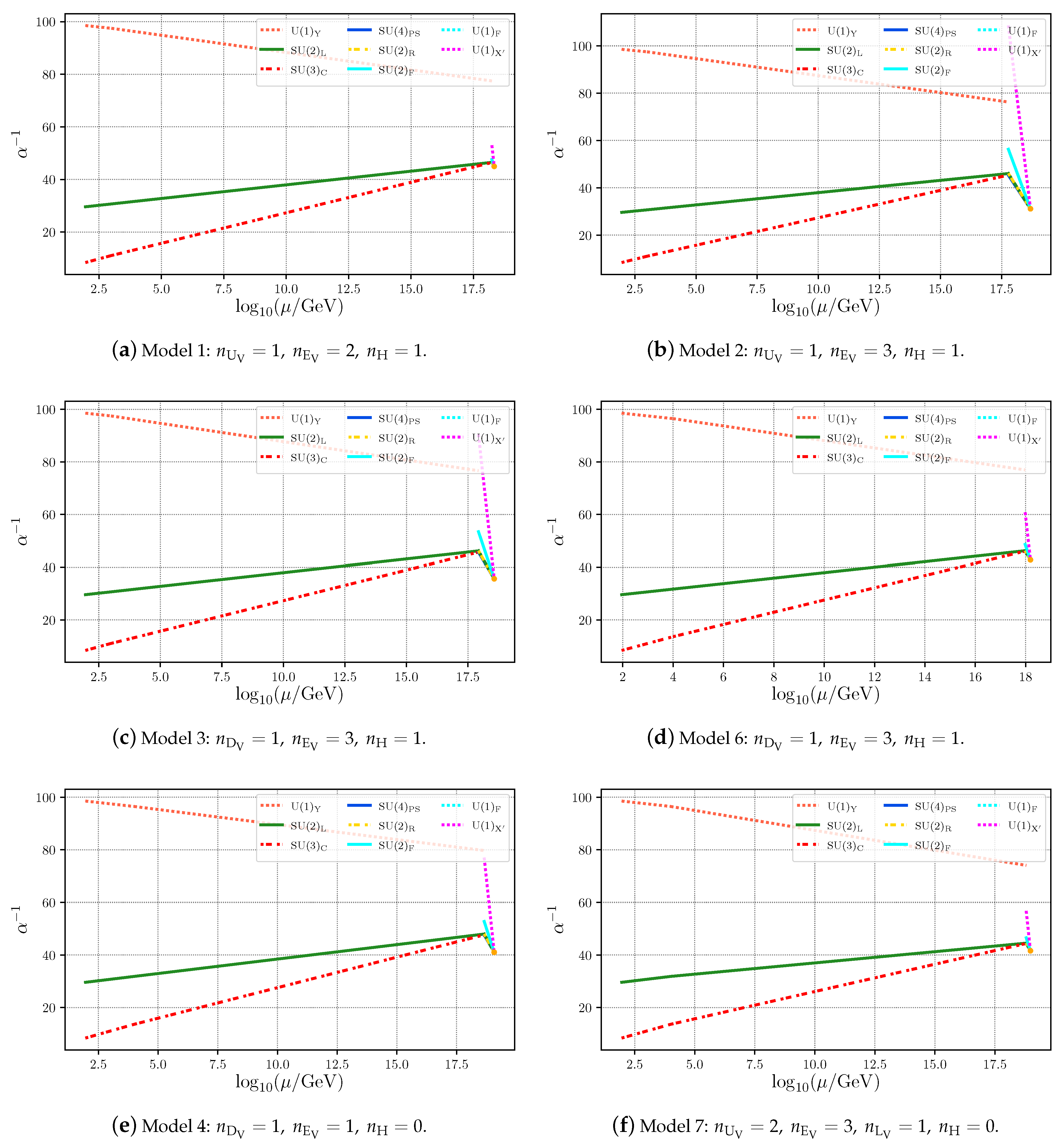
5. RG Evolution of Gauge Couplings
- 1.
- The first one corresponds to the running of the gauge couplings of the symmetry emergent from the orbifold compactification of the 10-dimensional theory as described above. In such a region, we label the beta-function coefficients as and denote the universal inverse structure constant at the GUT scale as . At this stage, all representations identified in Equation (20) contribute to the coefficients with the indicated multiplicity. Knowing that for we haveand that forwe obtain the coefficientsTaking into account the U(1) and U(1) charges and respective multiplicities also in Equation (20), the slopes of the RGEs of the Abelian inverse structure constants read aswhile U(1) and U(1) are considered to be trivially embedded into .
- 2.
- The second region corresponds to the stage after the three Wilson lines give VEVs to the SM singlet directions. The gauge group after this stage is that of the SM, and as discussed above, we only study the following possibilities:
- A scalar sector with either two or three Higgs doublets that we denote as in what follows;
- New exotic quarks containing either none or up to three generations of doublet VLQs denoted as ;
- New exotic up-type quarks containing either none or up to three generations of singlet VLQs and denoted as ;
- New exotic down-type quarks containing either none or up to three generations of singlet VLQs and denoted as ;
- New exotic leptons containing either none or up to three generations of doublet VLLs denoted as ;
- New exotic leptons containing either none or up to three generations of singlet VLLs denoted as .
Note that the choice of including up to three generations of vector-like fermions in the low-energy spectrum is not arbitrary. To see this, let us consider the possible bilinear terms involving the red and blue fields in Equation (20) that can be cast asIf we specialize on the first term, we can write a mass matrix in the basis aswhose rank is 4. Furthermore, and assuming real for illustration purposes, we see that has two degenerate eigenvalues which means that in the mass basis, we end up with one massless chiral fermion F as well as two vector-like states and . Inspired by the unified origin of our model under , in the limit where all can be thought as approximately degenerate, one can writewhere for the squared masses read asThis implies that, for a of the order of the compactification scale and for sufficiently small , we can have . In turn, it may result in up to two generations of vector-like fermions of the type not far from the TeV scale. Note that both and are doublets and so is . The exact same reasoning can be applied to the , and yielding up to two generations of the Pati–Salam fermions and up to one generation of and , motivating our choices in the bullet points above. Note that the doublet VLQs, , and VLLs belong to (two generations) and (one generation), while their singlet counterparts, , and are embedded in and . Similarly, all chiral matter belongs to the massless eigenstates and transforms according to the blue quantum numbers in Equation (20).With this in mind, the coefficients of the RGEs read aswith the various , encoding the number of extra Higgs and vector-like fermions at the low scale according to the label . - 3.
- Finally, we consider a third region below the mass threshold of the vector-like fermions and where the only New Physics states are either one or two additional Higgs doublets, i.e., a 2HDM or a 3HDM EW-scale theory. Note that the presence of three Higgs doublets can be advantageous for the generation of a realistic CKM mixing in the quark sector as discussed in refs. [16,17]. With this in mind, the beta-function coefficients in this region are
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | We are indebted to Stephen F. King for important discussions on this topic at early stages of this work. |
| 2 | The orbifold rotational conditions in Equation (11), are slightly different from the previous work in [52], which preserved the flavor symmetry . In this work the boundary conditions only preserve but, as will be seen below, these allow the Wilson lines to completely break the remaining symmetry into the SM one. This was not possible in the previous setup, making this one preferable. |
References
- Georgi, H.; Glashow, S.L. Unity of All Elementary Particle Forces. Phys. Rev. Lett. 1974, 32, 438–441. [Google Scholar] [CrossRef]
- Fritzsch, H.; Minkowski, P. Unified Interactions of Leptons and Hadrons. Annals Phys. 1975, 93, 193–266. [Google Scholar] [CrossRef]
- King, S.F.; Moretti, S.; Nevzorov, R. Exceptional supersymmetric standard model. Phys. Lett. 2006, B634, 278–284. [Google Scholar] [CrossRef]
- Buchmuller, W.; Napoly, O. Exceptional Coset Spaces and the Spectrum of Quarks and Leptons. Phys. Lett. B 1985, 163, 161. [Google Scholar] [CrossRef]
- Koca, M. Explicit Realization of E8. Lect. Notes Phys. 2005, 180, 356–359. [Google Scholar] [CrossRef]
- Slansky, R. Group Theory for Unified Model Building. Phys. Rept. 1981, 79, 1–128. [Google Scholar] [CrossRef]
- King, S.; Ross, G.G. Fermion masses and mixing angles from SU(3) family symmetry. Phys. Lett. B 2001, 520, 243–253. [Google Scholar] [CrossRef]
- King, S. Unified Models of Neutrinos, Flavour and CP Violation. Prog. Part. Nucl. Phys. 2017, 94, 217–256. [Google Scholar] [CrossRef]
- Hagedorn, C.; King, S.F.; Luhn, C. A SUSY GUT of Flavour with S4 × SU(5) to NLO. J. High Energy Phys. 2010, 06, 48. [Google Scholar] [CrossRef]
- Antusch, S.; de Medeiros Varzielas, I.; Maurer, V.; Sluka, C.; Spinrath, M. Towards predictive flavour models in SUSY SU(5) GUTs with doublet-triplet splitting. J. High Energy Phys. 2014, 9, 141. [Google Scholar] [CrossRef] [Green Version]
- Björkeroth, F.; de Anda, F.J.; de Medeiros Varzielas, I.; King, S.F. Towards a complete A4 × SU(5) SUSY GUT. J. High Energy Phys. 2015, 6, 141. [Google Scholar] [CrossRef]
- Björkeroth, F.; de Anda, F.J.; de Medeiros Varzielas, I.; King, S.F. Towards a complete Δ(27) × SO(10) SUSY GUT. Phys. Rev. D 2016, 94, 16006. [Google Scholar] [CrossRef]
- Björkeroth, F.; de Anda, F.J.; King, S.F.; Perdomo, E. A natural S4 × SO(10) model of flavour. J. High Energy Phys. 2017, 10, 148. [Google Scholar] [CrossRef]
- de Anda, F.J.; King, S.F.; Perdomo, E. SO(10) × S4 grand unified theory of flavour and leptogenesis. J. High Energy Phys. 2017, 12, 075. [Google Scholar] [CrossRef]
- Cárcamo Hernández, A.; Huong, D.; Kovalenko, S.; Morais, A.P.; Pasechnik, R.; Schmidt, I. How low-scale trinification sheds light in the flavor hierarchies, neutrino puzzle, dark matter, and leptogenesis. Phys. Rev. D 2020, 102, 95003. [Google Scholar] [CrossRef]
- Morais, A.P.; Pasechnik, R.; Porod, W. Prospects for New Physics from Gauge Left-Right-Colour-Family Grand Unification. Eur. Phys. J. 2020, C80, 1162. [Google Scholar] [CrossRef]
- Morais, A.P.; Pasechnik, R.; Porod, W. Grand Unified origin of gauge interactions and families replication in the Standard Model. Universe 2021, 7, 461. [Google Scholar] [CrossRef]
- Camargo-Molina, J.E.; Morais, A.P.; Ordell, A.; Pasechnik, R.; Sampaio, M.O.; Wessén, J. Reviving trinification models through an E6 -extended supersymmetric GUT. Phys. Rev. 2017, D95, 75031. [Google Scholar] [CrossRef]
- Camargo-Molina, J.E.; Morais, A.P.; Ordell, A.; Pasechnik, R.; Wessén, J. Scale hierarchies, symmetry breaking and particle spectra in SU(3)-family extended SUSY trinification. Phys. Rev. 2019, D99, 35041. [Google Scholar] [CrossRef]
- Camargo-Molina, J.E.; Morais, A.P.; Pasechnik, R.; Wessén, J. On a radiative origin of the Standard Model from Trinification. J. High Energy Phys. 2016, 9, 129. [Google Scholar] [CrossRef] [Green Version]
- Altarelli, G.; Feruglio, F.; Hagedorn, C. A SUSY SU(5) Grand Unified Model of Tri-Bimaximal Mixing from A4. J. High Energy Phys. 2008, 3, 52. [Google Scholar] [CrossRef]
- Burrows, T.; King, S. A(4) Family Symmetry from SU(5) SUSY GUTs in 6d. Nucl. Phys. B 2010, 835, 174–196. [Google Scholar] [CrossRef]
- Burrows, T.; King, S. A4 x SU(5) SUSY GUT of Flavour in 8d. Nucl. Phys. B 2011, 842, 107–121. [Google Scholar] [CrossRef]
- de Anda, F.J.; King, S.F. An S4 × SU(5) SUSY GUT of flavour in 6d. J. High Energy Phys. 2018, 7, 57. [Google Scholar] [CrossRef]
- Altarelli, G.; Feruglio, F.; Lin, Y. Tri-bimaximal neutrino mixing from orbifolding. Nucl. Phys. B 2007, 775, 31–44. [Google Scholar] [CrossRef]
- Adulpravitchai, A.; Schmidt, M.A. Flavored Orbifold GUT—An SO(10) x S4 model. J. High Energy Phys. 2011, 1, 106. [Google Scholar] [CrossRef]
- Adulpravitchai, A.; Blum, A.; Lindner, M. Non-Abelian Discrete Flavor Symmetries from T**2/Z(N) Orbifolds. J. High Energy Phys. 2009, 7, 53. [Google Scholar] [CrossRef]
- Asaka, T.; Buchmuller, W.; Covi, L. Gauge unification in six-dimensions. Phys. Lett. B 2001, 523, 199–204. [Google Scholar] [CrossRef]
- de Anda, F.J.; Valle, J.W.; Vaquera-Araujo, C.A. Flavour and CP predictions from orbifold compactification. Phys. Lett. B 2020, 801, 135195. [Google Scholar] [CrossRef]
- de Anda, F.J.; King, S.F.; Perdomo, E. SU(5) grand unified theory with A4 modular symmetry. Phys. Rev. D 2020, 101, 15028. [Google Scholar] [CrossRef] [Green Version]
- de Anda, F.J.; King, S.F. SU(3) × SO(10) in 6d. J. High Energy Phys. 2018, 10, 128. [Google Scholar] [CrossRef]
- Ibanez, L.E.; Mas, J.; Nilles, H.P.; Quevedo, F. Heterotic Strings in Symmetric and Asymmetric Orbifold Backgrounds. Nucl. Phys. B 1988, 301, 157–196. [Google Scholar] [CrossRef]
- Parr, E.; Vaudrevange, P.K.; Wimmer, M. Predicting the orbifold origin of the MSSM. Fortsch. Phys. 2020, 68, 2000032. [Google Scholar] [CrossRef]
- Adler, S.L. Should E(8) SUSY Yang-Mills be reconsidered as a family unification model? Phys. Lett. 2002, B533, 121–125. [Google Scholar] [CrossRef]
- Adler, S.L. Further Thoughts on Supersymmetric E(8) as a Family and Grand Unification Theory 2004. Available online: https://arxiv.org/abs/hep-ph/0401212 (accessed on 12 December 2022).
- Garibaldi, S. E8, the most exceptional group. Bull. Am. Math. Soc. 2016, 53, 643–671. [Google Scholar] [CrossRef]
- Thomas, S. Softly Broken N = 4 and E8. J. Phys. A 1986, 19, 1141. [Google Scholar] [CrossRef]
- Konshtein, S.; Fradkin, E. Asymptotically Supersymmetric Model of unified Interaction Based On E8. Pisma Zh. Eksp. Teor. Fiz. 1980, 32, 575–578. (In Russian) [Google Scholar]
- Baaklini, N. Supersymmetric Exceptional Gauge Unification. Phys. Rev. D 1980, 22, 3118–3127. [Google Scholar] [CrossRef]
- Baaklini, N. Supergrand Unification in E8. Phys. Lett. B 1980, 91, 376–378. [Google Scholar] [CrossRef]
- Barr, S.M. E8 family unification, mirror fermions, and new low-energy physics. Phys. Rev. D 1988, 37, 204. [Google Scholar] [CrossRef]
- Bars, I.; Gunaydin, M. Grand Unification With the Exceptional Group E8. Phys. Rev. Lett. 1980, 45, 859. [Google Scholar] [CrossRef]
- Koca, M. On Tumbling E8. Phys. Lett. B 1981, 107, 73–76. [Google Scholar] [CrossRef]
- Mahapatra, S.; Deo, B. Supergravity Induced E(8) Gauge Hierarchies. Phys. Rev. D 1988, 38, 3554. [Google Scholar] [CrossRef] [PubMed]
- Ong, C.L. Supersymmetric Models for Quarks and Leptons With Nonlinearly Realized E8 Symmetry. Phys. Rev. D 1985, 31, 3271. [Google Scholar] [CrossRef]
- Olive, D.I.; West, P.C. The N=4 Supersymmetric E(8) Gauge Theory and Coset Space: Dimensional Reduction. Nucl. Phys. B 1983, 217, 248–284. [Google Scholar] [CrossRef]
- Arkani-Hamed, N.; Gregoire, T.; Wacker, J.G. Higher dimensional supersymmetry in 4-D superspace. J. High Energy Phys. 2002, 3, 55. [Google Scholar] [CrossRef]
- Brink, L.; Schwarz, J.H.; Scherk, J. Supersymmetric Yang-Mills Theories. Nucl. Phys. B 1977, 121, 77–92. [Google Scholar] [CrossRef]
- Kawamura, Y. Triplet doublet splitting, proton stability and extra dimension. Prog. Theor. Phys. 2001, 105, 999–1006. [Google Scholar] [CrossRef]
- Hall, L.J.; Nomura, Y. Gauge unification in higher dimensions. Phys. Rev. D 2001, 64, 55003. [Google Scholar] [CrossRef]
- Hall, L.J.; Murayama, H.; Nomura, Y. Wilson lines and symmetry breaking on orbifolds. Nucl. Phys. B 2002, 645, 85–104. [Google Scholar] [CrossRef]
- Aranda, A.; de Anda, F.J.; King, S.F. Exceptional Unification of Families and Forces. Nucl. Phys. B 2020, 960, 115209. [Google Scholar] [CrossRef]
- Aranda, A.; de Anda, F.J. Complete E8 Unification in 10 Dimensions. Int. J. Mod. Phys. 2021, A36, 2150112. [Google Scholar] [CrossRef]
- Pati, J.C.; Salam, A. Unified Lepton-Hadron Symmetry and a Gauge Theory of the Basic Interactions. Phys. Rev. D 1973, 8, 1240–1251. [Google Scholar] [CrossRef]
- Braam, F.; Knochel, A.; Reuter, J. An Exceptional SSM from E6 Orbifold GUTs with intermediate LR symmetry. J. High Energy Phys. 2010, 6, 13. [Google Scholar] [CrossRef]
- Dixon, L.J.; Harvey, J.A.; Vafa, C.; Witten, E. Strings on Orbifolds. Nucl. Phys. 1985, B261, 678–686. [Google Scholar] [CrossRef]
- Dixon, L.J.; Harvey, J.A.; Vafa, C.; Witten, E. Strings on Orbifolds. 2. Nucl. Phys. 1986, B274, 285–314. [Google Scholar] [CrossRef]
- Groot Nibbelink, S.; Loukas, O.; Mütter, A.; Parr, E.; Vaudrevange, P.K. Tension Between a Vanishing Cosmological Constant and Non-Supersymmetric Heterotic Orbifolds. Fortsch. Phys. 2020, 68, 2000044. [Google Scholar] [CrossRef]
- De Anda, F.J.; King, S.F.; Perdomo, E.; Vaudrevange, P.K. Flavon alignments from orbifolding: SU(5) × SU(3) model with T6/Δ(54). J. High Energy Phys. 2019, 12, 55. [Google Scholar] [CrossRef]
- Fischer, M.; Ratz, M.; Torrado, J.; Vaudrevange, P.K. Classification of symmetric toroidal orbifolds. J. High Energy Phys. 2013, 1, 84. [Google Scholar] [CrossRef]
- Fischer, M.; Ramos-Sanchez, S.; Vaudrevange, P.K.S. Heterotic non-Abelian orbifolds. J. High Energy Phys. 2013, 7, 80. [Google Scholar] [CrossRef]
- Hosotani, Y. Dynamical Mass Generation by Compact Extra Dimensions. Phys. Lett. B 1983, 126, 309–313. [Google Scholar] [CrossRef]
- Hosotani, Y. Dynamical Gauge Symmetry Breaking as the Casimir Effect. Phys. Lett. B 1983, 129, 193–197. [Google Scholar] [CrossRef]
- Hosotani, Y.; Noda, S.; Takenaga, K. Dynamical gauge-Higgs unification in the electroweak theory. Phys. Lett. B 2005, 607, 276–285. [Google Scholar] [CrossRef]
- Hosotani, Y.; Noda, S.; Takenaga, K. Dynamical gauge symmetry breaking and mass generation on the orbifold T2/Z2. Phys. Rev. D 2004, 69, 125014. [Google Scholar] [CrossRef]
- Haba, N.; Hosotani, Y.; Kawamura, Y.; Yamashita, T. Dynamical symmetry breaking in gauge Higgs unification on orbifold. Phys. Rev. D 2004, 70, 15010. [Google Scholar] [CrossRef]
- Haba, N.; Harada, M.; Hosotani, Y.; Kawamura, Y. Dynamical rearrangement of gauge symmetry on the orbifold S1 / Z(2). Nucl. Phys. B 2003, 657, 169–213. [Google Scholar] [CrossRef]
- Dreiner, H.K. An Introduction to explicit R-parity violation. Adv. Ser. Direct. High Energy Phys. 2010, 21, 565–583. [Google Scholar] [CrossRef]
- Barbier, R.; Bérat, C.; Besancon, M.; Chemtob, M.; Deandrea, A.; Dudas, E.; Fayet, P.; Lavignac, S.; Moreau, G.; Perez, E.; et al. R-parity violating supersymmetry. Phys. Rept. 2005, 420, 1–202. [Google Scholar] [CrossRef]
- Branco, G.C.; Lavoura, L.; Silva, J.P. CP Violation. Int. Ser. Monogr. Phys. 1999, 103, 1–536. [Google Scholar] [CrossRef]
- Branco, G.C.; Ferreira, P.M.; Lavoura, L.; Rebelo, M.N.; Sher, M.; Silva, J.P. Theory and phenomenology of two-Higgs-doublet models. Phys. Rept. 2012, 516, 1–102. [Google Scholar] [CrossRef]
- Ivanov, I.P. Building and testing models with extended Higgs sectors. Prog. Part. Nucl. Phys. 2017, 95, 160–208. [Google Scholar] [CrossRef] [Green Version]
- Freitas, F.F.; Gonçalves, J.; Morais, A.P.; Pasechnik, R. Phenomenology of Vector-Like Leptons with Deep Learning at the Large Hadron Collider. J. High Energy Phys. 2021, 01, 076. [Google Scholar] [CrossRef]

| V | ||||
|---|---|---|---|---|
| Model | ||||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 1 | 1 | 0 | 0 | 2 | |||
| 2 | 1 | 1 | 0 | 0 | 3 | |||
| 3 | 1 | 0 | 1 | 0 | 3 |
| Model | ||||||||
|---|---|---|---|---|---|---|---|---|
| 4 | 0 | 0 | 1 | 0 | 1 | |||
| 5 | 1 | 1 | 0 | 0 | 3 | |||
| 6 | 1 | 0 | 1 | 0 | 3 | |||
| 7 | 0 | 2 | 0 | 1 | 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aranda, A.; Anda, F.J.d.; Morais, A.P.; Pasechnik, R. Gauge Couplings Evolution from the Standard Model, through Pati–Salam Theory, into E8 Unification of Families and Forces. Universe 2023, 9, 90. https://doi.org/10.3390/universe9020090
Aranda A, Anda FJd, Morais AP, Pasechnik R. Gauge Couplings Evolution from the Standard Model, through Pati–Salam Theory, into E8 Unification of Families and Forces. Universe. 2023; 9(2):90. https://doi.org/10.3390/universe9020090
Chicago/Turabian StyleAranda, Alfredo, Francisco J. de Anda, António P. Morais, and Roman Pasechnik. 2023. "Gauge Couplings Evolution from the Standard Model, through Pati–Salam Theory, into E8 Unification of Families and Forces" Universe 9, no. 2: 90. https://doi.org/10.3390/universe9020090
APA StyleAranda, A., Anda, F. J. d., Morais, A. P., & Pasechnik, R. (2023). Gauge Couplings Evolution from the Standard Model, through Pati–Salam Theory, into E8 Unification of Families and Forces. Universe, 9(2), 90. https://doi.org/10.3390/universe9020090







