Seven Hints That Early-Time New Physics Alone Is Not Sufficient to Solve the Hubble Tension
Abstract
:1. Introduction
2. Seven Hints
- ges of the oldest astrophysical objects;
- aryon Acoustic Oscillations sound horizon-Hubble constant degeneracy slope (compared to the same slope in the CMB);
- osmic chronometers;
- escending trends observed in a wide range of low-redshift data;
- arly integrated Sachs-Wolfe effect and the restrictions it imposes on early-time new physics;
- ractional matter density constraints from early-Universe physics insensitive and uncalibrated cosmic standards;
- alaxy power spectrum sound horizon- and equality wavenumber-based determinations of the Hubble constant.
2.1. Ages of the Oldest Astrophysical Objects
- the age of the Universe at any given redshift is self-evidently inversely proportional to the Hubble constant, ;
- it is possible for different cosmological models to lead to the same value of (at ), while predicting a completely different evolution at high redshift.
- create an OAO age-redshift catalog;
- choose a given model for the late-time expansion (the “null hypothesis” which will be the subject of the consistency test);
- impose (in a statistical sense) that the age of the Universe at any redshift within the chosen model exceeds the OAO ages—given the previous point 1), this will lead to an upper limit on ;
- the derived upper limit being in tension with local measurements would indicate an inconsistency in the chosen cosmological model and thus the need for at least some amount of late-time new physics (“new” relative to the chosen model)—conversely, absence of tension is at best an indication that there is no inconsistency yet, since it is in principle possible that OAO older than those present in the catalog may not yet have been identified.
2.2. BAO Sound Horizon-Hubble Constant Degeneracy Slope
2.3. Cosmic Chronometers
- regardless of whether one goes parametric or non-parametric, the value of inferred from CC is the cosmological (as opposed to local) one;
- as CC directly measure the late-time () expansion rate, a parametric analysis thereof requires no assumption whatsoever about early Universe physics (this is actually true for a non-parametric inference as well).
- should the value of inferred from CC within a certain model be in tension with local measurements, such a conclusion would be completely independent of whatever happened in the early Universe, including before and around recombination;
- assuming such a (residual) tension is physical (i.e., not due to systematics), resolving it would require introducing new (relative to the assumed parametric model) late-time ingredients to alter the cosmological value of , and/or new local physics to alter the local value of .
2.4. Descending Trends in Low-Redshift Data
- Has such a redshift evolution already been observed in current data?
- Has it been observed across different independent datasets, and if so is there a common trend across these independent datasets?
- Are the size and/or direction of this trend inconsistent with what one would expect if no new physics were at play, i.e., can it be attributed to new physics?
2.5. Early Integrated Sachs-Wolfe Effect
2.6. (Fractional) Matter Density Constraints from Early-Universe Physics Insensitive and Uncalibrated Cosmic Standards
2.7. Galaxy Power Spectrum Sound Horizon- and Equality Wavenumber-Based Determinations of the Hubble Constant
- the constraints on obtained from -marginalized analyses are model-dependent, although not to a large extent;
- the results depend to some extent on the assumed priors on , , and ;
- models which introduce additional energy density with significant pressure support (such as EDE or models increasing the effective number of relativistic species ) can lead to higher values even in -free analyses (for such models the -based value of is actually slightly lower than the -based value, although the error bars are huge).
3. Reflections on Promising Scenarios Moving Forward
- What about Occam’s razor? True, such a scenario may be viewed as unnecessary complicated, and aesthetically unpleasing, to the eyes of some. My view on this point is that, in the field of cosmology, Occam’s razor (and with it the concept of Bayesian evidence) is sometimes overused/abused. Not always is the simplest model the “most correct” one just because it fits the data better or with less parameters. Think, for example, about the many parameters for which we need to impose physical priors in cosmological analyses, to avoid inferring unphysical values thereof (the sum of the neutrino masses is an excellent example), which would otherwise be preferred by the data. If the true model chosen by Nature is actually as complicated as shown in Figure 11... then so be it! With a bit of poetic liberty, allow me to paraphrase Neil de Grasse Tyson and state that “Nature [the universe] is under no obligation to appear beautiful or simple in our eyes [make sense to you]”.
- You haven’t mentioned any concrete combination of early-plus-late-plus-local new physics models which does what you advocate. True once more, and this is left as an exercise to the reader and to aspiring Hubble tension solvers (including myself—so I hope to report on this in future work).
- Can all these early-plus-late-plus-local new physics ingredients come from one single underlying microphysical model, thus reducing the overall complexity? Maybe, why not? But once more, I am leaving this as an exercise to the reader and to aspiring Hubble tension solvers (this time likely not including myself).
4. Conclusions
- the CDM Universe appears a bit too young to accommodate the oldest astrophysical objects at high redshift, and this is a problem which cannot be fixed by new physics in the early Universe, but requires new physics at late times or in the local Universe;
- early-Universe new physics which only reduces the sound horizon cannot simultaneously agree with CMB, BAO, local , and WL data, and will necessarily introduce new tensions (or worsen existing ones) involving some of these observations;
- cosmic chronometers show a residual ≈2 tension with local measurements within CDM, a conclusion which is completely independent of early-Universe physics, and which therefore cannot be addressed by invoking the latter;
- if the tension is physical (in the sense of not being due to systematics) and calls for some amount of late-time new physics, evolving trends should be seen at intermediate redshifts between the CMB and local scales, and by now several independent hints thereof have appeared;
- the early ISW effect places very restrictive guard rails on what early-Universe physics may or may not do, and for models enhancing the pre-recombination expansion rate this often results in fixing the otherwise overpredicted eISW amplitude at the expense of worsening other tensions;
- early-Universe-independent uncalibrated cosmic standard constraints on and show a residual ≈2 tension with local measurements which cannot, by construction, be fixed by early-time new physics, but most involve late-time or local new physics;
- the good agreement between sound horizon- and equality wavenumber-based constraints on from galaxy power spectra measurements makes it relatively unlikely that a significant amount of new physics operating before recombination can be at play.
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| ACT | Atacama Cosmology Telescope |
| BBN | Big Bang Nucleosynthesis |
| BAO | Baryon Acoustic Oscillations |
| CC | Cosmic Chronometers |
| C.L. | Confidence Level |
| CMB | Cosmic Microwave Background |
| DE | Dark Energy |
| DM | Dark Matter |
| EDE | Early Dark Energy |
| EdS | Einstein-de Sitter |
| eISW | early Integrated Sachs-Wolfe |
| EoS | Equation of State |
| FLRS | Friedmann-Lemaître-Robertson-Walker |
| ISW | Integrated Sachs-Wolfe |
| LSS | Large-Scale Structure |
| MCMC | Markov Chain Monte Carlo |
| OAO | Old Astrophysical Objects |
| QSOs | Quasars |
| SNeIa | Type Ia Supernovae |
| UCS | Uncalibrated Cosmic Standards |
| WL | Weak Lensing |
| 1 | Or with an added, uncalled for, adverb “exclusively” between “calls” and “for”. |
| 2 | It has been argued that the number of objects an average human can hold in short-term memory is , a finding sometimes referred to as Miller’s law. Together with the many special and symbolic properties of the number seven, this is the reason behind my choice of focusing on seven hints. |
| 3 | Prior to 1998, and particularly in the 1980s, the leading cosmological model envisaged an Einstein-de Sitter (EdS) Universe, with a vanishing cosmological constant and no spatial curvature. The existence of old galaxies at high redshift (in conjunction with increasingly precise measurements of ), seemingly older than the EdS Universe, gave rise to an important “age crisis” [572,573,574], whose resolution eventually came with the discovery of cosmic acceleration in 1998. In fact, compared to an EdS Universe with the same total energy density, a CDM Universe where part of the matter content is replaced by dark energy naturally leads to an older Universe at any redshift, thus accommodating the otherwise puzzling OAO. |
| 4 | Note that, although Equation (1) is formally integrated up to , since in practice OAO can only form after recombination, the upper limit of the integral can be set to , the redshift of recombination. This prevents it from being sensitive to huge pre-BBN modifications to which could make arbitrarily large, although such modifications are not of interest in the discussion on the Hubble tension. |
| 5 | |
| 6 | Here by “model-independent” I really mean independent of any assumed underlying cosmological model, where the cosmological model-dependence of the “standard” CMB, BAO, SNeIa probes may be traded for dependence on other models, e.g., of more astrophysical/astronomical nature. |
| 7 | I follow the procedure outlined in gitlab.com/mmoresco/CCcovariance, accessed on 18 May 2023. |
| 8 | The OAO and CC datasets are mostly independent, except for 8 CC measurements with relatively large uncertainties [600], whose relative statistical weight in the conclusions is therefore low. Note that the reliability of these measurements has also been recently questioned by Kjerrgren and Mörtsell [601]. Given their low statistical weight in reaching my conclusions, a posteriori this should not present a concern. |
| 9 | This effective equation of state directly enters the second Friedmann equation (the acceleration equation) and includes contributions from all species, not only dark energy. |
| 10 | Of course the data need not be exactly in the form of , but other types of data (e.g., distance measurements) can be brought into this form, or conversely Equation (5) can be generalized to account for other types of data. |
| 11 | In principle, if some early-time new physics raised the CMB value of to be perfectly in agreement with the local value, and the late-time Universe were completely described by CDM, there should be no evolving trend at intermediate redshifts. |
| 12 | At this point there is no reason not to refer to this trend as a tension where, if one wants, the tension is with the mathematical requirement that be a (integration) constant. |
| 13 | In this case, rather than binning the data in redshift, the choice was to only use data above a certain redshift and examine the effect of the lower redshift cutoff. |
| 14 | The reliability of Hubble diagrams constructed out of QSOs data has been questioned (see e.g., Refs. [619,620]), but methods to overcome selection biases and astrophysical evolution in the QSOs parameters have also been tested [621,622,623,624,625]. Similar considerations hold for Gamma Ray Bursts as a cosmological probe [334,626,627,628,629]. |
| 15 | Tongue-in-cheek, one could say that this is the Hubble tension equivalent of the much more famous Fermi paradox. |
| 16 | In particular in Ref. [634] I argued that models raising the pre-recombination expansion rate, of which EDE can be considered a prototype (but certainly not the only example), naturally boost the eISW effect due to the enhanced potential decay. |
| 17 | In the case of SNeIa one simply needs to treat the SNeIa absolute magnitude as a free parameter to be marginalized over: however, this is what is routinely done anyway when analyzing uncalibrated SNeIa data (whereas it is much less common to analyze BAO data treating as a free parameter, rather an early-Universe model is usually assumed). |
| 18 | |
| 19 | In what follows, I will focus on galaxies as tracers of the LSS, and will therefore refer to “galaxy clustering”, although the subsequent discussion can apply to any LSS tracer. |
| 20 | See e.g., Refs. [4,7,240,575,583,588,621,662,663,664,665,666,667,668,669,670,671,672,673,674,675,676,677,678,679] for examples of recent analyses in these directions whose inferred central values of w (in some cases depending on the specific dataset combination or underlying model considered) lie slightly within the phantom regime, arising from a wide range of dataset combinations, mostly involving combinations of Planck CMB data with other external late-time measurements, and see Ref. [680] for a recent reassessment of this point. |
| 21 | Dark scattering-type scenarios studied in the literature include DM-DE scattering [293,688,689,690,691,692,693,694,695,696,697,698,699,700,701,702,703,704,705,706,707,708,709,710,711,712,713,714,715,716], DM-photon scattering [83,717,718,719], DM-neutrino scattering [71,720,721,722,723,724], DM-baryon scattering [725,726,727,728,729,730,731,732,733,734,735], DM self-scattering and scattering with dark radiation [66,136,736,737,738,739,740,741], “multi-interacting DM” scenarios featuring multiple similar interactions simultaneously [742], and DE-baryon scattering [743,744,745,746,747]. |
References
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational evidence from supernovae for an accelerating universe and a cosmological constant. Astron. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef]
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Castro, P.G.; Deustua, S.; Fabbro, S.; Goobar, A.; Groom, D.E.; et al. Measurements of Ω and Λ from 42 high redshift supernovae. Astrophys. J. 1999, 517, 565–586. [Google Scholar] [CrossRef]
- Troxel, M.A.; MacCrann, N.; Zuntz, J.; Eifler, T.F.; Krause, E.; Dodelson, S.; Gruen, D.; Blazek, J.; Friedrich, O.; Samuroff, S.; et al. Dark Energy Survey Year 1 results: Cosmological constraints from cosmic shear. Phys. Rev. D 2018, 98, 43528. [Google Scholar] [CrossRef]
- Aghanim, N. et al. [Planck Collaboration] Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6, Erratum in Astron. Astrophys. 2021, 652, C4. [Google Scholar]
- Bianchini, F.; Wu, W.L.K.; Ade, P.A.R.; Anderson, A.J.; Austermann, J.E.; Avva, J.S.; Beall, J.A.; Bender, A.N.; Benson, B.A.; Bleem, L.E.; et al. Constraints on Cosmological Parameters from the 500 deg2 SPTpol Lensing Power Spectrum. Astrophys. J. 2020, 888, 119. [Google Scholar] [CrossRef]
- Aiola, S.; Calabrese, E.; Maurin, L.; Naess, S.; Schmitt, B.L.; Abitbol, M.H.; Addison, G.E.; Ade, P.A.R.; Alonso, D.; Amiri, M.; et al. The Atacama Cosmology Telescope: DR4 Maps and Cosmological Parameters. JCAP 2020, 12, 47. [Google Scholar] [CrossRef]
- Alam, S.; Aubert, M.; Avila, S.; Balland, C.; Bautista, J.E.; Bershady, M.A.; Bizyaev, D.; Blanton, M.R.; Bolton, A.S.; Bovy, J.; et al. Completed SDSS-IV extended Baryon Oscillation Spectroscopic Survey: Cosmological implications from two decades of spectroscopic surveys at the Apache Point Observatory. Phys. Rev. D 2021, 103, 83533. [Google Scholar] [CrossRef]
- Asgari, M.; Lin, C.-A.; Joachimi, B.; Giblin, B.; Heymans, C.; Hildebrandt, H.; Kannawadi, A.; Stolzner, B.; Troster, T.; van den Busch, J.L.; et al. KiDS-1000 Cosmology: Cosmic shear constraints and comparison between two point statistics. Astron. Astrophys. 2021, 645, A104. [Google Scholar] [CrossRef]
- Mossa, V.; Stockel, K.; Cavanna, F.; Ferraro, F.; Aliotta, M.; Barile, F.; Bemmerer, D.; Best, A.; Boeltzig, A.; Broggini, C.; et al. The baryon density of the Universe from an improved rate of deuterium burning. Nature 2020, 587, 210–213. [Google Scholar] [CrossRef]
- Brout, D.; Scolnic, D.; Popovic, B.; Riess, A.G.; Zuntz, J.; Kessler, R.; Carr, A.; Davis, T.M.; Hinton, S.; Jones, D.; et al. The Pantheon+ Analysis: Cosmological Constraints. Astrophys. J. 2022, 938, 110. [Google Scholar] [CrossRef]
- Riess, A.G.; Yuan, W.L.; Macri, L.M.; Scolnic, D.; Brout, D.; Casertano, S.; Jones, D.O.; Murakami, Y.; Anand, G.S.; Breuval, L.; et al. A Comprehensive Measurement of the Local Value of the Hubble Constant with 1 km s−1 Mpc−1 Uncertainty from the Hubble Space Telescope and the SH0ES Team. Astrophys. J. Lett. 2022, 934, L7. [Google Scholar] [CrossRef]
- Fernández Arenas, D.; Terlevich, E.; Terlevich, R.; Melnick, J.; Chávez, R.; Bresolin, F.; Telles, E.; Plionis, M.; Basilakos, S. An independent determination of the local Hubble constant. Mon. Not. R. Astron. Soc. 2018, 474, 1250–1276. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.M.; Scolnic, D. Large Magellanic Cloud Cepheid Standards Provide a 1% Foundation for the Determination of the Hubble Constant and Stronger Evidence for Physics beyond ΛCDM. Astrophys. J. 2019, 876, 85. [Google Scholar]
- Wong, K.C.; Suyu, S.H.; Chen, G.C.F.; Rusu, C.E.; Millon, M.; Sluse, D.; Bonvin, V.; Fassnacht, C.D.; Taubenberger, S.; Auger, M.W.; et al. H0LiCOW–XIII. A 2.4 per cent measurement of H0 from lensed quasars: 5.3σ tension between early- and late-Universe probes. Mon. Not. R. Astron. Soc. 2020, 498, 1420–1439. [Google Scholar]
- Freedman, W.L.; Madore, B.F.; Hatt, D.; Hoyt, T.J.; Jang, I.-S.; Beaton, R.L.; Burns, C.R.; Lee, M.G.; Monson, A.J.; Neeley, J.R.; et al. The Carnegie-Chicago Hubble Program. VIII. An Independent Determination of the Hubble Constant Based on the Tip of the Red Giant Branch. Astrophys. J. 2019, 882, 34. [Google Scholar] [CrossRef]
- Yuan, W.; Riess, A.G.; Macri, L.M.; Casertano, S.; Scolnic, D. Consistent Calibration of the Tip of the Red Giant Branch in the Large Magellanic Cloud on the Hubble Space Telescope Photometric System and a Re-determination of the Hubble Constant. Astrophys. J. 2019, 886, 61. [Google Scholar] [CrossRef]
- Huang, C.D.; Riess, A.G.; Yuan, W.; Macri, L.M.; Zakamska, N.L.; Casertano, S.; Whitelock, P.A.; Hoffmann, S.L.; Filippenko, A.V.; Scolnic, D. Hubble Space Telescope Observations of Mira Variables in the Type Ia Supernova Host NGC 1559: An Alternative Candle to Measure the Hubble Constant. Astrophys. J. 2020, 889, 5. [Google Scholar]
- Pesce, D.W.; Braatz, J.A.; Reid, M.J.; Riess, A.G.; Scolnic, D.; Condon, J.J.; Gao, F.; Henkel, C.; Impellizzeri, C.M.V.; Kuo, C.Y.; et al. The Megamaser Cosmology Project. XIII. Combined Hubble constant constraints. Astrophys. J. Lett. 2020, 891, L1. [Google Scholar] [CrossRef]
- de Jaeger, T.; Stahl, B.E.; Zheng, W.; Filippenko, A.V.; Riess, A.G.; Galbany, L. A measurement of the Hubble constant from Type II supernovae. Mon. Not. R. Astron. Soc. 2020, 496, 3402–3411. [Google Scholar]
- Schombert, J.; McGaugh, S.; Lelli, F. Using the Baryonic Tully–Fisher Relation to Measure H o. Astron. J. 2020, 160, 71. [Google Scholar] [CrossRef]
- Blakeslee, J.P.; Jensen, J.B.; Ma, C.P.; Milne, P.A.; Greene, J.E. The Hubble Constant from Infrared Surface Brightness Fluctuation Distances. Astrophys. J. 2021, 911, 65. [Google Scholar]
- Verde, L.; Treu, T.; Riess, A.G. Tensions between the Early and the Late Universe. Nat. Astron. 2019, 3, 891. [Google Scholar] [CrossRef]
- Riess, A.G. The Expansion of the Universe is Faster than Expected. Nat. Rev. Phys. 2019, 2, 10–12. [Google Scholar] [CrossRef]
- Di Valentino, E.; Anchordoqui, L.A.; Akarsu, O.; Ali-Haimoud, Y.; Amendola, L.; Arendse, N.; Asgari, M.; Ballardini, M.; Basilakos, S.; Battistelli, E.; et al. Snowmass2021—Letter of interest cosmology intertwined II: The hubble constant tension. Astropart. Phys. 2021, 131, 102605. [Google Scholar]
- Di Valentino, E.; Mena, O.; Pan, S.; Visinelli, L.; Yang, W.; Melchiorri, A.; Mota, D.F.; Riess, A.G.; Silk, J. In the realm of the Hubble tension—A review of solutions. Class. Quant. Grav. 2021, 38, 153001. [Google Scholar]
- Perivolaropoulos, L.; Skara, F. Challenges for ΛCDM: An update. New Astron. Rev. 2022, 95, 101659. [Google Scholar]
- Schöneberg, N.; Franco Abellán, G.; Pérez Sánchez, A.; Witte, S.J.; Poulin, V.; Lesgourgues, J. The H0 Olympics: A fair ranking of proposed models. Phys. Rept. 2022, 984, 1–55. [Google Scholar]
- Shah, P.; Lemos, P.; Lahav, O. A buyer’s guide to the Hubble constant. Astron. Astrophys. Rev. 2021, 29, 9. [Google Scholar]
- Abdalla, E.; Abellan, G.F.; Aboubrahim, A.; Agnello, A.; Akarsu, O.; Akrami, Y.; Alestas, G.; Aloni, D.; Amendola, L.; Anchordoqui, L.A.; et al. Cosmology intertwined: A review of the particle physics, astrophysics, and cosmology associated with the cosmological tensions and anomalies. JHEAp 2022, 34, 49–211. [Google Scholar]
- Di Valentino, E. Challenges of the Standard Cosmological Model. Universe 2022, 8, 399. [Google Scholar] [CrossRef]
- Hu, J.P.; Wang, F.Y. Hubble Tension: The Evidence of New Physics. Universe 2023, 9, 94. [Google Scholar]
- Efstathiou, G. A Lockdown Perspective on the Hubble Tension (with comments from the SH0ES team). arXiv 2020, arXiv:2007.10716. [Google Scholar]
- Mortsell, E.; Goobar, A.; Johansson, J.; Dhawan, S. Sensitivity of the Hubble Constant Determination to Cepheid Calibration. Astrophys. J. 2022, 933, 212. [Google Scholar]
- Mortsell, E.; Goobar, A.; Johansson, J.; Dhawan, S. The Hubble Tension Revisited: Additional Local Distance Ladder Uncertainties. Astrophys. J. 2022, 935, 58. [Google Scholar] [CrossRef]
- Freedman, W.L. Measurements of the Hubble Constant: Tensions in Perspective. Astrophys. J. 2021, 919, 16. [Google Scholar]
- Wojtak, R.; Hjorth, J. Intrinsic tension in the supernova sector of the local Hubble constant measurement and its implications. Mon. Not. R. Astron. Soc. 2022, 515, 2790–2799. [Google Scholar] [CrossRef]
- Berezhiani, Z.; Dolgov, A.D.; Tkachev, I.I. Reconciling Planck results with low redshift astronomical measurements. Phys. Rev. D 2015, 92, 61303. [Google Scholar] [CrossRef]
- Sola, J.; Gomez-Valent, A.; de Cruz Pérez, J. Hints of dynamical vacuum energy in the expanding Universe. Astrophys. J. Lett. 2015, 811, L14. [Google Scholar] [CrossRef]
- Solà, J.; Gómez-Valent, A.; de Cruz Pérez, J. First evidence of running cosmic vacuum: Challenging the concordance model. Astrophys. J. 2017, 836, 43. [Google Scholar] [CrossRef]
- Solà Peracaula, J.; de Cruz Pérez, J.; Gómez-Valent, A. Dynamical dark energy vs. Λ = const in light of observations. EPL 2018, 121, 39001. [Google Scholar] [CrossRef]
- Di Valentino, E.; Melchiorri, A.; Silk, J. Reconciling Planck with the local value of H0 in extended parameter space. Phys. Lett. B 2016, 761, 242–246. [Google Scholar] [CrossRef]
- Huang, Q.G.; Wang, K. How the dark energy can reconcile Planck with local determination of the Hubble constant. Eur. Phys. J. C 2016, 76, 506. [Google Scholar] [CrossRef]
- Tram, T.; Vallance, R.; Vennin, V. Inflation Model Selection meets Dark Radiation. JCAP 2017, 1, 46. [Google Scholar] [CrossRef]
- Ko, P.; Tang, Y. Light dark photon and fermionic dark radiation for the Hubble constant and the structure formation. Phys. Lett. B 2016, 762, 462–466. [Google Scholar] [CrossRef]
- Karwal, T.; Kamionkowski, M. Dark energy at early times, the Hubble parameter, and the string axiverse. Phys. Rev. D 2016, 94, 103523. [Google Scholar] [CrossRef]
- Kumar, S.; Nunes, R.C. Probing the interaction between dark matter and dark energy in the presence of massive neutrinos. Phys. Rev. D 2016, 94, 123511. [Google Scholar] [CrossRef]
- Xia, D.M.; Wang, S. Constraining interacting dark energy models with latest cosmological observations. Mon. Not. R. Astron. Soc. 2016, 463, 952–956. [Google Scholar] [CrossRef]
- Ko, P.; Tang, Y. Residual Non-Abelian Dark Matter and Dark Radiation. Phys. Lett. B 2017, 768, 12–17. [Google Scholar] [CrossRef]
- Chacko, Z.; Cui, Y.; Hong, S.; Okui, T.; Tsai, Y. Partially Acoustic Dark Matter, Interacting Dark Radiation, and Large Scale Structure. JHEP 2016, 12, 108. [Google Scholar] [CrossRef]
- Prilepina, V.; Tsai, Y. Reconciling Large And Small-Scale Structure In Twin Higgs Models. JHEP 2017, 9, 33. [Google Scholar] [CrossRef]
- Zhao, G.B.; Raveri, M.; Pogosian, L.; Wang, Y.T.; Crittenden, R.G.; Handley, W.J.; Percival, W.J.; Beutler, F.; Brinkmann, J.; Chuang, C.-H.; et al. Dynamical dark energy in light of the latest observations. Nat. Astron. 2017, 1, 627–632. [Google Scholar] [CrossRef]
- Vagnozzi, S.; Giusarma, E.; Mena, O.; Freese, K.; Gerbino, M.; Ho, S.; Lattanzi, M. Unveiling ν secrets with cosmological data: Neutrino masses and mass hierarchy. Phys. Rev. D 2017, 96, 123503. [Google Scholar] [CrossRef]
- Kumar, S.; Nunes, R.C. Echo of interactions in the dark sector. Phys. Rev. D 2017, 96, 103511. [Google Scholar] [CrossRef]
- Benetti, M.; Graef, L.L.; Alcaniz, J.S. Do joint CMB and HST data support a scale invariant spectrum? JCAP 2017, 4, 3. [Google Scholar] [CrossRef]
- Feng, L.; Zhang, J.F.; Zhang, X. A search for sterile neutrinos with the latest cosmological observations. Eur. Phys. J. C 2017, 77, 418. [Google Scholar] [CrossRef]
- Solà Peracaula, J.; de Cruz Pérez, J.; Gomez-Valent, A. Possible signals of vacuum dynamics in the Universe. Mon. Not. R. Astron. Soc. 2018, 478, 4357–4373. [Google Scholar] [CrossRef]
- Zhao, M.M.; He, D.Z.; Zhang, J.F.; Zhang, X. Search for sterile neutrinos in holographic dark energy cosmology: Reconciling Planck observation with the local measurement of the Hubble constant. Phys. Rev. D 2017, 96, 43520. [Google Scholar] [CrossRef]
- Di Valentino, E.; Melchiorri, A.; Linder, E.V.; Silk, J. Constraining Dark Energy Dynamics in Extended Parameter Space. Phys. Rev. D 2017, 96, 23523. [Google Scholar] [CrossRef]
- Dirian, Y. Changing the Bayesian prior: Absolute neutrino mass constraints in nonlocal gravity. Phys. Rev. D 2017, 96, 83513. [Google Scholar] [CrossRef]
- Di Valentino, E.; Melchiorri, A.; Mena, O. Can interacting dark energy solve the H0 tension? Phys. Rev. D 2017, 96, 43503. [Google Scholar] [CrossRef]
- Solà, J.; Gómez-Valent, A.; de Cruz Pérez, J. The H0 tension in light of vacuum dynamics in the Universe. Phys. Lett. B 2017, 774, 317–324. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Barrow, J.D. Large-scale Stability and Astronomical Constraints for Coupled Dark-Energy Models. Phys. Rev. D 2018, 97, 43529. [Google Scholar] [CrossRef]
- Feng, L.; Zhang, J.F.; Zhang, X. Searching for sterile neutrinos in dynamical dark energy cosmologies. Sci. China Phys. Mech. Astron. 2018, 61, 50411. [Google Scholar] [CrossRef]
- Renk, J.; Zumalacárregui, M.; Montanari, F.; Barreira, A. Galileon gravity in light of ISW, CMB, BAO and H0 data. JCAP 2017, 10, 20. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Paliathanasis, A. Latest astronomical constraints on some non-linear parametric dark energy models. Mon. Not. R. Astron. Soc. 2018, 475, 2605–2613. [Google Scholar] [CrossRef]
- Buen-Abad, M.A.; Schmaltz, M.; Lesgourgues, J.; Brinckmann, T. Interacting Dark Sector and Precision Cosmology. JCAP 2018, 1, 8. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Mota, D.F. Novel approach toward the large-scale stable interacting dark-energy models and their astronomical bounds. Phys. Rev. D 2017, 96, 123508. [Google Scholar] [CrossRef]
- Raveri, M.; Hu, W.; Hoffman, T.; Wang, L.T. Partially Acoustic Dark Matter Cosmology and Cosmological Constraints. Phys. Rev. D 2017, 96, 103501. [Google Scholar] [CrossRef]
- Solà, J.; Gómez-Valent, A.; de Cruz Pérez, J. Vacuum dynamics in the Universe versus a rigid Λ=const. Int. J. Mod. Phys. A 2017, 32, 1730014. [Google Scholar] [CrossRef]
- Di Valentino, E.; Linder, E.V.; Melchiorri, A. Vacuum phase transition solves the H0 tension. Phys. Rev. D 2018, 97, 43528. [Google Scholar] [CrossRef]
- Di Valentino, E.; Bøehm, C.; Hivon, E.; Bouchet, F.R. Reducing the H0 and σ8 tensions with Dark Matter-neutrino interactions. Phys. Rev. D 2018, 97, 43513. [Google Scholar] [CrossRef]
- Khosravi, N.; Baghram, S.; Afshordi, N.; Altamirano, N. H0 tension as a hint for a transition in gravitational theory. Phys. Rev. D 2019, 99, 103526. [Google Scholar] [CrossRef]
- Santos, M.A.; Benetti, M.; Alcaniz, J.; Brito, F.A.; Silva, R. CMB constraints on β-exponential inflationary models. JCAP 2018, 3, 23. [Google Scholar] [CrossRef]
- Nunes, R.C.; Bonilla, A. Probing the properties of relic neutrinos using the cosmic microwave background, the Hubble Space Telescope and galaxy clusters. Mon. Not. R. Astron. Soc. 2018, 473, 4404–4409. [Google Scholar] [CrossRef]
- Benetti, M.; Graef, L.L.; Alcaniz, J.S. The H0 and σ8 tensions and the scale invariant spectrum. JCAP 2018, 7, 66. [Google Scholar] [CrossRef]
- Binder, T.; Gustafsson, M.; Kamada, A.; Sandner, S.M.R.; Wiesner, M. Reannihilation of self-interacting dark matter. Phys. Rev. D 2018, 97, 123004. [Google Scholar] [CrossRef]
- Bolejko, K. Emerging spatial curvature can resolve the tension between high-redshift CMB and low-redshift distance ladder measurements of the Hubble constant. Phys. Rev. D 2018, 97, 103529. [Google Scholar] [CrossRef]
- Feng, L.; Zhang, J.F.; Zhang, X. Search for sterile neutrinos in a universe of vacuum energy interacting with cold dark matter. Phys. Dark Univ. 2019, 23, 100261. [Google Scholar] [CrossRef]
- Mörtsell, E.; Dhawan, S. Does the Hubble constant tension call for new physics? JCAP 2018, 9, 25. [Google Scholar] [CrossRef]
- Vagnozzi, S.; Dhawan, S.; Gerbino, M.; Freese, K.; Goobar, A.; Mena, O. Constraints on the sum of the neutrino masses in dynamical dark energy models with w(z) ≥ −1 are tighter than those obtained in ΛCDM. Phys. Rev. D 2018, 98, 83501. [Google Scholar] [CrossRef]
- Nunes, R.C. Structure formation in f(T) gravity and a solution for H0 tension. JCAP 2018, 5, 52. [Google Scholar] [CrossRef]
- Poulin, V.; Boddy, K.K.; Bird, S.; Kamionkowski, M. Implications of an extended dark energy cosmology with massive neutrinos for cosmological tensions. Phys. Rev. D 2018, 97, 123504. [Google Scholar] [CrossRef]
- Kumar, S.; Nunes, R.C.; Yadav, S.K. Cosmological bounds on dark matter-photon coupling. Phys. Rev. D 2018, 98, 43521. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Xu, L.; Mota, D.F. Effects of anisotropic stress in interacting dark matter–Dark energy scenarios. Mon. Not. R. Astron. Soc. 2019, 482, 1858–1871. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Alam, U.; Pandey, K.L.; Das, S.; Pal, S. Are H0 and σ8 tensions generic to present cosmological data? Astrophys. J. 2019, 876, 143. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Di Valentino, E.; Nunes, R.C.; Vagnozzi, S.; Mota, D.F. Tale of stable interacting dark energy, observational signatures, and the H0 tension. JCAP 2018, 9, 19. [Google Scholar] [CrossRef]
- Poulin, V.; Smith, T.L.; Grin, D.; Karwal, T.; Kamionkowski, M. Cosmological implications of ultralight axionlike fields. Phys. Rev. D 2018, 98, 83525. [Google Scholar] [CrossRef]
- Akarsu, O.; Katirci, N.; Kumar, S.; Nunes, R.C.; Sami, M. Cosmological implications of scale-independent energy-momentum squared gravity: Pseudo nonminimal interactions in dark matter and relativistic relics. Phys. Rev. D 2018, 98, 63522. [Google Scholar] [CrossRef]
- Ócolgáin, E.; van Putten, M.H.P.M.; Yavartanoo, H. de Sitter Swampland, H0 tension & observation. Phys. Lett. B 2019, 793, 126–129. [Google Scholar]
- Yang, W.; Pan, S.; Herrera, R.; Chakraborty, S. Large-scale (in) stability analysis of an exactly solved coupled dark-energy model. Phys. Rev. D 2018, 98, 43517. [Google Scholar] [CrossRef]
- Banihashemi, A.; Khosravi, N.; Shirazi, A.H. Phase transition in the dark sector as a proposal to lessen cosmological tensions. Phys. Rev. D 2020, 101, 123521. [Google Scholar] [CrossRef]
- Dutta, K.; Ruchika; Roy, A.; Sen, A.A.; Sheikh-Jabbari, M.M. Beyond ΛCDM with low and high redshift data: Implications for dark energy. Gen. Rel. Grav. 2020, 52, 15. [Google Scholar] [CrossRef]
- D’Eramo, F.; Ferreira, R.Z.; Notari, A.; Bernal, J.L. Hot Axions and the H0 tension. JCAP 2018, 11, 14. [Google Scholar] [CrossRef]
- Guo, R.Y.; Zhang, J.F.; Zhang, X. Can the H0 tension be resolved in extensions to ΛCDM cosmology? JCAP 2019, 2, 54. [Google Scholar] [CrossRef]
- Graef, L.L.; Benetti, M.; Alcaniz, J.S. Primordial gravitational waves and the H0-tension problem. Phys. Rev. D 2019, 99, 43519. [Google Scholar] [CrossRef]
- Visinelli, L.; Vagnozzi, S. Cosmological window onto the string axiverse and the supersymmetry breaking scale. Phys. Rev. D 2019, 99, 63517. [Google Scholar] [CrossRef]
- Yang, W.; Mukherjee, A.; Di Valentino, E.; Pan, S. Interacting dark energy with time varying equation of state and the H0 tension. Phys. Rev. D 2018, 98, 123527. [Google Scholar] [CrossRef]
- El-Zant, A.; El Hanafy, W.; Elgammal, S. H0 Tension and the Phantom Regime: A Case Study in Terms of an Infrared f(T) Gravity. Astrophys. J. 2019, 871, 210. [Google Scholar] [CrossRef]
- Lin, M.X.; Raveri, M.; Hu, W. Phenomenology of Modified Gravity at Recombination. Phys. Rev. D 2019, 99, 43514. [Google Scholar] [CrossRef]
- Bengaly, C.A.P.; Andrade, U.; Alcaniz, J.S. How does an incomplete sky coverage affect the Hubble Constant variance? Eur. Phys. J. C 2019, 79, 768. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Di Valentino, E.; Saridakis, E.N.; Chakraborty, S. Observational constraints on one-parameter dynamical dark-energy parametrizations and the H0 tension. Phys. Rev. D 2019, 99, 43543. [Google Scholar] [CrossRef]
- Bonilla, A.; Nunes, R.C.; Abreu, E.M.C. Forecast on lepton asymmetry from future CMB experiments. Mon. Not. R. Astron. Soc. 2019, 485, 2486–2491. [Google Scholar] [CrossRef]
- Yang, W.; Shahalam, M.; Pal, B.; Pan, S.; Wang, A. Constraints on quintessence scalar field models using cosmological observations. Phys. Rev. D 2019, 100, 23522. [Google Scholar] [CrossRef]
- Banihashemi, A.; Khosravi, N.; Shirazi, A.H. Ginzburg-Landau Theory of Dark Energy: A Framework to Study Both Temporal and Spatial Cosmological Tensions Simultaneously. Phys. Rev. D 2019, 99, 83509. [Google Scholar] [CrossRef]
- Sola Peracaula, J.; Gomez-Valent, A.; de Cruz Pérez, J. Signs of Dynamical Dark Energy in Current Observations. Phys. Dark Univ. 2019, 25, 100311. [Google Scholar] [CrossRef]
- Poulin, V.; Smith, T.L.; Karwal, T.; Kamionkowski, M. Early Dark Energy Can Resolve The Hubble Tension. Phys. Rev. Lett. 2019, 122, 221301. [Google Scholar] [CrossRef] [PubMed]
- Farrugia, C.R.; Sultana, J.; Mifsud, J. Endowing Λ with a dynamic nature: Constraints in a spatially curved universe. Phys. Rev. D 2020, 102, 24013. [Google Scholar] [CrossRef]
- Carneiro, S.; de Holanda, P.C.; Pigozzo, C.; Sobreira, F. Is the H0 tension suggesting a fourth neutrino generation? Phys. Rev. D 2019, 100, 23505. [Google Scholar] [CrossRef]
- Kumar, S.; Nunes, R.C.; Yadav, S.K. Testing the warmness of dark matter. Mon. Not. R. Astron. Soc. 2019, 490, 1406–1414. [Google Scholar] [CrossRef]
- Kenworthy, W.D.; Scolnic, D.; Riess, A. The Local Perspective on the Hubble Tension: Local Structure Does Not Impact Measurement of the Hubble Constant. Astrophys. J. 2019, 875, 145. [Google Scholar] [CrossRef]
- Kreisch, C.D.; Cyr-Racine, F.Y.; Doré, O. Neutrino puzzle: Anomalies, interactions, and cosmological tensions. Phys. Rev. D 2020, 101, 123505. [Google Scholar] [CrossRef]
- Pandey, K.L.; Karwal, T.; Das, S. Alleviating the H0 and σ8 anomalies with a decaying dark matter model. JCAP 2020, 7, 26. [Google Scholar] [CrossRef]
- Martinelli, M.; Hogg, N.B.; Peirone, S.; Bruni, M.; Wands, D. Constraints on the interacting vacuum–geodesic CDM scenario. Mon. Not. R. Astron. Soc. 2019, 488, 3423–3438. [Google Scholar] [CrossRef]
- Kumar, S.; Nunes, R.C.; Yadav, S.K. Dark sector interaction: A remedy of the tensions between CMB and LSS data. Eur. Phys. J. C 2019, 79, 576. [Google Scholar] [CrossRef]
- Vattis, K.; Koushiappas, S.M.; Loeb, A. Dark matter decaying in the late Universe can relieve the H0 tension. Phys. Rev. D 2019, 99, 121302. [Google Scholar] [CrossRef]
- Pan, S.; Yang, W.; Singha, C.; Saridakis, E.N. Observational constraints on sign-changeable interaction models and alleviation of the H0 tension. Phys. Rev. D 2019, 100, 83539. [Google Scholar] [CrossRef]
- Akarsu, O.; Barrow, J.D.; Board, C.V.R.; Uzun, N.M.; Vazquez, J.A. Screening Λ in a new modified gravity model. Eur. Phys. J. C 2019, 79, 846. [Google Scholar] [CrossRef]
- Li, X.; Shafieloo, A.; Sahni, V.; Starobinsky, A.A. Revisiting Metastable Dark Energy and Tensions in the Estimation of Cosmological Parameters. Astrophys. J. 2019, 887, 153. [Google Scholar] [CrossRef]
- Alexander, S.; McDonough, E. Axion-Dilaton Destabilization and the Hubble Tension. Phys. Lett. B 2019, 797, 134830. [Google Scholar] [CrossRef]
- Agrawal, P.; Cyr-Racine, F.Y.; Pinner, D.; Randall, L. Rock ’n’ Roll Solutions to the Hubble Tension. arXiv 2019, arXiv:1904.01016. [Google Scholar]
- Yang, W.; Pan, S.; Paliathanasis, A.; Ghosh, S.; Wu, Y. Observational constraints of a new unified dark fluid and the H0 tension. Mon. Not. R. Astron. Soc. 2019, 490, 2071–2085. [Google Scholar] [CrossRef]
- Hooper, D.; Krnjaic, G.; McDermott, S.D. Dark Radiation and Superheavy Dark Matter from Black Hole Domination. JHEP 2019, 8, 1. [Google Scholar] [CrossRef]
- Adhikari, S.; Huterer, D. Super-CMB fluctuations and the Hubble tension. Phys. Dark Univ. 2020, 28, 100539. [Google Scholar] [CrossRef]
- Colgáin, E.O.; Yavartanoo, H. Testing the Swampland: H0 tension. Phys. Lett. B 2019, 797, 134907. [Google Scholar] [CrossRef]
- Blinov, N.; Kelly, K.J.; Krnjaic, G.Z.; McDermott, S.D. Constraining the Self-Interacting Neutrino Interpretation of the Hubble Tension. Phys. Rev. Lett. 2019, 123, 191102. [Google Scholar] [CrossRef]
- Keeley, R.E.; Joudaki, S.; Kaplinghat, M.; Kirkby, D. Implications of a transition in the dark energy equation of state for the H0 and σ8 tensions. JCAP 2019, 12, 35. [Google Scholar] [CrossRef]
- Lin, M.X.; Benevento, G.; Hu, W.; Raveri, M. Acoustic Dark Energy: Potential Conversion of the Hubble Tension. Phys. Rev. D 2019, 100, 63542. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Di Valentino, E.; Paliathanasis, A.; Lu, J. Challenging bulk viscous unified scenarios with cosmological observations. Phys. Rev. D 2019, 100, 103518. [Google Scholar] [CrossRef]
- Agrawal, P.; Obied, G.; Vafa, C. H0 tension, swampland conjectures, and the epoch of fading dark matter. Phys. Rev. D 2021, 103, 43523. [Google Scholar] [CrossRef]
- Li, X.; Shafieloo, A. A Simple Phenomenological Emergent Dark Energy Model can Resolve the Hubble Tension. Astrophys. J. Lett. 2019, 883, L3. [Google Scholar] [CrossRef]
- Martinelli, M.; Tutusaus, I. CMB tensions with low-redshift H0 and S8 measurements: Impact of a redshift-dependent type-Ia supernovae intrinsic luminosity. Symmetry 2019, 11, 986. [Google Scholar] [CrossRef]
- Gelmini, G.B.; Kusenko, A.; Takhistov, V. Possible Hints of Sterile Neutrinos in Recent Measurements of the Hubble Parameter. JCAP 2021, 6, 2. [Google Scholar] [CrossRef]
- Rossi, M.; Ballardini, M.; Braglia, M.; Finelli, F.; Paoletti, D.; Starobinsky, A.A.; Umiltà, C. Cosmological constraints on post-Newtonian parameters in effectively massless scalar-tensor theories of gravity. Phys. Rev. D 2019, 100, 103524. [Google Scholar] [CrossRef]
- Di Valentino, E.; Ferreira, R.Z.; Visinelli, L.; Danielsson, U. Late time transitions in the quintessence field and the H0 tension. Phys. Dark Univ. 2019, 26, 100385. [Google Scholar] [CrossRef]
- Yang, W.; Mena, O.; Pan, S.; Di Valentino, E. Dark sectors with dynamical coupling. Phys. Rev. D 2019, 100, 83509. [Google Scholar] [CrossRef]
- Archidiacono, M.; Hooper, D.C.; Murgia, R.; Bohr, S.; Lesgourgues, J.; Viel, M. Constraining Dark Matter-Dark Radiation interactions with CMB, BAO, and Lyman-α. JCAP 2019, 10, 55. [Google Scholar] [CrossRef]
- Desmond, H.; Jain, B.; Sakstein, J. Local resolution of the Hubble tension: The impact of screened fifth forces on the cosmic distance ladder. Phys. Rev. D 2019, 100, 43537, Erratum in Phys. Rev. D 2020, 101, 069904, Erratum in Phys. Rev. D 2020, 101, 129901. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Vagnozzi, S.; Di Valentino, E.; Mota, D.F.; Capozziello, S. Dawn of the dark: Unified dark sectors and the EDGES Cosmic Dawn 21-cm signal. JCAP 2019, 11, 44. [Google Scholar] [CrossRef]
- Nesseris, S.; Sapone, D.; Sypsas, S. Evaporating primordial black holes as varying dark energy. Phys. Dark Univ. 2020, 27, 100413. [Google Scholar] [CrossRef]
- Pan, S.; Yang, W.; Di Valentino, E.; Saridakis, E.N.; Chakraborty, S. Interacting scenarios with dynamical dark energy: Observational constraints and alleviation of the H0 tension. Phys. Rev. D 2019, 100, 103520. [Google Scholar] [CrossRef]
- Vagnozzi, S. New physics in light of the H0 tension: An alternative view. Phys. Rev. D 2020, 102, 23518. [Google Scholar] [CrossRef]
- Visinelli, L.; Vagnozzi, S.; Danielsson, U. Revisiting a negative cosmological constant from low-redshift data. Symmetry 2019, 11, 1035. [Google Scholar] [CrossRef]
- Hardy, E.; Parameswaran, S. Thermal Dark Energy. Phys. Rev. D 2020, 101, 23503. [Google Scholar] [CrossRef]
- Cai, Y.F.; Khurshudyan, M.; Saridakis, E.N. Model-independent reconstruction of f(T) gravity from Gaussian Processes. Astrophys. J. 2020, 888, 62. [Google Scholar] [CrossRef]
- Pan, S.; Yang, W.; Di Valentino, E.; Shafieloo, A.; Chakraborty, S. Reconciling H0 tension in a six parameter space? JCAP 2020, 6, 62. [Google Scholar] [CrossRef]
- Di Valentino, E.; Melchiorri, A.; Silk, J. Cosmological constraints in extended parameter space from the Planck 2018 Legacy release. JCAP 2020, 1, 13. [Google Scholar] [CrossRef]
- Xiao, L.; Zhang, L.; An, R.; Feng, C.; Wang, B. Fractional Dark Matter decay: Cosmological imprints and observational constraints. JCAP 2020, 1, 45. [Google Scholar] [CrossRef]
- Panpanich, S.; Burikham, P.; Ponglertsakul, S.; Tannukij, L. Resolving Hubble Tension with Quintom Dark Energy Model. Chin. Phys. C 2021, 45, 15108. [Google Scholar] [CrossRef]
- Di Valentino, E.; Melchiorri, A.; Mena, O.; Vagnozzi, S. Interacting dark energy in the early 2020s: A promising solution to the H0 and cosmic shear tensions. Phys. Dark Univ. 2020, 30, 100666. [Google Scholar] [CrossRef]
- Benetti, M.; Miranda, W.; Borges, H.A.; Pigozzo, C.; Carneiro, S.; Alcaniz, J.S. Looking for interactions in the cosmological dark sector. JCAP 2019, 12, 23. [Google Scholar] [CrossRef]
- Dutta, K.; Roy, A.; Ruchika; Sen, A.A.; Sheikh-Jabbari, M.M. Cosmology with low-redshift observations: No signal for new physics. Phys. Rev. D 2019, 100, 103501. [Google Scholar] [CrossRef]
- Ghosh, S.; Khatri, R.; Roy, T.S. Can dark neutrino interactions phase out the Hubble tension? Phys. Rev. D 2020, 102, 123544. [Google Scholar] [CrossRef]
- Solà Peracaula, J.; Gomez-Valent, A.; de Cruz Pérez, J.; Moreno-Pulido, C. Brans–Dicke Gravity with a Cosmological Constant Smoothes Out ΛCDM Tensions. Astrophys. J. Lett. 2019, 886, L6. [Google Scholar] [CrossRef]
- Escudero, M.; Witte, S.J. A CMB search for the neutrino mass mechanism and its relation to the Hubble tension. Eur. Phys. J. C 2020, 80, 294. [Google Scholar] [CrossRef]
- Yan, S.F.; Zhang, P.; Chen, J.W.; Zhang, X.Z.; Cai, Y.F.; Saridakis, E.N. Interpreting cosmological tensions from the effective field theory of torsional gravity. Phys. Rev. D 2020, 101, 121301. [Google Scholar] [CrossRef]
- Banerjee, S.; Benisty, D.; Guendelman, E.I. Running Dark Energy and Dark Matter from Dynamical Spacetime. Bulg. J. Phys. 2021, 48, 117–137. [Google Scholar]
- Liu, M.; Huang, Z. Band-limited Features in the Primordial Power Spectrum Do Not Resolve the Hubble Tension. Astrophys. J. 2020, 897, 166. [Google Scholar] [CrossRef]
- Anchordoqui, L.A.; Perez Bergliaffa, S.E. Hot thermal universe endowed with massive dark vector fields and the Hubble tension. Phys. Rev. D 2019, 100, 123525. [Google Scholar] [CrossRef]
- Lunardini, C.; Perez-Gonzalez, Y.F. Dirac and Majorana neutrino signatures of primordial black holes. JCAP 2020, 8, 14. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Nunes, R.C.; Mota, D.F. Dark calling Dark: Interaction in the dark sector in presence of neutrino properties after Planck CMB final release. JCAP 2020, 4, 8. [Google Scholar] [CrossRef]
- Di Valentino, E.; Melchiorri, A.; Mena, O.; Vagnozzi, S. Nonminimal dark sector physics and cosmological tensions. Phys. Rev. D 2020, 101, 63502. [Google Scholar] [CrossRef]
- Niedermann, F.; Sloth, M.S. New early dark energy. Phys. Rev. D 2021, 103, L041303. [Google Scholar] [CrossRef]
- Cheng, G.; Ma, Y.Z.; Wu, F.; Zhang, J.; Chen, X. Testing interacting dark matter and dark energy model with cosmological data. Phys. Rev. D 2020, 102, 43517. [Google Scholar] [CrossRef]
- Berghaus, K.V.; Karwal, T. Thermal Friction as a Solution to the Hubble Tension. Phys. Rev. D 2020, 101, 83537. [Google Scholar] [CrossRef]
- Sakstein, J.; Trodden, M. Early Dark Energy from Massive Neutrinos as a Natural Resolution of the Hubble Tension. Phys. Rev. Lett. 2020, 124, 161301. [Google Scholar] [CrossRef]
- Liu, M.; Huang, Z.; Luo, X.; Miao, H.; Singh, N.K.; Huang, L. Can Non-standard Recombination Resolve the Hubble Tension? Sci. China Phys. Mech. Astron. 2020, 63, 290405. [Google Scholar] [CrossRef]
- Anchordoqui, L.A.; Antoniadis, I.; Lüst, D.; Soriano, J.F.; Taylor, T.R. H0 tension and the String Swampland. Phys. Rev. D 2020, 101, 83532. [Google Scholar] [CrossRef]
- Hart, L.; Chluba, J. Updated fundamental constant constraints from Planck 2018 data and possible relations to the Hubble tension. Mon. Not. R. Astron. Soc. 2020, 493, 3255–3263. [Google Scholar] [CrossRef]
- Wang, K.; Huang, Q.G. Implications for cosmology from Ground-based Cosmic Microwave Background observations. JCAP 2020, 6, 45. [Google Scholar] [CrossRef]
- Alcaniz, J.; Bernal, N.; Masiero, A.; Queiroz, F.S. Light dark matter: A common solution to the lithium and H 0 problems. Phys. Lett. B 2021, 812, 136008. [Google Scholar] [CrossRef]
- Gehrlein, J.; Pierre, M. A testable hidden-sector model for Dark Matter and neutrino masses. JHEP 2020, 2, 68. [Google Scholar] [CrossRef]
- Akarsu, O.; Barrow, J.D.; Escamilla, L.A.; Vazquez, J.A. Graduated dark energy: Observational hints of a spontaneous sign switch in the cosmological constant. Phys. Rev. D 2020, 101, 63528. [Google Scholar] [CrossRef]
- Mazo, B.Y.D.V.; Romano, A.E.; Quintero, M.A.C. Combining gravitational and electromagnetic waves observations to investigate local structure and the Hubble tension. arXiv 2019, arXiv:1912.12465. [Google Scholar]
- Ding, Q.; Nakama, T.; Wang, Y. A gigaparsec-scale local void and the Hubble tension. Sci. China Phys. Mech. Astron. 2020, 63, 290403. [Google Scholar] [CrossRef]
- Ye, G.; Piao, Y.S. Is the Hubble tension a hint of AdS phase around recombination? Phys. Rev. D 2020, 101, 83507. [Google Scholar] [CrossRef]
- Pan, S.; Sharov, G.S.; Yang, W. Field theoretic interpretations of interacting dark energy scenarios and recent observations. Phys. Rev. D 2020, 101, 103533. [Google Scholar] [CrossRef]
- Amirhashchi, H.; Yadav, A.K.; Ahmad, N.; Yadav, V. Interacting dark sectors in anisotropic universe: Observational constraints and H0 tension. Phys. Dark Univ. 2022, 36, 101043. [Google Scholar] [CrossRef]
- Yang, W.; Di Valentino, E.; Pan, S.; Basilakos, S.; Paliathanasis, A. Metastable dark energy models in light of Planck 2018 data: Alleviating the H0 tension. Phys. Rev. D 2020, 102, 63503. [Google Scholar] [CrossRef]
- Li, X.; Shafieloo, A. Evidence for Emergent Dark Energy. Astrophys. J. 2020, 902, 58. [Google Scholar] [CrossRef]
- Beradze, R.; Gogberashvili, M. Can the quasi-molecular mechanism of recombination decrease the Hubble tension? Phys. Dark Univ. 2021, 32, 100841. [Google Scholar] [CrossRef]
- Perez, A.; Sudarsky, D.; Wilson-Ewing, E. Resolving the H0 tension with diffusion. Gen. Rel. Grav. 2021, 53, 7. [Google Scholar] [CrossRef]
- Lyu, M.Z.; Haridasu, B.S.; Viel, M.; Xia, J.Q. H0 Reconstruction with Type Ia Supernovae, Baryon Acoustic Oscillation and Gravitational Lensing Time-Delay. Astrophys. J. 2020, 900, 160. [Google Scholar] [CrossRef]
- Yang, W.; Di Valentino, E.; Mena, O.; Pan, S.; Nunes, R.C. All-inclusive interacting dark sector cosmologies. Phys. Rev. D 2020, 101, 83509. [Google Scholar] [CrossRef]
- Choi, G.; Suzuki, M.; Yanagida, T.T. Degenerate Sub-keV Fermion Dark Matter from a Solution to the Hubble Tension. Phys. Rev. D 2020, 101, 75031. [Google Scholar] [CrossRef]
- Dutta, B.; Ghosh, S.; Kumar, J. Contributions to ΔNeff from the dark photon of U(1)T3R. Phys. Rev. D 2020, 102, 15013. [Google Scholar] [CrossRef]
- Pan, S.; Yang, W.; Paliathanasis, A. Non-linear interacting cosmological models after Planck 2018 legacy release and the H0 tension. Mon. Not. R. Astron. Soc. 2020, 493, 3114–3131. [Google Scholar] [CrossRef]
- Sharov, G.S.; Sinyakov, E.S. Cosmological models, observational data and tension in Hubble constant. arXiv 2020, arXiv:2002.03599. [Google Scholar] [CrossRef]
- Lucca, M.; Hooper, D.C. Shedding light on dark matter-dark energy interactions. Phys. Rev. D 2020, 102, 123502. [Google Scholar] [CrossRef]
- D’Agostino, R.; Nunes, R.C. Measurements of H0 in modified gravity theories: The role of lensed quasars in the late-time Universe. Phys. Rev. D 2020, 101, 103505. [Google Scholar] [CrossRef]
- Hogg, N.B.; Bruni, M.; Crittenden, R.; Martinelli, M.; Peirone, S. Latest evidence for a late time vacuum–geodesic CDM interaction. Phys. Dark Univ. 2020, 29, 100583. [Google Scholar] [CrossRef]
- Benevento, G.; Hu, W.; Raveri, M. Can Late Dark Energy Transitions Raise the Hubble constant? Phys. Rev. D 2020, 101, 103517. [Google Scholar] [CrossRef]
- Barker, W.E.V.; Lasenby, A.N.; Hobson, M.P.; Handley, W.J. Systematic study of background cosmology in unitary Poincaré gauge theories with application to emergent dark radiation and H0 tension. Phys. Rev. D 2020, 102, 24048. [Google Scholar] [CrossRef]
- Zumalacarregui, M. Gravity in the Era of Equality: Towards solutions to the Hubble problem without fine-tuned initial conditions. Phys. Rev. D 2020, 102, 23523. [Google Scholar] [CrossRef]
- Hill, J.C.; McDonough, E.; Toomey, M.W.; Alexander, S. Early dark energy does not restore cosmological concordance. Phys. Rev. D 2020, 102, 43507. [Google Scholar] [CrossRef]
- Desmond, H.; Sakstein, J. Screened fifth forces lower the TRGB-calibrated Hubble constant too. Phys. Rev. D 2020, 102, 23007. [Google Scholar] [CrossRef]
- Benisty, D.; Guendemlan, E.I.; Nissimov, E.; Pacheva, S. ΛCDM as a Noether Symmetry in Cosmology. Int. J. Mod. Phys. D 2020, 26, 2050104. [Google Scholar] [CrossRef]
- Gómez-Valent, A.; Pettorino, V.; Amendola, L. Update on coupled dark energy and the H0 tension. Phys. Rev. D 2020, 101, 123513. [Google Scholar] [CrossRef]
- Akarsu, O.; Katırcı, N.; Kumar, S.; Nunes, R.C.; Öztürk, B.; Sharma, S. Rastall gravity extension of the standard ΛCDM model: Theoretical features and observational constraints. Eur. Phys. J. C 2020, 80, 1050. [Google Scholar] [CrossRef]
- Ballesteros, G.; Notari, A.; Rompineve, F. The H0 tension: ΔGN vs. ΔNeff. JCAP 2020, 11, 24. [Google Scholar] [CrossRef]
- Blinov, N.; Keith, C.; Hooper, D. Warm Decaying Dark Matter and the Hubble Tension. JCAP 2020, 6, 5. [Google Scholar] [CrossRef]
- Haridasu, B.S.; Viel, M. Late-time decaying dark matter: Constraints and implications for the H0-tension. Mon. Not. R. Astron. Soc. 2020, 497, 1757–1764. [Google Scholar] [CrossRef]
- Alestas, G.; Kazantzidis, L.; Perivolaropoulos, L. H0 tension, phantom dark energy, and cosmological parameter degeneracies. Phys. Rev. D 2020, 101, 123516. [Google Scholar] [CrossRef]
- Jedamzik, K.; Pogosian, L. Relieving the Hubble tension with primordial magnetic fields. Phys. Rev. Lett. 2020, 125, 181302. [Google Scholar] [CrossRef] [PubMed]
- Braglia, M.; Ballardini, M.; Emond, W.T.; Finelli, F.; Gumrukcuoglu, A.E.; Koyama, K.; Paoletti, D. Larger value for H0 by an evolving gravitational constant. Phys. Rev. D 2020, 102, 23529. [Google Scholar] [CrossRef]
- Chudaykin, A.; Gorbunov, D.; Nedelko, N. Combined analysis of Planck and SPTPol data favors the early dark energy models. JCAP 2020, 8, 13. [Google Scholar] [CrossRef]
- Izaurieta, F.; Lepe, S.; Valdivia, O. The Spin Tensor of Dark Matter and the Hubble Parameter Tension. Phys. Dark Univ. 2020, 30, 100662. [Google Scholar] [CrossRef]
- Ballardini, M.; Braglia, M.; Finelli, F.; Paoletti, D.; Starobinsky, A.A.; Umiltà, C. Scalar-tensor theories of gravity, neutrino physics, and the H0 tension. JCAP 2020, 10, 44. [Google Scholar] [CrossRef]
- Di Valentino, E.; Gariazzo, S.; Mena, O.; Vagnozzi, S. Soundness of Dark Energy properties. JCAP 2020, 7, 45. [Google Scholar] [CrossRef]
- Ivanov, M.M.; Ali-Haïmoud, Y.; Lesgourgues, J. H0 tension or T0 tension? Phys. Rev. D 2020, 102, 63515. [Google Scholar] [CrossRef]
- Gogoi, A.; Sharma, R.K.; Chanda, P.; Das, S. Early Mass-varying Neutrino Dark Energy: Nugget Formation and Hubble Anomaly. Astrophys. J. 2021, 915, 132. [Google Scholar] [CrossRef]
- Di Valentino, E.; Mukherjee, A.; Sen, A.A. Dark Energy with Phantom Crossing and the H0 Tension. Entropy 2021, 23, 404. [Google Scholar] [CrossRef] [PubMed]
- Banerjee, A.; Cai, H.; Heisenberg, L.; Colgáin, E.O.; Sheikh-Jabbari, M.M.; Yang, T. Hubble sinks in the low-redshift swampland. Phys. Rev. D 2021, 103, L081305. [Google Scholar] [CrossRef]
- Elizalde, E.; Khurshudyan, M.; Odintsov, S.D.; Myrzakulov, R. Analysis of the H0 tension problem in the Universe with viscous dark fluid. Phys. Rev. D 2020, 102, 123501. [Google Scholar] [CrossRef]
- Clark, S.J.; Vattis, K.; Koushiappas, S.M. Cosmological constraints on late-universe decaying dark matter as a solution to the H0 tension. Phys. Rev. D 2021, 103, 43014. [Google Scholar] [CrossRef]
- Solà Peracaula, J.; Gómez-Valent, A.; de Cruz Pérez, J.; Moreno-Pulido, C. Brans–Dicke cosmology with a Λ-term: A possible solution to ΛCDM tensions. Class. Quant. Grav. 2020, 37, 245003. [Google Scholar] [CrossRef]
- Niedermann, F.; Sloth, M.S. Resolving the Hubble tension with new early dark energy. Phys. Rev. D 2020, 102, 63527. [Google Scholar] [CrossRef]
- Ivanov, M.M.; McDonough, E.; Hill, J.C.; Simonović, M.; Toomey, M.W.; Alexander, S.; Zaldarriaga, M. Constraining Early Dark Energy with Large-Scale Structure. Phys. Rev. D 2020, 102, 103502. [Google Scholar] [CrossRef]
- D’Amico, G.; Senatore, L.; Zhang, P.; Zheng, H. The Hubble Tension in Light of the Full-Shape Analysis of Large-Scale Structure Data. JCAP 2021, 5, 72. [Google Scholar] [CrossRef]
- Elizalde, E.; Khurshudyan, M. Constraints on cosmic opacity from Bayesian machine learning: The hidden side of the H0 tension problem. Phys. Dark Univ. 2022, 37, 101114. [Google Scholar] [CrossRef]
- Di Valentino, E.; Linder, E.V.; Melchiorri, A. H0 ex machina: Vacuum metamorphosis and beyond H0. Phys. Dark Univ. 2020, 30, 100733. [Google Scholar] [CrossRef]
- Gonzalez, M.; Hertzberg, M.P.; Rompineve, F. Ultralight Scalar Decay and the Hubble Tension. JCAP 2020, 10, 28. [Google Scholar] [CrossRef]
- Capozziello, S.; Benetti, M.; Spallicci, A.D.A.M. Addressing the cosmological H0 tension by the Heisenberg uncertainty. Found. Phys. 2020, 50, 893–899. [Google Scholar] [CrossRef]
- Yang, W.; Di Valentino, E.; Pan, S.; Mena, O. Emergent Dark Energy, neutrinos and cosmological tensions. Phys. Dark Univ. 2021, 31, 100762. [Google Scholar] [CrossRef]
- Sekiguchi, T.; Takahashi, T. Early recombination as a solution to the H0 tension. Phys. Rev. D 2021, 103, 83507. [Google Scholar] [CrossRef]
- Nunes, R.C.; Bernui, A. BAO signatures in the 2-point angular correlations and the Hubble tension. Eur. Phys. J. C 2020, 80, 1025. [Google Scholar] [CrossRef]
- Berechya, D.; Leonhardt, U. Lifshitz cosmology: Quantum vacuum and Hubble tension. Mon. Not. R. Astron. Soc. 2021, 507, 3473–3485. [Google Scholar] [CrossRef]
- Benaoum, H.B.; Yang, W.; Pan, S.; Di Valentino, E. Modified emergent dark energy and its astronomical constraints. Int. J. Mod. Phys. D 2022, 31, 2250015. [Google Scholar] [CrossRef]
- Calderón, R.; Gannouji, R.; L’Huillier, B.; Polarski, D. Negative cosmological constant in the dark sector? Phys. Rev. D 2021, 103, 23526. [Google Scholar] [CrossRef]
- Ye, G.; Piao, Y.S. T0 censorship of early dark energy and AdS vacua. Phys. Rev. D 2020, 102, 83523. [Google Scholar] [CrossRef]
- Flores, M.M.; Kusenko, A. Primordial Black Holes from Long-Range Scalar Forces and Scalar Radiative Cooling. Phys. Rev. Lett. 2021, 126, 41101. [Google Scholar] [CrossRef]
- Niedermann, F.; Sloth, M.S. New Early Dark Energy is compatible with current LSS data. Phys. Rev. D 2021, 103, 103537. [Google Scholar] [CrossRef]
- Vázquez, J.A.; Tamayo, D.; Sen, A.A.; Quiros, I. Bayesian model selection on scalar ϵ-field dark energy. Phys. Rev. D 2021, 103, 43506. [Google Scholar] [CrossRef]
- Akarsu, O.; Barrow, J.D.; Uzun, N.M. Screening anisotropy via energy-momentum squared gravity: ΛCDM model with hidden anisotropy. Phys. Rev. D 2020, 102, 124059. [Google Scholar] [CrossRef]
- Pogosian, L.; Zhao, G.B.; Jedamzik, K. Recombination-independent determination of the sound horizon and the Hubble constant from BAO. Astrophys. J. Lett. 2020, 904, L17. [Google Scholar] [CrossRef]
- Smith, T.L.; Poulin, V.; Bernal, J.L.; Boddy, K.K.; Kamionkowski, M.; Murgia, R. Early dark energy is not excluded by current large-scale structure data. Phys. Rev. D 2021, 103, 123542. [Google Scholar] [CrossRef]
- Murgia, R.; Abellán, G.F.; Poulin, V. Early dark energy resolution to the Hubble tension in light of weak lensing surveys and lensing anomalies. Phys. Rev. D 2021, 103, 63502. [Google Scholar] [CrossRef]
- Vagnozzi, S.; Di Valentino, E.; Gariazzo, S.; Melchiorri, A.; Mena, O.; Silk, J. The galaxy power spectrum take on spatial curvature and cosmic concordance. Phys. Dark Univ. 2021, 33, 100851. [Google Scholar] [CrossRef]
- Anchordoqui, L.A. Decaying dark matter, the H0 tension, and the lithium problem. Phys. Rev. D 2021, 103, 35025. [Google Scholar] [CrossRef]
- Hashim, M.; El Hanafy, W.; Golovnev, A.; El-Zant, A.A. Toward a concordance teleparallel cosmology. Part I. Background dynamics. JCAP 2021, 7, 52. [Google Scholar] [CrossRef]
- Di Valentino, E. A combined analysis of the H0 late time direct measurements and the impact on the Dark Energy sector. Mon. Not. R. Astron. Soc. 2021, 502, 2065–2073. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Sáez-Chillón Gómez, D.; Sharov, G.S. Analyzing the H0 tension in F(R) gravity models. Nucl. Phys. B 2021, 966, 115377. [Google Scholar] [CrossRef]
- Carrillo González, M.; Liang, Q.; Sakstein, J.; Trodden, M. Neutrino-Assisted Early Dark Energy: Theory and Cosmology. JCAP 2021, 4, 63. [Google Scholar] [CrossRef]
- Das, A.; Ghosh, S. Flavor-specific interaction favors strong neutrino self-coupling in the early universe. JCAP 2021, 7, 38. [Google Scholar] [CrossRef]
- Braglia, M.; Ballardini, M.; Finelli, F.; Koyama, K. Early modified gravity in light of the H0 tension and LSS data. Phys. Rev. D 2021, 103, 43528. [Google Scholar] [CrossRef]
- Adi, T.; Kovetz, E.D. Can conformally coupled modified gravity solve the Hubble tension? Phys. Rev. D 2021, 103, 23530. [Google Scholar] [CrossRef]
- Banihashemi, A.; Khosravi, N.; Shafieloo, A. Dark energy as a critical phenomenon: A hint from Hubble tension. JCAP 2021, 6, 3. [Google Scholar] [CrossRef]
- Roy Choudhury, S.; Hannestad, S.; Tram, T. Updated constraints on massive neutrino self-interactions from cosmology in light of the H0 tension. JCAP 2021, 3, 84. [Google Scholar] [CrossRef]
- Mavromatos, N.E.; Solà Peracaula, J. Stringy-running-vacuum-model inflation: From primordial gravitational waves and stiff axion matter to dynamical dark energy. Eur. Phys. J. Special Top. 2021, 230, 2077–2110. [Google Scholar] [CrossRef]
- Cai, R.G.; Ding, J.F.; Guo, Z.K.; Wang, S.J.; Yu, W.W. Do the observational data favor a local void? Phys. Rev. D 2021, 103, 123539. [Google Scholar] [CrossRef]
- Haridasu, B.S.; Viel, M.; Vittorio, N. Sources of H0-tension in dark energy scenarios. Phys. Rev. D 2021, 103, 63539. [Google Scholar] [CrossRef]
- Shimon, M. Possible resolution of the Hubble tension with Weyl invariant gravity. JCAP 2022, 4, 48. [Google Scholar] [CrossRef]
- Brinckmann, T.; Chang, J.H.; LoVerde, M. Self-interacting neutrinos, the Hubble parameter tension, and the cosmic microwave background. Phys. Rev. D 2021, 104, 63523. [Google Scholar] [CrossRef]
- Alestas, G.; Kazantzidis, L.; Perivolaropoulos, L. w—M phantom transition at zt < 0.1 as a resolution of the Hubble tension. Phys. Rev. D 2021, 103, 83517. [Google Scholar]
- Yin, L. Reducing the H0 tension with exponential acoustic dark energy. Eur. Phys. J. C 2022, 82, 78. [Google Scholar] [CrossRef]
- Moshafi, H.; Baghram, S.; Khosravi, N. CMB lensing in a modified ΛCDM model in light of the H0 tension. Phys. Rev. D 2021, 104, 63506. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Di Valentino, E.; Mena, O.; Melchiorri, A. 2021-H0 odyssey: Closed, phantom and interacting dark energy cosmologies. JCAP 2021, 10, 8. [Google Scholar] [CrossRef]
- Seto, O.; Toda, Y. Comparing early dark energy and extra radiation solutions to the Hubble tension with BBN. Phys. Rev. D 2021, 103, 123501. [Google Scholar] [CrossRef]
- Sinha, S. Differentiating dark interactions with perturbation. Phys. Rev. D 2021, 103, 123547. [Google Scholar] [CrossRef]
- Gao, L.Y.; Zhao, Z.W.; Xue, S.S.; Zhang, X. Relieving the H 0 tension with a new interacting dark energy model. JCAP 2021, 7, 5. [Google Scholar] [CrossRef]
- Cai, R.G.; Guo, Z.K.; Li, L.; Wang, S.J.; Yu, W.W. Chameleon dark energy can resolve the Hubble tension. Phys. Rev. D 2021, 103, 121302. [Google Scholar] [CrossRef]
- Di Valentino, E.; Pan, S.; Yang, W.; Anchordoqui, L.A. Touch of neutrinos on the vacuum metamorphosis: Is the H0 solution back? Phys. Rev. D 2021, 103, 123527. [Google Scholar] [CrossRef]
- Marra, V.; Perivolaropoulos, L. Rapid transition of Geff at zt ≃0.01 as a possible solution of the Hubble and growth tensions. Phys. Rev. D 2021, 104, L021303. [Google Scholar] [CrossRef]
- Solà Peracaula, J.; Gómez-Valent, A.; de Cruz Perez, J.; Moreno-Pulido, C. Running vacuum against the H0 and σ8 tensions. EPL 2021, 134, 19001. [Google Scholar] [CrossRef]
- Kumar, S. Remedy of some cosmological tensions via effective phantom-like behavior of interacting vacuum energy. Phys. Dark Univ. 2021, 33, 100862. [Google Scholar] [CrossRef]
- Ren, X.; Wong, T.H.T.; Cai, Y.F.; Saridakis, E.N. Data-driven Reconstruction of the Late-time Cosmic Acceleration with f(T) Gravity. Phys. Dark Univ. 2021, 32, 100812. [Google Scholar] [CrossRef]
- Escudero, M.; Witte, S.J. The hubble tension as a hint of leptogenesis and neutrino mass generation. Eur. Phys. J. C 2021, 81, 515. [Google Scholar] [CrossRef]
- Levi Said, J.; Mifsud, J.; Sultana, J.; Adami, K.Z. Reconstructing teleparallel gravity with cosmic structure growth and expansion rate data. JCAP 2021, 6, 15. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Saez-Chillon Gomez, D.; Sharov, G.S. Modeling and testing the equation of state for (Early) dark energy. Phys. Dark Univ. 2021, 32, 100837. [Google Scholar] [CrossRef]
- Ye, G.; Hu, B.; Piao, Y.S. Implication of the Hubble tension for the primordial Universe in light of recent cosmological data. Phys. Rev. D 2021, 104, 63510. [Google Scholar] [CrossRef]
- Elizalde, E.; Gluza, J.; Khurshudyan, M. An approach to cold dark matter deviation and the H0 tension problem by using machine learning. arXiv 2021, arXiv:2104.01077. [Google Scholar]
- Dhawan, S.; Alsing, J.; Vagnozzi, S. Non-parametric spatial curvature inference using late-Universe cosmological probes. Mon. Not. R. Astron. Soc. 2021, 506, L1–L5. [Google Scholar] [CrossRef]
- Acquaviva, G.; Akarsu, O.; Katirci, N.; Vazquez, J.A. Simple-graduated dark energy and spatial curvature. Phys. Rev. D 2021, 104, 23505. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Aresté Saló, L.; de Haro, J. Theoretical and observational bounds on some interacting vacuum energy scenarios. Phys. Rev. D 2021, 103, 83520. [Google Scholar] [CrossRef]
- Paul, A.; Chatterjee, A.; Ghoshal, A.; Pal, S. Shedding light on dark matter and neutrino interactions from cosmology. JCAP 2021, 10, 17. [Google Scholar] [CrossRef]
- Geng, C.Q.; Hsu, Y.T.; Lu, J.R.; Yin, L. A Dark Energy model from Generalized Proca Theory. Phys. Dark Univ. 2021, 32, 100819. [Google Scholar] [CrossRef]
- Hashim, M.; El-Zant, A.A.; El Hanafy, W.; Golovnev, A. Toward a concordance teleparallel cosmology. Part II. Linear perturbation. JCAP 2021, 7, 53. [Google Scholar] [CrossRef]
- Alestas, G.; Antoniou, I.; Perivolaropoulos, L. Hints for a Gravitational Transition in Tully–Fisher Data. Universe 2021, 7, 366. [Google Scholar] [CrossRef]
- Fung, L.W.H.; Li, L.; Liu, T.; Luu, H.N.; Qiu, Y.C.; Tye, S.H.H. Hubble constant in the axi-Higgs universe. Phys. Rev. Res. 2023, 5, L022059. [Google Scholar] [CrossRef]
- Mavromatos, N.E.; Solà Peracaula, J. Inflationary physics and trans-Planckian conjecture in the stringy running vacuum model: From the phantom vacuum to the true vacuum. Eur. Phys. J. Plus 2021, 136, 1152. [Google Scholar] [CrossRef]
- Teng, Y.P.; Lee, W.; Ng, K.W. Constraining the dark-energy equation of state with cosmological data. Phys. Rev. D 2021, 104, 83519. [Google Scholar] [CrossRef]
- Thiele, L.; Guan, Y.; Hill, J.C.; Kosowsky, A.; Spergel, D.N. Can small-scale baryon inhomogeneities resolve the Hubble tension? An investigation with ACT DR4. Phys. Rev. D 2021, 104, 63535. [Google Scholar] [CrossRef]
- Zhou, Z.; Liu, G.; Mu, Y.; Xu, L. Can phantom transition at z ∼ 1 restore the Cosmic concordance? Mon. Not. R. Astron. Soc. 2022, 511, 595–606. [Google Scholar] [CrossRef]
- Linares Cedeño, F.X.; Roy, N.; Ureña López, L.A. Tracker phantom field and a cosmological constant: Dynamics of a composite dark energy model. Phys. Rev. D 2021, 104, 123502. [Google Scholar] [CrossRef]
- Gu, Y.; Wu, L.; Zhu, B. Axion dark radiation: Hubble tension and the Hyper-Kamiokande neutrino experiment. Phys. Rev. D 2022, 105, 95008. [Google Scholar] [CrossRef]
- Lucca, M. Dark energy–dark matter interactions as a solution to the S8 tension. Phys. Dark Univ. 2021, 34, 100899. [Google Scholar] [CrossRef]
- Krishnan, C.; Mohayaee, R.; Colgáin, E.O.; Sheikh-Jabbari, M.M.; Yin, L. Does Hubble tension signal a breakdown in FLRW cosmology? Class. Quant. Grav. 2021, 38, 184001. [Google Scholar] [CrossRef]
- Okamatsu, F.; Sekiguchi, T.; Takahashi, T. H0 tension without CMB data: Beyond the ΛCDM. Phys. Rev. D 2021, 104, 23523. [Google Scholar] [CrossRef]
- Jin, S.J.; Wang, L.F.; Wu, P.J.; Zhang, J.F.; Zhang, X. How can gravitational-wave standard sirens and 21-cm intensity mapping jointly provide a precise late-universe cosmological probe? Phys. Rev. D 2021, 104, 103507. [Google Scholar] [CrossRef]
- Krishnan, C.; Mohayaee, R.; Colgáin, E.O.; Sheikh-Jabbari, M.M.; Yin, L. Hints of FLRW breakdown from supernovae. Phys. Rev. D 2022, 105, 63514. [Google Scholar] [CrossRef]
- Dinda, B.R. Cosmic expansion parametrization: Implication for curvature and H0 tension. Phys. Rev. D 2022, 105, 63524. [Google Scholar] [CrossRef]
- Adil, S.A.; Gangopadhyay, M.R.; Sami, M.; Sharma, M.K. Late-time acceleration due to a generic modification of gravity and the Hubble tension. Phys. Rev. D 2021, 104, 103534. [Google Scholar] [CrossRef]
- de Araujo, J.C.N.; De Felice, A.; Kumar, S.; Nunes, R.C. Minimal theory of massive gravity in the light of CMB data and the S8 tension. Phys. Rev. D 2021, 104, 104057. [Google Scholar] [CrossRef]
- Beltrán Jiménez, J.; Bettoni, D.; Figueruelo, D.; Teppa Pannia, F.A.; Tsujikawa, S. Probing elastic interactions in the dark sector and the role of S8. Phys. Rev. D 2021, 104, 103503. [Google Scholar] [CrossRef]
- Karwal, T.; Raveri, M.; Jain, B.; Khoury, J.; Trodden, M. Chameleon early dark energy and the Hubble tension. Phys. Rev. D 2022, 105, 63535. [Google Scholar] [CrossRef]
- Lucca, M. Multi-interacting dark energy and its cosmological implications. Phys. Rev. D 2021, 104, 83510. [Google Scholar] [CrossRef]
- Bag, S.; Sahni, V.; Shafieloo, A.; Shtanov, Y. Phantom Braneworld and the Hubble Tension. Astrophys. J. 2021, 923, 212. [Google Scholar] [CrossRef]
- Jiang, J.Q.; Piao, Y.S. Testing AdS early dark energy with Planck, SPTpol, and LSS data. Phys. Rev. D 2021, 104, 103524. [Google Scholar] [CrossRef]
- Ghosh, S.; Kumar, S.; Tsai, Y. Free-streaming and coupled dark radiation isocurvature perturbations: Constraints and application to the Hubble tension. JCAP 2022, 5, 14. [Google Scholar] [CrossRef]
- Nunes, R.C.; Di Valentino, E. Dark sector interaction and the supernova absolute magnitude tension. Phys. Rev. D 2021, 104, 63529. [Google Scholar] [CrossRef]
- Gómez-Valent, A.; Zheng, Z.; Amendola, L.; Pettorino, V.; Wetterich, C. Early dark energy in the pre- and postrecombination epochs. Phys. Rev. D 2021, 104, 83536. [Google Scholar] [CrossRef]
- Li, B.; Shapiro, P.R. Precision cosmology and the stiff-amplified gravitational-wave background from inflation: NANOGrav, Advanced LIGO-Virgo and the Hubble tension. JCAP 2021, 10, 24. [Google Scholar] [CrossRef]
- Hart, L.; Chluba, J. Varying fundamental constants principal component analysis: Additional hints about the Hubble tension. Mon. Not. R. Astron. Soc. 2022, 510, 2206–2227. [Google Scholar] [CrossRef]
- Pogosian, L.; Raveri, M.; Koyama, K.; Martinelli, M.; Silvestri, A.; Zhao, G.B.; Li, J.; Peirone, S.; Zucca, A. Imprints of cosmological tensions in reconstructed gravity. Nat. Astron. 2022, 6, 1484–1490. [Google Scholar] [CrossRef]
- Cyr-Racine, F.Y.; Ge, F.; Knox, L. Symmetry of Cosmological Observables, a Mirror World Dark Sector, and the Hubble Constant. Phys. Rev. Lett. 2022, 128, 201301. [Google Scholar] [CrossRef] [PubMed]
- Ye, G.; Zhang, J.; Piao, Y.S. Alleviating both H0 and S8 tensions: Early dark energy lifts the CMB-lockdown on ultralight axion. Phys. Lett. B 2023, 839, 137770. [Google Scholar] [CrossRef]
- Normann, B.D.; Brevik, I.H. Can the Hubble tension be resolved by bulk viscosity? Mod. Phys. Lett. A 2021, 36, 2150198. [Google Scholar] [CrossRef]
- Anchordoqui, L.A.; Di Valentino, E.; Pan, S.; Yang, W. Dissecting the H0 and S8 tensions with Planck + BAO + supernova type Ia in multi-parameter cosmologies. JHEAp 2021, 32, 28–64. [Google Scholar] [CrossRef]
- Staicova, D.; Benisty, D. Constraining the dark energy models using Baryon Acoustic Oscillations: An approach independent of H0·rd. Astron. Astrophys. 2022, 668, A135. [Google Scholar] [CrossRef]
- Chang, C.F. Imprint of early dark energy in stochastic gravitational wave background. Phys. Rev. D 2022, 105, 23508. [Google Scholar] [CrossRef]
- Drees, M.; Zhao, W. U(1)Lμ−Lτ for Light Dark Matter, gμ-2, the 511 keV excess and the Hubble Tension. Phys. Lett. B 2022, 827, 136948. [Google Scholar] [CrossRef]
- Rashkovetskyi, M.; Muñoz, J.B.; Eisenstein, D.J.; Dvorkin, C. Small-scale clumping at recombination and the Hubble tension. Phys. Rev. D 2021, 104, 103517. [Google Scholar] [CrossRef]
- Qiu, X.W.; Zhao, Z.W.; Wang, L.F.; Zhang, J.F.; Zhang, X. A forecast of using fast radio burst observations to constrain holographic dark energy. JCAP 2022, 2, 6. [Google Scholar] [CrossRef]
- Liu, W.; Anchordoqui, L.A.; Di Valentino, E.; Pan, S.; Wu, Y.; Yang, W. Constraints from high-precision measurements of the cosmic microwave background: The case of disintegrating dark matter with Λ or dynamical dark energy. JCAP 2022, 2, 12. [Google Scholar] [CrossRef]
- Rezaei, M.; Solà Peracaula, J.; Malekjani, M. Cosmographic approach to Running Vacuum dark energy models: New constraints using BAOs and Hubble diagrams at higher redshifts. Mon. Not. R. Astron. Soc. 2021, 509, 2593–2608. [Google Scholar] [CrossRef]
- Nilsson, N.A.; Park, M.I. Tests of standard cosmology in Hořava gravity, Bayesian evidence for a closed universe, and the Hubble tension. Eur. Phys. J. C 2022, 82, 873. [Google Scholar] [CrossRef]
- Akarsu, O.; Kumar, S.; Özülker, E.; Vazquez, J.A. Relaxing cosmological tensions with a sign switching cosmological constant. Phys. Rev. D 2021, 104, 123512. [Google Scholar] [CrossRef]
- Luongo, O.; Muccino, M.; Colgáin, E.O.; Sheikh-Jabbari, M.M.; Yin, L. Larger H0 values in the CMB dipole direction. Phys. Rev. D 2022, 105, 103510. [Google Scholar] [CrossRef]
- Mawas, E.; Street, L.; Gass, R.; Wijewardhana, L.C.R. Interacting dark energy axions in light of the Hubble tension. arXiv 2021, arXiv:2108.13317. [Google Scholar]
- Perivolaropoulos, L.; Skara, F. Hubble tension or a transition of the Cepheid SnIa calibrator parameters? Phys. Rev. D 2021, 104, 123511. [Google Scholar] [CrossRef]
- Hill, J.C.; Calabrese, E.; Aiola, S.; Battaglia, N.; Bolliet, B.; Choi, S.K.; Devlin, M.J.; Duivenvoorden, A.J.; Dunkley, J.; Ferraro, S.; et al. Atacama Cosmology Telescope: Constraints on prerecombination early dark energy. Phys. Rev. D 2022, 105, 123536. [Google Scholar] [CrossRef]
- Feng, L.; Guo, R.Y.; Zhang, J.F.; Zhang, X. Cosmological search for sterile neutrinos after Planck 2018. Phys. Lett. B 2022, 827, 136940. [Google Scholar] [CrossRef]
- Poulin, V.; Smith, T.L.; Bartlett, A. Dark energy at early times and ACT data: A larger Hubble constant without late-time priors. Phys. Rev. D 2021, 104, 123550. [Google Scholar] [CrossRef]
- Theodoropoulos, A.; Perivolaropoulos, L. The Hubble Tension, the M Crisis of Late Time H(z) Deformation Models and the Reconstruction of Quintessence Lagrangians. Universe 2021, 7, 300. [Google Scholar] [CrossRef]
- Hogg, N.B.; Bruni, M. Shan–Chen interacting vacuum cosmology. Mon. Not. R. Astron. Soc. 2022, 511, 4430–4443. [Google Scholar] [CrossRef]
- Drepanou, N.; Lymperis, A.; Saridakis, E.N.; Yesmakhanova, K. Kaniadakis holographic dark energy and cosmology. Eur. Phys. J. C 2022, 82, 449. [Google Scholar] [CrossRef]
- Khosravi, N.; Farhang, M. Phenomenological gravitational phase transition: Early and late modifications. Phys. Rev. D 2022, 105, 63505. [Google Scholar] [CrossRef]
- Aghababaei, S.; Moradpour, H.; Vagenas, E.C. Hubble tension bounds the GUP and EUP parameters. Eur. Phys. J. Plus 2021, 136, 997. [Google Scholar] [CrossRef]
- Petronikolou, M.; Basilakos, S.; Saridakis, E.N. Alleviating H0 tension in Horndeski gravity. Phys. Rev. D 2022, 106, 124051. [Google Scholar] [CrossRef]
- Guo, R.Y.; Feng, L.; Yao, T.Y.; Chen, X.Y. Exploration of interacting dynamical dark energy model with interaction term including the equation-of-state parameter: Alleviation of the H0 tension. JCAP 2021, 12, 36. [Google Scholar] [CrossRef]
- Di Valentino, E.; Gariazzo, S.; Giunti, C.; Mena, O.; Pan, S.; Yang, W. Minimal dark energy: Key to sterile neutrino and Hubble constant tensions? Phys. Rev. D 2022, 105, 103511. [Google Scholar] [CrossRef]
- Castello, S.; Högås, M.; Mörtsell, E. A cosmological underdensity does not solve the Hubble tension. JCAP 2022, 7, 3, Erratum in JCAP 2022, 09, E01. [Google Scholar] [CrossRef]
- Bansal, S.; Kim, J.H.; Kolda, C.; Low, M.; Tsai, Y. Mirror twin Higgs cosmology: Constraints and a possible resolution to the H0 and S8 tensions. JHEP 2022, 5, 50. [Google Scholar] [CrossRef]
- Alestas, G.; Camarena, D.; Di Valentino, E.; Kazantzidis, L.; Marra, V.; Nesseris, S.; Perivolaropoulos, L. Late-transition versus smooth H(z)-deformation models for the resolution of the Hubble crisis. Phys. Rev. D 2022, 105, 63538. [Google Scholar] [CrossRef]
- De Simone, B.; Nielson, V.; Rinaldi, E.; Dainotti, M.G. A new perspective on cosmology through Supernovae Ia and Gamma Ray Bursts. In Proceedings of the 16th Marcel Grossmann Meeting on Recent Developments in Theoretical and Experimental General Relativity, Astrophysics and Relativistic Field Theories, Online, 5–10 July 2021. [Google Scholar]
- Clark, S.J.; Vattis, K.; Fan, J.; Koushiappas, S.M. H0 and S8 tensions necessitate early and late time changes to ΛCDM. Phys. Rev. D 2023, 107, 83527. [Google Scholar] [CrossRef]
- Aloni, D.; Berlin, A.; Joseph, M.; Schmaltz, M.; Weiner, N. A Step in understanding the Hubble tension. Phys. Rev. D 2022, 105, 123516. [Google Scholar] [CrossRef]
- Luu, H.N. Axion-Higgs cosmology: Cosmic microwave background and cosmological tensions. Phys. Rev. D 2023, 107, 23513. [Google Scholar] [CrossRef]
- Tiwari, P.; Kothari, R.; Jain, P. Superhorizon Perturbations: A Possible Explanation of the Hubble–Lemaître Tension and the Large-scale Anisotropy of the Universe. Astrophys. J. Lett. 2022, 924, L36. [Google Scholar] [CrossRef]
- Gariazzo, S.; Di Valentino, E.; Mena, O.; Nunes, R.C. Late-time interacting cosmologies and the Hubble constant tension. Phys. Rev. D 2022, 106, 23530. [Google Scholar] [CrossRef]
- Benetti, M.; Graef, L.L.; Vagnozzi, S. Primordial gravitational waves from NANOGrav: A broken power-law approach. Phys. Rev. D 2022, 105, 43520. [Google Scholar] [CrossRef]
- Belgacem, E.; Prokopec, T. Quantum origin of dark energy and the Hubble tension. Phys. Lett. B 2022, 831, 137174. [Google Scholar] [CrossRef]
- Duan, W.F.; Li, S.P.; Li, X.Q.; Yang, Y.D. Linking anomalies to Hubble tension via a single right-handed neutrino *. Chin. Phys. C 2023, 47, 33102. [Google Scholar] [CrossRef]
- Dialektopoulos, K.; Said, J.L.; Mifsud, J.; Sultana, J.; Adami, K.Z. Neural network reconstruction of late-time cosmology and null tests. JCAP 2022, 2, 23. [Google Scholar] [CrossRef]
- Gómez-Valent, A. Measuring the sound horizon and absolute magnitude of SNIa by maximizing the consistency between low-redshift data sets. Phys. Rev. D 2022, 105, 43528. [Google Scholar] [CrossRef]
- Corona, M.A.; Murgia, R.; Cadeddu, M.; Archidiacono, M.; Gariazzo, S.; Giunti, C.; Hannestad, S. Pseudoscalar sterile neutrino self-interactions in light of Planck, SPT and ACT data. JCAP 2022, 6, 10. [Google Scholar] [CrossRef]
- Niedermann, F.; Sloth, M.S. Hot new early dark energy: Towards a unified dark sector of neutrinos, dark energy and dark matter. Phys. Lett. B 2022, 835, 137555. [Google Scholar] [CrossRef]
- Niedermann, F.; Sloth, M.S. Hot new early dark energy. Phys. Rev. D 2022, 105, 63509. [Google Scholar] [CrossRef]
- Di Valentino, E.; Melchiorri, A. Neutrino Mass Bounds in the Era of Tension Cosmology. Astrophys. J. Lett. 2022, 931, L18. [Google Scholar] [CrossRef]
- Renzi, F.; Hogg, N.B.; Giarè, W. The resilience of the Etherington–Hubble relation. Mon. Not. R. Astron. Soc. 2022, 513, 4004–4014. [Google Scholar] [CrossRef]
- Takahashi, F.; Yin, W. Cosmological implications of ns ≈ 1 in light of the Hubble tension. Phys. Lett. B 2022, 830, 137143. [Google Scholar] [CrossRef]
- Spallicci, A.D.A.M.; Benetti, M.; Capozziello, S. The Heisenberg Limit at Cosmological Scales. Found. Phys. 2022, 52, 23. [Google Scholar] [CrossRef]
- Akarsu, O.; Di Valentino, E.; Kumar, S.; Ozyigit, M.; Sharma, S. Testing spatial curvature and anisotropic expansion on top of the ΛCDM model. Phys. Dark Univ. 2023, 39, 101162. [Google Scholar] [CrossRef]
- Saridakis, E.N.; Yang, W.; Pan, S.; Anagnostopoulos, F.K.; Basilakos, S. Observational constraints on soft dark energy and soft dark matter: Challenging ΛCDM cosmology. Nucl. Phys. B 2023, 986, 116042. [Google Scholar] [CrossRef]
- McDonough, E.; Lin, M.X.; Hill, J.C.; Hu, W.; Zhou, S. Early dark sector, the Hubble tension, and the swampland. Phys. Rev. D 2022, 106, 43525. [Google Scholar] [CrossRef]
- Sen, A.A.; Adil, S.A.; Sen, S. Do cosmological observations allow a negative Λ? Mon. Not. R. Astron. Soc. 2022, 518, 1098–1105. [Google Scholar] [CrossRef]
- La Posta, A.; Louis, T.; Garrido, X.; Hill, J.C. Constraints on prerecombination early dark energy from SPT-3G public data. Phys. Rev. D 2022, 105, 83519. [Google Scholar] [CrossRef]
- Greene, K.L.; Cyr-Racine, F.Y. Hubble distancing: Focusing on distance measurements in cosmology. JCAP 2022, 6, 2. [Google Scholar] [CrossRef]
- Herold, L.; Ferreira, E.G.M.; Komatsu, E. New Constraint on Early Dark Energy from Planck and BOSS Data Using the Profile Likelihood. Astrophys. J. Lett. 2022, 929, L16. [Google Scholar] [CrossRef]
- Sakr, Z.; Sapone, D. Can varying the gravitational constant alleviate the tensions? JCAP 2022, 3, 34. [Google Scholar] [CrossRef]
- Cao, M.D.; Zheng, J.; Qi, J.Z.; Zhang, X.; Zhu, Z.H. A New Way to Explore Cosmological Tensions Using Gravitational Waves and Strong Gravitational Lensing. Astrophys. J. 2022, 934, 108. [Google Scholar] [CrossRef]
- Solomon, R.; Agarwal, G.; Stojkovic, D. Environment dependent electron mass and the Hubble constant tension. Phys. Rev. D 2022, 105, 103536. [Google Scholar] [CrossRef]
- Colaço, L.R.; Holanda, R.F.L.; Nunes, R.C. Varying-α in scalar-tensor theory: Implications in light of the supernova absolute magnitude tension and forecast from GW standard sirens. arXiv 2022, arXiv:2201.04073. [Google Scholar]
- Banihashemi, A.; Khosravi, N. Fluctuations in the Ginzburg–Landau Theory of Dark Energy: Internal (In)consistencies in the Planck Data Set. Astrophys. J. 2022, 931, 148. [Google Scholar] [CrossRef]
- Alestas, G.; Perivolaropoulos, L.; Tanidis, K. Constraining a late time transition of Geff using low-z galaxy survey data. Phys. Rev. D 2022, 106, 23526. [Google Scholar] [CrossRef]
- Wang, H.; Piao, Y.S. Testing dark energy after pre-recombination early dark energy. Phys. Lett. B 2022, 832, 137244. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K. Did the Universe experience a pressure non-crushing type cosmological singularity in the recent past? EPL 2022, 137, 39001. [Google Scholar] [CrossRef]
- Perivolaropoulos, L. Is the Hubble Crisis Connected with the Extinction of Dinosaurs? Universe 2022, 8, 263. [Google Scholar] [CrossRef]
- Roy, N.; Goswami, S.; Das, S. Quintessence or phantom: Study of scalar field dark energy models through a general parametrization of the Hubble parameter. Phys. Dark Univ. 2022, 36, 101037. [Google Scholar] [CrossRef]
- Dainotti, M.G.; De Simone, B.; Schiavone, T.; Montani, G.; Rinaldi, E.; Lambiase, G.; Bogdan, M.; Ugale, S. On the Evolution of the Hubble Constant with the SNe Ia Pantheon Sample and Baryon Acoustic Oscillations: A Feasibility Study for GRB-Cosmology in 2030. Galaxies 2022, 10, 24. [Google Scholar] [CrossRef]
- Heisenberg, L.; Villarrubia-Rojo, H.; Zosso, J. Simultaneously solving the H0 and σ8 tensions with late dark energy. Phys. Dark Univ. 2023, 39, 101163. [Google Scholar] [CrossRef]
- Hazra, D.K.; Antony, A.; Shafieloo, A. One spectrum to cure them all: Signature from early Universe solves major anomalies and tensions in cosmology. JCAP 2022, 8, 63. [Google Scholar] [CrossRef]
- Wang, Y.J.; Qi, J.Z.; Wang, B.; Zhang, J.F.; Cui, J.L.; Zhang, X. Cosmological model-independent measurement of cosmic curvature using distance sum rule with the help of gravitational waves. Mon. Not. R. Astron. Soc. 2022, 516, 5187–5195. [Google Scholar] [CrossRef]
- Heisenberg, L.; Villarrubia-Rojo, H.; Zosso, J. Can late-time extensions solve the H0 and σ8 tensions? Phys. Rev. D 2022, 106, 43503. [Google Scholar] [CrossRef]
- Qi, J.Z.; Cui, Y.; Hu, W.H.; Zhang, J.F.; Cui, J.L.; Zhang, X. Strongly lensed type Ia supernovae as a precise late-Universe probe of measuring the Hubble constant and cosmic curvature. Phys. Rev. D 2022, 106, 23520. [Google Scholar] [CrossRef]
- Sharma, R.K.; Pandey, K.L.; Das, S. Implications of an Extended Dark Energy Model with Massive Neutrinos. Astrophys. J. 2022, 934, 113. [Google Scholar] [CrossRef]
- Lee, B.H.; Lee, W.; Colgáin, E.O.; Sheikh-Jabbari, M.M.; Thakur, S. Is local H 0 at odds with dark energy EFT? JCAP 2022, 4, 4. [Google Scholar] [CrossRef]
- Benisty, D.; Mifsud, J.; Levi Said, J.; Staicova, D. On the robustness of the constancy of the Supernova absolute magnitude: Non-parametric reconstruction & Bayesian approaches. Phys. Dark Univ. 2023, 39, 101160. [Google Scholar]
- Aboubrahim, A.; Klasen, M.; Nath, P. Analyzing the Hubble tension through hidden sector dynamics in the early universe. JCAP 2022, 4, 42. [Google Scholar] [CrossRef]
- Hoshiya, K.; Toda, Y. Electron mass variation from dark sector interactions and compatibility with cosmological observations. Phys. Rev. D 2023, 107, 43505. [Google Scholar] [CrossRef]
- Sabla, V.I.; Caldwell, R.R. Microphysics of early dark energy. Phys. Rev. D 2022, 106, 63526. [Google Scholar] [CrossRef]
- Heeck, J.; Thapa, A. Explaining lepton-flavor non-universality and self-interacting dark matter with Lμ−Lτ. Eur. Phys. J. C 2022, 82, 480. [Google Scholar] [CrossRef]
- Benevento, G.; Kable, J.A.; Addison, G.E.; Bennett, C.L. An Exploration of an Early Gravity Transition in Light of Cosmological Tensions. Astrophys. J. 2022, 935, 156. [Google Scholar] [CrossRef]
- Smith, T.L.; Lucca, M.; Poulin, V.; Abellan, G.F.; Balkenhol, L.; Benabed, K.; Galli, S.; Murgia, R. Hints of early dark energy in Planck, SPT, and ACT data: New physics or systematics? Phys. Rev. D 2022, 106, 43526. [Google Scholar] [CrossRef]
- Ye, G.; Piao, Y.S. Improved constraints on primordial gravitational waves in light of the H0 tension and BICEP/Keck data. Phys. Rev. D 2022, 106, 43536. [Google Scholar] [CrossRef]
- Mazo, B.Y.D.V.; Romano, A.E.; Quintero, M.A.C. H0 tension or M overestimation? Eur. Phys. J. C 2022, 82, 610. [Google Scholar] [CrossRef]
- Jin, S.J.; Li, T.N.; Zhang, J.F.; Zhang, X. Precisely measuring the Hubble constant and dark energy using only gravitational-wave dark sirens. arXiv 2022, arXiv:2202.11882. [Google Scholar]
- Cai, R.G.; Guo, Z.K.; Wang, S.J.; Yu, W.W.; Zhou, Y. No-go guide for late-time solutions to the Hubble tension: Matter perturbations. Phys. Rev. D 2022, 106, 63519. [Google Scholar] [CrossRef]
- Jiang, J.Q.; Piao, Y.S. Toward early dark energy and ns=1 with Planck, ACT, and SPT observations. Phys. Rev. D 2022, 105, 103514. [Google Scholar] [CrossRef]
- Mehrabi, A.; Levi Said, J. Gaussian discriminators between ΛCDM and wCDM cosmologies using expansion data. Eur. Phys. J. C 2022, 82, 806. [Google Scholar] [CrossRef]
- Ren, X.; Yan, S.F.; Zhao, Y.; Cai, Y.F.; Saridakis, E.N. Gaussian processes and effective field theory of f(T) gravity under the H0 tension. Astrophys. J. 2022, 932, 2. [Google Scholar] [CrossRef]
- Anchordoqui, L.A.; Barger, V.; Marfatia, D.; Soriano, J.F. Decay of multiple dark matter particles to dark radiation in different epochs does not alleviate the Hubble tension. Phys. Rev. D 2022, 105, 103512. [Google Scholar] [CrossRef]
- Adhikari, S. The Hubble tension in the non-flat Super-ΛCDM model. Phys. Dark Univ. 2022, 36, 101005. [Google Scholar] [CrossRef]
- Simon, T.; Franco Abellán, G.; Du, P.; Poulin, V.; Tsai, Y. Constraining decaying dark matter with BOSS data and the effective field theory of large-scale structures. Phys. Rev. D 2022, 106, 23516. [Google Scholar] [CrossRef]
- Nunes, R.C.; Vagnozzi, S.; Kumar, S.; Di Valentino, E.; Mena, O. New tests of dark sector interactions from the full-shape galaxy power spectrum. Phys. Rev. D 2022, 105, 123506. [Google Scholar] [CrossRef]
- Harko, T.; Asadi, K.; Moshafi, H.; Sheikhahmadi, H. Observational constraints on the interacting dark energy — Dark matter (IDM) cosmological models. Phys. Dark Univ. 2022, 38, 101131. [Google Scholar] [CrossRef]
- Davari, Z.; Khosravi, N. Can decaying dark matter scenarios alleviate both H0 and σ8 tensions? Mon. Not. R. Astron. Soc. 2022, 516, 4373–4382. [Google Scholar] [CrossRef]
- Qi, J.Z.; Hu, W.H.; Cui, Y.; Zhang, J.F.; Zhang, X. Cosmological Parameter Estimation Using Current and Future Observations of Strong Gravitational Lensing. Universe 2022, 8, 254. [Google Scholar] [CrossRef]
- Fondi, E.; Melchiorri, A.; Pagano, L. No evidence for EDE from Planck data in extended scenarios. Astrophys. J. Lett. 2022, 931, L18. [Google Scholar] [CrossRef]
- Hu, J.P.; Wang, F.Y. Revealing the late-time transition of H0: Relieve the Hubble crisis. Mon. Not. R. Astron. Soc. 2022, 517, 576–581. [Google Scholar] [CrossRef]
- Gómez-Valent, A. Fast test to assess the impact of marginalization in Monte Carlo analyses and its application to cosmology. Phys. Rev. D 2022, 106, 63506. [Google Scholar] [CrossRef]
- Jin, S.J.; Zhu, R.Q.; Wang, L.F.; Li, H.L.; Zhang, J.F.; Zhang, X. Impacts of gravitational-wave standard siren observations from Einstein Telescope and Cosmic Explorer on weighing neutrinos in interacting dark energy models. Commun. Theor. Phys. 2022, 74, 105404. [Google Scholar] [CrossRef]
- Archidiacono, M.; Castorina, E.; Redigolo, D.; Salvioni, E. Unveiling dark fifth forces with linear cosmology. JCAP 2022, 10, 74. [Google Scholar] [CrossRef]
- Berghaus, K.V.; Karwal, T. Thermal friction as a solution to the Hubble and large-scale structure tensions. Phys. Rev. D 2023, 107, 103515. [Google Scholar] [CrossRef]
- Yusofi, E.; Ramzanpour, M.A. Cosmological Constant Problem and H0 Tension in Void-dominated Cosmology. arXiv 2022, arXiv:2204.12180. [Google Scholar]
- Carneiro, S.; Pigozzo, C.; Alcaniz, J.S. Redshift systematics and the H0 tension problem. Eur. Phys. J. Plus 2022, 137, 537. [Google Scholar] [CrossRef]
- Ye, G.; Jiang, J.Q.; Piao, Y.S. Toward inflation with ns=1 in light of the Hubble tension and implications for primordial gravitational waves. Phys. Rev. D 2022, 106, 103528. [Google Scholar] [CrossRef]
- Kumar, S.; Nunes, R.C.; Yadav, P. Updating non-standard neutrinos properties with Planck-CMB data and full-shape analysis of BOSS and eBOSS galaxies. JCAP 2022, 9, 60. [Google Scholar] [CrossRef]
- Camarena, D.; Marra, V.; Sakr, Z.; Clarkson, C. A void in the Hubble tension? The end of the line for the Hubble bubble. Class. Quant. Grav. 2022, 39, 184001. [Google Scholar] [CrossRef]
- Alvi, S.; Brinckmann, T.; Gerbino, M.; Lattanzi, M.; Pagano, L. Do you smell something decaying? Updated linear constraints on decaying dark matter scenarios. JCAP 2022, 11, 15. [Google Scholar] [CrossRef]
- Garcia-Arroyo, G.; Cervantes-Cota, J.L.; Nucamendi, U. Neutrino mass and kinetic gravity braiding degeneracies. JCAP 2022, 8, 9. [Google Scholar] [CrossRef]
- Aljaf, M.; Elizalde, E.; Khurshudyan, M.; Myrzakulov, K.; Zhadyranova, A. Solving the H0 tension in f(T) gravity through Bayesian machine learning. Eur. Phys. J. C 2022, 82, 1130. [Google Scholar] [CrossRef]
- Schiavone, T.; Montani, G.; Dainotti, M.G.; De Simone, B.; Rinaldi, E.; Lambiase, G. Running Hubble Constant from the SNe Ia Pantheon Sample? In Proceedings of the 17th Italian-Korean Symposium on Relativistic Astrophysics, Online, 2–6 August 2022. [Google Scholar]
- Vagnozzi, S.; Roy, R.; Tsai, Y.D.; Visinelli, L.; Afrin, M.; Allahyari, A.; Bambhaniya, P.; Dey, D.; Ghosh, S.G.; Joshi, P.S.; et al. Horizon-scale tests of gravity theories and fundamental physics from the Event Horizon Telescope image of Sagittarius A. Class. Quant. Grav. 2023, 40, 165007. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Oikonomou, V.K. Integral F(R) gravity and saddle point condition as a remedy for the H0-tension. Nucl. Phys. B 2022, 980, 115850. [Google Scholar] [CrossRef]
- Sandoval-Orozco, R.; Escamilla-Rivera, C. Cosmological piecewise functions to treat the local Hubble tension. Eur. Phys. J. Plus 2022, 137, 819. [Google Scholar] [CrossRef]
- Kojima, K.; Okubo, Y. Early dark energy from a higher-dimensional gauge theory. Phys. Rev. D 2022, 106, 63540. [Google Scholar] [CrossRef]
- Yao, Y.H.; Meng, X.H. A new coupled three-form dark energy model and implications for the H0 tension. Phys. Dark Univ. 2020, 30, 100729. [Google Scholar] [CrossRef]
- Mohseni Sadjadi, H.; Anari, V. Early dark energy and the screening mechanism. Eur. Phys. J. Plus 2023, 138, 84. [Google Scholar] [CrossRef]
- Oikonomou, V.K.; Lymperiadou, E.C. Effects of a Geometrically Realized Early Dark Energy Era on the Spectrum of Primordial Gravitational Waves. Symmetry 2022, 14, 1143. [Google Scholar] [CrossRef]
- Hernández-Jiménez, R.; Moreno, C.; Bellini, M.; Ortiz, C. Cosmological Boundary Flux Parameter. Phys. Dark Univ. 2022, 38, 101137. [Google Scholar] [CrossRef]
- Schöneberg, N.; Franco Abellán, G. A step in the right direction? Analyzing the Wess Zumino Dark Radiation solution to the Hubble tension. JCAP 2022, 12, 1. [Google Scholar] [CrossRef]
- Seto, O.; Toda, Y. Big bang nucleosynthesis constraints on varying electron mass solution to the Hubble tension. Phys. Rev. D 2023, 107, 83512. [Google Scholar] [CrossRef]
- Reeves, A.; Herold, L.; Vagnozzi, S.; Sherwin, B.D.; Ferreira, E.G.M. Restoring cosmological concordance with early dark energy and massive neutrinos? Mon. Not. R. Astron. Soc. 2023, 520, 3688–3695. [Google Scholar] [CrossRef]
- Zhai, Z.; Percival, W.J. Sample variance for supernovae distance measurements and the Hubble tension. Phys. Rev. D 2022, 106, 103527. [Google Scholar] [CrossRef]
- Joseph, M.; Aloni, D.; Schmaltz, M.; Sivarajan, E.N.; Weiner, N. A Step in Understanding the S8 Tension. arXiv 2022, arXiv:2207.03500. [Google Scholar] [CrossRef]
- Aluri, P.K.; Cea, P.; Chingangbam, P.; Chu, M.-C.; Clowes, R.G.; Hutsemekers, D.; Kochappan, J.P.; Lopez, A.M.; Liu, L.; Martens, N.C.; et al. Is the observable Universe consistent with the cosmological principle? Class. Quant. Grav. 2023, 40, 94001. [Google Scholar] [CrossRef]
- Adil, A.; Albrecht, A.; Knox, L. Quintessential cosmological tensions. Phys. Rev. D 2023, 107, 63521. [Google Scholar] [CrossRef]
- Trivedi, O. Another look on the connections of Hubble tension with the Heisenberg Uncertainty Principle. Phys. Dark Univ. 2023, 39, 101150. [Google Scholar] [CrossRef]
- Akarsu, O.; Colgain, E.O.; Özulker, E.; Thakur, S.; Yin, L. Inevitable manifestation of wiggles in the expansion of the late Universe. Phys. Rev. D 2023, 107, 123526. [Google Scholar] [CrossRef]
- Wang, D. Pantheon+ tomography and Hubble tension. arXiv 2022, arXiv:2207.10927. [Google Scholar]
- Rezaei, M.; Sola Peracaula, J. Running vacuum versus holographic dark energy: A cosmographic comparison. Eur. Phys. J. C 2022, 82, 765. [Google Scholar] [CrossRef]
- Gómez-Valent, A.; Zheng, Z.; Amendola, L.; Wetterich, C.; Pettorino, V. Coupled and uncoupled early dark energy, massive neutrinos, and the cosmological tensions. Phys. Rev. D 2022, 106, 103522. [Google Scholar] [CrossRef]
- El Bourakadi, K. Hubble tension and Reheating: Hybrid Inflation Implications. arXiv 2022, arXiv:2208.01162. [Google Scholar]
- Moshafi, H.; Firouzjahi, H.; Talebian, A. Multiple Transitions in Vacuum Dark Energy and H 0 Tension. Astrophys. J. 2022, 940, 121. [Google Scholar] [CrossRef]
- Simon, T.; Zhang, P.; Poulin, V.; Smith, T.L. Updated constraints from the effective field theory analysis of the BOSS power spectrum on early dark energy. Phys. Rev. D 2023, 107, 63505. [Google Scholar] [CrossRef]
- Buen-Abad, M.A.; Chacko, Z.; Kilic, C.; Marques-Tavares, G.; Youn, T. Stepped partially acoustic dark matter, large scale structure, and the Hubble tension. JHEP 2023, 6, 12. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K. Dissimilar donuts in the sky? Effects of a pressure singularity on the circular photon orbits and shadow of a cosmological black hole. EPL 2022, 139, 59003. [Google Scholar] [CrossRef]
- Rezazadeh, K.; Ashoorioon, A.; Grin, D. Cascading Dark Energy. arXiv 2022, arXiv:2208.07631. [Google Scholar]
- Perivolaropoulos, L.; Skara, F. A Reanalysis of the Latest SH0ES Data for H0: Effects of New Degrees of Freedom on the Hubble Tension. Universe 2022, 8, 502. [Google Scholar] [CrossRef]
- Escudero, H.G.; Kuo, J.L.; Keeley, R.E.; Abazajian, K.N. Early or phantom dark energy, self-interacting, extra, or massive neutrinos, primordial magnetic fields, or a curved universe: An exploration of possible solutions to the H0 and σ8 problems. Phys. Rev. D 2022, 106, 103517. [Google Scholar] [CrossRef]
- Banerjee, S.; Petronikolou, M.; Saridakis, E.N. Alleviating the H0 tension with new gravitational scalar tensor theories. Phys. Rev. D 2023, 108, 24012. [Google Scholar] [CrossRef]
- Cruz, J.S.; Niedermann, F.; Sloth, M.S. A grounded perspective on new early dark energy using ACT, SPT, and BICEP/Keck. JCAP 2023, 2, 41. [Google Scholar] [CrossRef]
- Oikonomou, V.K.; Tsyba, P.; Razina, O. Probing Our Universe’s Past Using Earth’s Geological and Climatological History and Shadows of Galactic Black Holes. Universe 2022, 8, 484. [Google Scholar] [CrossRef]
- Naidoo, K.; Jaber, M.; Hellwing, W.A.; Bilicki, M. A dark matter solution to the H0 and σ8 tensions, and the integrated Sachs-Wolfe void anomaly. arXiv 2022, arXiv:2209.08102. [Google Scholar]
- Wang, H.; Piao, Y.S. A fraction of dark matter faded with early dark energy? arXiv 2022, arXiv:2209.09685. [Google Scholar]
- Kumar, S.; Nunes, R.C.; Yadav, P. New cosmological constraints on f(T) gravity in light of full Planck-CMB and type Ia supernovae data. Phys. Rev. D 2023, 107, 63529. [Google Scholar] [CrossRef]
- de Sá, R.; Benetti, M.; Graef, L.L. An empirical investigation into cosmological tensions. Eur. Phys. J. Plus 2022, 137, 1129. [Google Scholar] [CrossRef]
- Di Valentino, E.; Giarè, W.; Melchiorri, A.; Silk, J. Health checkup test of the standard cosmological model in view of recent cosmic microwave background anisotropies experiments. Phys. Rev. D 2022, 106, 103506. [Google Scholar] [CrossRef]
- Secco, L.F.; Karwal, T.; Hu, W.; Krause, E. Role of the Hubble scale in the weak lensing versus CMB tension. Phys. Rev. D 2023, 107, 83532. [Google Scholar] [CrossRef]
- Schöneberg, N.; Verde, L.; Gil-Marín, H.; Brieden, S. BAO+BBN revisited—Growing the Hubble tension with a 0.7 km/s/Mpc constraint. JCAP 2022, 11, 39. [Google Scholar] [CrossRef]
- Jusufi, K.; Sheykhi, A. Entropic corrections to Friedmann equations and bouncing universe due to the zero-point length. Phys. Lett. B 2023, 836, 137621. [Google Scholar] [CrossRef]
- Jiang, J.Q.; Ye, G.; Piao, Y.S. Return of Harrison-Zeldovich spectrum in light of recent cosmological tensions. arXiv 2022, arXiv:2210.06125. [Google Scholar]
- Zhou, Z.; Mu, Y.; Liu, G.; Xu, L.; Lu, J. Equality scale-based and sound horizon-based analysis of the Hubble tension. Phys. Rev. D 2023, 107, 63536. [Google Scholar] [CrossRef]
- Zhao, Z.W.; Wang, L.F.; Zhang, J.G.; Zhang, J.F.; Zhang, X. Probing the interaction between dark energy and dark matter with future fast radio burst observations. arxiv 2022, arXiv:2210.07162. [Google Scholar] [CrossRef]
- Yang, W.; Giarè, W.; Pan, S.; Di Valentino, E.; Melchiorri, A.; Silk, J. Revealing the effects of curvature on the cosmological models. Phys. Rev. D 2023, 107, 63509. [Google Scholar] [CrossRef]
- Cardona, W.; Sabogal, M.A. Holographic energy density, dark energy sound speed, and tensions in cosmological parameters: H0 and S8. JCAP 2023, 2, 45. [Google Scholar] [CrossRef]
- Herold, L.; Ferreira, E.G.M. Resolving the Hubble tension with Early Dark Energy. arXiv 2022, arXiv:2210.16296. [Google Scholar] [CrossRef]
- Holm, E.B.; Herold, L.; Hannestad, S.; Nygaard, A.; Tram, T. Decaying dark matter with profile likelihoods. Phys. Rev. D 2023, 107, L021303. [Google Scholar] [CrossRef]
- Akarsu, O.; Kumar, S.; Özülker, E.; Vazquez, J.A.; Yadav, A. Relaxing cosmological tensions with a sign switching cosmological constant: Improved results with Planck, BAO and Pantheon data. arXiv 2022, arXiv:2211.05742. [Google Scholar] [CrossRef]
- Cai, T.; Ding, Q.; Wang, Y. Reconciling cosmic dipolar tensions with a gigaparsec void. arXiv 2022, arXiv:2211.06857. [Google Scholar]
- Pan, S.; Yang, W.; Di Valentino, E.; Mota, D.F.; Silk, J. IWDM: The fate of an interacting non-cold dark matter—Vacuum scenario. arXiv 2022, arXiv:2211.11047. [Google Scholar] [CrossRef]
- Gangopadhyay, M.R.; Pacif, S.K.J.; Sami, M.; Sharma, M.K. Generic Modification of Gravity, Late Time Acceleration and Hubble Tension. Universe 2023, 9, 83. [Google Scholar] [CrossRef]
- Kuzmichev, V.E.; Kuzmichev, V.V. The Hubble tension from the standpoint of quantum cosmology. arXiv 2022, arXiv:2211.16394. [Google Scholar]
- Schiavone, T.; Montani, G.; Bombacigno, F. f(R) gravity in the Jordan frame as a paradigm for the Hubble tension. Mon. Not. R. Astron. Soc. 2023, 522, L72–L77. [Google Scholar] [CrossRef]
- Bernardo, R.C.; Lee, Y.R. Hubble constant by natural selection: Evolution chips in the Hubble tension. arXiv 2022, arXiv:2212.02203. [Google Scholar] [CrossRef]
- Bansal, S.; Barron, J.; Curtin, D.; Tsai, Y. Precision Cosmological Constraints on Atomic Dark Matter. arXiv 2022, arXiv:2212.02487. [Google Scholar]
- Lee, N.; Ali-Haïmoud, Y.; Schöneberg, N.; Poulin, V. What It Takes to Solve the Hubble Tension through Modifications of Cosmological Recombination. Phys. Rev. Lett. 2023, 130, 161003. [Google Scholar] [CrossRef] [PubMed]
- Di Valentino, E.; Nilsson, N.A.; Park, M.I. A new test of dynamical dark energy models and cosmic tensions in Hořava gravity. Mon. Not. R. Astron. Soc. 2023, 519, 5043–5058. [Google Scholar] [CrossRef]
- Lin, M.X.; McDonough, E.; Hill, J.C.; Hu, W. Dark matter trigger for early dark energy coincidence. Phys. Rev. D 2023, 107, 103523. [Google Scholar] [CrossRef]
- Haridasu, B.S.; Khoraminezhad, H.; Viel, M. Scrutinizing Early Dark Energy models through CMB lensing. arXiv 2022, arXiv:2212.09136. [Google Scholar]
- Brinckmann, T.; Chang, J.H.; Du, P.; LoVerde, M. Confronting interacting dark radiation scenarios with cosmological data. Phys. Rev. D 2023, 107, 123517. [Google Scholar] [CrossRef]
- Gao, L.Y.; Xue, S.S.; Zhang, X. Dark energy and matter interacting scenario can relieve H0 and S8 tensions. arXiv 2022, arXiv:2212.13146. [Google Scholar]
- de Jesus, A.S.; Pinto-Neto, N.; Queiroz, F.S.; Silk, J.; da Silva, D.R. The hubble rate trouble: An effective field theory of dark matter. Eur. Phys. J. C 2023, 83, 203. [Google Scholar] [CrossRef]
- Perivolaropoulos, L.; Skara, F. On the homogeneity of SnIa absolute magnitude in the Pantheon+ sample. Mon. Not. R. Astron. Soc. 2023, 520, 5110–5125. [Google Scholar] [CrossRef]
- Bouchè, F.; Capozziello, S.; Salzano, V. Addressing Cosmological Tensions by Non-Local Gravity. Universe 2023, 9, 27. [Google Scholar] [CrossRef]
- Brissenden, L.; Dimopoulos, K.; Sánchez López, S. Non-oscillating Early Dark Energy and Quintessence from Alpha-Attractors. arXiv 2023, arXiv:2301.03572. [Google Scholar]
- Dahmani, S.; Bouali, A.; Bojaddaini, I.E.; Errahmani, A.; Ouali, T. Smoothing the H0 tension with a dynamical dark energy model. arXiv 2023, arXiv:2301.04200. [Google Scholar] [CrossRef]
- Khodadi, M.; Schreck, M. Hubble tension as a guide for refining the early Universe: Cosmologies with explicit local Lorentz and diffeomorphism violation. Phys. Dark Univ. 2023, 39, 101170. [Google Scholar] [CrossRef]
- Bernui, A.; Di Valentino, E.; Giarè, W.; Kumar, S.; Nunes, R.C. Exploring the H0 tension and the evidence for dark sector interactions from 2D BAO measurements. Phys. Rev. D 2023, 107, 103531. [Google Scholar] [CrossRef]
- Thakur, R.K.; Gupta, S.; Nigam, R.; Thiruvikraman, P. Investigating the Hubble Tension Through Hubble Parameter Data. Res. Astron. Astrophys. 2023, 23, 65017. [Google Scholar] [CrossRef]
- Mandal, S.; Sokoliuk, O.; Mishra, S.S.; Sahoo, P.K. H0 tension in torsion-based modified gravity. Nucl. Phys. B 2023, 993, 116285. [Google Scholar] [CrossRef]
- Jin, S.J.; Xing, S.S.; Shao, Y.; Zhang, J.F.; Zhang, X. Joint constraints on cosmological parameters using future multi-band gravitational wave standard siren observations*. Chin. Phys. C 2023, 47, 65104. [Google Scholar] [CrossRef]
- Kumar, S.; Nunes, R.C.; Pan, S.; Yadav, P. New late-time constraints on f(R) gravity. Phys. Dark Univ. 2023, 42, 101281. [Google Scholar] [CrossRef]
- Tiwari, Y.; Ghosh, B.; Jain, R.K. Horndeski Helping Hubble: Towards a possible solution to the Hubble tension with Horndeski gravity. arXiv 2023, arXiv:2301.09382. [Google Scholar]
- Dainotti, M.; De Simone, B.; Montani, G.; Schiavone, T.; Lambiase, G. The Hubble constant tension: Current status and future perspectives through new cosmological probes. In Proceedings of the CORFU2022: 22th Hellenic School and Workshops on Elementary Particle Physics and Gravity, Corfu, Greece, 28 August–1 October 2022. [Google Scholar]
- Bassi, A.; Adil, S.A.; Rajvanshi, M.P.; Sen, A.A. Cosmological evolution in bimetric gravity: Observational constraints and LSS signatures. Eur. Phys. J. C 2023, 83, 525. [Google Scholar] [CrossRef]
- Ben-Dayan, I.; Kumar, U. Emergent Unparticles Dark Energy can restore cosmological concordance. arXiv 2023, arXiv:2302.00067. [Google Scholar]
- de Cruz Perez, J.; Sola Peracaula, J.; Singh, C.P. Running vacuum in Brans-Dicke theory: A possible cure for the σ8 and H0 tensions. arXiv 2023, arXiv:2302.04807. [Google Scholar]
- Ballardini, M.; Ferrari, A.G.; Finelli, F. Phantom scalar-tensor models and cosmological tensions. JCAP 2023, 4, 29. [Google Scholar] [CrossRef]
- Giani, L.; Piattella, O.F. Induced non-local cosmology. Phys. Dark Univ. 2023, 40, 101219. [Google Scholar] [CrossRef]
- Rebouças, J.A.; Gordon, J.; de Souza, D.H.F.; Zhong, K.; Miranda, V.; Rosenfeld, R.; Eifler, T.; Krause, E. Early dark energy constraints with late-time expansion marginalization. arXiv 2023, arXiv:2302.07333. [Google Scholar]
- Cruz, J.S.; Hannestad, S.; Holm, E.B.; Niedermann, F.; Sloth, M.S.; Tram, T. Profiling Cold New Early Dark Energy. arXiv 2023, arXiv:2302.07934. [Google Scholar] [CrossRef]
- Carrillo González, M.; Liang, Q.; Sakstein, J.; Trodden, M. Neutrino-Assisted Early Dark Energy is a Natural Resolution of the Hubble Tension. arXiv 2023, arXiv:2302.09091. [Google Scholar]
- Goldstein, S.; Hill, J.C.; Iršič, V.; Sherwin, B.D. Canonical Hubble-Tension-Resolving Early Dark Energy Cosmologies are Inconsistent with the Lyman-α Forest. arXiv 2023, arXiv:2303.00746. [Google Scholar]
- Yao, Y.H.; Wang, J.C.; Meng, X.H. Observational constraints on non-cold dark matter and phenomenological emergent dark energy. arXiv 2023, arXiv:2303.00961. [Google Scholar]
- Borges, H.A.; Pigozzo, C.; Hepp, P.; Baraúna, L.O.; Benetti, M. Testing the growth rate in homogeneous and inhomogeneous interacting vacuum models. JCAP 2023, 6, 9. [Google Scholar] [CrossRef]
- Das, A.; Ghosh, S. The magnificent ACT of flavor-specific neutrino self-interaction. arXiv 2023, arXiv:2303.08843. [Google Scholar]
- Staicova, D. Model selection results from different BAO datasets—DE models and ΩKCDM. arXiv 2023, arXiv:2303.11271. [Google Scholar]
- Jiang, J.Q.; Ye, G.; Piao, Y.S. Impact of the Hubble tension on the r-ns contour. arXiv 2023, arXiv:2303.12345. [Google Scholar]
- Högås, M.; Mörtsell, E. Impact of symmetron screening on the Hubble tension: New constraints using cosmic distance ladder data. Phys. Rev. D 2023, 108, 24007. [Google Scholar] [CrossRef]
- Jusufi, K.; Sheykhi, A.; Capozziello, S. Apparent dark matter as a non-local manifestation of emergent gravity. Phys. Dark Univ. 2023, 42, 101270. [Google Scholar] [CrossRef]
- Hill, J.C.; Bolliet, B. Did the Universe Reheat After Recombination? arXiv 2023, arXiv:2304.03750. [Google Scholar]
- Nojiri, S.; Odintsov, S.D.; Sáez-Chillón Gómez, D. Unifying inflation with early and late dark energy in Einstein–Gauss–Bonnet gravity. Phys. Dark Univ. 2023, 41, 101238. [Google Scholar] [CrossRef]
- Sola Peracaula, J.; Gomez-Valent, A.; de Cruz Perez, J.; Moreno-Pulido, C. Running Vacuum in the Universe: Phenomenological Status in Light of the Latest Observations, and Its Impact on the σ8 and H0 Tensions. Universe 2023, 9, 262. [Google Scholar] [CrossRef]
- Sakr, Z. Extensions to ΛCDM at Intermediate Redshifts to Solve the Tensions? In Proceedings of the CORFU2022: 22th Hellenic School and Workshops on Elementary Particle Physics and Gravity, Corfu, Greece, 28 August–1 October 2022. [Google Scholar]
- Sakr, Z. One matter density discrepancy to alleviate them all or further trouble for ΛCDM model. arXiv 2023, arXiv:2305.02846. [Google Scholar]
- Cruz, J.S.; Niedermann, F.; Sloth, M.S. Cold New Early Dark Energy pulls the trigger on the H0 and S8 tensions: A simultaneous solution to both tensions without new ingredients. arXiv 2023, arXiv:2305.08895. [Google Scholar]
- Perivolaropoulos, L. On the isotropy of SnIa absolute magnitudes in the Pantheon+ and SH0ES samples. arXiv 2023, arXiv:2305.12819. [Google Scholar]
- Allali, I.J.; Rompineve, F.; Hertzberg, M.P. Dark Sectors with Mass Thresholds Face Cosmological Datasets. arXiv 2023, arXiv:2305.14166. [Google Scholar] [CrossRef]
- Dialektopoulos, K.F.; Mukherjee, P.; Levi Said, J.; Mifsud, J. Neural network reconstruction of scalar-tensor cosmology. arXiv 2023, arXiv:2305.15500. [Google Scholar]
- Dialektopoulos, K.F.; Mukherjee, P.; Levi Said, J.; Mifsud, J. Neural network reconstruction of cosmology using the Pantheon Compilation. arXiv 2023, arXiv:2305.15499. [Google Scholar]
- Gómez-Valent, A.; Mavromatos, N.E.; Solà Peracaula, J. Stringy Running Vacuum Model and current Tensions in Cosmology. arXiv 2023, arXiv:2305.15774. [Google Scholar]
- Bisnovatyi-Kogan, G.S.; Nikishin, A.M. Eliminating the Hubble Tension in the Presence of the Interconnection between Dark Energy and Matter in the Modern Universe. Astron. Rep. 2023, 67, 115–124. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K.; Sharov, G.S. Early dark energy with power-law F(R) gravity. Phys. Lett. B 2023, 843, 137988. [Google Scholar] [CrossRef]
- Ye, G.; Jiang, J.Q.; Piao, Y.S. Shape of CMB lensing in the early dark energy cosmology. arXiv 2023, arXiv:2305.18873. [Google Scholar]
- Jin, S.J.; Zhang, Y.Z.; Song, J.Y.; Zhang, J.F.; Zhang, X. The Taiji-TianQin-LISA network: Precisely measuring the Hubble constant using both bright and dark sirens. arXiv 2023, arXiv:2305.19714. [Google Scholar]
- Takahashi, T.; Toda, Y. Impact of big bang nucleosynthesis on the H0 tension. arXiv 2023, arXiv:2306.00454. [Google Scholar]
- Li, Y.H.; Zhang, X. IDECAMB: An implementation of interacting dark energy cosmology in CAMB. arXiv 2023, arXiv:2306.01593. [Google Scholar]
- Buen-Abad, M.A.; Chacko, Z.; Kilic, C.; Marques-Tavares, G.; Youn, T. Stepped Partially Acoustic Dark Matter: Likelihood Analysis and Cosmological Tensions. arXiv 2023, arXiv:2306.01844. [Google Scholar]
- Flores, M.M.; Kouvaris, C.; Kusenko, A. Defrosting and Blast Freezing Dark Matter. arXiv 2023, arXiv:2306.04056. [Google Scholar]
- Ruchika; Rathore, H.; Roy Choudhury, S.; Rentala, V. A gravitational constant transition within cepheids as supernovae calibrators can solve the Hubble tension. arXiv 2023, arXiv:2306.05450. [Google Scholar]
- Greene, K.; Cyr-Racine, F.Y. Thomson scattering: One rate to rule them all. arXiv 2023, arXiv:2306.06165. [Google Scholar]
- Adil, S.A.; Akarsu, O.; Di Valentino, E.; Nunes, R.C.; Ozulker, E.; Sen, A.A.; Specogna, E. Omnipotent dark energy: A phenomenological answer to the Hubble tension. arXiv 2023, arXiv:2306.08046. [Google Scholar]
- Montani, G.; De Angelis, M.; Bombacigno, F.; Carlevaro, N. Metric f(R) gravity with dynamical dark energy as a paradigm for the Hubble Tension. arXiv 2023, arXiv:2306.11101. [Google Scholar]
- Schöneberg, N.; Franco Abellán, G.; Simon, T.; Bartlett, A.; Patel, Y.; Smith, T.L. The weak, the strong and the ugly—A comparative analysis of interacting stepped dark radiation. arXiv 2023, arXiv:2306.12469. [Google Scholar]
- Farhang, M.; Khosravi, N. Reconstruction of A Scale-Dependent Gravitational Phase Transition. arXiv 2023, arXiv:2306.14014. [Google Scholar]
- Escamilla-Rivera, C.; de Albornoz-Caratozzolo, J.M.; Nájera, S. Fab-Four cosmography to tackle the Hubble tension. arXiv 2023, arXiv:2306.14855. [Google Scholar] [CrossRef]
- Yadav, V. Measuring Hubble constant in an anisotropic extension of ΛCDM model. arXiv 2023, arXiv:2306.16135. [Google Scholar]
- Lombriser, L. Cosmology in Minkowski space. Class. Quant. Grav. 2023, 40, 155005. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, C.; Wang, Z.; Cui, M.; Tsai, Y.L.S.; Yuan, Q.; Fan, Y.Z. Primordial magnetic field as a common solution of nanohertz gravitational waves and Hubble tension. arXiv 2023, arXiv:2306.17124. [Google Scholar]
- Singh, N.K.; Kashyap, G. Unimodular Theory of Gravity in Light of the Latest Cosmological Data. arXiv 2023, arXiv:2306.17754. [Google Scholar]
- Nygaard, A.; Holm, E.B.; Tram, T.; Hannestad, S. Decaying Dark Matter and the Hubble Tension. arXiv 2023, arXiv:2307.00418. [Google Scholar]
- Sarkar, A.; Ghosh, B. Early Dark Energy Motivated Quintessential α-Attractor Inflaton Potential. arXiv 2023, arXiv:2307.00603. [Google Scholar]
- Sengupta, R.; Paul, P.; Paul, B.C.; Kalam, M. Can extended Chaplygin gas source a Hubble tension resolved emergent universe? arXiv 2023, arXiv:2307.02602. [Google Scholar]
- Ferrari, A.G.; Ballardini, M.; Finelli, F.; Paoletti, D.; Mauri, N. Cosmological effects of the Galileon term in Scalar-Tensor Theories. arXiv 2023, arXiv:2307.02987. [Google Scholar]
- Patil, T.; Ruchika; Panda, S. Coupled Quintessence scalar field model in light of observational datasets. arXiv 2023, arXiv:2307.03740. [Google Scholar]
- Benaoum, H.B.; García, L.A.; Castañeda, L. Early dark energy induced by non-linear electrodynamics. arXiv 2023, arXiv:2307.05917. [Google Scholar]
- Frion, E.; Camarena, D.; Giani, L.; Miranda, T.; Bertacca, D.; Marra, V.; Piattella, O.F. Bayesian analysis of Unified Dark Matter models with fast transition: Can they alleviate the H0 tension? arXiv 2023, arXiv:2307.06320. [Google Scholar]
- Brax, P.; Ouazzani, A. Two-field Screening and its Cosmological Dynamics. arXiv 2023, arXiv:2307.06781. [Google Scholar]
- Liu, G.; Zhou, Z.; Mu, Y.; Xu, L. Alleviating Cosmological Tensions with a Coupled Scalar Fields Model. arXiv 2023, arXiv:2307.07228. [Google Scholar]
- D’Agostino, R.; Nunes, R.C. Cosmographic view on the H0 and σ8 tensions. Phys. Rev. D 2023, 108, 23523. [Google Scholar] [CrossRef]
- Lu, Z.; Imtiaz, B.; Zhang, D.; Cai, Y.F. Testing the coupling of dark radiations in light of the Hubble tension. arXiv 2023, arXiv:2307.09863. [Google Scholar]
- Akarsu, O.; Di Valentino, E.; Kumar, S.; Nunes, R.C.; Vazquez, J.A.; Yadav, A. ΛsCDM model: A promising scenario for alleviation of cosmological tensions. arXiv 2023, arXiv:2307.10899. [Google Scholar]
- Bousder, M.; Riadsolh, A.; Fatimy, A.E.; Belkacemi, M.E.; Ez-Zahraouy, H. Implications of the NANOGrav results for primordial black holes and Hubble tension. arXiv 2023, arXiv:2307.10940. [Google Scholar]
- Kable, J.A.; Benevento, G.; Addison, G.E.; Bennett, C.L. Cosmological Tensions and the Transitional Planck Mass Model. arXiv 2023, arXiv:2307.12174. [Google Scholar]
- Adil, S.A.; Mukhopadhyay, U.; Sen, A.A.; Vagnozzi, S. Dark energy in light of the early JWST observations: Case for a negative cosmological constant? arXiv 2023, arXiv:2307.12763. [Google Scholar]
- Das, S.; Nasiri, A.; Yazdi, Y.K. Aspects of Everpresent Λ (II): Cosmological Tests of Current Models. arXiv 2023, arXiv:2307.13743. [Google Scholar]
- Kalita, S.; Rabha, C. Possible combinations of early and late time cosmologies through BAO scales. Eur. Phys. J. C 2023, 83, 671. [Google Scholar] [CrossRef]
- Peng, Z.Y.; Piao, Y.S. Testing the ns—H0 scaling relation with Planck-independent CMB data. arXiv 2023, arXiv:2308.01012. [Google Scholar]
- Basilakos, S.; Lymperis, A.; Petronikolou, M.; Saridakis, E.N. Alleviating both H0 and σ8 tensions in Tsallis cosmology. arXiv 2023, arXiv:2308.01200. [Google Scholar]
- Banerjee, T.; Mandal, G.; Biswas, A.; Biswas, S.K. Gravitationally induced matter creation and cosmological consequences. arXiv 2023, arXiv:2308.00804. [Google Scholar]
- M, N.K.; Nelleri, S.; Poonthottathil, N. Testing the dynamical stability and validity of generalized second law within the phantom dynamical dark energy model. arXiv 2023, arXiv:2308.03084. [Google Scholar]
- Santana, Z.C.; Holanda, R.F.L.; Silva, R. Non-Parametric Analysis for the Dark Matter Density Evolution. arXiv 2023, arXiv:2308.05165. [Google Scholar]
- Wei, J.J.; Melia, F. Investigating Cosmological Models and the Hubble Tension using Localized Fast Radio Bursts. arXiv 2023, arXiv:2308.05918. [Google Scholar]
- Hoerning, G.A.; Landim, R.G.; Ponte, L.O.; Rolim, R.P.; Abdalla, F.B.; Abdalla, E. Constraints on interacting dark energy revisited: Alleviating the Hubble tension. arXiv 2023, arXiv:2308.05807. [Google Scholar]
- Harada, J. Dark energy in conformal Killing gravity. arXiv 2023, arXiv:2308.07634. [Google Scholar]
- Bernal, J.L.; Verde, L.; Riess, A.G. The trouble with H0. JCAP 2016, 10, 19. [Google Scholar] [CrossRef]
- Addison, G.E.; Watts, D.J.; Bennett, C.L.; Halpern, M.; Hinshaw, G.; Weiland, J.L. Elucidating ΛCDM: Impact of Baryon Acoustic Oscillation Measurements on the Hubble Constant Discrepancy. Astrophys. J. 2018, 853, 119. [Google Scholar] [CrossRef]
- Lemos, P.; Lee, E.; Efstathiou, G.; Gratton, S. Model independent H(z) reconstruction using the cosmic inverse distance ladder. Mon. Not. R. Astron. Soc. 2019, 483, 4803–4810. [Google Scholar] [CrossRef]
- Aylor, K.; Joy, M.; Knox, L.; Millea, M.; Raghunathan, S.; Wu, W.L.K. Sounds Discordant: Classical Distance Ladder & ΛCDM -based Determinations of the Cosmological Sound Horizon. Astrophys. J. 2019, 874, 4. [Google Scholar]
- Schöneberg, N.; Lesgourgues, J.; Hooper, D.C. The BAO+BBN take on the Hubble tension. JCAP 2019, 10, 29. [Google Scholar] [CrossRef]
- Knox, L.; Millea, M. Hubble constant hunter’s guide. Phys. Rev. D 2020, 101, 43533. [Google Scholar] [CrossRef]
- Arendse, N.; Wojtak, R.J.; Agnello, A.; Chen, G.C.F.; Fassnacht, C.D.; Sluse, D.; Hilbert, S.; Millon, M.; Bonvin, V.; Wong, K.C.; et al. Cosmic dissonance: Are new physics or systematics behind a short sound horizon? Astron. Astrophys. 2020, 639, A57. [Google Scholar] [CrossRef]
- Efstathiou, G. To H0 or not to H0? Mon. Not. R. Astron. Soc. 2021, 505, 3866–3872. [Google Scholar] [CrossRef]
- Cai, R.G.; Guo, Z.K.; Wang, S.J.; Yu, W.W.; Zhou, Y. No-go guide for the Hubble tension: Late-time solutions. Phys. Rev. D 2022, 105, L021301. [Google Scholar] [CrossRef]
- Keeley, R.E.; Shafieloo, A. Ruling Out New Physics at Low Redshift as a solution to the H0 Tension. arXiv 2022, arXiv:2206.08440. [Google Scholar]
- Di Valentino, E.; Bridle, S. Exploring the Tension between Current Cosmic Microwave Background and Cosmic Shear Data. Symmetry 2018, 10, 585. [Google Scholar] [CrossRef]
- Di Valentino, E.; Anchordoqui, L.A.; Akarsu, O.; Ali-Haimoud, Y.; Amendola, L.; Arendse, N.; Asgari, M.; Ballardini, M.; Basilakos, S.; Battistelli, E.; et al. Cosmology Intertwined III: fσ8 and S8. Astropart. Phys. 2021, 131, 102604. [Google Scholar] [CrossRef]
- Nunes, R.C.; Vagnozzi, S. Arbitrating the S8 discrepancy with growth rate measurements from redshift-space distortions. Mon. Not. R. Astron. Soc. 2021, 505, 5427–5437. [Google Scholar] [CrossRef]
- Huterer, D. Growth of cosmic structure. Astron. Astrophys. Rev. 2023, 31, 2. [Google Scholar] [CrossRef]
- Sakr, Z. Untying the Growth Index to Relieve the σ8 Discomfort. Universe 2023, 9, 366. [Google Scholar] [CrossRef]
- Jaffe, A.H. H0 and odds on cosmology. Astrophys. J. 1996, 471, 24. [Google Scholar] [CrossRef]
- Ostriker, J.P.; Steinhardt, P.J. The Observational case for a low density universe with a nonzero cosmological constant. Nature 1995, 377, 600–602. [Google Scholar] [CrossRef]
- Dunlop, J.; Peacock, J.; Spinrad, H.; Dey, A.; Jimenez, R.; Stern, D.; Windhorst, R. A 3.5-Gyr-old galaxy at redshift 1.55. Nature 1996, 381, 581. [Google Scholar] [CrossRef]
- Vagnozzi, S.; Pacucci, F.; Loeb, A. Implications for the Hubble tension from the ages of the oldest astrophysical objects. JHEAp 2022, 36, 27–35. [Google Scholar] [CrossRef]
- Pacucci, F.; Baldassare, V.; Cappelluti, N.; Fan, X.H.; Ferrara, A.; Haiman, Z.; Natarajan, P.; Ozel, F.; Schneider, R.; Tremblay, G.R.; et al. Detecting the Birth of Supermassive Black Holes Formed from Heavy Seeds. Bull. Am. Astron. Soc. 2019, 51, 117. [Google Scholar]
- Valcin, D.; Jimenez, R.; Verde, L.; Bernal, J.L.; Wandelt, B.D. The age of the Universe with globular clusters: Reducing systematic uncertainties. JCAP 2021, 8, 17. [Google Scholar] [CrossRef]
- Borghi, N.; Moresco, M.; Cimatti, A.; Huchet, A.; Quai, S.; Pozzetti, L. Toward a Better Understanding of Cosmic Chronometers: Stellar Population Properties of Passive Galaxies at Intermediate Redshift. Astrophys. J. 2022, 927, 164. [Google Scholar] [CrossRef]
- Borghi, N.; Moresco, M.; Cimatti, A. Toward a Better Understanding of Cosmic Chronometers: A New Measurement of H(z) at z ∼ 0.7. Astrophys. J. Lett. 2022, 928, L4. [Google Scholar] [CrossRef]
- Pacucci, F.; Loeb, A. The search for the farthest quasar: Consequences for black hole growth and seed models. Mon. Not. R. Astron. Soc. 2021, 509, 1885–1891. [Google Scholar] [CrossRef]
- Pacucci, F.; Natarajan, P.; Volonteri, M.; Cappelluti, N.; Urry, C.M. Conditions for Optimal Growth of Black Hole Seeds. Astrophys. J. Lett. 2017, 850, L42. [Google Scholar] [CrossRef]
- Jimenez, R.; Cimatti, A.; Verde, L.; Moresco, M.; Wandelt, B. The local and distant Universe: Stellar ages and H0. JCAP 2019, 3, 43. [Google Scholar] [CrossRef]
- Vagnozzi, S.; Loeb, A.; Moresco, M. Eppur è piatto? The Cosmic Chronometers Take on Spatial Curvature and Cosmic Concordance. Astrophys. J. 2021, 908, 84. [Google Scholar] [CrossRef]
- Valcin, D.; Bernal, J.L.; Jimenez, R.; Verde, L.; Wandelt, B.D. Inferring the Age of the Universe with Globular Clusters. JCAP 2020, 12, 2. [Google Scholar] [CrossRef]
- Wei, J.J.; Melia, F. Exploring the Hubble Tension and Spatial Curvature from the Ages of Old Astrophysical Objects. Astrophys. J. 2022, 928, 165. [Google Scholar] [CrossRef]
- Costa, A.A.; Ren, Z.; Yin, Z. A bias using the ages of the oldest astrophysical objects to address the Hubble tension. arXiv 2023, arXiv:2306.01234. [Google Scholar]
- Bernal, J.L.; Verde, L.; Jimenez, R.; Kamionkowski, M.; Valcin, D.; Wandelt, B.D. The trouble beyond H0 and the new cosmic triangles. Phys. Rev. D 2021, 103, 103533. [Google Scholar] [CrossRef]
- Moresco, M.; Amati, L.; Amendola, L.; Birrer, S.; Blakeslee, J.P.; Cantiello, M.; Cimatti, A.; Darling, J.; Della Valle, M.; Fishbach, M.; et al. Unveiling the Universe with emerging cosmological probes. Living Rev. Rel. 2022, 25, 6. [Google Scholar] [CrossRef]
- Boylan-Kolchin, M.; Weisz, D.R. Uncertain times: The redshift–time relation from cosmology and stars. Mon. Not. R. Astron. Soc. 2021, 505, 2764–2783. [Google Scholar] [CrossRef]
- Cimatti, A.; Moresco, M. Revisiting oldest stars as cosmological probes: New constraints on the Hubble constant. arXiv 2023, arXiv:2302.07899. [Google Scholar] [CrossRef]
- Jimenez, R.; Moresco, M.; Verde, L.; Wandelt, B.D. Cosmic Chronometers with Photometry: A new path to H(z). arXiv 2023, arXiv:2306.11425. [Google Scholar]
- Capozziello, S.; Sarracino, G.; Spallicci, A.D.A.M. Questioning the H0 tension via the look-back time. Phys. Dark Univ. 2023, 40, 101201. [Google Scholar] [CrossRef]
- Jedamzik, K.; Pogosian, L.; Zhao, G.B. Why reducing the cosmic sound horizon alone can not fully resolve the Hubble tension. Commun. in Phys. 2021, 4, 123. [Google Scholar] [CrossRef]
- Jimenez, R.; Loeb, A. Constraining cosmological parameters based on relative galaxy ages. Astrophys. J. 2002, 573, 37–42. [Google Scholar] [CrossRef]
- Thomas, D.; Maraston, C.; Schawinski, K.; Sarzi, M.; Silk, J. Environment and self-regulation in galaxy formation. Mon. Not. R. Astron. Soc. 2010, 404, 1775. [Google Scholar] [CrossRef]
- Yu, H.; Ratra, B.; Wang, F.Y. Hubble Parameter and Baryon Acoustic Oscillation Measurement Constraints on the Hubble Constant, the Deviation from the Spatially Flat ΛCDM Model, the Deceleration–Acceleration Transition Redshift, and Spatial Curvature. Astrophys. J. 2018, 856, 3. [Google Scholar] [CrossRef]
- Gómez-Valent, A.; Amendola, L. H0 from cosmic chronometers and Type Ia supernovae, with Gaussian Processes and the novel Weighted Polynomial Regression method. JCAP 2018, 4, 51. [Google Scholar] [CrossRef]
- Moresco, M.; Jimenez, R.; Verde, L.; Pozzetti, L.; Cimatti, A.; Citro, A. Setting the Stage for Cosmic Chronometers. I. Assessing the Impact of Young Stellar Populations on Hubble Parameter Measurements. Astrophys. J. 2018, 868, 84. [Google Scholar] [CrossRef]
- Moresco, M.; Jimenez, R.; Verde, L.; Cimatti, A.; Pozzetti, L. Setting the Stage for Cosmic Chronometers. II. Impact of Stellar Population Synthesis Models Systematics and Full Covariance Matrix. Astrophys. J. 2020, 898, 82. [Google Scholar] [CrossRef]
- Simon, J.; Verde, L.; Jimenez, R. Constraints on the redshift dependence of the dark energy potential. Phys. Rev. D 2005, 71, 123001. [Google Scholar] [CrossRef]
- Kjerrgren, A.A.; Mortsell, E. On the use of galaxies as clocks and the universal expansion. Mon. Not. R. Astron. Soc. 2022, 518, 585–591. [Google Scholar]
- Krishnan, C.; Colgáin, E.O.; Sheikh-Jabbari, M.M.; Yang, T. Running Hubble Tension and a H0 Diagnostic. Phys. Rev. D 2021, 103, 103509. [Google Scholar] [CrossRef]
- Birrer, S.; Amara, A.; Refregier, A. The mass-sheet degeneracy and time-delay cosmography: Analysis of the strong lens RXJ1131-1231. JCAP 2016, 8, 20. [Google Scholar] [CrossRef]
- Kochanek, C.S. Overconstrained gravitational lens models and the Hubble constant. Mon. Not. R. Astron. Soc. 2020, 493, 1725–1735. [Google Scholar] [CrossRef]
- Blum, K.; Castorina, E.; Simonović, M. Could Quasar Lensing Time Delays Hint to a Core Component in Halos, Instead of H0 Tension? Astrophys. J. Lett. 2020, 892, L27. [Google Scholar] [CrossRef]
- Birrer, S.; Shajib, A.J.; Galan, A.; Millon, M.; Treu, T.; Agnello, A.; Auger, M.; Chen, G.C.F.; Christensen, L.; Collett, T.; et al. TDCOSMO—IV. Hierarchical time-delay cosmography – joint inference of the Hubble constant and galaxy density profiles. Astron. Astrophys. 2020, 643, A165. [Google Scholar] [CrossRef]
- Krishnan, C.; Colgáin, E.O.; Ruchika; Sen, A.A.; Sheikh-Jabbari, M.M.; Yang, T. Is there an early Universe solution to Hubble tension? Phys. Rev. D 2020, 102, 103525. [Google Scholar] [CrossRef]
- Reid, M.J.; Pesce, D.W.; Riess, A.G. An Improved Distance to NGC 4258 and its Implications for the Hubble Constant. Astrophys. J. Lett. 2019, 886, L27. [Google Scholar] [CrossRef]
- Pesce, D.W.; Braatz, J.A.; Reid, M.J.; Condon, J.J.; Gao, F.; Henkel, C.; Kuo, C.Y.; Lo, K.Y.; Zhao, W. The Megamaser Cosmology Project. XI. A geometric distance to CGCG 074-064. Astrophys. J. 2020, 890, 118. [Google Scholar] [CrossRef]
- Dainotti, M.G.; De Simone, B.; Schiavone, T.; Montani, G.; Rinaldi, E.; Lambiase, G. On the Hubble constant tension in the SNe Ia Pantheon sample. Astrophys. J. 2021, 912, 150. [Google Scholar] [CrossRef]
- Jia, X.D.; Hu, J.P.; Wang, F.Y. Evidence of a decreasing trend for the Hubble constant. Astron. Astrophys. 2023, 674, A45. [Google Scholar] [CrossRef]
- Huterer, D.; Cooray, A. Uncorrelated estimates of dark energy evolution. Phys. Rev. D 2005, 71, 23506. [Google Scholar] [CrossRef]
- Malekjani, M.; Conville, R.M.; Colgáin, E.O.; Pourojaghi, S.; Sheikh-Jabbari, M.M. Negative Dark Energy Density from High Redshift Pantheon+ Supernovae. arXiv 2023, arXiv:2301.12725. [Google Scholar]
- Horstmann, N.; Pietschke, Y.; Schwarz, D.J. Inference of the cosmic rest-frame from supernovae Ia. Astron. Astrophys. 2022, 668, A34. [Google Scholar] [CrossRef]
- Risaliti, G.; Lusso, E. Cosmological constraints from the Hubble diagram of quasars at high redshifts. Nat. Astron. 2019, 3, 272–277. [Google Scholar] [CrossRef]
- Lusso, E.; Risaliti, G.; Nardini, E.; Bargiacchi, G.; Benetti, M.; Bisogni, S.; Capozziello, S.; Civano, F.; Eggleston, L.; Elvis, M.; et al. Quasars as standard candles III. Validation of a new sample for cosmological studies. Astron. Astrophys. 2020, 642, A150. [Google Scholar] [CrossRef]
- Colgáin, E.O.; Sheikh-Jabbari, M.M.; Solomon, R.; Bargiacchi, G.; Capozziello, S.; Dainotti, M.G.; Stojkovic, D. Revealing intrinsic flat ΛCDM biases with standardizable candles. Phys. Rev. D 2022, 106, L041301. [Google Scholar] [CrossRef]
- Colgáin, E.O.; Sheikh-Jabbari, M.M.; Solomon, R.; Dainotti, M.G.; Stojkovic, D. Putting Flat ΛCDM In The (Redshift) Bin. arXiv 2022, arXiv:2206.11447. [Google Scholar]
- Velten, H.; Gomes, S. Is the Hubble diagram of quasars in tension with concordance cosmology? Phys. Rev. D 2020, 101, 43502. [Google Scholar] [CrossRef]
- Mehrabi, A.; Basilakos, S. Does ΛCDM really be in tension with the Hubble diagram data? Eur. Phys. J. C 2020, 80, 632. [Google Scholar] [CrossRef]
- Bargiacchi, G.; Benetti, M.; Capozziello, S.; Lusso, E.; Risaliti, G.; Signorini, M. Quasar cosmology: Dark energy evolution and spatial curvature. Mon. Not. R. Astron. Soc. 2022, 515, 1795–1806. [Google Scholar] [CrossRef]
- Dainotti, M.G.; Bardiacchi, G.; Lenart, A.L.; Capozziello, S.; Colgain, E.O.; Solomon, R.; Stojkovic, D.; Sheikh-Jabbari, M.M. Quasar Standardization: Overcoming Selection Biases and Redshift Evolution. Astrophys. J. 2022, 931, 106. [Google Scholar] [CrossRef]
- Lenart, A.L.; Bargiacchi, G.; Dainotti, M.G.; Nagataki, S.; Capozziello, S. A Bias-free Cosmological Analysis with Quasars Alleviating H 0 Tension. Astrophys. J. Suppl. 2023, 264, 46. [Google Scholar] [CrossRef]
- Bargiacchi, G.; Dainotti, M.G.; Nagataki, S.; Capozziello, S. Gamma-Ray Bursts, Quasars, Baryonic Acoustic Oscillations, and Supernovae Ia: New statistical insights and cosmological constraints. Mon. Not. R. Astron. Soc. 2023, 521, 3909–3924. [Google Scholar] [CrossRef]
- Dainotti, M.G.; Bargiacchi, G.; Bogdan, M.; Lenart, A.L.; Iwasaki, K.; Capozziello, S.; Zhang, B.; Fraija, N. Reducing the Uncertainty on the Hubble Constant up to 35% with an Improved Statistical Analysis: Different Best-fit Likelihoods for Type Ia Supernovae, Baryon Acoustic Oscillations, Quasars, and Gamma-Ray Bursts. Astrophys. J. 2023, 951, 63. [Google Scholar] [CrossRef]
- Wei, J.J.; Wu, X.F. Gamma-ray burst cosmology: Hubble diagram and star formation history. Int. J. Mod. Phys. D 2016, 26, 1730002. [Google Scholar] [CrossRef]
- Dainotti, M.G.; Nielson, V.; Sarracino, G.; Rinaldi, E.; Nagataki, S.; Capozziello, S.; Gnedin, O.Y.; Bargiacchi, G. Optical and X-ray GRB Fundamental Planes as cosmological distance indicators. Mon. Not. R. Astron. Soc. 2022, 514, 1828–1856. [Google Scholar] [CrossRef]
- Dainotti, M.G.; Sarracino, G.; Capozziello, S. Gamma-ray bursts, supernovae Ia, and baryon acoustic oscillations: A binned cosmological analysis. Publ. Astron. Soc. Jap. 2022, 74, 1095–1113. [Google Scholar] [CrossRef]
- Dainotti, M.G.; Lenart, A.L.; Chraya, A.; Sarracino, G.; Nagataki, S.; Fraija, N.; Capozziello, S.; Bogdan, M. The Gamma-ray Bursts fundamental plane correlation as a cosmological tool. Mon. Not. R. Astron. Soc. 2023, 518, 2201–2240. [Google Scholar] [CrossRef]
- Colgáin, E.O.; Sheikh-Jabbari, M.M.; Solomon, R. High redshift ΛCDM cosmology: To bin or not to bin? Phys. Dark Univ. 2023, 40, 101216. [Google Scholar] [CrossRef]
- Colgáin, E.O.; Pourojaghi, S.; Sheikh-Jabbari, M.M.; Sherwin, D. MCMC Marginalisation Bias and ΛCDM tensions. arXiv 2023, arXiv:2307.16349. [Google Scholar]
- Adil, S.A.; Akarsu, O.; Malekjani, M.; Colgáin, E.O.; Pourojaghi, S.; Sen, A.A.; Sheikh-Jabbari, M.M. S8 increases with effective redshift in ΛCDM cosmology. arXiv 2023, arXiv:2303.06928. [Google Scholar]
- Esposito, M.; Iršič, V.; Costanzi, M.; Borgani, S.; Saro, A.; Viel, M. Weighing cosmic structures with clusters of galaxies and the intergalactic medium. Mon. Not. R. Astron. Soc. 2022, 515, 857–870. [Google Scholar] [CrossRef]
- Vagnozzi, S. Consistency tests of ΛCDM from the early integrated Sachs-Wolfe effect: Implications for early-time new physics and the Hubble tension. Phys. Rev. D 2021, 104, 63524. [Google Scholar] [CrossRef]
- Calabrese, E.; Slosar, A.; Melchiorri, A.; Smoot, G.F.; Zahn, O. Cosmic Microwave Weak lensing data as a test for the dark universe. Phys. Rev. D 2008, 77, 123531. [Google Scholar] [CrossRef]
- Specogna, E.; Di Valentino, E.; Levi Said, J.; Nguyen, N.M. Exploring the Growth Index γL: Insights from Different CMB Dataset Combinations and Approaches. arXiv 2023, arXiv:2305.16865. [Google Scholar]
- Giarè, W.; Mena, O.; Di Valentino, E. On the lensing impact on cosmic relics & tensions. arXiv 2023, arXiv:2307.14204. [Google Scholar]
- Kable, J.A.; Addison, G.E.; Bennett, C.L. Deconstructing the Planck TT Power Spectrum to Constrain Deviations from ΛCDM. Astrophys. J. 2020, 905, 164. [Google Scholar] [CrossRef]
- Ruiz-Granda, M.; Vielva, P. Constraining CMB physical processes using Planck 2018 data. JCAP 2022, 11, 43. [Google Scholar] [CrossRef]
- Kamionkowski, M.; Riess, A.G. The Hubble Tension and Early Dark Energy. arXiv 2022, arXiv:2211.04492. [Google Scholar] [CrossRef]
- Poulin, V.; Smith, T.L.; Karwal, T. The Ups and Downs of Early Dark Energy solutions to the Hubble tension: A review of models, hints and constraints circa 2023. arXiv 2023, arXiv:2302.09032. [Google Scholar]
- Handley, W.; Lemos, P. Quantifying the global parameter tensions between ACT, SPT and Planck. Phys. Rev. D 2021, 103, 63529. [Google Scholar] [CrossRef]
- Giarè, W.; Renzi, F.; Mena, O.; Di Valentino, E.; Melchiorri, A. Is the Harrison-Zel’dovich spectrum coming back? ACT preference for ns ∼ 1 and its discordance with Planck. Mon. Not. R. Astron. Soc. 2023, 521, 2911–2918. [Google Scholar] [CrossRef]
- Zhai, Y.; Giarè, W.; van de Bruck, C.; Di Valentino, E.; Mena, O.; Nunes, R.C. A consistent view of interacting dark energy from multiple CMB probes. JCAP 2023, 7, 32. [Google Scholar] [CrossRef]
- Di Valentino, E.; Gariazzo, S.; Giarè, W.; Mena, O. Weighing neutrinos at the damping tail. arXiv 2023, arXiv:2305.12989. [Google Scholar]
- Giarè, W.; Pan, S.; Di Valentino, E.; Yang, W.; de Haro, J.; Melchiorri, A. Inflationary Potential as seen from Different Angles: Model Compatibility from Multiple CMB Missions. arXiv 2023, arXiv:2305.15378. [Google Scholar]
- Abazajian, K.N.; Adshead, P.; Ahmed, Z.; Allen, S.W.; Alonso, D.; Arnold, K.S.; Baccigalupi, C.; Bartlett, J.G.; Battaglia, N.; Benson, B.A.; et al. CMB-S4 Science Book, First Edition. arXiv 2016, arXiv:1610.02743. [Google Scholar]
- Ade, P.; Aguirre, J.; Ahmed, Z.; Aiola, S.; Ali, A.; Alonso, D.; Alvarez, M.A.; Arnold, K.; Ashton, P.; Austermann, J.; et al. The Simons Observatory: Science goals and forecasts. JCAP 2019, 2, 56. [Google Scholar] [CrossRef]
- Abitbol, M.H.; Adachi, S.; Ade, P.; Aguirre, J.; Ahmed, Z.; Aiola, S.; Ali, A.; Alonso, D.; Alvarez, M.A.; Arnold, K.; et al. The Simons Observatory: Astro2020 Decadal Project Whitepaper. Bull. Am. Astron. Soc. 2019, 51, 147. [Google Scholar]
- Lin, W.; Chen, X.; Mack, K.J. Early Universe Physics Insensitive and Uncalibrated Cosmic Standards: Constraints on Ωm and Implications for the Hubble Tension. Astrophys. J. 2021, 920, 159. [Google Scholar] [CrossRef]
- Lin, W.; Mack, K.J.; Hou, L. Investigating the Hubble Constant Tension—Two Numbers in the Standard Cosmological Model. Astrophys. J. Lett. 2020, 904, L22. [Google Scholar] [CrossRef]
- Smith, T.L.; Poulin, V.; Simon, T. Assessing the robustness of sound horizon-free determinations of the Hubble constant. arXiv 2022, arXiv:2208.12992. [Google Scholar]
- Baxter, E.J.; Sherwin, B.D. Determining the Hubble Constant without the Sound Horizon Scale: Measurements from CMB Lensing. Mon. Not. R. Astron. Soc. 2021, 501, 1823–1835. [Google Scholar] [CrossRef]
- Philcox, O.H.E.; Farren, G.S.; Sherwin, B.D.; Baxter, E.J.; Brout, D.J. Determining the Hubble constant without the sound horizon: A 3.6% constraint on H0 from galaxy surveys, CMB lensing, and supernovae. Phys. Rev. D 2022, 106, 63530. [Google Scholar] [CrossRef]
- Philcox, O.H.E.; Sherwin, B.D.; Farren, G.S.; Baxter, E.J. Determining the Hubble Constant without the Sound Horizon: Measurements from Galaxy Surveys. Phys. Rev. D 2021, 103, 23538. [Google Scholar] [CrossRef]
- Farren, G.S.; Philcox, O.H.E.; Sherwin, B.D. Determining the Hubble constant without the sound horizon: Perspectives with future galaxy surveys. Phys. Rev. D 2022, 105, 63503. [Google Scholar] [CrossRef]
- Ivezić, V.; Kahn, S.M.; Tyson, J.A.; Abel, B.; Acosta, E.; Allsman, R.; Alonso, D.; AlSayyad, Y.; Anderson, S.F.; Andrew, J.; et al. LSST: From Science Drivers to Reference Design and Anticipated Data Products. Astrophys. J. 2019, 873, 111. [Google Scholar] [CrossRef]
- Amendola, L.; Appleby, S.; Bacon, D.; Baker, T.; Baldi, M.; Bartolo, N.; Blanchard, A.; Bonvin, C.; Borgani, S.; Branchini, E.; et al. Cosmology and fundamental physics with the Euclid satellite. Living Rev. Rel. 2013, 16, 6. [Google Scholar] [CrossRef]
- Spergel, D.; Gehrels, N.; Baltay, C.; Bennett, D.; Breckinridge, J.; Donahue, M.; Dressler, A.; Gaudi, B.S.; Greene, T.; Guyon, O.; et al. Wide-Field InfrarRed Survey Telescope-Astrophysics Focused Telescope Assets WFIRST-AFTA 2015 Report. arXiv 2015, arXiv:1503.03757. [Google Scholar]
- Aghamousa, A.; Aguilar, J.; Ahlen, S.; Alam, S.; Allen, L.E.; Allende Prieto, C.; Annis, J.; Bailey, S.; Balland, C.; Ballester, O.; et al. The DESI Experiment Part I: Science, Targeting, and Survey Design. arXiv 2016, arXiv:1611.00036. [Google Scholar]
- Bacon, D.J.; Battye, R.A.; Bull, P.; Camera, S.; Ferreira, P.G.; Harrison, I.; Parkinson, D.; Pourtsidou, A.; Santos, M.G.; Wolz, L.; et al. Cosmology with Phase 1 of the Square Kilometre Array: Red Book 2018: Technical specifications and performance forecasts. Publ. Astron. Soc. Austral. 2020, 37, e007. [Google Scholar]
- D’Amico, G.; Senatore, L.; Zhang, P. Limits on wCDM from the EFTofLSS with the PyBird code. JCAP 2021, 1, 6. [Google Scholar] [CrossRef]
- Chudaykin, A.; Dolgikh, K.; Ivanov, M.M. Constraints on the curvature of the Universe and dynamical dark energy from the Full-shape and BAO data. Phys. Rev. D 2021, 103, 23507. [Google Scholar] [CrossRef]
- Brieden, S.; Gil-Marín, H.; Verde, L. Model-agnostic interpretation of 10 billion years of cosmic evolution traced by BOSS and eBOSS data. JCAP 2022, 8, 24. [Google Scholar] [CrossRef]
- Carrilho, P.; Moretti, C.; Pourtsidou, A. Cosmology with the EFTofLSS and BOSS: Dark energy constraints and a note on priors. JCAP 2023, 1, 28. [Google Scholar] [CrossRef]
- Semenaite, A.; Sánchez, A.G.; Pezzotta, A.; Hou, J.; Eggemeier, A.; Crocce, M.; Zhao, C.; Brownstein, J.R.; Rossi, G.; Schneider, D.P. Beyond – ΛCDM constraints from the full shape clustering measurements from BOSS and eBOSS. Mon. Not. R. Astron. Soc. 2023, 521, 5013–5025. [Google Scholar] [CrossRef]
- Abbott, T.M.C.; Abdalla, F.B.; Avila, S.; Banerji, M.; Baxter, E.; Bechtol, K.; Becker, M.R.; Bertin, E.; Blazek, J.; Bridle, S.L.; et al. Dark Energy Survey Year 1 Results: Constraints on Extended Cosmological Models from Galaxy Clustering and Weak Lensing. Phys. Rev. D 2019, 99, 123505. [Google Scholar] [CrossRef]
- Tröster, T.; Asgari, M.; Blake, C.; Cataneo, M.; Heymans, C.; Hildebrandt, H.; Joachimi, B.; Lin, C.-A.; Sanchez, A.G.; Wright, A.H.; et al. KiDS-1000 Cosmology: Constraints beyond flat ΛCDM. Astron. Astrophys. 2021, 649, A88. [Google Scholar] [CrossRef]
- Abbott, T.M.C.; Aguena, M.; Alarcon, A.; Alves, O.; Amon, A.; Annis, J.; Avila, S.; Bacon, D.; Baxter, E.; Bechtol, K.; et al. Dark Energy Survey Year 3 results: Constraints on extensions to ΛCDM with weak lensing and galaxy clustering. Phys. Rev. D 2023, 107, 83504. [Google Scholar] [CrossRef]
- Scolnic, D.M.; Jones, D.O.; Rest, A.; Pan, Y.C.; Chornock, R.; Foley, R.J.; Huber, M.E.; Kessler, R.; Narayan, G.; Riess, A.G.; et al. The Complete Light-curve Sample of Spectroscopically Confirmed SNe Ia from Pan-STARRS1 and Cosmological Constraints from the Combined Pantheon Sample. Astrophys. J. 2018, 859, 101. [Google Scholar] [CrossRef]
- Moresco, M.; Jimenez, R.; Verde, L.; Cimatti, A.; Pozzetti, L.; Maraston, C.; Thomas, D. Constraining the time evolution of dark energy, curvature and neutrino properties with cosmic chronometers. JCAP 2016, 12, 39. [Google Scholar] [CrossRef]
- Yang, W.; Di Valentino, E.; Pan, S.; Wu, Y.; Lu, J. Dynamical dark energy after Planck CMB final release and H0 tension. Mon. Not. R. Astron. Soc. 2021, 501, 5845–5858. [Google Scholar] [CrossRef]
- Grillo, C.; Rosati, P.; Suyu, S.H.; Caminha, G.B.; Mercurio, A.; Halkola, A. On the accuracy of time-delay cosmography in the Frontier Fields Cluster MACS J1149.5+2223 with supernova Refsdal. Astrophys. J. 2020, 898, 87. [Google Scholar] [CrossRef]
- Cao, S.; Ryan, J.; Ratra, B. Cosmological constraints from H ii starburst galaxy, quasar angular size, and other measurements. Mon. Not. R. Astron. Soc. 2022, 509, 4745–4757. [Google Scholar] [CrossRef]
- Khadka, N.; Ratra, B. Using quasar X-ray and UV flux measurements to constrain cosmological model parameters. Mon. Not. R. Astron. Soc. 2020, 497, 263–278. [Google Scholar] [CrossRef]
- Khadka, N.; Ratra, B. Determining the range of validity of quasar X-ray and UV flux measurements for constraining cosmological model parameters. Mon. Not. R. Astron. Soc. 2021, 502, 6140–6156. [Google Scholar] [CrossRef]
- Cao, S.; Ryan, J.; Ratra, B. Using Pantheon and DES supernova, baryon acoustic oscillation, and Hubble parameter data to constrain the Hubble constant, dark energy dynamics, and spatial curvature. Mon. Not. R. Astron. Soc. 2021, 504, 300–310. [Google Scholar] [CrossRef]
- Khadka, N.; Ratra, B. Do quasar X-ray and UV flux measurements provide a useful test of cosmological models? Mon. Not. R. Astron. Soc. 2022, 510, 2753–2772. [Google Scholar] [CrossRef]
- Cao, S.; Ratra, B. H0 = 69.8 ± 1.3 km s−1 Mpc−1, Ωm0 = 0.288 ± 0.017, and other constraints from lower-redshift, non-CMB, expansion-rate data. Phys. Rev. D 2023, 107, 103521. [Google Scholar] [CrossRef]
- Escamilla, L.A.; Giarè, W.; Di Valentino, E.; Nunes, R.C.; Vagnozzi, S. The state of the dark energy equation of state circa 2023. arXiv 2023, arXiv:2307.14802. [Google Scholar]
- Oikonomou, V.K. Effects of the axion through the Higgs portal on primordial gravitational waves during the electroweak breaking. Phys. Rev. D 2023, 107, 64071. [Google Scholar] [CrossRef]
- Espinosa, J.R.; Grojean, C.; Panico, G.; Pomarol, A.; Pujolàs, O.; Servant, G. Cosmological Higgs-Axion Interplay for a Naturally Small Electroweak Scale. Phys. Rev. Lett. 2015, 115, 251803. [Google Scholar] [CrossRef]
- Dev, P.S.B.; Ferrer, F.; Zhang, Y.; Zhang, Y. Gravitational Waves from First-Order Phase Transition in a Simple Axion-Like Particle Model. JCAP 2019, 11, 6. [Google Scholar] [CrossRef]
- Im, S.H.; Jeong, K.S. Freeze-in Axion-like Dark Matter. Phys. Lett. B 2019, 799, 135044. [Google Scholar] [CrossRef]
- Bauer, M.; Rostagni, G.; Spinner, J. Axion-Higgs portal. Phys. Rev. D 2023, 107, 15007. [Google Scholar] [CrossRef]
- Oikonomou, V.K. Flat energy spectrum of primordial gravitational waves versus peaks and the NANOGrav 2023 observation. Phys. Rev. D 2023, 108, 43516. [Google Scholar] [CrossRef]
- Amon, A.; Efstathiou, G. A non-linear solution to the S8 tension? Mon. Not. R. Astron. Soc. 2022, 516, 5355–5366. [Google Scholar] [CrossRef]
- Simpson, F. Scattering of dark matter and dark energy. Phys. Rev. D 2010, 82, 83505. [Google Scholar] [CrossRef]
- Xu, X.D.; Wang, B.; Abdalla, E. The signature of the scattering between dark sectors in large scale cosmic microwave background anisotropies. Phys. Rev. D 2012, 85, 83513. [Google Scholar] [CrossRef]
- Richarte, M.G.; Xu, L. Interacting parametrized post-Friedmann method. Gen. Rel. Grav. 2016, 48, 39. [Google Scholar] [CrossRef]
- Boehmer, C.G.; Tamanini, N.; Wright, M. Interacting quintessence from a variational approach Part II: Derivative couplings. Phys. Rev. D 2015, 91, 123003. [Google Scholar] [CrossRef]
- Tamanini, N. Phenomenological models of dark energy interacting with dark matter. Phys. Rev. D 2015, 92, 43524. [Google Scholar] [CrossRef]
- Koivisto, T.S.; Saridakis, E.N.; Tamanini, N. Scalar-Fluid theories: Cosmological perturbations and large-scale structure. JCAP 2015, 9, 47. [Google Scholar] [CrossRef]
- Pourtsidou, A.; Tram, T. Reconciling CMB and structure growth measurements with dark energy interactions. Phys. Rev. D 2016, 94, 43518. [Google Scholar] [CrossRef]
- Dutta, J.; Khyllep, W.; Tamanini, N. Scalar-Fluid interacting dark energy: Cosmological dynamics beyond the exponential potential. Phys. Rev. D 2017, 95, 23515. [Google Scholar] [CrossRef]
- Kumar, S.; Nunes, R.C. Observational constraints on dark matter–dark energy scattering cross section. Eur. Phys. J. C 2017, 77, 734. [Google Scholar] [CrossRef]
- Linton, M.S.; Pourtsidou, A.; Crittenden, R.; Maartens, R. Variable sound speed in interacting dark energy models. JCAP 2018, 4, 43. [Google Scholar] [CrossRef]
- Bose, B.; Baldi, M.; Pourtsidou, A. Modelling Non-Linear Effects of Dark Energy. JCAP 2018, 4, 32. [Google Scholar] [CrossRef]
- Bose, B.; Taruya, A. The one-loop matter bispectrum as a probe of gravity and dark energy. JCAP 2018, 10, 19. [Google Scholar] [CrossRef]
- Asghari, M.; Beltrán Jiménez, J.; Khosravi, S.; Mota, D.F. On structure formation from a small-scales-interacting dark sector. JCAP 2019, 4, 42. [Google Scholar] [CrossRef]
- Kase, R.; Tsujikawa, S. Scalar-Field Dark Energy Nonminimally and Kinetically Coupled to Dark Matter. Phys. Rev. D 2020, 101, 63511. [Google Scholar] [CrossRef]
- Kase, R.; Tsujikawa, S. Weak cosmic growth in coupled dark energy with a Lagrangian formulation. Phys. Lett. B 2020, 804, 135400. [Google Scholar] [CrossRef]
- Chamings, F.N.; Avgoustidis, A.; Copeland, E.J.; Green, A.M.; Pourtsidou, A. Understanding the suppression of structure formation from dark matter-dark energy momentum coupling. Phys. Rev. D 2020, 101, 43531. [Google Scholar] [CrossRef]
- Asghari, M.; Khosravi, S.; Mollazadeh, A. Perturbation level interacting dark energy model and its consequence on late-time cosmological parameters. Phys. Rev. D 2020, 101, 43503. [Google Scholar] [CrossRef]
- Amendola, L.; Tsujikawa, S. Scaling solutions and weak gravity in dark energy with energy and momentum couplings. JCAP 2020, 6, 20. [Google Scholar] [CrossRef]
- De Felice, A.; Nakamura, S.; Tsujikawa, S. Suppressed cosmic growth in coupled vector-tensor theories. Phys. Rev. D 2020, 102, 63531. [Google Scholar] [CrossRef]
- Beltrán Jiménez, J.; Bettoni, D.; Figueruelo, D.; Teppa Pannia, F.A.; Tsujikawa, S. Velocity-dependent interacting dark energy and dark matter with a Lagrangian description of perfect fluids. JCAP 2021, 3, 85. [Google Scholar] [CrossRef]
- Figueruelo, D.; Aparicio Resco, M.; Teppa Pannia, F.A.; Beltran Jimenez, J.; Bettoni, D.; Maroto, A.L.; Abramo, L.R.; Alcaniz, J.; Benitez, N.; Bonoli, S.; et al. J-PAS: Forecasts for dark matter—Dark energy elastic couplings. JCAP 2021, 7, 22. [Google Scholar] [CrossRef]
- Carrilho, P.; Moretti, C.; Bose, B.; Markovič, K.; Pourtsidou, A. Interacting dark energy from redshift-space galaxy clustering. JCAP 2021, 10, 4. [Google Scholar] [CrossRef]
- Linton, M.S.; Crittenden, R.; Pourtsidou, A. Momentum transfer models of interacting dark energy. JCAP 2022, 8, 75. [Google Scholar] [CrossRef]
- Mancini Spurio, A.; Pourtsidou, A. KiDS-1000 cosmology: Machine learning–Accelerated constraints on interacting dark energy with CosmoPower. Mon. Not. R. Astron. Soc. 2022, 512, L44–L48. [Google Scholar] [CrossRef]
- Carrilho, P.; Carrion, K.; Bose, B.; Pourtsidou, A.; Hidalgo, J.C.; Lombriser, L.; Baldi, M. On the road to per cent accuracy VI: The non-linear power spectrum for interacting dark energy with baryonic feedback and massive neutrinos. Mon. Not. R. Astron. Soc. 2022, 512, 3691–3702. [Google Scholar] [CrossRef]
- Poulin, V.; Bernal, J.L.; Kovetz, E.D.; Kamionkowski, M. Sigma-8 tension is a drag. Phys. Rev. D 2023, 107, 123538. [Google Scholar] [CrossRef]
- Cardona, W.; Figueruelo, D. Momentum transfer in the dark sector and lensing convergence in upcoming galaxy surveys. JCAP 2022, 12, 10. [Google Scholar] [CrossRef]
- Piga, L.; Marinucci, M.; D’Amico, G.; Pietroni, M.; Vernizzi, F.; Wright, B.S. Constraints on modified gravity from the BOSS galaxy survey. JCAP 2023, 4, 38. [Google Scholar] [CrossRef]
- Jiménez, J.B.; Di Dio, E.; Figueruelo, D. A smoking gun from the power spectrum dipole for elastic interactions in the dark sector. arXiv 2022, arXiv:2212.08617. [Google Scholar]
- Wilkinson, R.J.; Lesgourgues, J.; Boehm, C. Using the CMB angular power spectrum to study Dark Matter-photon interactions. JCAP 2014, 4, 26. [Google Scholar] [CrossRef]
- Stadler, J.; Bœhm, C. Constraints on γ-CDM interactions matching the Planck data precision. JCAP 2018, 10, 9. [Google Scholar] [CrossRef]
- Yadav, S.K. Constraints on dark matter-photon coupling in the presence of time-varying dark energy. Mod. Phys. Lett. A 2019, 35, 1950358. [Google Scholar] [CrossRef]
- Serra, P.; Zalamea, F.; Cooray, A.; Mangano, G.; Melchiorri, A. Constraints on neutrino—Dark matter interactions from cosmic microwave background and large scale structure data. Phys. Rev. D 2010, 81, 43507. [Google Scholar] [CrossRef]
- Wilkinson, R.J.; Boehm, C.; Lesgourgues, J. Constraining Dark Matter-Neutrino Interactions using the CMB and Large-Scale Structure. JCAP 2014, 5, 11. [Google Scholar] [CrossRef]
- Escudero, M.; Mena, O.; Vincent, A.C.; Wilkinson, R.J.; Bœhm, C. Exploring dark matter microphysics with galaxy surveys. JCAP 2015, 9, 34. [Google Scholar] [CrossRef]
- Stadler, J.; Bœhm, C.; Mena, O. Comprehensive Study of Neutrino-Dark Matter Mixed Damping. JCAP 2019, 8, 14. [Google Scholar] [CrossRef]
- Hooper, D.C.; Lucca, M. Hints of dark matter-neutrino interactions in Lyman-α data. Phys. Rev. D 2022, 105, 103504. [Google Scholar] [CrossRef]
- Dvorkin, C.; Blum, K.; Kamionkowski, M. Constraining Dark Matter-Baryon Scattering with Linear Cosmology. Phys. Rev. D 2014, 89, 23519. [Google Scholar] [CrossRef]
- Gluscevic, V.; Boddy, K.K. Constraints on Scattering of keV–TeV Dark Matter with Protons in the Early Universe. Phys. Rev. Lett. 2018, 121, 81301. [Google Scholar] [CrossRef]
- Boddy, K.K.; Gluscevic, V. First Cosmological Constraint on the Effective Theory of Dark Matter-Proton Interactions. Phys. Rev. D 2018, 98, 83510. [Google Scholar] [CrossRef]
- Xu, W.L.; Dvorkin, C.; Chael, A. Probing sub-GeV Dark Matter-Baryon Scattering with Cosmological Observables. Phys. Rev. D 2018, 97, 103530. [Google Scholar] [CrossRef]
- Boddy, K.K.; Gluscevic, V.; Poulin, V.; Kovetz, E.D.; Kamionkowski, M.; Barkana, R. Critical assessment of CMB limits on dark matter-baryon scattering: New treatment of the relative bulk velocity. Phys. Rev. D 2018, 98, 123506. [Google Scholar] [CrossRef]
- Ali-Haïmoud, Y. Testing dark matter interactions with CMB spectral distortions. Phys. Rev. D 2021, 103, 43541. [Google Scholar] [CrossRef]
- Buen-Abad, M.A.; Essig, R.; McKeen, D.; Zhong, Y.M. Cosmological constraints on dark matter interactions with ordinary matter. Phys. Rept. 2022, 961, 1–35. [Google Scholar] [CrossRef]
- Nguyen, D.V.; Sarnaaik, D.; Boddy, K.K.; Nadler, E.O.; Gluscevic, V. Observational constraints on dark matter scattering with electrons. Phys. Rev. D 2021, 104, 103521. [Google Scholar] [CrossRef]
- Rogers, K.K.; Dvorkin, C.; Peiris, H.V. Limits on the Light Dark Matter–Proton Cross Section from Cosmic Large-Scale Structure. Phys. Rev. Lett. 2022, 128, 171301. [Google Scholar] [CrossRef]
- Driskell, T.; Nadler, E.O.; Mirocha, J.; Benson, A.; Boddy, K.K.; Morton, T.D.; Lashner, J.; An, R.; Gluscevic, V. Structure formation and the global 21-cm signal in the presence of Coulomb-like dark matter-baryon interactions. Phys. Rev. D 2022, 106, 103525. [Google Scholar] [CrossRef]
- He, A.; Ivanov, M.M.; An, R.; Gluscevic, V. S8 Tension in the Context of Dark Matter-Baryon Scattering. arXiv 2023, arXiv:2301.08260. [Google Scholar]
- Foot, R.; Vagnozzi, S. Dissipative hidden sector dark matter. Phys. Rev. D 2015, 91, 23512. [Google Scholar] [CrossRef]
- Foot, R.; Vagnozzi, S. Diurnal modulation signal from dissipative hidden sector dark matter. Phys. Lett. B 2015, 748, 61–66. [Google Scholar] [CrossRef]
- Cyr-Racine, F.Y.; Sigurdson, K.; Zavala, J.; Bringmann, T.; Vogelsberger, M.; Pfrommer, C. ETHOS—An effective theory of structure formation: From dark particle physics to the matter distribution of the Universe. Phys. Rev. D 2016, 93, 123527. [Google Scholar] [CrossRef]
- Vogelsberger, M.; Zavala, J.; Cyr-Racine, F.Y.; Pfrommer, C.; Bringmann, T.; Sigurdson, K. ETHOS–An effective theory of structure formation: Dark matter physics as a possible explanation of the small-scale CDM problems. Mon. Not. R. Astron. Soc. 2016, 460, 1399–1416. [Google Scholar] [CrossRef]
- Foot, R.; Vagnozzi, S. Solving the small-scale structure puzzles with dissipative dark matter. JCAP 2016, 7, 13. [Google Scholar] [CrossRef]
- Archidiacono, M.; Bohr, S.; Hannestad, S.; Jørgensen, J.H.; Lesgourgues, J. Linear scale bounds on dark matter–dark radiation interactions and connection with the small scale crisis of cold dark matter. JCAP 2017, 11, 10. [Google Scholar] [CrossRef]
- Becker, N.; Hooper, D.C.; Kahlhoefer, F.; Lesgourgues, J.; Schöneberg, N. Cosmological constraints on multi-interacting dark matter. JCAP 2021, 2, 19. [Google Scholar] [CrossRef]
- Vagnozzi, S.; Visinelli, L.; Mena, O.; Mota, D.F. Do we have any hope of detecting scattering between dark energy and baryons through cosmology? Mon. Not. R. Astron. Soc. 2020, 493, 1139–1152. [Google Scholar] [CrossRef]
- Jiménez, J.B.; Bettoni, D.; Figueruelo, D.; Teppa Pannia, F.A. On cosmological signatures of baryons-dark energy elastic couplings. JCAP 2020, 8, 20. [Google Scholar] [CrossRef]
- Vagnozzi, S.; Visinelli, L.; Brax, P.; Davis, A.C.; Sakstein, J. Direct detection of dark energy: The XENON1T excess and future prospects. Phys. Rev. D 2021, 104, 63023. [Google Scholar] [CrossRef]
- Benisty, D.; Davis, A.C. Dark energy interactions near the Galactic Center. Phys. Rev. D 2022, 105, 24052. [Google Scholar] [CrossRef]
- Ferlito, F.; Vagnozzi, S.; Mota, D.F.; Baldi, M. Cosmological direct detection of dark energy: Non-linear structure formation signatures of dark energy scattering with visible matter. Mon. Not. R. Astron. Soc. 2022, 512, 1885–1905. [Google Scholar] [CrossRef]
- Pourtsidou, A.; Skordis, C.; Copeland, E.J. Models of dark matter coupled to dark energy. Phys. Rev. D 2013, 88, 83505. [Google Scholar] [CrossRef]
- Skordis, C.; Pourtsidou, A.; Copeland, E.J. Parametrized post-Friedmannian framework for interacting dark energy theories. Phys. Rev. D 2015, 91, 83537. [Google Scholar] [CrossRef]
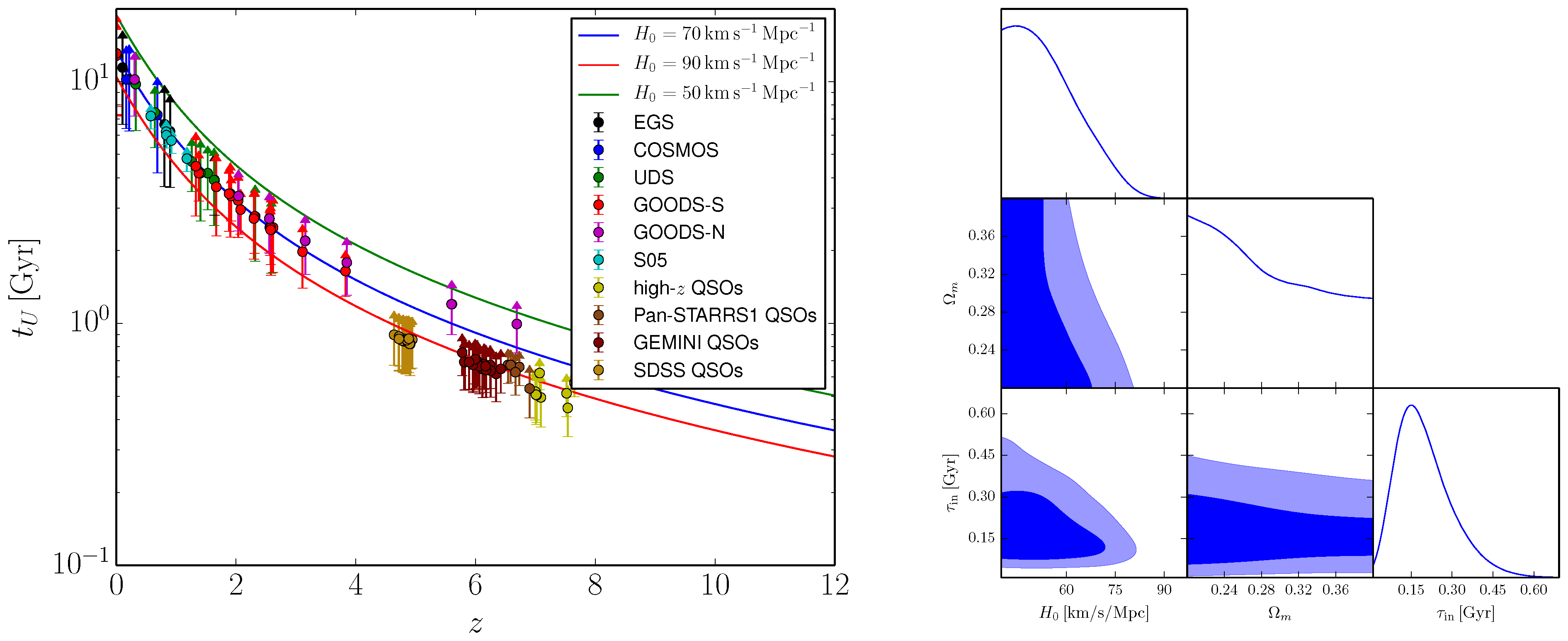
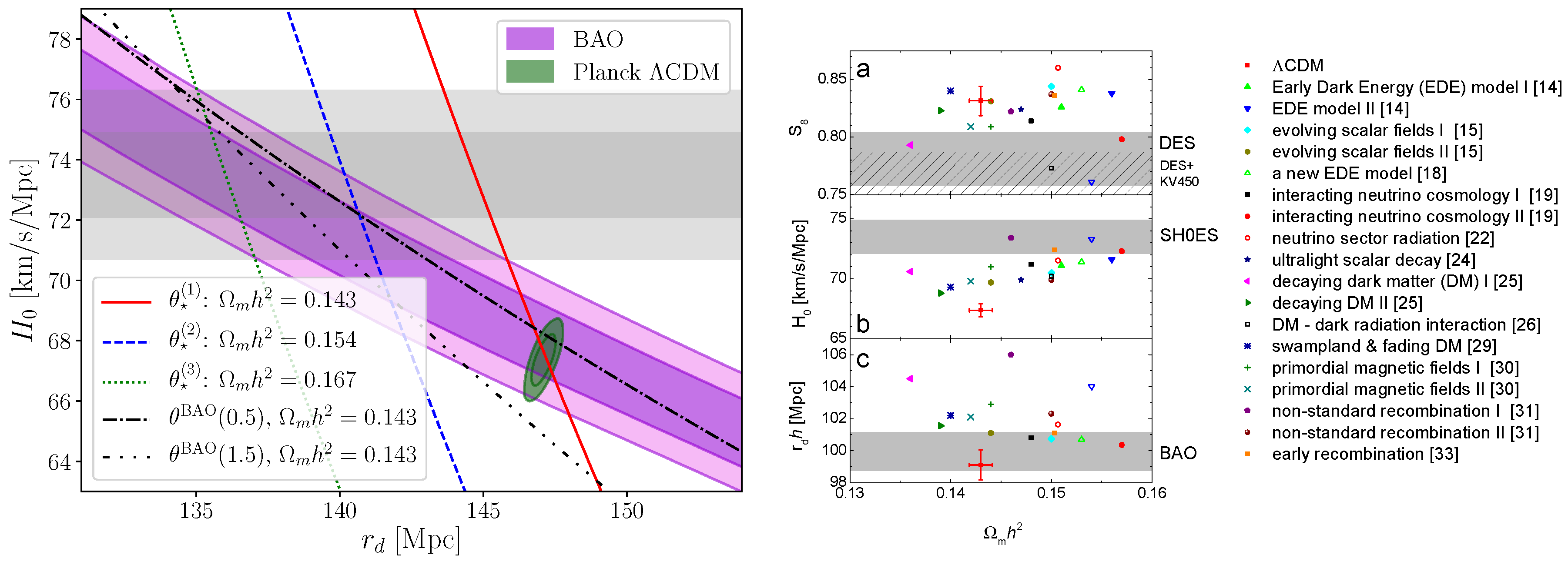


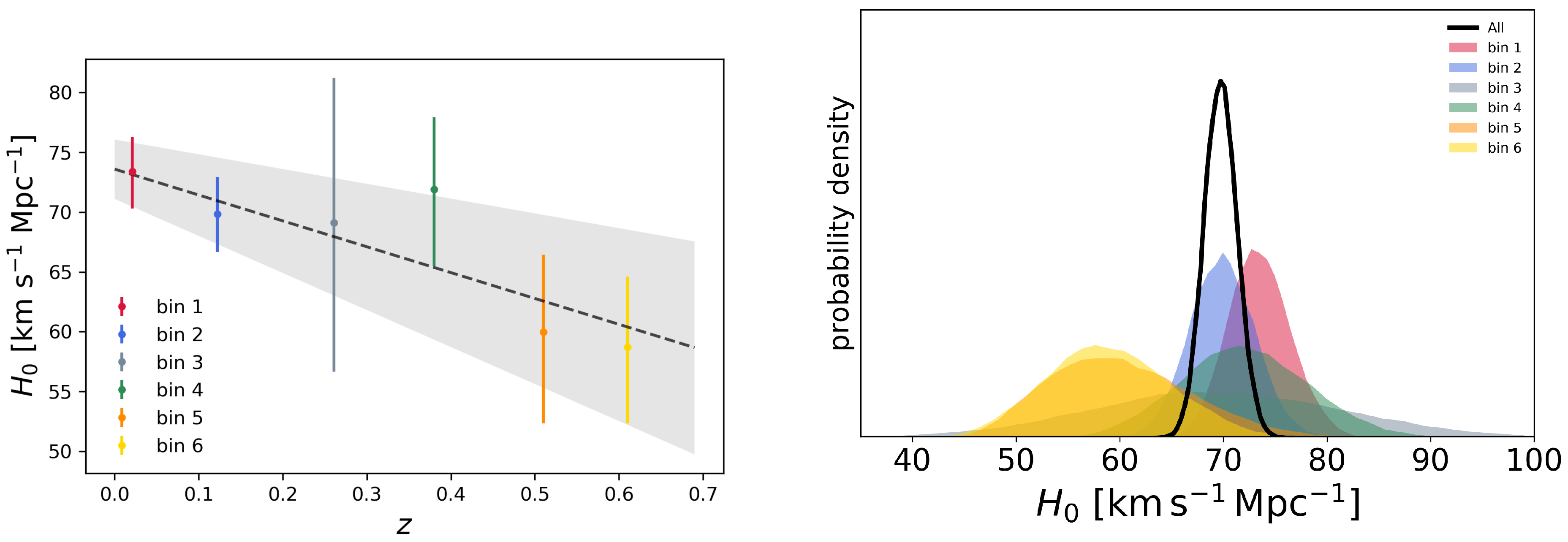
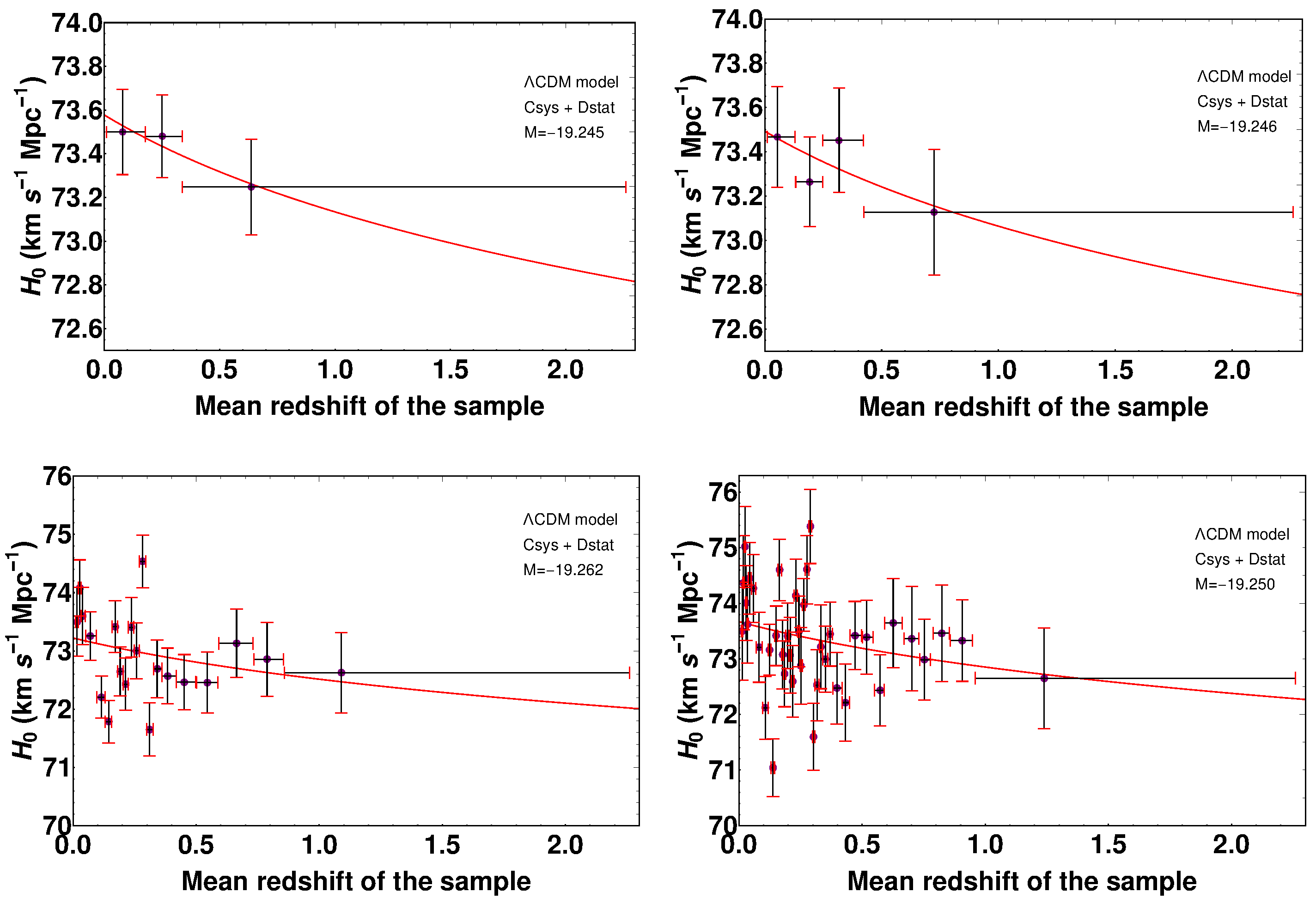
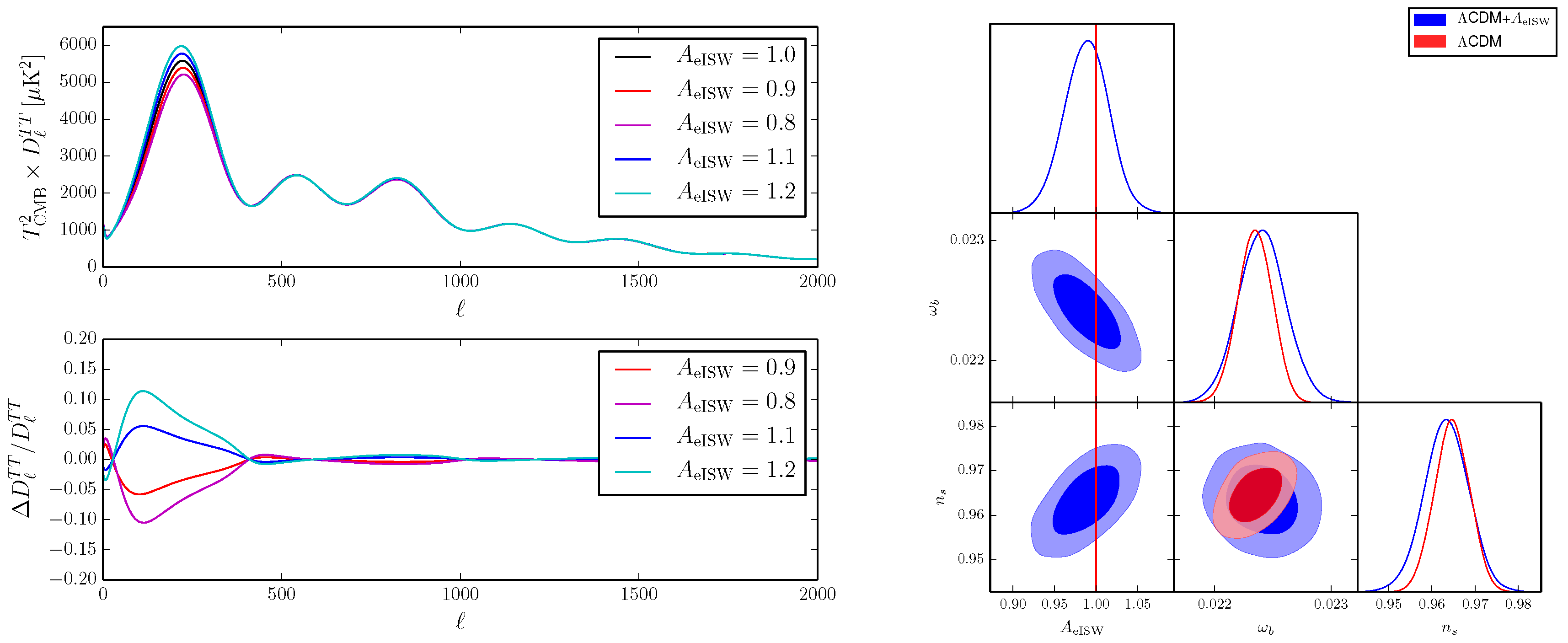
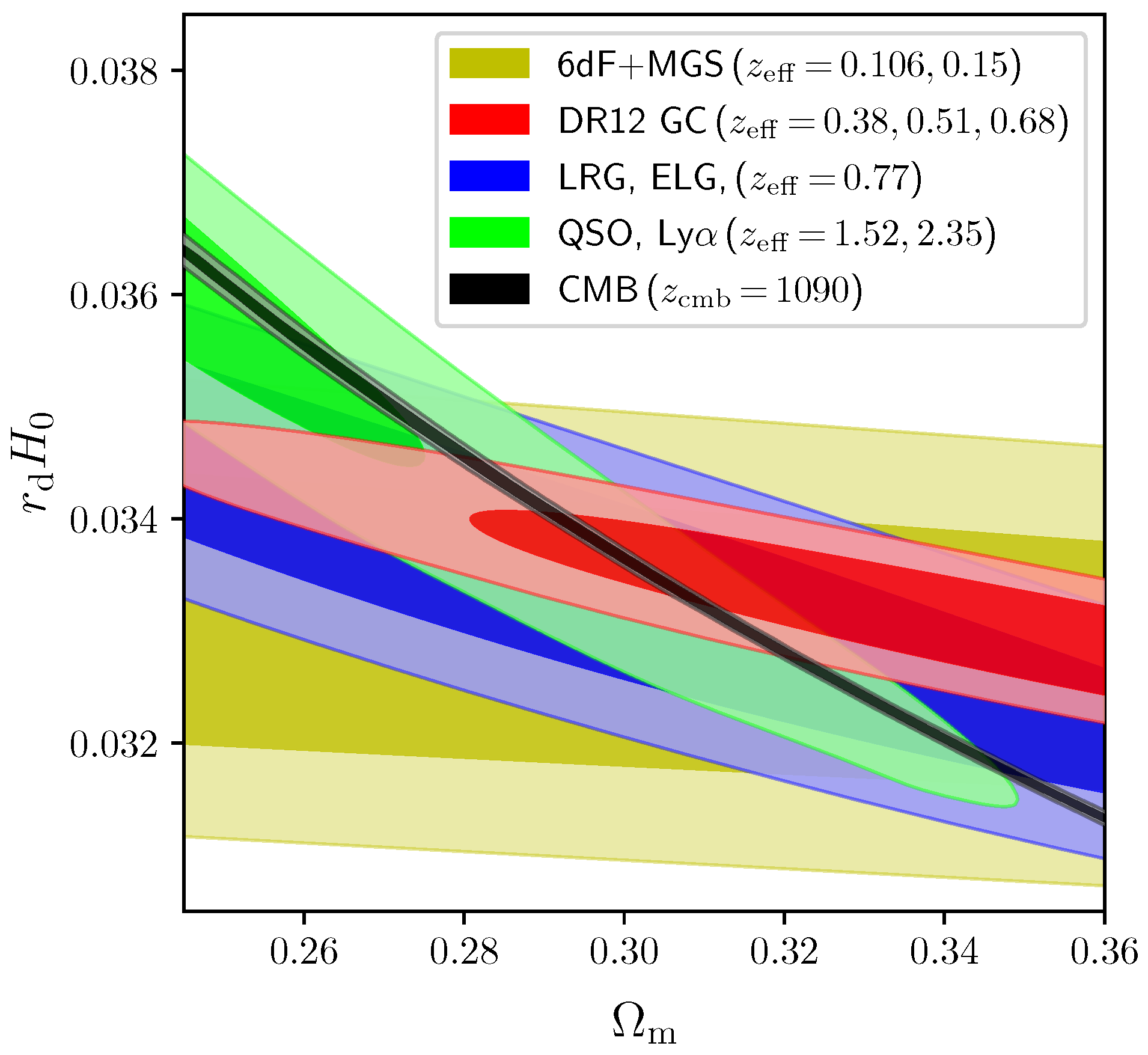

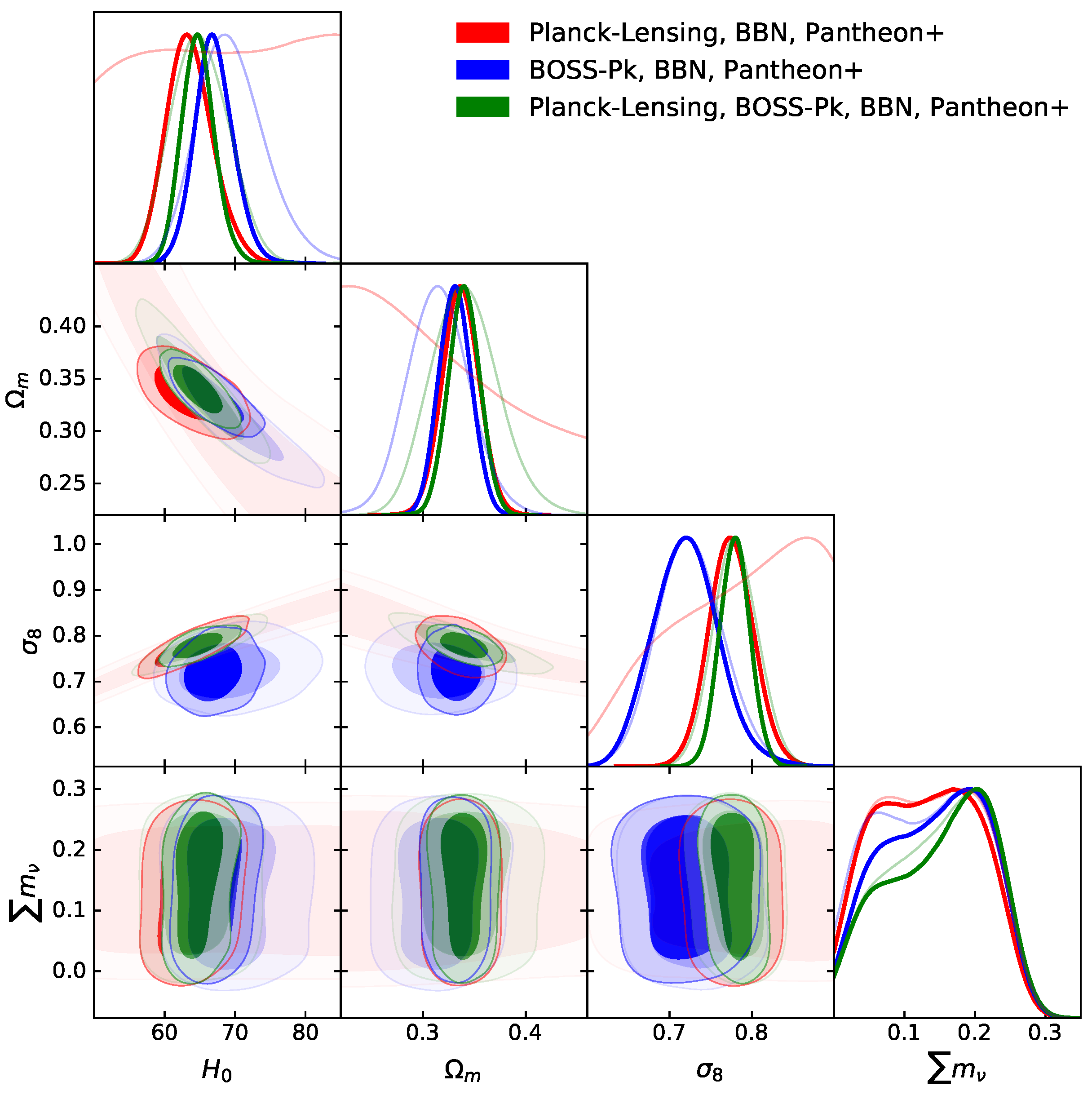
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vagnozzi, S. Seven Hints That Early-Time New Physics Alone Is Not Sufficient to Solve the Hubble Tension. Universe 2023, 9, 393. https://doi.org/10.3390/universe9090393
Vagnozzi S. Seven Hints That Early-Time New Physics Alone Is Not Sufficient to Solve the Hubble Tension. Universe. 2023; 9(9):393. https://doi.org/10.3390/universe9090393
Chicago/Turabian StyleVagnozzi, Sunny. 2023. "Seven Hints That Early-Time New Physics Alone Is Not Sufficient to Solve the Hubble Tension" Universe 9, no. 9: 393. https://doi.org/10.3390/universe9090393
APA StyleVagnozzi, S. (2023). Seven Hints That Early-Time New Physics Alone Is Not Sufficient to Solve the Hubble Tension. Universe, 9(9), 393. https://doi.org/10.3390/universe9090393






