Construction of Multiscale Genome-Scale Metabolic Models: Frameworks and Challenges
Abstract
1. Introduction
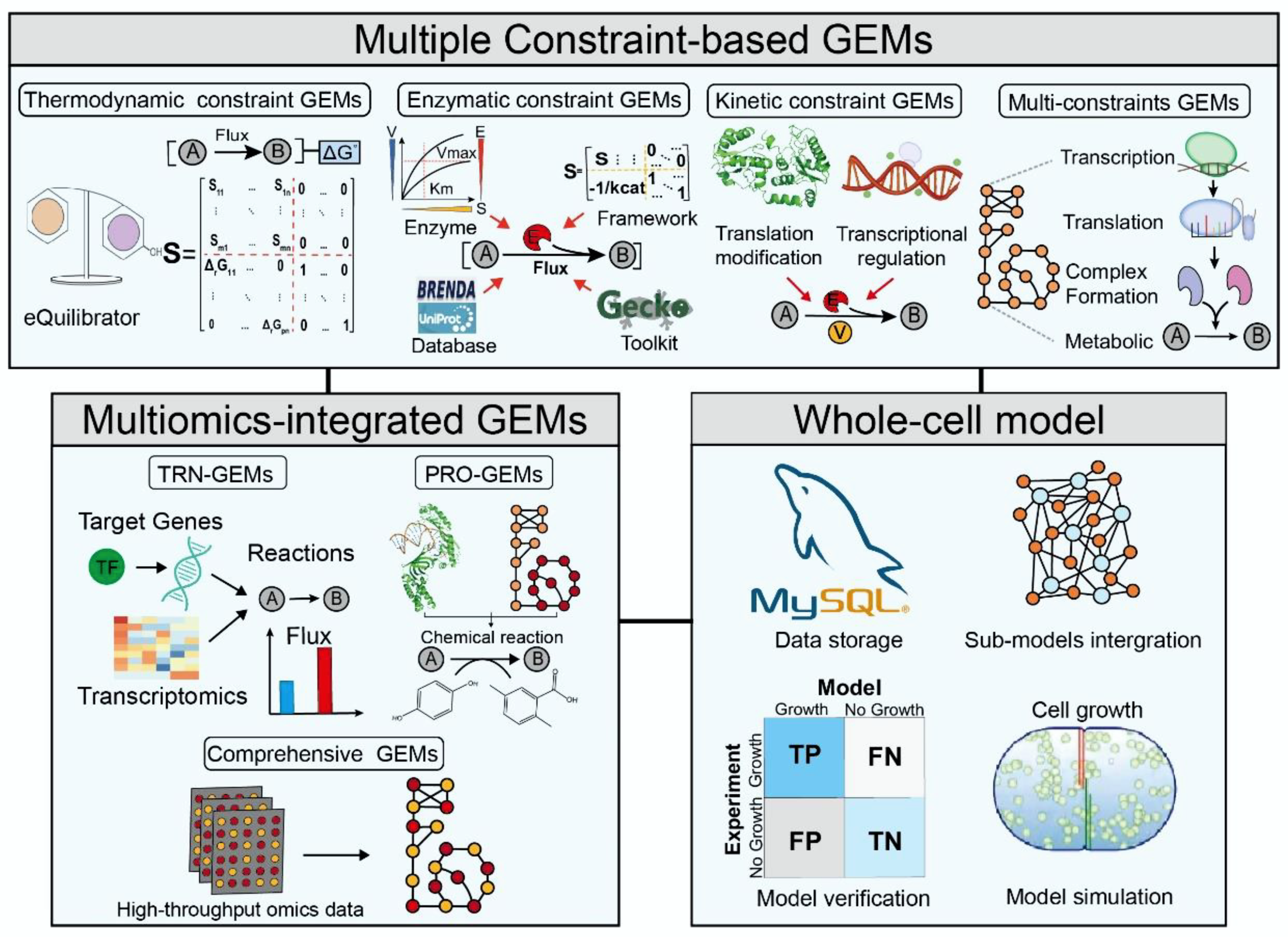
2. Constraint-Based GEMs
2.1. Thermodynamic Constraint GEMs
2.2. Enzymatic Constraint GEMs
2.3. Kinetic Constraint GEMs
2.4. Multiconstraint GEMs
3. Multiomics-Integrated GEMs
3.1. TRN-Integrated GEMs
3.2. PRO-Integrated GEMs
3.3. Comprehensive Metabolic Models
4. Whole-Cell Model
4.1. Construction of Whole-Cell Models
4.2. Application of Whole-Cell Models
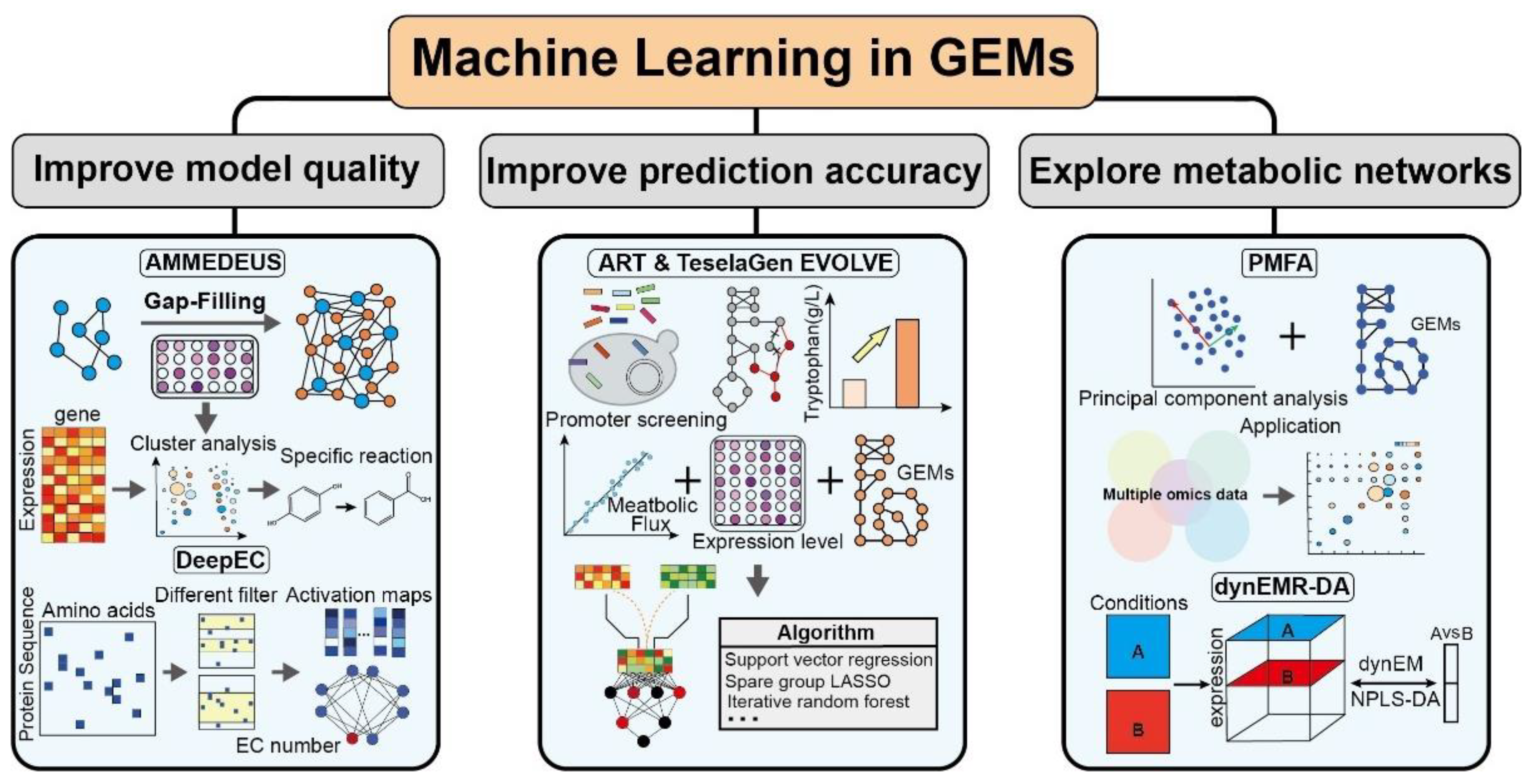
5. Machine Learning in GEMs
5.1. Improving the Model Quality
5.2. Improving the Prediction Accuracy
5.3. Exploring Metabolic Networks
6. Conclusions and Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kim, W.J.; Kim, H.U.; Lee, S.Y. Current state and applications of microbial genome-scale metabolic models. Curr. Opin. Syst. Biol. 2017, 2, 10–18. [Google Scholar] [CrossRef]
- Zielinski, D.C.; Patel, A.; Palsson, B.O. The Expanding Computational Toolbox for Engineering Microbial Phenotypes at the Genome Scale. Microorganisms 2020, 8, 2050. [Google Scholar] [CrossRef] [PubMed]
- Panikov, N.S. Genome-Scale Reconstruction of Microbial Dynamic Phenotype: Successes and Challenges. Microorganisms 2021, 9, 2352. [Google Scholar] [CrossRef] [PubMed]
- Edwards, J.S.; Palsson, B.O. Systems Properties of the Haemophilus influenzae Rd Metabolic Genotype. J. Biol. Chem. 1999, 274, 17410–17416. [Google Scholar] [CrossRef]
- Gu, C.; Kim, G.B.; Kim, W.J.; Kim, H.U.; Lee, S.Y. Current status and applications of genome-scale metabolic models. Genome Biol. 2019, 20, 121. [Google Scholar] [CrossRef]
- Mao, Z.; Huang, T.; Yuan, Q.; Ma, H. Construction and analysis of an integrated biological network of Escherichia coli. Syst. Microbiol. Biomanuf. 2021, 2, 165–176. [Google Scholar] [CrossRef]
- Lu, H.; Kerkhoven, E.J.; Nielsen, J. Multiscale models quantifying yeast physiology: Towards a whole-cell model. Trends Biotechnol. 2021, 40, 291–305. [Google Scholar] [CrossRef]
- Kocabaş, P.; Çalık, P.; Çalık, G.; Özdamar, T.H. Analyses of extracellular protein production in Bacillus subtilis—I: Genome-scale metabolic model reconstruction based on updated gene-enzyme-reaction data. Biochem. Eng. J. 2017, 127, 229–241. [Google Scholar] [CrossRef]
- Edwards, J.S.; Palsson, B.O. The Escherichia coli MG1655 in silico metabolic genotype: Its definition, characteristics, and capabilities. Proc. Natl. Acad. Sci. USA. 2000, 97, 5528–5533. [Google Scholar] [CrossRef]
- Fang, X.; Lloyd, C.J.; Palsson, B.O. Reconstructing organisms in silico: Genome-scale models and their emerging applications. Nat. Rev. Microbiol. 2020, 18, 731–743. [Google Scholar] [CrossRef]
- O’Brien, E.J.; Lerman, J.; Chang, R.; Hyduke, D.R.; Palsson, B. Genome-scale models of metabolism and gene expression extend and refine growth phenotype prediction. Mol. Syst. Biol. 2013, 9, 693. [Google Scholar] [CrossRef] [PubMed]
- Chen, K.; Gao, Y.; Mih, N.; O’Brien, E.J.; Yang, L.; Palsson, B.O. Thermosensitivity of growth is determined by chaperone-mediated proteome reallocation. Proc. Natl. Acad. Sci. USA 2017, 114, 11548–11553. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.; Mih, N.; Anand, A.; Park, J.H.; Tan, J.; Yurkovich, J.T.; Monk, J.M.; Lloyd, C.J.; Sandberg, T.E.; Seo, S.W.; et al. Cellular responses to reactive oxygen species are predicted from molecular mechanisms. Proc. Natl. Acad. Sci. USA 2019, 116, 14368–14373. [Google Scholar] [CrossRef] [PubMed]
- Du, B.; Yang, L.; Lloyd, C.J.; Fang, X.; Palsson, B.O. Genome-scale model of metabolism and gene expression provides a multi-scale description of acid stress responses in Escherichia coli. PLOS Comput. Biol. 2019, 15, e1007525. [Google Scholar] [CrossRef] [PubMed]
- Österlund, T.; Nookaew, I.; Nielsen, J. Fifteen years of large scale metabolic modeling of yeast: Developments and impacts. Biotechnol. Adv. 2011, 30, 979–988. [Google Scholar] [CrossRef]
- Massaiu, I.; Pasotti, L.; Sonnenschein, N.; Rama, E.; Cavaletti, M.; Magni, P.; Calvio, C.; Herrgård, M.J. Integration of enzymatic data in Bacillus subtilis genome-scale metabolic model improves phenotype predictions and enables in silico design of poly-gamma-glutamic acid production strains. Microb. Cell Fact. 2019, 18, 3. [Google Scholar] [CrossRef]
- Orth, J.D.; Thiele, I.; Palsson, B.Ø. What is flux balance analysis? Nat. Biotechnol. 2010, 28, 245–248. [Google Scholar] [CrossRef]
- Mahadevan, R.; Edwards, J.S.; Doyle, F.J., III. Dynamic Flux Balance Analysis of Diauxic Growth in Escherichia coli. Biophys. J. 2002, 83, 1331–1340. [Google Scholar] [CrossRef]
- Zur, H.; Ruppin, E.; Shlomi, T. iMAT: An integrative metabolic analysis tool. Bioinformatics 2010, 26, 3140–3142. [Google Scholar] [CrossRef]
- Jensen, P.A.; Papin, J. Functional integration of a metabolic network model and expression data without arbitrary thresholding. Bioinformatics 2010, 27, 541–547. [Google Scholar] [CrossRef]
- Ravi, S.; Gunawan, R. ΔFBA—Predicting metabolic flux alterations using genome-scale metabolic models and differential transcriptomic data. PLOS Comput. Biol. 2021, 17, e1009589. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, B.J.; Ebrahim, A.; Metz, T.O.; Adkins, J.N.; Palsson, B.; Hyduke, D.R. GIM3E: Condition-specific models of cellular metabolism developed from metabolomics and expression data. Bioinformatics 2013, 29, 2900–2908. [Google Scholar] [CrossRef] [PubMed]
- Mahamkali, V.; McCubbin, T.; Beber, M.E.; Noor, E.; Marcellin, L.K.; Nielsen, L.K. multiTFA: A Python package for multi-variate thermodynamics-based flux analysis. Bioinformatics 2021, 37, 3064–3066. [Google Scholar] [CrossRef] [PubMed]
- Di Filippo, M.; Pescini, D.; Galuzzi, B.G.; Bonanomi, M.; Gaglio, D.; Mangano, E.; Consolandi, C.; Alberghina, L.; Vanoni, M.; Damiani, C. INTEGRATE: Model-based multi-omics data integration to characterize multi-level metabolic regulation. PLOS Comput. Biol. 2022, 18, e1009337. [Google Scholar] [CrossRef] [PubMed]
- Arkin, A.P.; Cottingham, R.W.; Henry, C.S.; Harris, N.L.; Stevens, R.L.; Maslov, S.; Dehal, P.; Ware, D.; Perez, F.; Canon, S.; et al. KBase: The United States Department of Energy Systems Biology Knowledgebase. Nat. Biotechnol. 2018, 36, 566–569. [Google Scholar] [CrossRef] [PubMed]
- Seaver, S.M.; Liu, F.; Zhang, Q.; Jeffryes, J.; Faria, J.P.; Edirisinghe, J.N.; Mundy, M.; Chia, N.; Noor, E.; Beber, M.E. The ModelSEED biochemistry database for the integration of metabolic annotations and the reconstruction, comparison and analysis of metabolic models for plants, fungi and microbes. Nucleic Acids Res. 2020, 49, D575–D588. [Google Scholar] [CrossRef]
- Machado, D.; Andrejev, S.; Tramontano, M.; Patil, K.R. Fast automated reconstruction of genome-scale metabolic models for microbial species and communities. Nucleic Acids Res. 2018, 46, 7542–7553. [Google Scholar] [CrossRef]
- Lieven, C.; Beber, M.E.; Olivier, B.G.; Bergmann, F.T.; Ataman, M.; Babaei, P.; Bartell, J.A.; Blank, L.M.; Chauhan, S.; Correia, K.; et al. Publisher Correction: MEMOTE for standardized genome-scale metabolic model testing. Nat. Biotechnol. 2020, 38, 504. [Google Scholar] [CrossRef]
- Kim, Y.; Kim, G.B.; Lee, S.Y. Machine learning applications in genome-scale metabolic modeling. Curr. Opin. Syst. Biol. 2021, 25, 42–49. [Google Scholar] [CrossRef]
- Min, S.; Lee, B.; Yoon, S. Deep learning in bioinformatics. Briefings Bioinform. 2016, 18, 851–869. [Google Scholar] [CrossRef]
- Lawson, C.E.; Martí, J.M.; Radivojevic, T.; Jonnalagadda, S.V.R.; Gentz, R.; Hillson, N.J.; Peisert, S.; Kim, J.; Simmons, B.A.; Petzold, C.J.; et al. Machine learning for metabolic engineering: A review. Metab. Eng. 2020, 63, 34–60. [Google Scholar] [CrossRef]
- Zampieri, G.; Vijayakumar, S.; Yaneske, E.; Angione, C. Machine and deep learning meet genome-scale metabolic modeling. PLOS Comput. Biol. 2019, 15, e1007084. [Google Scholar] [CrossRef] [PubMed]
- Dai, Z.; Locasale, J.W. Thermodynamic constraints on the regulation of metabolic fluxes. J. Biol. Chem. 2018, 293, 19725–19739. [Google Scholar] [CrossRef] [PubMed]
- Beard, D.A.; Liang, S.-D.; Qian, H. Energy Balance for Analysis of Complex Metabolic Networks. Biophys. J. 2002, 83, 79–86. [Google Scholar] [CrossRef]
- Kümmel, A.; Panke, S.; Heinemann, M. Putative regulatory sites unraveled by network-embedded thermodynamic analysis of metabolome data. Mol. Syst. Biol. 2006, 2, 2006-0034. [Google Scholar] [CrossRef] [PubMed]
- Henry, C.S.; Broadbelt, L.J.; Hatzimanikatis, V. Thermodynamics-Based Metabolic Flux Analysis. Biophys. J. 2007, 92, 1792–1805. [Google Scholar] [CrossRef]
- Mavrovouniotis, M. Estimation of standard Gibbs energy changes of biotransformations. J. Biol. Chem. 1991, 266, 14440–14445. [Google Scholar] [CrossRef]
- Beber, M.E.; Gollub, M.G.; Mozaffari, D.; Shebek, K.M.; Flamholz, A.I.; Milo, R.; Noor, E. eQuilibrator 3.0: A database solution for thermodynamic constant estimation. Nucleic Acids Res. 2021, 50, D603–D609. [Google Scholar] [CrossRef]
- Hädicke, O.; Von Kamp, A.; Aydogan, T.; Klamt, S. OptMDFpathway: Identification of metabolic pathways with maximal thermodynamic driving force and its application for analyzing the endogenous CO2 fixation potential of Escherichia coli. PLOS Comput. Biol. 2018, 14, e1006492. [Google Scholar] [CrossRef]
- Xu, Z.; Sun, J.; Wu, Q.; Zhu, D. Find_tfSBP: Find thermodynamics-feasible and smallest balanced pathways with high yield from large-scale metabolic networks. Sci. Rep. 2017, 7, 17334. [Google Scholar] [CrossRef]
- Salvy, P.; Fengos, G.; Ataman, M.; Pathier, T.; Soh, K.C.; Hatzimanikatis, V. pyTFA and matTFA: A Python package and a Matlab toolbox for Thermodynamics-based Flux Analysis. Bioinformatics 2018, 35, 167–169. [Google Scholar] [CrossRef] [PubMed]
- Henry, C.S.; Jankowski, M.D.; Broadbelt, L.J.; Hatzimanikatis, V. Genome-Scale Thermodynamic Analysis of Escherichia coli Metabolism. Biophys. J. 2006, 90, 1453–1461. [Google Scholar] [CrossRef] [PubMed]
- Henry, C.S.; Zinner, J.F.; Cohoon, M.P.; Stevens, R.L. iBsu1103: A new genome-scale metabolic model of Bacillus subtilis based on SEED annotations. Genome Biol. 2009, 10, 1–15. [Google Scholar] [CrossRef] [PubMed]
- Kiparissides, A.; Hatzimanikatis, V. Thermodynamics-based Metabolite Sensitivity Analysis in metabolic networks. Metab. Eng. 2017, 39, 117–127. [Google Scholar] [CrossRef][Green Version]
- Krumholz, E.W.; Libourel, I.G. Thermodynamic Constraints Improve Metabolic Networks. Biophys. J. 2017, 113, 679–689. [Google Scholar] [CrossRef]
- Beg, Q.K.; Vazquez, A.; Ernst, J.; de Menezes, M.A.; Bar-Joseph, Z.; Barabási, A.-L.; Oltvai, Z.N. Intracellular crowding defines the mode and sequence of substrate uptake by Escherichia coli and constrains its metabolic activity. Proc. Natl. Acad. Sci. USA 2007, 104, 12663–12668. [Google Scholar] [CrossRef]
- Adadi, R.; Volkmer, B.; Milo, R.; Heinemann, M.; Shlomi, T. Prediction of Microbial Growth Rate versus Biomass Yield by a Metabolic Network with Kinetic Parameters. PLOS Comput. Biol. 2012, 8, e1002575. [Google Scholar] [CrossRef]
- Sánchez, B.J.; Zhang, C.; Nilsson, A.; Lahtvee, P.-J.; Kerkhoven, E.J.; Nielsen, J. Improving the phenotype predictions of a yeast genome-scale metabolic model by incorporating enzymatic constraints. Mol. Syst. Biol. 2017, 13, 935. [Google Scholar] [CrossRef]
- Steuer, R.; Gross, T.; Selbig, J.; Blasius, B. Structural kinetic modeling of metabolic networks. Proc. Natl. Acad. Sci. USA 2006, 103, 11868–11873. [Google Scholar] [CrossRef]
- Jamshidi, N.; Palsson, B. Formulating genome-scale kinetic models in the post-genome era. Mol. Syst. Biol. 2008, 4, 171. [Google Scholar] [CrossRef]
- Miskovic, L.; Hatzimanikatis, V. Production of biofuels and biochemicals: In need of an ORACLE. Trends Biotechnol. 2010, 28, 391–397. [Google Scholar] [CrossRef] [PubMed]
- Chakrabarti, A.; Miskovic, L.; Soh, K.C.; Hatzimanikatis, V. Towards kinetic modeling of genome-scale metabolic networks without sacrificing stoichiometric, thermodynamic and physiological constraints. Biotechnol. J. 2013, 8, 1043–1057. [Google Scholar] [CrossRef]
- Tran, L.M.; Rizk, M.L.; Liao, J.C. Ensemble Modeling of Metabolic Networks. Biophys. J. 2008, 95, 5606–5617. [Google Scholar] [CrossRef] [PubMed]
- Yang, X.; Mao, Z.; Zhao, X.; Wang, R.; Zhang, P.; Cai, J.; Xue, C.; Ma, H. Integrating thermodynamic and enzymatic constraints into genome-scale metabolic models. Metab. Eng. 2021, 67, 133–144. [Google Scholar] [CrossRef] [PubMed]
- Salvy, P.; Hatzimanikatis, V. The ETFL formulation allows multi-omics integration in thermodynamics-compliant metabolism and expression models. Nat. Commun. 2020, 11, 30. [Google Scholar] [CrossRef]
- Jensen, P.A.; Lutz, K.A.; Papin, J.A. TIGER: Toolbox for integrating genome-scale metabolic models, expression data, and transcriptional regulatory networks. BMC Syst. Biol. 2011, 5, 147. [Google Scholar] [CrossRef]
- Marmiesse, L.; Peyraud, R.; Cottret, L. FlexFlux: Combining metabolic flux and regulatory network analyses. BMC Syst. Biol. 2015, 9, 93. [Google Scholar] [CrossRef]
- Chandrasekaran, S.; Price, N.D. Probabilistic integrative modeling of genome-scale metabolic and regulatory networks in Escherichia coli and Mycobacterium tuberculosis. Proc. Natl. Acad. Sci. USA 2010, 107, 17845–17850. [Google Scholar] [CrossRef]
- Motamedian, E.; Mohammadi, M.; Shojaosadati, S.A.; Heydari, M. TRFBA: An algorithm to integrate genome-scale metabolic and transcriptional regulatory networks with incorporation of expression data. Bioinformatics 2017, 33, 1057–1063. [Google Scholar] [CrossRef]
- Shen, F.; Sun, R.; Yao, J.; Li, J.; Liu, Q.; Price, N.D.; Liu, C.; Wang, Z. OptRAM: In-silico strain design via integrative regulatory-metabolic network modeling. PLOS Comput. Biol. 2019, 15, e1006835. [Google Scholar] [CrossRef]
- Brunk, E.; Mih, N.; Monk, J.; Zhang, Z.; O’Brien, E.J.; Bliven, S.E.; Chen, K.; Chang, R.L.; Bourne, P.E.; Palsson, B.O. Systems biology of the structural proteome. BMC Syst. Biol. 2016, 10, 26. [Google Scholar] [CrossRef] [PubMed]
- Mondeel, T.D.G.A.; Cremazy, F.; Barberis, M. GEMMER: GEnome-wide tool for Multi-scale Modeling data Extraction and Representation for Saccharomyces cerevisiae. Bioinformatics 2018, 34, 2147–2149. [Google Scholar] [CrossRef] [PubMed]
- Arakawa, K.; Yamada, Y.; Shinoda, K.; Nakayama, Y.; Tomita, M. GEM System: Automatic prototyping of cell-wide metabolic pathway models from genomes. BMC Bioinform. 2006, 7, 168. [Google Scholar] [CrossRef] [PubMed]
- Karp, P.D.; Midford, P.E.; Billington, R.; Kothari, A.; Krummenacker, M.; Latendresse, M.; Ong, W.K.; Subhraveti, P.; Caspi, R.; Fulcher, C.; et al. Pathway Tools version 23.0 update: Software for pathway/genome informatics and systems biology. Briefings Bioinform. 2019, 22, 109–126. [Google Scholar] [CrossRef]
- Karr, J.R.; Sanghvi, J.C.; Macklin, D.N.; Arora, A.; Covert, M.W. WholeCellKB: Model organism databases for comprehensive whole-cell models. Nucleic Acids Res. 2012, 41, D787–D792. [Google Scholar] [CrossRef]
- Clerx, M.; Cooling, M.T.; Cooper, J.; Garny, A.; Moyle, K.; Nickerson, D.P.; Nielsen, P.M.F.; Sorby, H. CellML 2.0. J. Integr. Bioinf. 2020, 17, 20200021. [Google Scholar] [CrossRef]
- Takahashi, K.; Ishikawa, N.; Sadamoto, Y.; Sasamoto, H.; Ohta, S.; Shiozawa, A.; Miyoshi, F.; Naito, Y.; Nakayama, Y.; Tomita, M. E-Cell 2: Multi-platform E-Cell simulation system. Bioinformatics 2003, 19, 1727–1729. [Google Scholar] [CrossRef]
- Matsuoka, Y.; Funahashi, A.; Ghosh, S.; Kitano, H. Modeling and Simulation Using CellDesigner. Transcr. Factor Regul. Netw. 2014, 1164, 121–145. [Google Scholar] [CrossRef]
- Mendes, P.; Hoops, S.; Sahle, S.; Gauges, R.; Dada, J.; Kummer, U. Computational Modeling of Biochemical Networks Using COPASI. In Systems Biology; Humana Press: Totova, NJ, USA, 2009; Volume 500, pp. 17–59. [Google Scholar] [CrossRef]
- Pahle, J. Biochemical simulations: Stochastic, approximate stochastic and hybrid approaches. Briefings Bioinform. 2008, 10, 53–64. [Google Scholar] [CrossRef]
- Zhou, J.; Fan, X.; Cao, L.; Sun, H.; Xia, J.; Yan, X. Review of construction methods for whole-cell computational models. Syst. Microbiol. Biomanufact. 2021, 2, 259–270. [Google Scholar] [CrossRef]
- Lee, R.; Karr, J.R.; Covert, M.W. WholeCellViz: Data visualization for whole-cell models. BMC Bioinform. 2013, 14, 253. [Google Scholar] [CrossRef] [PubMed]
- Ryu, J.Y.; Kim, H.U.; Lee, S.Y. Deep learning enables high-quality and high-throughput prediction of enzyme commission numbers. Proc. Natl. Acad. Sci. USA 2019, 116, 13996–14001. [Google Scholar] [CrossRef] [PubMed]
- Heckmann, D.; Lloyd, C.J.; Mih, N.; Ha, Y.; Zielinski, D.C.; Haiman, Z.B.; Desouki, A.A.; Lercher, M.J.; Palsson, B.O. Machine learning applied to enzyme turnover numbers reveals protein structural correlates and improves metabolic models. Nat. Commun. 2018, 9, 5252. [Google Scholar] [CrossRef]
- Culley, C.; Vijayakumar, S.; Zampieri, G.; Angione, C. A mechanism-aware and multiomic machine-learning pipeline characterizes yeast cell growth. Proc. Natl. Acad. Sci. USA 2020, 117, 18869–18879. [Google Scholar] [CrossRef] [PubMed]
- John, P.C.S.; Strutz, J.; Broadbelt, L.J.; Tyo, K.E.J.; Bomble, Y.J. Bayesian inference of metabolic kinetics from genome-scale multiomics data. PLOS Comput. Biol. 2019, 15, e1007424. [Google Scholar] [CrossRef]
- Sridhara, V.; Meyer, A.G.; Rai, P.; Barrick, J.E.; Ravikumar, P.; Segrè, D.; Wilke, C.O. Predicting growth conditions from internal metabolic fluxes in an in-silico model of E. coli. PLoS ONE 2014, 9, e114608. [Google Scholar] [CrossRef]
- Folch-Fortuny, A.; Marques, R.; Isidro, I.A.; Oliveira, R.; Ferrer, A. Principal elementary mode analysis (PEMA). Mol. BioSyst. 2016, 12, 737–746. [Google Scholar] [CrossRef]
- Folch-Fortuny, A.; Teusink, B.; Hoefsloot, H.C.; Smilde, A.K.; Ferrer, A. Dynamic elementary mode modelling of non-steady state flux data. BMC Syst. Biol. 2018, 12, 71. [Google Scholar] [CrossRef]
- Wu, S.G.; Wang, Y.; Jiang, W.; Oyetunde, T.; Yao, R.; Zhang, X.; Shimizu, K.; Tang, Y.J.; Bao, F.S. Rapid Prediction of Bacterial Heterotrophic Fluxomics Using Machine Learning and Constraint Programming. PLOS Comput. Biol. 2016, 12, e1004838. [Google Scholar] [CrossRef]
- Bekiaris, P.S.; Klamt, S. Automatic construction of metabolic models with enzyme constraints. BMC Bioinform. 2020, 21, 19. [Google Scholar] [CrossRef]
- Yizhak, K.; Benyamini, T.; Liebermeister, W.; Ruppin, E.; Shlomi, T. Integrating quantitative proteomics and metabolomics with a genome-scale metabolic network model. Bioinformatics 2010, 26, i255–i260. [Google Scholar] [CrossRef] [PubMed]
- Noor, E.; Flamholz, A.; Bar-Even, A.; Davidi, D.; Milo, R.; Liebermeister, W. The Protein Cost of Metabolic Fluxes: Prediction from Enzymatic Rate Laws and Cost Minimization. PLOS Comput. Biol. 2016, 12, e1005167. [Google Scholar] [CrossRef] [PubMed]
- Zeng, H.; Yang, A. Modelling overflow metabolism in Escherichia coli with flux balance analysis incorporating differential proteomic efficiencies of energy pathways. BMC Syst. Biol. 2019, 13, 3. [Google Scholar] [CrossRef]
- Vazquez, A.; Beg, Q.K.; Demenezes, M.A.; Ernst, J.; Bar-Joseph, Z.; Barabási, A.-L.; Boros, L.G.; Oltvai, Z.N. Impact of the solvent capacity constraint on E. coli metabolism. BMC Syst. Biol. 2008, 2, 7. [Google Scholar] [CrossRef] [PubMed]
- Ye, C.; Luo, Q.; Guo, L.; Gao, C.; Xu, N.; Zhang, L.; Liu, L.; Chen, X. Improving lysine production through construction of an Escherichia coli enzyme-constrained model. Biotechnol. Bioeng. 2020, 117, 3533–3544. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Sun, Y.; Liu, Z.; Dong, F.; Li, Y.; Wang, Y. Genome-scale modeling for Bacillus coagulans to understand the metabolic characteristics. Biotechnol. Bioeng. 2020, 117, 3545–3558. [Google Scholar] [CrossRef] [PubMed]
- Zhou, J.; Zhuang, Y.; Xia, J. Integration of enzyme constraints in a genome-scale metabolic model of Aspergillus niger improves phenotype predictions. Microb. Cell Fact. 2021, 20, 125. [Google Scholar] [CrossRef]
- Chassagnole, C.; Noisommit-Rizzi, N.; Schmid, J.W.; Mauch, K.; Reuss, M. Dynamic modeling of the central carbon metabolism of Escherichia coli. Biotechnol. Bioeng. 2002, 79, 53–73. [Google Scholar] [CrossRef]
- Saa, P.; Nielsen, L.K. A probabilistic framework for the exploration of enzymatic capabilities based on feasible kinetics and control analysis. Biochim. Biophys. Acta (BBA) -Gen. Subj. 2016, 1860, 576–587. [Google Scholar] [CrossRef][Green Version]
- Saa, P.; Nielsen, L.K. Construction of feasible and accurate kinetic models of metabolism: A Bayesian approach. Sci. Rep. 2016, 6, 29635. [Google Scholar] [CrossRef]
- Lee, Y.; Rivera, J.G.L.; Liao, J.C. Ensemble Modeling for Robustness Analysis in engineering non-native metabolic pathways. Metab. Eng. 2014, 25, 63–71. [Google Scholar] [CrossRef]
- Andreozzi, S.; Miskovic, L.; Hatzimanikatis, V. Ischrunk—In Silico Approach to Characterization and Reduction of Uncertainty in the Kinetic Models of Genome-scale Metabolic Networks. Metab. Eng. 2016, 33, 158–168. [Google Scholar] [CrossRef] [PubMed]
- Smith, R.W.; Van Rosmalen, R.P.; Dos Santos, V.A.P.M.; Fleck, C. DMPy: A Python package for automated mathematical model construction of large-scale metabolic systems. BMC Syst. Biol. 2018, 12, 72. [Google Scholar] [CrossRef]
- Haiman, Z.B.; Zielinski, D.C.; Koike, Y.; Yurkovich, J.T.; Palsson, B.O. MASSpy: Building, simulating, and visualizing dynamic biological models in Python using mass action kinetics. PLOS Comput. Biol. 2021, 17, e1008208. [Google Scholar] [CrossRef] [PubMed]
- Khodayari, A.; Maranas, C.D. A genome-scale Escherichia coli kinetic metabolic model k-ecoli457 satisfying flux data for multiple mutant strains. Nat. Commun. 2016, 7, 13806. [Google Scholar] [CrossRef] [PubMed]
- Gauvry, E.; Mathot, A.-G.; Couvert, O.; Leguérinel, I.; Jules, M.; Coroller, L. Differentiation of Vegetative Cells into Spores: A Kinetic Model Applied to Bacillus subtilis. Appl. Environ. Microbiol. 2019, 85, e00322-19. [Google Scholar] [CrossRef]
- Dash, S.; Khodayari, A.; Zhou, J.; Holwerda, E.K.; Olson, D.G.; Lynd, L.R.; Maranas, C.D. Development of a core Clostridium thermocellum kinetic metabolic model consistent with multiple genetic perturbations. Biotechnol. Biofuels 2017, 10, 108. [Google Scholar] [CrossRef]
- Thiele, I.; Jamshidi, N.; Fleming, R.M.; Palsson, B. Genome-scale reconstruction of Escherichia coli’s transcriptional and translational machinery: A knowledge base, its mathematical formulation, and its functional characterization. PLoS Comput. Biol. 2009, 5, e1000312. [Google Scholar] [CrossRef]
- Liu, J.K.; O’Brien, E.J.; Lerman, J.A.; Zengler, K.; Palsson, B.O.; Feist, A.M. Reconstruction and modeling protein translocation and compartmentalization in Escherichia coli at the genome-scale. BMC Syst. Biol. 2014, 8, 110. [Google Scholar] [CrossRef]
- King, Z.; Lloyd, C.J.; Feist, A.M.; Palsson, B.O. Next-generation genome-scale models for metabolic engineering. Curr. Opin. Biotechnol. 2015, 35, 23–29. [Google Scholar] [CrossRef]
- Lloyd, C.J.; Ebrahim, A.; Yang, L.; King, Z.; Catoiu, E.; O’Brien, E.J.; Liu, J.K.; Palsson, B.O. COBRAme: A computational framework for genome-scale models of metabolism and gene expression. PLOS Comput. Biol. 2018, 14, e1006302. [Google Scholar] [CrossRef] [PubMed]
- Lerman, J.; Hyduke, D.R.; Latif, H.; Portnoy, V.A.; Lewis, N.; Orth, J.D.; Rutledge, A.; Smith, R.; Adkins, J.; Zengler, K.; et al. In silico method for modelling metabolism and gene product expression at genome scale. Nat. Commun. 2012, 3, 929. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.K.; Lloyd, C.; Al-Bassam, M.M.; Ebrahim, A.; Kim, J.-N.; Olson, C.; Aksenov, A.; Dorrestein, P.; Zengler, K. Predicting proteome allocation, overflow metabolism, and metal requirements in a model acetogen. PLOS Comput. Biol. 2019, 15, e1006848. [Google Scholar] [CrossRef] [PubMed]
- Oftadeh, O.; Salvy, P.; Masid, M.; Curvat, M.; Miskovic, L.; Hatzimanikatis, V. A genome-scale metabolic model of Saccharomyces cerevisiae that integrates expression constraints and reaction thermodynamics. Nat. Commun. 2021, 12, 4790. [Google Scholar] [CrossRef] [PubMed]
- Cruz, F.; Faria, J.P.; Rocha, M.; Rocha, I.; Dias, O. A review of methods for the reconstruction and analysis of integrated genome-scale models of metabolism and regulation. Biochem. Soc. Trans. 2020, 48, 1889–1903. [Google Scholar] [CrossRef]
- Faria, J.; Overbeek, R.; Xia, F.; Rocha, M.; Rocha, I.; Henry, C.S. Genome-scale bacterial transcriptional regulatory networks: Reconstruction and integrated analysis with metabolic models. Briefings Bioinform. 2013, 15, 592–611. [Google Scholar] [CrossRef]
- Chandrasekaran, S.; Price, N.D. Metabolic Constraint-Based Refinement of Transcriptional Regulatory Networks. PLOS Comput. Biol. 2013, 9, e1003370. [Google Scholar] [CrossRef]
- Wang, Z.; Danziger, S.A.; Heavner, B.D.; Ma, S.; Smith, J.J.; Li, S.; Herricks, T.; Simeonidis, E.; Baliga, N.S.; Aitchison, J.D.; et al. Combining inferred regulatory and reconstructed metabolic networks enhances phenotype prediction in yeast. PLOS Comput. Biol. 2017, 13, e1005489. [Google Scholar] [CrossRef]
- Ma, S.; Minch, K.J.; Rustad, T.R.; Hobbs, S.; Zhou, S.-L.; Sherman, D.R.; Price, N.D. Integrated Modeling of Gene Regulatory and Metabolic Networks in Mycobacterium tuberculosis. PLOS Comput. Biol. 2015, 11, e1004543. [Google Scholar] [CrossRef]
- Friedman, A.; Perrimon, N. Genetic Screening for Signal Transduction in the Era of Network Biology. Cell 2007, 128, 225–231. [Google Scholar] [CrossRef]
- Nyfeler, B.; Michnick, S.W.; Hauri, H.-P. Capturing protein interactions in the secretory pathway of living cells. Proc. Natl. Acad. Sci. USA 2005, 102, 6350–6355. [Google Scholar] [CrossRef]
- Chen, Y.; Nielsen, J. Mathematical modeling of proteome constraints within metabolism. Curr. Opin. Syst. Biol. 2021, 25, 50–56. [Google Scholar] [CrossRef]
- Rose, P.W.; Bi, C.; Bluhm, W.F.; Christie, C.H.; Dimitropoulos, D.; Dutta, S.; Green, R.K.; Goodsell, D.S.; Prlic, A.; Quesada, M.; et al. The RCSB Protein Data Bank: New resources for research and education. Nucleic Acids Res. 2012, 41, D475–D482. [Google Scholar] [CrossRef] [PubMed]
- Chang, R.L.; Andrews, K.; Kim, D.; Li, Z.; Godzik, A.; Palsson, B.O. Structural Systems Biology Evaluation of Metabolic Thermotolerance in Escherichia coli. Science 2013, 340, 1220–1223. [Google Scholar] [CrossRef] [PubMed]
- Elsemman, I.E.; Prado, A.R.; Grigaitis, P.; Albornoz, M.G.; Harman, V.; Holman, S.W.; van Heerden, J.; Bruggeman, F.J.; Bisschops, M.M.M.; Sonnenschein, N.; et al. Whole-cell modeling in yeast predicts compartment-specific proteome constraints that drive metabolic strategies. Nat. Commun. 2022, 13, 801. [Google Scholar] [CrossRef] [PubMed]
- Carrera, J.; Estrela, R.; Luo, J.; Rai, N.; Tsoukalas, A.; Tagkopoulos, I. An integrative, multi-scale, genome-wide model reveals the phenotypic landscape of Escherichia coli. Mol. Syst. Biol. 2014, 10, 735. [Google Scholar] [CrossRef]
- Monk, J.M.; Lloyd, C.J.; Brunk, E.; Mih, N.; Sastry, A.; King, Z.; Takeuchi, R.; Nomura, W.; Zhang, Z.; Mori, H.; et al. iML1515, a knowledgebase that computes Escherichia coli traits. Nat. Biotechnol. 2017, 35, 904–908. [Google Scholar] [CrossRef]
- Lu, H.; Li, F.; Sánchez, B.; Zhu, Z.; Li, G.; Domenzain, I.; Marcišauskas, S.; Anton, P.M.; Lappa, D.; Lieven, C.; et al. A consensus S. cerevisiae metabolic model Yeast8 and its ecosystem for comprehensively probing cellular metabolism. Nat. Commun. 2019, 10, 3586. [Google Scholar] [CrossRef]
- Carrera, J.; Covert, M.W. Why build whole-cell models? Trends Cell Biol. 2015, 25, 719–722. [Google Scholar] [CrossRef]
- Karr, J.R.; Takahashi, K.; Funahashi, A. The principles of whole-cell modeling. Curr. Opin. Microbiol. 2015, 27, 18–24. [Google Scholar] [CrossRef]
- Hamilton, J.; Reed, J.L. Software platforms to facilitate reconstructing genome-scale metabolic networks. Environ. Microbiol. 2013, 16, 49–59. [Google Scholar] [CrossRef] [PubMed]
- Saha, R.; Chowdhury, A.; Maranas, C.D. Recent advances in the reconstruction of metabolic models and integration of omics data. Curr. Opin. Biotechnol. 2014, 29, 39–45. [Google Scholar] [CrossRef] [PubMed]
- Kolpakov, F.; Akberdin, I.; Kiselev, I.; Kolmykov, S.; Kondrakhin, Y.; Kulyashov, M.; Kutumova, E.; Pintus, S.; Ryabova, A.; Sharipov, R.; et al. BioUML—Towards a universal research platform. Nucleic Acids Res. 2022, gkac286. [Google Scholar] [CrossRef] [PubMed]
- Smith, R.N.; Aleksic, J.; Butano, D.; Carr, A.; Contrino, S.; Hu, F.; Lyne, M.; Lyne, R.; Kalderimis, A.; Rutherford, K.; et al. InterMine: A flexible data warehouse system for the integration and analysis of heterogeneous biological data. Bioinformatics 2012, 28, 3163–3165. [Google Scholar] [CrossRef]
- Malik-Sheriff, R.S.; Glonț, M.; Nguyen, T.V.N.; Tiwari, K.; Roberts, M.G.; Xavier, A.; Vu, M.T.; Men, J.; Maire, M.; Kananathan, S.; et al. BioModels—15 years of sharing computational models in life science. Nucleic Acids Res. 2019, 48, D407–D415. [Google Scholar] [CrossRef]
- Norsigian, C.J.; Pusarla, N.; McConn, J.L.; Yurkovich, J.T.; Dräger, A.; Palsson, B.O.; King, Z. BiGG Models 2020: Multi-strain genome-scale models and expansion across the phylogenetic tree. Nucleic Acids Res. 2019, 48, D402–D406. [Google Scholar] [CrossRef]
- Salis, H.; Sotiropoulos, V.; Kaznessis, Y.N. Multiscale Hy3S: Hybrid stochastic simulation for supercomputers. BMC Bioinform. 2006, 7, 93. [Google Scholar] [CrossRef]
- Takahashi, K.; Kaizu, K.; Hu, B.; Tomita, M. A multi-algorithm, multi-timescale method for cell simulation. Bioinformatics 2004, 20, 538–546. [Google Scholar] [CrossRef]
- Holzhütter, H.; Drasdo, D.; Preusser, T.; Lippert, J.; Henney, A.M. The virtual liver: A multidisciplinary, multilevel challenge for systems biology. WIREs Syst. Biol. Med. 2012, 4, 221–235. [Google Scholar] [CrossRef]
- Kou, S.; Cheng, D.; Sun, F.; Hsing, I.-M. Microfluidics and microbial engineering. Lab. Chip 2015, 16, 432–446. [Google Scholar] [CrossRef]
- Henry, C.S.; DeJongh, M.; Best, A.A.; Frybarger, P.M.; Linsay, B.; Stevens, R.L. High-throughput generation, optimization and analysis of genome-scale metabolic models. Nat. Biotechnol. 2010, 28, 977–982. [Google Scholar] [CrossRef] [PubMed]
- Karr, J.; Phillips, N.C.; Covert, M.W. Whole Cell SimDB: A hybrid relational/HDF database for whole-cell model predictions. Database 2014, 2014, bau095. [Google Scholar] [CrossRef] [PubMed]
- Karr, J.R.; Sanghvi, J.C.; Macklin, D.N.; Gutschow, M.V.; Jacobs, J.M.; Bolival, B.; Assad-Garcia, N.; Glass, J.I.; Covert, M.W. A Whole-Cell Computational Model Predicts Phenotype from Genotype. Cell 2012, 150, 389–401. [Google Scholar] [CrossRef] [PubMed]
- Macklin, D.N.; Ahn-Horst, T.A.; Choi, H.; Ruggero, N.A.; Carrera, J.; Mason, J.C.; Sun, G.; Agmon, E.; DeFelice, M.M.; Maayan, I.; et al. Simultaneous cross-evaluation of heterogeneous E. coli datasets via mechanistic simulation. Science 2020, 369, eaav3751. [Google Scholar] [CrossRef]
- Ye, C.; Xu, N.; Gao, C.; Liu, G.-Q.; Xu, J.; Zhang, W.; Chen, X.; Nielsen, J.; Liu, L. Comprehensive understanding of Saccharomyces cerevisiae phenotypes with whole-cell model WM_S288C. Biotechnol. Bioeng. 2020, 117, 1562–1574. [Google Scholar] [CrossRef]
- Zhang, J.; Petersen, S.D.; Radivojevic, T.; Ramirez, A.; Pérez-Manríquez, A.; Abeliuk, E.; Sánchez, B.J.; Costello, Z.; Chen, Y.; Fero, M.J.; et al. Combining mechanistic and machine learning models for predictive engineering and optimization of tryptophan metabolism. Nat. Commun. 2020, 11, 4880. [Google Scholar] [CrossRef]
- Costello, Z.; Martin, H.G. A machine learning approach to predict metabolic pathway dynamics from time-series multiomics data. NPJ Syst. Biol. Appl. 2018, 4, 19. [Google Scholar] [CrossRef]
- Bhadra, S.; Blomberg, P.; Castillo, S.; Rousu, J. Principal metabolic flux mode analysis. Bioinformatics 2018, 34, 2409–2417. [Google Scholar] [CrossRef]
- Szappanos, B.; Kovács, K.; Szamecz, B.; Honti, F.; Costanzo, M.; Baryshnikova, A.; Gelius-Dietrich, G.; Lercher, M.; Jelasity, M.; Myers, C.L.; et al. An integrated approach to characterize genetic interaction networks in yeast metabolism. Nat. Genet. 2011, 43, 656–662. [Google Scholar] [CrossRef]
- Tokuyama, K.; Ohno, S.; Yoshikawa, K.; Hirasawa, T.; Tanaka, S.; Furusawa, C.; Shimizu, H. Increased 3-hydroxypropionic acid production from glycerol, by modification of central metabolism in Escherichia coli. Microb. Cell Factories 2014, 13, 64. [Google Scholar] [CrossRef]
- Kim, T.Y.; Park, J.M.; Kim, H.U.; Cho, K.M.; Lee, S.Y. Design of homo-organic acid producing strains using multi-objective optimization. Metab. Eng. 2014, 28, 63–73. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Qi, H.; Wang, C.; Wen, J. Model-driven intracellular redox status modulation for increasing isobutanol production in Escherichia coli. Biotechnol. Biofuels 2015, 8, 108. [Google Scholar] [CrossRef] [PubMed]
- Mienda, B.S.; Salihu, R.; Adamu, A.; Idris, S. Genome-scale metabolic models as platforms for identification of novel genes as antimicrobial drug targets. Futur. Microbiol. 2018, 13, 455–467. [Google Scholar] [CrossRef] [PubMed]
- Rau, M.H.; Zeidan, A.A. Constraint-based modeling in microbial food biotechnology. Biochem. Soc. Trans. 2018, 46, 249–260. [Google Scholar] [CrossRef]

| Model Type | Year | Algorithm/Framework | Language | Task | Reference |
|---|---|---|---|---|---|
| Constraint-based models | 2007 | TMFA | MATLAB | Thermodynamic constraint model | [36] |
| 2019 | MatTFA, pyTFA | MATLAB, Python | Toolkit of build thermodynamic constraint model | [41] | |
| 2007 | FBAwMC | MATLAB | Calculation scheme of enzyme concentration | [46] | |
| 2012 | MOMENT | MATLAB | Enzymatic constraint model | [47] | |
| 2017 | GECKO | MATLAB | Comprehensive framework for enzyme constraint models | [48] | |
| 2006 | Structural Kinetic Modeling | MATLAB | Dynamic analysis of metabolic systems | [49] | |
| 2008 | MASS framework | MATLAB | Evaluate the dynamic properties of the model and formulate a timescale hierarchy | [50] | |
| 2010 | ORACLE | MATLAB | Introducing the state space of the enzyme into the model | [51] | |
| 2008 | Ensemble Modelling | MATLAB | Framework for Steady-State kinetics model | [52] | |
| 2016 | ABC-GRASP | MATLAB | Framework for modeling uncertain dynamics data | [53] | |
| 2021 | ETGEM | Python | Framework of enzyme constraints and thermodynamic constraints | [54] | |
| 2020 | Expression and Thermodynamics Flux models | Python | Multi-omics integrated framework | [55] | |
| Multi-scale Integrated models | 2011 | TIGER | MATLAB | Integrate TRN and GEM platforms | [56] |
| 2015 | FlexFlux | Java | Integrate TRN and GEM platforms | [57] | |
| 2010 | Probabilistic Regulation of Metabolism | MATLAB | Toolkit of integrate TRN and GEM | [58] | |
| 2017 | TRFBA | MATLAB | Toolkit of integrate TRN and GEM | [59] | |
| 2019 | OptRAM | MATLAB | Predict optimal metabolic flux in TRN-integrated GEM | [60] | |
| 2016 | GEM-PRO | MATLAB | Integration of protein structure with GEM | [61] | |
| 2019 | GEMMER | Python + Java | Database for multiscale modeling | [62] | |
| Whole cell model | 2006 | GEM System | Java | Toolbox for building metabolic pathways in whole-cell models | [63] |
| 2021 | Pathway Tools | Python + Java | Software for pathway and genetic data | [64] | |
| 2013 | WholeCellKB | Python + SQL | Database of whole-cell models | [65] | |
| 2020 | CellML | XML | Mathematical models describing cellular physiological systems | [66] | |
| 2003 | E-Cell | C++ | Multiplatform cell simulation system | [67] | |
| 2014 | CellDesigner | SBML | modeling tool for biochemical networks | [68] | |
| 2009 | Complex pathway simulator | SBML | Software for biochemical network modeling and simulation | [69] | |
| 2009 | Biochemical simulations | Python | Random mixture algorithm | [70] | |
| 2014 | WholeCellSimDB | Python + Java | Database of whole-cell model predictions. | [71] | |
| 2013 | WholeCellViz | Java + SOL | visualization for whole-cell models | [72] | |
| Machine learning-based models | 2019 | DeepEC | Python | EC number prediction by deep learning | [73] |
| 2020 | ART, TeselaGen EVOLVE | Python | Multi-level training datasets for accurate prediction | [74] | |
| 2020 | BEMKL, bagged random forest, multimodal artificial neural network, sparse group lasso, NSGA-II, iterative random forests | Python | Multiomics and multimodal algorithms to predict phenotypes | [75] | |
| 2020 | AMMEDEUS | Python | Tools to identify changes in model structure | [76] | |
| 2014 | regularized multinomial logistic regression | MATLAB | Tool for phenotypic inverse prediction of growth conditions | [77] | |
| 2016 | primary elementary modal analysis | Python | Identifying metabolic patterns in fluxomics based on metabolic pathways | [78] | |
| 2018 | dynEMR-DA | MATLAB | Algorithm for environment-driven dynamic performance discrimination | [79] | |
| 2016 | support vector machines, k-nearest neighbors, decision trees | MATLAB | Method for rapid prediction of bacterial heterotrophic fluxomics | [80] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bi, X.; Liu, Y.; Li, J.; Du, G.; Lv, X.; Liu, L. Construction of Multiscale Genome-Scale Metabolic Models: Frameworks and Challenges. Biomolecules 2022, 12, 721. https://doi.org/10.3390/biom12050721
Bi X, Liu Y, Li J, Du G, Lv X, Liu L. Construction of Multiscale Genome-Scale Metabolic Models: Frameworks and Challenges. Biomolecules. 2022; 12(5):721. https://doi.org/10.3390/biom12050721
Chicago/Turabian StyleBi, Xinyu, Yanfeng Liu, Jianghua Li, Guocheng Du, Xueqin Lv, and Long Liu. 2022. "Construction of Multiscale Genome-Scale Metabolic Models: Frameworks and Challenges" Biomolecules 12, no. 5: 721. https://doi.org/10.3390/biom12050721
APA StyleBi, X., Liu, Y., Li, J., Du, G., Lv, X., & Liu, L. (2022). Construction of Multiscale Genome-Scale Metabolic Models: Frameworks and Challenges. Biomolecules, 12(5), 721. https://doi.org/10.3390/biom12050721









