Untethered Origami Worm Robot with Diverse Multi-Leg Attachments and Responsive Motions under Magnetic Actuation
Abstract
:1. Introduction
2. Materials and Methods
2.1. Fabrication of Soft Robot
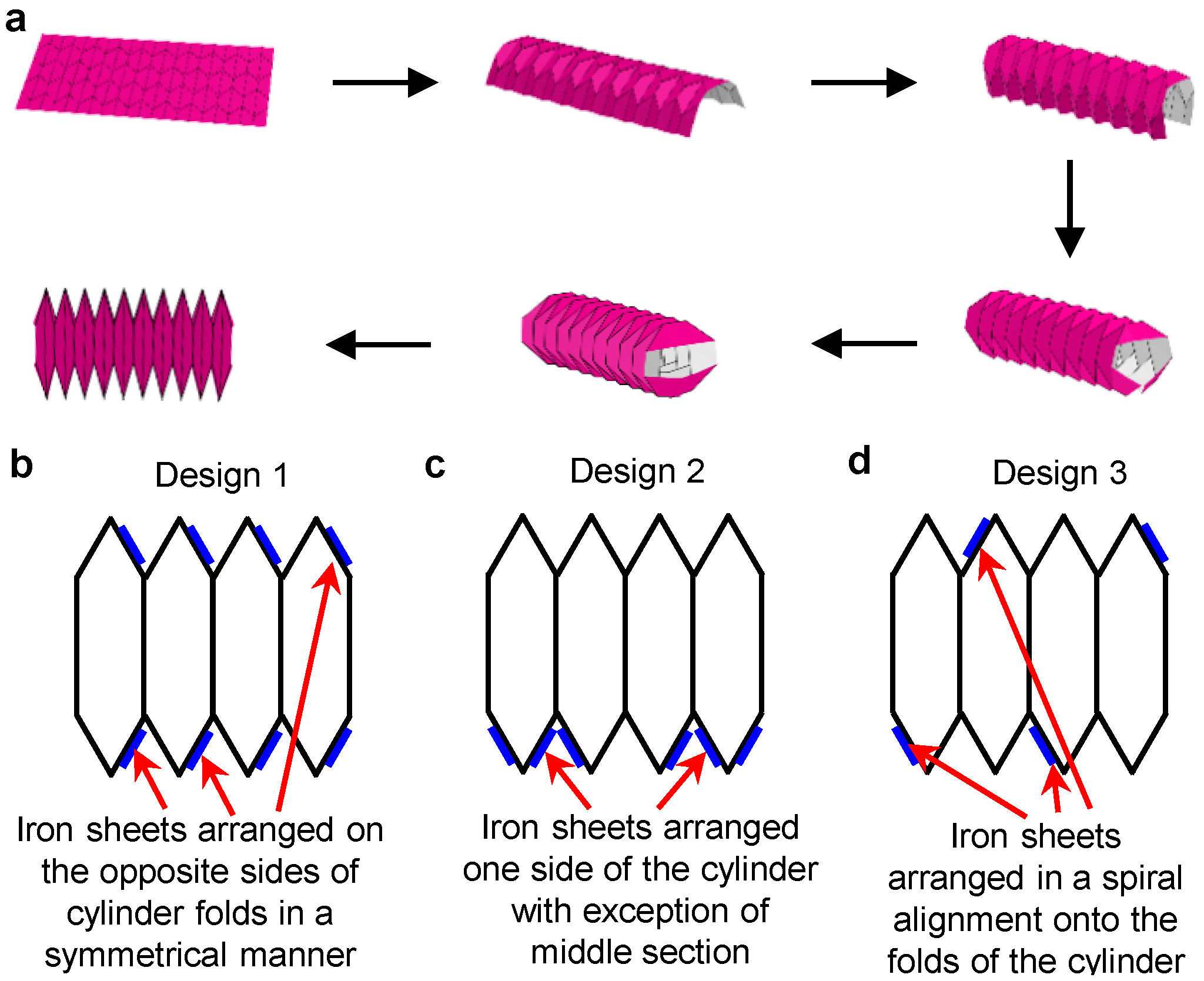
2.2. Configuration of Iron Sheets in the Soft Robot
2.3. Actuating Magnet
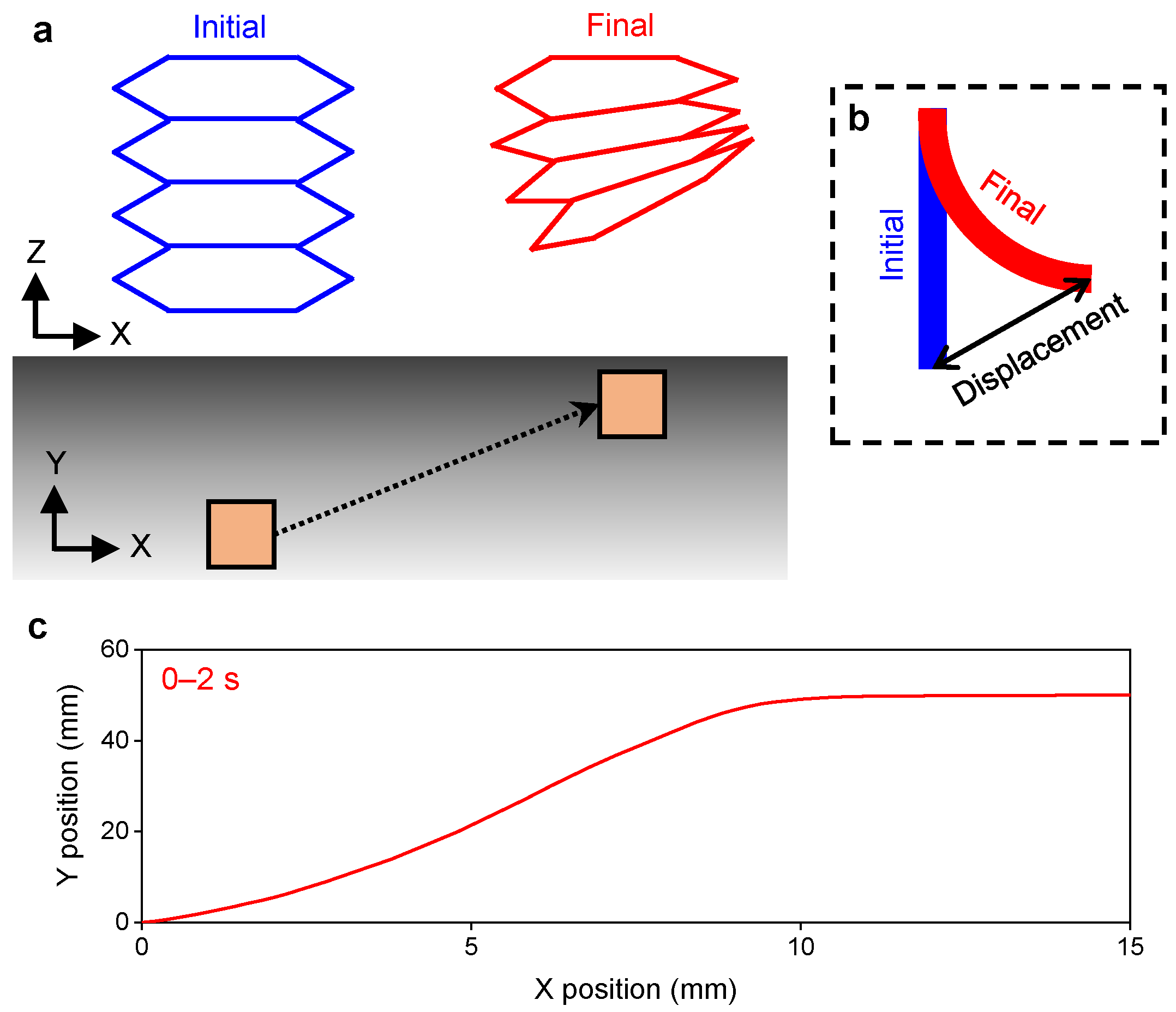
3. Results and Discussions
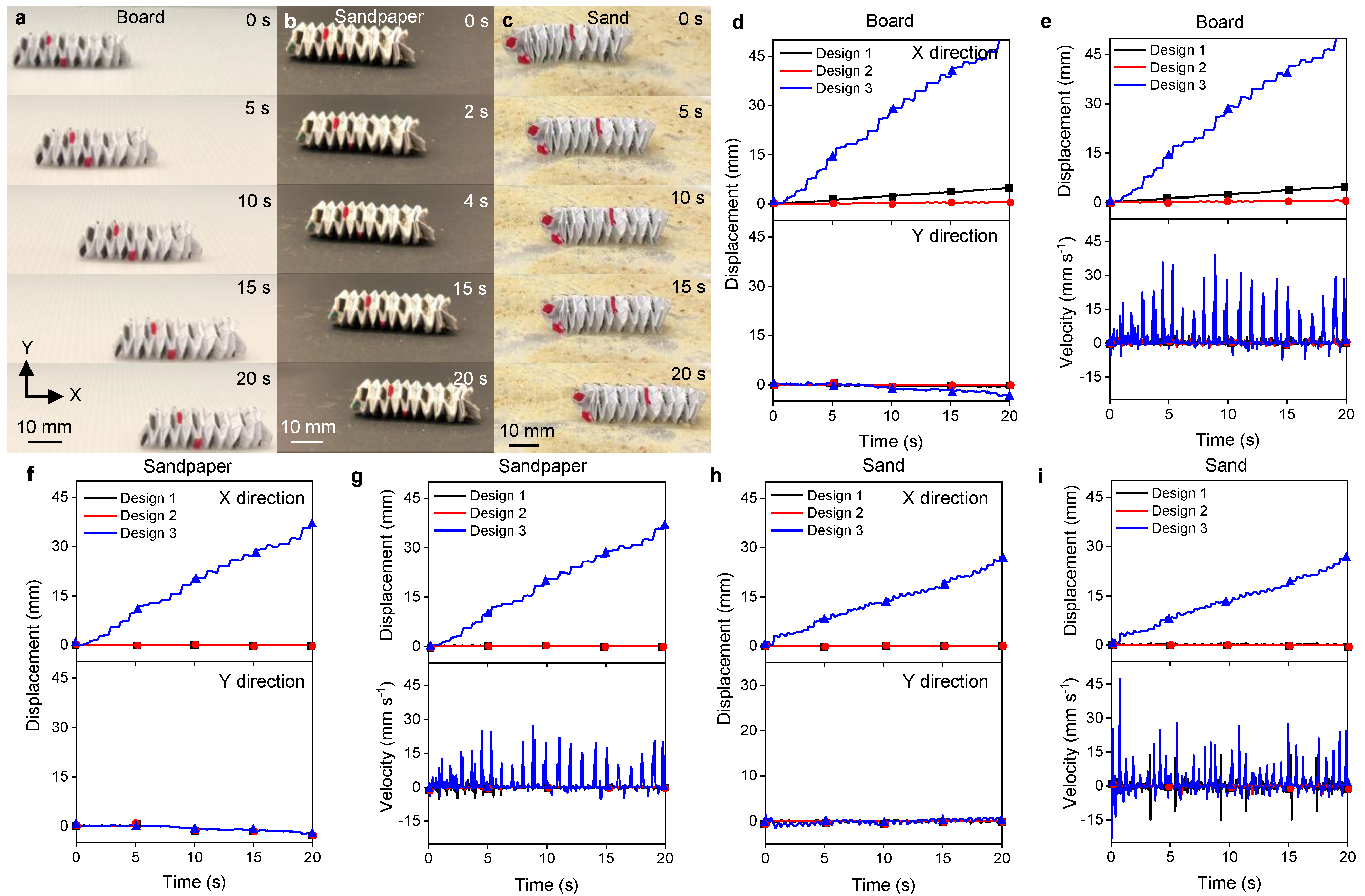
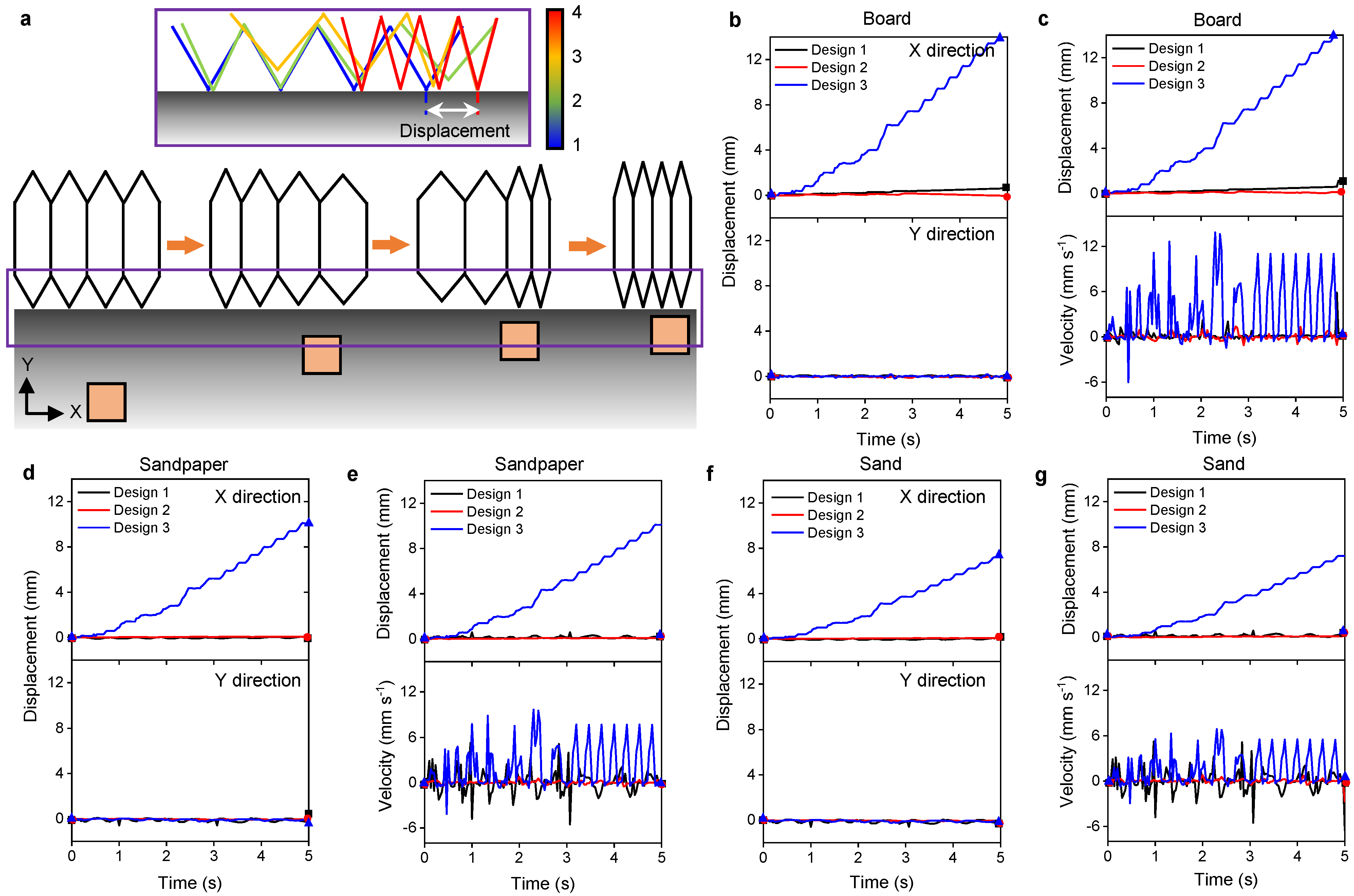
3.1. Peristaltic Motion
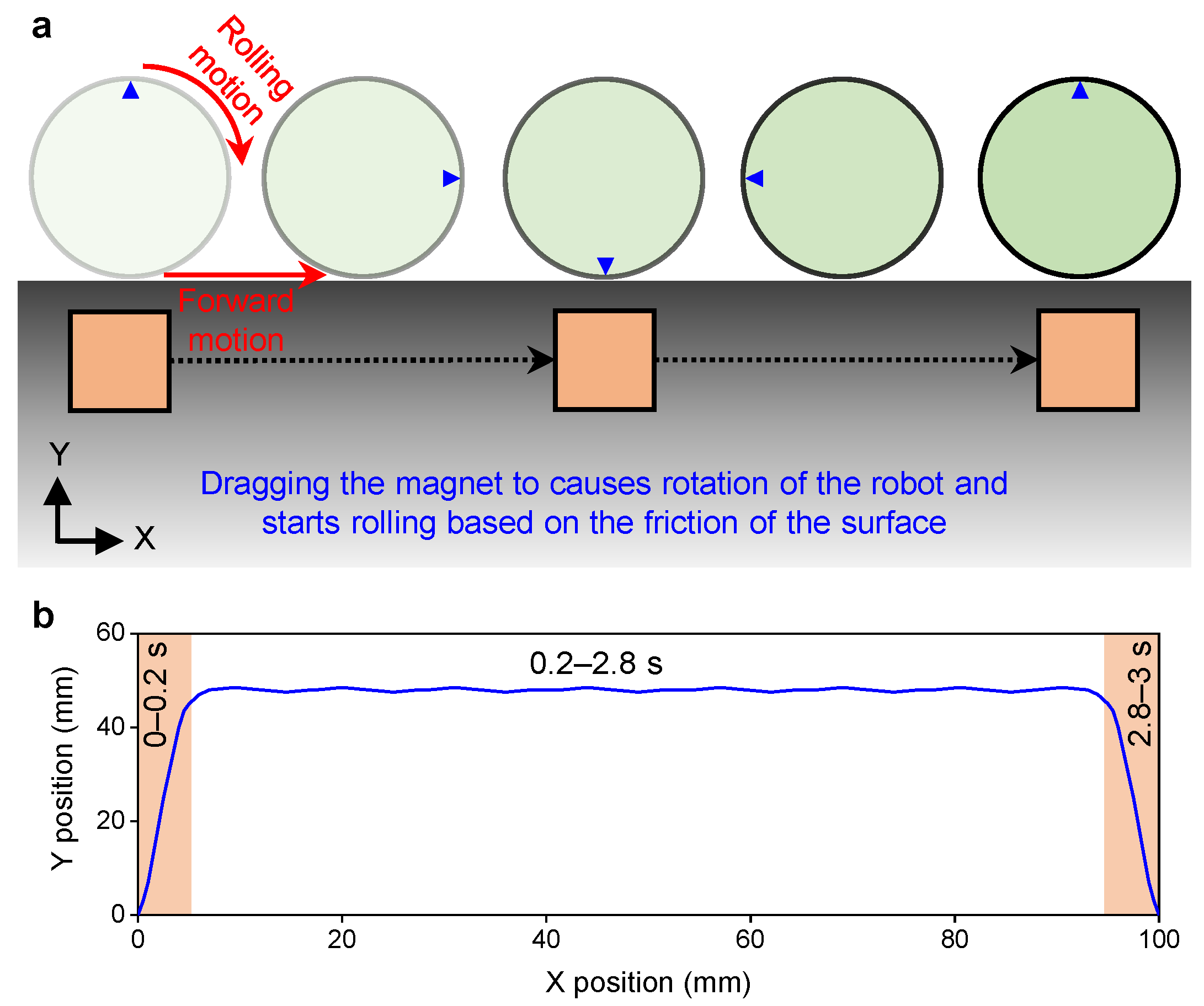
3.1.1. Rolling Motion
3.1.2. Turning Motion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Banerjee, H.; Pusalkar, N.; Ren, H. Single-motor controlled tendon-driven peristaltic soft origami robot. J. Mech. Robot. 2018, 10, 064501. [Google Scholar] [CrossRef] [Green Version]
- Yang, H.; Yeow, B.S.; Chang, T.H.; Li, K.; Fu, F.; Ren, H.; Chen, P.Y. Graphene Oxide-Enabled Synthesis of Metal Oxide Origamis for Soft Robotics. ACS Nano 2019, 13, 5410–5420. [Google Scholar] [CrossRef]
- Yang, H.; Yeow, B.S.; Li, Z.; Li, K.; Chang, T.H.; Jing, L.; Li, Y.; Ho, J.S.; Ren, H.; Chen, P.Y. Multifunctional metallic backbones for origami robotics with strain sensing and wireless communication capabilities. Sci. Robot. 2019, 4, 59. [Google Scholar] [CrossRef] [PubMed]
- Paez, L.; Agarwal, G.; Paik, J. Design and analysis of a soft pneumatic actuator with origami shell reinforcement. Soft Robot. 2016, 3, 109–119. [Google Scholar] [CrossRef]
- Zhai, Z.; Wang, Y.; Jiang, H. Origami-inspired, on-demand deployable and collapsible mechanical metamaterials with tunable stiffness. Proc. Natl. Acad. Sci. USA 2018, 115, 2032–2037. [Google Scholar] [CrossRef] [Green Version]
- Reid, A.; Lechenault, F.; Rica, S.; Adda-Bedia, M. Geometry and design of origami bellows with tunable response. Phys. Rev. E 2017, 95, 013002. [Google Scholar] [CrossRef] [Green Version]
- Wang, F.; Gong, H.; Chen, X.; Chen, C. Folding to curved surfaces: A generalized design method and mechanics of origami-based cylindrical structures. Sci. Rep. 2016, 6, 33312. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schenk, M.; Viquerat, A.D.; Seffen, K.A.; Guest, S.D. Review of inflatable booms for deployable space structures: Packing and rigidization. J. Spacecr. Rocket. 2014, 51, 762–778. [Google Scholar] [CrossRef] [Green Version]
- Cowan, B.; Von Lockette, P.R. Fabrication, characterization, and heuristic trade space exploration of magnetically actuated Miura-Ori origami structures. Smart Mater. Struct. 2017, 26, 045015. [Google Scholar] [CrossRef]
- Kuribayashi, K.; Tsuchiya, K.; You, Z.; Tomus, D.; Umemoto, M.; Ito, T.; Sasaki, M. Self-deployable origami stent grafts as a biomedical application of Ni-rich TiNi shape memory alloy foil. Mater. Sci. Eng. A 2006, 419, 131–137. [Google Scholar] [CrossRef]
- Camarillo, D.B.; Milne, C.F.; Carlson, C.R.; Zinn, M.R.; Salisbury, J.K. Mechanics modeling of tendon-driven continuum manipulators. IEEE Trans. Robot. 2008, 24, 1262–1273. [Google Scholar] [CrossRef]
- Dong, S. Review on piezoelectric, ultrasonic, and magnetoelectric actuators. J. Adv. Dielectr. 2012, 2, 1230001. [Google Scholar] [CrossRef]
- Conway, N.J.; Traina, Z.J.; Kim, S.G. A strain amplifying piezoelectric MEMS actuator. J. Micromech. Microeng. 2007, 17, 781. [Google Scholar] [CrossRef]
- Bowen, L.; Springsteen, K.; Frecker, M.; Simpson, T. Trade space exploration of magnetically actuated origami mechanisms. J. Mech. Robot. 2016, 8, 031012. [Google Scholar] [CrossRef]
- Onal, C.D.; Wood, R.J.; Rus, D. An origami-inspired approach to worm robots. IEEE/ASME Trans. Mechatron. 2012, 18, 430–438. [Google Scholar] [CrossRef]
- Kalairaj, M.S.; Banerjee, H.; Lim, C.M.; Chen, P.Y.; Ren, H. Hydrogel-matrix encapsulated Nitinol actuation with self-cooling mechanism. RSC Adv. 2019, 9, 34244–34255. [Google Scholar] [CrossRef] [Green Version]
- Kalairaj, M.S.; Yeow, B.S.; Lim, C.M.; Ren, H. Nitinol actuated soft structures towards transnasal drug delivery: A pilot cadaver study. Med Biol. Eng. Comput. 2020, 58, 611–623. [Google Scholar] [CrossRef]
- Kalairaj, M.S.; Yeow, B.S.; Lim, C.M.; Ren, H. Needle-size bending actuators based on controlled nitinol curvatures and elastic structures. J. Mech. Robot. 2020, 12, 031015. [Google Scholar] [CrossRef]
- Banerjee, H.; Sivaperuman Kalairaj, M.; Chang, T.H.; Fu, F.; Chen, P.Y.; Ren, H. Highly Stretchable Flame-Retardant Skin for Soft Robotics with Hydrogel–Montmorillonite-Based Translucent Matrix. Soft Robot. 2021. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.; Ke, X.; Jiang, Q.; Ding, H.; Wu, Z. Programmable and reprocessable multifunctional elastomeric sheets for soft origami robots. Sci. Robot. 2021, 6, eabd6107. [Google Scholar] [CrossRef]
- Tang, D.; Zhang, C.; Sun, H.; Dai, H.; Xie, J.; Fu, J.; Zhao, P. Origami-inspired magnetic-driven soft actuators with programmable designs and multiple applications. Nano Energy 2021, 89, 106424. [Google Scholar] [CrossRef]
- Salerno, M.; Firouzeh, A.; Paik, J. A low profile electromagnetic actuator design and model for an origami parallel platform. J. Mech. Robot. 2017, 9, 041005. [Google Scholar] [CrossRef]
- Cai, C.J.; Xiao, X.; Kalairaj, M.S.; Lee, I.J.J.; Mugilvannan, A.K.; Yeow, B.S.; Tan, J.H.; Huang, H.; Ren, H. Diversified and untethered motion generation via crease patterning from magnetically actuated caterpillar-inspired origami robot. IEEE/ASME Trans. Mechatron. 2020, 26, 1678–1688. [Google Scholar] [CrossRef]
- Xiang, X.; Lu, G.; Ruan, D.; You, Z.; Zolghadr, M. Large deformation of an arc-Miura structure under quasi-static load. Compos. Struct. 2017, 182, 209–222. [Google Scholar] [CrossRef]
- Fang, H.; Zhang, Y.; Wang, K. Origami-based earthworm-like locomotion robots. Bioinspir. Biomimet. 2017, 12, 065003. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Fang, H.; Xu, J. Yoshimura-origami Based Earthworm-like Robot With 3-dimensional Locomotion Capability. Front. Robot. AI 2021, 8, 271. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sivaperuman Kalairaj, M.; Cai, C.J.; S, P.; Ren, H. Untethered Origami Worm Robot with Diverse Multi-Leg Attachments and Responsive Motions under Magnetic Actuation. Robotics 2021, 10, 118. https://doi.org/10.3390/robotics10040118
Sivaperuman Kalairaj M, Cai CJ, S P, Ren H. Untethered Origami Worm Robot with Diverse Multi-Leg Attachments and Responsive Motions under Magnetic Actuation. Robotics. 2021; 10(4):118. https://doi.org/10.3390/robotics10040118
Chicago/Turabian StyleSivaperuman Kalairaj, Manivannan, Catherine Jiayi Cai, Pavitra S, and Hongliang Ren. 2021. "Untethered Origami Worm Robot with Diverse Multi-Leg Attachments and Responsive Motions under Magnetic Actuation" Robotics 10, no. 4: 118. https://doi.org/10.3390/robotics10040118






