A Review of Aeroengines’ Bolt Preload Formation Mechanism and Control Technology
Abstract
1. Introduction
2. Bolt Preload Formation Mechanism
2.1. Preload Formation Mechanism of a Single Bolt
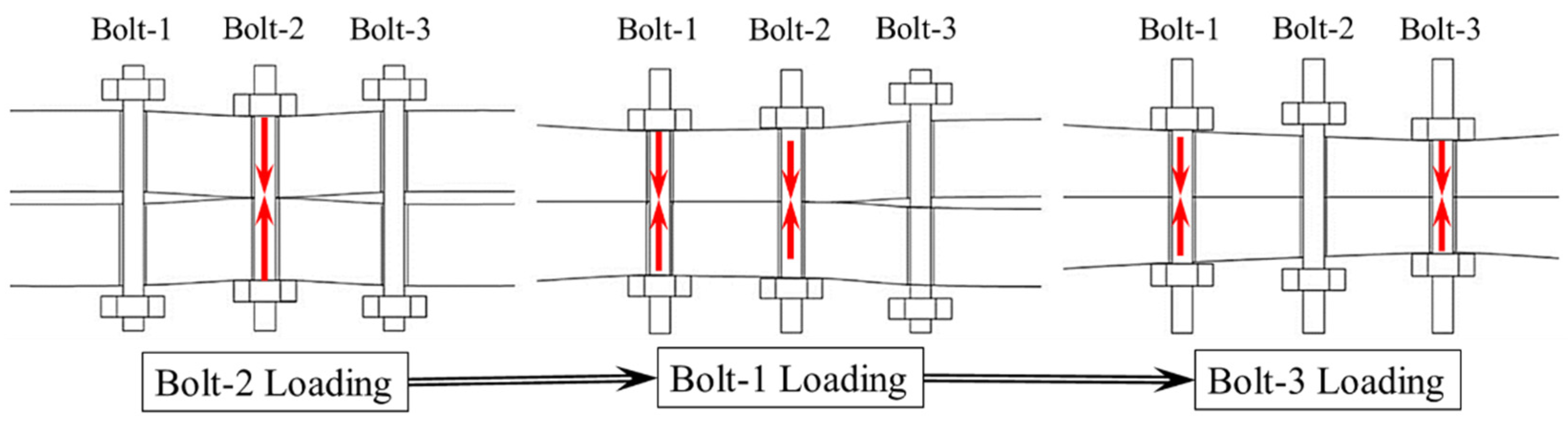
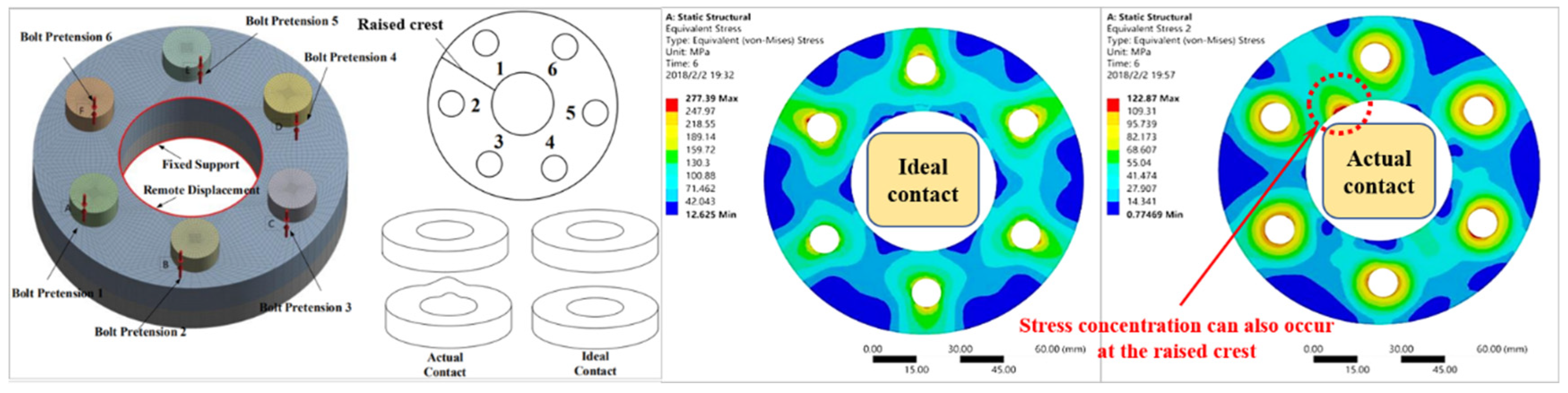
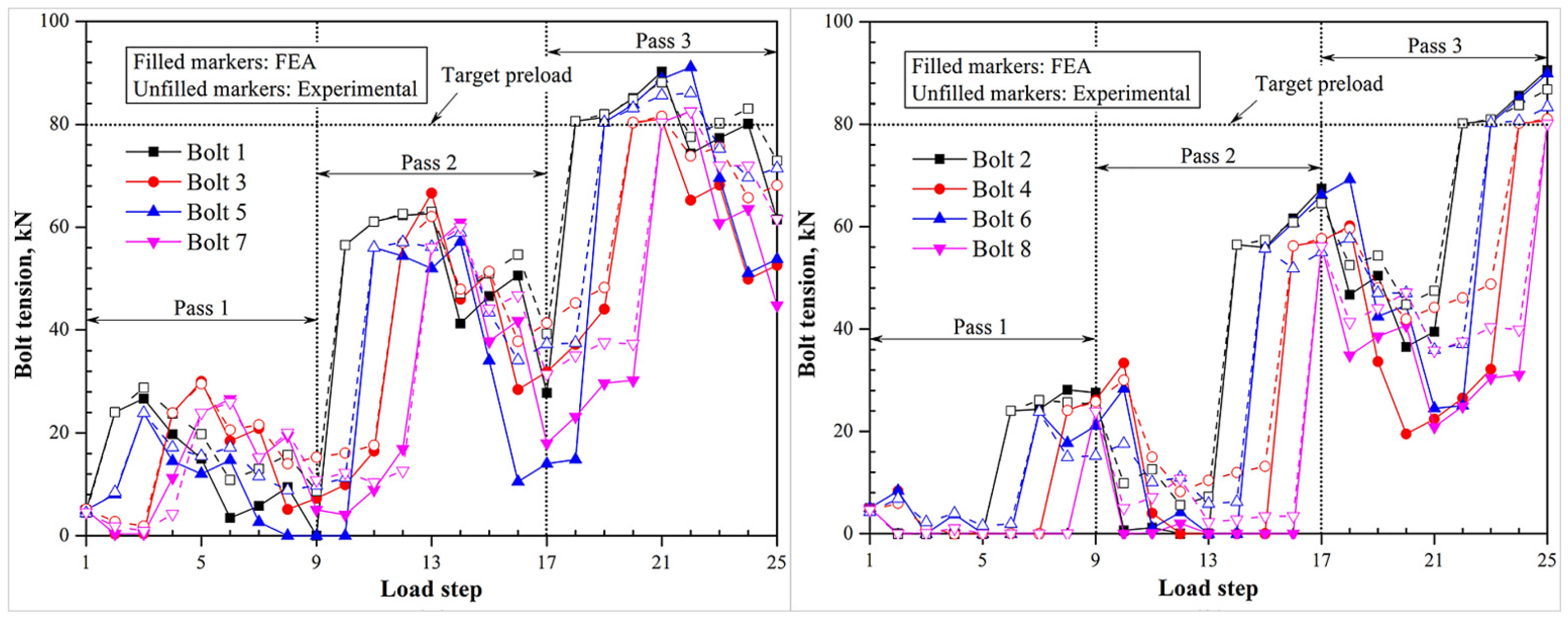
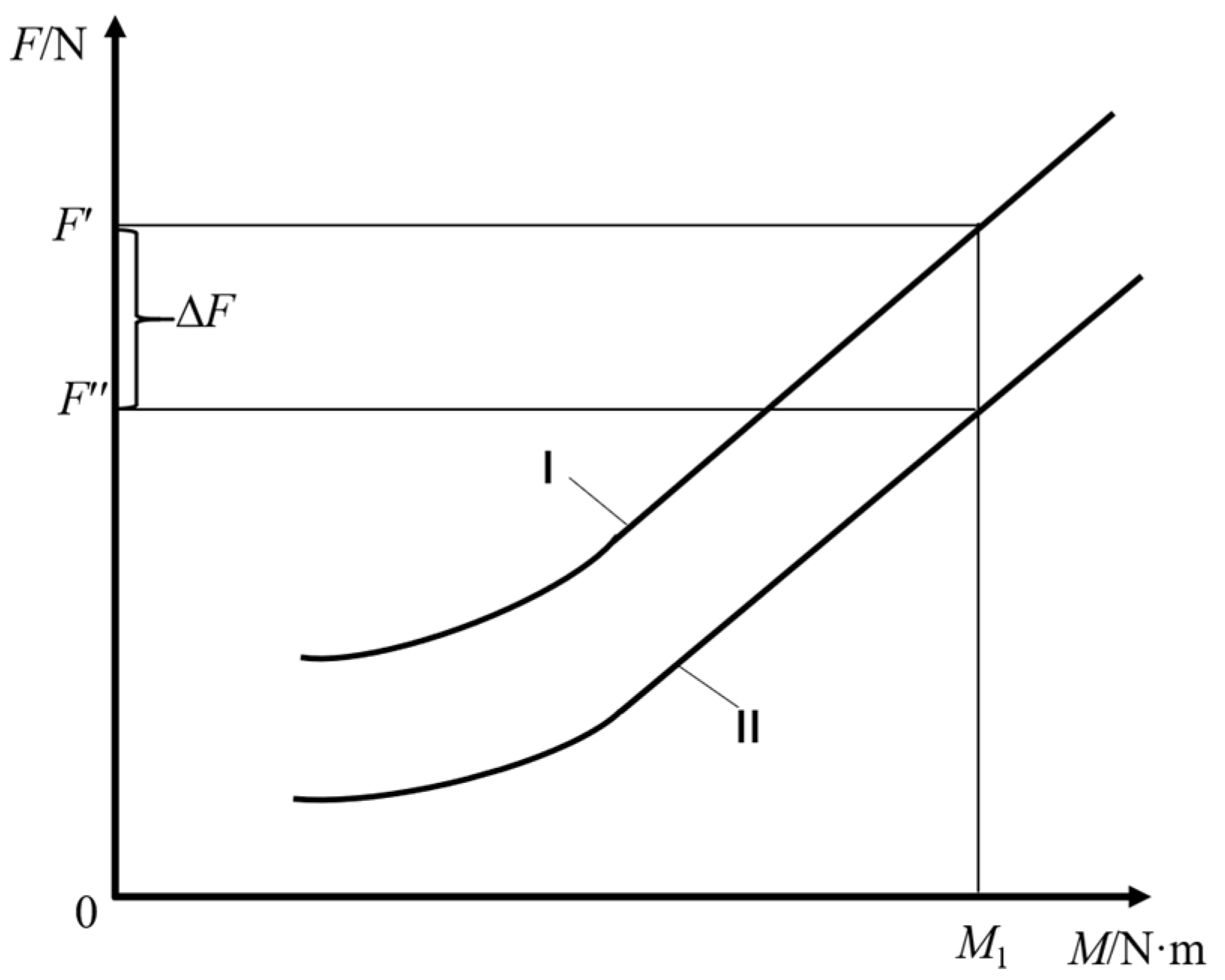
2.2. Preload Formation Mechanism of Multiple Bolts
3. Bolt Tightening Process
3.1. Bolt Tightening Process Parameters
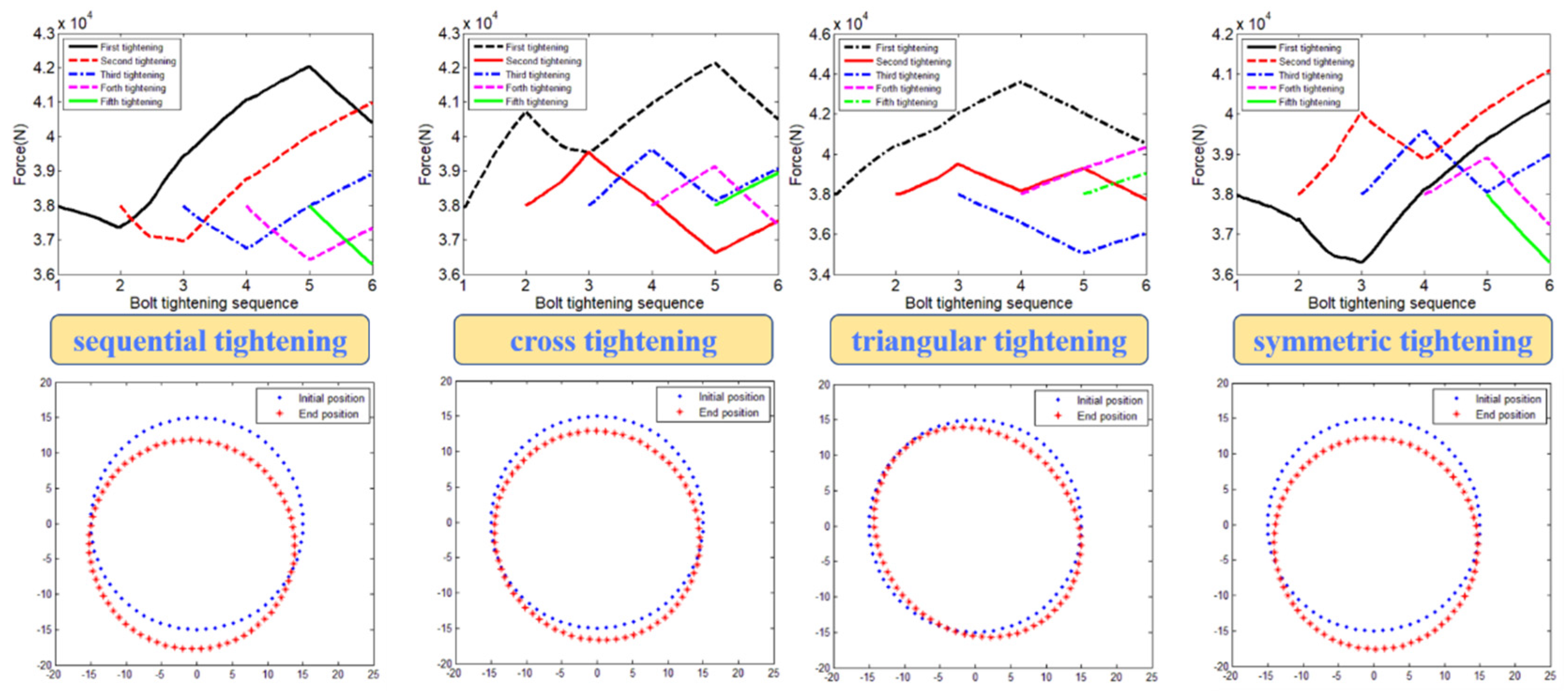
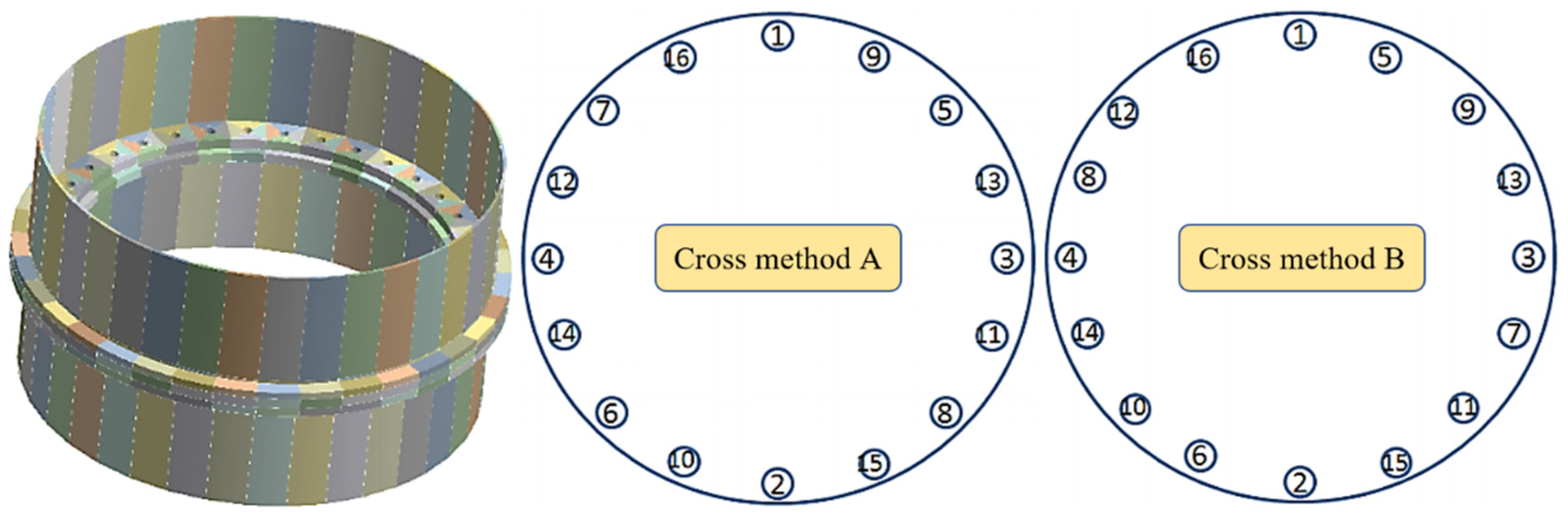
3.2. Bolt Tightening Method
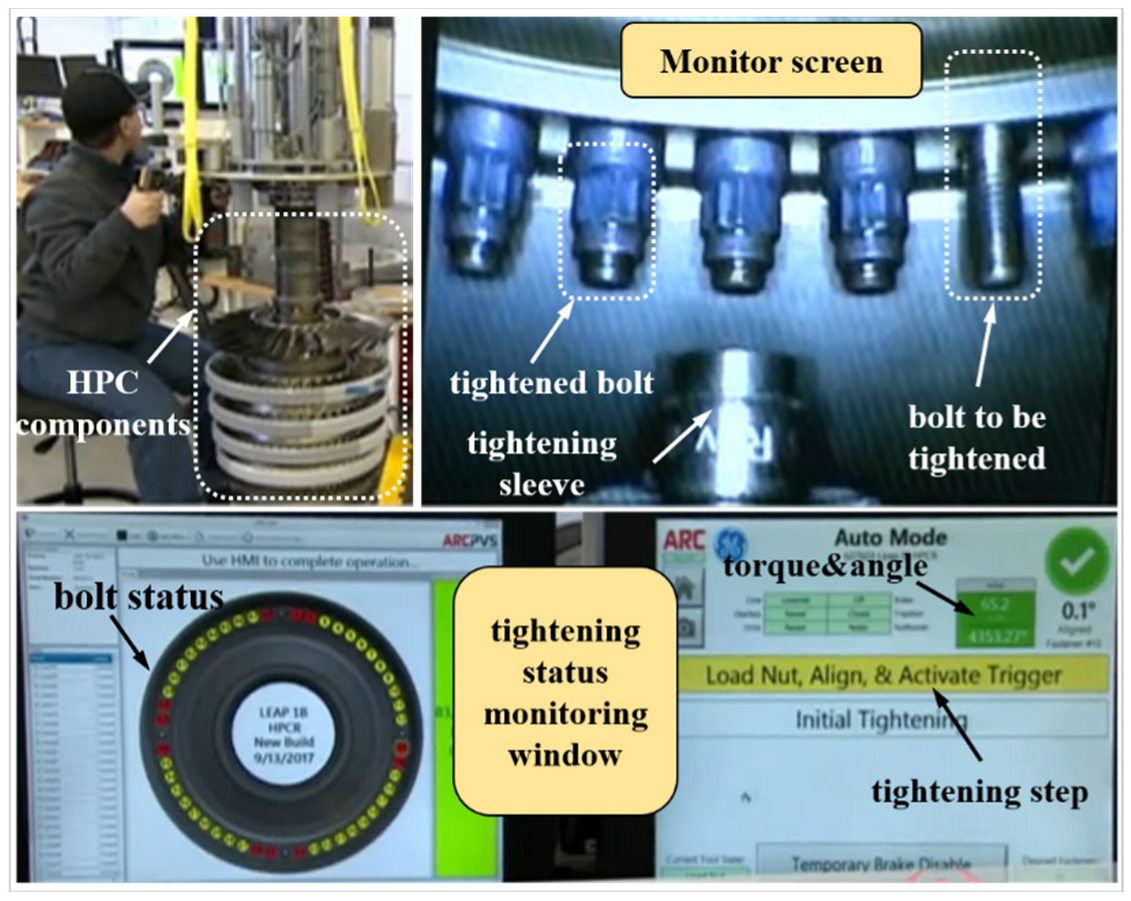
4. Bolt Tightening Equipment
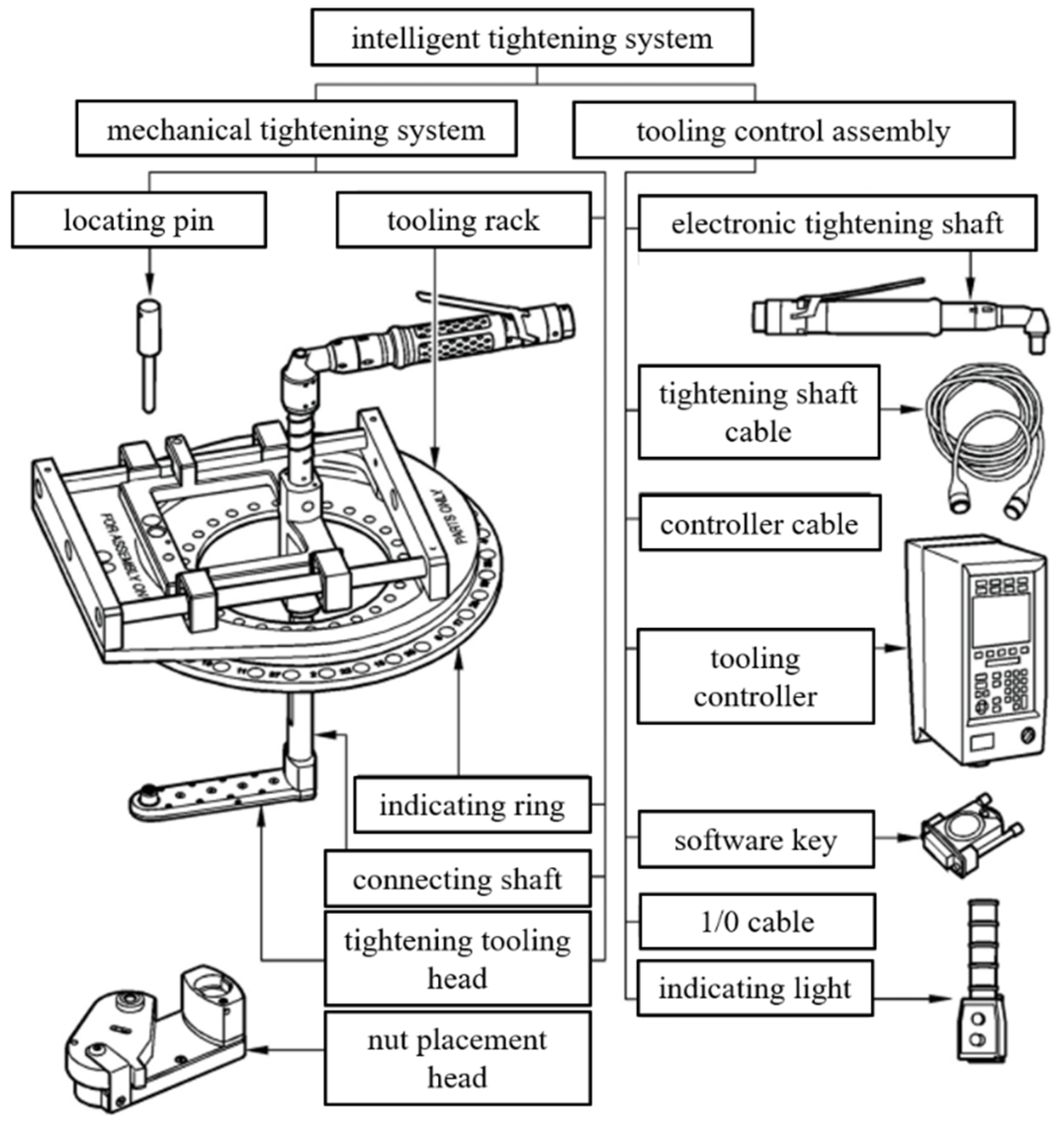
4.1. Open-Space Tightening Equipment
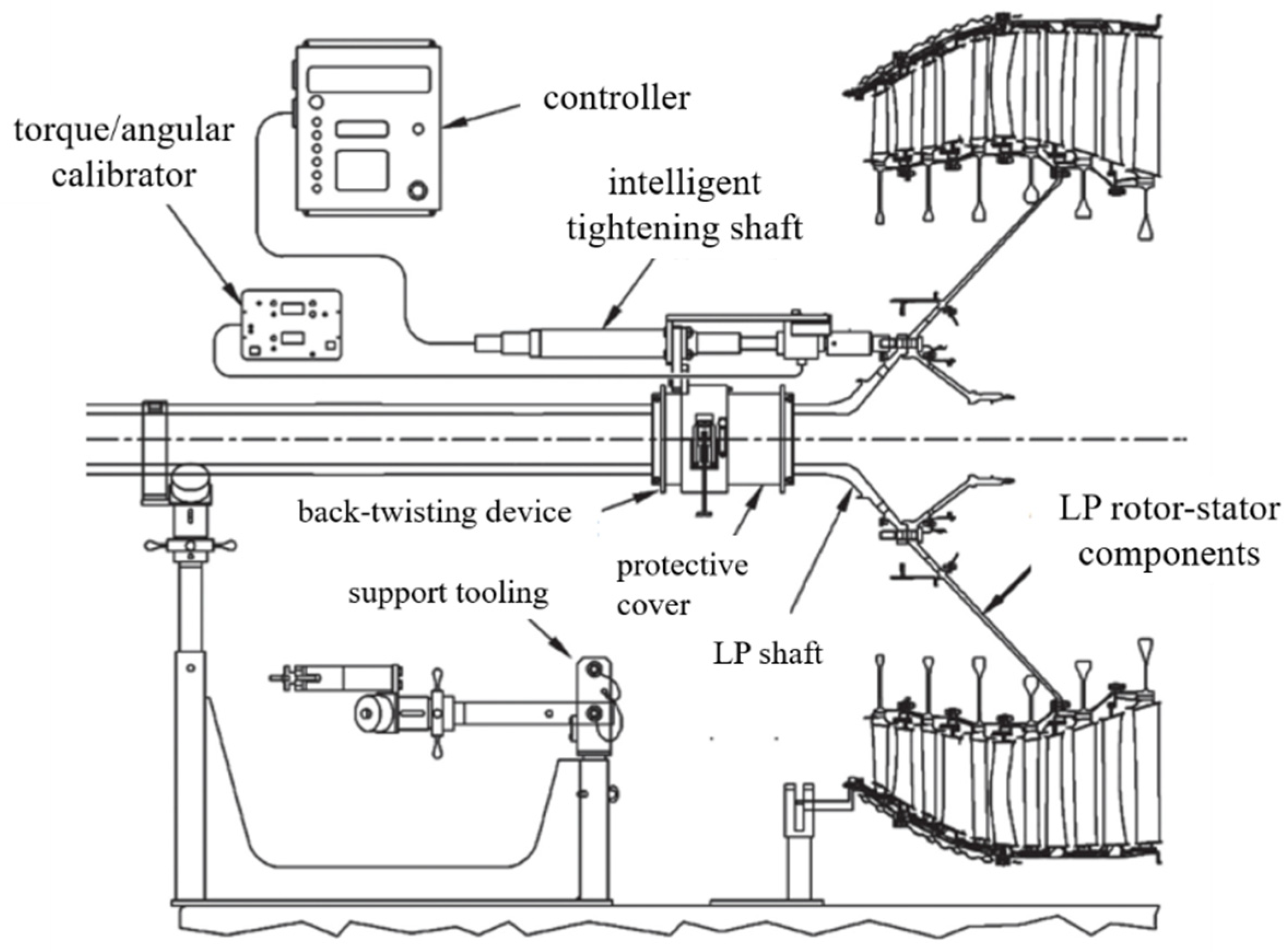
4.2. Narrow-Space Tightening Equipment
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, Y.; Liang, C.; Shi, L. General Design of Aviation Gas Turbine; Science Press: Beijing, China, 2021. [Google Scholar]
- Yan, Q. Research on Assembly Tightness Detection Method for Aeroengine Bolt-Jointed Rotor; Chang’an University: Xi’an, China, 2019. [Google Scholar]
- Zhao, G.; Wang, Y.; Zhao, X.; Li, S.; Teng, G. Modeling and application test of contact stiffness of bolt connection structure of disk and drum. J. Aeronaut. Dyn. 2022, 37, 76–86. [Google Scholar]
- Jiao, J.; Mo, R.; Xu, G.; Fu, X.; Sun, H.; Chang, Z. Influence of position error of bolt hole on assembly mechanical characteristic of short precision bolted connection structure. J. Aeronaut. Dyn. 2021, 36, 935–947. [Google Scholar]
- Li, X.; Chen, G.; Yu, P.; Yang, M. Nonlinear stiffness mechanism analysis and numerical simulation of rabbet-bolted connection structure. J. Aeronaut. Dyn. 2021, 36, 358–368. [Google Scholar]
- Wang, K.; Yan, M.; Sun, Z.; Su, D.; Hui, A.; Liu, H. Influence factors of nonlinear stiffness of bolted flange connection structure under different loads. J. Aeronaut. Dyn. 2021, 36, 2503–2514. [Google Scholar]
- Ai, Y.; Lai, C.; Han, Y.; Sun, D.; Wu, L. Experiment on sealing characteristics of bolts flanged connections for aero-engines. J. Aeronaut. Dyn. 2018, 33, 2315–2323. [Google Scholar]
- Yu, P.; Zhao, Z.; Hou, L.; Chen, G. Analysis on analytical modelling and damping characteristics of bolted joint structure with spigot. J. Aeronaut. Dyn. 2021, 36, 2490–2502. [Google Scholar]
- Deng, W.; Mo, R.; Chen, K.; Feng, X.; Xia, H.; Sun, H.; Chang, Z. Prediction of rotor blade tip assembly clearance based on measured data for aero-engine. J. Aeronaut. Dyn. 2022, 37, 1273–1283. [Google Scholar]
- Bai, S.; Zhai, X.; AI, Y.; Ma, W. The influence of Bolted Connection on the Coaxial Tolerance and Dynamic Analysis in Aero-Engine Casing. J. Aeronaut. Dyn. 2010, 6, 35–37. [Google Scholar]
- Hong, J.; Xu, X.; Su, Z.; Ma, Y. Joint stiffness loss and vibration characteristics of high-speed rotor. J. Beihang Univ. 2019, 45, 18–25. [Google Scholar]
- Fan, Z. Study on the Influence of Bolt Preload on the Non-Continuous Rotor Dynamics of Aeroengine; Harbin Institute of Technology: Harbin, China, 2020. [Google Scholar]
- Sun, W.; Li, T.; Yang, D.; Sun, Q.; Huo, J. Dynamic investigation of aeroengine high pressure rotor system considering assembly characteristics of bolted joints. Eng. Fail. Anal. 2020, 112, 104510. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, D.; Hong, J.; Wu, F.; Jiang, G.; Huang, H. Analysis of Whole Aeroengine Vibration Control Technology. Aircr. Engine 2013, 39, 1–8+13. [Google Scholar]
- Sun, S.; Li, Y. Research on the Influence of Manufacturing Error of Aeroengine Rotor Installation Edge on Assembly Performance. Mod. Manuf. Technol. Equip. 2020, 56, 126–131. [Google Scholar]
- Zhang, B. Study on Loosening Regular Pattern of Bolt Connection of Low Pressure Turbine Shaft and Disc; Dalian University of Technology: Dalian, China, 2019. [Google Scholar]
- Zhang, X. Experimental Research on Evolution Law of Aero-Engine Bolt Connection Loosening Characteristics; Dalian University of Technology: Dalian, China, 2021. [Google Scholar]
- Zhang, B. Research on Precise Control of Pre Preload of Shaft Disk Connection of Low Pressure Turbine of Aero Engine; Dalian University of Technology: Dalian, China, 2018. [Google Scholar]
- HB/Z 251-1993; Relationship between Tightening Torque and Axial Force of Bolted Connections. China National Aviation Industry Corporation: Beijing, China, 1994.
- GB/T 16823.3-2010; Fasteners Torque-Clamp Force Testing. Standards Press of China: Beijing, China, 2011.
- Motosh, N. Development of Design Charts for Bolts Preloaded up to the Plastic Range. J. Eng. Ind. 1976, 98, 849–851. [Google Scholar] [CrossRef]
- Gong, H.; Liu, J.; Ding, X. Calculation of the effective bearing contact radius for precision tightening of bolted joints. Adv. Mech. Eng. 2016, 8, 1–8. [Google Scholar] [CrossRef]
- Ganeshmurthy, S.; Nassar, S. Finite Element Simulation of Process Control of Bolt Tightening for Joints with Non-Parallel Contact. In Volume 2: Computer Technology and Bolted Joints; American Society of Mechanical Engineers: Toronto, ON, Canada, 2012; pp. 343–351. [Google Scholar]
- Nassar, S.; Matin, P.; Barber, G. Thread Friction Torque in Bolted Joints. In Analysis of Bolted Joints; ASMEDC: San Diego, CA, USA, 2004; pp. 145–154. [Google Scholar]
- Ganeshmurthy, S.; Nassar, S. Study of Two-Stage Tightening of Threaded Fasteners Using Various Tool Speed Combinations. In Volume 2: Computer Applications/Technology and Bolted Joints; ASMEDC: San Antonio, TX, USA, 2007; pp. 295–308. [Google Scholar]
- Eccles, W.; Sherrington, I.; Arnell, R. Frictional changes during repeated tightening of zinc plated threaded fasteners. Tribol. Int. 2010, 43, 700–707. [Google Scholar] [CrossRef]
- Graboń, W.; Mucha, J.; Osetek, M.; Szlachta, J. Influence of different thermochemical treatments of bolts on tightening parameters of a bolted joint. Strength Mater. 2016, 48, 495–506. [Google Scholar] [CrossRef]
- Pi, Z. Study on Reliability Design and Tightening Technology of Screw Connection; Wuhan University of Technology: Wuhan, China, 2012. [Google Scholar]
- Meng, A.; Yang, X.; Nassar, S. Effect of Bearing Friction and Hole Clearance on the Clamp Load-Deformation Correlation in Bolted Joints. SAE Trans. 2007, 699–706. [Google Scholar] [CrossRef]
- Liu, C.; Tu, D. Research on Influence Factors of friction coefficient of electro galvanized bolts. Lubr. Seal. 2014, 39, 38–42. [Google Scholar]
- Croccolo, D.; De Agostinis, M.; Vincenzi, N. Failure analysis of bolted joints: Effect of friction coefficients in torque–preloading relationship. Eng. Fail. Anal. 2011, 18, 364–373. [Google Scholar] [CrossRef]
- Zou, Q.; Sun, T.; Nassar, S.; Barber, G.; El-Khiamy, H.; Zhu, D. Contact Mechanics Approach to Determine Effective Radius in Bolted Joints. J. Tribol. 2005, 127, 30–36. [Google Scholar] [CrossRef]
- Nassar, S.; Ranganathan, R.; Ganeshmurthy, S.; Barber, G. Effect of Tightening Speed on the Torque-Tension and Wear Pattern in Bolted Connections. In Volume 2: Computer Technology; Vancouver, B.C., Ed.; ASMEDC: Toronto, ONT, Canada, 2006; pp. 169–182. [Google Scholar]
- Nassar, S.; Sun, T.; Zou, Q. The Effect of Coating and Tightening Speed on the Torque-Tension Relationship in Threaded Fasteners; SAE Technical Paper: Detroit, Michigan, 2006. [Google Scholar]
- Nassar, S.; Yang, X. Novel Formulation of the Tightening and Breakaway Torque Components in Threaded Fasteners. J. Press. Vessel Technol. 2007, 129, 653–663. [Google Scholar] [CrossRef]
- Nassar, S.; Sun, T. Surface roughness effect on the torque-tension relationship in threaded fasteners. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2007, 221, 95–103. [Google Scholar] [CrossRef]
- Chen, D. Study on the Bolt Pretension and Residual Torque Forming under Geometric Deviations; Dalian University: Dalian, China, 2019. [Google Scholar]
- Liu, Y. Analysis of Formation Mechanism of Bolt Pretension and Its Fatigue Performance Influence under Manufacturing Deviation; Dalian University of Technology: Dalian, China, 2021. [Google Scholar]
- Bibel, G. Experimental and analytical study of elastic interaction in a pipe flange. In Proceedings of the International Conference on Pressure Vessel Technology, Dusseldorf, Germany, 31 May–5 June 1992. [Google Scholar]
- Bibel, G.; Ezell, R. An Improved Flange Bolt-Up Procedure Using Experimentally Determined Elastic Interaction Coefficients. J. Press. Vessel Technol. 1992, 114, 439–443. [Google Scholar] [CrossRef]
- Takaki, T.; Fukuoka, T. Effective Bolting Up Procedure Using Finite Element Analysis and Elastic Interaction Coefficient Method. In Analysis of Bolted Joints; ASMEDC: San Diego, CA, USA, 2004; pp. 155–162. [Google Scholar]
- Alkelani, A.; Nassar, S.; Housari, B. Formulation of Elastic Interaction BETWEEN Bolts during the Tightening of Flat-Face Gasketed Joints. J. Mech. Des. 2009, 131, 021004. [Google Scholar] [CrossRef]
- Nassar, S.; Alkelani, A. Clamp Load Loss due to Elastic Interaction and Gasket Creep Relaxation in Bolted Joints. J. Press. Vessel Technol. 2006, 128, 394–401. [Google Scholar] [CrossRef]
- Nassar, S.; Yang, X. Novel Formulation of Bolt Elastic Interaction in Gasketed Joints. J. Press. Vessel Technol. 2009, 131, 051204. [Google Scholar] [CrossRef]
- Nassar, S.; Wu, Z.; Yang, X. Achieving Uniform Clamp Load in Gasketed Bolted Joints Using a Nonlinear Finite Element Model. J. Press. Vessel Technol. 2010, 132, 031205. [Google Scholar] [CrossRef]
- Abasolo, M.; Aguirrebeitia, J.; Avilés, R. Methodology for the Optimization of Bolting Sequences for Wind Generator Flanges. J. Press. Vessel Technol. 2014, 136, 061202. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, J.; Liu, H.; Xu, S. Modeling and numerical analysis of multi-bolt elastic interaction with bolt stress relaxation. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2016, 230, 2579–2587. [Google Scholar] [CrossRef]
- Grzejda, R. Determination of bolt forces and normal contact pressure between elements in the system with many bolts for its assembly conditions. Adv. Sci. Technol. Res. J. 2019, 13, 116–121. [Google Scholar] [CrossRef]
- Ye, Y. Study on Pre-Preload and Deformation of Bolted Connection with Rabbet Considering Actual Contact; Dalian University of Technology: Dalian, China, 2019. [Google Scholar]
- Ibrahim, R.; Pettit, C. Uncertainties and dynamics problems of bolted joints and other fasteners. J. Sound Vib. 2005, 279, 857–936. [Google Scholar] [CrossRef]
- Hou, B. Mechanical Properties of Bolted Connection Structure under Real Contact Surface Topography; Dalian University of Technology: Dalian, China, 2020. [Google Scholar]
- Chen, C.; Yang, G.; Chang, D.; Hong, J. Assembly Connection Design Orienting to Sealing Performance of Joint Surface. J. Xi’an Jiaotong Univ. 2012, 46, 75–83. [Google Scholar]
- Hao, K.; Chen, Z.; Zhang, S.; Liang, Q.; Jiang, E.; Wang, R. Research on the Tightening Order of Multiple bolts for Cylindrical Parts. Mech. Res. Appl. 2016, 29, 20–21+25. [Google Scholar]
- Chen, S. Research on the Influence of Manufacturing Error of Mounting Edge of Bolts-Stop Connection on the Assembly Performance; Dalian University of Technology: Dalian, China, 2021. [Google Scholar]
- ANSI/ASME PCC-1-2019; Guidelines for Pressure Boundary Bolted Flange Joint Assembly. ASME: New York, NY, USA, 2019.
- Tsuji, H.; Nakano, M. Bolt Preload Control for Bolted Flange Joint. In Analysis of Bolted Joints; Vancouver, B.C., Ed.; ASMEDC: Toronto, ON, Canada, 2002; pp. 163–170. [Google Scholar]
- Fukuoka, T.; Takaki, T. Finite Element Simulation of Bolt-Up Process of Pipe Flange Connections with Spiral Wound Gasket. J. Press. Vessel Technol. 2003, 125, 371–378. [Google Scholar] [CrossRef]
- Kumakura, S.; Saito, K. Tightening Sequence for Bolted Flange Joint Assembly. In Analysis of Bolted Joints; ASMEDC: Cleveland, OH, USA, 2003; pp. 9–16. [Google Scholar]
- Zou, Q.; Sun, T.; Nassar, S.; Barber, G.; Gumul, A. Effect of Lubrication on Friction and Torque-Tension Relationship in Threaded Fasteners. Tribol. Trans. 2007, 50, 127–136. [Google Scholar] [CrossRef]
- Lian, J.; Qian, Y.; Dong, L. Study on the influence of assembly technology on the preload of shaft system in super high speed test. Aerosp. Manuf. Technol. 2018, 2, 52–56. [Google Scholar]
- Wang, C.; Du, L.; Jin, W.; Hu, X.; Huang, B. Influence of thread lubrication on torque coefficient of thread pair and Diskussion on tightening technology. Intern. Combust. Engine Accessories 2018, 23, 50–52. [Google Scholar]
- Wang, W.; Xiao, Q.; Li, P.; Xu, X. Application of reverse engineering and finite element simulation in bolt assembly process research. Mach. Tools Hydraul. 2012, 40, 127–131+139. [Google Scholar]
- Ma, Y.; Wang, W.; Xu, H.; Xu, X.; Liu, X. The Research of Bolt-up Process Based on ANSYS. Modul. Mach. Tool Autom. Mach. Technol. 2013, 6, 106–109. [Google Scholar]
- Zhu, L.; Bouzid, A.; Hong, J. Numerical and Experimental Study of Elastic Interaction in Bolted Flange Joints. J. Press. Vessel Technol. 2017, 139, 021211. [Google Scholar] [CrossRef]
- Huang, W. Study on Pre Preload for Connecting Bolts of Aeroengine Low Pressure Rotor; Dalian University of Technology: Dalian, China, 2018. [Google Scholar]
- Duffey, T. Optimal bolt preload for dynamic loading. Int. J. Mech. Sci. 1993, 35, 257–265. [Google Scholar] [CrossRef]
- Piluso, V.; Faella, C. Experimental analysis of bolted connections: Snug versus. J. Struct. Eng. 1998, 124, 765–774. [Google Scholar]
- Hu, D. Research on Modeling Technique and Simulation for 3D Solid of Parametric Thread; Dalian Jiaotong University: Dalian, China, 2005. [Google Scholar]
- Li, G.; Wang, H.; Xiong, Z. Assembly accuracy study of large optical units in high power laser system. J. Mech. Eng. 2015, 51, 116–122. [Google Scholar] [CrossRef]
- Li, X.; Meng, Q.; Du, Y.; Chen, F.; Wang, H.; Zhao, B. Influence of Tightening Strategy on Pre-preload of Aero-engine Singe-Bolt Connection. J. Mech. Eng. 2020, 56, 231–241. [Google Scholar]
- Li, X.; Han, Y.; Chen, F.; Wang, H.; Zhao, B. Fluctuation test of tightening torque coefficient of aeroengine bolts. J. Aeronaut. Dyn. 2022, 1–13. [Google Scholar] [CrossRef]
- Zhao, B.; Zhang, S.; Wang, H. Analyses of preload variations in aeroengine rotor bolted connections. J. Tsinghua Univ. 2020, 61, 1144–1151. [Google Scholar]
- Zhao, B.; Zhang, S.; Wang, H.; Wu, D.; Qiao, T.; Huang, X. Evaluation and analysis of the preload of the connecting bolts of the ninth stage plate assembly. China Mech. Eng. 2020, 31, 1570–1576. [Google Scholar]
- Bickford, J. Introduction to the Design and Behavior of Bolted Joints 4/e; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Defeo, J.; Juran, J. Juran’s Quality Handbook: The Complete Guide to Performance Excellence 6/e; McGraw Hill Professional: New York, NY, USA, 2010. [Google Scholar]
- Persson, E.; Roloff, A. Ultrasonic tightening control of a screw joint: A comparison of the clamp force accuracy from different tightening methods. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2015, 230, 1989–1996. [Google Scholar] [CrossRef]
- Toth, G. Torque and angle controlled tightening over the yield point of a screw-based on Monte-Carlo simulations. J. Mech. Des. 2004, 126, 729–736. [Google Scholar] [CrossRef]
- Chu, T. Bolt Tightening Methods and Preload Control. Chem. Equip. Pipeline 2005, 42, 40–42. [Google Scholar]
- Sun, G.; Zhao, Z.; Yang, F.; Li, H. Application Experiment of “Torque–Angle” Method for Engine Disk and Shaft Connection. Aeronaut. Manuf. Technol. 2019, 62, 76–79+93. [Google Scholar]
- Fukuoka. Evaluation of the tightening process of elastic angle control method and proposal of a practical tightening operation. Nippon Kikai Gakkai Ronbunshu C Hen Trans. Jpn. Soc. Mech. Eng. Part C 2006, 72, 1370–1377. [Google Scholar]
- Dubois, G.; Piraprez, E. High strength friction grip bolts optimal parameters for the combined method of tightening. J. Constr. Steel Res. 1994, 28, 1–22. [Google Scholar] [CrossRef]
- Hashimura, S.; Komatsu, K.; Inoue, C.; Nakao, T. A New Tightening Method of Bolt/Nut Assembly to Control the Clamping Force. J. Adv. Mech. Des. Syst. Manuf. 2008, 2, 896–902. [Google Scholar] [CrossRef]
- Nassar, S.; Veeram, A. Ultrasonic control of fastener tightening using varying wave speed. J. Press. Vessel Technol. -Trans. ASME 2006, 128, 427–432. [Google Scholar] [CrossRef]
- Tang, X. Research on Multi-Sensor Bolt Pre Preload Detection Method; Zhejiang University of Technology: HangZhou, China, 2009. [Google Scholar]
- Mizutani, Y.; Onishi, T.; Mayuzumi, M. Plastic-region tightening of bolts controlled by acoustic emission method. Proc. ICAE 2007, 6, 120–125. [Google Scholar]
- Huang, Y.; Liu, L.; Yeung, T.; Hung, Y. Real-time monitoring of clamping force of a bolted joint by use of automatic digital image correlation. Opt. Laser Technol. 2009, 41, 408–414. [Google Scholar] [CrossRef]
- Fukuoka, T.; Xu, Q. Finite element simulation of the tightening process of bolted joint with a bolt heater. J. Press. Vessel. Technol. -Trans. ASME 2002, 124, 457–464. [Google Scholar] [CrossRef]
- Grzejda, R.; Parus, A. Experimental studies of the process of tightening an asymmetric multi-bolted connection. IEEE Access 2021, 9, 47372–47379. [Google Scholar] [CrossRef]
- BiliBili Bullet Screen Video Network. Rolls-RoyceSuper Efficient Trent XWB Assembly Process of Series Turbofan Engine. 2018. Available online: https://www.bilibili.com/video/BV16s411J7ux/ (accessed on 27 March 2022).
- BiliBili Bullet Screen Video Network. The Whole Process of ej200 Engine Assembly. 2018. Available online: https://www.bilibili.com/video/BV1Wt411y7MF/ (accessed on 27 March 2022).
- Sun, G.; Wang, W.; Yang, F.; Zhao, Z.; Li, H. Analysis of low pressure turbine balancing technology for high bypass ratio turbofan engine. Aviat. Maint. Eng. 2019, 1, 42–45. [Google Scholar]
- MARPOSS. Aerospace Industry-MRO and Frock-Marposs. 2014. Available online: https://www.marposs.com/chi/application/aerospace-industry-mro-and-tooling (accessed on 28 March 2022).
- Li, L.; Zhang, Y.; Zhou, S.; Wang, X.; Chen, J.; Xiang, H.; Chen, D.; Wang, P. A Nut Placing and Tightening Device, Method and System. CN105522537B, 17 May 2017. [Google Scholar]
- Chen, D.; Wang, J.; Lin, L.; Zhang, Y.; Zhang, X.; Ouyang, K.; Ren, J. Nut Tightening Device. CN108237393B, 27 August 2019. [Google Scholar]
- Sun, G.; Wang, T.; Lv, Y. Advanced assembly technology and equipment of turbofan engine. Aviat. Manuf. Technol. 2017, 22, 72–77. [Google Scholar]
- Ge Aviation. Leap Engines–CFM International Jet Engines. 2017. Available online: https://www.cfmaeroengines.com/engines/leap/ (accessed on 28 March 2022).
- Gao, H.; Zhou, T. The Invention Relates to a Tightening Device and Method for a Rear Nut of an Aero-Engine High-Pressure Rotor Sealing Disk. CN108000127B, 17 May 2019. [Google Scholar]
- Gao, H.; Zhou, T. A Tightening Device and Method for Front Nut of Sealing Disk of High-Pressure Rotor of Aeroengine. CN108080939B, 29 October 2019. [Google Scholar]
- Sun, W.; Wang, L.; Yang, Y.; Yuan, B.; Sun, Q. The Invention Relates to an Aircraft Engine Connecting Rod Mechanism Alignment Nut Tightening Device and Method. CN110561097B, 11 December 2020. [Google Scholar]
- Sun, Q.; Yang, Y.; Yuan, B.; Ding, J.; Sun, W. The Invention Relates to an Internal Nut Flexible Shaft Transmission Tightening Device and Method for an Aircraft Engine. CN110561098A, 13 December 2019. [Google Scholar]
- Sun, Q.; Yuan, B.; Yang, Y.; Liu, C.; Sun, W. The Invention Relates to a Universal Joint Transmission Tightening Device and Method for an Internal Nut of an Aeroengine. CN110497190A, 26 November 2019. [Google Scholar]
- Lian, Y.; Wang, C.; Chen, Q.; Pan, Z.; Zhou, S. Tightening Device and Tightening Method for Rear Axle Nut of High Pressure Turbine of Aeroengine. CN110640446B, 17 August 2021. [Google Scholar]
- Wang, J.; Chen, D.; Tang, F.; Guo, L.; Zhou, X. A Tightening Device. CN113547319A, 26 October 2021. [Google Scholar]
- Ye, C.; Wang, H.; Yu, X.; Liu, Y.; Wang, R. A Fast Large Torque Tightening Device with a Narrow and Long Space Blind Cavity. CN212286614U, 5 January 2021. [Google Scholar]

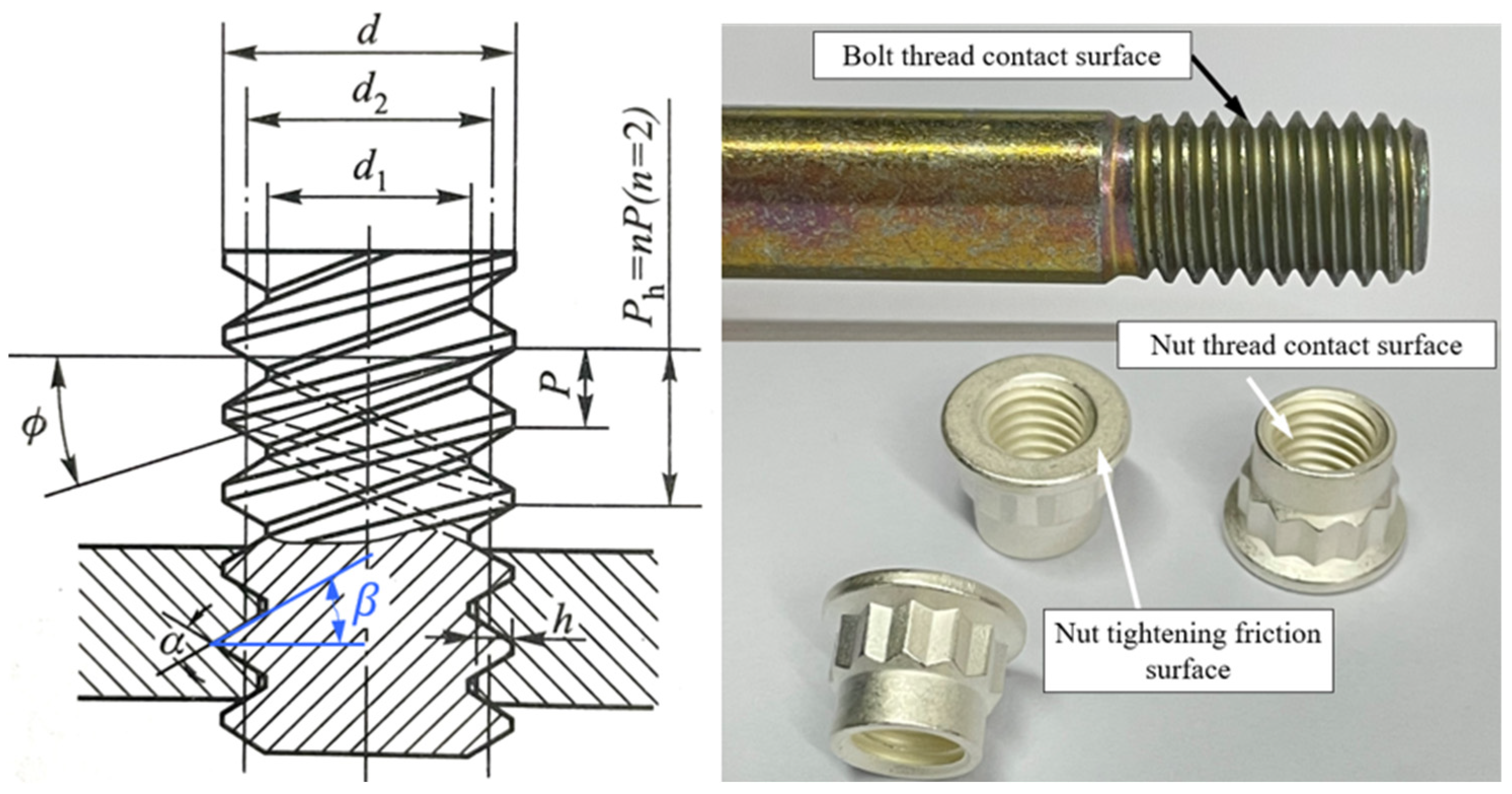
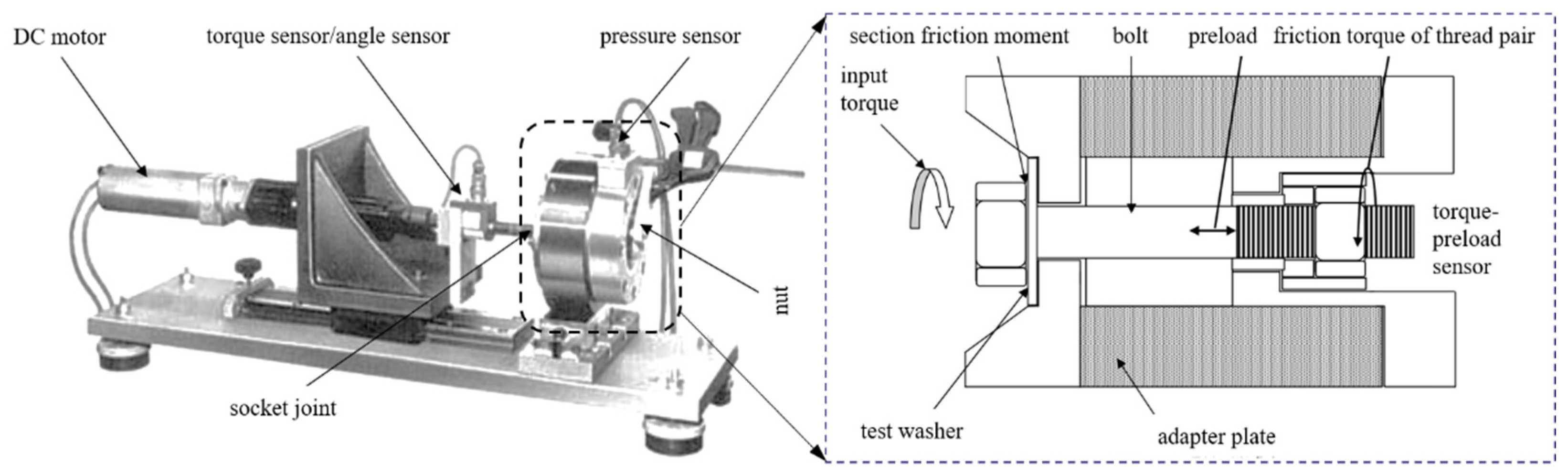
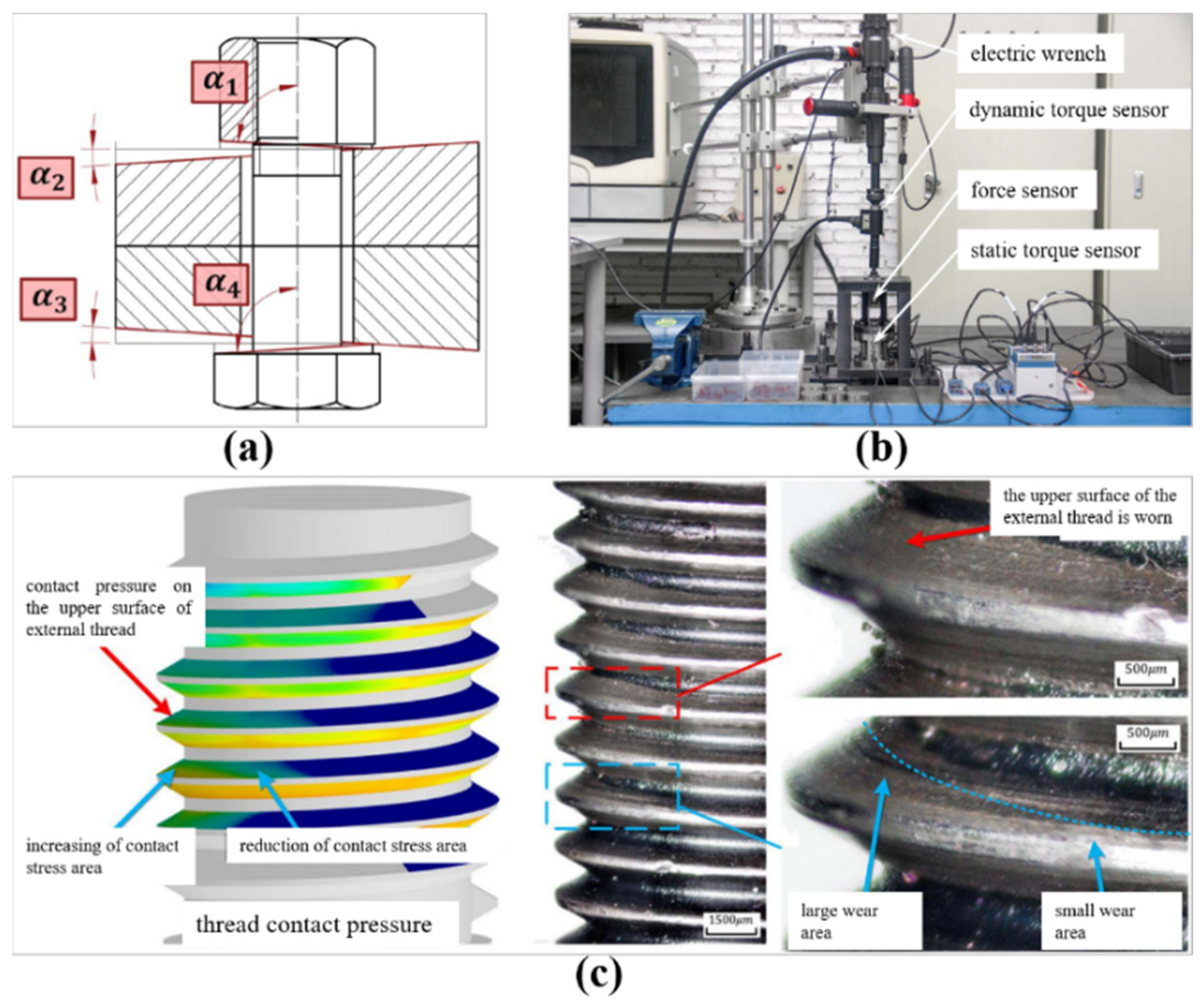









| Tightening Method | Principle Description | Advantages and Disadvantages | |
|---|---|---|---|
| Open-loop control | Torque method | Taking torque as the control quantity, preload is achieved by applying a certain torque value to the bolt or nut | The control and operation of this method are very simple, but it is greatly affected by friction conditions, resulting in large dispersion of preload and low utilization of bolt material |
| Torque angle method | Taking the rotation angle as the control quantity, the pre-tightening is achieved by applying a fixed rotation angle to the bolt or nut after the fitting torque is reached | This method reduces the influence of the friction coefficient on the preload, and the preload control precision is high, but the initial fitting torque and control angle are not easy to determine | |
| Semi-closed-loop control | Yield point method | Taking the curvature of the torque–angle relationship curve as the control quantity, the real-time control computer calculates the curvature until the material yield point is reached | The bolt preload dispersion is very small, and the bolt material utilization rate is high, but the fastening will exceed the material yield point, and the fastening equipment cost is high |
| Pre-stretching control method | Pre-extension of bolts required by design is achieved by hydraulic, electric, and heating methods, and then the nuts are tightened | This method can effectively improve the precision of preload control and the strength of the bolt connection, but the preloading device needs to be customized, and the cost is high | |
| Closed-loop control | Elongation detection method | Directly controls the elongation of bolts. During tightening, measures the elongation of bolts by means of ultrasonication, dial indicator, etc., until it reaches the set value | The dispersion of the bolt preload is very small, and the bolt material utilization rate is high, but the bolt end face needs to be processed, and the cost is high |
| Bolt loading method | Applies the tension load of the design clamping force value directly to the bolt, and then tightens the nut | This method can achieve direct control of axial tension, with high precision, but high cost | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Li, X.; Han, Y.; Zhang, P.; Zhang, Z.; Zhang, M.; Zhao, G. A Review of Aeroengines’ Bolt Preload Formation Mechanism and Control Technology. Aerospace 2023, 10, 307. https://doi.org/10.3390/aerospace10030307
Li Z, Li X, Han Y, Zhang P, Zhang Z, Zhang M, Zhao G. A Review of Aeroengines’ Bolt Preload Formation Mechanism and Control Technology. Aerospace. 2023; 10(3):307. https://doi.org/10.3390/aerospace10030307
Chicago/Turabian StyleLi, Zhaoyu, Xiaoqiang Li, Yujie Han, Pengfei Zhang, Zongjiang Zhang, Mingming Zhang, and Gang Zhao. 2023. "A Review of Aeroengines’ Bolt Preload Formation Mechanism and Control Technology" Aerospace 10, no. 3: 307. https://doi.org/10.3390/aerospace10030307
APA StyleLi, Z., Li, X., Han, Y., Zhang, P., Zhang, Z., Zhang, M., & Zhao, G. (2023). A Review of Aeroengines’ Bolt Preload Formation Mechanism and Control Technology. Aerospace, 10(3), 307. https://doi.org/10.3390/aerospace10030307







