Optimizing the Location of the Piezoelectric Actuator and Analyzing Its Effect on the Dynamics of Asymmetric Flexible Spacecraft
Abstract
:1. Introduction
- Considering the self-physical properties and unilaterally installed on the flexible appendages of the actuator, the ODE–PDEs coupling dynamics model is derived for the rigid–flexible electromechanical coupled asymmetric flexible spacecraft.
- We use GA to optimize the installation position of the PZT actuators on the cantilever beam with end loads. The optimization covers the entire continuous interval of the beam length.
- We analyze how the installation position of the actuator and its own physical characteristics affect the asymmetric characteristics of the system. We also study how optimizing the actuator position can improve the control performance of the distributed controller.
2. Dynamics of Asymmetric Flexible Spacecraft with Integrated PZT Actuator
2.1. Actuator Model
2.2. Dynamics of Asymmetric Flexible Spacecraft
3. Optimal Placement of the PZT Actuators
3.1. Design of Optimization Criteria
3.2. Solution Approaches
4. Numerical Simulations
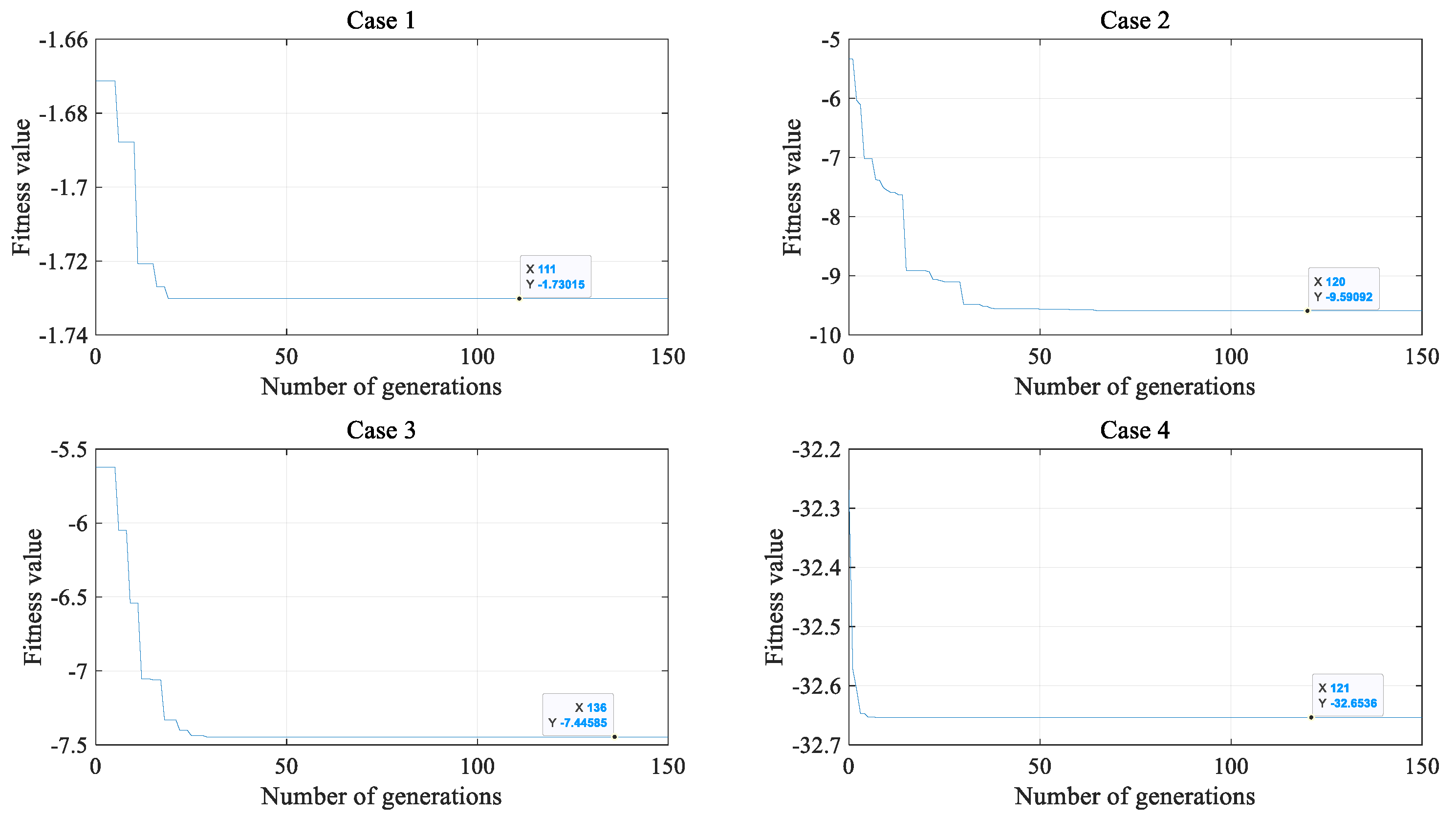
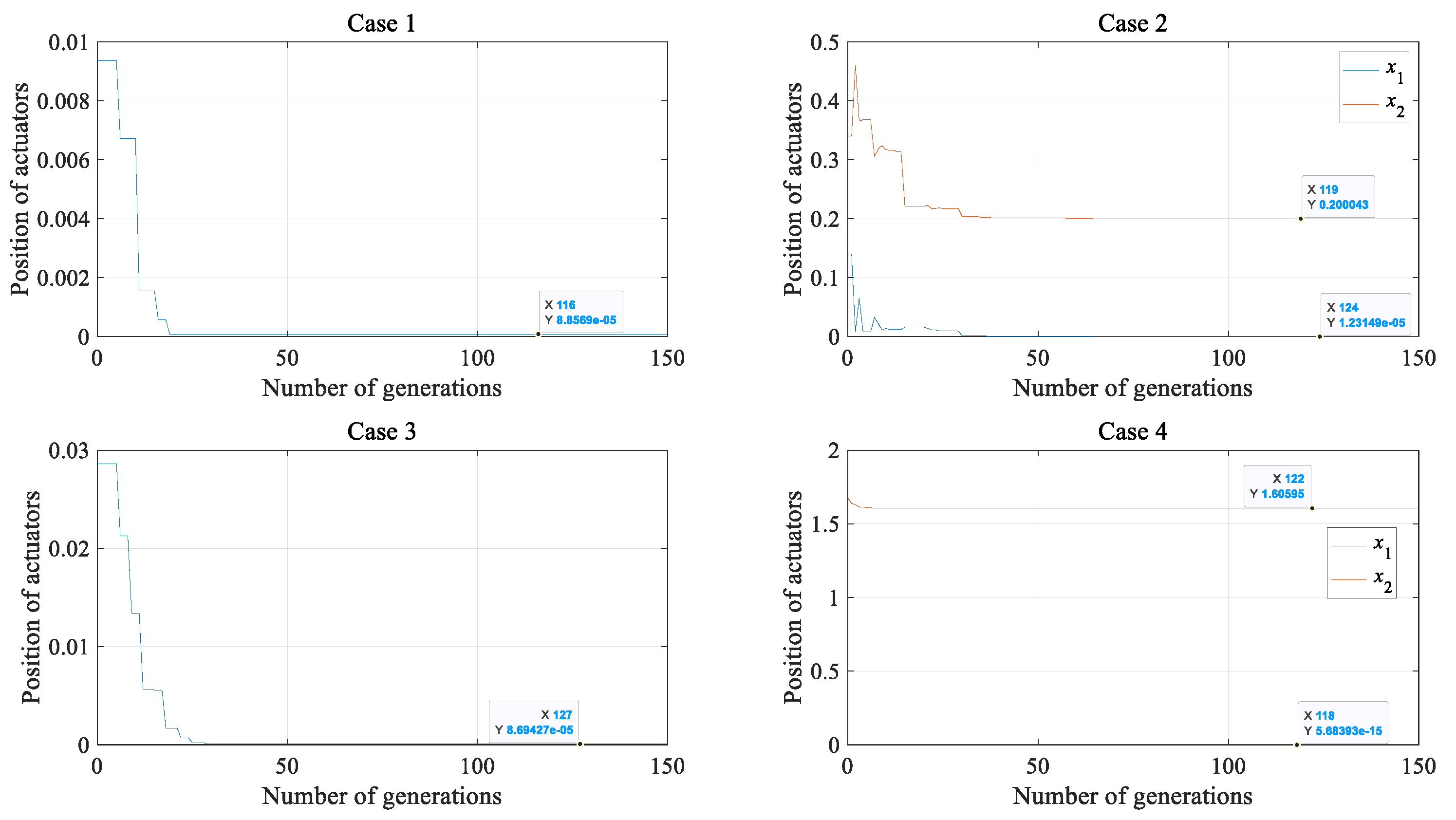
4.1. Actuator Position Optimization
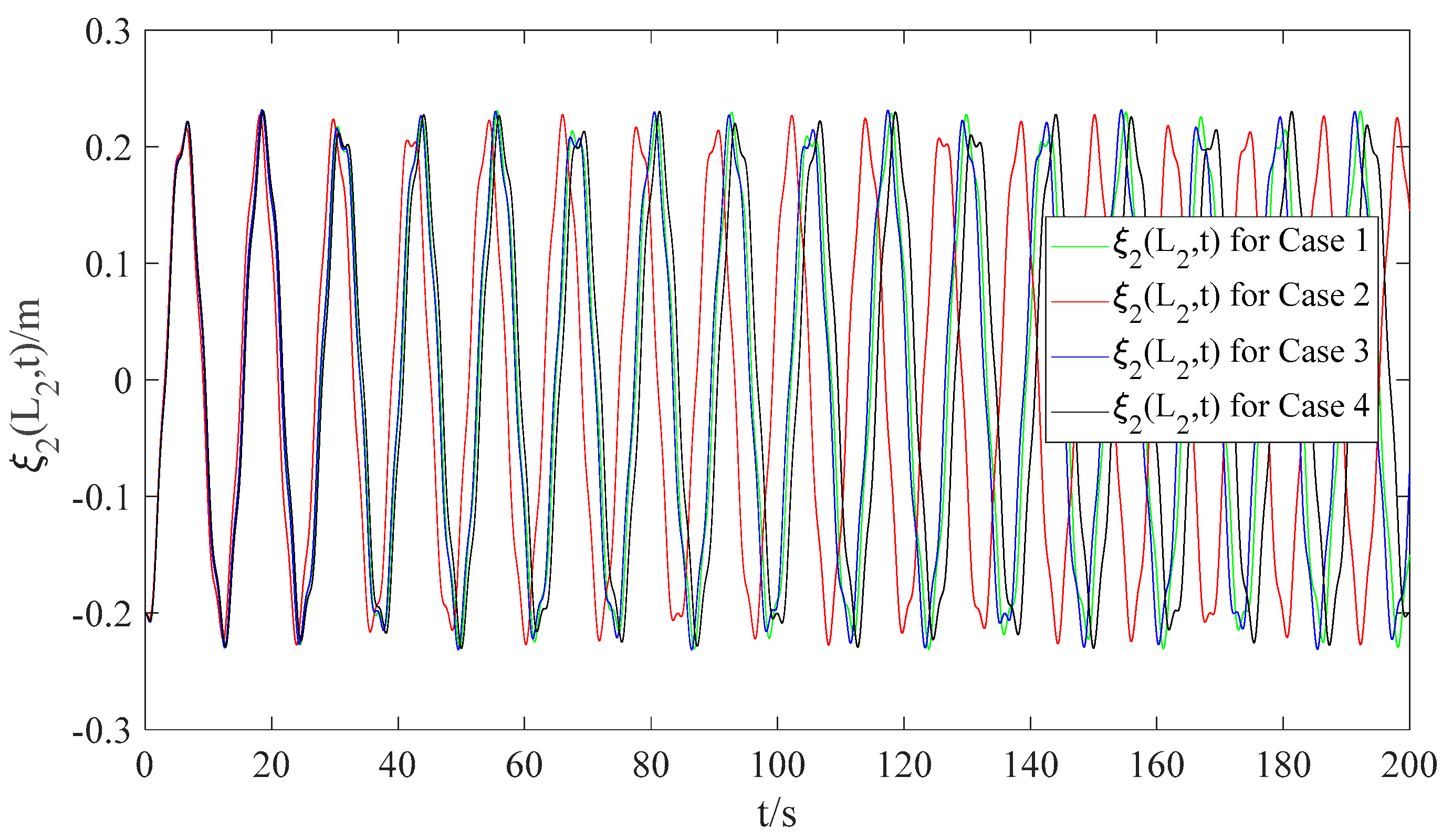
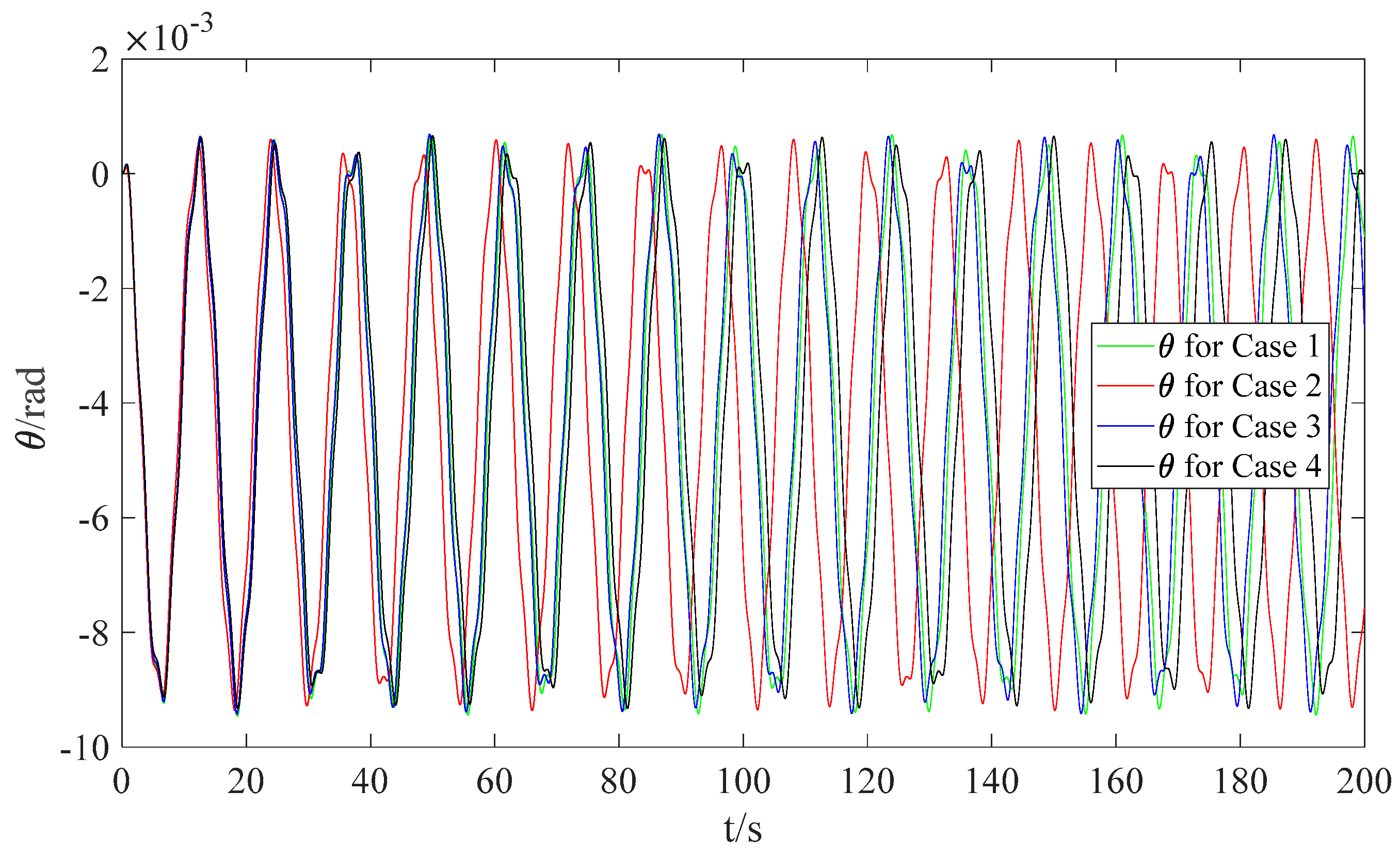
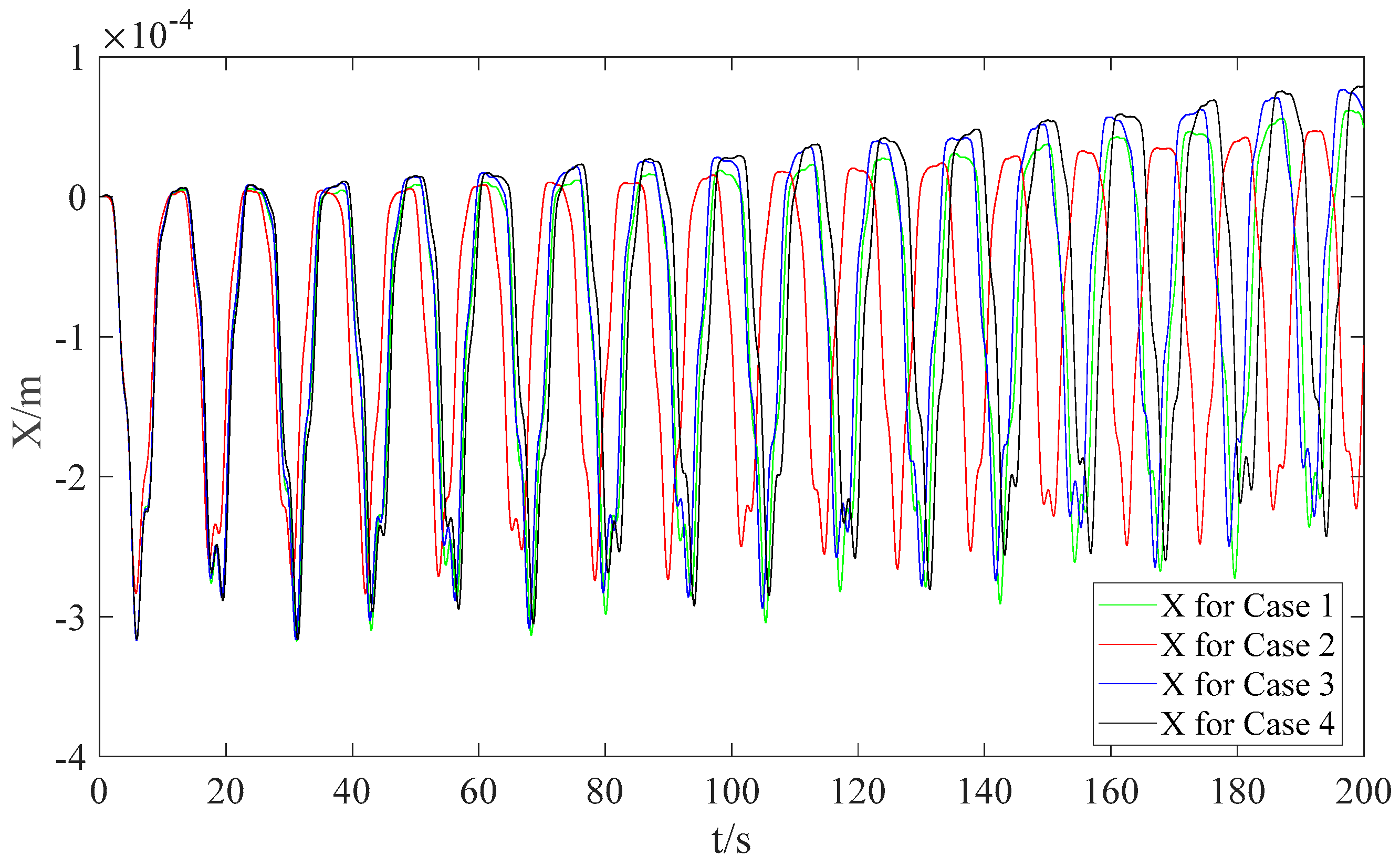
4.2. Influence of Actuator Position on Dynamic Characteristics
- Beam 1: This beam has no actuator attached, and its length is .
- Beams 2–4: These beams also have a length of and the actuators are installed at positions , , and , respectively.
- Beam 5: Similar to Beam 1, this beam has no actuator, but its length is changed to .
- Beams 6–8: These beams have a length of and the actuators are installed at positions , , and , respectively.
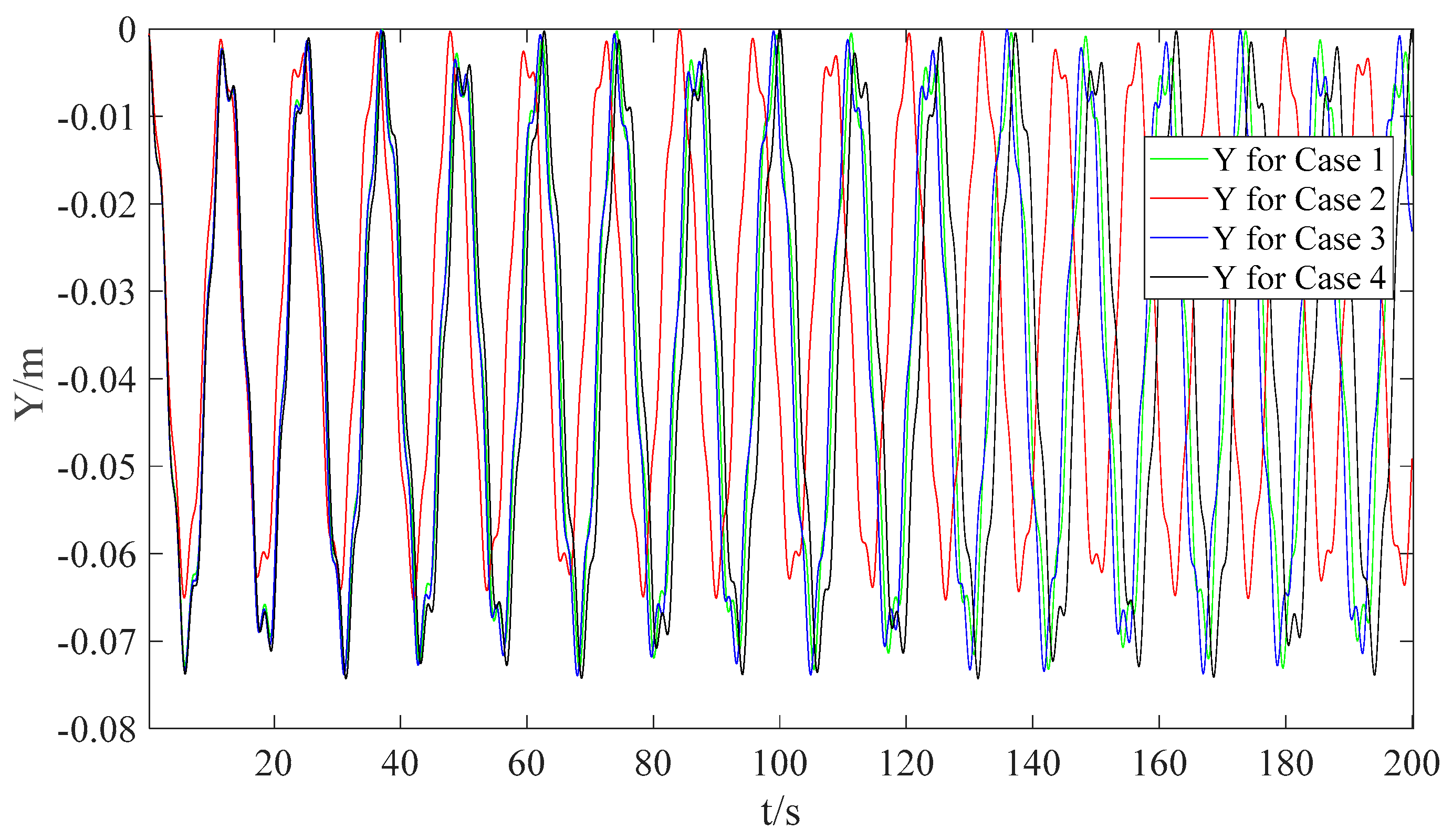
- The flexible appendages on both sides of the central hub are symmetrically configured with a length of , and there is no actuator attached.
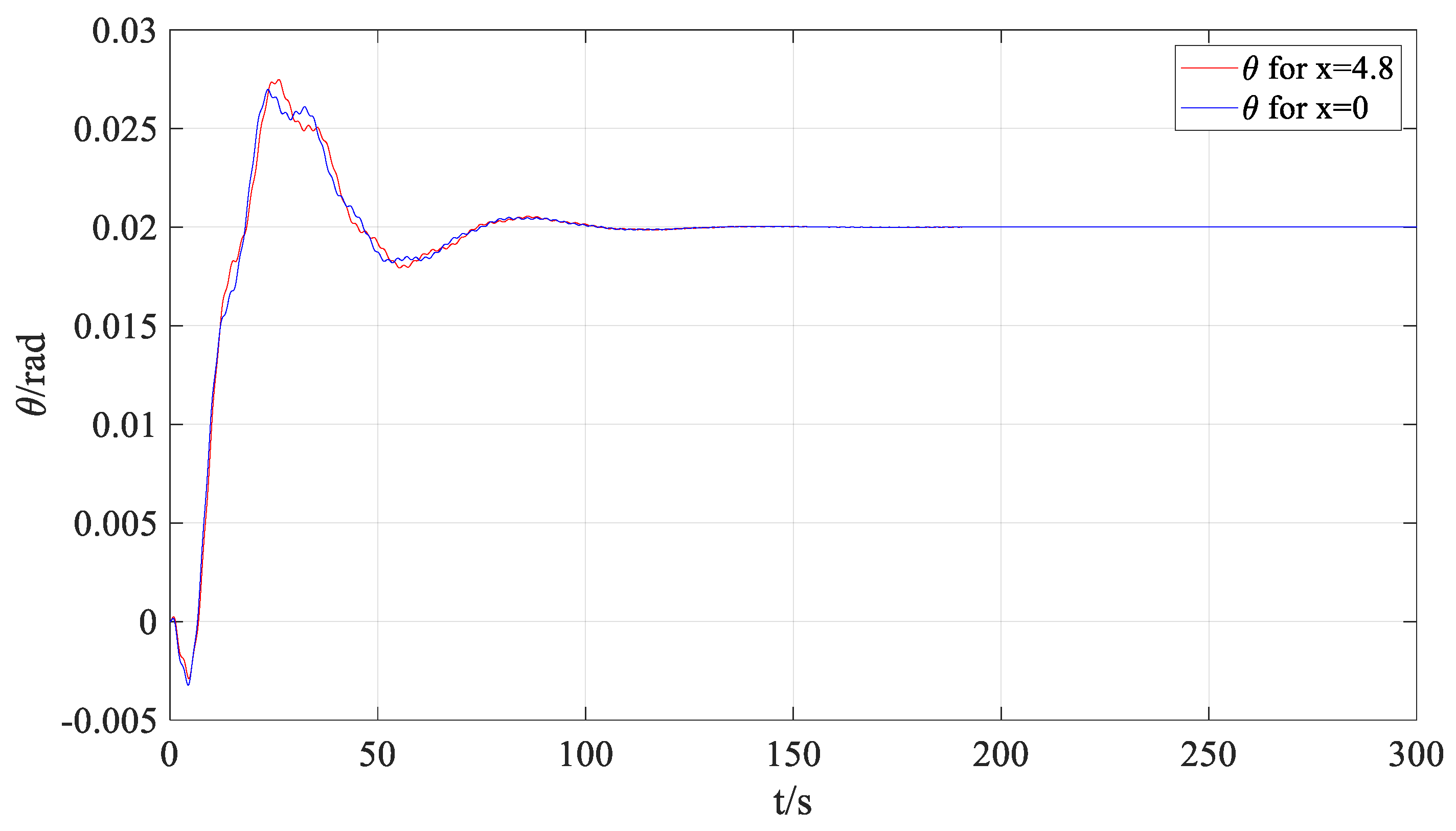
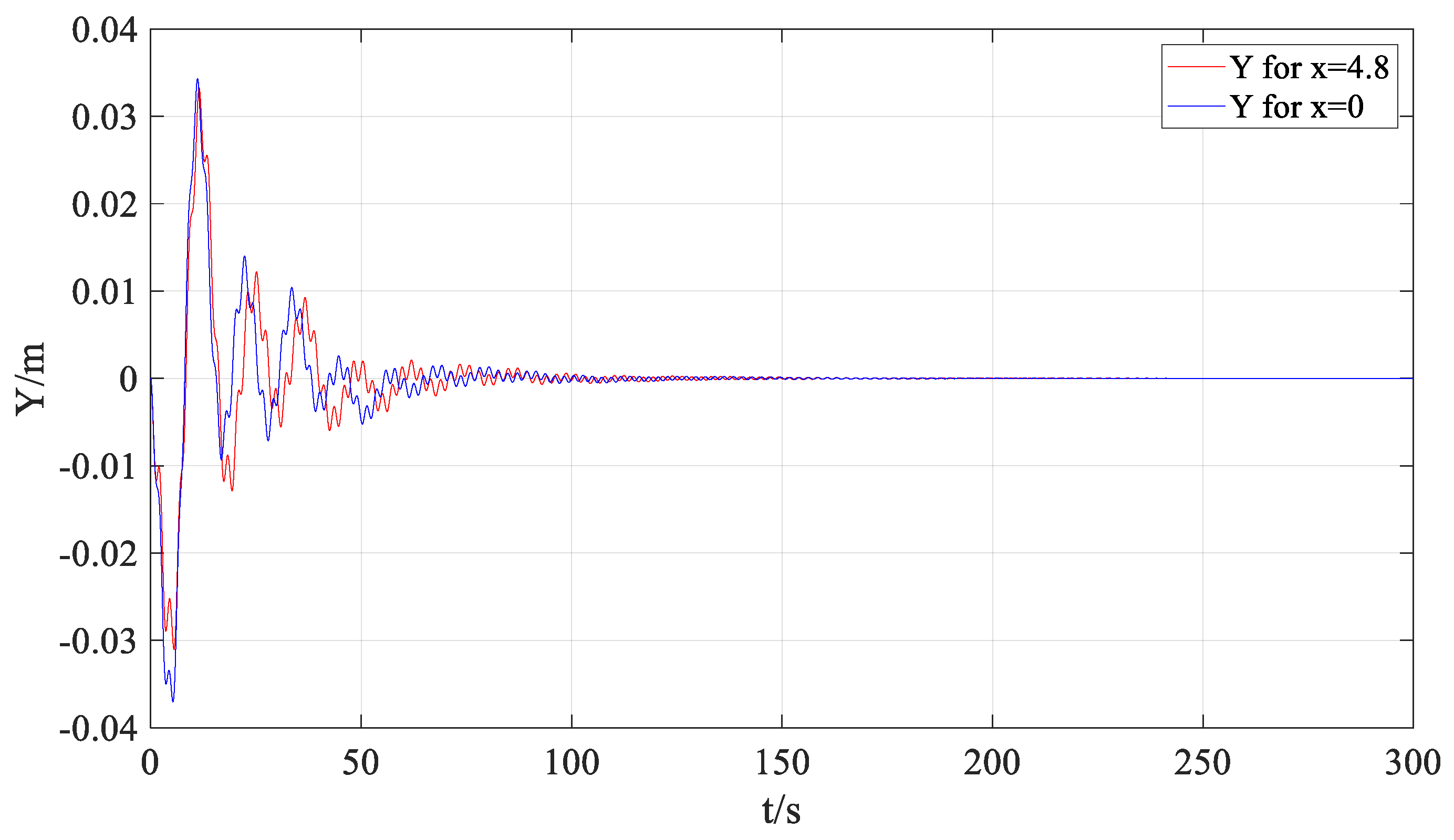
- The flexible appendages on both sides of the central hub are symmetrically configured with a length of , and the actuators are installed at the root x = 0 m.
- The flexible appendages on both sides of the central hub are symmetrically configured with a length of , and the actuators are installed at the middle point x = 2.4 m.
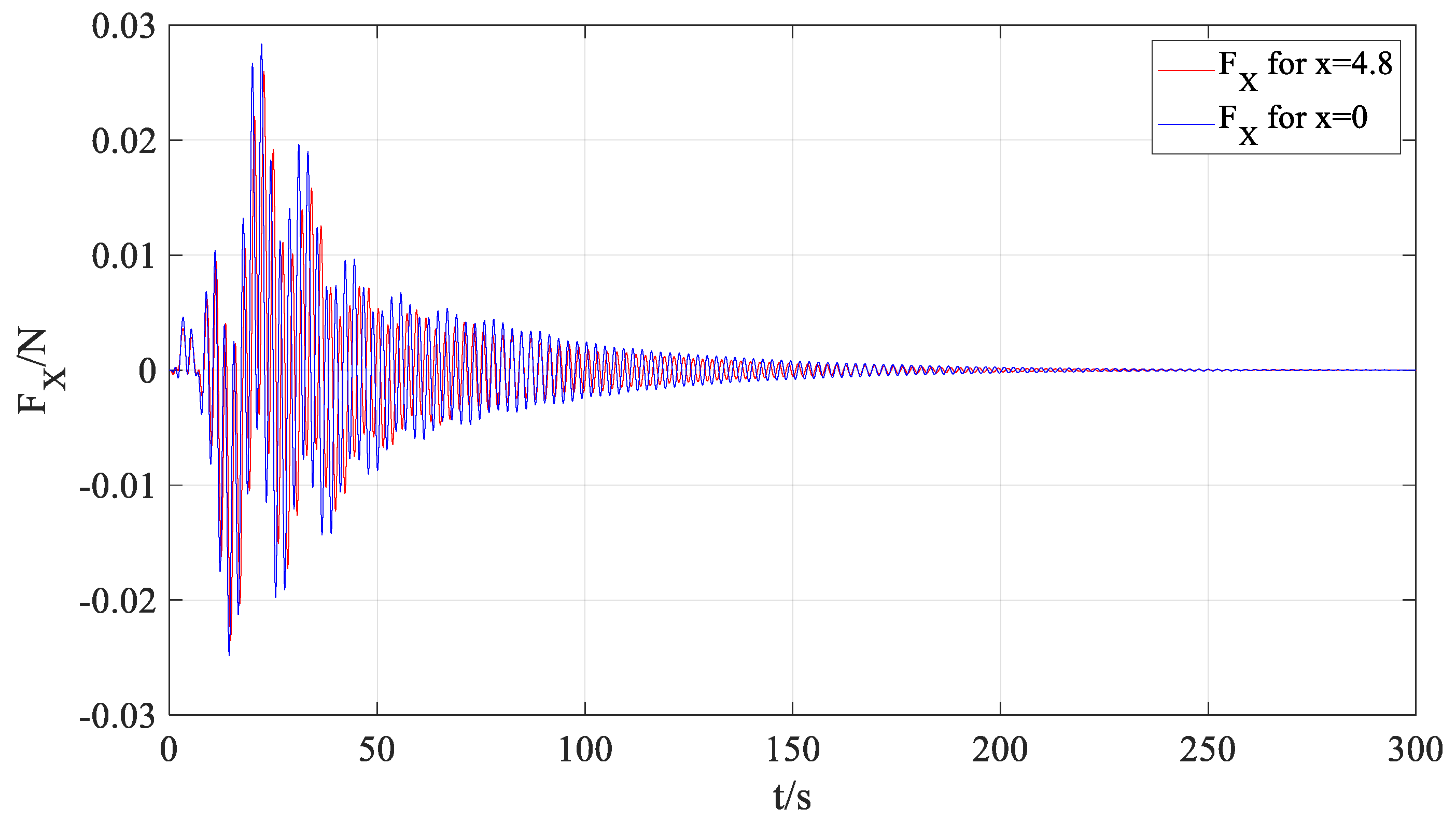
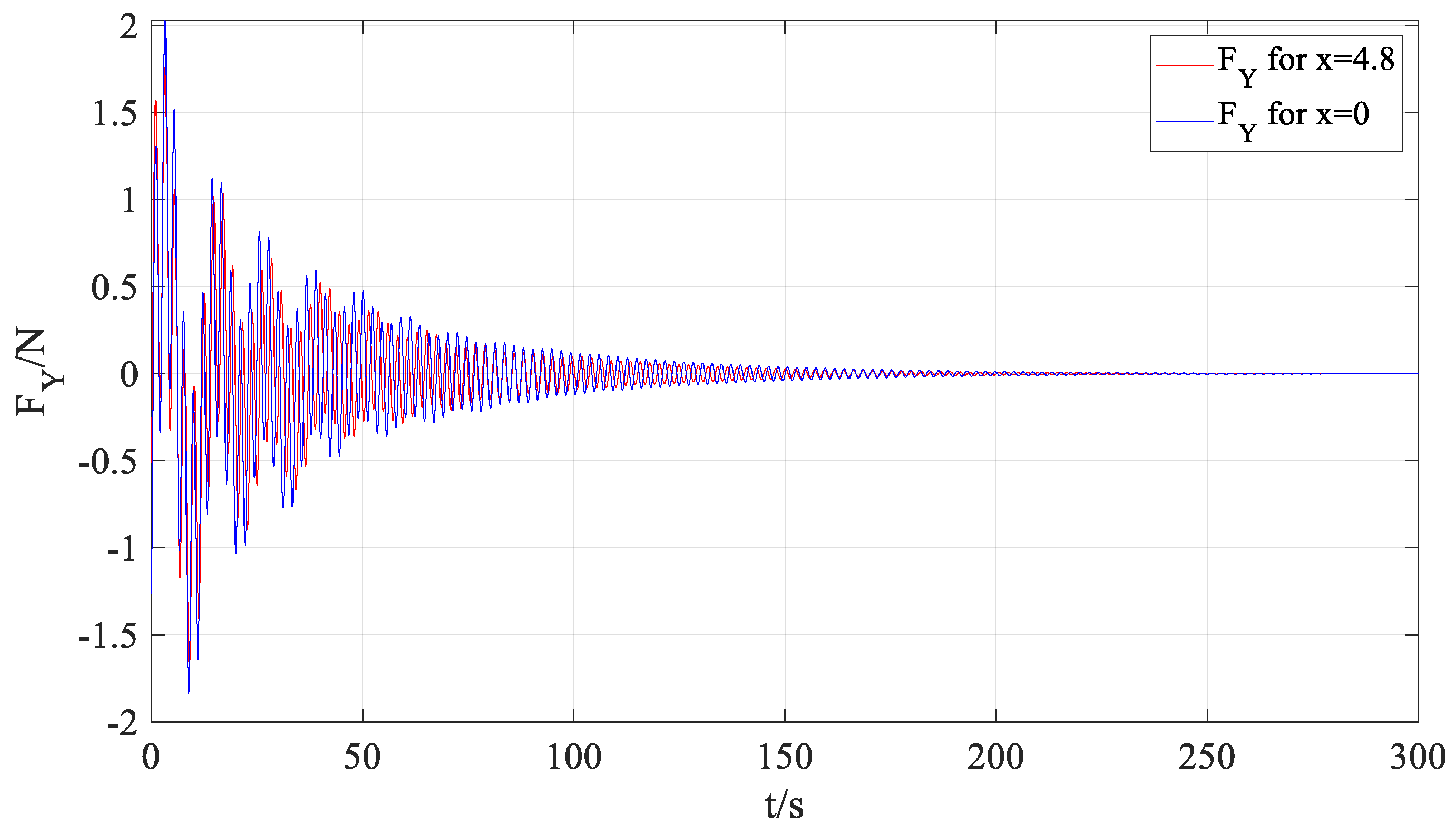
- The flexible appendages on both sides of the central hub are symmetrically configured with a length of , and the actuators are installed at the end point x = 4.8 m.
- 5.
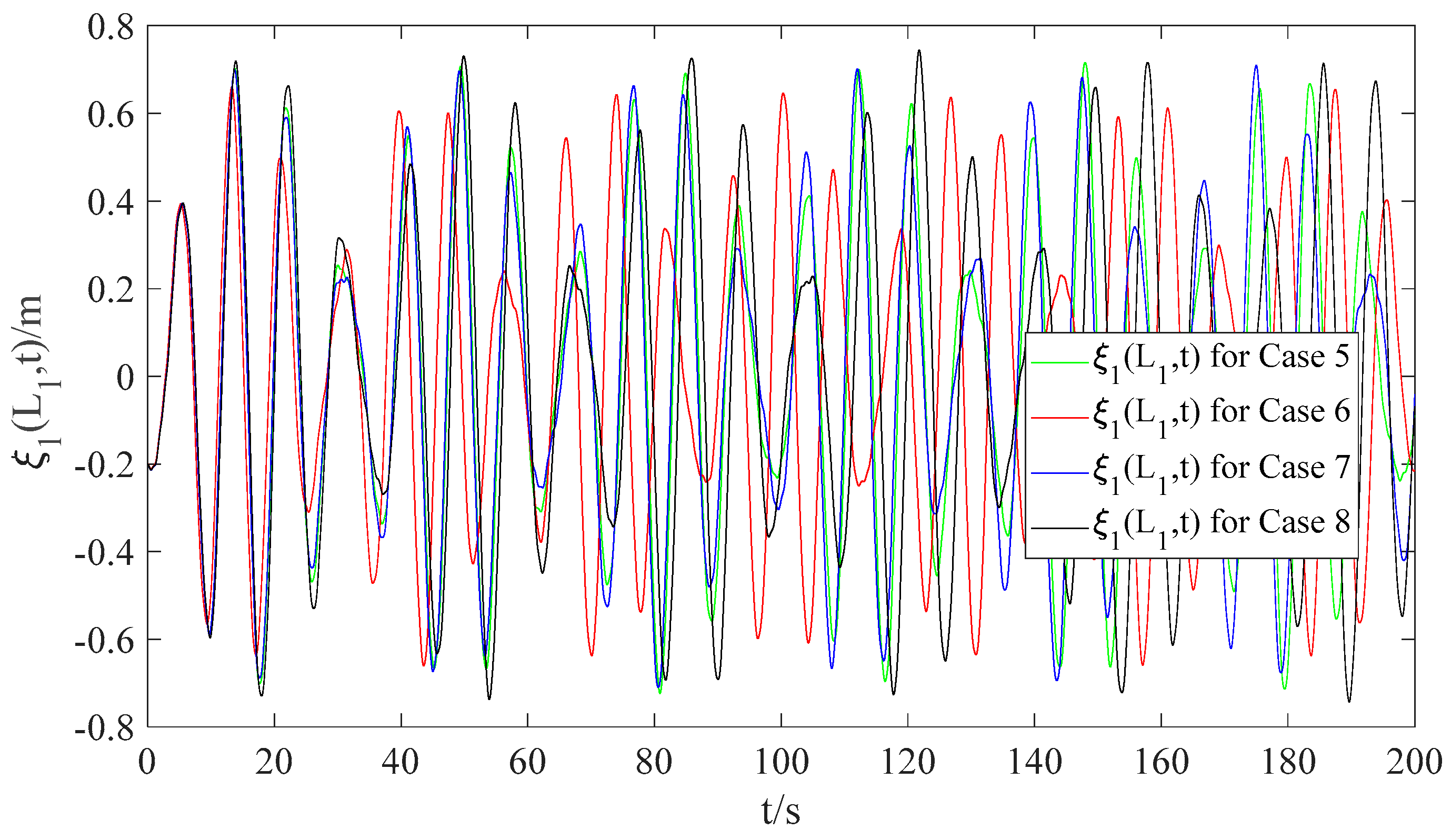
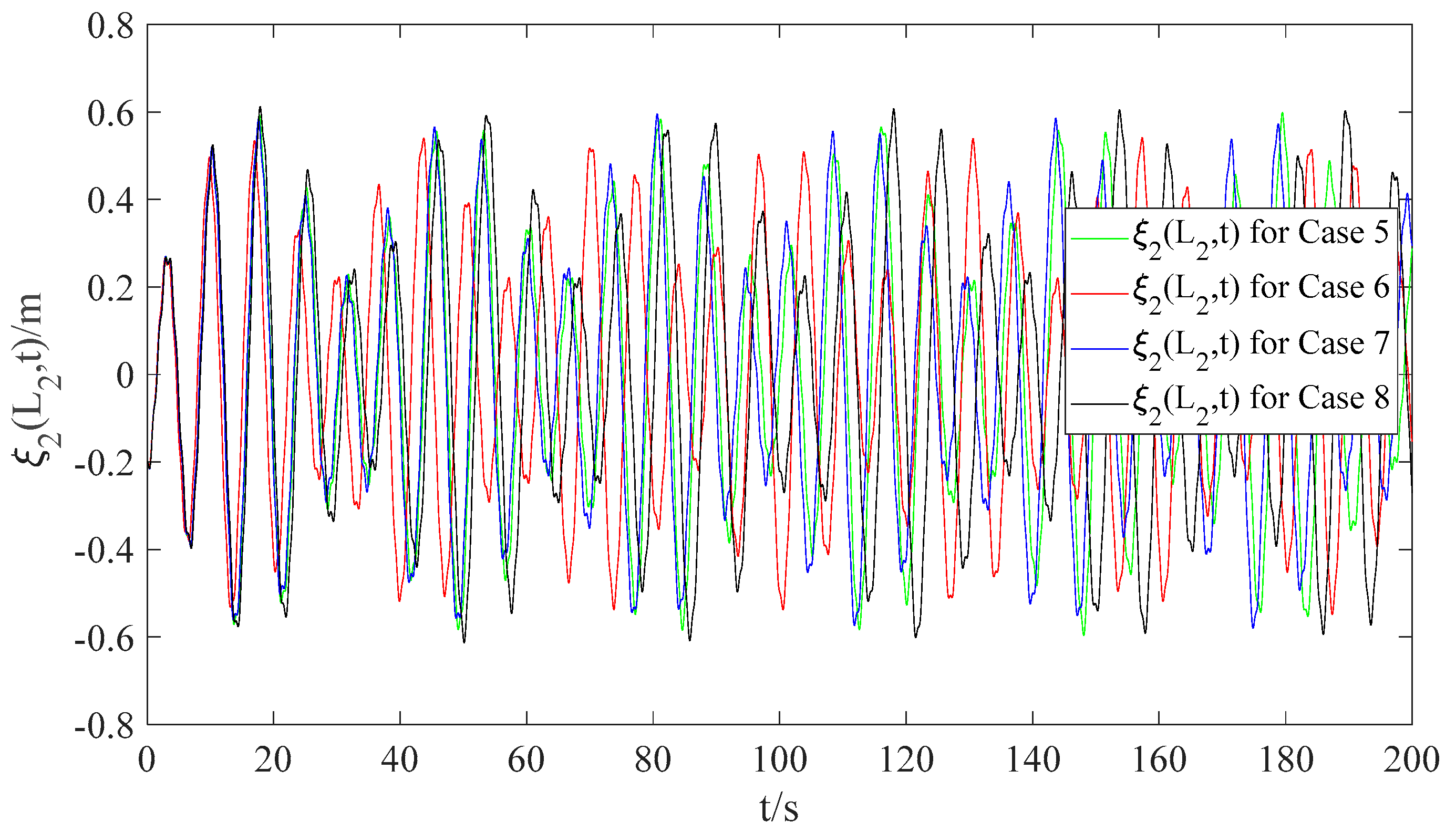
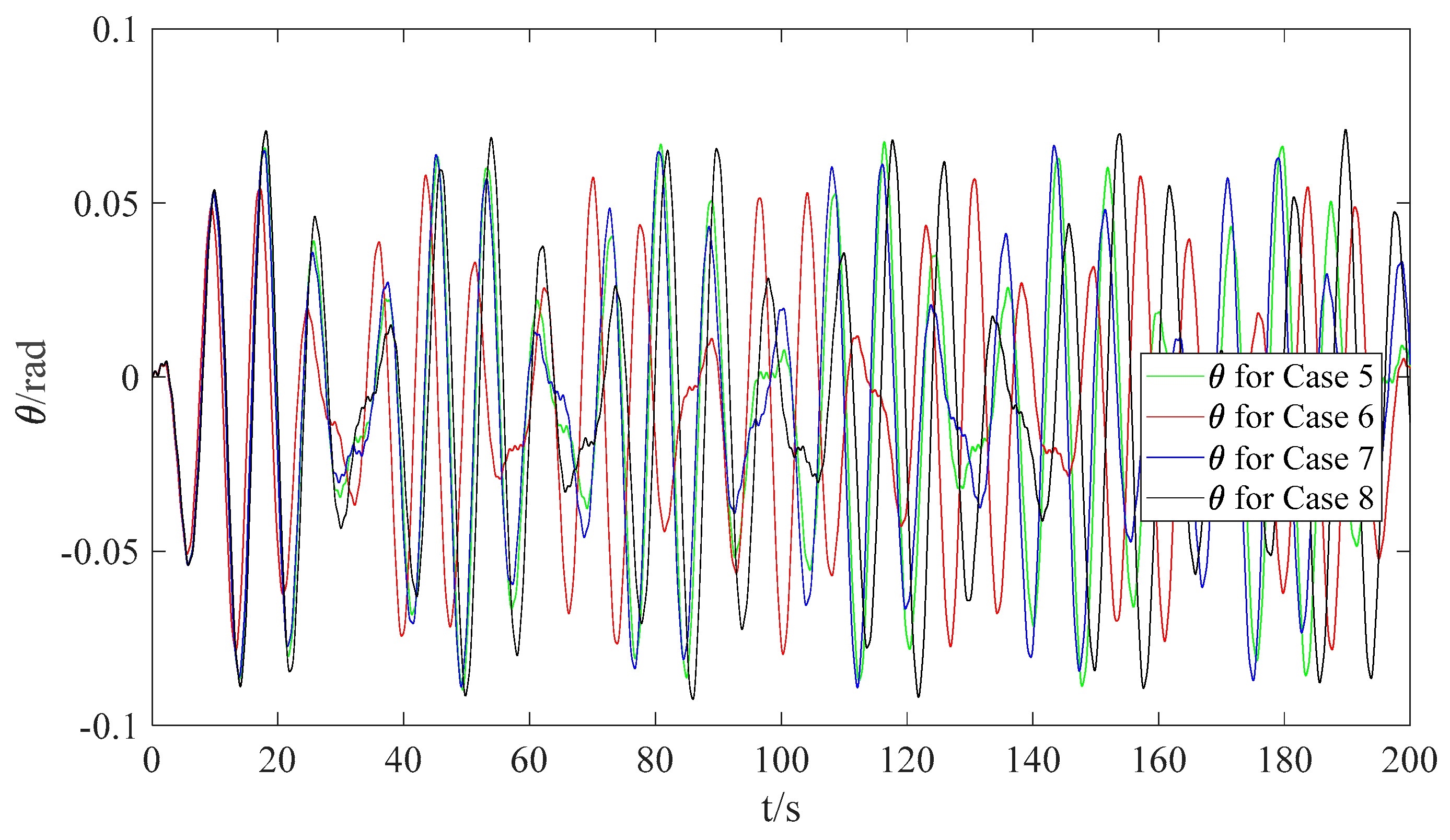
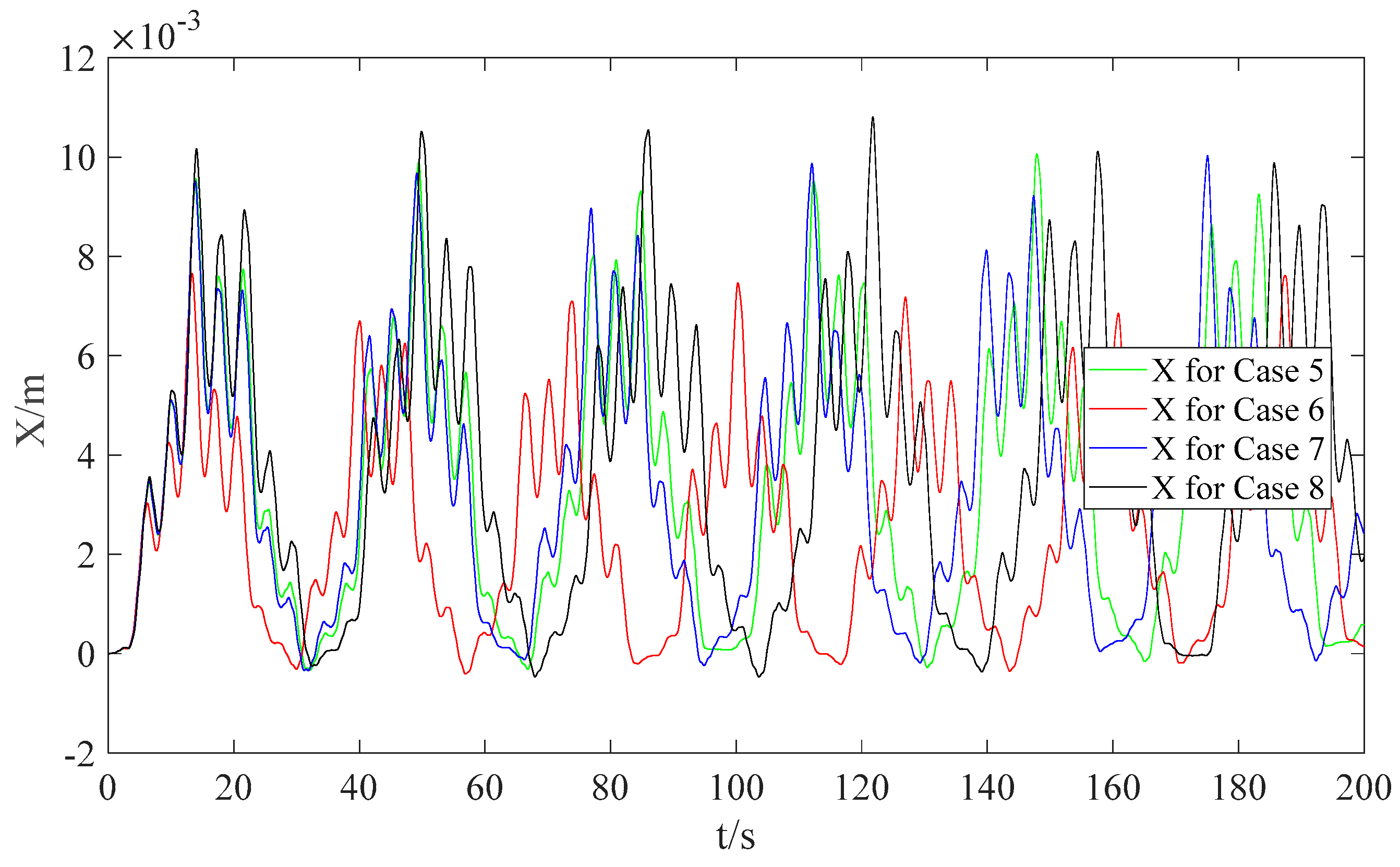
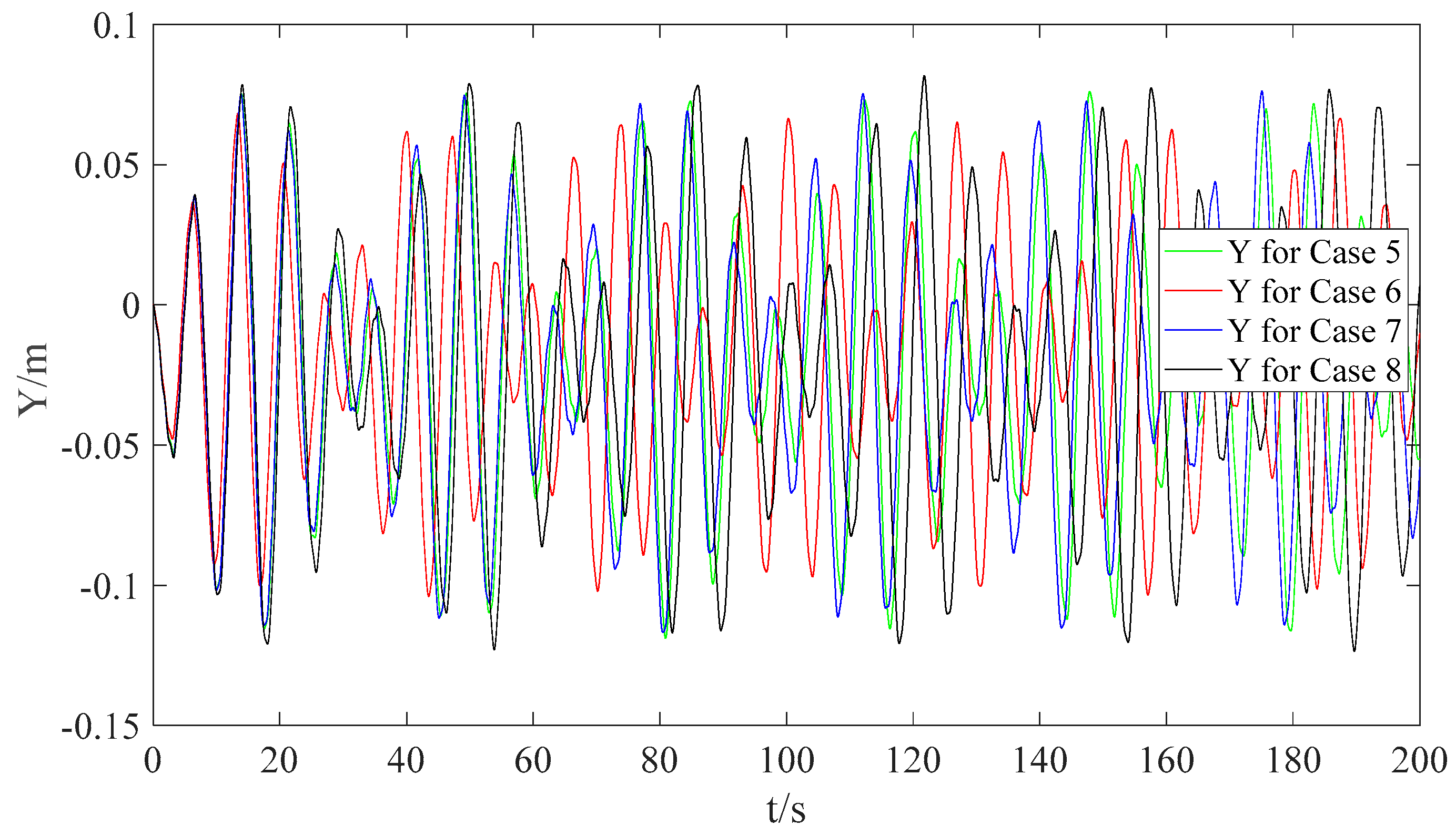
- The flexible appendages on both sides of the central hub are asymmetrically configured with lengths of and , respectively, with no actuator attached.
- 6.
- The flexible appendages on both sides of the central hub are asymmetrically configured with lengths of and , respectively, with the actuators installed at the root x = 0 m.
- 7.
- The flexible appendages on both sides of the central hub are asymmetrically configured with lengths of and , respectively, and the actuators are installed at the middle points x = 2.4 m and x = 1.4 m, respectively.
- 8.
- The flexible appendages on both sides of the central hub are asymmetrically configured with lengths of and , respectively, with the actuators installed at the end points x = 4.8 m and x = 2.8 m, respectively.
4.3. Influence of Actuator Position on Control Performance
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- Beam 1: No actuator, .
- Beam 2: , .
- Beam 3: , .
- Beam 4: , .
- Beam 5: No actuator, .
- Beam 6: , .
- Beam 7: , .
- Beam 8: , .
References
- Zhang, Z.; Li, X.; Li, Y.; Hu, G.; Wang, X.; Zhang, G.; Tao, H. Modularity, reconfigurability, and autonomy for the future in spacecraft: A review. Chin. J. Aeronaut. 2023, 36, 282–315. [Google Scholar] [CrossRef]
- Li, D.; Zhong, L.; Zhu, W.; Xu, Z.; Tang, Q.; Zhan, W. A Survey of Space Robotic Technologies for On-Orbit Assembly. Space Sci. Technol. 2022, 2022, 9849170. [Google Scholar] [CrossRef]
- Li, Y.K.; Li, S.; Xin, M. Dynamic Modeling and Attitude Control of Large-Scale Flexible Parallel Multibody Spacecraft. J. Guid. Control Dyn. 2022, 45, 2304–2317. [Google Scholar] [CrossRef]
- Cao, K.; Li, S.; She, Y.C.; Biggs, J.D.; Liu, Y.F.; Bian, L.L. Dynamics and on-orbit assembly strategies for an orb-shaped solar array. Acta Astronaut. 2021, 178, 881–893. [Google Scholar] [CrossRef]
- Li, Y.K.; Li, D.Y.; Zhu, W.S.; Sun, J.; Zhang, X.L.; Li, S. Constrained Motion Planning of 7-DOF Space Manipulator via Deep Reinforcement Learning Combined with Artificial Potential Field. Aerospace 2022, 9, 163. [Google Scholar] [CrossRef]
- Astone, P.; Bassan, M.; Bonifazi, P.; Carelli, P.; Coccia, E.; Fafone, V.; Febo, L.; Frasca, S.; Marini, A.; Mauceli, E.; et al. The Rome Group Resonant-Mass Detectors Explorer and Nautilus. In Second Workshop Gravitational Wave Data Analysis; Atlantica Séguier Frontières: Biarritz, France, 1997; p. 21. [Google Scholar]
- Li, J.; Zhang, L.; Li, S.; Mao, Q.; Mao, Y. Active disturbance rejection control for piezoelectric smart structures: A review. Machines 2023, 11, 174. [Google Scholar] [CrossRef]
- da Fonseca, I.M.; Rade, D.A.; Goes, L.C.; de Paula Sales, T. Attitude and vibration control of a satellite containing flexible solar arrays by using reaction wheels, and piezoelectric transducers as sensors and actuators. Acta Astronaut. 2017, 139, 357–366. [Google Scholar] [CrossRef]
- Callipari, F.; Sabatini, M.; Angeletti, F.; Iannelli, P.; Gasbarri, P. Active vibration control of large space structures: Modelling and experimental testing of offset piezoelectric stack actuators. Acta Astronaut. 2022, 198, 733–745. [Google Scholar] [CrossRef]
- Qing, X.; Li, W.; Wang, Y.; Sun, H. Piezoelectric transducer-based structural health monitoring for aircraft applications. Sensors 2019, 19, 545. [Google Scholar] [CrossRef]
- Liu, X.; Cai, G.; Peng, F.; Zhang, H. Dynamic model and active vibration control of a membrane antenna structure. J. Vib. Control 2018, 24, 4282–4296. [Google Scholar] [CrossRef]
- Zorić, N.D.; Tomović, A.M.; Obradović, A.M.; Radulović, R.D.; Petrović, G.R. Active vibration control of smart composite plates using optimized self-tuning fuzzy logic controller with optimization of placement, sizing and orientation of PFRC actuators. J. Sound Vib. 2019, 456, 173–198. [Google Scholar] [CrossRef]
- Yang, C.; Zhang, X.; Huang, X.; Cheng, Z.; Zhang, X.; Hou, X. Optimal sensor placement for deployable antenna module health monitoring in SSPS using genetic algorithm. Acta Astronaut. 2017, 140, 213–224. [Google Scholar] [CrossRef]
- Angeletti, F.; Gasbarri, P.; Sabatini, M. Optimal design and robust analysis of a net of active devices for micro-vibration control of an on-orbit large space antenna. Acta Astronaut. 2019, 164, 241–253. [Google Scholar] [CrossRef]
- Goncalves, J.F.; De Leon, D.M.; Perondi, E.A. Topology optimization of embedded piezoelectric actuators considering control spillover effects. J. Sound Vib. 2017, 388, 20–41. [Google Scholar] [CrossRef]
- Rad, H.K.; Salarieh, H.; Alasty, A.; Vatankhah, R. Boundary control of anti-symmetric vibration of satellite with flexible appendages in planar motion with exponential stability. Acta Astronaut. 2018, 147, 219–230. [Google Scholar] [CrossRef]
- Ataei, M.M.; Salarieh, H.; Pishkenari, H.N.; Jalili, H. Boundary control design for vibration suppression and attitude control of flexible satellites with multi-section appendages. Acta Astronaut. 2020, 173, 22–30. [Google Scholar] [CrossRef]
- Chen, T.; Wen, H.; Wei, Z.T. Distributed attitude tracking for multiple flexible spacecraft described by partial differential equations. Acta Astronaut. 2019, 159, 637–645. [Google Scholar] [CrossRef]
- Li, W.P.; Huang, H. Integrated optimization of actuator placement and vibration control for piezoelectric adaptive trusses. J. Sound Vib. 2013, 332, 17–32. [Google Scholar] [CrossRef]
- Angeletti, F.; Iannelli, P.; Gasbarri, P.; Sabatini, M. End-to-end design of a robust attitude control and vibration suppression system for large space smart structures. Acta Astronaut. 2021, 187, 416–428. [Google Scholar] [CrossRef]
- Biglar, M.; Gromada, M.; Stachowicz, F.; Trzepiecinski, T. Optimal configuration of piezoelectric sensors and actuators for active vibration control of a plate using a genetic algorithm. Acta Mech. 2015, 226, 3451–3462. [Google Scholar] [CrossRef]
- Bruant, I.; Proslier, L. Optimal location of piezoelectric actuators for active vibration control of thin axially functionally graded beams. Int. J. Mech. Mater. Des. 2016, 12, 173–192. [Google Scholar] [CrossRef]
- Liu, X.; Cai, G.P.; Peng, F.J.; Zhang, H. Piezoelectric Actuator Placement Optimization and Active Vibration Control of a Membrane Structure. Acta Mech. Solida Sin. 2018, 31, 66–79. [Google Scholar] [CrossRef]
- Cao, K.; Li, S.; Li, Y.K.; Xin, M. Dynamic Modeling and Distributed Control of Asymmetric Flexible Spacecraft. J. Guid. Control Dyn. 2022, 46, 141–151. [Google Scholar] [CrossRef]
- Nakka, Y.K.; Chung, S.J.; Allison, J.T.; Aldrich, J.B.; Alvarez-Salazar, O.S. Nonlinear Attitude Control of a Spacecraft with Distributed Actuation of Solar Arrays. J. Guid. Control Dyn. 2019, 42, 458–475. [Google Scholar] [CrossRef]
- Meirovitch, L. Fundamentals of Vibrations; McGraw-Hill: Boston, MA, USA, 2001. [Google Scholar]
- Leleu, S.; Abou-Kandil, H.; Bonnassieux, Y. Piezoelectric actuators and sensors location for active control of flexible structures. In Proceedings of the 17th IEEE Instrumentation and Measurement Technology Conference, Baltimore, MD, USA, 1–4 May 2000; Volume 2, pp. 818–823. [Google Scholar]
- Moheimani, S.O.R.; Ryall, T.G. Considerations on placement of piezoceramic actuators that are used in structural vibration control. In Proceedings of the 38th IEEE Conference on Decision and Control (Cat. No. 99CH36304), Phoenix, AZ, USA, 7–10 December 1999; Volume 2, pp. 1118–1123. [Google Scholar]

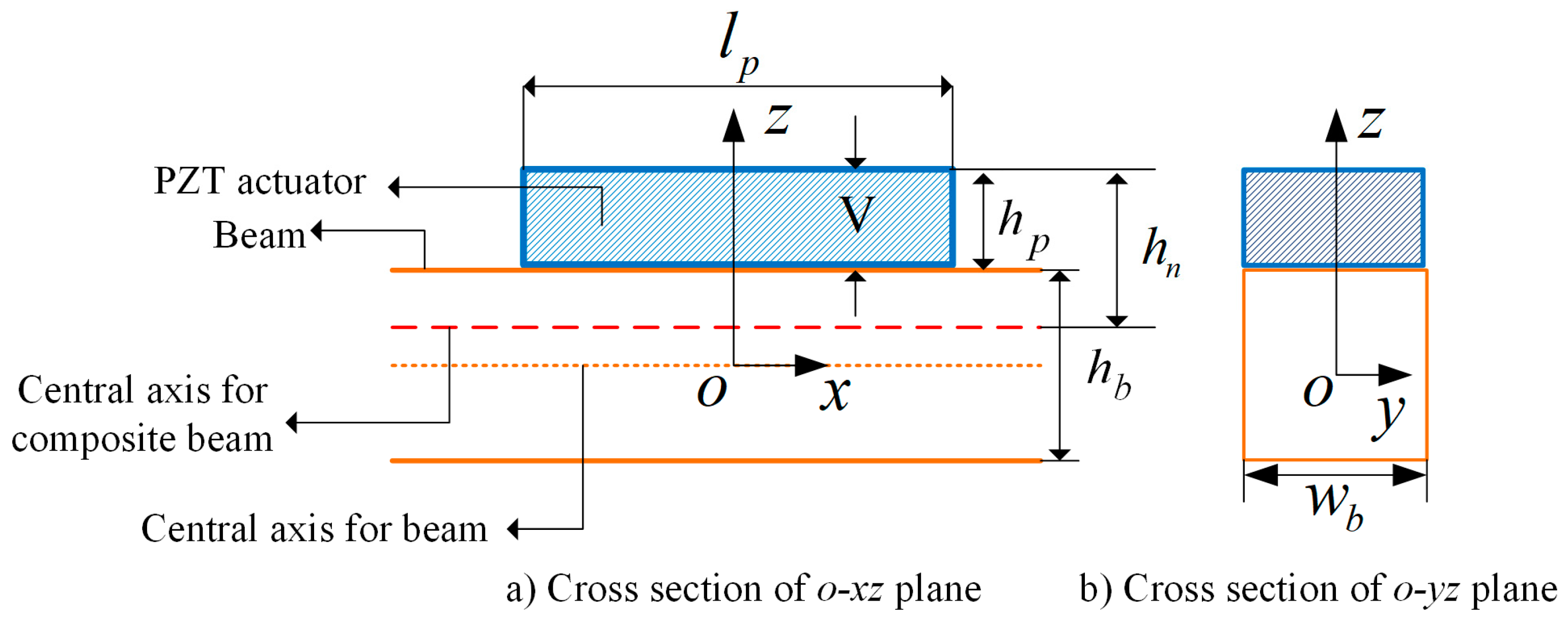
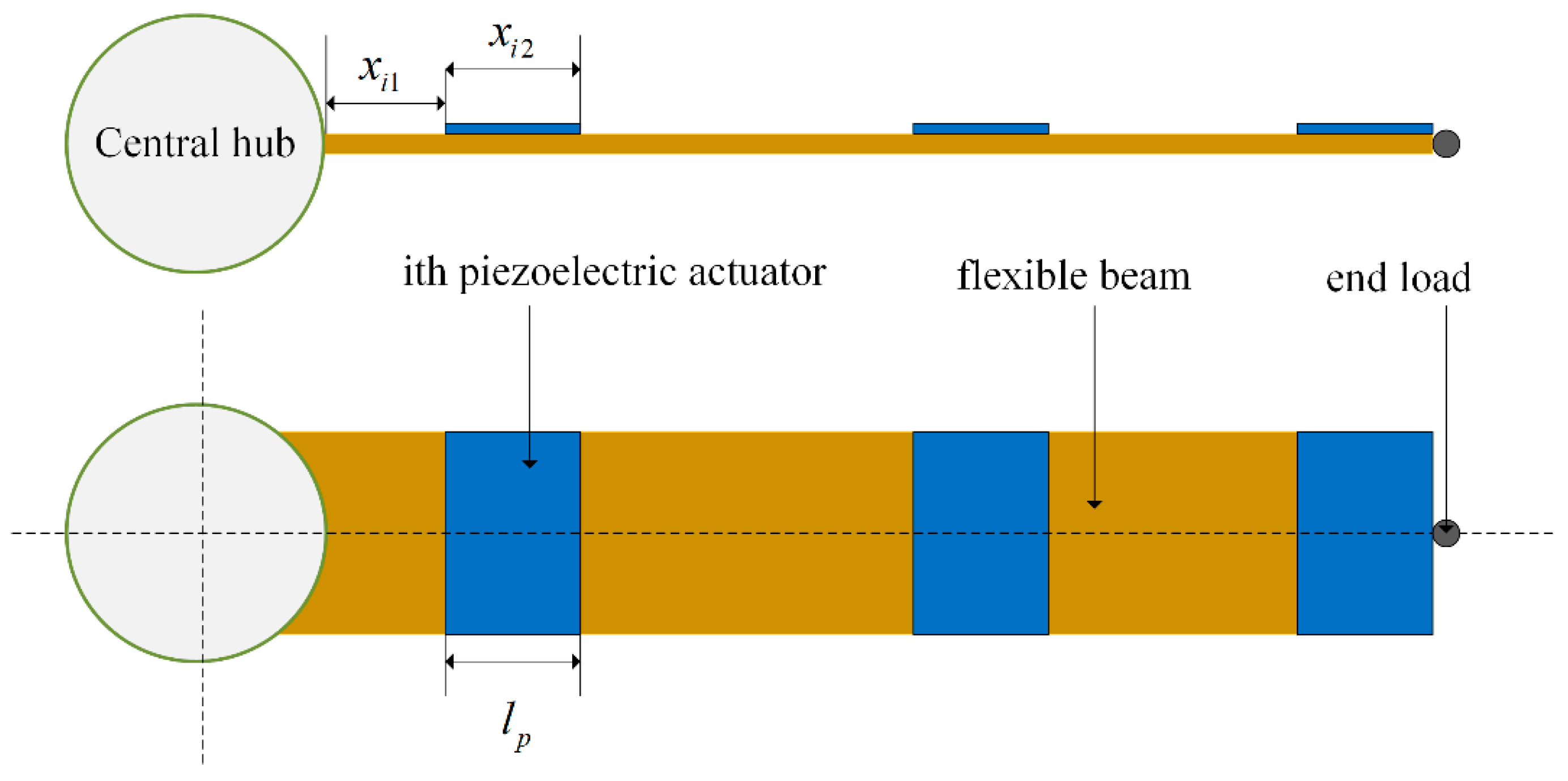
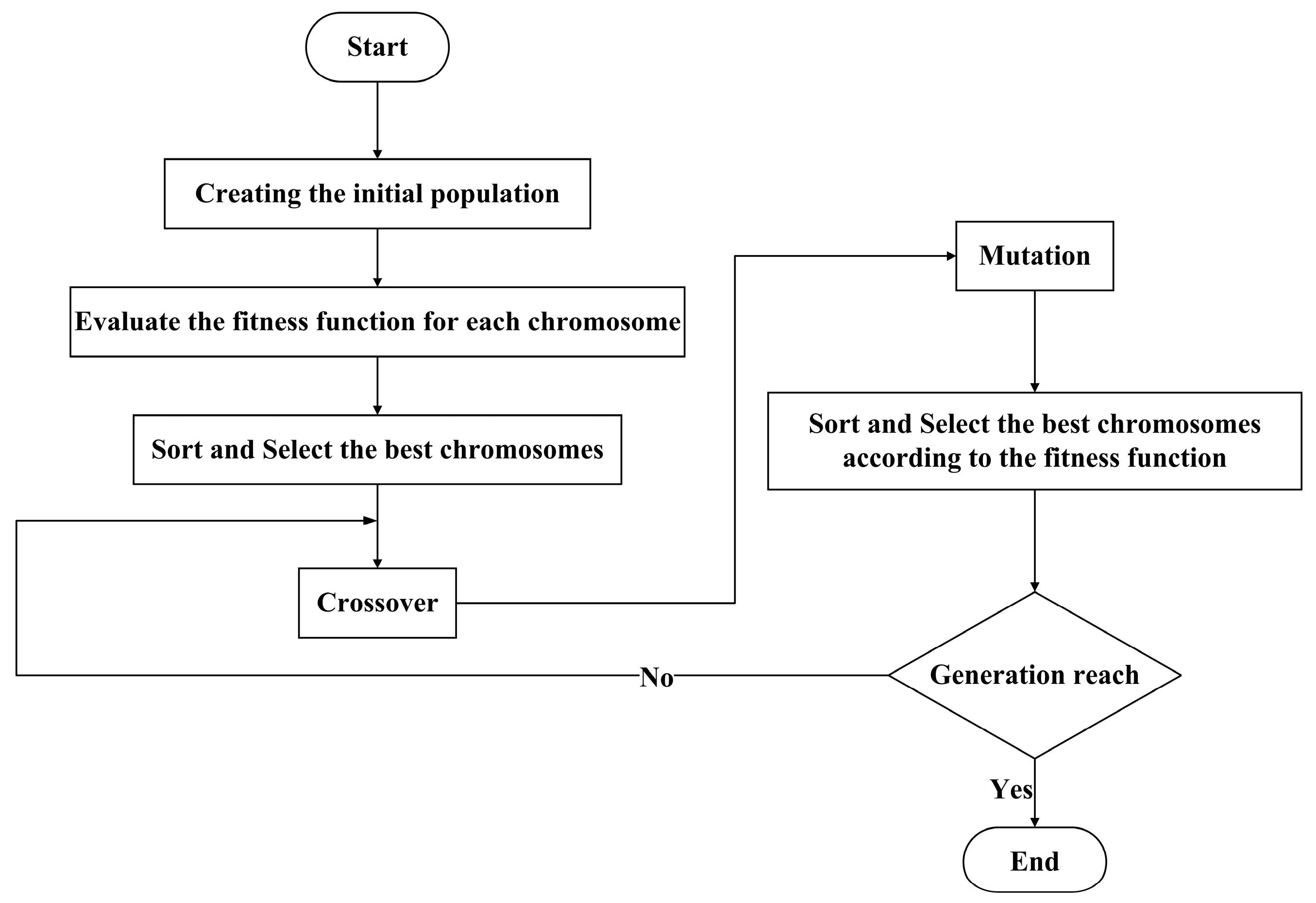





| Parameters | Value |
|---|---|
| Width () | 0.05 |
| Height () | 0.035 |
| ) | 6500 |
| Damping | 0.002 |
| Case Number | Optimal Installation Position | Optimal Fitness Value |
|---|---|---|
| 1 | ||
| 2 | ||
| 3 | ||
| 4 |
| Case Number | Length of the Right Beam | Length of the Left Beam | Actuator Installation Position on the Right Beam | Actuator Installation Position on the Left Beam |
|---|---|---|---|---|
| 1 | 5 m | 5 m | No Actuator | No Actuator |
| 2 | 5 m | 5 m | 0 m | 0 m |
| 3 | 5 m | 5 m | 2.4 m | 2.4 m |
| 4 | 5 m | 5 m | 4.8 m | 4.8 m |
| 5 | 5 m | 3 m | No Actuator | No Actuator |
| 6 | 5 m | 3 m | 0 m | 0 m |
| 7 | 5 m | 3 m | 2.4 m | 1.4 m |
| 8 | 5 m | 3 m | 4.8 m | 2.8 m |
| Component | Material Property | Value |
|---|---|---|
| Central hub | Mass (kg) | 200 |
| Radius (m) | 2 | |
| Moment of inertia (kg⋅m2) | 160 | |
| Flexible beam | Young’s modulus (GPa) | 0.689 |
| Width (m) | 0.05 | |
| Height (m) | 0.035 | |
| Density (kg/m3) | 6500 | |
| PZT actuator | Young’s modulus (GPa) | 0.63 |
| Length (m) | 0.2 | |
| Width (m) | 0.05 | |
| Height (m) | 0.005 | |
| Density(kg/m3) | 7650 | |
| (m/V) | 1 10−12 |
| 0 | 0 | 0 | 0 | 0 | 0 | 0.2 | 0.02 | 0.2 | 0.02 | 0 | 0 | 0 | 0 |
| Integral Variables | Integral Value for Case 4 | Integral Value for Case 2 |
|---|---|---|
| ) | 19.0538 | 18.9485 |
| () | 0.4857 | 0.5752 |
| () | 29.8984 | 34.7184 |
| () | 193.9818 | 190.8399 |
| () | 193.9818 | 190.8399 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, K.; Xie, R.; Zhou, J.; Zhang, X.; Wang, J.; Li, S. Optimizing the Location of the Piezoelectric Actuator and Analyzing Its Effect on the Dynamics of Asymmetric Flexible Spacecraft. Aerospace 2023, 10, 716. https://doi.org/10.3390/aerospace10080716
Cao K, Xie R, Zhou J, Zhang X, Wang J, Li S. Optimizing the Location of the Piezoelectric Actuator and Analyzing Its Effect on the Dynamics of Asymmetric Flexible Spacecraft. Aerospace. 2023; 10(8):716. https://doi.org/10.3390/aerospace10080716
Chicago/Turabian StyleCao, Kai, Renyuan Xie, Jianmin Zhou, Xiaowei Zhang, Jingji Wang, and Shuang Li. 2023. "Optimizing the Location of the Piezoelectric Actuator and Analyzing Its Effect on the Dynamics of Asymmetric Flexible Spacecraft" Aerospace 10, no. 8: 716. https://doi.org/10.3390/aerospace10080716
APA StyleCao, K., Xie, R., Zhou, J., Zhang, X., Wang, J., & Li, S. (2023). Optimizing the Location of the Piezoelectric Actuator and Analyzing Its Effect on the Dynamics of Asymmetric Flexible Spacecraft. Aerospace, 10(8), 716. https://doi.org/10.3390/aerospace10080716







