Simulation of Shock-to-Detonation Transition by OpenFOAM
Abstract
:1. Introduction
2. Governing Equations
3. Results
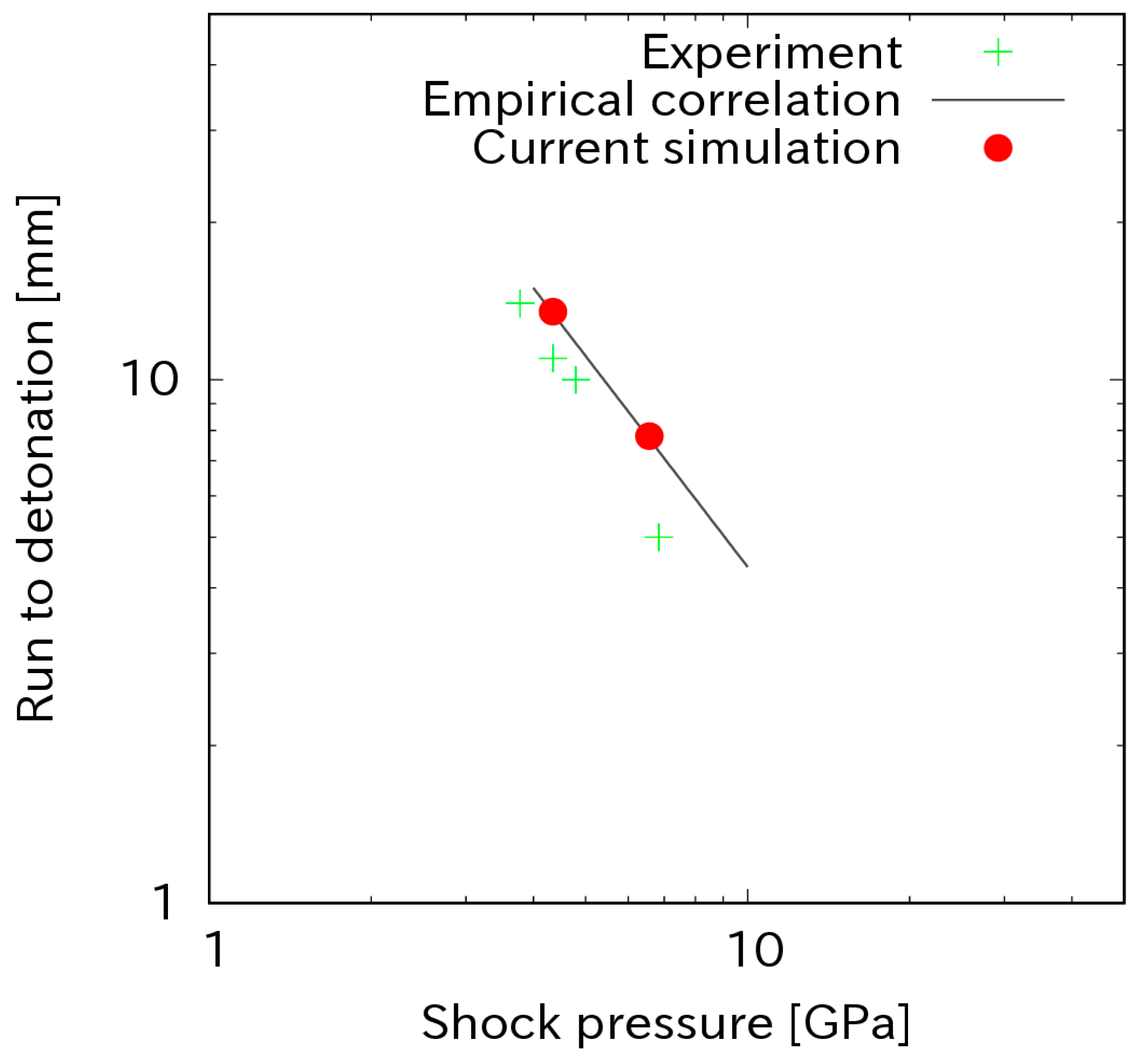
3.1. Pop Plot Calculation
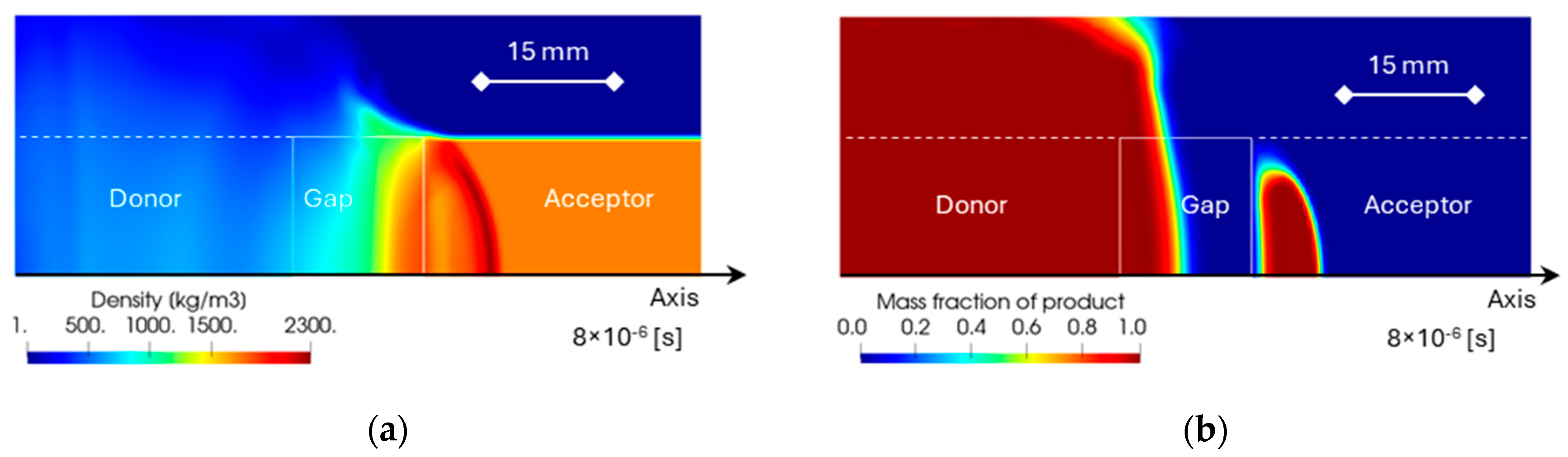
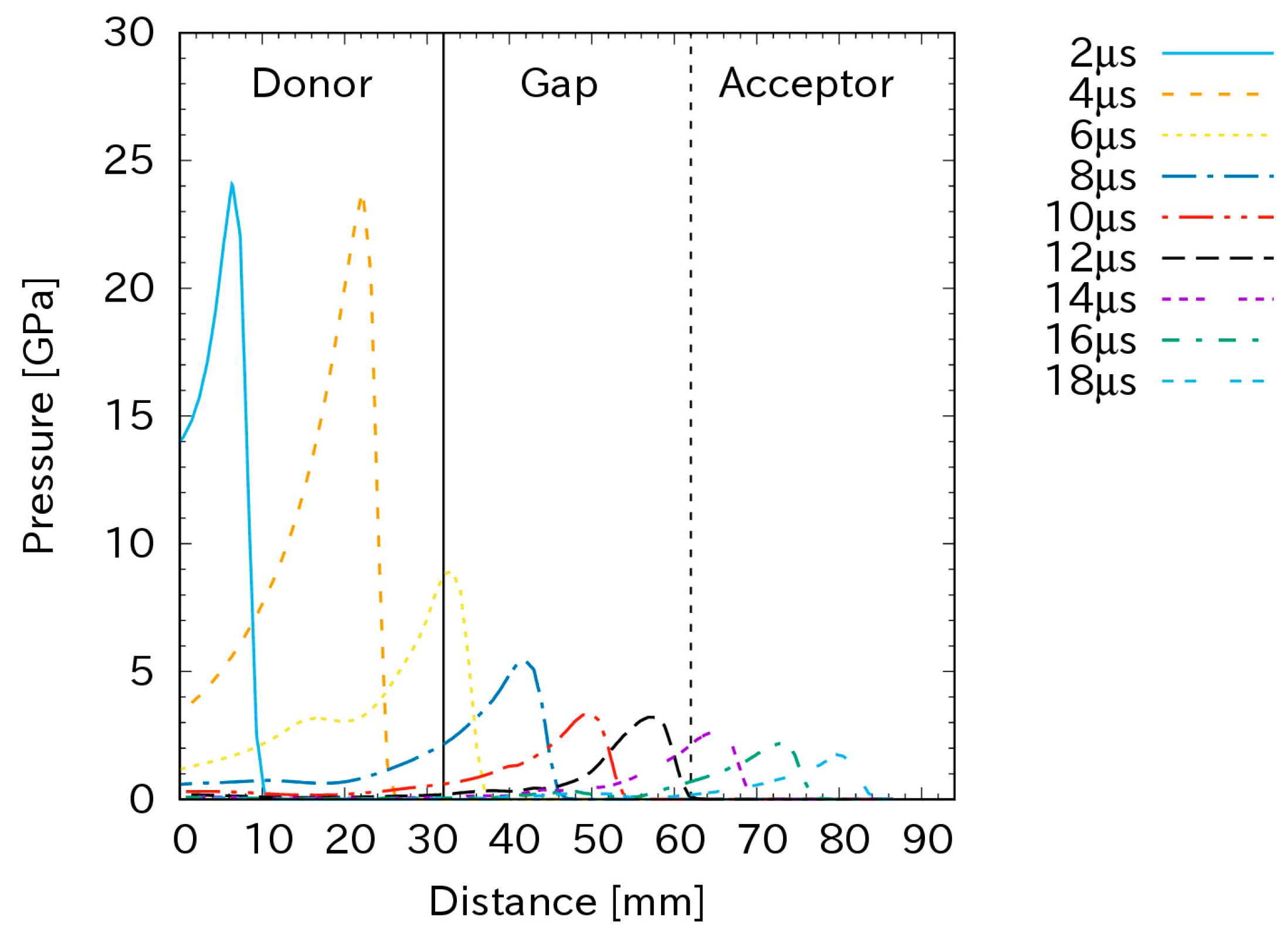
3.2. Sympathetic Detonation Simulation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, L.; Wang, C.; Feng, C.; Lu, F.; Lu, J.; Wang, X.; Guo, X. Study on random initiation phenomenon for sympathetic detonation of explosive. Def. Technol. 2013, 9, 224–228. [Google Scholar] [CrossRef]
- Saburi, T.; Kobota, S.; Wada, Y.; Yoshida, M. Development of numerical code for physical hazard analysis of high-energy materials. Sci. Tech. Energetic Mater. 2013, 74, 124–131. [Google Scholar]
- Kim, K.H.; Yoh, J.J. A particle level-set based Eulerian method for multi-material detonation simulation of high explosive and metal confinements. Proc. Combust. Inst. 2013, 34, 2025–2033. [Google Scholar] [CrossRef]
- Yang, G.; Fu, Y.; Hu, D.; Han, X. Feasibility analysis of SPH method in the simulation of condensed explosives detonation with ignition and growth model. Comput. Fluids 2013, 88, 51–59. [Google Scholar] [CrossRef]
- Ansys Inc. Ansys LS-DYNA. Multiphysics Solver. Available online: https://www.ansys.com/products/structures/ansys-ls-dyna (accessed on 9 January 2020).
- Ansys Inc. Ansys Autodyn. Short Duration, Severe Loading Simulations. Available online: https://www.ansys.com/products/structures/ansys-autodyn (accessed on 9 January 2020).
- Yuan, P.; Zhao, Y. Numerical simulation of damage to ship structure by underwater contact explosion shock wave. Chem. Eng. Trans. 2017, 62, 673–678. [Google Scholar]
- Chen, J.K.; Ching, H.K.; Allahdadi, F.A. Shock-induced detonation of high explosives by high velocity impact. J. Mech. Mater. Struct. 2007, 2, 1701–1721. [Google Scholar] [CrossRef]
- Openfoam. Available online: https://openfoam.org/ (accessed on 9 January 2020).
- Menikoff, R. JWL Equation of State; Technical Report 2017, LA-UR-15-29536; Los Alamos National Laboratory (LANL): Los Alamos, NM, USA, 2015. [Google Scholar]
- Segletes, S.B. An Analysis on the Stability of the Mie-Gruneisen Equation of State for Describing the Behavior of Shock-Loaded Materials; Technical Report 1991, BRL-TR-3214; U.S. Army Laboratory Command: Adelphi, ML, USA, 1991. [Google Scholar]
- Tarver, C.T. Modeling detonation experiments on Triaminotrinitrobenzene (TATB)-based explosives LX-17, PBX 9502, and ultrafine TATB. Energetic Mater. 2012, 30, 220–251. [Google Scholar] [CrossRef]
- Lorenzo, M.D.; Pelanti, M.P.; Lafon, P. HLLC-type and path-conservative schemes for a single-velocity six-equation two-phase flow model: A comparative study. Appl. Math. Comput. 2018, 333, 95–117. [Google Scholar] [CrossRef]
- Shima, E.; Kitamura, K. Parameter-free simple low-dissipation AUSM-family scheme for all speeds. AIAA 2011, 49, 1693–1709. [Google Scholar] [CrossRef]
- Urtiew, P.A.; Vandersall, K.S.; Tarver, C.M.; Gacia, F.; Forbes, J.W. Shock initiation of composition B and C-4 explosives: Experiments and modeling. In Proceedings of the International Conference of Shock Waves in Condensed Matter, Saint Petersburg, Russia, 3–8 September 2006. [Google Scholar]
- Gibbs, T.R.; Popolato, A. LASL Explosive Property Data; University of California Press: Berkeley, CA, USA, 1980; p. 19. [Google Scholar]
- Kubota, S.; Liu, Z.; Otsuki, S.; Nakayama, Y.; Ogata, Y.; Yoshida, M. A numerical study of sympathetic detonation in gap test. Mater. Sci. Forum 2004, 465–466, 163–168. [Google Scholar] [CrossRef]
- Kim, B.; Kim, M.; Yoh, J.J. Shock to detonation transition analysis using experiments and models. Proc. Combust. Inst. 2017, 36, 2699–2707. [Google Scholar] [CrossRef]




| Parameter | Reactant | Product | Unit |
|---|---|---|---|
| A | 778.1 | 5.242 | [Mbar] |
| B | −0.05301 | 0.07678 | (Mbar] |
| R1 | 11.3 | 4.2 | [-] |
| R2 | 1.13 | 1.1 | [-] |
| ω | 0.8938 | 0.5 | [-] |
| E0 | - | 4.9505 | [MJ/kg] |
| ρ0 | 1717.0 | - | [kgm−3] |
| Parameter | Reactant | Unit |
|---|---|---|
| I | 4.0 × 1012 | [s−1] |
| a | 0.0367 | [-] |
| b | 0.667 | [-] |
| x | 7.0 | [-] |
| G1 | 140.0 × 106 | [Mbar−2s−1] |
| c | 0.667 | [-] |
| d | 1.0 | [-] |
| y | 2.0 | [-] |
| G2 | 1000.0 × 106 | Mbar−3s−1 |
| e | 0.222 | [-] |
| g | 1.0 | [-] |
| z | 3.0 | [-] |
| λImax | 0.022 | [-] |
| λG1max | 0.7 | [-] |
| λG2min | 0.0 | [-] |
| Parameter | Value | Unit |
|---|---|---|
| c0 | 5240.0 | (ms−1) |
| a | 0.48 | (-) |
| s | 1.4 | (-) |
| Γ0 | 1.97 | (-) |
| ρ0 | 2703.0 | (kgm−3) |
| Parameter | Value | Unit |
|---|---|---|
| c0 | 2180.0 | (ms−1) |
| a | 0.0 | (-) |
| s | 1.41 | (-) |
| Γ0 | 0.85 | (-) |
| ρ0 | 1182.0 | (kgm−3) |
| c0 | 2180.0 | (ms−1) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dinh, T.X.; Yoshida, M.; Ishikura, S. Simulation of Shock-to-Detonation Transition by OpenFOAM. Aerospace 2025, 12, 214. https://doi.org/10.3390/aerospace12030214
Dinh TX, Yoshida M, Ishikura S. Simulation of Shock-to-Detonation Transition by OpenFOAM. Aerospace. 2025; 12(3):214. https://doi.org/10.3390/aerospace12030214
Chicago/Turabian StyleDinh, Thien Xuan, Masatake Yoshida, and Shuichi Ishikura. 2025. "Simulation of Shock-to-Detonation Transition by OpenFOAM" Aerospace 12, no. 3: 214. https://doi.org/10.3390/aerospace12030214
APA StyleDinh, T. X., Yoshida, M., & Ishikura, S. (2025). Simulation of Shock-to-Detonation Transition by OpenFOAM. Aerospace, 12(3), 214. https://doi.org/10.3390/aerospace12030214





