Design of an Orbital Infrastructure to Guarantee Continuous Communication to the Lunar South Pole Region
Abstract
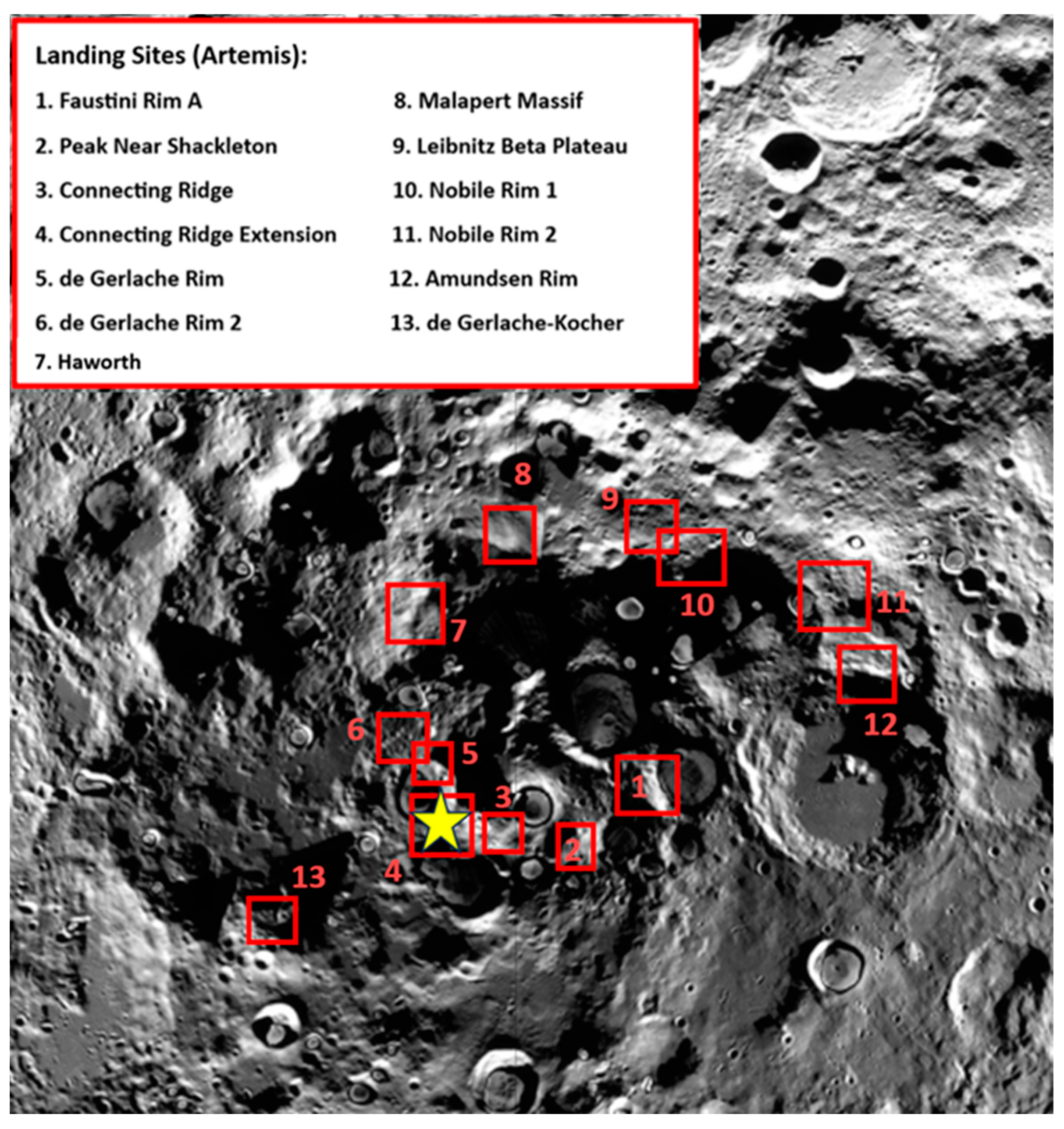
:1. Introduction
2. Multi-Body Constellation Design
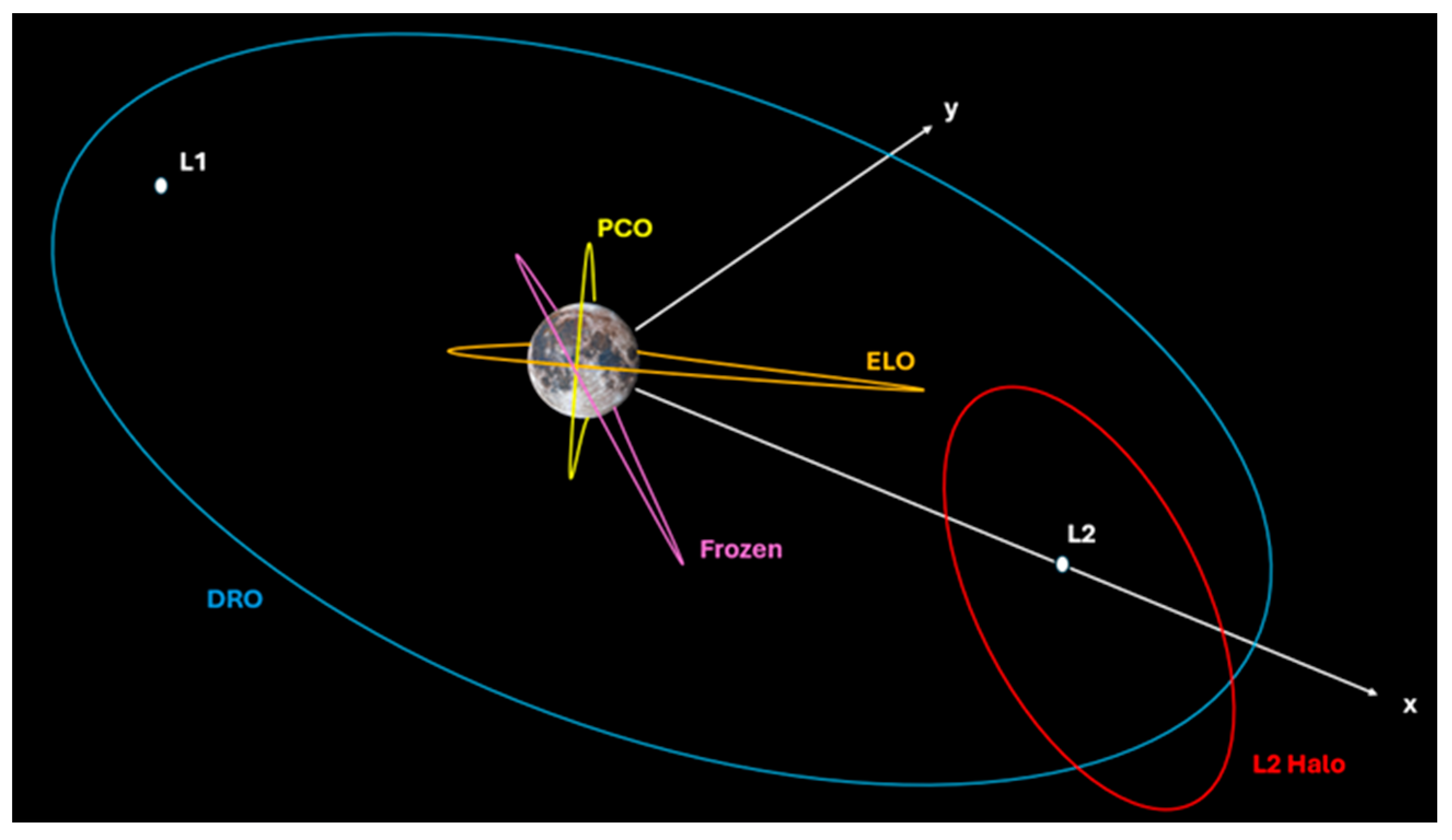
2.1. Possible Cislunar Orbits
- Low Lunar Orbits (LLOs) are typically circular orbits or slightly elliptical with an average altitude of around 100 km and any possible inclination; these orbits turn out to be the perfect candidate for surface access.
- Elliptical Lunar Orbits (ELOs) are equatorial orbits with a low perilune distance providing favourable access to the surface, but compared to the previous one they reduce access costs from Earth.
- Prograde Circular Orbits (PCOs) have a radius up to 5000 km; they are highly stable and require almost zero corrections to be maintained.
- Frozen Lunar Orbits are orbits with constant average eccentricity and an argument of periapsis values; this kind of orbit was discovered by analysing gravitational potential and looking for regions, corresponding to defined orbital parameters, in which effects due to perturbations are cancelled out or minimised. Those orbits exist for only certain combinations of energy, eccentricity, and inclination, and although they can provide high stability over time, research into them is not trivial. An in-depth study of this kind of orbit may be found in [9].
- Earth–Moon Liberation Point Halo Orbits are periodic orbits around the collinear liberation points which remain relatively fixed in the Earth–Moon plane, rotating at the same rate as that of the Moon around the Earth and that of the Moon around its own axis. Farquhar coined the term halo in 1966, noting how these orbits create a halo about the Moon by observing them from Earth [10]. Within a full ephemeris model, they may be slightly unstable, but their strengths include continuous Earth visibility, relatively easy access from Earth, and in certain cases predictable behaviour.
- Distant Retrograde Orbits (DROs) are a stable family of planar orbits about the smaller primary within the three-body problem [11].
2.2. Lunar Constellation
- The street-of-coverage design, typically involving satellites in polar orbits with a separation between the orbital planes selected such that the ground coverage of satellites in adjacent planes overlaps to provide full coverage.
- The Walker/Mozhaev constellation consisting of evenly distributed satellites in evenly distributed planes in circular orbits.
- The Flower Constellations (FCs) conceived by [14] based on placing all satellites on the same trajectory in a rotating reference frame. When viewed from the rotating frame, the common trajectory appears as multi-petaled “flowers”. An FC is generally characterised by repeatable ground tracks and suitable phasing mechanics. The basic requirements that an FC must have can be summarised as follows:
- -
- Identical orbit shape: anomalistic period, argument of perigee, height of perigee, and inclination;
- -
- Compatibility: the orbital period is evaluated in such a way as to yield a perfectly repeated ground track;
- -
- An equally displaced node line along the equatorial plane for each satellite in a complete FC.
Architectures for Lunar South Pole Coverage
- Sufficiently large semi-major axis values to produce continuous single and double coverage with a minimal number of satellites;
- Large eccentricity values to focus coverage near apoapsis for longer contact durations;
- Inclination values to orient the coverage swaths over the poles (rather than the equator);
- The argument of periapsis values set to either 90° or 270° depending on whether apoapsis should be over the south pole or north pole, respectively.
2.3. Candidate Orbits: Halo Southern Families
3. Dynamical Results
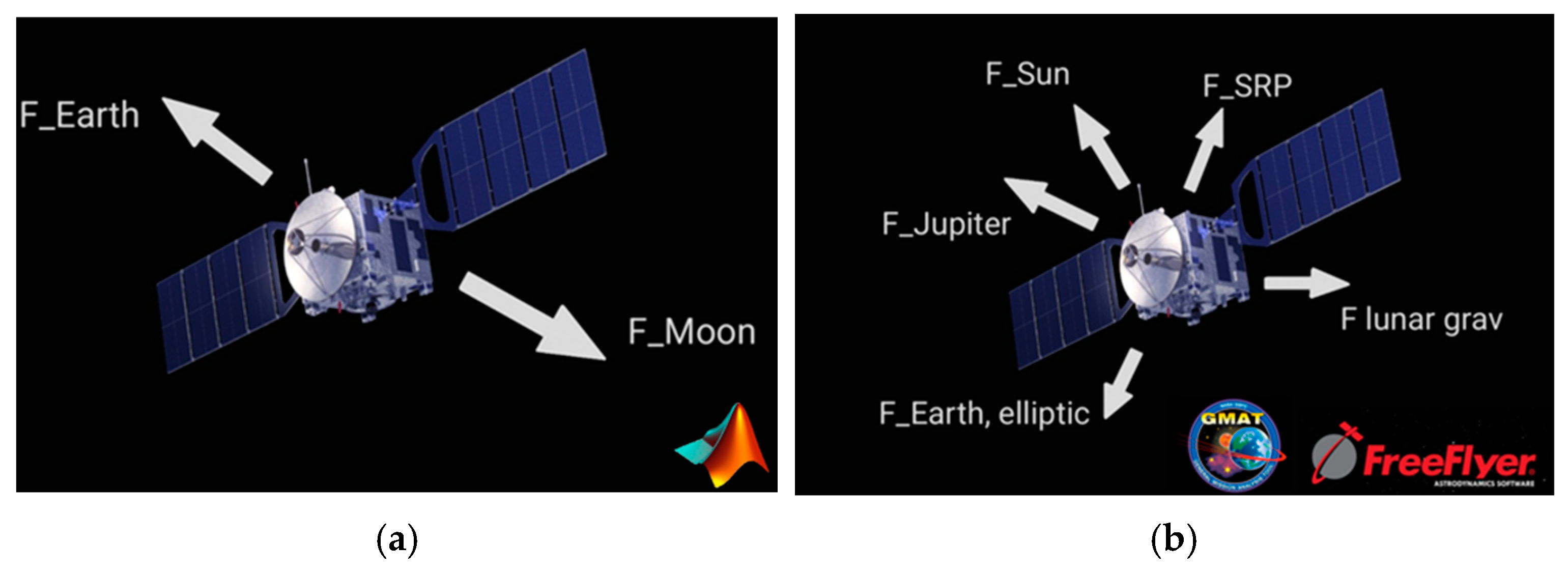
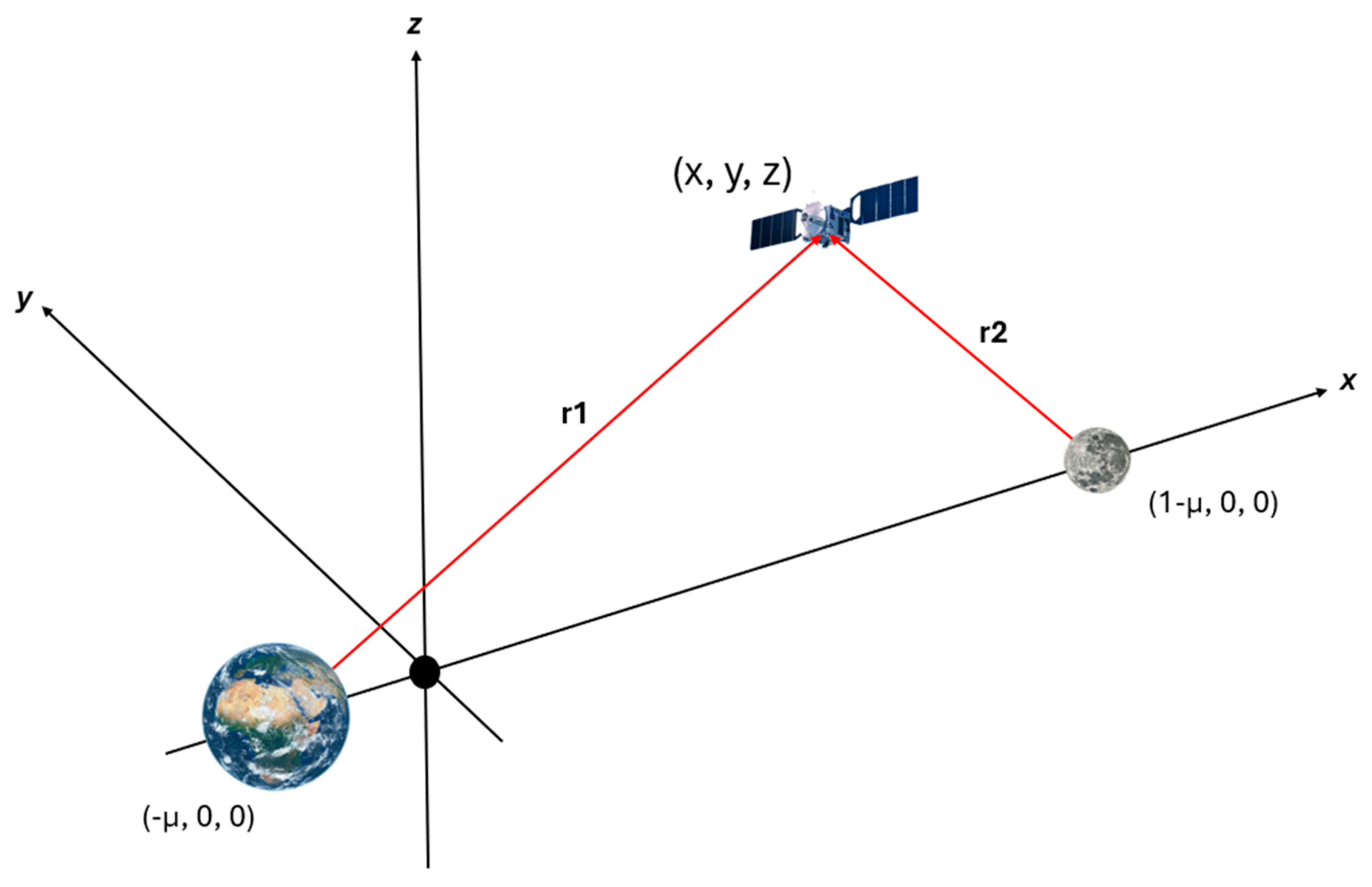
3.1. The Circular Restricted Three-Body Problem
- The masses are normalised so that m1 = 1 − µ and m2 = µ, where µ = m2/(m1 + m2) is the mass parameter of the system.
- The angular velocity of the comoving rotating frame is normalised to one.
- The distance between m1 and m2 has their coordinates in the reference system as (−µ, 0, 0) and (1 − µ, 0, 0) on the x-axis, as viewable in Figure 3. In this way, the distances of the third body to m1 and m2 are given by the following:
- Lastly, the introduction of the non-dimensional time τ is useful during the integration procedurewhere t* is the characteristic time of the systemso the equations of motion expressed in a non-dimensional form becomethereby demonstrating the effective potential, and Ux, Uy and Uz are the partial derivatives of U with respect to the position variables. Table 1 offers the main parameters of the Earth–Moon system.
- The eigenvalues of the monodromy matrix always appear in reciprocal pairs.
- One pair of eigenvalues is always equal to unity due to the periodicity of the orbit and the existence of a family of such orbits with precisely periodic behaviour. The other two reciprocal pairs of eigenvalues (λi, 1/λi) are combined into a single metric for the purpose of describing the stability; it is therefore possible to introduce the stability index as
3.1.1. Preliminary Results
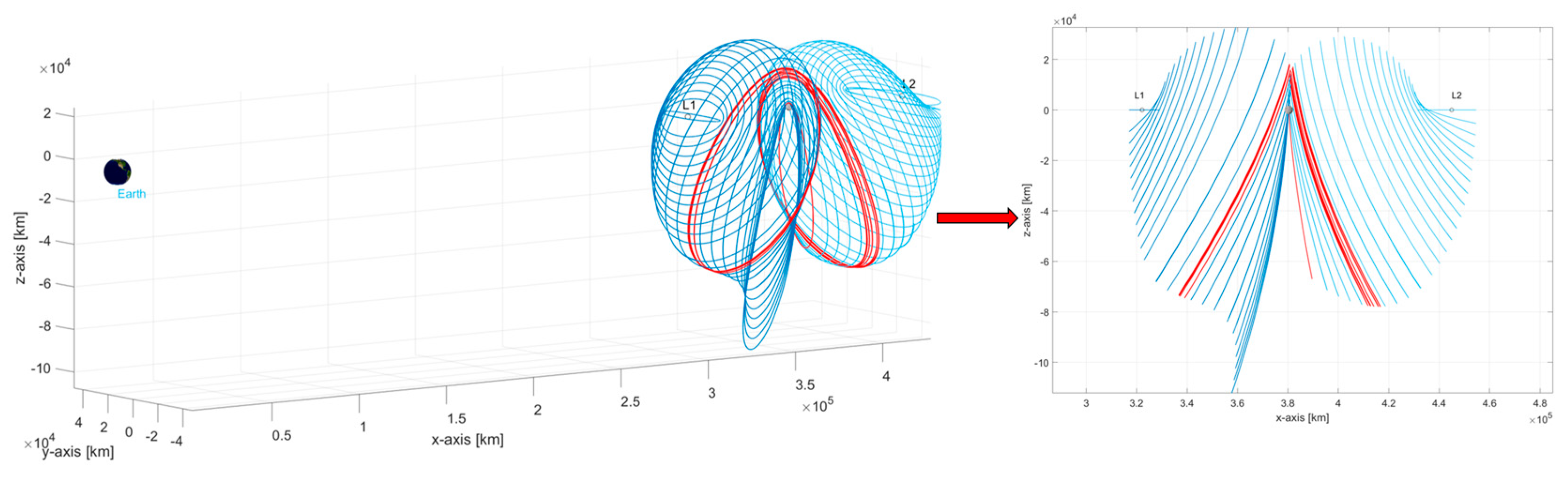
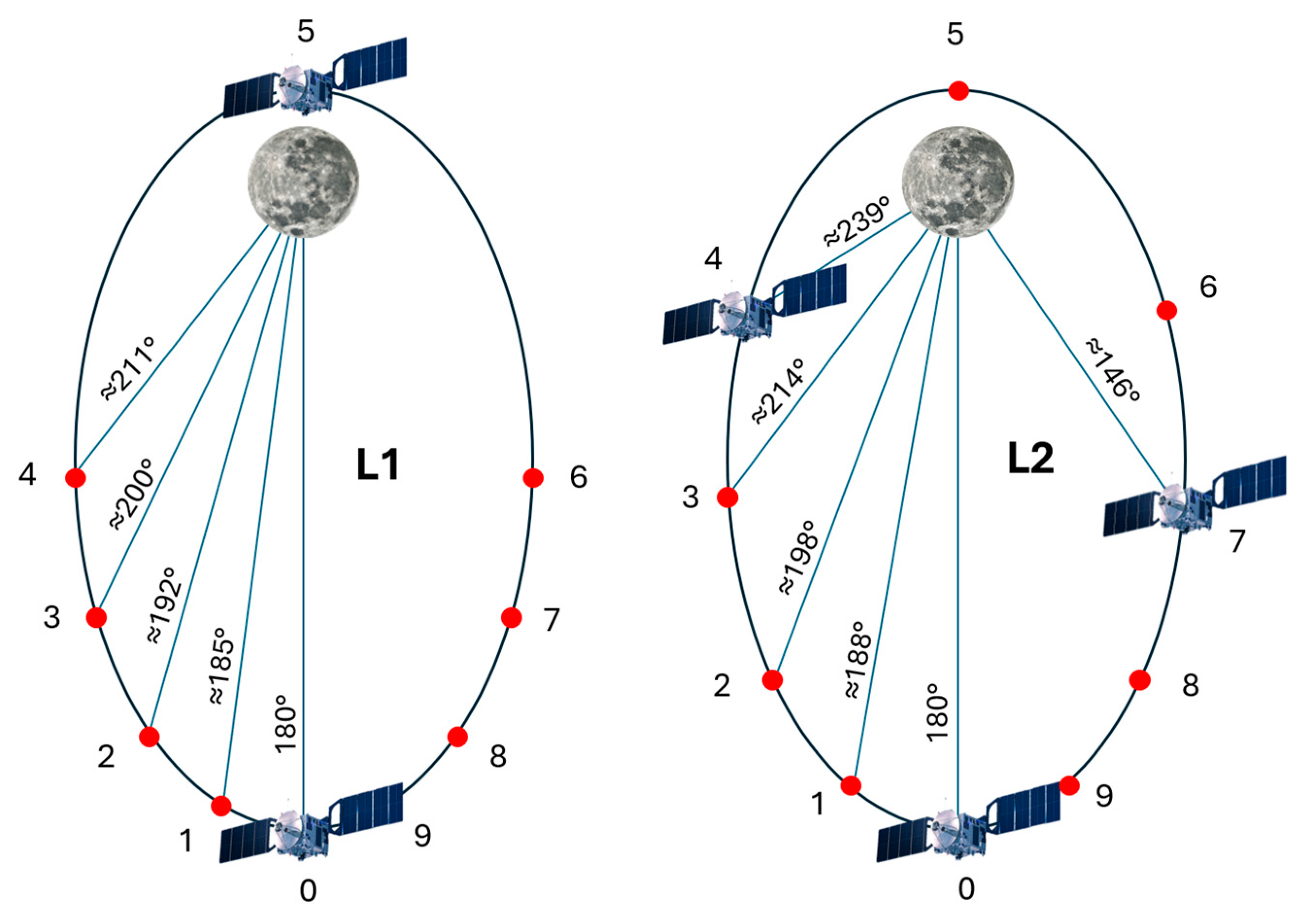
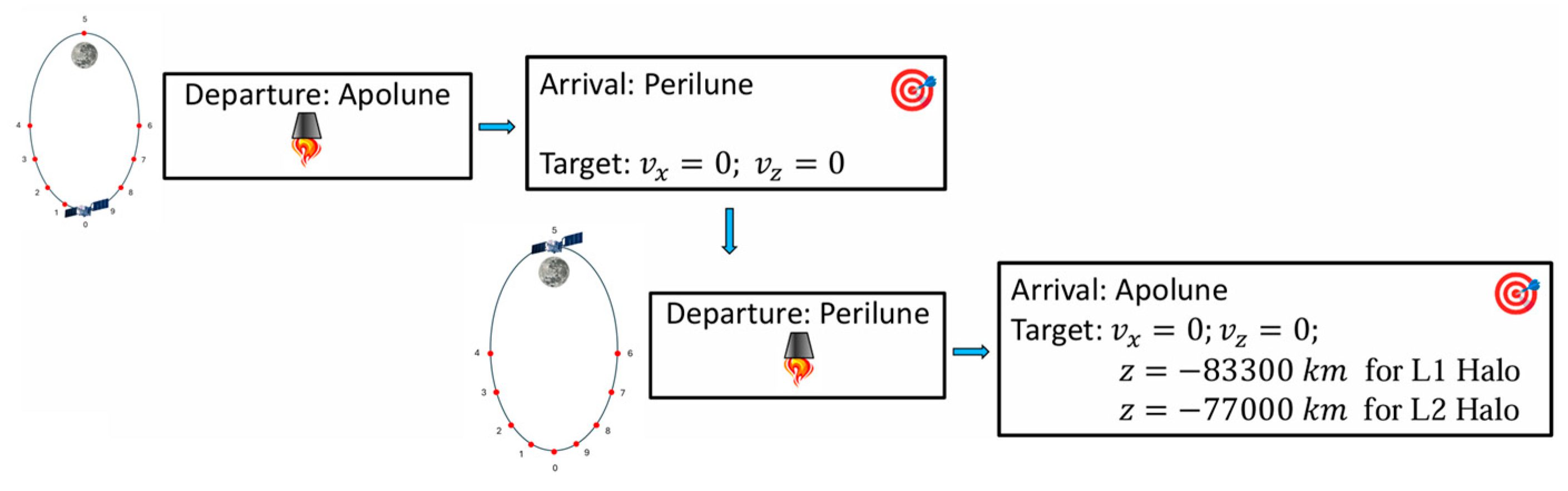
3.1.2. Orbit Selection
3.2. High-Fidelity Simulations
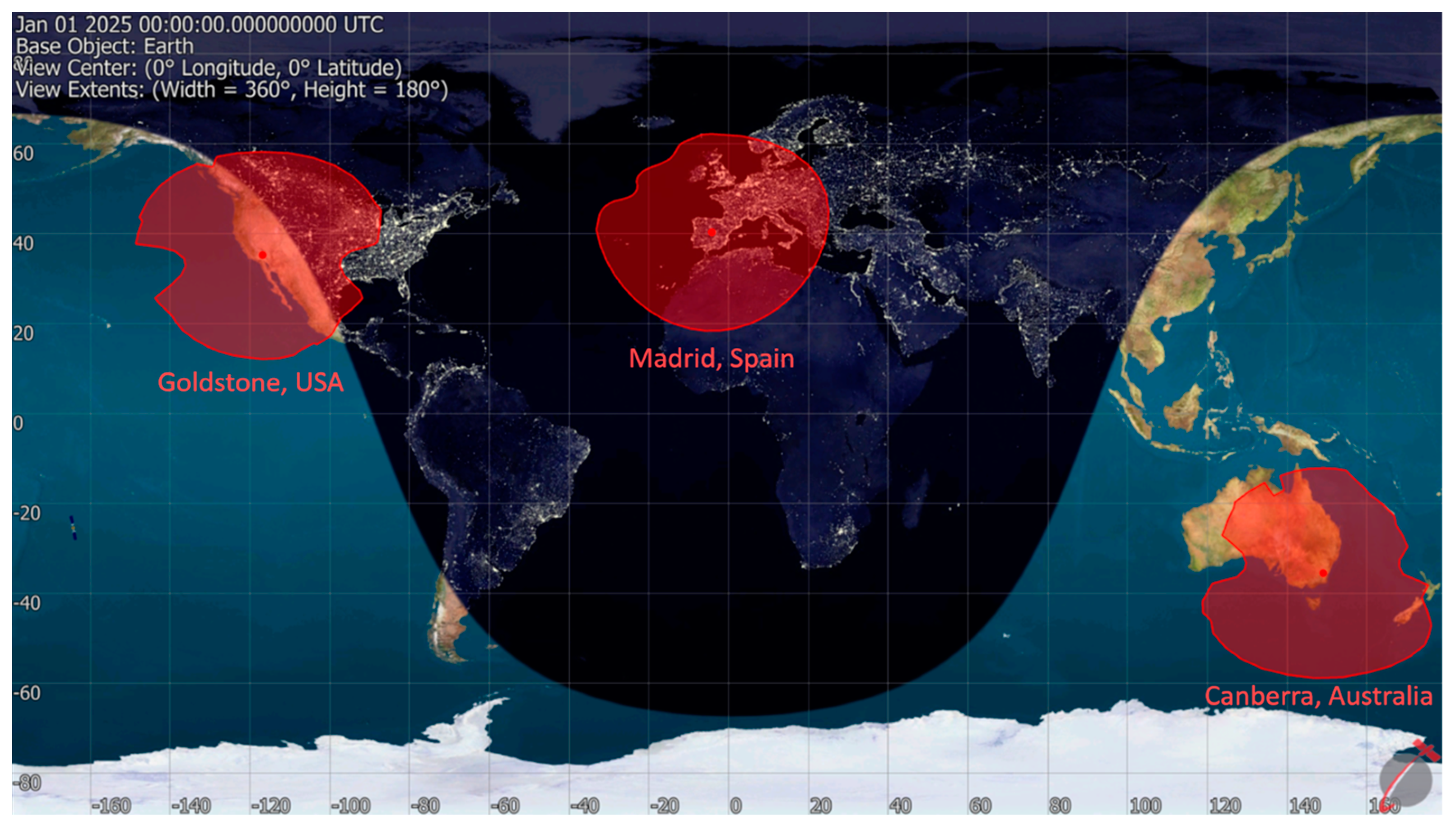
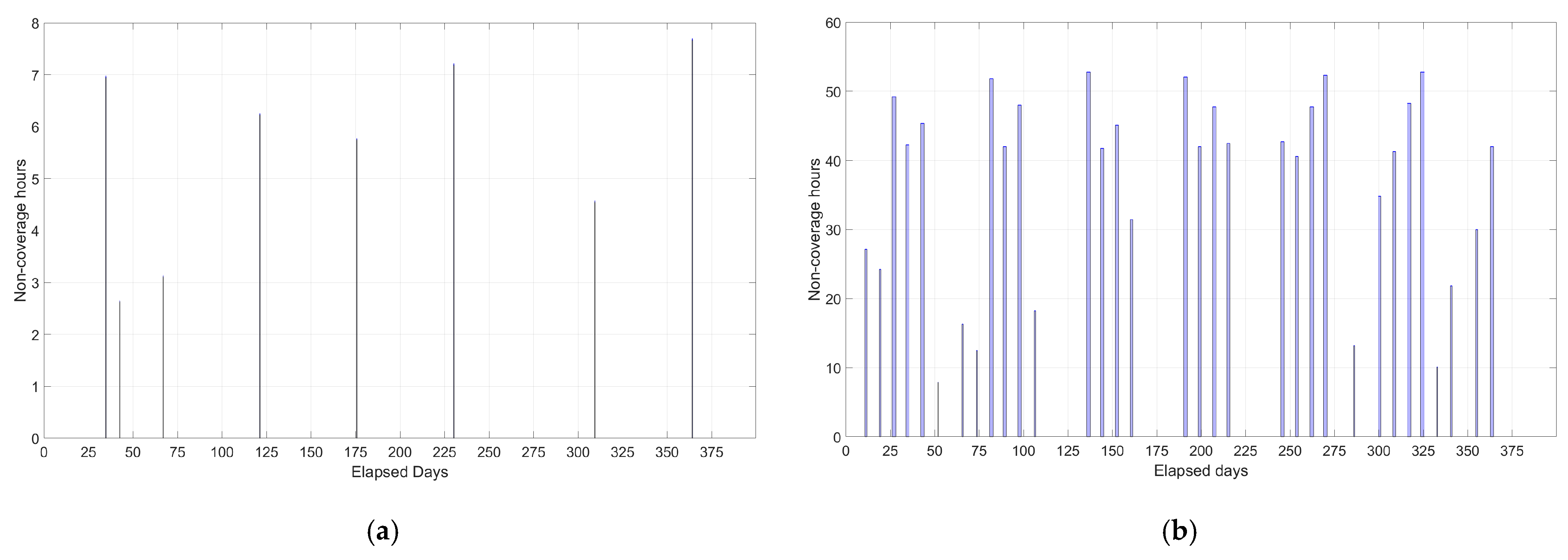
3.2.1. Earth Visibility
3.2.2. Constellation Design and Coverage Analysis
4. Discussion
- Improvement of the preliminary simulation within the CR3BP moving to a more detailed analysis exploiting the Bicircular Restricted Four-Body Problem (BCR4BP) which considers the solar influence; this could surely enhance the stability analysis and enrich the library of initial state vectors for orbits around the Lagrange point of the Earth–Moon system.
- Searching for combinations of orbits with periods as similar as possible to overcome the phase synchronisation issues observed in the previous sections. Ensuring the best possible phase control means minimising the need for corrective manoeuvres to restore the nominal conditions of the constellation, thus reducing costs.
- Refining the gravitational model within the high-fidelity simulations.
- Introducing a detailed satellite geometry such as the spacecraft surface area to conduct highly realistic assessments on station keeping and the propulsion system.
- Improvement of the communication analysis by equipping the satellite with a suitable antenna and introducing possible functional and performance requirements for the entire telecommunication infrastructure. Also, from a communication point of view, it will be interesting to provide an order of magnitude regarding the maximum tolerable coverage gaps for lunar ground facilities.
- Observing the contribution of the Gateway project and understanding if it could contribute to the telecom constellation.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| 0.8234 | 0 | 0.1262312 | 11.9732 |
| 0.8234 | −0.0224 | 0.1341195 | 11.9877 |
| 0.8235 | −0.0344 | 0.1435988 | 12.0061 |
| 0.8237 | −0.0464 | 0.1560969 | 12.0340 |
| 0.8242 | −0.0584 | 0.1691586 | 12.0632 |
| 0.8250 | −0.0704 | 0.18236 | 12.0922 |
| 0.8260 | −0.0824 | 0.19626 | 12.1247 |
| 0.8273 | −0.0944 | 0.209415 | 12.1505 |
| 0.8289 | −0.1064 | 0.221528 | 12.1633 |
| 0.8307 | −0.1184 | 0.23342 | 12.1629 |
| 0.8329 | −0.1304 | 0.24348 | 12.1229 |
| 0.8355 | −0.1424 | 0.2527 | 12.0447 |
| 0.8389 | −0.1544 | 0.259948 | 11.8798 |
| 0.8510 | −0.1765 | 0.2628 | 11.0794 |
| 0.8629 | −0.1858 | 0.2504 | 10.2873 |
| 0.8744 | −0.1904 | 0.2345 | 9.5822 |
| 0.8749 | −0.1914 | 0.2325 | 9.5670 |
| 0.8759 | −0.1918 | 0.2308 | 9.5110 |
| 0.8808 | −0.1935 | 0.2225 | 9.2445 |
| 0.8869 | −0.1957 | 0.2110 | 8.9374 |
| 0.8989 | −0.2002 | 0.1865 | 8.4202 |
| 0.9109 | −0.2060 | 0.1590 | 8.0392 |
| 0.9246 | −0.2180 | 0.1232 | 7.8781 |
| 0.9305 | −0.2300 | 0.104359 | 8.0312 |
| 0.9330 | −0.2420 | 0.09355 | 8.2753 |
| 0.9335 | −0.2540 | 0.087415 | 8.5496 |
| 0.9329 | −0.2660 | 0.083856 | 8.8269 |
| 0.9315 | −0.2780 | 0.0821 | 9.0984 |
| 0.9292 | −0.2914 | 0.081687 | 9.3894 |
| 1.1809 | 0 | −0.155865 | 14.9095 |
| 1.1807 | −0.0139 | −0.1569 | 14.9022 |
| 1.1802 | −0.0259 | −0.15942 | 14.8847 |
| 1.1794 | −0.0379 | −0.16349 | 14.8567 |
| 1.1782 | −0.0499 | −0.168392 | 14.8172 |
| 1.1767 | −0.0619 | −0.17489 | 14.7704 |
| 1.1746 | −0.0739 | −0.180805 | 14.7072 |
| 1.1721 | −0.0859 | −0.187706 | 14.6328 |
| 1.1690 | −0.0979 | −0.19417 | 14.5411 |
| 1.1654 | −0.1099 | −0.20112 | 14.4345 |
| 1.1611 | −0.1219 | −0.20725 | 14.3031 |
| 1.1561 | −0.1339 | −0.2129 | 14.1451 |
| 1.1503 | −0.1459 | −0.21792 | 13.9512 |
| 1.1435 | −0.1579 | −0.2219 | 13.7039 |
| 1.1354 | −0.1699 | −0.2247 | 13.3782 |
| 1.12345 | −0.1837 | −0.22512 | 12.8289 |
| 1.1113 | −0.1934 | −0.22204 | 12.1739 |
| 1.0994 | −0.1993 | −0.2153 | 11.4665 |
| 1.0874 | −0.2020 | −0.2054 | 10.6961 |
| 1.0824 | −0.20207 | −0.20108 | 10.3581 |
| 1.0804 | −0.20212 | −0.1988 | 10.2266 |
| 1.0754 | −0.2022 | −0.1926 | 9.9076 |
| 1.0724 | −0.2015 | −0.1895 | 9.6924 |
| 1.0694 | −0.20105 | −0.1860 | 9.4966 |
| 1.0634 | −0.2003 | −0.1770 | 9.1155 |
| 1.0514 | −0.1968 | −0.1589 | 8.3465 |
| 1.0394 | −0.1919 | −0.1381 | 7.6086 |
| 1.0274 | −0.1856 | 0.1146 | 6.9067 |
| 1.0118 | −0.1739 | −0.0799 | 5.9981 |
References
- Cohen, B.A. Lunar Mission Priorities for the Decade 2023–2033; NASA: Washington, DC, USA, 2020. [Google Scholar]
- Tai, W.; Cosby, M.; Lanucara, M. Lunar Communications Architecture Study Report (v1. 3). Interagency Operations Advisory Group, Lunar Communications Architecture Working Group. 2022. Available online: https://www.ioag.org/Public%20Documents/Lunar%20communications%20architecture%20study%20report%20FINAL%20v1.3.pdf (accessed on 14 February 2025).
- Trabacchin, N.; Ochner, P.; Colombatti, G. A Physical and Spectroscopic Survey of the Lunar South Pole with the Galileo Telescope of the Asiago Astrophysical Observatory. Aerospace 2024, 11, 693. [Google Scholar] [CrossRef]
- ESA Moonlight. Available online: https://connectivity.esa.int/esa-moonlight (accessed on 31 January 2025).
- Ely, T.A. Coverage and Control of Constellations of Elliptical Inclined Frozen Lunar Orbits. In Proceedings of the 2005 AAS/AIAA Astrodynamics Specialist Conference, Lake Tahoe, CA, USA, 7–11 August 2005. [Google Scholar]
- Lu, Y.; Yang, Y.; Li, X.; Zhu, Z.; Li, H.; Dong, G. Lunar Polar Orbit Relay Constellation and Its Deployment and Maintenance. In Proceedings of the 2012 Second International Conference on Intelligent System Design and Engineering Application, Sanya, China, 6–7 January 2012; pp. 333–336. [Google Scholar] [CrossRef]
- Grebow, D. Generating Periodic Orbits in the Circular Restricted Three-Body Problem with Applications to Lunar South Pole Coverage. Master’s Thesis, School of Aeronautics and Astronautics, Purdue University, West Lafayette, IN, USA, 2006; pp. 8–14. [Google Scholar]
- Whitley, R.; Martinez, R. Options for staging orbits in cislunar space. In Proceedings of the 2016 IEEE Aerospace Conference, Big Sky, MT, USA, 5–12 March 2016; pp. 1–9. [Google Scholar]
- Cinelli, M.; Ortore, E.; Mengali, G.; Quarta, A.A.; Circi, C. Lunar orbits for telecommunication and navigation services. Astrodynamics 2024, 8, 209–220. [Google Scholar]
- FARQUHAR, R.W. Lunar communications with libration-point satellites. J. Spacecr. Rocket. 1967, 4, 1383–1384. [Google Scholar] [CrossRef]
- Hénon, M. Numerical exploration of the restricted problem, V. Astron. Astrophys. 1969, 1, 223–238. [Google Scholar]
- GRAIL. Available online: https://www.jpl.nasa.gov/missions/gravity-recovery-and-interior-laboratory-grail/ (accessed on 31 January 2025).
- SELENE (Selenological and Engineering Explorer). Available online: https://www.eoportal.org/satellite-missions/selene (accessed on 31 January 2025).
- Mortari, D.; Wilkins, M.P.; Bruccoleri, C. The Flower Constellations. J. Astronaut. Sci. 2004, 52, 107–127. [Google Scholar] [CrossRef]
- Mortari, D.; De Sanctis, M.; Lucente, M. Design of Flower Constellations for Telecom Munication Services. Proc. IEEE 2011, 99, 2008–2019. [Google Scholar] [CrossRef]
- Ely, T.A. Stable constellations of frozen elliptical inclined lunar orbits. J. Astronaut. Sci. 2005, 53, 301–316. [Google Scholar] [CrossRef]
- Ely, T.A.; Lieb, E. Constellations of elliptical inclined lunar orbits providing polar and global coverage. J. Astronaut. Sci. 2006, 54, 53–67. [Google Scholar] [CrossRef]
- Shirobokov, M.; Tromov, S.; Ovchinnikov, M. Lunar Frozen Orbits for Small Satellite Communication/Navigation Constellations. In Proceedings of the International Astronautical Congress, IAC, Dubai, United Arab Emirates, 25–29 October 2021. [Google Scholar]
- Nallapu, R.; Vance, L.D.; Xu, Y.; Thangavelatham, J. Automated design architecture for lunar constellations. In Proceedings of the 2020 IEEE Aerospace Conference, Big Sky, MT, USA, 7–14 March 2020; pp. 1–11. [Google Scholar]
- Lee, D.E. White Paper: Gateway Destination Orbit Model: A Continuous 15 Year Nrho Reference Trajectory; No. JSC-E-DAA-TN72594; NASA: Washington, DC, USA, 2019. [Google Scholar]
- Zimovan, E.M.; Howell, K.C.; Davis, D.C. Near rectilinear halo orbits and their application in cis-lunar space. In Proceedings of the 3rd IAA Conference on Dynamics and Control of Space Systems, Moscow, Russia, 30 May–1 June 2017; Volume 20, p. 40. [Google Scholar]
- MATLAB R2023a. Available online: https://it.mathworks.com/products/new_products/release2023a.html (accessed on 31 January 2025).
- General Mission Analysis Tool R2022a. Available online: http://gmatcentral.org (accessed on 31 January 2025).
- FreeFlyer University. Available online: https://ai-solutions.com/freeflyer-astrodynamic-software/freeflyer-university/ (accessed on 12 December 2024).
- Qual è la distanza tra la Terra e la Luna. Available online: https://www.focus.it/scienza/spazio/apollo-11-lunar-laser-ranging-misura-la-distanza-tra-la-terra-e-la-luna#:~:text=Questi%20specchi%20 (accessed on 14 February 2025).
- Breakwell, J.V.; Brown, J.V. The ‘halo’family of 3-dimensional periodic orbits in the Earth-Moon restricted 3-body problem. Celest. Mech. 1979, 20, 389–404. [Google Scholar]
- Connor Howell, K. Three-dimensional, periodic, ‘halo’orbits. Celest. Mech. 1984, 32, 53–71. [Google Scholar] [CrossRef]
- Howell, K.C.; Breakwell, J.V. Almost rectilinear halo orbits. Celest. Mech. 1984, 32, 29–52. [Google Scholar] [CrossRef]
- Gao, Z.Y.; Hou, X.Y. Coverage analysis of lunar communication/navigation constellations based on halo orbits and distant retrograde orbits. J. Navig. 2020, 73, 932–952. [Google Scholar] [CrossRef]
- Trofimov, S.; Shirobokov, M.; Tselousova, A.; Ovchinnikov, M. Transfers from near-rectilinear halo orbits to low-perilune orbits and the Moon’s surface. Acta Astronaut. 2020, 167, 260–271. [Google Scholar] [CrossRef]
- Lara, M.; Ferrer, S.; De Saedeleer, B. Lunar analytical theory for polar orbits in a 50-degree zonal model plus third-body effect. J. Astronaut. Sci. 2009, 57, 561–577. [Google Scholar]
- Chang’e 4. Available online: https://en.wikipedia.org/wiki/Chang%27e_4 (accessed on 31 January 2025).
- Lunar South Pole Atlas. Available online: https://www.lpi.usra.edu/lunar/lunar-south-pole-atlas/ (accessed on 31 January 2025).





| Feature | Value |
|---|---|
| Moon mass | 7.3477 × 1022 [kg] |
| Earth mass | 5.97219 × 1024 [kg] |
| µ [24] | 0.0121505856 |
| Moon radius | 1734.4 [km] |
| Earth–Moon average distance (r12) [25] | 385,000.6 [km] |
| Characteristic time (t*) | 4.3651274 [days] |
| Orbit | Period (days) | Sinodic Res. | Sidereal Res. | CTP [%] | Perilune Distance | |||
|---|---|---|---|---|---|---|---|---|
| L1 | 0.9246 | −0.2180 | 0.1232 | 7.8781 | 3.75 | 3.48 | 80.31 | 3905.9 |
| L2 | 1.0694 | −0.20105 | −0.1860 | 9.4966 | 3.11 | 2.88 | 42.32 | 13,798.6 |
| L2 | 1.0634 | −0.2003 | −0.1770 | 9.1155 | 3.24 | 3.00 | 47.22 | 12,005.7 |
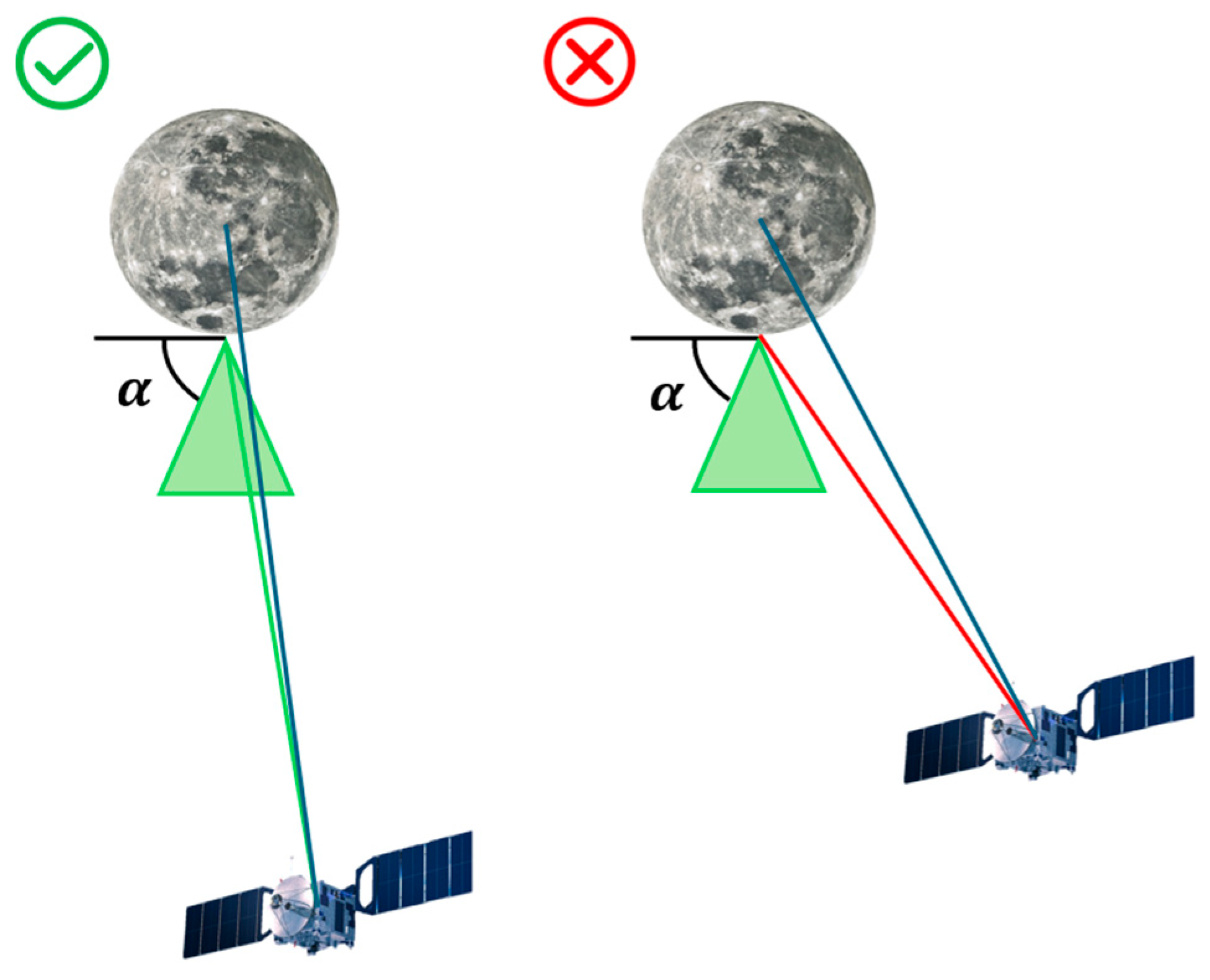
| Antenna Minimum Elevation Angle α [°] | Associated Latitude [°] |
|---|---|
| 15 | −18 |
| 20 | −22 |
| 40 | −42 |
| 60 | −60 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trabacchin, N.; Colombatti, G. Design of an Orbital Infrastructure to Guarantee Continuous Communication to the Lunar South Pole Region. Aerospace 2025, 12, 289. https://doi.org/10.3390/aerospace12040289
Trabacchin N, Colombatti G. Design of an Orbital Infrastructure to Guarantee Continuous Communication to the Lunar South Pole Region. Aerospace. 2025; 12(4):289. https://doi.org/10.3390/aerospace12040289
Chicago/Turabian StyleTrabacchin, Nicolò, and Giacomo Colombatti. 2025. "Design of an Orbital Infrastructure to Guarantee Continuous Communication to the Lunar South Pole Region" Aerospace 12, no. 4: 289. https://doi.org/10.3390/aerospace12040289
APA StyleTrabacchin, N., & Colombatti, G. (2025). Design of an Orbital Infrastructure to Guarantee Continuous Communication to the Lunar South Pole Region. Aerospace, 12(4), 289. https://doi.org/10.3390/aerospace12040289







