A Numerical Investigation on Stress Modal Analysis of Composite Laminated Thin Plates
Abstract
1. Introduction
2. Theoretical Background
- Dynamic stress responses are straightforward related to material’s strength, e.g., in the criteria of vibration fatigue under random loadings [72]; and vibration fatigue failure of aircraft composite materials is frequently stress-induced high-cycle fatigue;
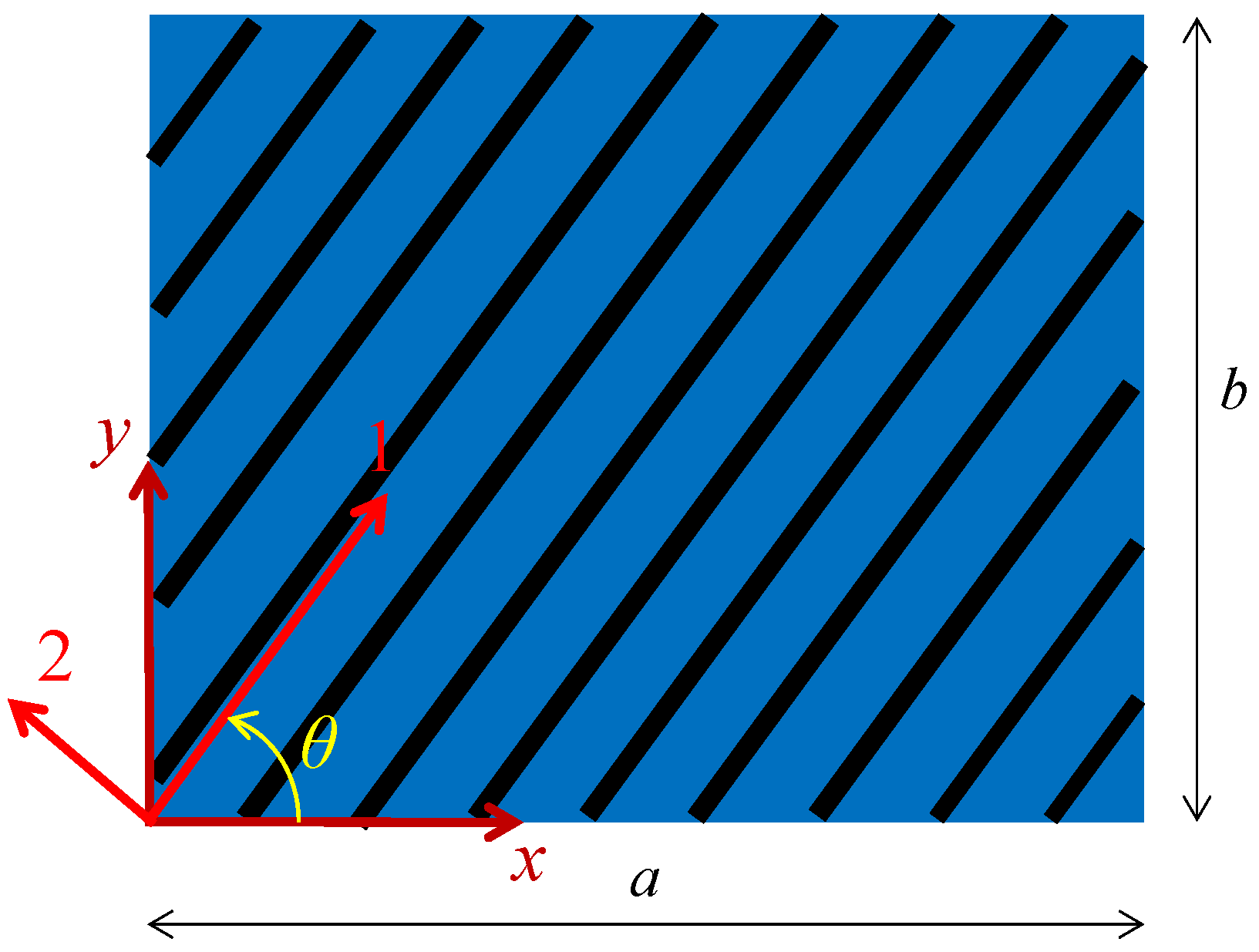
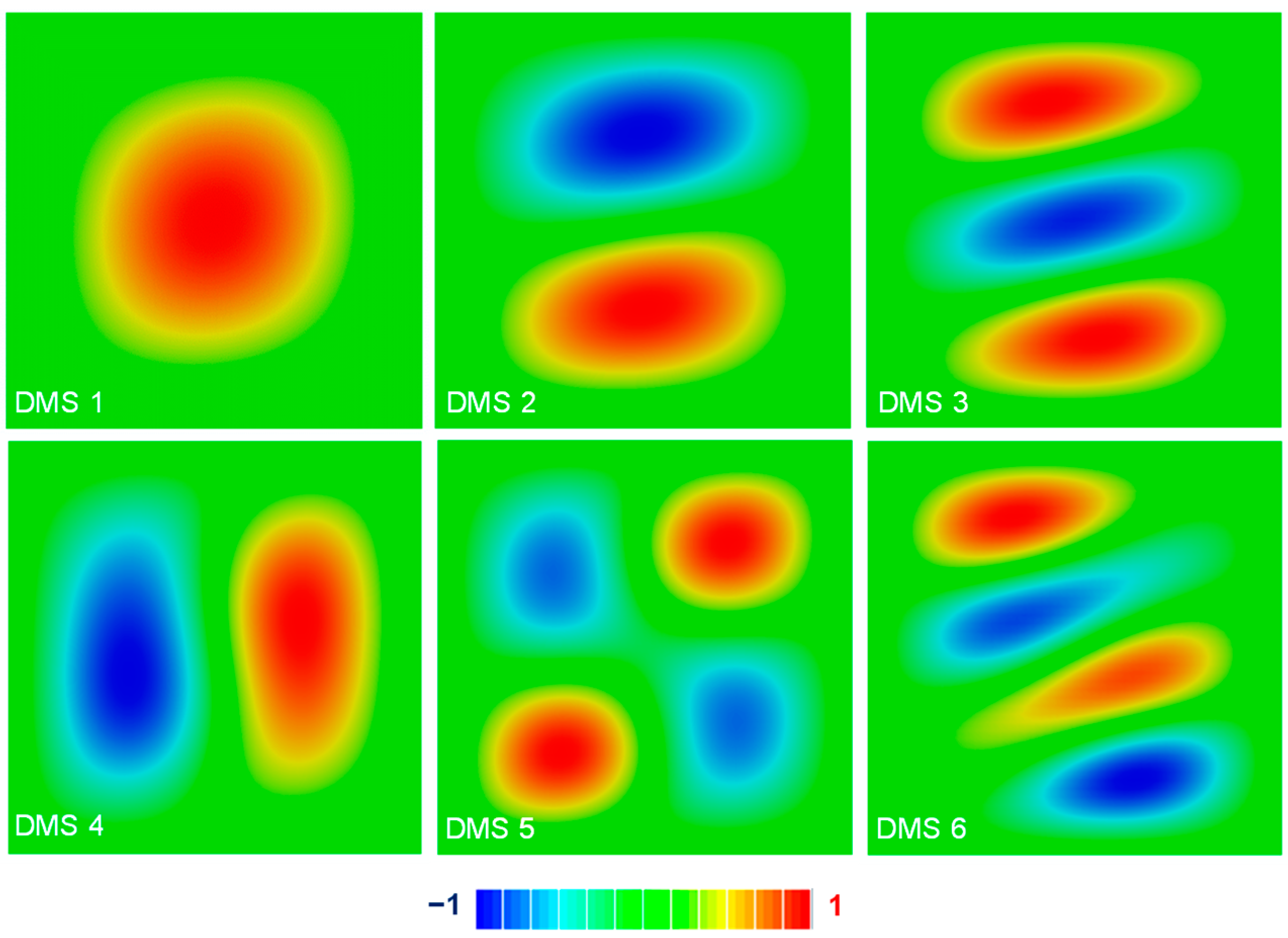
- Although the displacement fields of composite laminates are continuous, the stress distribution of each lamina may experience severe discontinuity due to the severe anisotropy. The SMSs can embody the orientation effect of each lamina, i.e., the same strain along different directions may result in different stresses because of the orthotropic properties;
- The strain modes measured at laminate’s surface in modal testing cannot reflect the internal stress state at a mode shape; while the internal SMSs can be easily captured in computational modal analysis, which will be useful for internal damage assessment for each layer.
- (a)
- The thickness distribution of the plate is uniform.
- (b)
- The material is linear elastic and 2-D orthotropic.
- (c)
- The damping is light, so that it can be neglected.
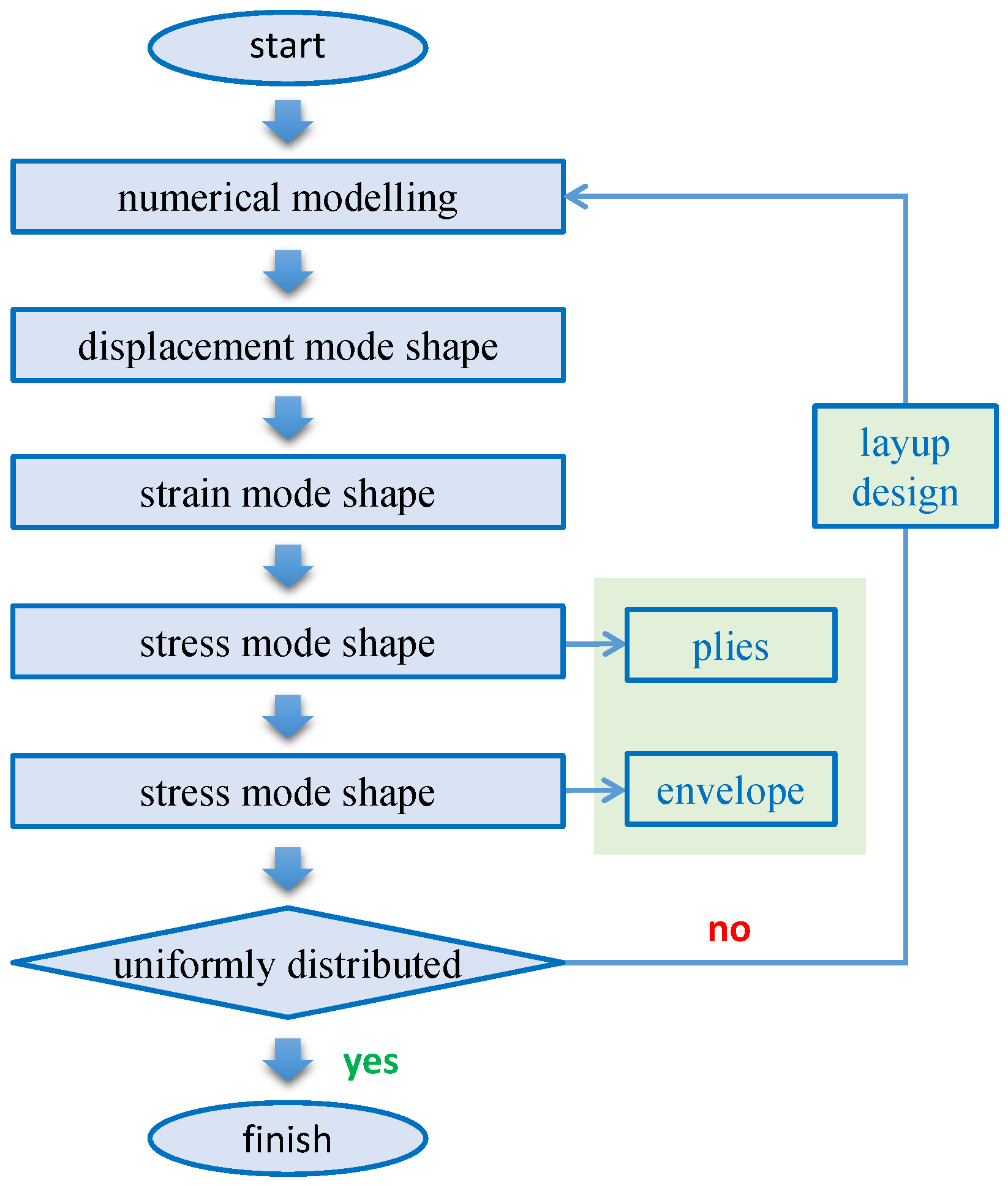
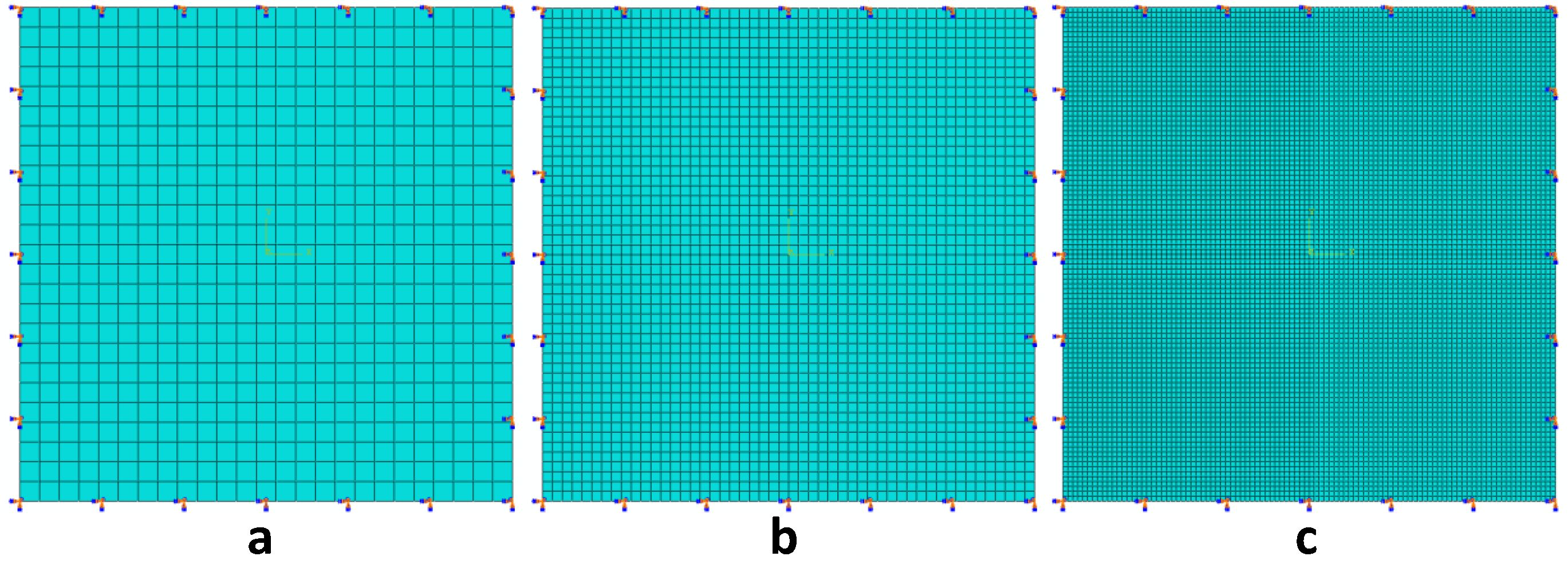
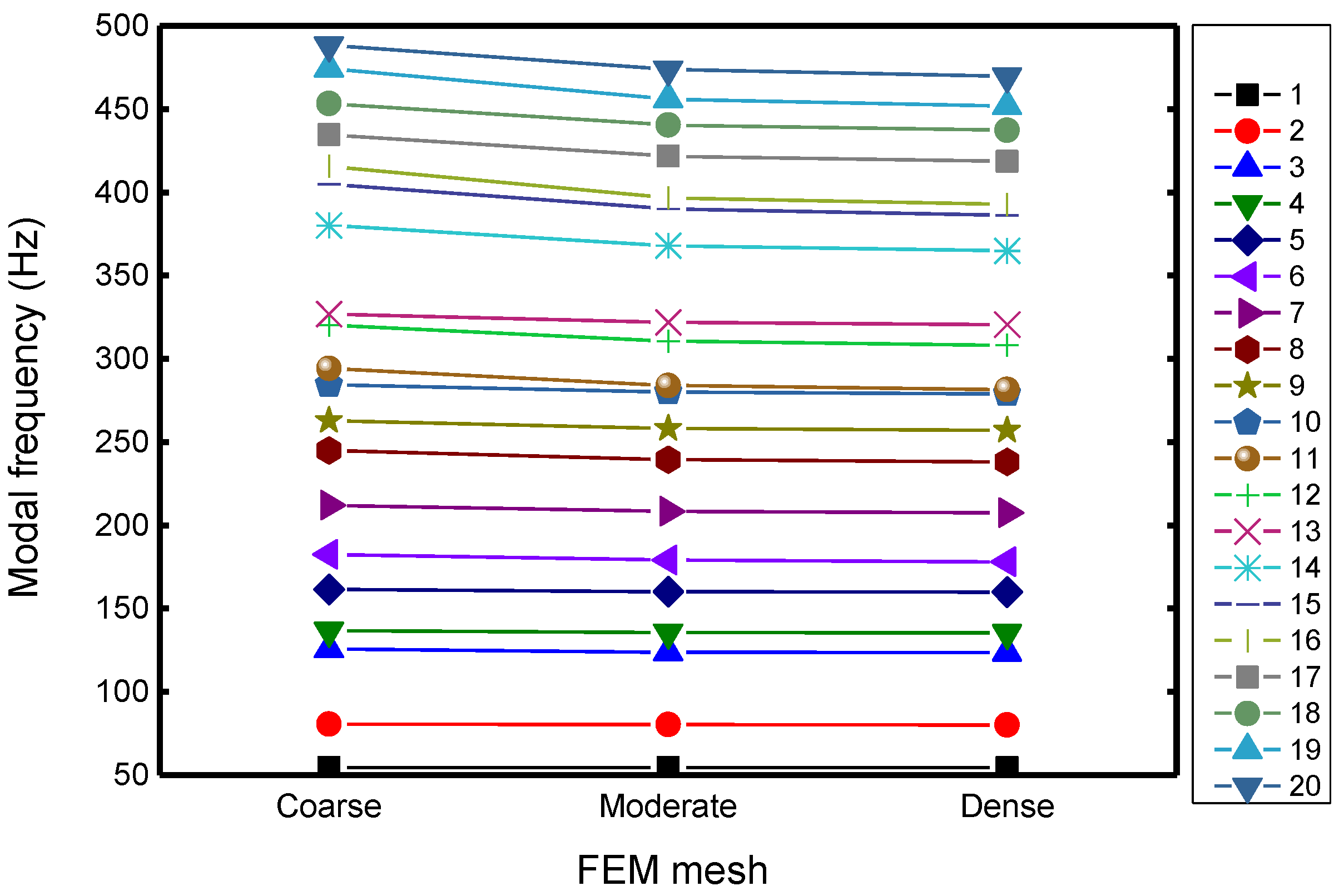
3. FEM Modelling
4. Results and Discussion
- (1)
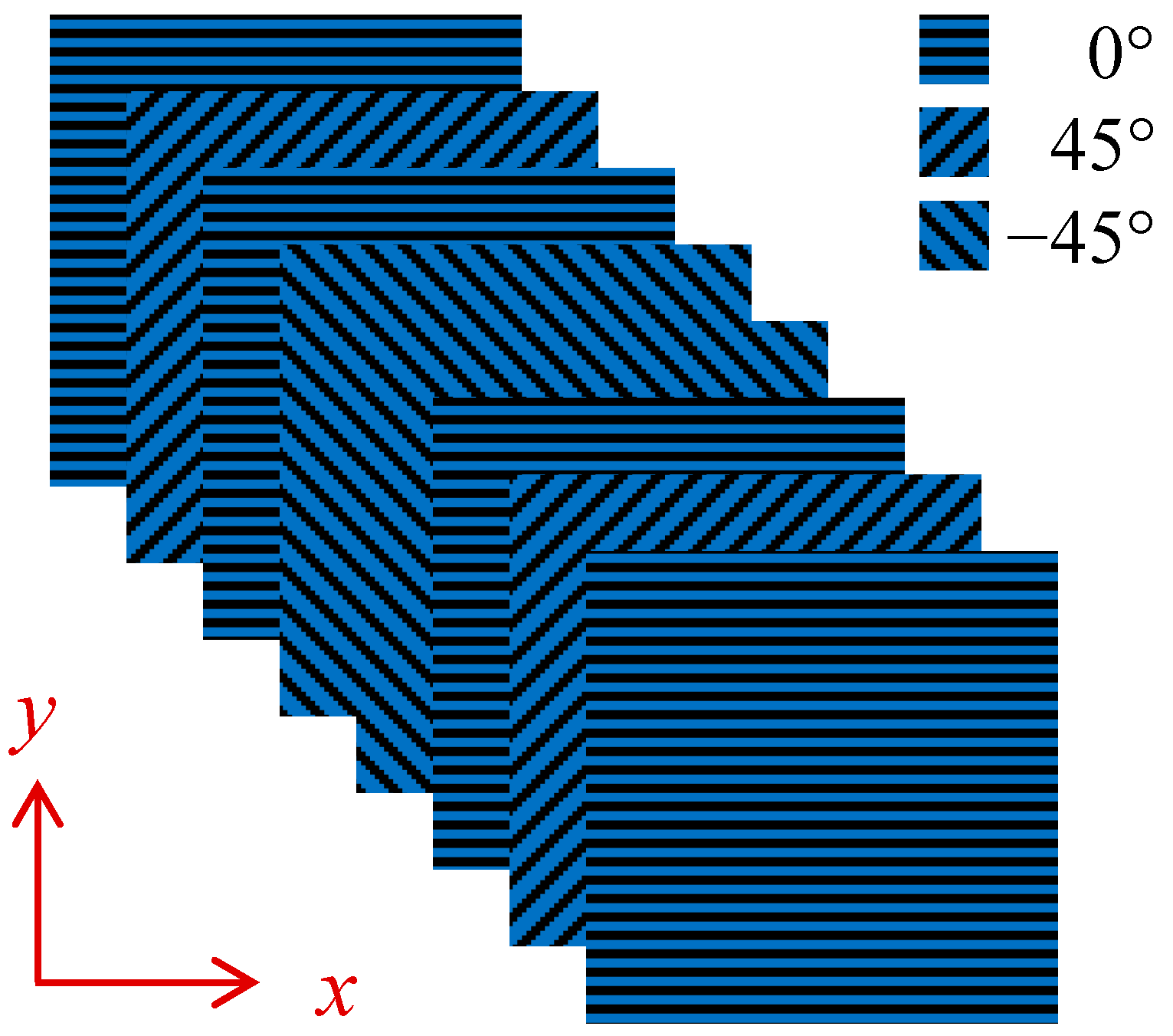
- The stress mode distributions in different laminas will be different due to the orthotropic elastic properties. Because the maximum damage envelope of all layers is critically important in evaluating the strength of laminated plates (e.g., in the failure criteria of damage mechanics); therefore, herein, the envelope SMSs are considered.
- (2)
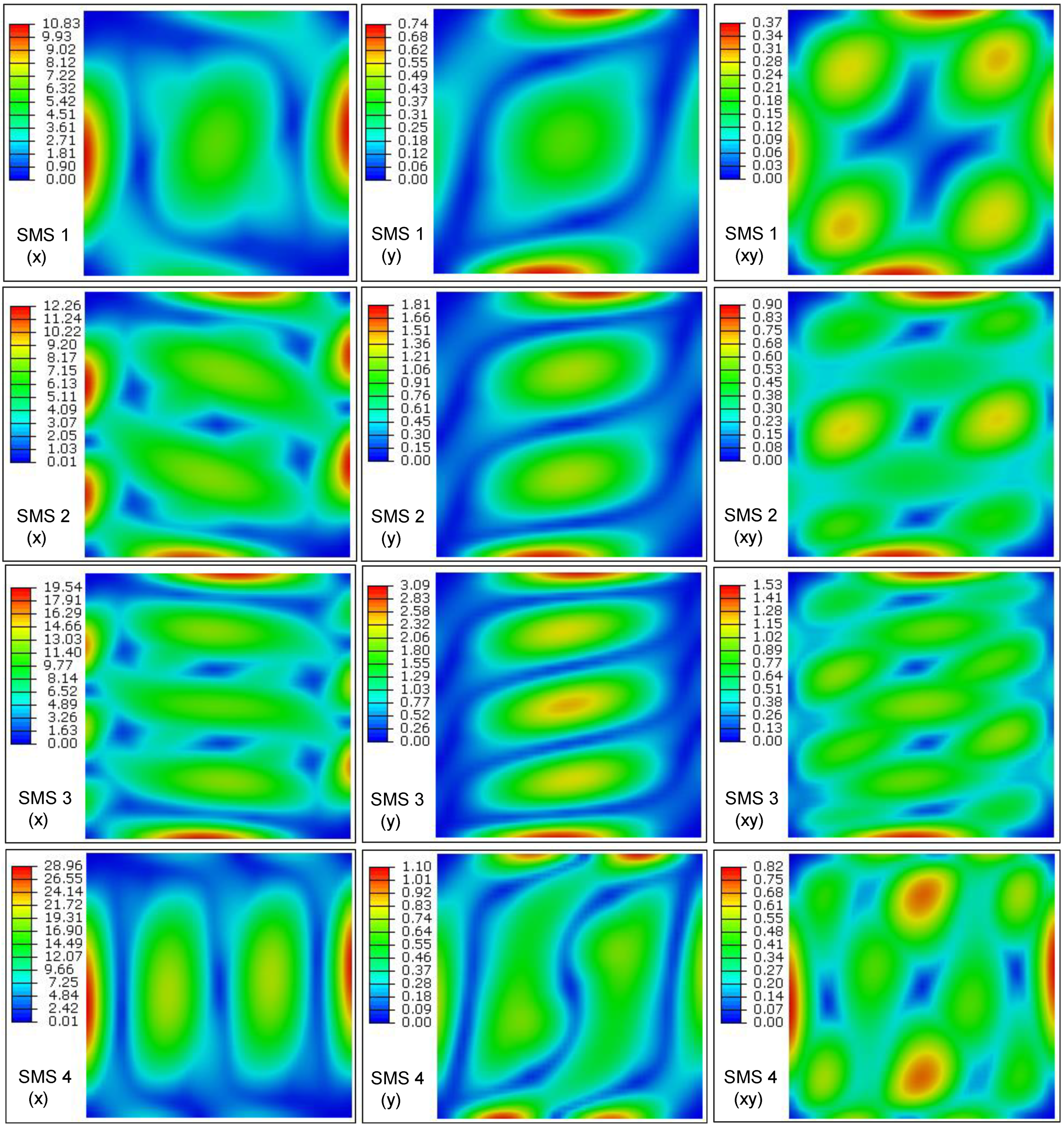
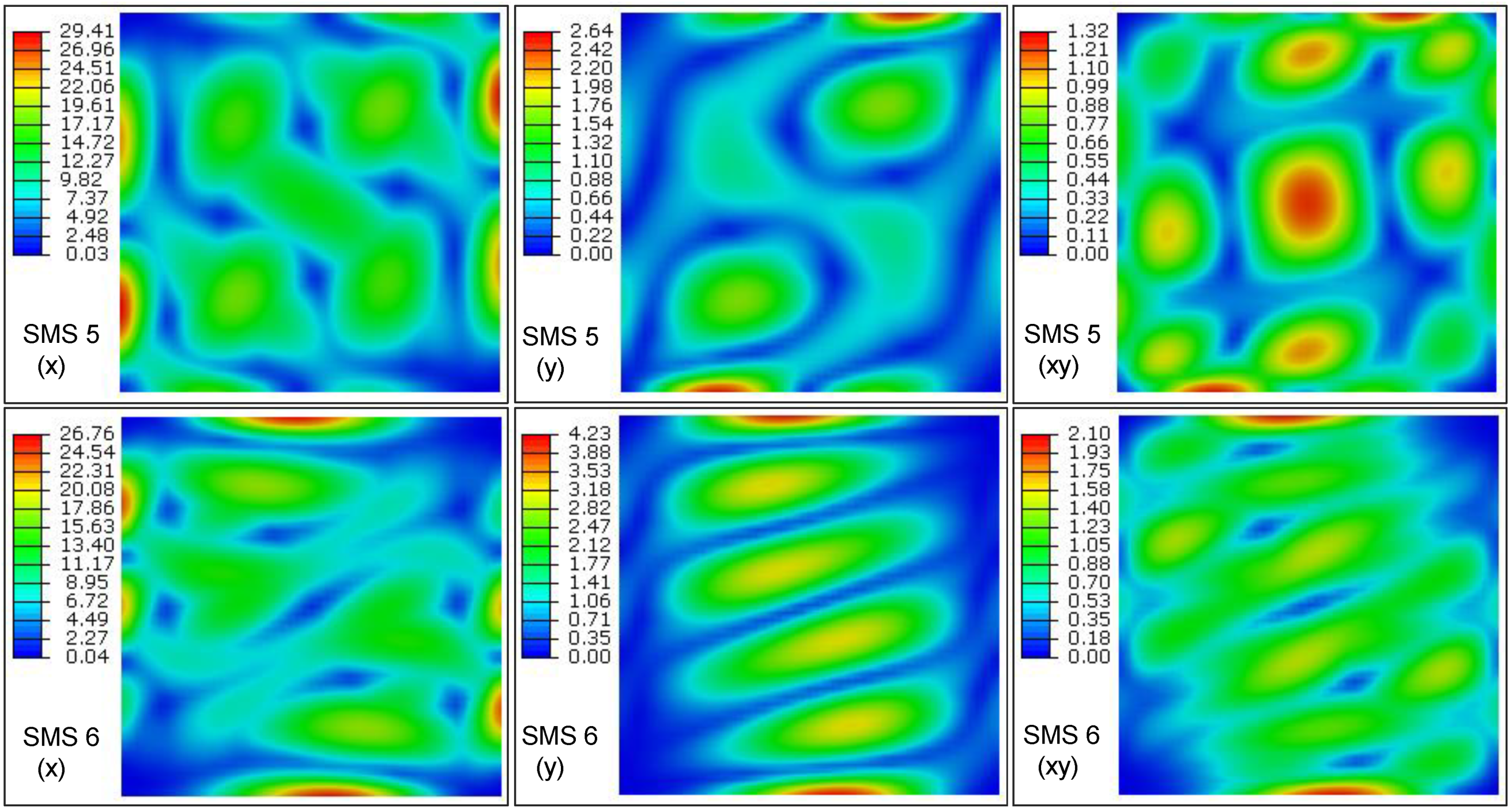
- The SMSs have different distributions with different stress components (e.g., for thin plates along the three important directions) and the invariants. For simplicity but without loss of generality, present study only considers the in-plane stresses, which are the most important stress components in damage criteria of composite laminates. For instance, the Tsai–Hill failure criterion can be written as , for the multi-axial random stress states (plane stress) in composite structures [78].
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| C | elastic matrix |
| D | damping matrix |
| f | exciting force vector |
| j | imaginary unit |
| K | stiffness matrix |
| M | mass matrix |
| H | displacement frequency response function |
| Hσ | stress frequency response function |
| S | compliance matrix |
| x | nodal displacement vector |
| w | out-of-plane deflection |
| ϕ | displacement mode shape |
| Φ | displacement modal matrix |
| ϕσ | stress mode shape |
| Φσ | stress modal matrix |
| ω | modal frequency |
| ε | strain vector |
| σ | stress vector |
| κ | modal curvature |
| FEM | finite element method |
| FRF | frequency response function |
| SFRF | stress frequency response function |
| SMA | stress modal analysis |
| SMSs | stress mode shapes |
References
- Reddy, J.N. Mechanics of Laminated Composite Plates and Shells; CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Kessler, S.S.; Spearing, S.; Atalla, M.J.; Cesnik, C.E.; Soutis, C. Damage detection in composite materials using frequency response methods. Compos. Part B Eng. 2002, 33, 87–95. [Google Scholar] [CrossRef]
- Jayaprakash, K.; Muthukumar, M.; Desai, Y.M.; Naik, N.K. Vibration Induced Fatigue Analysis of [0n/90n]s Simply Supported Composite Plate Under Central Patch Impulse Loading. J. Eng. Mater. Technol. 2015, 137. [Google Scholar] [CrossRef]
- Zhou, S.; Sun, Y.; Guo, L. Random fatigue life prediction of carbon fibre-reinforced composite laminate based on hybrid time-frequency domain method. Adv. Compos. Mater. 2016, 26, 1–15. [Google Scholar] [CrossRef]
- Fan, Z.; Jiang, Y.; Zhang, S.; Chen, X. Experimental Research on Vibration Fatigue of CFRP and Its Influence Factors Based on Vibration Testing. Shock. Vib. 2017, 2017, 1–18. [Google Scholar] [CrossRef]
- Guida, M.; Marulo, F.; Abrate, S. Advances in crash dynamics for aircraft safety. Prog. Aerosp. Sci. 2018, 98, 106–123. [Google Scholar] [CrossRef]
- Zhao, W.; Kapania, R.K. Prestressed Vibration of Stiffened Variable-Angle Tow Laminated Plates. AIAA J. 2019, 57, 2575–2593. [Google Scholar] [CrossRef]
- Wu, Z.; Fang, G.; Fu, M.; Chen, X.; Liang, J.; Lv, D. Random fatigue damage accumulation analysis of composite thin-wall structures based on residual stiffness method. Compos. Struct. 2019, 211, 546–556. [Google Scholar] [CrossRef]
- Guida, M.; Sellitto, A.; Marulo, F.; Riccio, A. Analysis of the Impact Dynamics of Shape Memory Alloy Hybrid Composites for Advanced Applications. Materials 2019, 12, 153. [Google Scholar] [CrossRef]
- Riccio, A.; Saputo, S.; Sellitto, A.; Russo, A.; Di Caprio, F.; Di Palma, L. An Insight on the Crashworthiness Behavior of a Full-Scale Composite Fuselage Section at Different Impact Angles. Aerospace 2019, 6, 72. [Google Scholar] [CrossRef]
- Cawley, P.; Adams, R.D. A Vibration Technique for Non-Destructive Testing of Fibre Composite Structures. J. Compos. Mater. 1979, 13, 161–175. [Google Scholar] [CrossRef]
- Mondal, S.; Patra, A.; Chakraborty, S.; Mitra, N. Dynamic performance of sandwich composite plates with circular hole/cut-out: A mixed experimental–numerical study. Compos. Struct. 2015, 131, 479–489. [Google Scholar] [CrossRef]
- Murugan, R.; Ramesh, R.; Padmanabhan, K. Investigation on Mechanical Behaviour and Vibration Characteristics of Thin Walled Glass/Carbon Hybrid Composite Beams Under Fixed-Free Boundary Condition. Mech. Adv. Mater. Struct. 2016, 23, 909–916. [Google Scholar] [CrossRef]
- Sahoo, S.S.; Panda, S.K.; Sen, D. Effect of Delamination on Static and Dynamic Behavior of Laminated Composite Plate. AIAA J. 2016, 54, 2530–2544. [Google Scholar] [CrossRef]
- Mandal, A.; Ray, C.; Haldar, S. Free vibration analysis of laminated composite skew plates with cut-out. Arch. Appl. Mech. 2017, 87, 1511–1523. [Google Scholar] [CrossRef]
- Chaubey, A.K.; Kumar, A.; Chakrabarti, A. Vibration of Laminated Composite Shells with Cutouts and Concentrated Mass. AIAA J. 2018, 56, 1662–1678. [Google Scholar] [CrossRef]
- Yurddaskal, M.; Ozmen, U.; Kir, M.; Baba, B.O. The effect of foam properties on vibration response of curved sandwich composite panels. Compos. Struct. 2018, 183, 278–285. [Google Scholar] [CrossRef]
- Ewins, D.J. Modal Testing: Theory and Practice; Research Studies Press: Letchworth, UK, 1984. [Google Scholar]
- He, J.; Fu, Z.-F. Modal Analysis; Butterworth-Heinemann: Oxford, UK, 2001. [Google Scholar]
- Kranjc, T.; Slavič, J.; Boltežar, M. A comparison of strain and classic experimental modal analysis. J. Vib. Control. 2016, 22, 371–381. [Google Scholar] [CrossRef]
- Kim, S.; Cho, H.; Joo, H.; Shin, S.; Kwak, J. Equivalent Structural Modeling Using Laminated Composite Shell Analysis for the Nozzle Component of a Launch Vehicle Engine. J. Aerosp. Eng. 2018, 31, 04018078. [Google Scholar] [CrossRef]
- Zhao, W.; Gupta, A.; Regan, C.D.; Miglani, J.; Kapania, R.K.; Seiler, P.J. Component data assisted finite element model updating of composite flying-wing aircraft using multi-level optimization. Aerosp. Sci. Technol. 2019, 95, 105486. [Google Scholar] [CrossRef]
- Vo-Duy, T.; Ho-Huu, V.; Dang-Trung, H.; Nguyen-Thoi, T. A two-step approach for damage detection in laminated composite structures using modal strain energy method and an improved differential evolution algorithm. Compos. Struct. 2016, 147, 42–53. [Google Scholar] [CrossRef]
- Kim, Y.; Cho, H.; Park, S.; Kim, H.; Shin, S. Advanced Structural Analysis Based on Reduced-Order Modeling for Gas Turbine Blade. AIAA J. 2018, 56, 3369–3373. [Google Scholar] [CrossRef]
- Česnik, M.; Slavič, J.; Boltežar, M. Uninterrupted and accelerated vibrational fatigue testing with simultaneous monitoring of the natural frequency and damping. J. Sound Vib. 2012, 331, 5370–5382. [Google Scholar] [CrossRef]
- Anwar, W.; Khan, M.Z.; Israr, A.; Mehmood, S.; Anjum, N.A. Effect of structural dynamic characteristics on fatigue and damage tolerance of aerospace grade composite materials. Aerosp. Sci. Technol. 2017, 64, 39–51. [Google Scholar] [CrossRef]
- Zhou, Y.; Hang, X.; Wu, S.; Fei, Q.; Trisovic, N. Frequency-dependent random fatigue of panel-type structures made of ceramic matrix composites. Acta Mech. Solida Sin. 2017, 30, 165–173. [Google Scholar] [CrossRef]
- Boudjemai, A.; Amri, R.; Mankour, A.; Salem, H.; Bouanane, M.; Boutchicha, D. Modal analysis and testing of hexagonal honeycomb plates used for satellite structural design. Mater. Des. 2012, 35, 266–275. [Google Scholar] [CrossRef]
- Fan, W.; Qiao, P. Vibration-based damage identification methods: A review and comparative study. Struct. Health Monit. 2011, 10, 83–111. [Google Scholar] [CrossRef]
- Rucevskis, S.; Janeliukstis, R.; Akishin, P.; Chate, A. Mode shape-based damage detection in plate structure without baseline data. Struct. Control Health Monit. 2016, 23, 1180–1193. [Google Scholar] [CrossRef]
- Cao, S.; Ouyang, H.; Cheng, L. Baseline-free adaptive damage localization of plate-type structures by using robust PCA and Gaussian smoothing. Mech. Syst. Signal Process. 2019, 122, 232–246. [Google Scholar] [CrossRef]
- Yam, L.Y.; Leung, T.P.; Li, D.B.; Xue, K.Z. Theoretical and experimental study of modal strain analysis. J. Sound Vib. 1996, 191, 251–260. [Google Scholar] [CrossRef]
- Li, Y.Y.; Cheng, L.; Yam, L.H.; Wong, W.O. Identification of damage locations for plate-like structures using damage sensitive indices: Strain modal approach. Comput. Struct. 2002, 80, 1881–1894. [Google Scholar] [CrossRef]
- Lee, E.-T.; Rahmatalla, S.; Eun, H.-C. Damage detection by mixed measurements using accelerometers and strain gages. Smart Mater. Struct. 2013, 22, 075014. [Google Scholar] [CrossRef]
- Shadan, F.; Khoshnoudian, F.; Esfandiari, A. Structural Damage Identification Based on Strain Frequency Response Functions. Int. J. Struct. Stab. Dyn. 2018, 18, 1850159. [Google Scholar] [CrossRef]
- Su, W.; Cesnik, C.E.S. Strain-Based Analysis for Geometrically Nonlinear Beams: A Modal Approach. J. Aircr. 2014, 51, 890–903. [Google Scholar] [CrossRef][Green Version]
- Skafte, A.; Aenlle, M.L.; Brincker, R. A general procedure for estimating dynamic displacements using strain measurements and operational modal analysis. Smart Mater. Struct. 2016, 25, 025020. [Google Scholar] [CrossRef]
- Wang, Z.-C.; Geng, D.; Ren, W.-X.; Liu, H.-T. Strain modes based dynamic displacement estimation of beam structures with strain sensors. Smart Mater. Struct. 2014, 23, 125045. [Google Scholar] [CrossRef]
- Česnik, M.; Slavič, J.; Čermelj, P.; Boltežar, M. Frequency-based structural modification for the case of base excitation. J. Sound Vib. 2013, 332, 5029–5039. [Google Scholar] [CrossRef]
- Xie, C.; Xue, P. An accurate and efficient computational method for structural dynamic stresses under random loading. Aerosp. Sci. Technol. 2016, 59, 11–17. [Google Scholar] [CrossRef]
- Braccesi, C.; Cianetti, F.; Tomassini, L. Fast evaluation of stress state spectral moments. Int. J. Mech. Sci. 2017, 127, 4–9. [Google Scholar] [CrossRef]
- Braccesi, C.; Cianetti, F.; Tomassini, L. An innovative modal approach for frequency domain stress recovery and fatigue damage evaluation. Int. J. Fatigue 2016, 91, 382–396. [Google Scholar] [CrossRef]
- Mršnik, M.; Slavič, J.; Boltežar, M. Vibration fatigue using modal decomposition. Mech. Syst. Signal Process. 2018, 98, 548–556. [Google Scholar] [CrossRef]
- Li, F.; Wu, H.; Wu, P. Vibration fatigue dynamic stress simulation under non-stationary state. Mech. Syst. Signal Process. 2021, 146, 107006. [Google Scholar] [CrossRef]
- Zhou, Y.; Fei, Q.; Wu, S. Utilization of modal stress approach in random-vibration fatigue evaluation. In Proceedings of the Institution of Mechanical Engineers, Part G. J. Aerosp. Eng. 2017, 213, 2603–2615. [Google Scholar]
- Zhou, Y.; Tao, J. Theoretical and numerical investigation of stress mode shapes in multi-axial random fatigue. Mech. Syst. Signal Process. 2019, 127, 499–512. [Google Scholar] [CrossRef]
- Zhou, Y. Local Finite Element Refinement for Accurate Dynamic Stress via Modal Information Only. AIAA J. 2020, 58, 3593–3606. [Google Scholar] [CrossRef]
- Lestari, W.; Qiao, P.; Hanagud, S. Curvature Mode Shape-based Damage Assessment of Carbon/Epoxy Composite Beams. J. Intell. Mater. Syst. Struct. 2006, 18, 189–208. [Google Scholar] [CrossRef]
- Qiao, P.; Lu, K.; Lestari, W.; Wang, J. Curvature mode shape-based damage detection in composite laminated plates. Compos. Struct. 2007, 80, 409–428. [Google Scholar] [CrossRef]
- Frieden, J.; Cugnoni, J.; Botsis, J.; Gmür, T.; Coric, D. High-speed internal strain measurements in composite structures under dynamic load using embedded FBG sensors. Compos. Struct. 2010, 92, 1905–1912. [Google Scholar] [CrossRef]
- Shariyat, M.; Alipour, M.M. Differential transform vibration and modal stress analyses of circular plates made of two-directional functionally graded materials resting on elastic foundations. Arch. Appl. Mech. 2010, 81, 1289–1306. [Google Scholar] [CrossRef]
- Dos Santos, F.L.M.; Peeters, B.; Lau, J.; Desmet, W.; Góes, L.C.S. The use of strain gauges in vibration-based damage detection. J. Phys. Conf. Ser. 2015, 628, 012119. [Google Scholar] [CrossRef]
- Yang, Z.-B.; Radzieński, M.; Kudela, P.; Ostachowicz, W. Two-dimensional modal curvature estimation via Fourier spectral method for damage detection. Compos. Struct. 2016, 148, 155–167. [Google Scholar] [CrossRef]
- Ooijevaar, T.H.; Warnet, L.L.; Loendersloot, R.; Akkerman, R.; Tinga, T. Impact damage identification in composite skin-stiffener structures based on modal curvatures. Struct. Control Health Monit. 2015, 23, 198–217. [Google Scholar] [CrossRef]
- Loutas, T.; Bourikas, A. Strain sensors optimal placement for vibration-based structural health monitoring. The effect of damage on the initially optimal configuration. J. Sound Vib. 2017, 410, 217–230. [Google Scholar] [CrossRef]
- Zhen, W.; Wanji, C. Free and Forced Vibration of Laminated Composite Beams. AIAA J. 2018, 56, 2877–2886. [Google Scholar] [CrossRef]
- Esposito, M.; Gherlone, M. Composite wing box deformed-shape reconstruction based on measured strains: Optimization and comparison of existing approaches. Aerosp. Sci. Technol. 2020, 99, 105758. [Google Scholar] [CrossRef]
- Müzel, S.D.; Bonhin, E.P.; Guimarães, N.M.; Guidi, E.S. Application of the Finite Element Method in the Analysis of Composite Materials: A Review. Polymers 2020, 12, 818. [Google Scholar] [CrossRef] [PubMed]
- Rezaiee-Pajand, M.; Arabi, E.; Masoodi, A.R. Nonlinear analysis of FG-sandwich plates and shells. Aerosp. Sci. Technol. 2019, 87, 178–189. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Masoodi, A.R. Hygro-thermo-elastic nonlinear analysis of functionally graded porous composite thin and moderately thick shallow panels. Mech. Adv. Mater. Struct. 2020, 1–19. [Google Scholar] [CrossRef]
- Srinivas, S. A refined analysis of composite laminates. J. Sound Vib. 1973, 30, 495–507. [Google Scholar] [CrossRef]
- Messina, A. Three-Dimensional Free Vibration Analysis of Cross-Ply Laminated Rectangular Plates through 2D and Exact Models. Mech. Adv. Mater. Struct. 2012, 19, 250–264. [Google Scholar] [CrossRef]
- Grover, N.; Singh, B.; Maiti, D. Analytical and finite element modeling of laminated composite and sandwich plates: An assessment of a new shear deformation theory for free vibration response. Int. J. Mech. Sci. 2013, 67, 89–99. [Google Scholar] [CrossRef]
- Sayyad, A.S.; Ghugal, Y.M. On the free vibration analysis of laminated composite and sandwich plates: A review of recent literature with some numerical results. Compos. Struct. 2015, 129, 177–201. [Google Scholar] [CrossRef]
- Sayyad, A.S.; Ghugal, Y.M. On the free vibration of angle-ply laminated composite and soft core sandwich plates. J. Sandw. Struct. Mater. 2017, 19, 679–711. [Google Scholar] [CrossRef]
- Vidal, P.; Gallimard, L.; Polit, O. Free vibration analysis of composite plates based on a variable separation method. Compos. Struct. 2019, 230, 111493. [Google Scholar] [CrossRef]
- Tiwari, P.; Barman, S.K.; Maiti, D.K.; Maity, D. Free Vibration Analysis of Delaminated Composite Plate Using 3D Degenerated Element. J. Aerosp. Eng. 2019, 32, 04019070. [Google Scholar] [CrossRef]
- Almitani, K.H.; Abdelrahman, A.A.; Eltaher, M.A. Influence of the perforation configuration on dynamic behaviors of multilayered beam structure. Structures 2020, 28, 1413–1426. [Google Scholar] [CrossRef]
- Li, D.; Wang, Z.; Zhang, C. A multi-level and multi-site mesh refinement method for the 2D problems with microstructures. Mech. Adv. Mater. Struct. 2019, 1–18. [Google Scholar] [CrossRef]
- Melo, J.D.D.; Bi, J.; Tsai, S.W. A novel invariant-based design approach to carbon fiber reinforced laminates. Compos. Struct. 2017, 159, 44–52. [Google Scholar] [CrossRef]
- Carrera, E.; Fiordilino, G.; Nagaraj, M.; Pagani, A.; Montemurro, M. A global/local approach based on CUF for the accurate and efficient analysis of metallic and composite structures. Eng. Struct. 2019, 188, 188–201. [Google Scholar] [CrossRef]
- Benasciutti, D.; Tovo, R. Frequency-based analysis of random fatigue loads: Models, hypotheses, reality. Mater. Werkst. 2018, 49, 345–367. [Google Scholar] [CrossRef]
- Cornwell, P.; Doebling, S.; Farrar, C. Application of the strain energy damage detection method to plate-like structures. J. Sound Vib. 1999, 224, 359–374. [Google Scholar] [CrossRef]
- Fan, W.; Qiao, P. A strain energy-based damage severity correction factor method for damage identification in plate-type structures. Mech. Syst. Signal Process. 2012, 28, 660–678. [Google Scholar] [CrossRef]
- Xu, W.; Cao, M.; Ostachowicz, W.; Radzieński, M.; Xia, N. Two-dimensional curvature mode shape method based on wavelets and Teager energy for damage detection in plates. J. Sound Vib. 2015, 347, 266–278. [Google Scholar] [CrossRef]
- Hu, H.; Wang, B.-T.; Lee, C.-H.; Su, J.-S. Damage detection of surface cracks in composite laminates using modal analysis and strain energy method. Compos. Struct. 2006, 74, 399–405. [Google Scholar] [CrossRef]
- Zhou, Y.; Sun, Y.; Huang, T. SPH-FEM Design of Laminated Plies under Bird-Strike Impact. Aerospace 2019, 6, 112. [Google Scholar] [CrossRef]
- Wijker, J.J. Random Vibrations in Spacecraft Structures Design: Theory and Applications; Gladwell, G.M.L., Ed.; Springer: London, UK, 2009. [Google Scholar]
- Preumont, A. Twelve Lectures on Structural Dynamics; Gladwell, G.M.L., Ed.; Springer: London, UK, 2013. [Google Scholar]
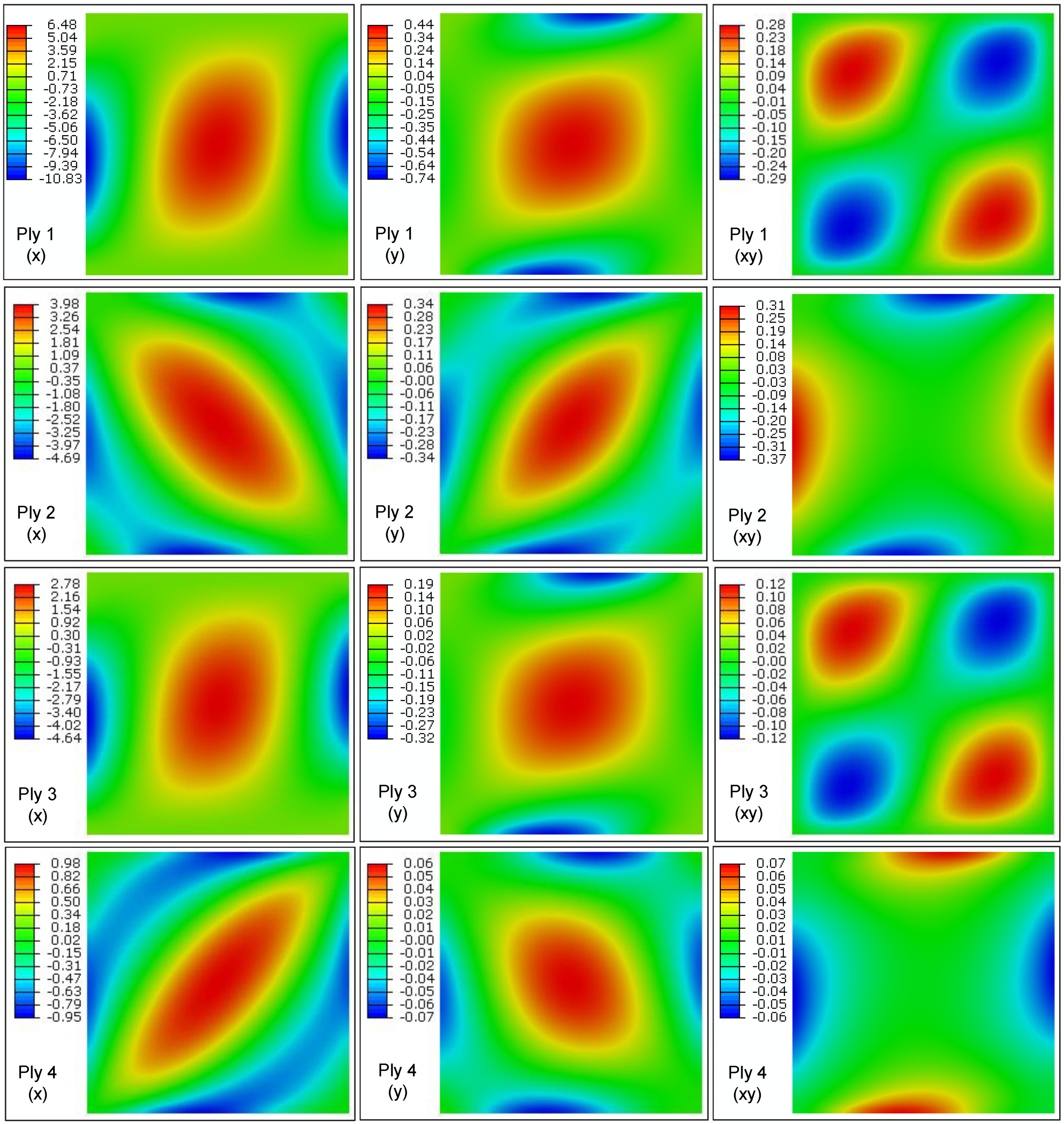
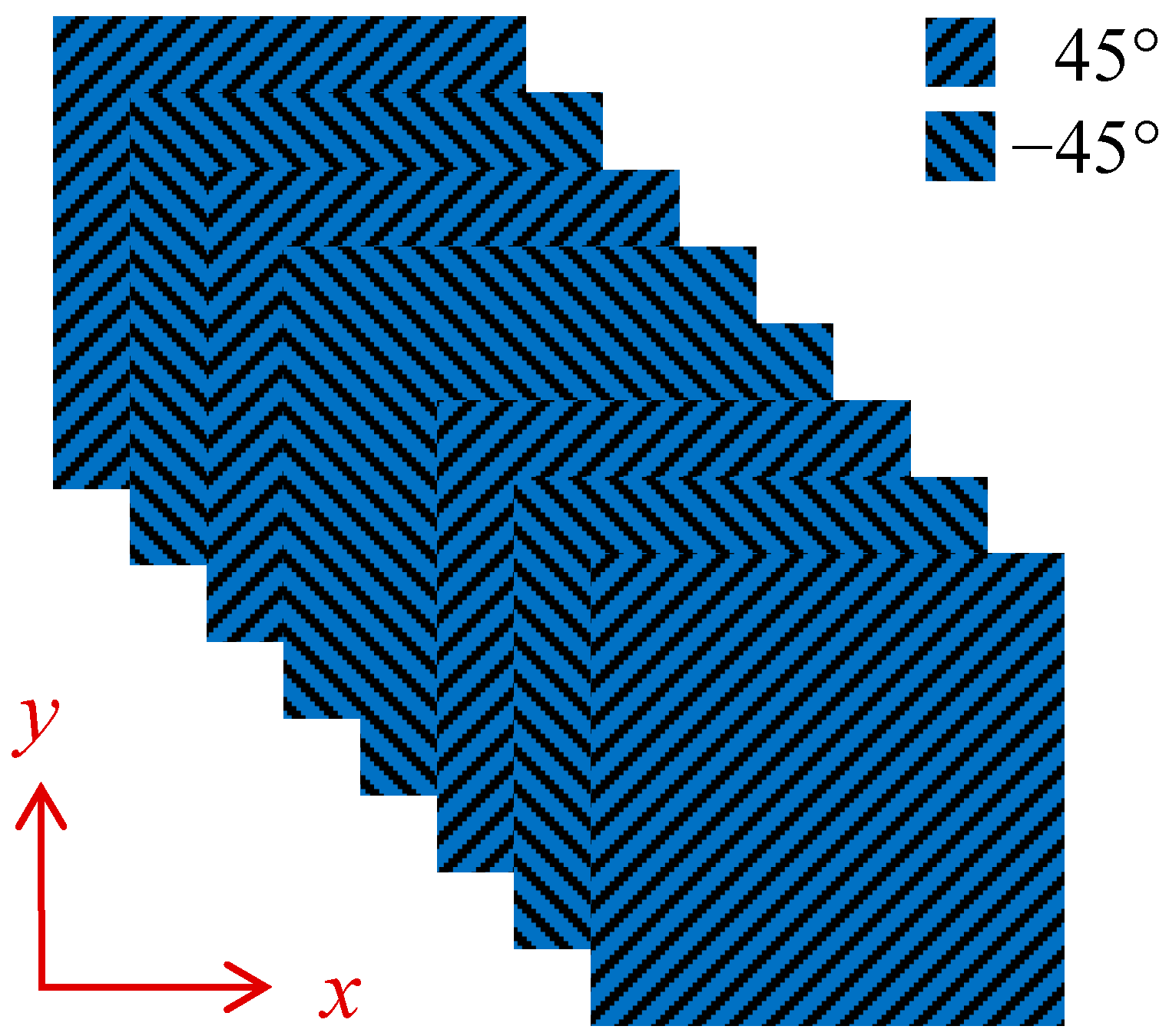
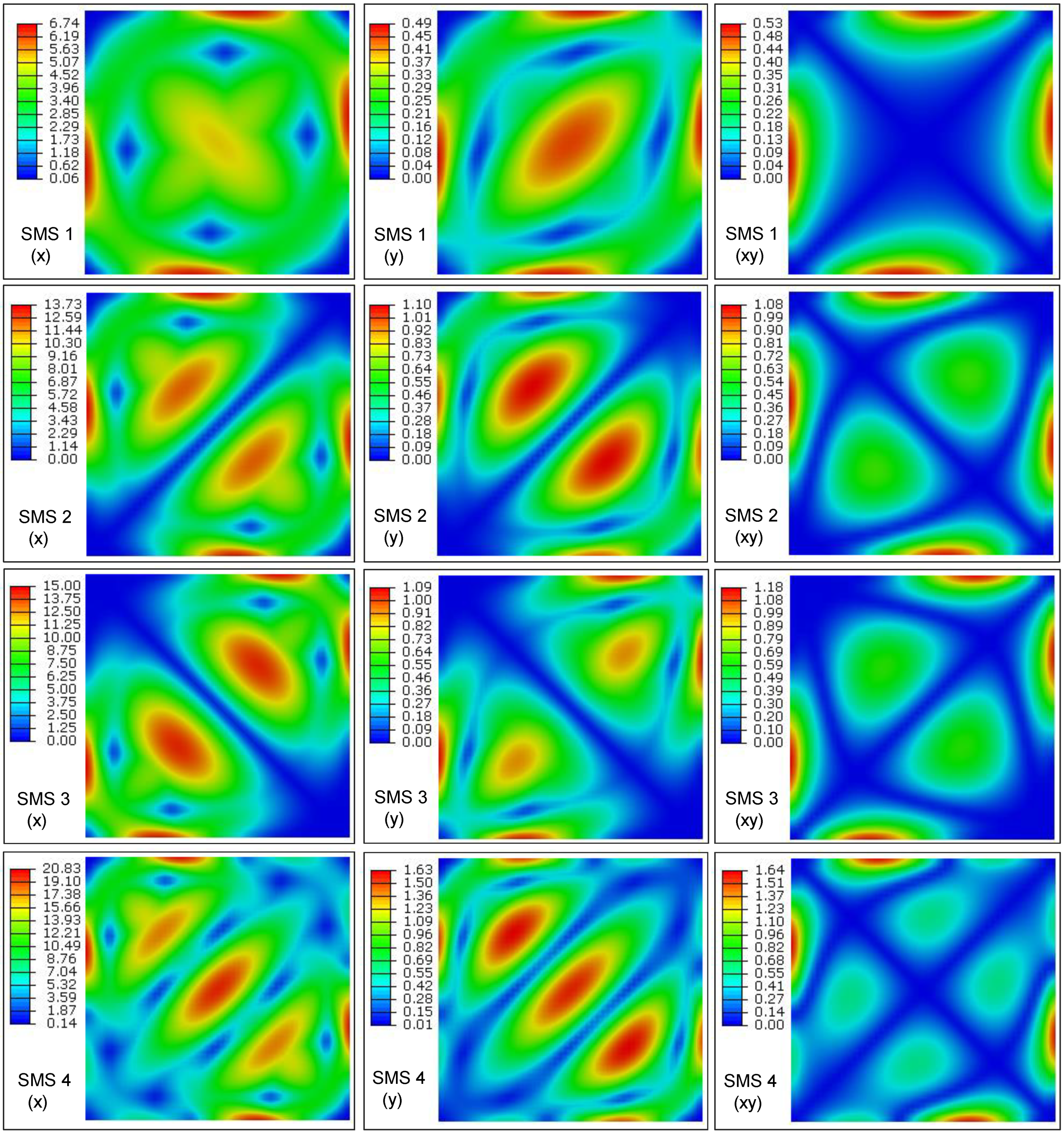

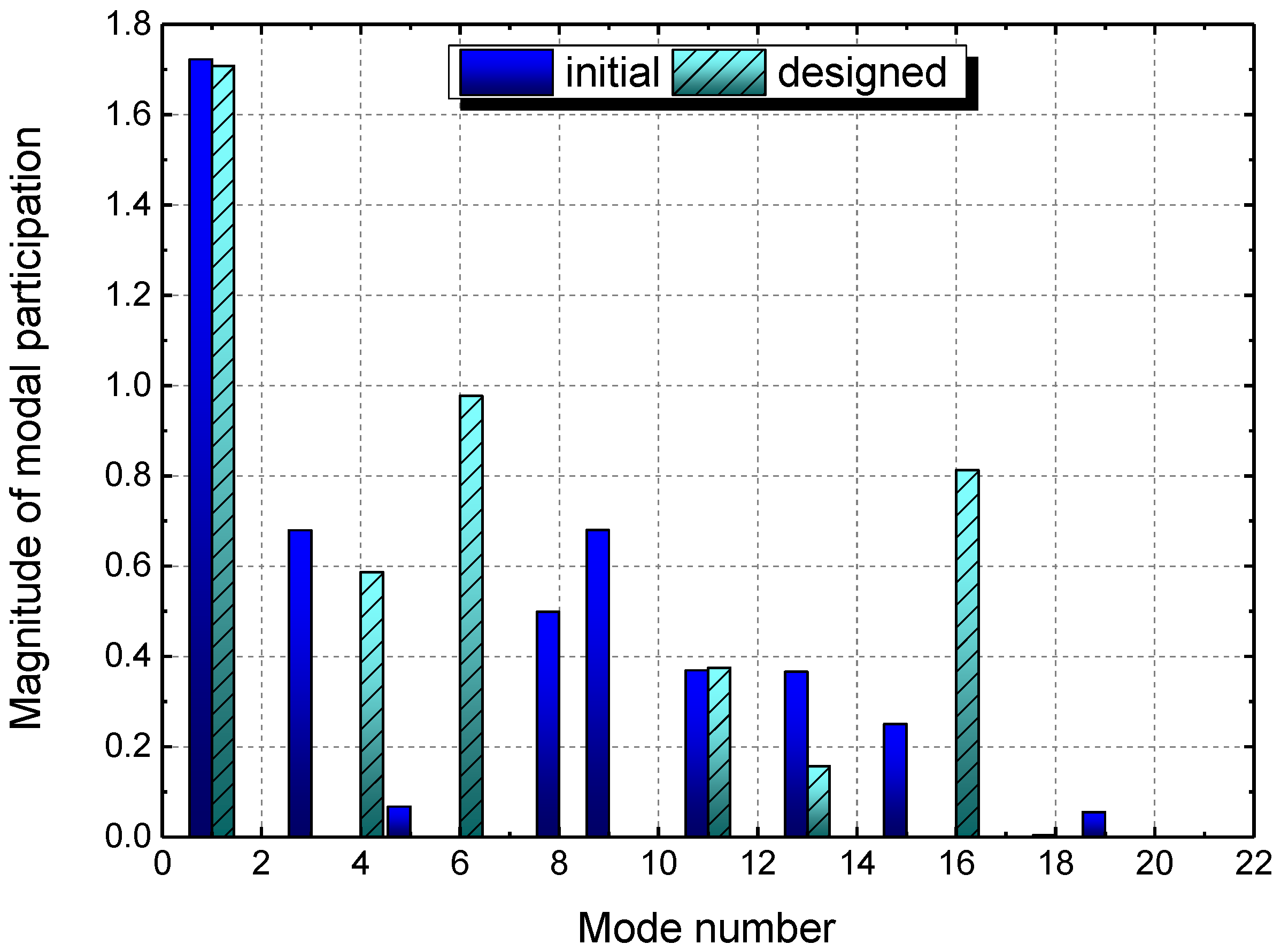
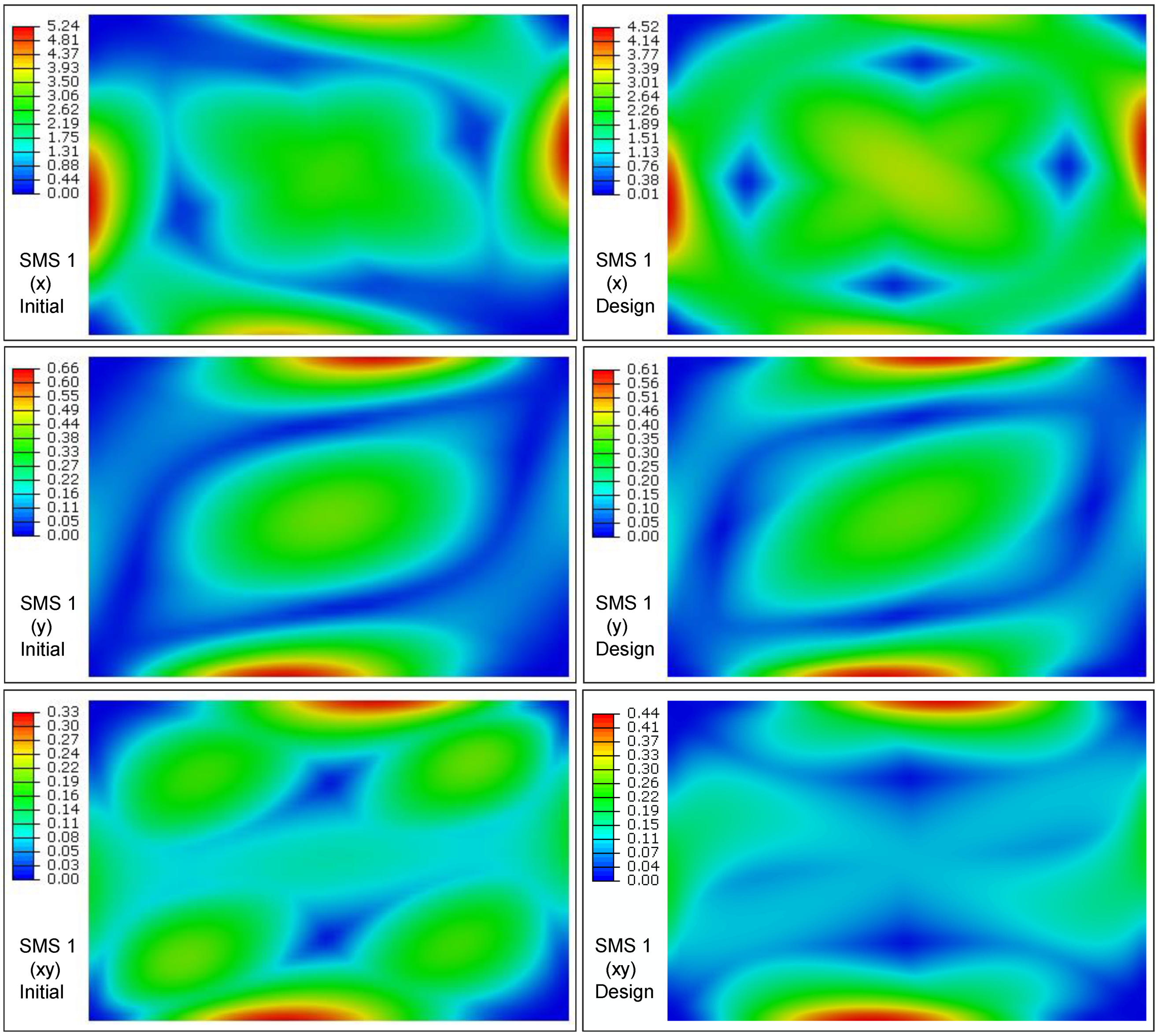
| Property/Notation | Value |
|---|---|
| Longitudinal modulus E11 (GPa) | 181 |
| Transverse modulus E22 (GPa) | 10.3 |
| Shear modulus G12 (GPa) | 7.17 |
| Poisson’s ratio ν12 | 0.28 |
| Mass density ρ (kg/m3) | 1600 |
| Length a = b (mm) | 500 |
| Thickness h (mm) | 1.2 |
| Mode Number | Maximum Magnitude of the Stress Mode | |||||
|---|---|---|---|---|---|---|
| Initial Layup | Designed Layup | |||||
| x | y | xy | x | y | xy | |
| 1 | 10.83 | 0.74 | 0.37 | 6.74 | 0.49 | 0.53 |
| 2 | 12.26 | 1.82 | 0.90 | 13.73 | 1.10 | 1.08 |
| 3 | 19.54 | 3.09 | 1.53 | 15.00 | 1.09 | 1.18 |
| 4 | 28.96 | 1.10 | 0.82 | 20.83 | 1.63 | 1.64 |
| 5 | 29.41 | 2.64 | 1.32 | 26.61 | 1.94 | 2.10 |
| 6 | 26.76 | 4.24 | 2.11 | 29.86 | 1.83 | 1.98 |
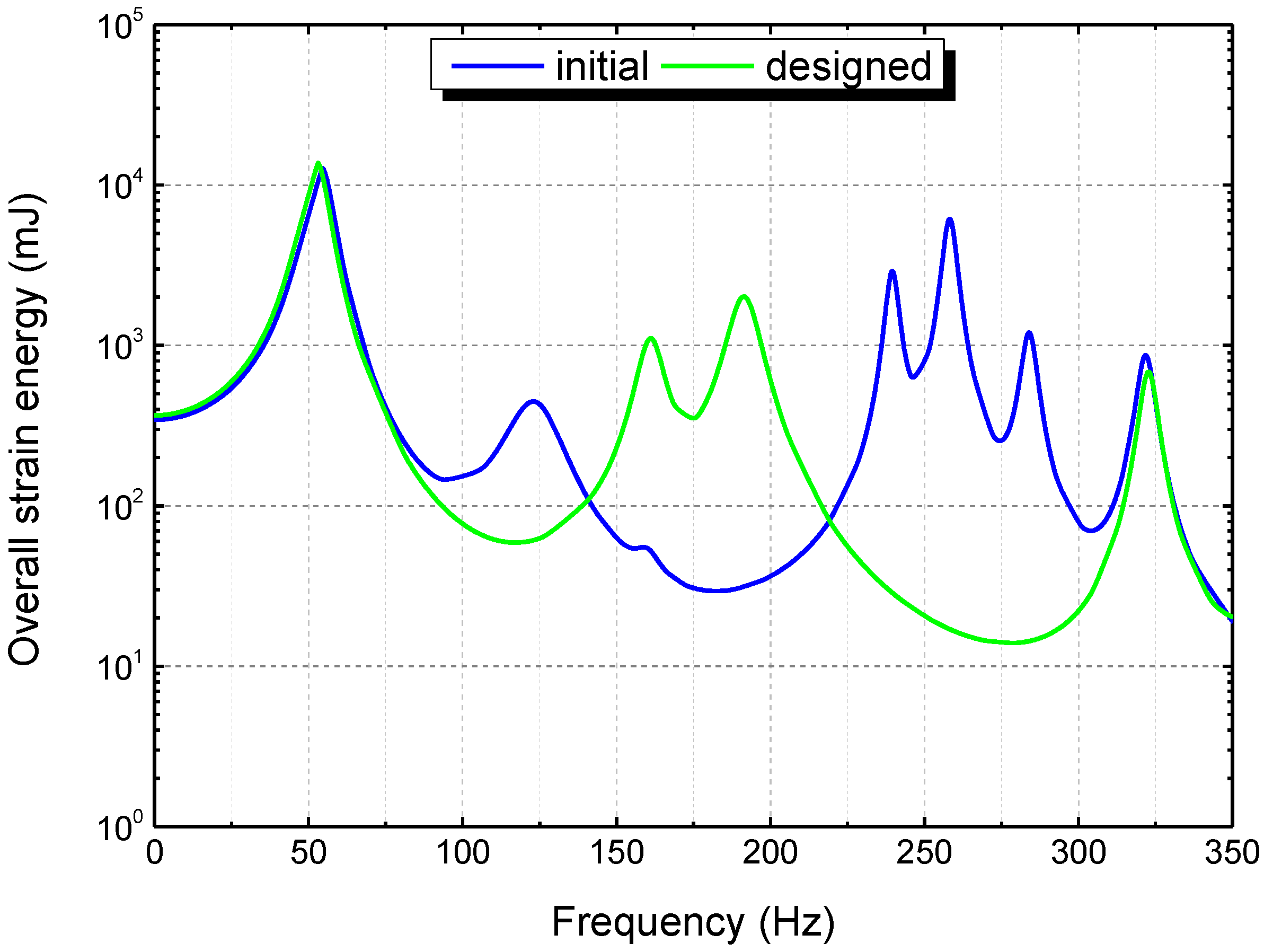
| Mode Number | Modal Frequency (Hz) | ||
|---|---|---|---|
| Initial Layup | Designed Layup | Percent of Change (%) | |
| 1 | 54.4 | 52.9 | −2.7 |
| 2 | 80.2 | 101.4 | 26.5 |
| 3 | 123.8 | 111.2 | −10.2 |
| 4 | 135.6 | 161.2 | 18.9 |
| 5 | 160.1 | 183.5 | 14.6 |
| 6 | 179.0 | 191.5 | 7.0 |
| 7 | 208.4 | 235.1 | 12.8 |
| 8 | 239.6 | 259.6 | 8.4 |
| 9 | 258.2 | 289.5 | 12.1 |
| 10 | 280.1 | 295.6 | 5.5 |
| 11 | 284.0 | 322.8 | 13.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Y.; Sun, Y.; Zeng, W. A Numerical Investigation on Stress Modal Analysis of Composite Laminated Thin Plates. Aerospace 2021, 8, 63. https://doi.org/10.3390/aerospace8030063
Zhou Y, Sun Y, Zeng W. A Numerical Investigation on Stress Modal Analysis of Composite Laminated Thin Plates. Aerospace. 2021; 8(3):63. https://doi.org/10.3390/aerospace8030063
Chicago/Turabian StyleZhou, Yadong, Youchao Sun, and Weili Zeng. 2021. "A Numerical Investigation on Stress Modal Analysis of Composite Laminated Thin Plates" Aerospace 8, no. 3: 63. https://doi.org/10.3390/aerospace8030063
APA StyleZhou, Y., Sun, Y., & Zeng, W. (2021). A Numerical Investigation on Stress Modal Analysis of Composite Laminated Thin Plates. Aerospace, 8(3), 63. https://doi.org/10.3390/aerospace8030063








