Thermochemistry of Combustion in Polyvinyl Alcohol + Hydroxylammonium Nitrate
Abstract
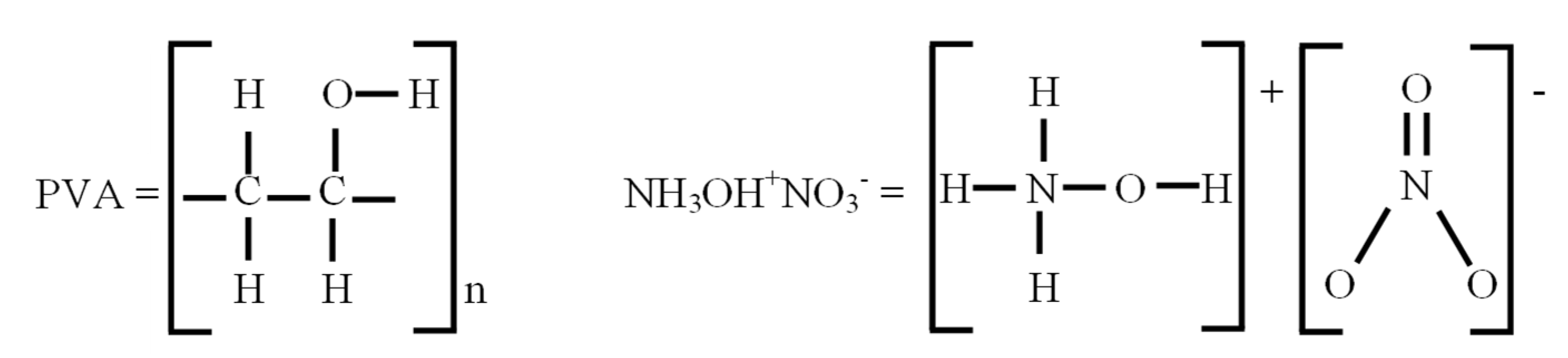
1. Introduction
- (1)
- Upon application of the external voltage, the combustion in PVA + HAN is confined to the electrode surfaces. This observation, plus the fact that HAN is an ionic conductor, strongly suggests that the reactions involved at the electrodes are electrolytic in nature.
- (2)
- If the anode and cathode have the same area, combustion occurs preferentially at the anode. This observation suggests that the oxidizing agent supporting combustion at the anode is stronger than the oxidizing agent supporting combustion at the cathode.
- (3)
- Nonetheless, if the cathode is smaller than the anode, combustion is also observed at the cathode. This suggests a local electric field effect. In particular, the theory of electrostatics predicts that the strength of the electric field in the vicinity of a charged metal electrode increases as the size of the electrode decreases, in which case the value of the field in the vicinity of the electrode may exceed the electric breakdown strength of the plastisol, causing an electric discharge, which enhances the combustion.
- (4)
- The PVA + HAN plastisol fails to ignite in air when exposed to an open flame. This observation suggests that the oxidizing agents supporting combustion at the anode and at the cathode are stronger than molecular oxygen.
- (5)
- The products of PVA + HAN are known to include the molecular gases, carbon monoxide, carbon dioxide, water, hydrogen, and nitrogen. The first two gases necessarily have their origin in PVA. Water and hydrogen can come from either PVA or HAN, whereas nitrogen can have its origin only in HAN.
2. Case A: A Low Energy Scenario Where the Product Distribution Is Rich in Molecular Hydrogen
2.1. Chemistry of the Overall Reaction
2.2. Anodic Oxygen + Cathodic Hydroxyl Radical Mechanism
2.2.1. Electrolysis Reaction
2.2.2. Combustion at the Anode Produces
2.2.3. Combustion at the Cathode Produces
2.2.4. Overall Reaction Heat
2.3. The Anodic Ozone + Cathodic Hydrogen Peroxide Mechanism
2.3.1. Electrolysis Reaction
2.3.2. Combustion at the Anode Produces
2.3.3. Combustion at the Cathode Produces
2.3.4. Overall Reaction Heat
3. Case B: A High Energy Scenario Where the Product Distribution Is Poor in Molecular Hydrogen
3.1. Chemistry of the Overall Reaction
3.2. The Anodic Oxygen + Cathodic Hydroxyl Radical Mechanism
3.2.1. Electrolysis Reaction
3.2.2. Combustion at the Anode Produces and
3.2.3. Combustion at the Cathode Produces and
3.2.4. Overall Reaction Heat
3.3. The Anodic Ozone + Cathodic Hydrogen Peroxide Mechanism
3.3.1. Electrolysis Reaction
3.3.2. Combustion at the Anode Produces and
3.3.3. Combustion at the Cathode Produces and
3.3.4. Overall Reaction Heat
4. Performance Characteristics
4.1. Energy Efficiency
4.2. Mass Transfer Rate
5. Discussion
- (1)
- (2)
- (3)
6. Conclusions
- (1)
- The proposed mechanisms account for the appearance of carbon monoxide, carbon dioxide, water, molecular nitrogen, and molecular hydrogen among the products.
- (2)
- The heat of oxidation of PVA at the cathode can be endothermic. According to Table 2, this occurs in Case A, where the cathodic oxidizing species, , is weak and the products of combustion are poor in the oxides.
- (3)
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| PVA | polyvinyl alcohol |
| HAN | hydroxylammonium nitrate |
Nomenclauture
| specific mass transfer rate | |
| electrical efficiency | |
| electrolytic conversion fraction | |
| number of moles of species “X” | |
| heat content of species “X” | |
| electrolytic enthalpy | |
| heat of combustion at the anode | |
| heat of combustion at the cathode | |
| total reaction enthalpy | |
| electrolytic enthalpy per mole of HAN | |
| anodic combustion enthalpy per mole of HAN | |
| cathodic combustion enthalpy per mole of HAN | |
| total reaction enthalpy per mole of HAN |
References
- Yetter, R.A.; Yang, V.; Wu, M.-H.; Wang, Y.; Milius, D.; Aksay, I.A.; Dryer, F.L. Combustion Issues and Approaches for Chemical Microthrusters. Int. J. Energ. Mater. 2007, 6, 393–424. [Google Scholar] [CrossRef]
- Gohardani, A.S.; Stanojev, J.; Demaire, A.; Anflo, K.; Persson, M.; Wignborg, N.; Nilsson, C. Green Space Propulsion: Opportunities and Prospects. Prog. Aerosp. Sci. 2014, 71, 128–149. [Google Scholar] [CrossRef]
- Amroussee, R.; Katsimi, P.; Itouyama, N.; Azuma, N.; Kagawa, H.; Hatai, K.; Ikeda, H.; Hori, K. New HAN-Based Mixtures for Reaction Control System and Low Toxic Spacecraft Propulsion System: Thermal Decomposition and Possible Thruster Applications. Combust. Flame 2015, 162, 2686–2692. [Google Scholar] [CrossRef]
- Amroussee, R.; Katsumi, T.; Azuma, N.; Hori, K. Hydroxylammonium Nitrate (HAN)—Based Green Propellant as Alternative Energy Resource for Potential Hydrazine Substitution: From Lab Scale to Pilot Plant Scale-Up. Combust. Flame 2017, 176, 334–348. [Google Scholar] [CrossRef]
- Kidd, F.G., III; Taylor, N.R.; Lemmer, K.M. Decomposition of Hydroxylammonium Nitrate in a Low Pressure Flowing Capillary System. J. Mol. Liq. 2018, 262, 396–404. [Google Scholar] [CrossRef]
- Amrousee, R.; Hori, K.; Fetimi, W.; Farhat, K. HAN and AND as Liquid Ionic Monopropellants: Thermal and Catalytic Decomposition Processes. Appl. Catal. B Environ. 2012, 127, 121–128. [Google Scholar] [CrossRef]
- Risha, G.A.; Yetter, R.A.; Yang, V. Electrolytic-Induced Decomposition and Ignition of HAN-Based Liquid Monopropellants. Int. J. Energetic Mater. Chem. Propuls. 2007, 6, 575–588. [Google Scholar] [CrossRef]
- Wu, M.-H.; Yetter, R.A. A Novel Electrolytic Ignition Monopropellant Microthruster Based on Low Temperature Co-Fired Ceramic Tape Technology. Lab Chip 2009, 9, 910–916. [Google Scholar] [CrossRef] [PubMed]
- Koh, K.S.; Chin, J.; Chik, T.F.W.K. Role of Electrodes in Ambient Electrolytic Decomposition of Hydroxylammonium Nitrate (HAN) Solutions. Propuls. Power Res. 2013, 2, 194–200. [Google Scholar] [CrossRef]
- Khare, P.; Yang, V.; Meng, H.; Risha, G.A.; Yetter, R.A. Thermal and Electrolytic Decomposition and Ignition of HAN—Water Solutions. Combust. Sci. Technol. 2015, 187, 1065–1078. [Google Scholar] [CrossRef]
- Chai, W.S.; Cheah, K.H.; Koh, K.S.; Chin, J.; Chik, T.F.W.K. Parametric Studies of Electrolytic Decomposition of Hydroxylammonium Nitrate (HAN) Energetic Ionic Liquid in Microractor Using Image Processing Technique. Chem. Eng. J. 2016, 296, 19–27. [Google Scholar] [CrossRef]
- Baird, J.K.; Lang, J.R.; Hiatt, A.T.; Frederick, R.A., Jr. Electrolytic Combustion in the Polyvinyl Alcohol + Hydroxylammonium Nitrate Solid Propellant. J. Propuls. Power 2017, 33, 1589–1590. [Google Scholar] [CrossRef]
- Baird, J.K.; Huang, S.; Frederick, R.A., Jr. Space Charge Limited Conduction in Polyvinyl Alcohol + Hydroxylammonium Nitrate Solid Propellant. J. Propuls. Power 2020, 36, 479–484. [Google Scholar] [CrossRef]
- Birk, J.P. Chemistry; Houghton Mifflin, Co.: Palo Alto, CA, USA, 1994; p. 292. [Google Scholar]
- Pilling, M.J.; Smith, I.W.M. (Eds.) Modern Gas Kinetics: Theory Experiment and Application; Blackwell Scientific Publishers: Oxford, UK, 1987. [Google Scholar]
- Bruce, P.G. (Ed.) Solid State Electrochemistry; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Baird, J.K.; Lee, N.; Miller, G. The G-Value in Plasma and Radiation Chemistry. J. Appl. Phys. 1990, 68, 3661–3668. [Google Scholar] [CrossRef]
- Miller, G.P.; Baird, J.K. Radiofrequency Plasma Decomposition of Ammonia: A Comparison with Radiation Chemistry Using the G-Value. J. Phys. Chem. 1993, 97, 10984–10988. [Google Scholar] [CrossRef]
- Wayne, R.P. Principles and Applications of Photochemistry; Oxford Science Publications: Oxford, UK, 1988. [Google Scholar]
- Glasscock, M.S.; Drew, P.D.; Rovey, J.L.; Polzin, K.A. Thermodynamic Properties of Hydroxylammonium Nitrate—Based Electric Solid Propellants. J. Thermodyn. Heat Transf. 2020, 34, 522–529. [Google Scholar] [CrossRef]
| −ΔH(X) | C2H4O | NH3OH+ | NO3− | H2O | CO2 | CO |
| (kJ/mol) | 2742 | 1818 | 1009 | 918 | 1598 | 1072 |
| −ΔH(X) | H2 | N2 | O | O3 | H2O2 | OH |
| (kJ/mol) | 436 | 942 | zero | 636 | 1060 | 459 |
| Case | Oxidizing Species Anode/Cathode | ΔeHm | ΔaHm | ΔcHm | ΔTotHm |
|---|---|---|---|---|---|
| A | O/OH | 772 | −1306 | −33 | −567 |
| O3/H2O2 | 65 | −670 | 38 | −567 | |
| B | O/OH | 772 | −1374 | −206.33 | −808.33 |
| O3/H2O2 | 65 | −738 | −135.33 | −808.33 |
| Case | fe (W/A) | E (%) | dm/dt ((mg/s)/A) | H2 (mol%) |
|---|---|---|---|---|
| A | 5.88 | 2.9 | 1.82 | 39.2 |
| B | 8.38 | 4.2 | 1.34 | 24.7 |
| Case | NCO2 | NCO | NH2O | NN2 | NH2 | NHAN | mol% H2 |
|---|---|---|---|---|---|---|---|
| A | 3 | 15 | 8 | 5 | 20 | 5 | 39.2 |
| B | 36 | 10 | 61 | 30 | 45 | 30 | 24.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baird, J.K.; Frederick, R.A., Jr. Thermochemistry of Combustion in Polyvinyl Alcohol + Hydroxylammonium Nitrate. Aerospace 2021, 8, 142. https://doi.org/10.3390/aerospace8050142
Baird JK, Frederick RA Jr. Thermochemistry of Combustion in Polyvinyl Alcohol + Hydroxylammonium Nitrate. Aerospace. 2021; 8(5):142. https://doi.org/10.3390/aerospace8050142
Chicago/Turabian StyleBaird, James K., and Robert A. Frederick, Jr. 2021. "Thermochemistry of Combustion in Polyvinyl Alcohol + Hydroxylammonium Nitrate" Aerospace 8, no. 5: 142. https://doi.org/10.3390/aerospace8050142
APA StyleBaird, J. K., & Frederick, R. A., Jr. (2021). Thermochemistry of Combustion in Polyvinyl Alcohol + Hydroxylammonium Nitrate. Aerospace, 8(5), 142. https://doi.org/10.3390/aerospace8050142





