\({\mathcal{L}_1}\) Adaptive Loss Fault Tolerance Control of Unmanned Hypersonic Aircraft with Elasticity
Abstract
:1. Introduction
2. Problem Formulation
2.1. Dynamic Motion Equations
2.2. Loss Fault and Model Simplification
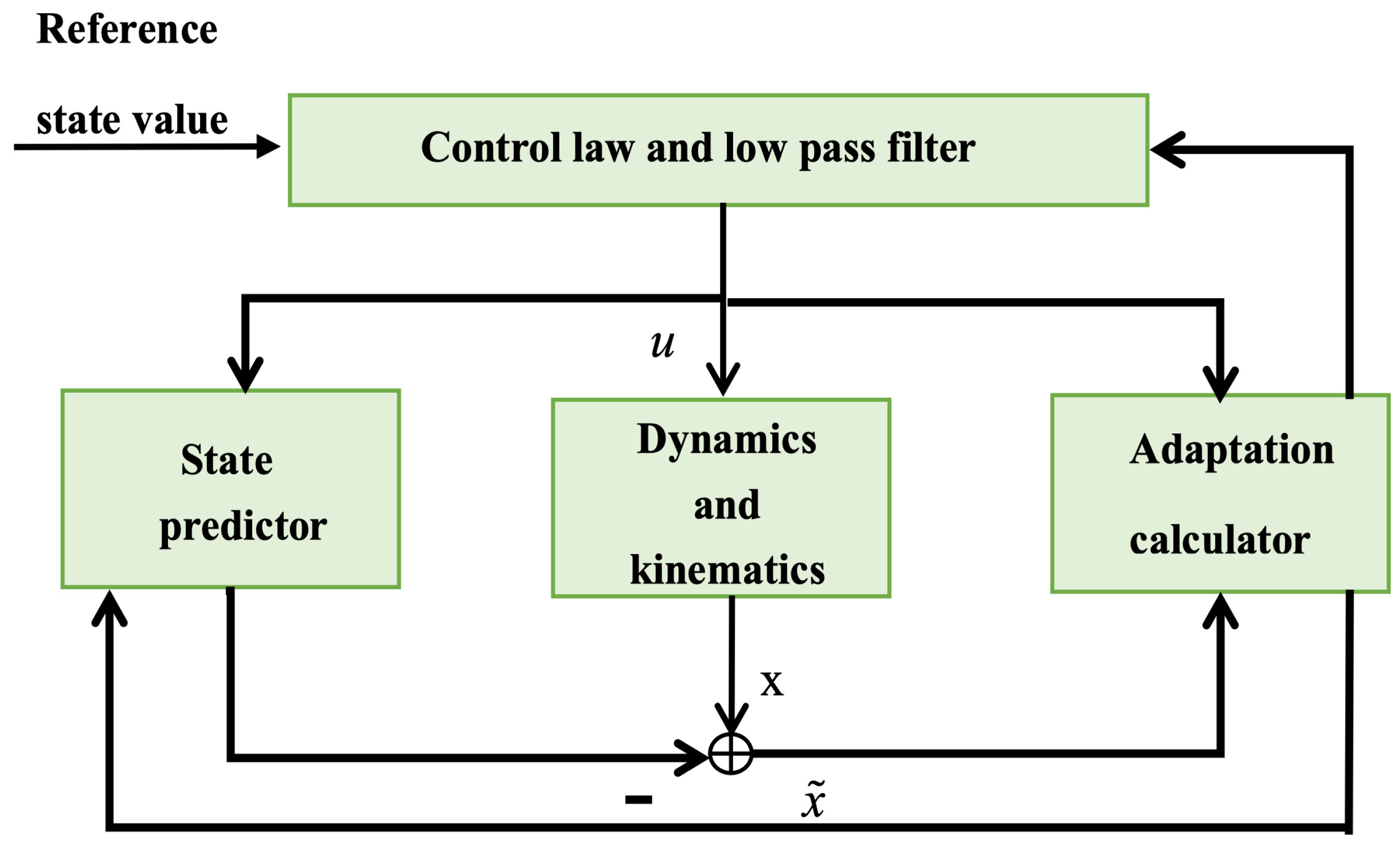
3. Adaptive Controller Design
3.1. Velocity Controller
3.2. Altitude Controller
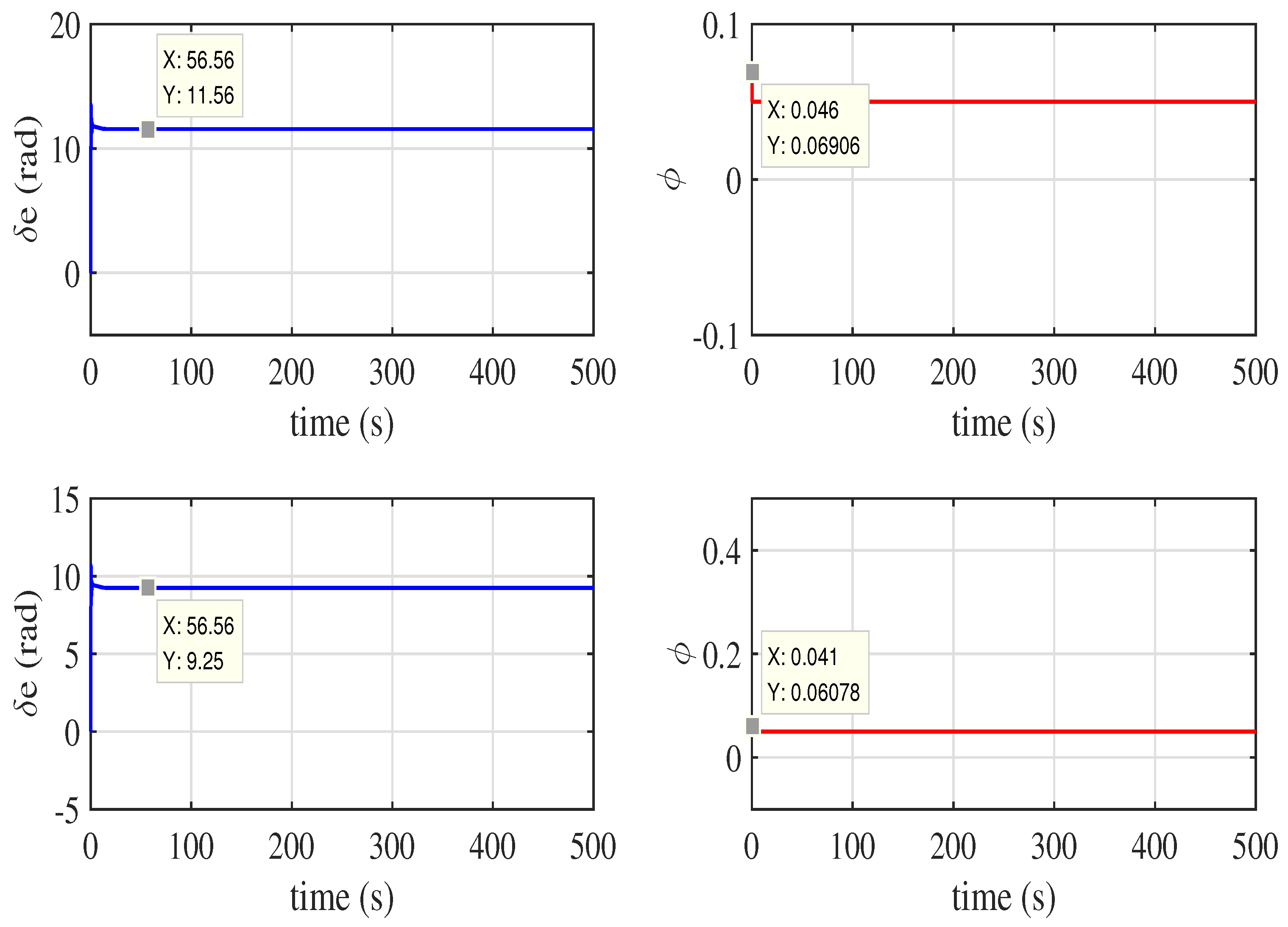
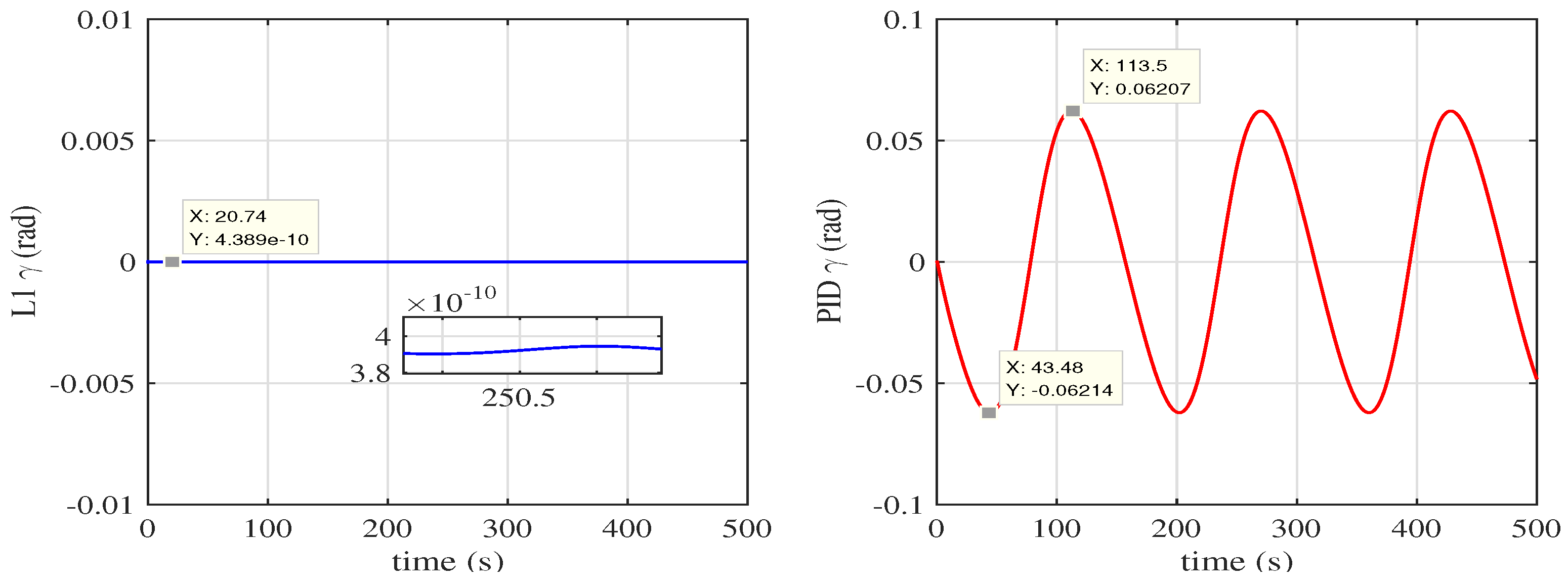
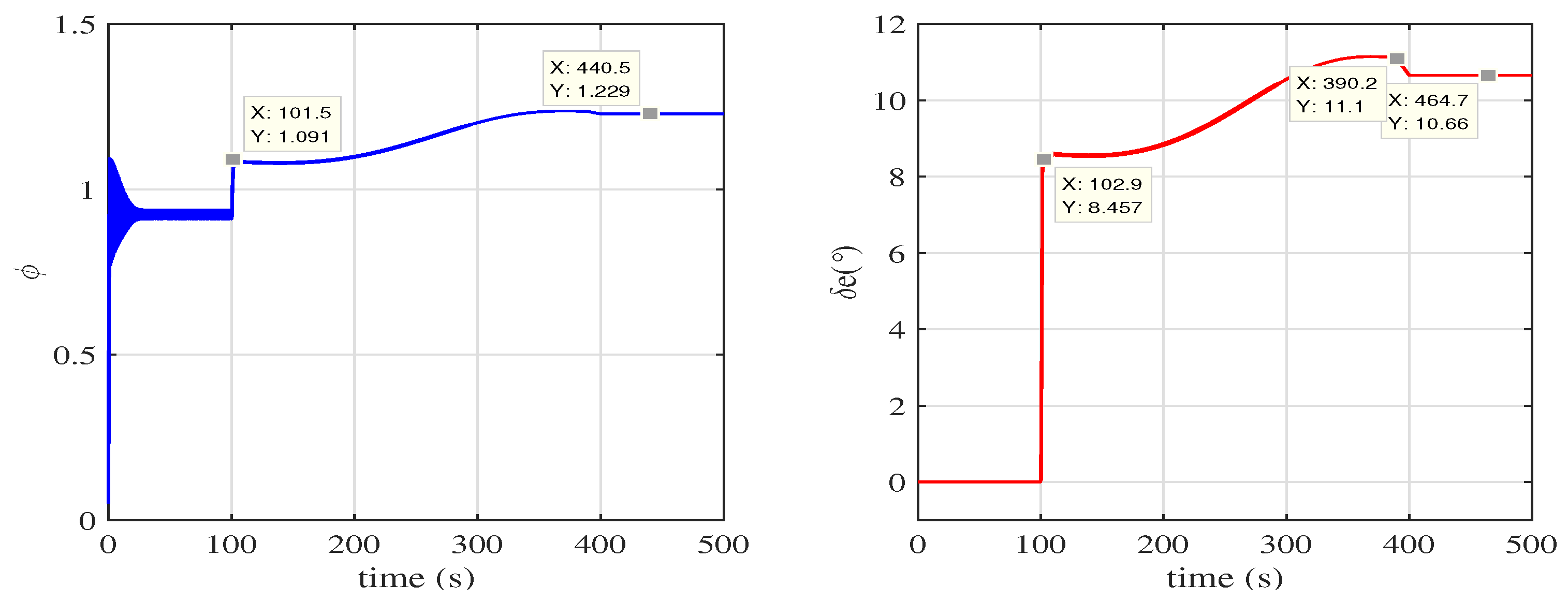
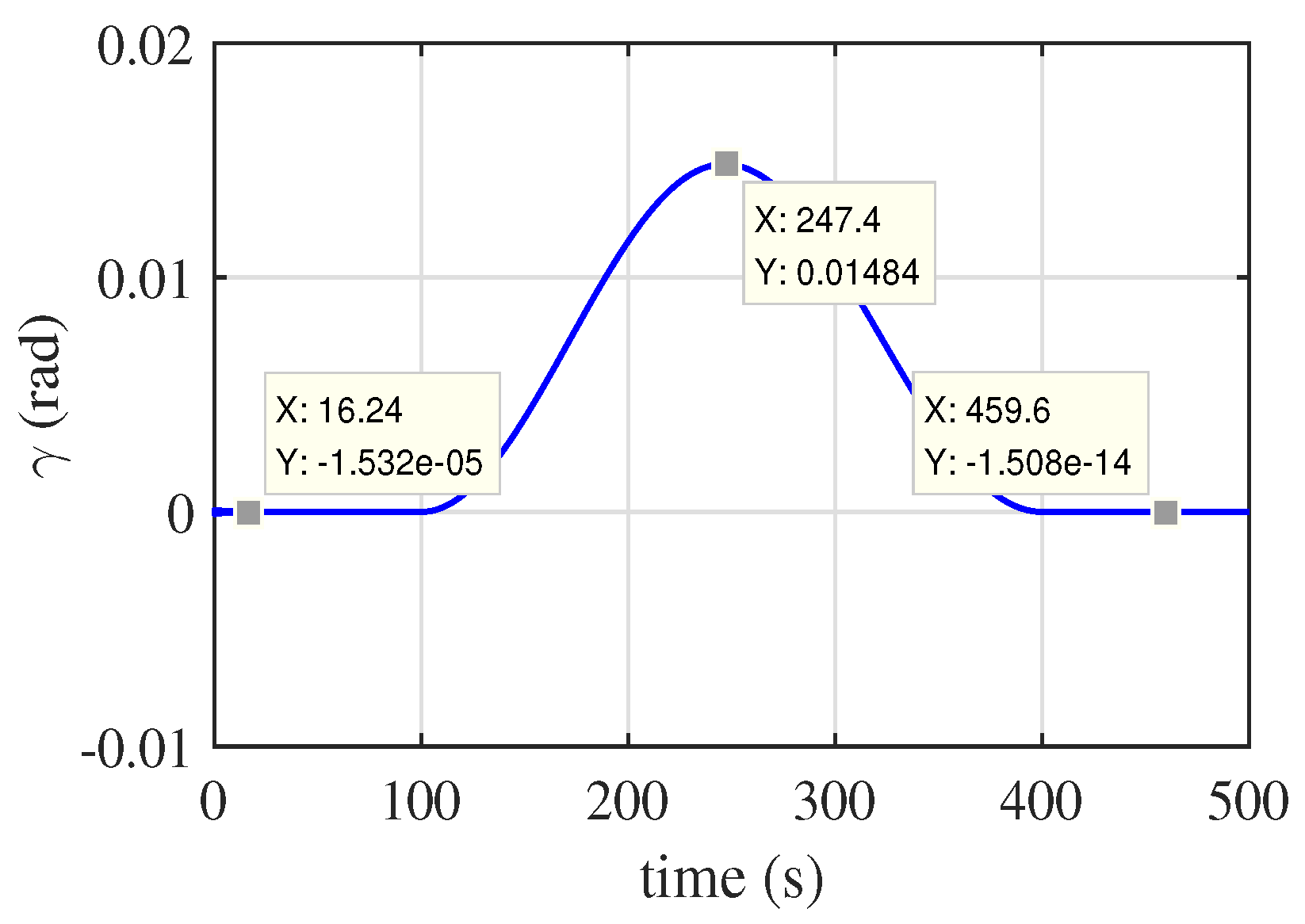
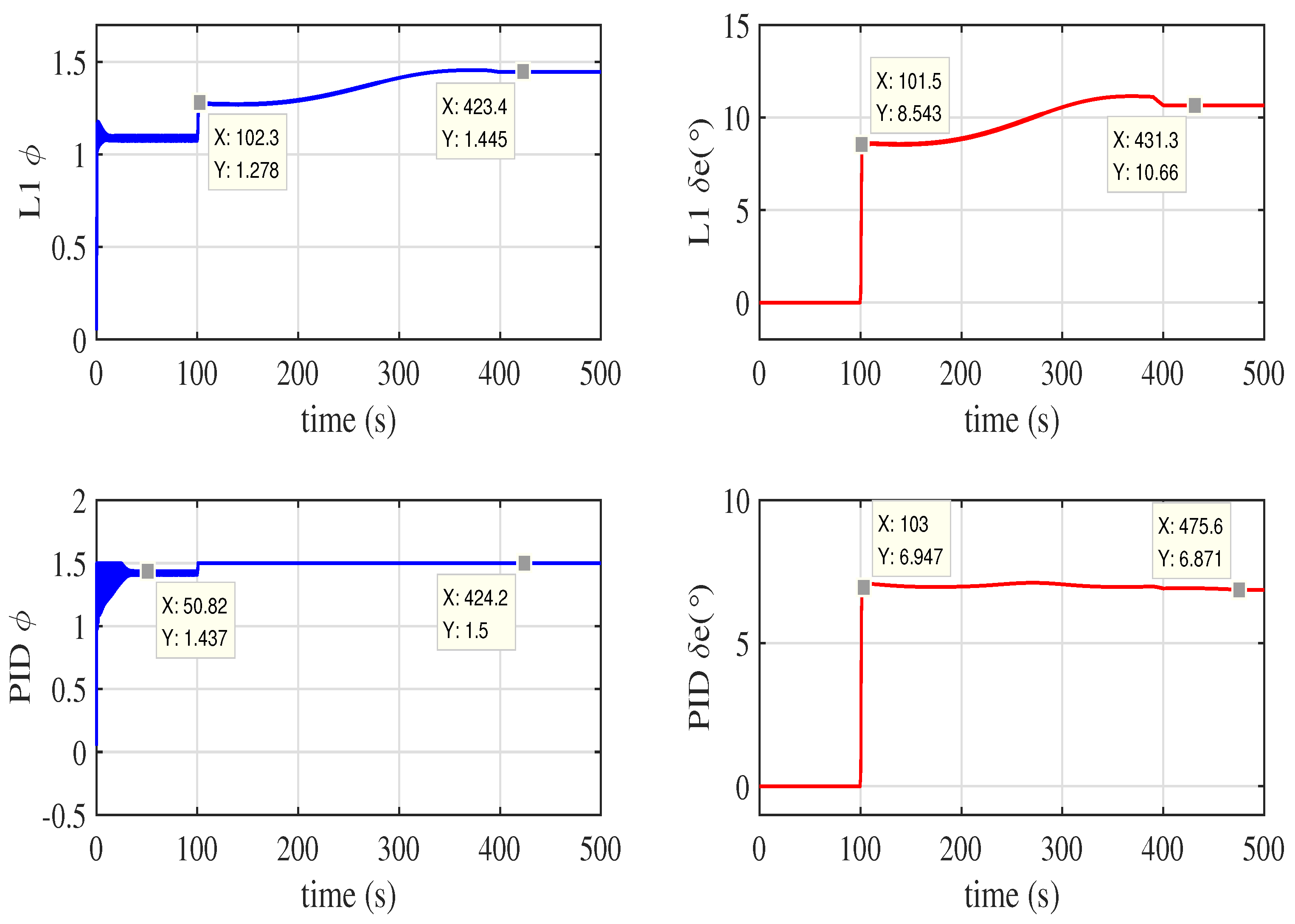
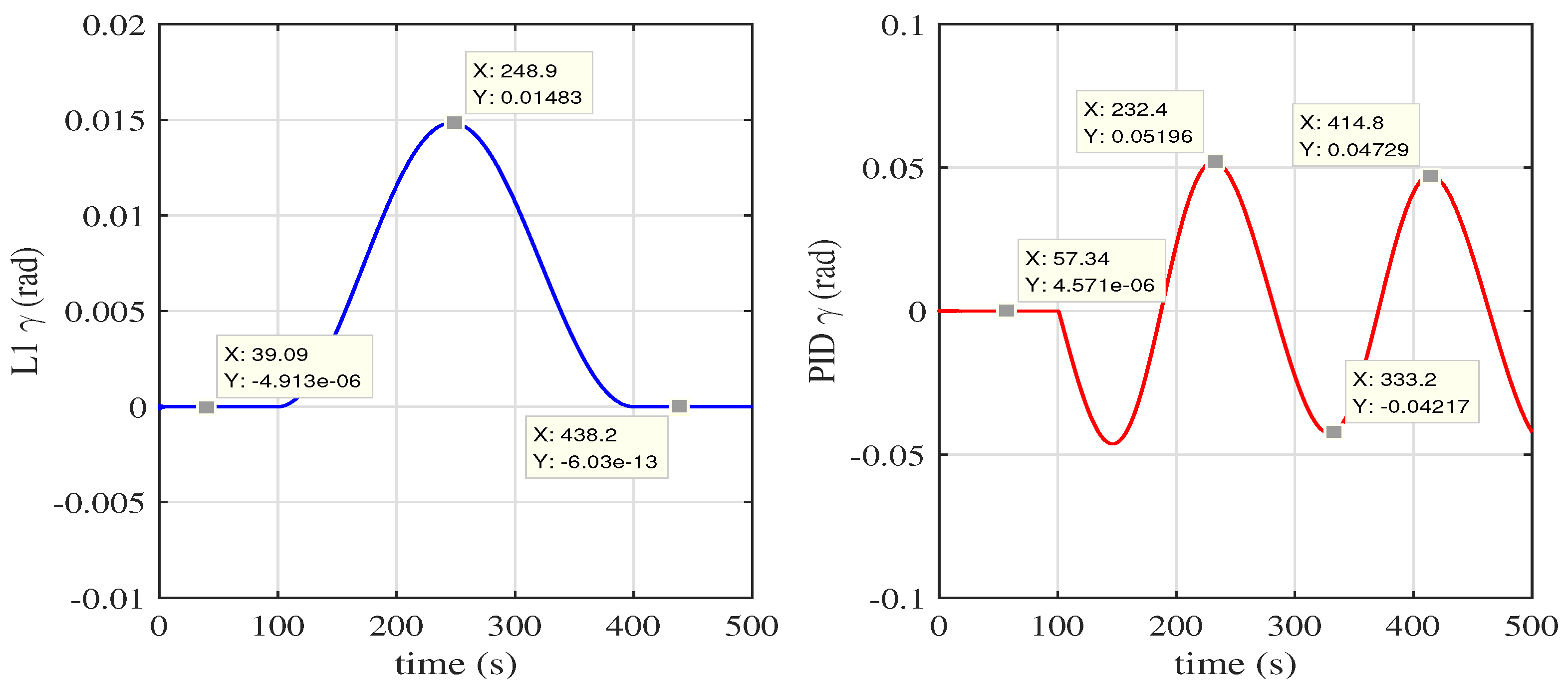
4. Simulation
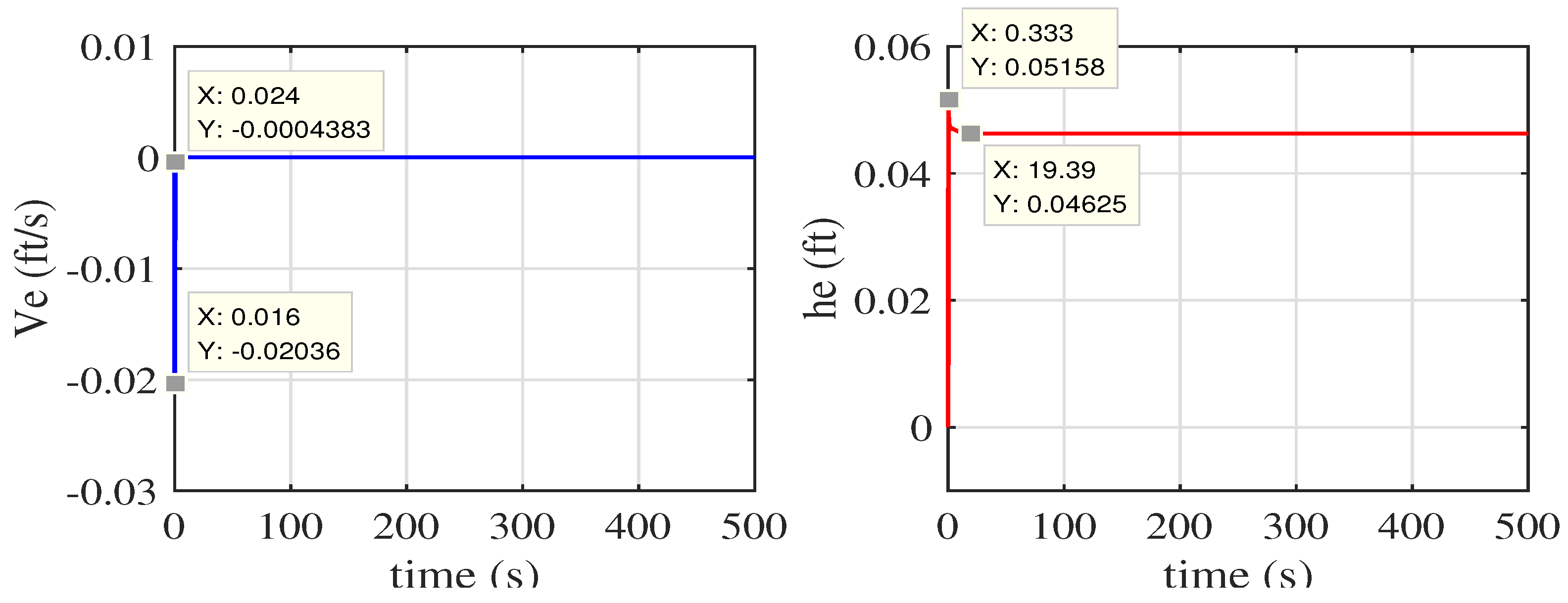
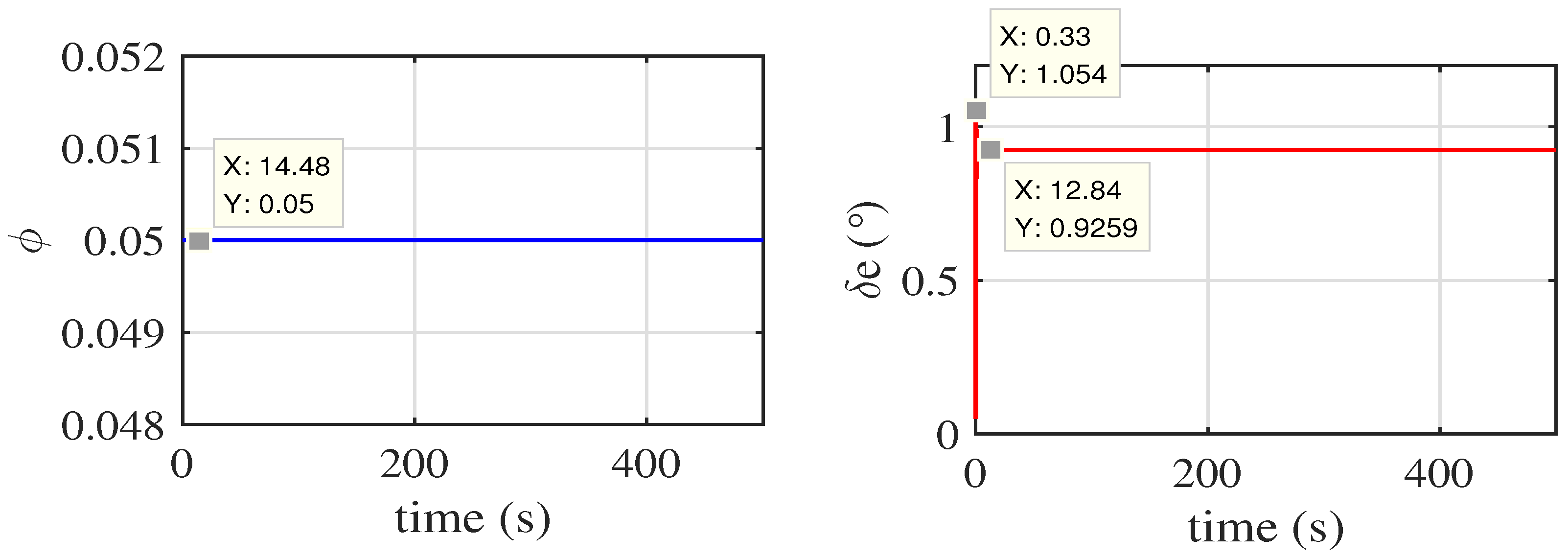
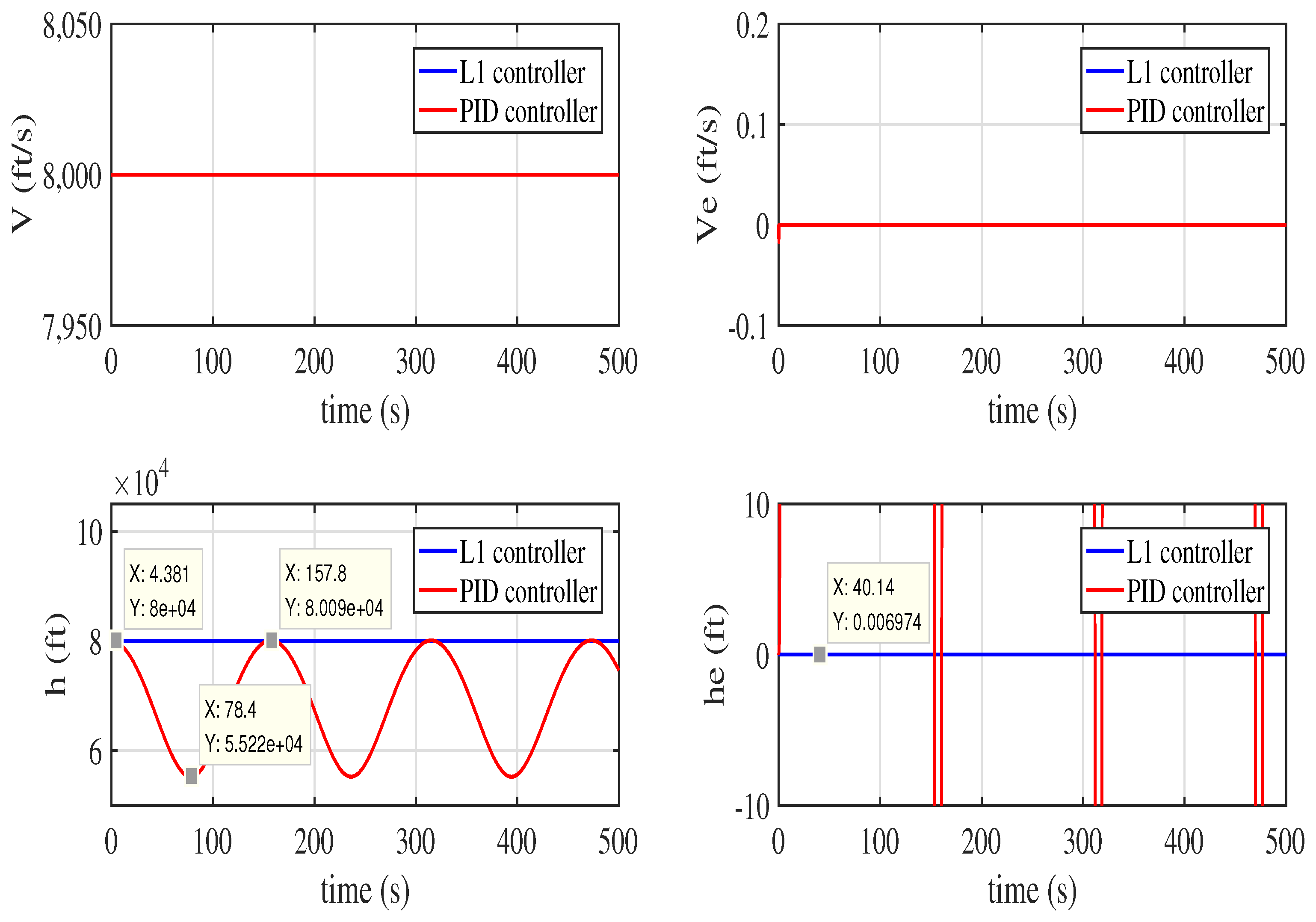
4.1. Horizontal Flight
4.2. Pitching Maneuver
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Lewis, M. X-51 Screams into the Future. Aerosapce Am. 2010, 4, 26–31. [Google Scholar]
- Laurent, S.; Francois, F. Promethee—The French Military Hypersonic Propulsion Program. In Proceedings of the 12th AIAA International Space Planes and Hypersonic Systems and Technologies, Norfolk, VA, USA, 28–30 July 2012. [Google Scholar]
- Park, C. Hypersonic Aerothermodynamics: Past, Present and Future. Int. J. Aeronaut. Space Sci. 2013, 14, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Wei, H.; Zhao-bo, D.; Li, Y. Supersonic mixing in airbreathing propulsion systems for hypersonic flights. Prog. Aerosp. Sci. 2019, 109. [Google Scholar]
- Hambling, D. China, Russia and the US in hypersonic arms race. New Sci. 2019, 243, 12. [Google Scholar] [CrossRef]
- Parker, J.T.; Serrani, A.; Yurkovich, S.; Bolender, M.A.; Doman, D.B. Control-oriented modeling of an air-breathing hypersonic vehicle. J. Guid. Control Dyn. 2007, 30, 856–869. [Google Scholar] [CrossRef]
- Fiorentini, L. Nonlinear Adaptive Controller Design for Air-Breathing Hypersonic Vehicles; The Ohio State University: Columbus, OH, USA, 2010. [Google Scholar]
- Linqi, Y.; Qun, Z.; Xiuyun, Z. Adaptive Control for a Non-minimum Phase Hypersonic Vehicle Model. In Proceedings of the 34th Chinese Control Conference, Hangzhou, China, 28–30 July 2015. [Google Scholar]
- Liu, Y.; Tong, Y.; Jin, F. Control law design of hypersonic vehicles using the elastic surrogate model. J. Low Freq. Noise Vib. Act. Control 2020, 39, 216–229. [Google Scholar] [CrossRef] [Green Version]
- Zhou, D.; Lu, Z.; Guo, T.; Shen, E.; Wu, J.; Chen, G. Fluid-thermal modeling of hypersonic vehicles via a gas-kinetic BGK scheme-based integrated algorithm. Aerosp. Sci. Technol. 2020, 99. [Google Scholar] [CrossRef]
- Li, Z.; Zhou, W.; Liu, H. Robust Controller Design of Non-minimum Phase Hypersonic Aircrafts Model based on Quantitative Feedback Theory. J. Astronaut. Sci. 2020, 67, 137–163. [Google Scholar] [CrossRef]
- Jia, J.; Fu, D.; He, Z.; Yang, J.; Hu, L. Hypersonic aerodynamic interference investigation for a two-stage-to-orbit model. ACTA Astronaut. 2020, 168, 138–145. [Google Scholar] [CrossRef]
- An, H.; Guo, Z.; Wang, G.; Wang, C. Low-complexity hypersonic flight control with asymmetric angle of attack constraint. Nonlinear Dyn. 2020. [Google Scholar] [CrossRef]
- Cheng, Z.; Chen, F.; Gong, J. Self-repairing control of air-breathing hypersonic vehicle with actuator fault and backlash. Aerosapce Sci. Technol. 2020, 97. [Google Scholar] [CrossRef]
- Sun, J.G.; Li, C.M.; Guo, Y.; Wang, C.Q.; Li, P. Adaptive fault tolerant control for hypersonic vehicle with input saturation and state constraints. ACTA Astronaut. 2020, 167, 302–313. [Google Scholar] [CrossRef]
- Tang, X.; Zhai, D.; Li, X. Adaptive fault-tolerance control based finite-time backstepping for hypersonic flight vehicle with full state constrains. Inf. Sci. 2020, 507, 53–66. [Google Scholar] [CrossRef]
- Dong, C.; Liu, Y.; Wang, Q. Barrier Lyapunov function based adaptive finite-time control for hypersonic flight vehicles with state constraints. ISA Trans. 2020, 9, 163–176. [Google Scholar] [CrossRef]
- Wang, A.; Gong, H.; Chen, F. Fault estimation and compensation for hypersonic flight vehicle via type-2 fuzzy technique and cuckoo search algorithm. Int. J. Adv. Robot. Syst. 2020, 17. [Google Scholar] [CrossRef] [Green Version]
- Thu, K.M.; Gavrilov, A.I. Designing and modeling of quadcopter control system using L1 adaptive control. Int. Symp. Intell. Syst. 2017, 103, 528–535. [Google Scholar]
- Zuo, Z.; Ru, P. Augmented L1 adaptive tracking control of quad-rotor unmanned aircrafts. IEEE Trans. Aerosp. Electron. Syst. 2014, 5, 3090–3101. [Google Scholar] [CrossRef]
- Xu, D.; Whidborne, J.; Cooke, A. Fault tolerant control of a quadrotor using L1 adaptive control. Int. J. Intell. Unmaaned Syst. 2016, 4, 43–66. [Google Scholar] [CrossRef] [Green Version]
- Li, M.; Zuo, Z.; Liu, H. Adaptive fault tolerant control for trajectory tracking of a quadrotor helicopter. Trans. Inst. Meas. Control 2018, 40, 3560–3569. [Google Scholar] [CrossRef]
- Cao, C.; Hovakimyan, N. L1 adaptive control theory. Soc. Ind. Appl. Math. 2010. [Google Scholar] [CrossRef]
- Sigthorsson, D.O.; Jankovsky, P.; Serrani, A.; Yurkovich, S.; Bolender, M.A.; Doman, D.B. Robust linear output feedback control of an airbreathing hypersonic vehicle. J. Guid. Control Dyn. 2008, 31, 1052–1066. [Google Scholar] [CrossRef]



| Parameter | Explanation | Parameter | Explanation |
|---|---|---|---|
| m | mass | dynamic pressure | |
| V | velocity | air density | |
| h | altitude | air density at nominal altitude | |
| track angle | nominal altitude | ||
| pitch angle | inverse of the air density exponential decay rate | ||
| Q | pitch angle rate | generalized force | |
| attack angle | and | six elastic states | |
| T | thrust | damping factor | |
| D | drag | natural oscillation frequency | |
| L | lift | n | loss rate |
| M | pitching torque | reference pitch angle | |
| g | acceleration due to gravity | uncertainties | |
| moment of inertia along the longitudinal direction | adaptive variable | ||
| fuel equivalent ratio | velocity gain | ||
| elevator deflection angle | control law | ||
| mean aerodynamic chord | adaption gain | ||
| S | reference area | the solution of Lyapunov equation | |
| thrust-to-moment coupling coefficient | low-filter |
| Mark | Value | Mark | Value |
|---|---|---|---|
| 5.9598 | −0.07402 | ||
| 0.7341 | 0.9102 | ||
| −0.02438 | 1.084 × 10 | ||
| −0.03410 | −0.01988 | ||
| −0.01737 | 0.001293 | ||
| −0.06758 | 2.5523 × 10 | ||
| 7.9641 | 0.002707 | ||
| −0.08928 | 8.8374 × 10 | ||
| 0.3497 | 9.5685 × 10 | ||
| 2.7562 × 10 | 1.3834 × 10 | ||
| 0.03902 | −2.4875 × 10 | ||
| −9.3415 × 10 | 4.112 × 10 | ||
| −6.7015 × 10 | 1.0924 × 10 | ||
| −1.8813 × 10 | 8.5621 × 10 | ||
| 6.8888 | −7.4826 × 10 | ||
| 5.139 | 0.103 | ||
| 0.1628 | −1.9277 × 10 | ||
| −1.3642 | −4.2624 × 10 | ||
| 7.1776 × 10 | 3.2963 × 10 | ||
| −0.03022 | 3.0022 × 10 | ||
| −0.01067 | 6.5423 × 10 | ||
| −14.038 | 0.03728 | ||
| −1.5839 | −0.02164 | ||
| 0.6934 | 0.0027609 | ||
| 0.1990 | −0.0034979 | ||
| 1.0929 | −0.005331 | ||
| 0.9714 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Shi, S. \({\mathcal{L}_1}\) Adaptive Loss Fault Tolerance Control of Unmanned Hypersonic Aircraft with Elasticity. Aerospace 2021, 8, 176. https://doi.org/10.3390/aerospace8070176
Li Z, Shi S. \({\mathcal{L}_1}\) Adaptive Loss Fault Tolerance Control of Unmanned Hypersonic Aircraft with Elasticity. Aerospace. 2021; 8(7):176. https://doi.org/10.3390/aerospace8070176
Chicago/Turabian StyleLi, Zhaoying, and Shuai Shi. 2021. "\({\mathcal{L}_1}\) Adaptive Loss Fault Tolerance Control of Unmanned Hypersonic Aircraft with Elasticity" Aerospace 8, no. 7: 176. https://doi.org/10.3390/aerospace8070176
APA StyleLi, Z., & Shi, S. (2021). \({\mathcal{L}_1}\) Adaptive Loss Fault Tolerance Control of Unmanned Hypersonic Aircraft with Elasticity. Aerospace, 8(7), 176. https://doi.org/10.3390/aerospace8070176






