Wind Tunnel Studies on Hover and Forward Flight Performances of a Coaxial Rigid Rotor
Abstract
:1. Introduction
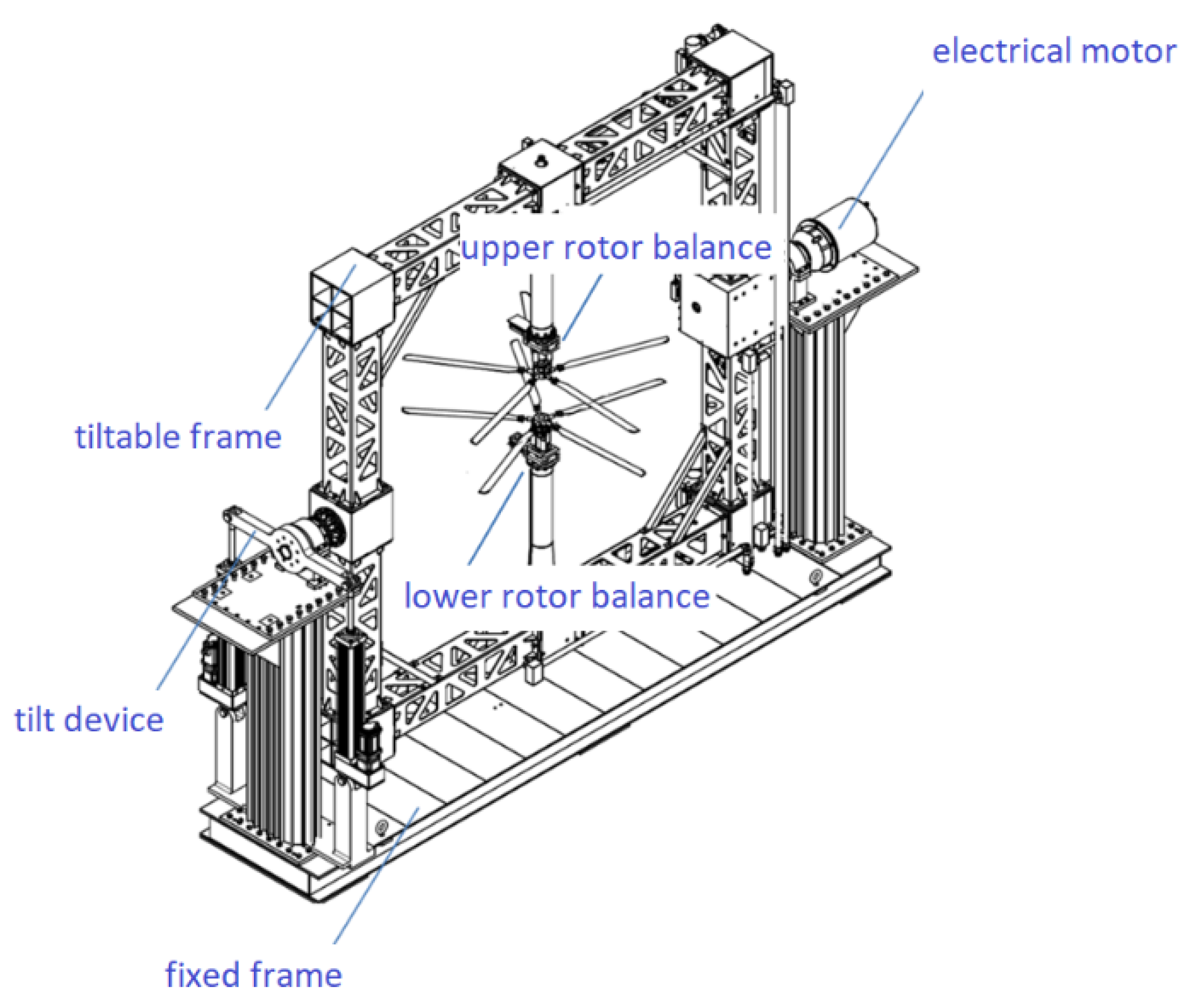
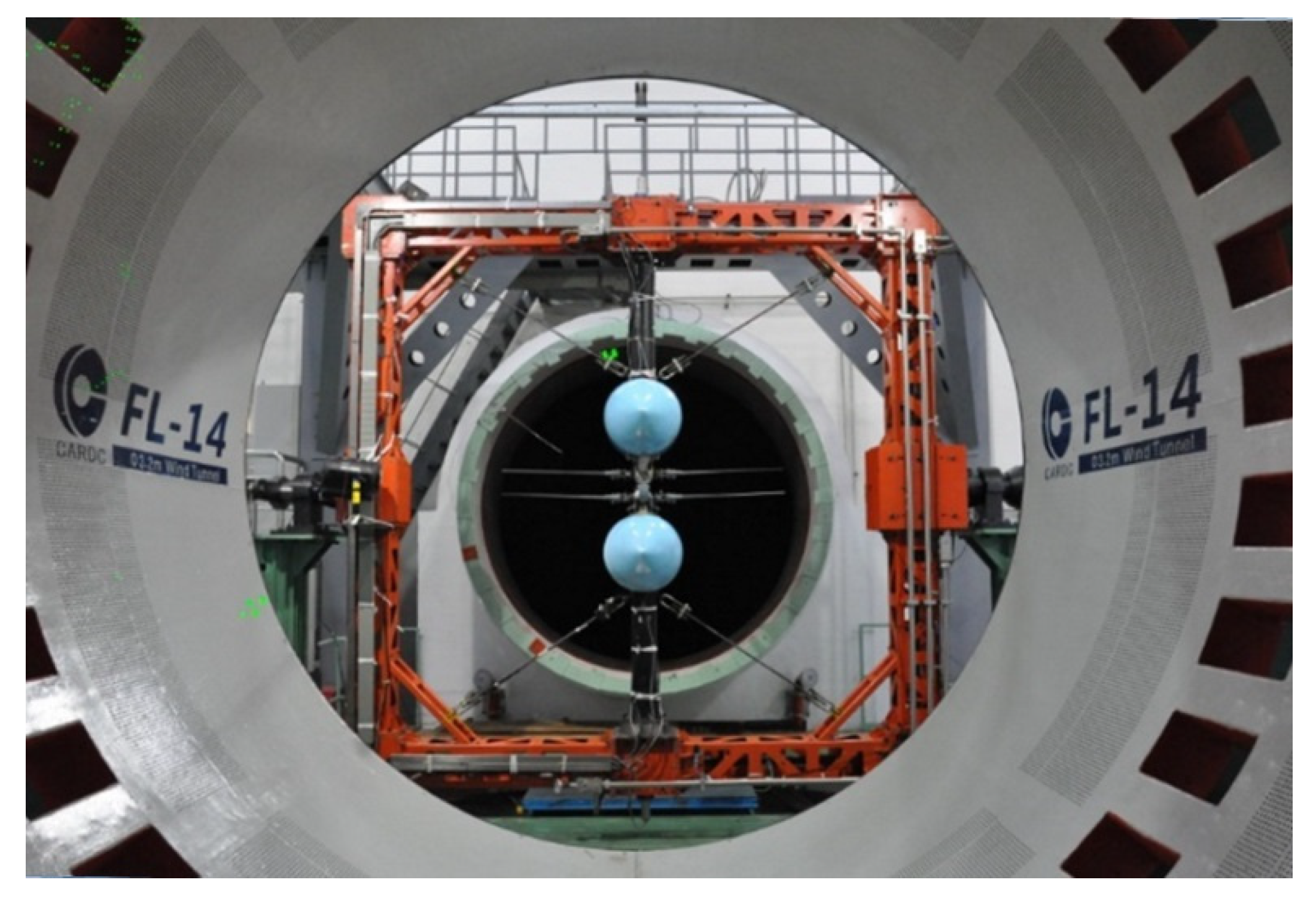
2. Experimental Setup
2.1. Test Apparatus
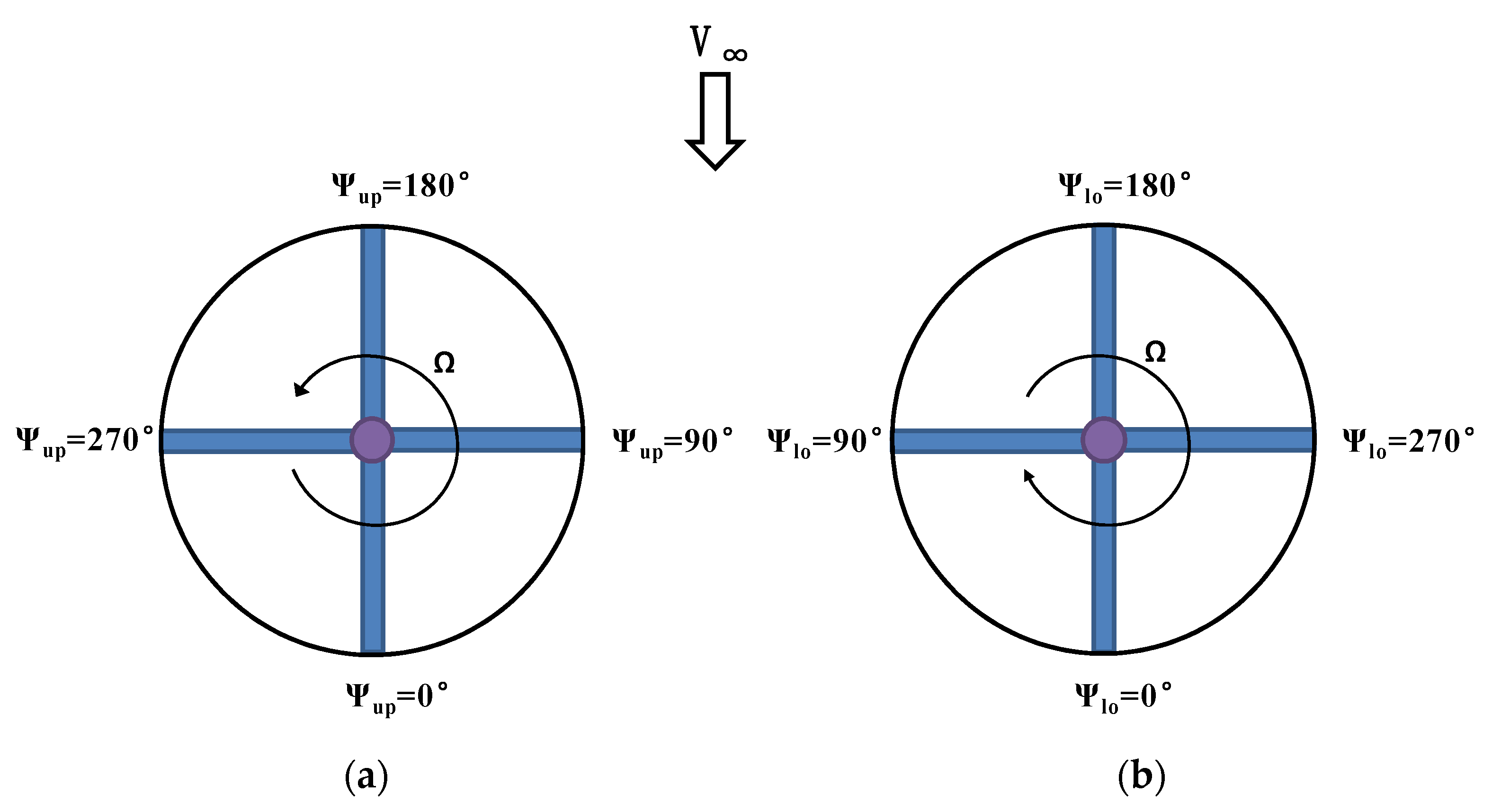
2.2. Test Model and Procedure
2.3. Experimental Content
3. Results and Discussion
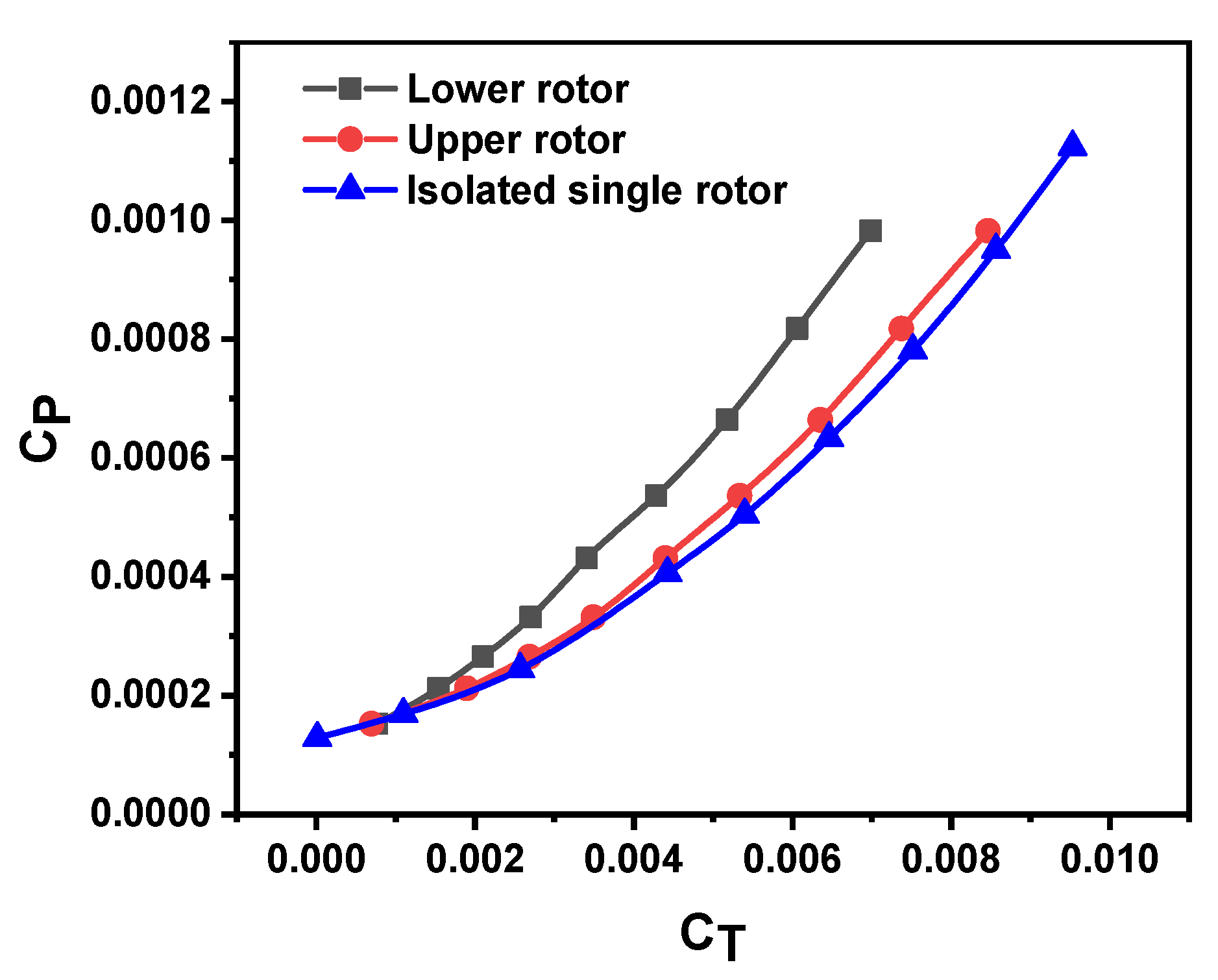
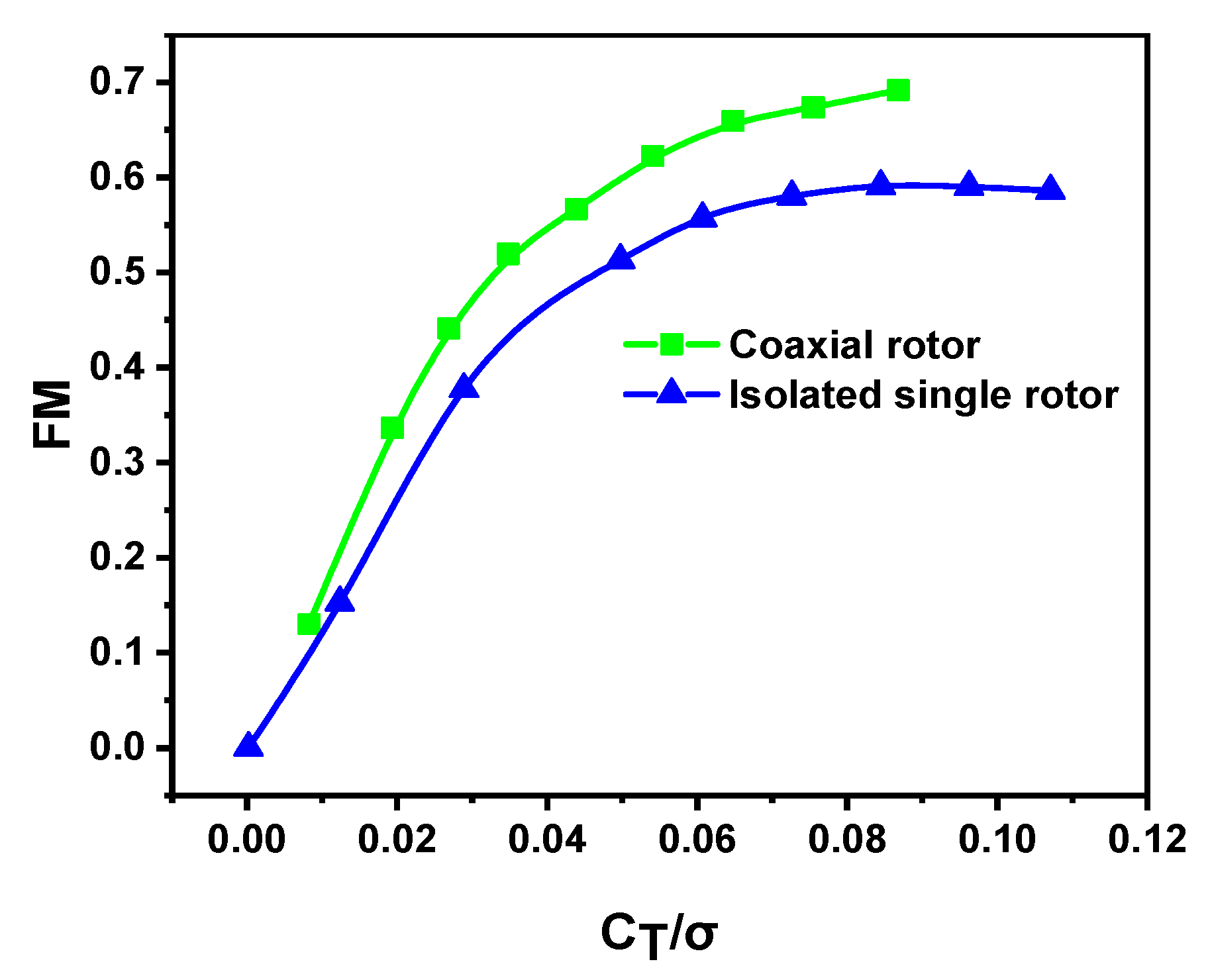
3.1. Rotor Hover Performance
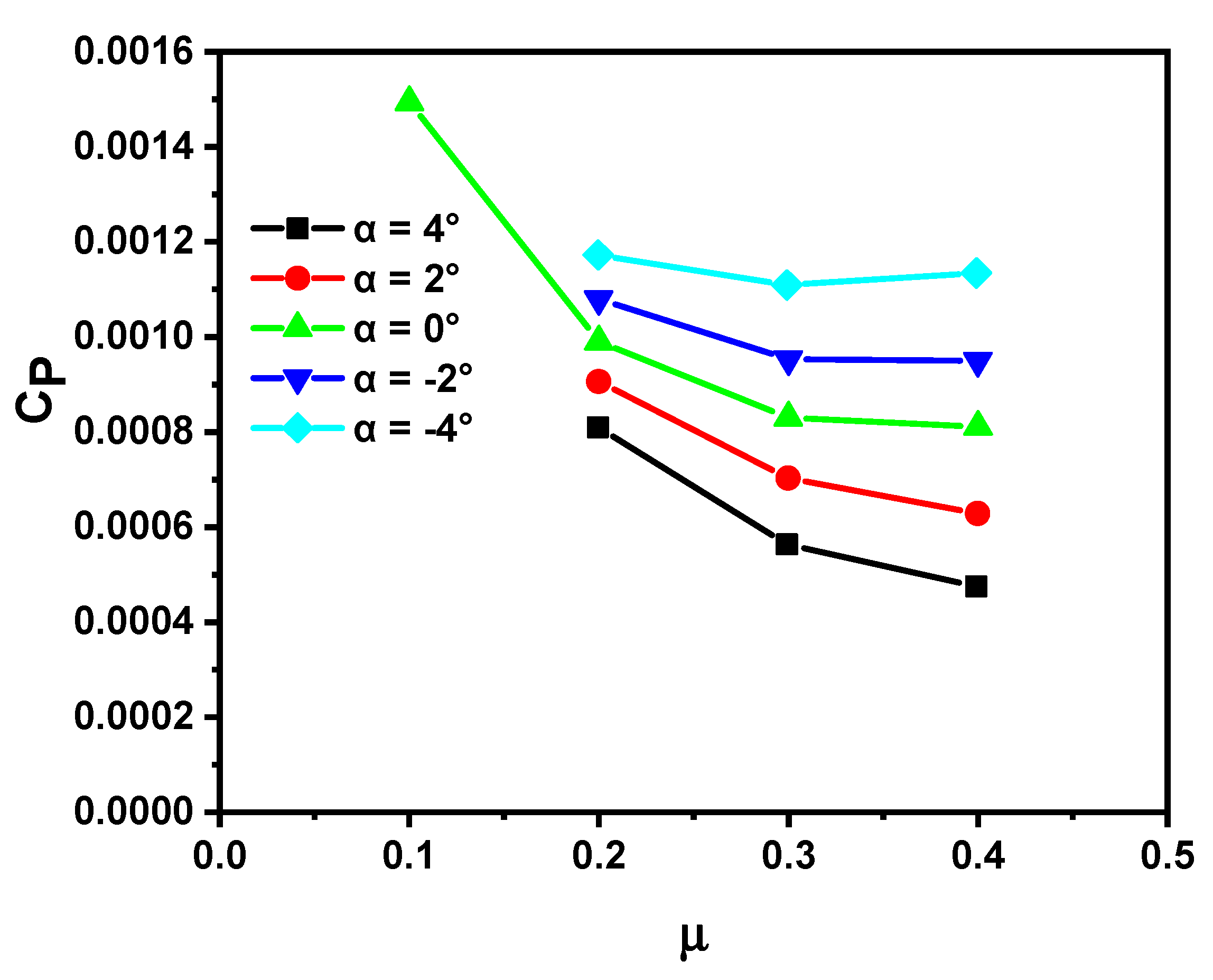
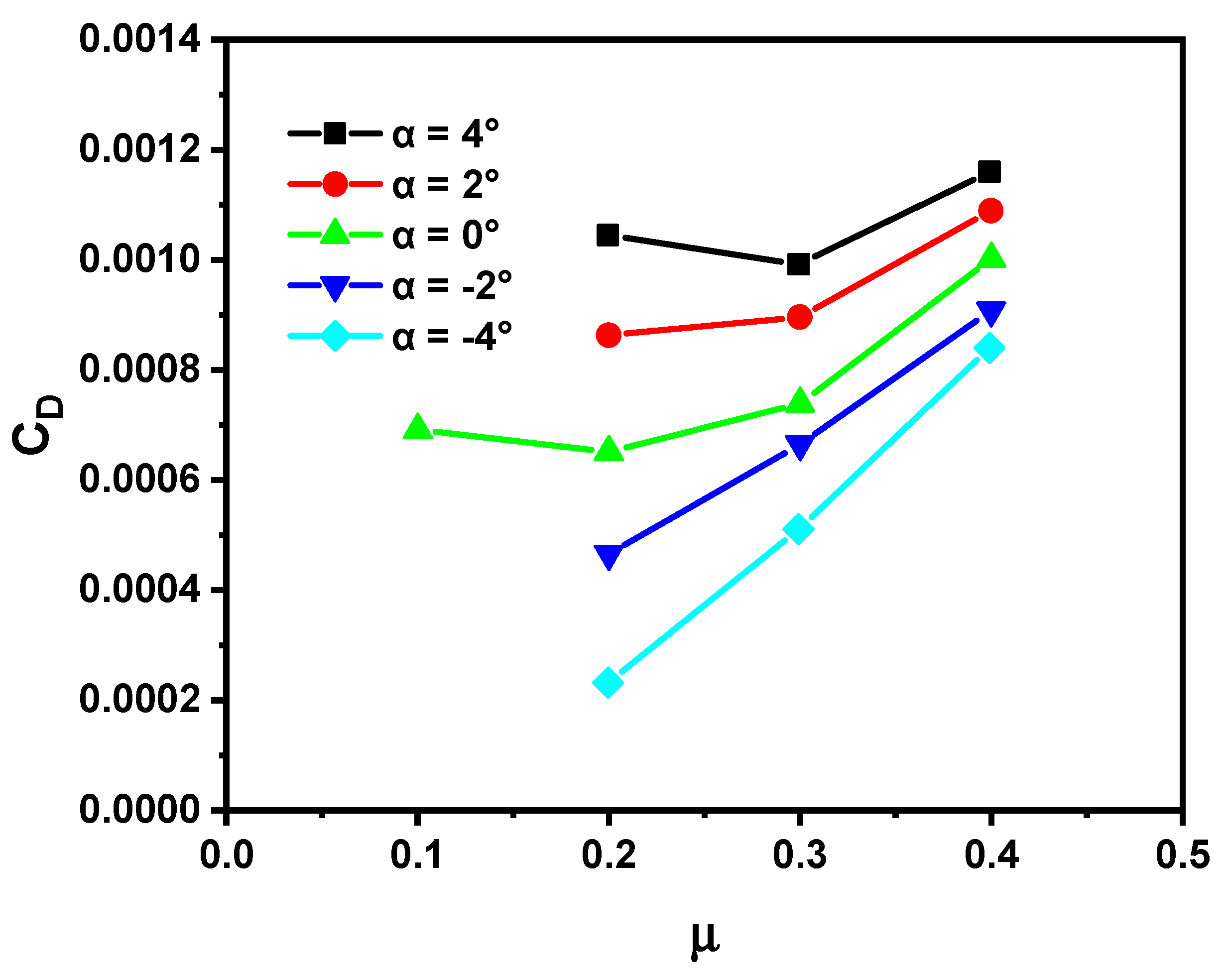
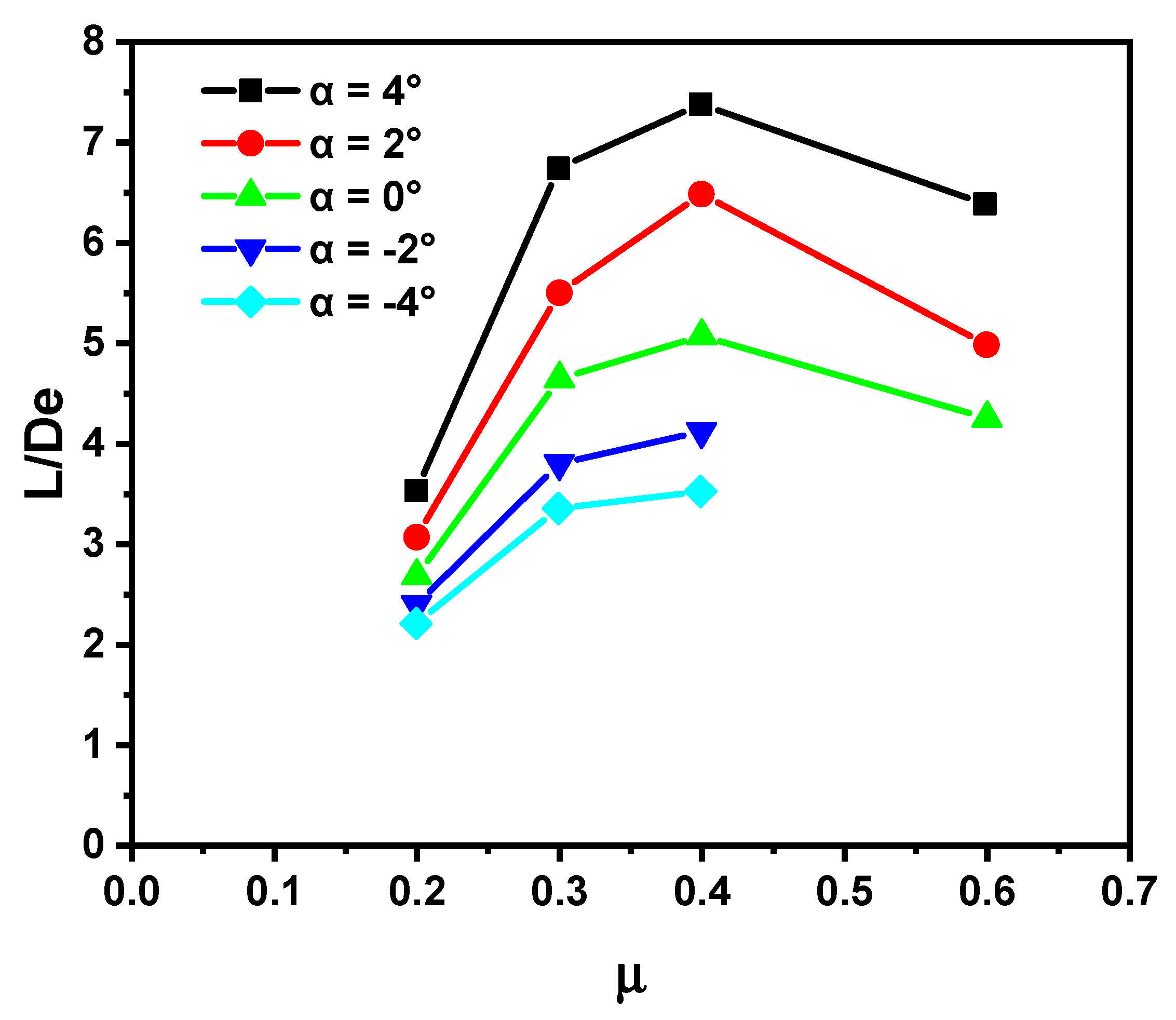
3.2. Forward Flight Rotor Performance
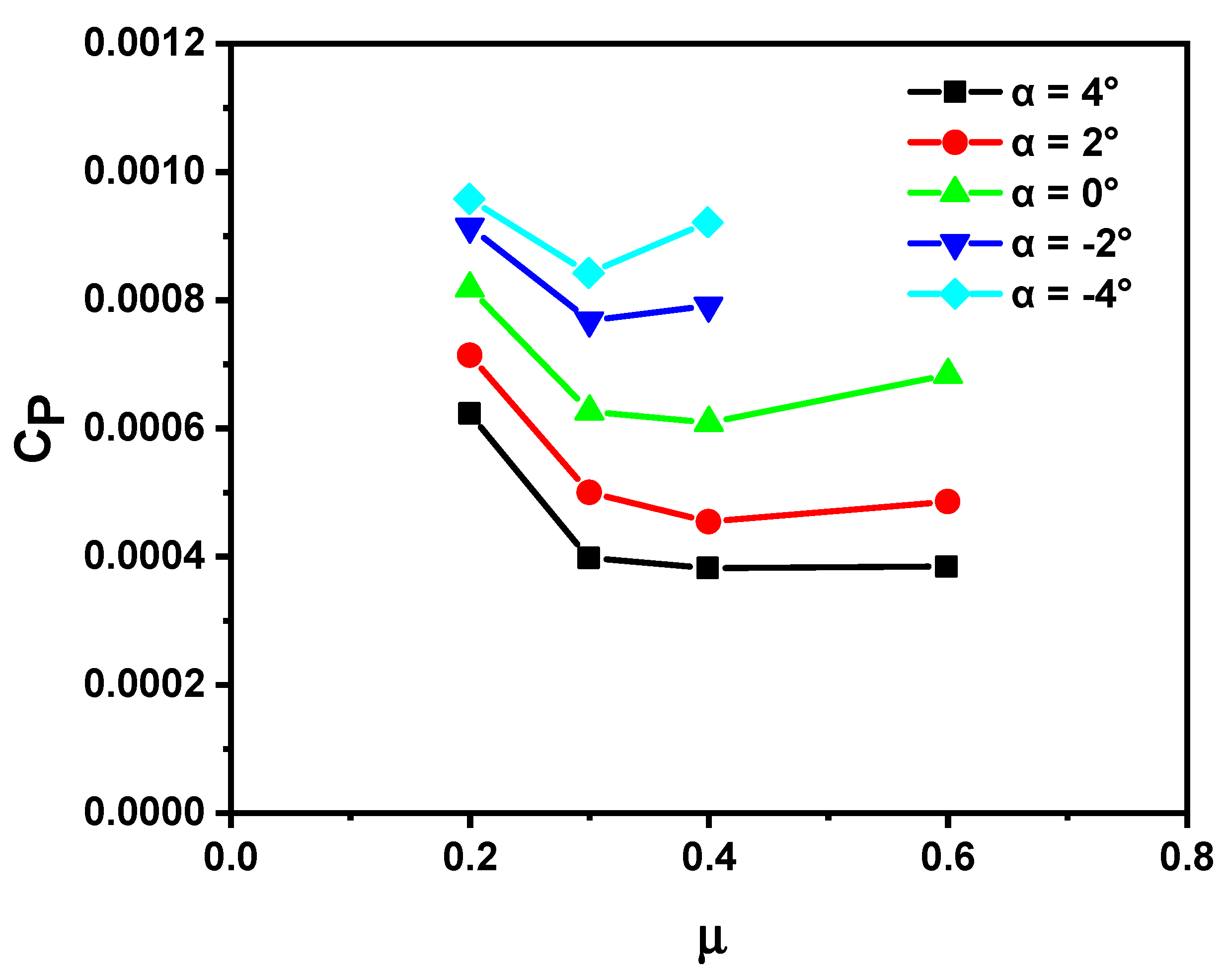
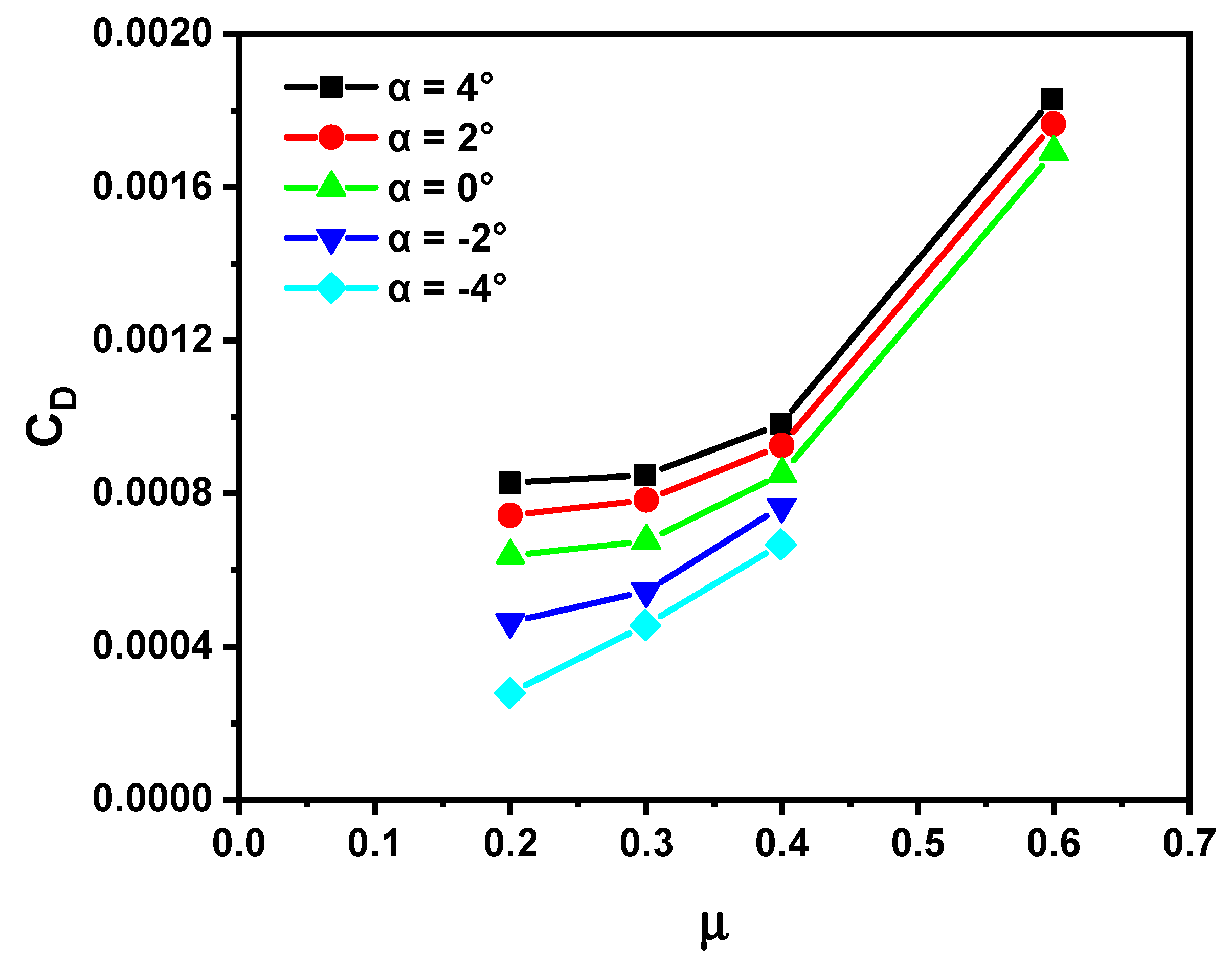
3.2.1. Tilt Angle Sweep Rotor Performance
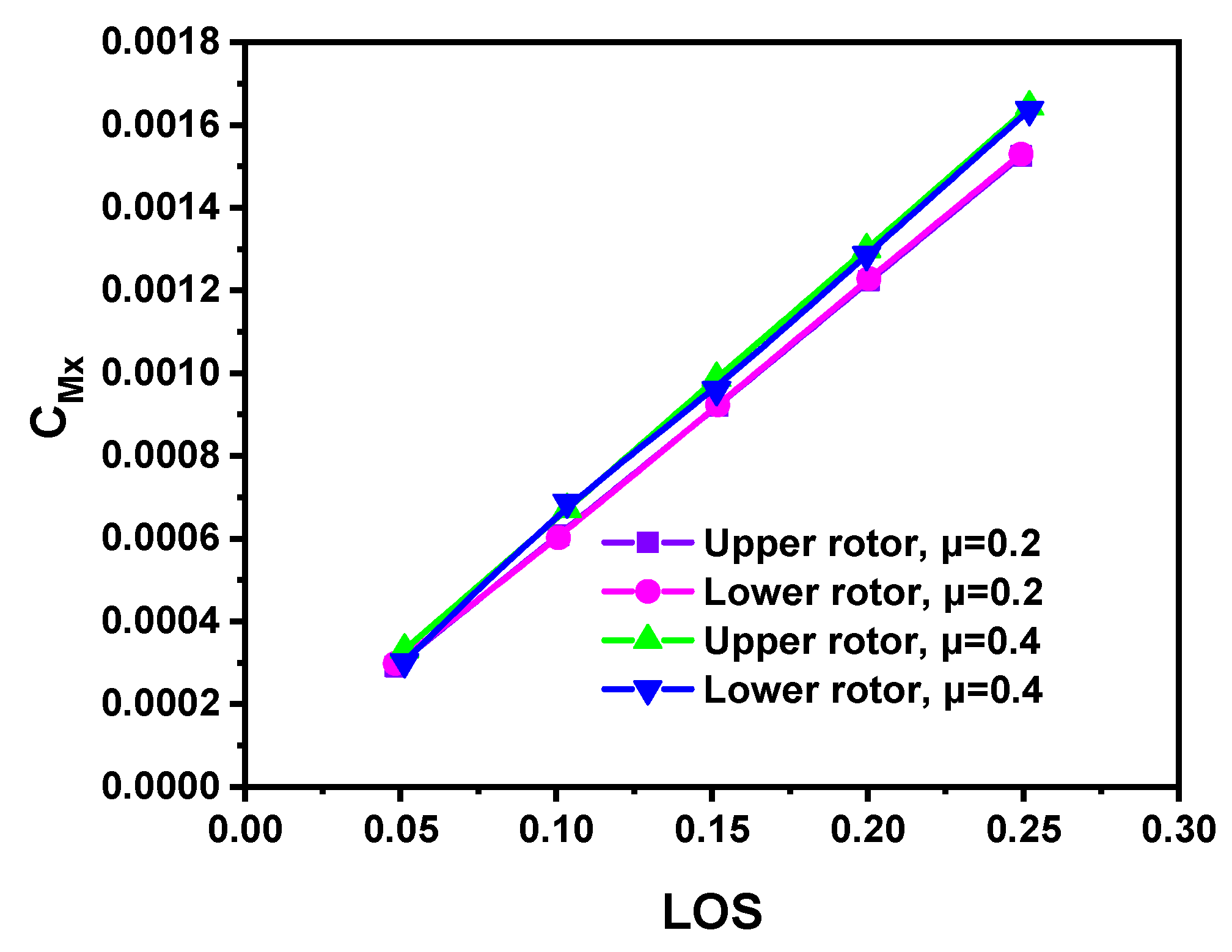
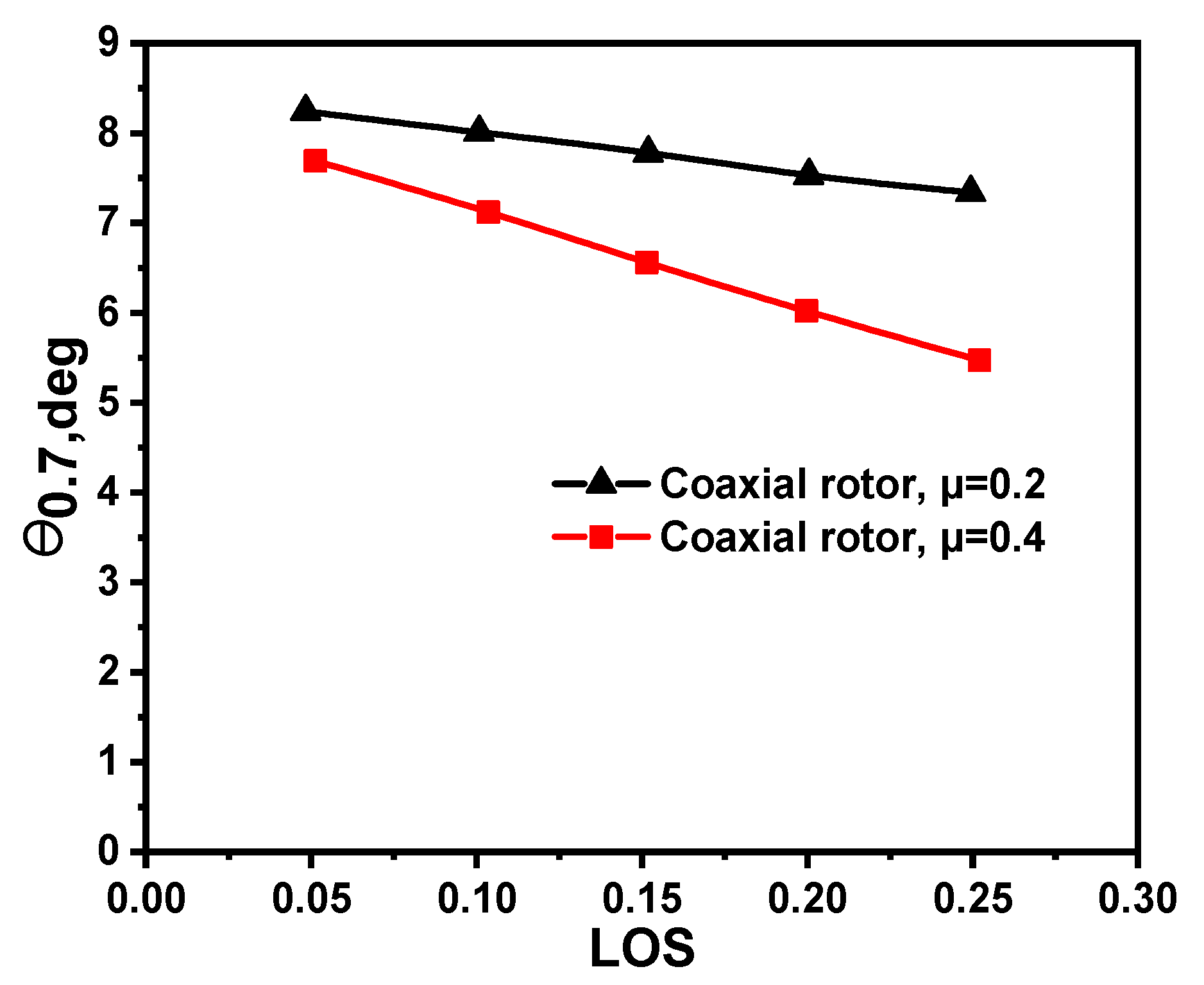
3.2.2. Lift Offset Sweep Rotor Performance
3.2.3. Effect of Interference between Upper and Lower Rotors on Rotor Forward Flight Performance
4. Conclusions
- (1)
- The hover test results demonstrate that the FM values of the upper and lower rotors are lower than that of the isolated single rotor and FM of the lower rotor is lower than that of the upper rotor. Moreover, the coaxial rotor configuration can contribute to better hover efficiency under the same blade loading condition.
- (2)
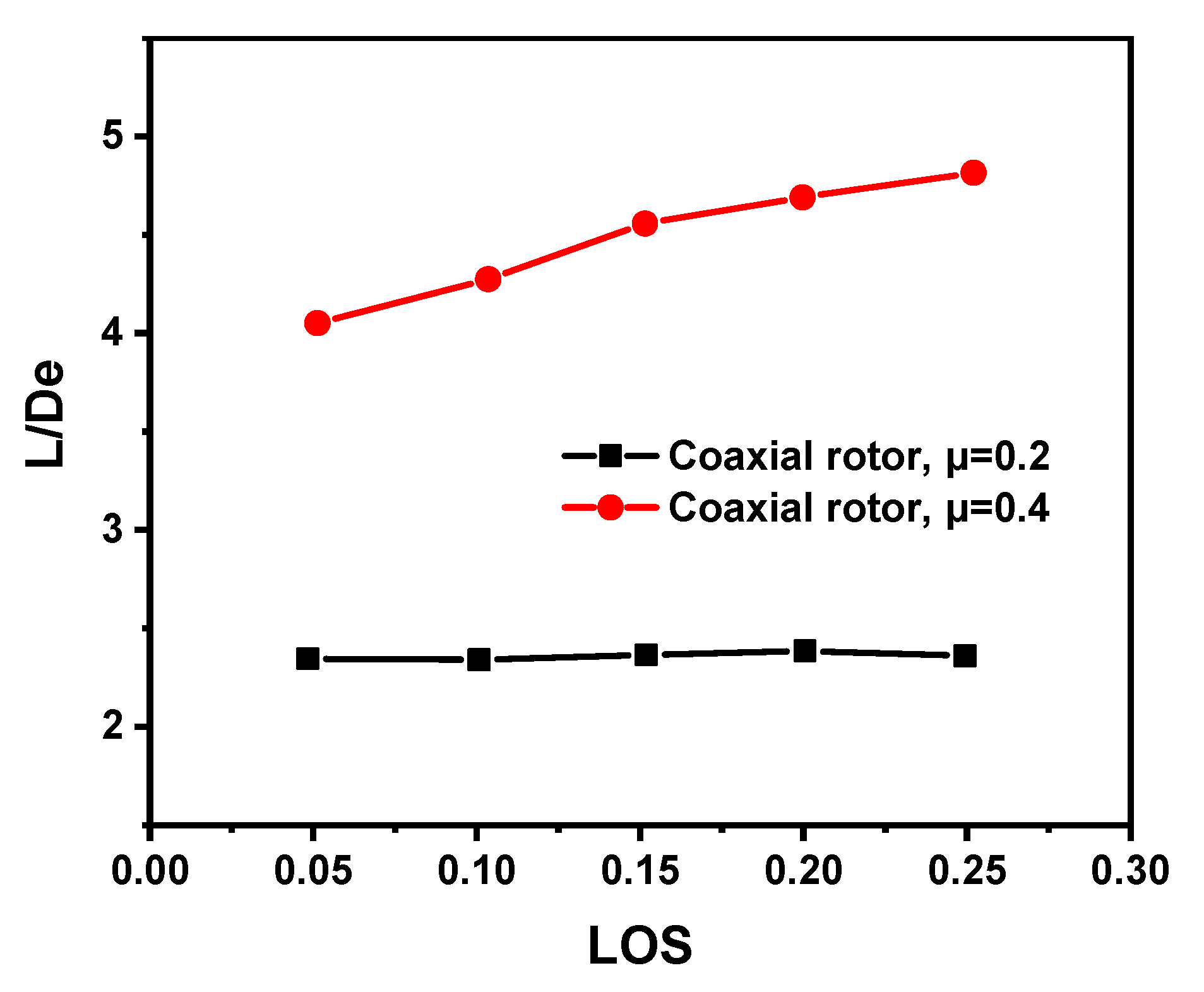
- The effective L/De ratio of the coaxial rigid rotor does not monotonously increase as the advance ratio increases. The increases of the required power and drag in the case with a high advance ratio of 0.6 led to the decreasing L/De ratio of the rotor system with an advance ratio of above 0.4. Moreover, the L/De ratio of the rotor is relatively high when the rotor shaft is tilted backward.
- (3)
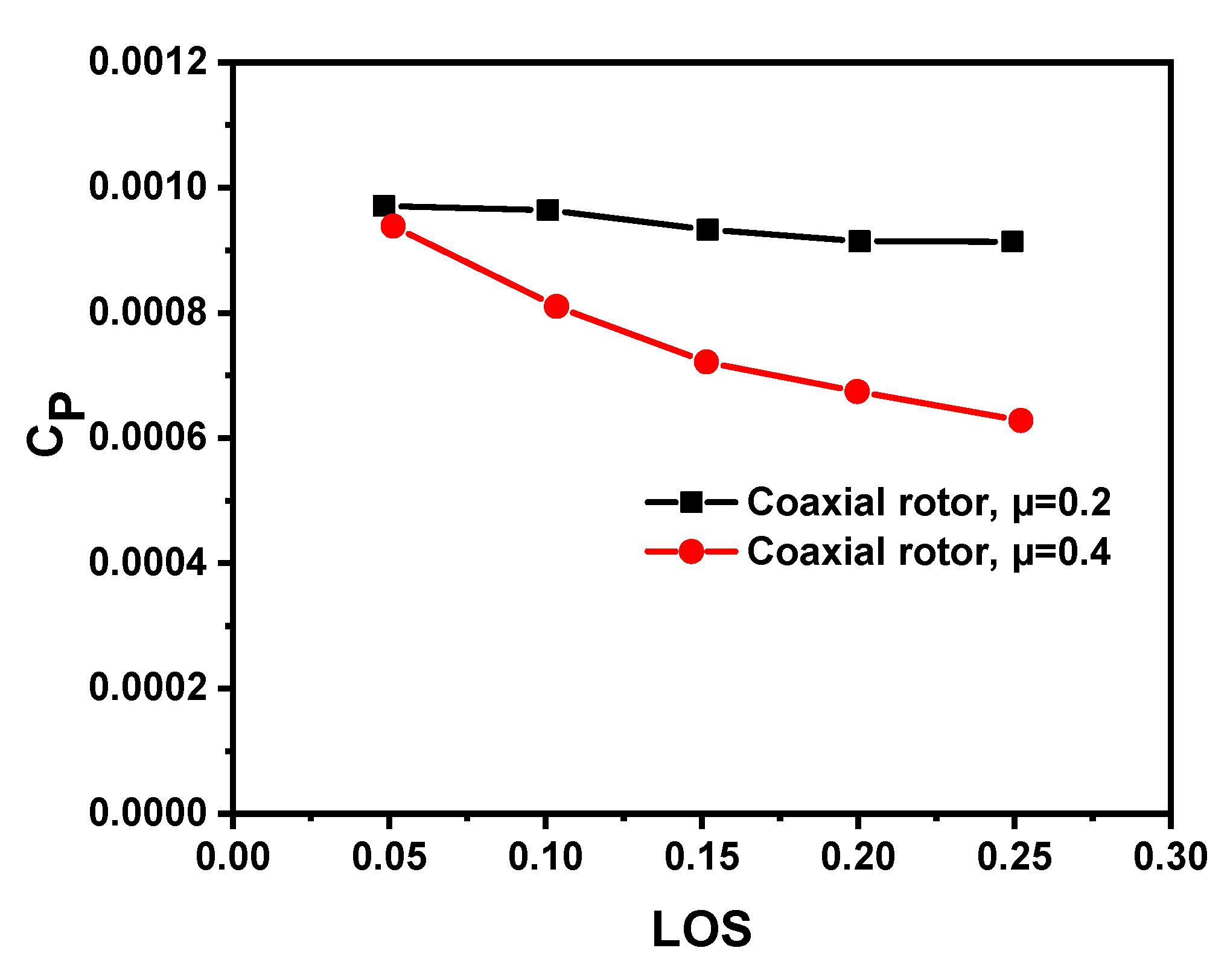
- The increase in lift offset will reduce the total pitch whilst maintaining the same rotor lift, resulting in a decrease in the power required by the rotor. Moreover, the rotor drag is increased when there is an increase in the attack angle of the forward edge blade in the high dynamic pressure area of the advancing side. When the effect of the reduced rotor power is greater than that of the increased rotor drag, the L/De ratio increases as the lift offset increases. At a lower advance ratio, forward efficiency does not obviously benefit from the increasing lift offset and it even decreases when the lift offset becomes exceedingly high. At a higher advance ratio of 0.4, the benefit of the decreased rotor power is greater than the effect of the increased rotor drag, and the rotor obtains approximately 20% better overall forward efficiency with increased lift offset.
- (4)
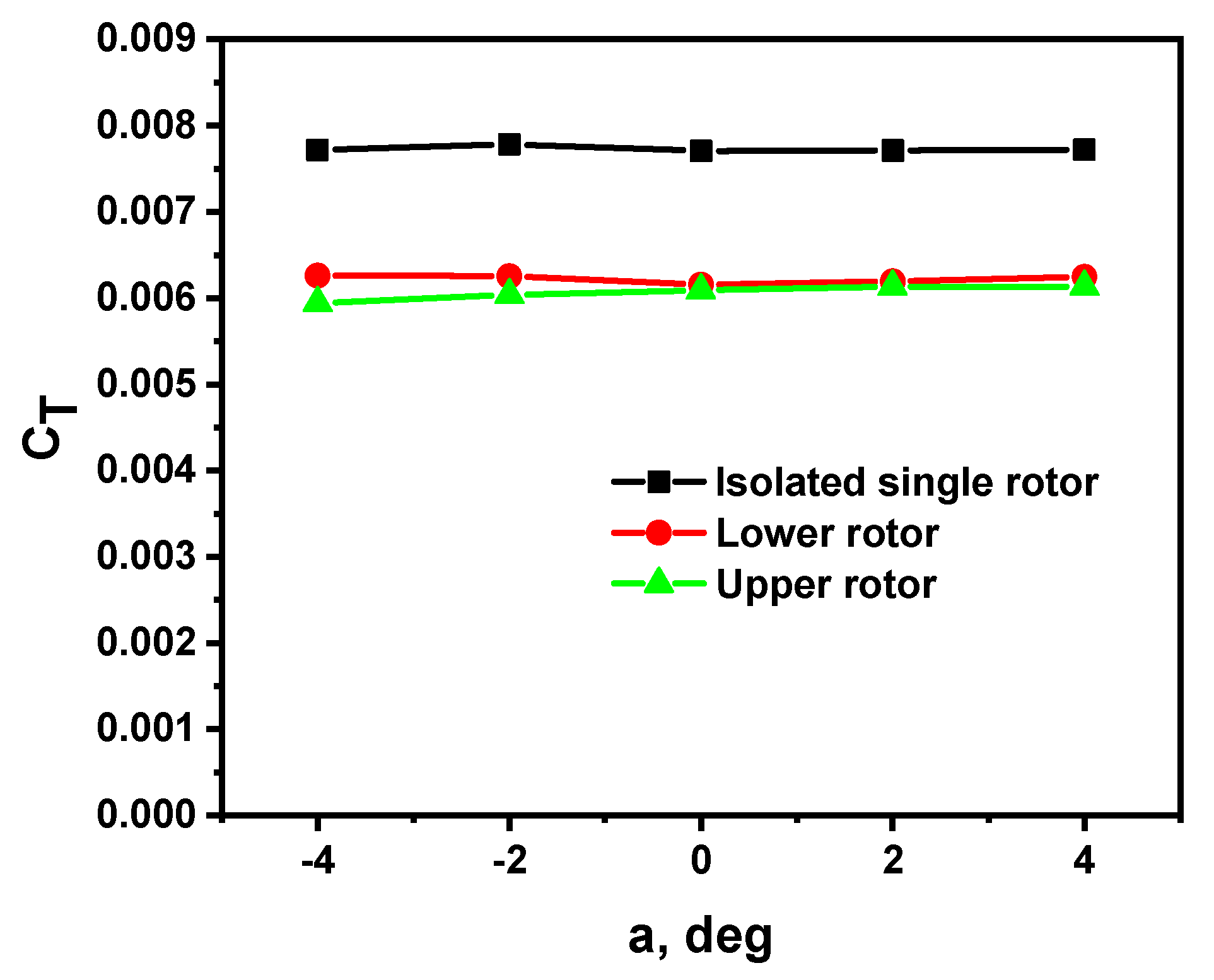
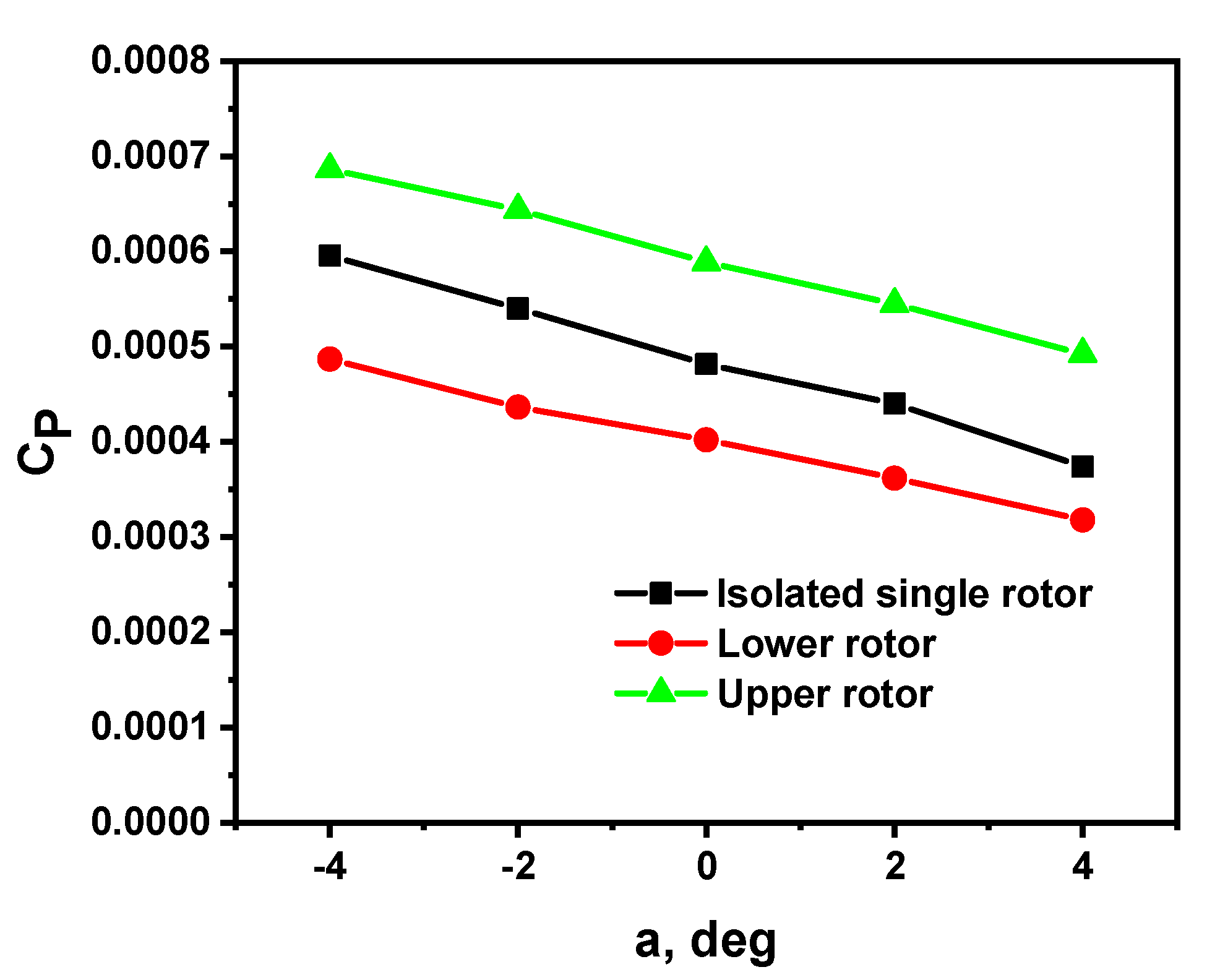
- In the forward flight state, the L/De ratios of the upper and lower rotors are smaller than that of the isolated single rotor and that of the upper rotor is lower than that of the lower rotor. The difference in the forward state’s efficiency of upper and lower rotors is significantly different from the case in the hover flight state.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| T | Rotor thrust force, N |
| D | Rotor drag force, N |
| L | Rotor lift force, N |
| P | Rotor power, W |
| Rotor rolling moment, N·m | |
| ρ | Air density, kg/m3 |
| R | Rotor radius, m |
| α | Rotor shaft tilt angle, deg. |
| σ | Rotor solidity |
| Rotor thrust coefficient | |
| Rotor power coefficient | |
| Rotor lift force coefficient | |
| Rotor drag force coefficient | |
| Rotor rolling moment coefficient | |
| LOS | Lateral lift offset |
| L/De | Effective lift-to-drag ratio |
| FM | Figure of merit |
| /σ | Blade loading coefficient |
| μ | Advance ratio |
| ρ | Air density, kg/m3 |
| ω | Rotor angular velocity, rad/s |
| Ψ | Blade azimuthal angle, deg. |
| Blade pitch angle of upper rotor, deg. | |
| Blade pitch angle of lower rotor, deg. | |
| Coupled collective pitch, deg. | |
| Coupled longitudinal cyclic pitch, deg. | |
| Coupled lateral cyclic pitch, deg. | |
| Differential collective pitch, deg. | |
| Differential longitudinal cyclic pitch, deg. | |
| Differential lateral cyclic pitch, deg. | |
| Collective pitch of upper rotor, deg. | |
| Longitudinal cyclic pitch of upper rotor, deg. | |
| Lateral cyclic pitch of upper rotor, deg. | |
| Collective pitch of lower rotor, deg. | |
| Longitudinal cyclic pitch of lower rotor, deg. | |
| Lateral cyclic pitch of lower rotor, deg. | |
| Rotor phase lag angle, deg. | |
| Φ | Diameter |
| Subscripts | |
| up | Upper rotor |
| lo | Lower rotor |
| hub | Rotor hub |
| ∞ | Free-stream conditions |
References
- Leishman, G.J. Principles of Helicopter Aerodynamics, 2nd ed.; Cambridge University Press: Cambridge, UK, 2016. [Google Scholar]
- Blackwell, R.; Millott, T. Dynamic Design Characteristics of the Sikorsky X2 TD Aircraft. In Proceedings of the American Helicopter Society 65th Annual Forum, Montréal, QC, Canada, 29 April–1 May 2008. [Google Scholar]
- Ruddell, A.J. Advancing Blade Concept (ABC) Development. J. Am. Helicopter Soc. 1976, 22, 13–23. [Google Scholar] [CrossRef]
- Cheney, M.C. The ABC Helicopter. J. Am. Helicopter Soc. 1969, 14, 10–19. [Google Scholar] [CrossRef]
- Paglino, V.M.; Beno, E.A. Full-Scale Wind-Tunnel Investigation of the Advancing Blade Concept Rotor System; United Technologies Corp, Sikorsky Aircraft Div: Stratford, CT, USA, 1971. [Google Scholar]
- Felker, F.F. Performance and Loads Data from a Wind Tunnel Test of a Full-Scale, Coaxial, Hingeless Rotor Helicopter; National Aeronuatics and Space Administration Ames Research Center: Moffett Field, CA, USA, 1981.
- Cameron, C.G.; Sirohi, J. Performance and Loads of a Lift Offset Rotor: Hover and Wind Tunnel Testing. J. Am. Helicopter Soc. 2019, 64, 1–12. [Google Scholar] [CrossRef]
- Cameron, C.; Sirohi, J. Performance and loads of a model coaxial rotor part II prediction validations with measurements. In Proceedings of the AHS 72nd Annual Forum, West Palm Beach, FL, USA, 17–19 May 2016. [Google Scholar]
- Cameron, C.G.; Uehara, D.; Sirohi, J. Transient Hub Loads and Blade Deformation of a Mach-Scale Coaxial Rotor in Hover. In Proceedings of 56th AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, AIAA SciTech, Kissimmee, FL, USA, 5–9 January 2015. [Google Scholar]
- Lorber, F.P.; Law, G.K.; O’Neill, J.J.; Matalanis, C.; Bowles, P. Overview of S-97 RaiderTM Scale Model Tests. In Proceedings of the American Helicopter Society 72nd Annual Forum, West Palm Beach, FL, USA, 17–19 May 2016. [Google Scholar]
- Lorber, F.P.; Bowles, P.; Fox, E.; Wang, Z.K.; Hein, B.; Mayrides, B. Wind tunnel testing for the SB > 1 DEFIANT™ joint multi-role technology demonstrator. In Proceedings of the AHS International 73rd Annual Forum & Technology Display, Fort Worth, TX, USA, 9–11 May 2017. [Google Scholar]
- Ruddell, A.; Groth, W.; McCutcheon, R. Advancing Blade Concept (ABC) TM Technology Demonstrator; Technical Report USAVRADCOM-TR-81-D-5; U.S. ArmyResearch and Technology Labotratories (AVRADCOM): Maryland, MD, USA, 1981. [Google Scholar]
- Yeo, H.; Johnson, W. Investigation of Maximum Blade Loading Capability of Lift-Offset Rotors. J. Am. Helicopter Soc. 2014, 59, 1–12. [Google Scholar] [CrossRef]
- Bagai, A. Aerodynamic Design of the X2 Technology Demonstrator Main Rotor Blade. In Proceedings of the American Helicopter Society 64th Annual Forum, Alexandria, VA, USA, 29 April–1 May 2008. [Google Scholar]
- Leishman, J.G.; Ananthan, S. Aerodynamic optimization of a coaxial proprotor. In Proceedings of the 62th American Helicopter Society Annual Forum, American Helicopter Society, Phoenix, AZ, USA, 9–11 May 2006; Volume 1, p. 64. [Google Scholar]
- Rand, O.; Khromov, V. Aerodynamic optimization of coaxial rotor in hover and axial flight. In Proceedings of the 27th International Congress of the Aeronautical Sciences, Nice, France, 19–24 September 2010; pp. 1–13. [Google Scholar]
- Leishman, J.G.; Rosen, K.M. Challenges in the aerodynamic optimization of high-efficiency proprotors. J. Am. Helicopter Soc. 2011, 56, 12004. [Google Scholar] [CrossRef]
- Syal, M.; Leishman, J.G. Aerodynamic optimization study of a coaxial rotor in hovering flight. J. Am. Helicopter Soc. 2012, 57, 1–15. [Google Scholar] [CrossRef]
- Saetti, U.; Enciu, J.; Horn, J.F. Performance and design optimization of the f-helix evtol concept. In Proceedings of the Vertical Flight Society’s 75th Annual Forum and Technology Display, Philadelphia, PA, USA, 13–16 May 2019. [Google Scholar]
- Syal, M. Contributions to the Aerodynamic Optimization of a Coaxial Rotor System. Master’s Thesis, University of Maryland, College Park, MD, USA, 2008. [Google Scholar]
- Knight, M.; Hefner, R.A. Static Thrust Analysis of the Lifting Airscrew; NASA Technical Report NACA TN 626; NASA: Washington, DC, USA, 1937. Available online: https://ntrs.nasa.gov/citations/19930081433 (accessed on 25 July 2021).
- Berry, B.; Chopra, I. High-Advance Ratio Wind Tunnel Testing of a Model Rotor with Pressure Measurements. In Proceedings of the 5th Decennial AHS Aeromechanics Specialists’ Conference Proceedings, San Francisco, CA, USA, 22–24 January 2014. [Google Scholar]
- Wang, X.; Trollinger, L.; Chopra, I. Refined Performance Results on a Slowed Mach-Scaled Rotor at High Advance Ratios. J. Am. Helicopter Soc. 2020, 65, 1–13. [Google Scholar] [CrossRef]
- Wang, X.; Bauknecht, A.; Maurya, S.; Chopra, I. Slowed Hingeless Rotor Wind Tunnel Tests and Validation at High Advance Ratios. J. Aircr. 2021, 58, 153–166. [Google Scholar] [CrossRef]






| Measurement Parameters | Maximum Capacity | Measured Standard Deviation of Error | |
|---|---|---|---|
| Value | % Capacity | ||
| Normal force or lift, N | 2200 | 0.66 | 0.03 |
| Side force, N | 500 | 0.3 | 0.06 |
| Axial force or drag, N | 500 | 0.25 | 0.05 |
| Pitching moment, N·m | 200 | 0.08 | 0.04 |
| Rolling moment, N·m | 250 | 0.05 | 0.02 |
| Torque, N·m | 340 | 0.27 | 0.08 |
| Rotor Parameters | Value |
|---|---|
| Rotor radius, R (m) | 1 |
| Root cut out (m) | 0.18 |
| Number of blades | 8 (4 for the upper rotor and 4 for the lower rotor) |
| Chord (m) | 0.07 |
| Twist angle (°) | −12 |
| Plan form | Untapered |
| Precone angle (°) | 2 |
| Rotational direction | Counterclockwise for the upper rotor, clockwise for the lower rotor. |
| Solidity, σ | 0.178 (coaxial rotor) |
| Airfoil | NACA0026, NACA0020, and NACA0012 |
| Nominal rotation speed | 1860 RPM |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, C.; Huang, M.; Peng, X.; Zhang, G.; Tang, M.; Wang, H. Wind Tunnel Studies on Hover and Forward Flight Performances of a Coaxial Rigid Rotor. Aerospace 2021, 8, 205. https://doi.org/10.3390/aerospace8080205
Wang C, Huang M, Peng X, Zhang G, Tang M, Wang H. Wind Tunnel Studies on Hover and Forward Flight Performances of a Coaxial Rigid Rotor. Aerospace. 2021; 8(8):205. https://doi.org/10.3390/aerospace8080205
Chicago/Turabian StyleWang, Chang, Minqi Huang, Xianmin Peng, Guichuan Zhang, Min Tang, and Haowen Wang. 2021. "Wind Tunnel Studies on Hover and Forward Flight Performances of a Coaxial Rigid Rotor" Aerospace 8, no. 8: 205. https://doi.org/10.3390/aerospace8080205
APA StyleWang, C., Huang, M., Peng, X., Zhang, G., Tang, M., & Wang, H. (2021). Wind Tunnel Studies on Hover and Forward Flight Performances of a Coaxial Rigid Rotor. Aerospace, 8(8), 205. https://doi.org/10.3390/aerospace8080205





