Analytical Study on Lift Performance of a Bat-Inspired Foldable Flapping Wing: Effect of Wing Arrangement

Abstract

In this work, we use a three-dimensional computational fluid dynamics (CFD) simulation to comprehend how the two wing arrangement variables, i.e., inner/outer wing proportion and mid-stroke dihedral, affect the lift characteristic of a bat-inspired span foldable flapping wing. The employed flapping mechanism is based on previous work. In this study, the structure parameters of the flapping mechanism remain unchanged across all simulations. Based on the CFD results, the tendency and work point regarding maximum lift generation can be found by changing both of the variables. As a result, when modifying the inner/outer wing proportion without changing the total wing shape and area, the maximum time-averaged lift appears in the case of the inner wing occupying half of the semi-span. In addition, when changing the dihedral, the maximum time-averaged lift was obtained when the inner wing dihedral was equal to zero. To discuss the lift variation of the foldable flapping wing, pressure distribution and vorticity of the flow field at certain time points were provided corresponding to the instantaneous lift curves. The conclusions of this research are able to help understand the wing arrangement of birds and bats issued from natural selection, and also support the future design of flapping wing micro-aerial-vehicles.

1. Introduction

Birds and bats have shown superior flight performance because of the high aerodynamic efficiency at low Reynolds numbers. The unsteady aerodynamics of the flapping wings is one of the most important references for flapping wing aerial vehicles (FWAVs) design. Substantial research has been carried out on understanding the flight mechanism of flying creatures as well as FWAVs. In order to understand the flight mechanism of flying birds and bats, computational fluid dynamic (CFD) simulations and wind tunnel tests have been performed [1,2,3,4]. Mulijres et al. [1] measured the flapping motion of a small nectar-feeding bat with the digital particle image velocimetry (DPIV) technic. Strong leading-edge vortices (LEV) can be observed at the front of the wing which contributes more than 40% of the total lift. Warrick and Tobalske [2] also equipped the DPIV technic to investigate the wake structure and dynamics of hummingbirds at different flight speeds. Riskin et al. [3] made a comparison of flight kinematics of 27 bats in six pteropodid species. The research provided the relationship of parameters including lift coefficient, wing flapping period, and angle of attack with respect to body mass. Power input and aerodynamic force analysis during the flapping period was proceeded with the simplified flapping robotic bat wing designed by Behlman et al. [4]. The robotic wing consists of a 2 degrees of freedom (2-DOF) scapula and a 1-DOF (flexion/extension) elbow and wrist. The energy input and aerodynamic force output of the flapping wing were able to measure directly. In terms of the aerodynamic characteristics of FWAVs, many researchers analyzed the influence factors [5,6,7]. The performance influenced by wing shape, aspect ratio, angle of attack, was analyzed by Gong et al. [5]. Mazaheri and Ebrahimi [6] who performed a wind tunnel test to discover the relationship of the lift and thrust with respect to angles of attack and flapping frequencies at four different flight speeds. Yang [7] carried out a fluid–structure interaction analysis and designed a bird-like FWAV, which achieved a fully autonomous flight.

Besides the traditional factors that influence the aerodynamics of flapping wings as summarized above, Shkarayev and Silin [8] performed the analysis of two flapping wing models with variables, dihedral and bending stiffness, of the single degree of freedom flapping wings. The effect of these variables on aerodynamic force generation was investigated through experimental tests. Wolf et al. [9] proposed research into the kinematics and aerodynamics of flying bats by wind tunnel tests with help of high-speed photography. Three main factors which most affect the aerodynamic characteristics of the flying bats were studied, including flight speed, downstroke ratio, and span ratio. The span ratio is defined as the ratio of wingspan during mid-downstroke and mid-upstroke. A higher span ratio means lower negative lift during upstroke, resulting in a higher average lift in one flapping cycle. Wing folding is one major solution to achieve a higher span ratio during flapping. Through the published works to date, there are two main ways to achieve wing folding. One way is to employ a structure with an electric servomechanism to retract/protract the wing periodically. Ramezani et al. [10,11] designed a bat-inspired UAV named Bat Bot (B2). The wing mechanism had 5-DOFs and was able to achieve symmetric and asymmetric motion, which gave the UAV the ability to process more flexible maneuvers during flight. They also demonstrated a fabricated prototype, which was able to achieve indoor flight, including straight flight, diving, and bank turns. This kind of structure can process the asymmetrical maneuver but will add more complexity to the control system. The other way to achieve wing folding is to design a purely mechanical structure to enable outer wing folding. Many works have published design concepts of foldable wing structures [12,13,14]. Ryu et al. [12] and Hou et al. [13] proposed a similar kind of structure that can achieve a asymmetry flapping kinematic. Experiment results showed that the structure provided a higher average lift compared with a non-folding flapping wing.

In terms of the optimization of folding wing structures, Ryu et al. [12] performed an amplitude optimization after the design of a folding mechanism. Nevertheless, compared with the wing arrangement of flying creatures, many key parameters regarding the flapping wing design have been rarely studied. For example, wing folding during upstroke can obviously decrease negative lift, but does more folding area during upstroke, mean more average lift produced in a flapping cycle? Besides the factors such as flight speed, angle of attack (AOA), flapping frequency, and flapping amplitude, are there any other factors that can play an important role in the aerodynamic characteristics during flapping? Focusing on the above problems, in this paper, two wing arrangement variables, inner/outer wing proportion and mid-stroke dihedral, were selected for analysis. We considered a numerical approach by the use of unsteady three-dimensional CFD simulations. The simulation model and CFD method are introduced in Section 2. Simulation results and analytical discussion are provided in Section 3. The tendency and work point regarding maximum lift generation were found by changing both of the variables through simulations. Pressure distribution and vorticity of the flow field at specific time points were also provided corresponding to the instantaneous lift results to further discuss the lift variation of the foldable flapping wing. The main conclusions from this work are given in Section 4. The research can help to understand the wing arrangement of birds and bats issued from natural selection, and also support the future design of FWAVs.

2. Numerical Simulation Method

2.1. Model Design

The CAD model built for simulation is shown in Figure 1. A bat-inspired wing was designed with inner and outer wing segments. The semi-span length of the wing was 0.2 m and the area of the half-wing was 0.0125 m². The inner/outer wing proportion can be modified for different analyses. The flapping mechanism was designed based on the research of Ryu et al. [12], whose mechanism could achieve an outer wing enfolding during upstroke, which could improve the average lift in one flapping cycle. The flapping angle of inner and outer wings were derived from cosine law. The schematic of the flapping mechanism is shown in Figure 2, where L₁ is the input link and L₄ is the output link. The inner wing and outer wing surfaces in Figure 1 adhered with L_inner and L_outer. The length of L₁–L₄ and the coordinates of points A and O are the main factors of the flapping design. In this work, we defined the coordinate of point O as (0, 0) and the position of point A as (y_AO, z_AO), the values of other influence factors are given in

Table 1. Parameters of the flapping mechanism.

Structural Parameters	Length (mm)
L1	7
L2	11.17
L3	8
L4	11
yAO	7
zAO	11

.

Previous works have found that the flexibility of the wing has an obvious contribution to thrust but very little effect on lift. [7,15,16] Meanwhile, the rigid wing model has already been employed in the design process of many published FWAVs, [17,18] so the simplified rigid wing model was used for simulations. The flapping motion of this research was derived from cosine law based on Ryu et al. [12]. In order to easily implement the motion into the simulation software, fourth-order Fourier functions were employed to reflect the flapping angle of both inner and outer wings. Equations of motions are provided as follows

$$\begin{array}{c}\hfill {\theta }_{inner}=0.1632+0.2307\cos \left(t·\omega \right)-0.6398sin\left(t·\omega \right)+0.1331\cos \left(2t·\omega \right)+0.0495\sin \left(2t·\omega \right)\\ \hfill +0.0205\cos \left(3t·\omega \right)+0.0486\sin \left(3t·\omega \right)-0.0134\cos \left(4t·\omega \right)+0.0141sin\left(4t·\omega \right)\end{array}$$

(1)

$$\begin{array}{c}\hfill {\theta }_{outer}=-0.6787+0.6647\cos \left(t·\omega \right)+0.0659sin\left(t·\omega \right)+0.1219\cos \left(2t·\omega \right)+0.0723\sin \left(2t·\omega \right)\\ \hfill -0.0088\cos \left(3t·\omega \right)+0.0508\sin \left(3t·\omega \right)-0.0163\cos \left(4t·\omega \right)+0.0105sin\left(4t·\omega \right)\end{array}$$

(2)

$$\omega =2\pi f$$

(3)

where, ${\theta }_{inner}$ and ${\theta }_{outer}$ are the flapping angle of inner and outer wings in radians; $\omega$ is the angular frequency and f is the flapping frequency. The schematic of the flapping motion is shown in Figure 3, the angle as well as angular velocity around the x-axis are defined to be positive in the anti-clockwise direction.

Based on the research of Bullen and McKenzie [19], according to the designed wing area and wing span, the flapping frequency of the model was selected to be 10 Hz and the flight speed was selected to be 7 m/s. The Reynolds number was calculated as follows:

$$Re=\frac{\rho V\overline{c}}{\mu }=30109$$

(4)

where V is the flight speed, $\overline{c}$ is the mean aerodynamic chord, $\rho =1.225\mathrm{kg}/{\mathrm{m}}^3$ is the air density and $\mu =1.78\times {10}^{-5}\mathrm{Pa}·\mathrm{s}$ is the dynamic viscosity.

Some initial discussion had been made based on previous research. First, the flapping mechanism employed in this research, designed by Ryu et al. [12], had an asymmetry flapping period, which meat the period of upstroke and downstroke was unequal. Define the dimensionless time t* = time · f. As can be obtained from Figure 3b,c, the upstroke period of the inner and outer wing was t* = 0−0.45 and t* = 0.25−0.7, respectively. The upstroke period was about 55% of the total flapping period. According to Wolf et al. [9] and Chen et al. [20], when the downstroke period occupies less time compared with the upstroke period in one flapping cycle, the average flapping speed during downstroke is faster than that in upstroke, and the aerodynamic lift can experience a certain increase. Thus, the flapping wing could gain more in lift from the asymmetric feature. Second, there is a stable phase lag between the inner and outer wings. The phase lag of the outer wing relative to the inner wing segment was about $\pi /2$, this caused the inner and outer wings to have different upstroke and downstroke periods, as shown in Figure 3b. In order to make the analysis clear, in this paper, the upstroke and downstroke period of the inner wing was selected for separation of the period of the model wing flapping, because the motion of the outer wing can be treated as an additional motion for lift enhancement.

2.2. Simulation Setup

Three dimensional CFD simulation was conducted to investigate the unsteady aerodynamics of the flapping wing UAV. The simulation environment was established by using the software Ansys Fluent v18.2. A rectangular far field was applied as the computational domain. The size of the inlet and outlet was 25 × 50 $\overline{c}$ and the length of the field in the flow direction was 50 $\overline{c}$. Boundary conditions of the far field and the wing surfaces were velocity inlet, pressure outlet, and no-slip wall. The symmetry boundary at y = 0 was used in order to reduce the computational cost. An unstructured grid was adopted as shown in Figure 4.

The unsteady pressure solver was employed in the simulation. Incompressible Reynolds-average Navier-Strokes (RANS) equations were solved by using the finite volume method with a SST k-ω turbulence model [18,21,22]. The SIMPLE scheme was employed for pressure–velocity coupling. The spatial discretization with the second-order upwind scheme and the transient formulation with first-order implicit scheme were adopted. The grid generation method as well as the solver have been employed many times, and validation tests can be found in our previous works [18,21,22].

Grid and time-step convergence studies were performed. Three types of grids and three types of time-step sizes were tested. It was found that there was no obvious difference in the instantaneous lift with the three types of grids as well as time-step sizes, as shown in Figure 5. The time-averaged lift for all validation cases are provided in

Table 2. Time-averaged lift results of the convergence studies.

Validation Cases			Lift (N)	Difference
No.	Grid Number	Time Step (s)
1	1.7 million (Medium)	0.0002	0.2313
2	1.2 million (Coarse)	0.0002	0.2324	0.47%
3	2.6 million (Fine)	0.0002	0.2310	−0.15%
4	1.7 million (Medium)	0.0005	0.2285	−1.22%
5	1.7 million (Medium)	0.0001	0.2319	0.23%

. The maximum difference of the average lift of all cases was only 1.22%, which is negligible in this analysis. Finally, the total number of grids we selected for all cases was around 1.7 million. The minimum grid size on the wing surface was 0.015 $\overline{c}$. The time step size 0.0002 s was selected for the unsteady simulation of the transient flapping motion.

The wing kinematics were defined in simulation cases followed by Equations (1)–(3) as described in Section 2.1. The dynamic mesh was employed for transient simulation of the flapping wing. User-defined functions (UDF) were activated to simulate the flapping motions of the inner and outer wings. The motion of the inner wing was pure rotation relative to the origin point, while the motion of the outer wing consisted of both rotation and translation relative to the tip chord of the inner wing. Since the wing model was considered to be rigid, the CG motion function was selected for the UDF script file which contained the angular velocity of the inner and outer wings. The angular velocity of the inner wing was calculated by taking the derivative of Equation (1). The motion of the outer wing was obtained by setting the inner wing as the relative zone in the dynamic mesh module, the relative angular velocity of the outer wing was then equal to the difference between the angular velocity of the outer and inner wings. The diffusion smoothing and the remeshing functions were activated to ensure the quality of the nearby meshes were maintained during the movement of the flapping wing.

3. Results and Discussion

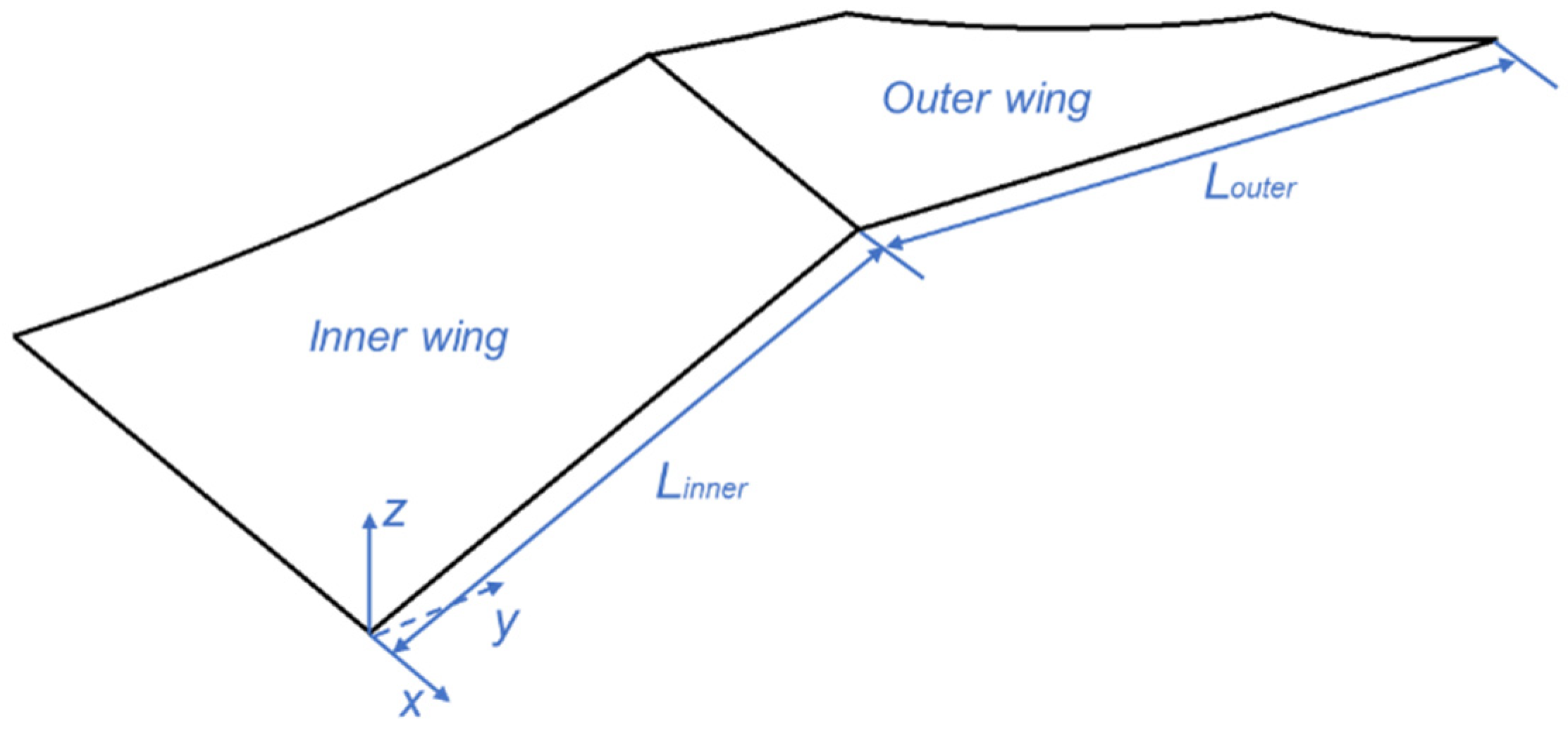
3.1. Effect of Inner/Outer Wing Proportion

From the instantaneous lift of flapping wings published in previous studies, it can be observed that positive lift is generated during downstroke of a cycle, while the upstroke will generate negative lift, resulting in a reduction in average lift [15,18,21]. In nature, birds and bats usually reduce the wing area in the process of upstroke by folding the outer wing. Such a design can directly reduce the negative lift. Intuitively, it seems that the larger the folded area is, the more the reduction in the negative lift. However, this does not seem to be the case. According to the research of [23,24], statistics of the span proportion of the inner and outer wings of several bat wings found that the proportion of the span of the inner wings were all about 50%. Based on this phenomenon, this paper firstly analyzed the influence of the inner/outer wingspan proportion change on the lift performance of the flapping wing.

Table 3. List of cases for analysis of the effect of inner/outer wing proportion.

Case No.	Span Proportion		Area Proportion
	Inner Wing	Outer Wing	Inner Wing	Outer Wing
1.1	30%	70%	41.0%	59.0%
1.2	40%	60%	54.1%	45.9%
1.3	50%	50%	65.9%	34.1%
1.4	60%	40%	76.9%	23.1%
1.5	70%	30%	86.7%	13.3%

gives the numbers defined for five cases in subsequent analysis.

Figure 6 shows the average lift of the flapping wing in one flapping cycle. The reason we selected the span proportion but not the area proportion of the inner and outer wings for discussion was because there is a more obvious dependency between span proportion and the average lift of the flapping wing. According to the data in Table 3 and Figure 6a, there was a clear division when considering the span extent of the inner wing: when the inner wing span proportion was lower than the outer wing (inner/outer wing ratio < 1), the average lift increased with the increase in inner wing proportion, and vice versa. As for area proportion, there was no such clear correlation. When the proportion of the inner wing area to the total area exceeded that of the outer wing (case 1.2), the average lift still increased. The peak of the average lift with respect to the area ratio did not appear on a typical value (inner/outer wing area ratio = 1.933). Therefore, the span proportion was selected for discussion in the following content.

It can be seen from Figure 6b that when the AOA was equal to 0° and 6°, the average lift achieved the maximum value when the inner wing occupied 50% of the total span. With an increase in the AOA, the average lift of arrangements with a higher inner wing proportion increased faster. For an AOA that was equal to 12°, the average lift had almost no difference after the inner wing proportion exceeded 50%. For example, the average lift in the case of a 70% inner wing proportion was only 0.43% different from that in the case of a 50% inner wing proportion. Based on the results it can be concluded that cases where the inner wing span occupied 50% of the total wing span had the best lift characteristic when the AOA was in the range from 0° to 12°, which is the range of the AOA the bird- and bat-inspired FWAVs are normally in [7,18].

In order to further discuss the reason for the lift change caused by variation of the proportion of the inner and outer wings, considering cases with the angle of attack of 6° as example, Figure 7 gives the instantaneous lift with respect to the non-dimensional time t* (t* = t/T, where t is time and T is the flapping period). The sixth flapping cycle of the instantaneous lift in the simulation was extracted for analysis because the lift curve showed good convergence characteristics after calculating six periods. It was found that the trend of the instantaneous lift of this flapping mechanism in one flapping cycle was significantly different from that of the single degree of freedom flapping wings which normally had an instantaneous lift curve similar to the sinusoidal function in previous results [18,21]. The instantaneous lift curve obtained in this study had an obvious positive peak between t* = 0−0.45, but no deep negative lift trough: two small negative trough time points were observed between t* = 0.45−1 in the process of the upstroke. The lift curves of the flapping process have an obvious asymmetrical characteristic.

As can be seen from Figure 7, first, with the increase of the inner wingspan proportion from 30% to 70%, the time to reach the peak during downstroke was slightly delayed with the increase of the proportion of the outer wings. The maximum positive lift increases gradually with the increase in the inner wing proportion, and the maximum value (in case 1.5) increases by 6.8% compared with the minimum value (in case 1.1).

To further analyze the reason for the change of lift in one flapping cycle, the vorticity of the flow field was calculated based on the Q criterion [25]. Figure 8 shows the vorticity of Q = 15,000 at t* = 0.2 and 0.35. This Q value was adopted for all vortices structure plots shown in the following content because it offered the best clarity. Combined with the angular velocity provided in Figure 2c, when t* = 0.2, the inner wing begins the rapid downward flapping process, and from the corresponding Figure 8a–c it can be seen that the leading edge of the inner wing has begun to generate obvious LEV, but because the outer wing is still in the upstroke at this time, there is no obvious LEV on the upper surface of the outer wing. Therefore, because the inner wing in case 1.4 is longer, a more significant LEV structure is developed, resulting in a higher lift. When t* = 0.35, as shown in Figure 8d,f, there are still stable LEV structures on the upper wing surface of case 1.1 due to it having a shorter inner wing span. For cases 1.3 and 1.4 with a larger inner wingspan, the LEV on the outer wing has been separated into shed vortices, which can no longer provide lift increases for the flapping wing. This earlier separation phenomenon is due to the larger inner wingspan resulting in a higher linear downward flapping velocity at the inner wing tip, which will transmit to the outer wing as a translation motion as described in Section 2.2. This is why the linear flapping speed of the outer wing in cases 1.3 and 1.4 exceed the speed which can keep a stable LEV and result in the separation of the LEV. Therefore, the instantaneous lift of models with a larger inner wingspan have entered into a downward trend at this time point.

Second, it can be seen that during the upstroke (t* = 0.45 − 1), although the negative trough of the instantaneous lift is far smaller than the positive peak, the relative change of the instantaneous lift with respect to the variation of the inner/outer wingspan proportion is still obvious. The change of negative lift can be divided into two typical stages. The first stage is t* = 0.45 − 0.85. During this period, with the increase of the proportion of the inner wingspan, the downward trend of lift becomes rapid, and all cases listed in Table 3 produced local minimum points in this process (near t* = 0.65). The second stage is t* = 0.85 − 1, where the trend of lift is just opposite to that of the first stage. With the increase of the proportion of the inner wingspan, the aerodynamic lift rises rapidly, and the instantaneous lift of case 1.1 turns positive firstly. At this stage, arrangements with an inner wingspan less than 50% show a second trough (at around t* = 0.95).

To further investigate the generation of the lift trough near the two time points t* = 0.65 and 0.95, Figure 9 shows the vorticity at the corresponding times. It can be seen from Figure 9a–c that when t* = 0.65, the circumstance is just opposite to that when t* = 0.2: the inner wing is in the process of accelerating the upstroke, so a strong LEV is generated on the lower surface of the inner wing. The larger the span proportion of the inner wing, the stronger the LEV is, so the greater the negative lift generated. At the same time, it can be seen from Figure 9b,c that there are shedding vortices near the leading edge of the outer wing section, which may be the LEV generated in previous time points.

In order to prove this hypothesis, Figure 10 shows the vorticity at time t* = 0.55, in which the leading-edge vortices structure can be observed at the leading edge of the outer wing. This is because for arrangements with the inner wing segment occupying a longer proportion, the outer wing has inherited a large z-direction translation speed due to the large linear velocity at the tip of the inner wing. Therefore, although the outer wing is still in the process of the downstroke, it has already started to move upward. This phenomenon is also one of the reasons why the instantaneous lift of the arrangement with a large span proportion of the inner wing decreases faster during the upstroke.

When t* = 0.95, it can be seen from Figure 3 that the inner wing has almost reached the highest point of flapping, which means the angular velocity of the inner wing approaches zero at this time, but the outer wing is still in the process of accelerating flapping up at this time. It can be seen from Figure 9d, f that case 1.1 produces strong LEV near the wing tip, but the strength of the LEV near the tip of the other cases gradually decreases as the proportion of the outer wing decreases, thus no strong negative lift is produced in this period.

To further analyze the contribution of the inner and outer wings to the lift generated during the flapping process, the instantaneous lift and lift coefficient curves of the inner and outer wings are given in Figure 11 separately. During downstroke, there are obvious lift peaks for both the inner and outer wings, which means both wings have made a contribution to the positive lift. However, in comparison, the contribution of the outer wing segment is slightly larger than that of the inner wing. From curves of lift coefficient, it can be seen that the maximum positive lift coefficient of the outer wing section during downstroke is about 2.06 times that of the inner wing. During upstroke, the contributions of inner and outer wings are obviously different at t* = 0.45−0.85. The negative lift trough near t* = 0.65 is only related to the inner wing, the larger the proportion of the inner wingspan, the greater the negative lift. At t* = 0.95 an obvious negative lift trough appears for the outer wing, while the lift of the inner wing is almost zero at this time. These trends are consistent with the previous analysis results based on vorticity analysis. In addition, through the comparison of the lift and lift coefficient curves, it was found that the trend of the lift and lift coefficient of the outer wing section is opposite. With the increase in the span proportion of the outer wing section, the lift produced by the outer wing gradually decreases while the lift coefficient is increasing. This means the smaller the proportion of the outer wing segment, the higher the efficiency of the lift generating during flapping. However, it can be seen from Figure 6 that a decrease in the span of the outer wing will lead to a decrease in the time-averaged lift when exceeding the threshold. Therefore, when applying this flapping mechanism to the design of FWAVs, further considerations should be made to discuss the relationship between the efficiency and the required lift to select a suitable scheme.

3.2. Effect of Dihedral

As concluded from previous works, flapping frequency, flapping amplitude, AOA, and mid-stroke dihedral are the main factors that affect lift characteristics. Besides of flapping frequency and AOA which have been proved to be linearly related to the lift in the condition of small angle and low frequency [7,18], Ryu et al. [12] performed amplitude optimization in their research, but no research into the effect of dihedral. An upward dihedral has always been employed in the design of FWAVs in previous works [26,27]. Shkarayev and Silin [8] analyzed the effect of three positive dihedrals and found that the adoption of a dihedral was beneficial for lift generation. Previous analyses only focused on single degree of freedom FWAVs, and seldom in foldable flapping wings as discussed in this work.

On the premise that the flapping design is unchanged in one cycle, there are two possible results of lift changing caused by the variation of a dihedral for the specific flapping mechanism that are discussed in this paper. First, the change of dihedral will lead to a change of the projected area of the wing on the normal plane of the lift vector, and then result in the change of lift. The reduction in the projected area, due to the large dihedral, will directly lead to a reduction in the average lift. Second, since the outer wing segment has a large downward flapping angle (more than 90 degrees in specific cases) in the flapping process, it may cause the flapping wing to benefit from the unsteady aerodynamic effect, such as the clap and fling [28,29], and then increase the lift. In order to determine the effect of dihedral variation, further research was carried out. Due to the foldable design, the inner and outer wings have different dihedrals in the neutral position. Because the flapping stroke is defined by the motion of the inner wing, the dihedral of the inner wing was selected as the dihedral of the wing model in the following content for concise expression.

By analyzing the kinematic characteristics of the flapping mechanism, it was found that the upper and lower limits of the amplitude changed by changing the position of point A (Figure 2) while keeping the angular velocity unchanged. This section analyzed the lift characteristics of seven cases with different dihedrals δ. The number of each case and its corresponding dihedral is shown in

Table 4. List of cases for analysis of effect of dihedral.

Case No.	Dihedral, δ (Deg)
	Inner Wing	Outer Wing
2.1	−40	−71
2.2	−20	−51
2.3	−10	−41
2.4	0	−31
2.5	10	−21
2.6	20	−11
2.7	40	9

. Results in the previous section show that the arrangement with the inner wing equipped with 50% of the span always has the maximum lift. Therefore, case 1.3 was selected as the standard case, cases 2.1–2.7 are then established to analyze the influence of the deviation of dihedrals on lift characteristics.

Figure 12 shows the average lift of cases 2.1–2.7 with the change of dihedral. It can be seen that the lift reached the maximum in case 2.4. The average lift decreased when the dihedral increases or decreases. Figure 13 shows the instantaneous lift of all the cases. In contrast to the situation when the span proportion of the inner/outer wing changes, the difference between the curves near the peak with the change of dihedral is very obvious. First, the peak position has a phase difference in cases with different dihedrals. Second, when the dihedral is negative the positive lift peak increases significantly with the increase in the dihedral. The maximum transient lift of case 2.4 is 36.2% higher than that of case 2.1. On the contrary, when the dihedral is positive, the maximum positive lift begins to decrease with the increase in the dihedral. When the dihedral is +40° (case 2.7), the maximum transient lift decreases by 8.7% compared with case 2.4.

The spanwise distribution of pressure variation at section x = −0.25 $\overline{c}$ and vorticity of three typical cases: case 2.1, 2.4, and 2.7 at t* = 0.35 are provided in Figure 14. The white line in the figures represents the position of the wing surface. It can be seen from Figure 14a,c,e that the pressure on the upper surface in case 2.7 near the wing root is obviously lower than in the other two cases. Additionally, the LEV in case 2.1 (Figure 14b) has shown an indication of separation while in case 2.7 (Figure 14f), the LEV seems to remain stable at the same time. However, combining the results in Figure 12, it can be observed that although there is a stronger LEV on the leading edge of case 2.7, it still offers a comparatively low average lift. The reason the lift of cases 2.1 and 2.7 become significantly smaller could be due to the large dihedral, which leads to a smaller projected area on the normal plane of lift. Based on the results of the average lift in Figure 12, it can be concluded that the lift produced by the flapping wing based on the mechanisms discussed in this paper are mostly related to the projected area of the wing in the normal plane of lift, which is consistent with the first hypothesis we made at the beginning of this section: the projected area of the wing on the normal plane of lift is the main factor affecting the average lift. This also explains the phenomenon that the lift peak gradually moves backward as the dihedral increases in Figure 13: when the dihedral is negative, the greater the negative value, the earlier the time it reaches the maximum projected area; and when the dihedral is positive, the larger the dihedral, the later the time it reaches the maximum projected area.

The result is comparatively different from previous research on single DOF flapping wings which found that a positive dihedral is better for increasing the average lift. As concluded by Shkarayev and Silin [8], the aerodynamic force is probably benefited from the near fling effect [28], which increases the deformation of the wing and lowers the pressure on the upper surface during flapping because of the closer distance of the pair of wings. From the spanwise distribution of pressure variation of the typical cases in Figure 15, it can be seen that the pressure near the root (marked with red circles in the figure) of the upper surface becomes lower with an increase in the dihedral, which matches the statement in previous works. The reasons that our result is difference from previous works may be because:

• the flapping mechanism discussed in this paper has a different characteristic compared with the single DOF flapping mechanism in previous research; or,
• the rigid model selected in this work neglects the flexible effect, which will contribute more aerodynamic forces from the clap and fling effect.

Furthermore, the foldable mechanism adopted in this research has an asymmetric flapping design, resulting in different inertia characteristics during upstroke and downstroke, which could result in new phenomena when employed in the FWAVs. Experiment tests [30] or fluid–structure interaction analyses [31,32] are needed to determine whether there are similar effects in previous works which can help increase lift.

Besides of the discussion of absolute lift value, there are also some other interesting phenomena. In combination with Figure 12 and Figure 13, it was found that although case 2.7 (dihedral = 40°) had a positive lift peak 24.4% larger than case 2.1 (dihedral = −40°), the average lift of the two cases had no significant difference. Although the dihedrals of the two cases have a symmetrical appearance, it should be noted that the dihedral of the model is defined by the inner wing dihedral at the neutral position. From the view of the dihedral of the outer wing provided in Table 4, the projection area at the neutral position of the outer wing on the normal plane of lift of case 2.7 should be much larger than that of case 2.1 but resulted in no significant difference in average lift. To study the reasons, we further analyzed the lift difference by directly using the lift of case 2.7 minus the lift of case 2.1, as shown in Figure 16. It can be seen from Figure 16 that the instantaneous lift of case 2.7 is kept lower than that of case 2.1 except in the period around the positive peak (t* = 0.3 − 0.5). It is known from Figure 14 that when t* = 0.35, the reason that the instantaneous lift of case 2.7 is greater than that of case 2.1 is due to the fact that the projection area of the wing on the normal plane of lift is larger. Therefore, we only selected the other two time points: t* = 0.25 and t* = 0.6 to further analyze why the instantaneous lift of case 2.1 was larger.

The reason for the large instantaneous lift of case 2.1 at time t* = 0.25 is the same as the reason for the large instantaneous lift of case 2.7 at time t* = 0.35, the projection area of the wing surface on the normal plane of lift at this time is comparatively larger as can be seen from Figure 15a,e. When t* = 0.6, the outer wing is turned into the upstroke so to see a low-pressure region at the lower surface of the outer wing is expected. However, as can be seen by comparing Figure 17a,b, the negative pressure under the lower wing surface in case 2.1 is more obvious than that in case 2.7. This is possibly also due to the near fling effect as discussed previously in Figure 15. In general, this low-pressure region should induce a negative gain to the lift. However, in case 2.7, because the outer wing arrives at a flapping angle larger than 90°, the induced force provides a positive component to the lift. Although the projection area of the outer wing in the low-pressure region on the normal plane of lift is very small due to the large flapping angle of the wing at this specific time, it can be seen from the instantaneous lift curve in Figure 13 that the positive lift generated by the outer wing at this time is sufficient to offset the negative lift generated by the inner wing, so the instantaneous lift of case 2.1 at this time is close to zero. This phenomenon provides new ideas for the design of future bionic flapping mechanisms.

4. Conclusions

This paper provides the aerodynamic analysis of a bat-inspired foldable flapping wing mechanism. Three-dimensional CFD simulations were performed for two variables, inner/outer wing proportion and dihedral. A parametric study of each factor on lift characteristics was performed. It was found that when changing inner/outer wing proportion, the maximum time-averaged lift appears in cases where the inner wing occupied half of the semi-span. In cases where the inner wingspan proportion was expanded from 30% to 50%, the lift increased by 11.2%. The arrangement with maximum average lift matched the arrangement of real bats. The other interesting finding was that the significant changes in the mid-stroke dihedral can lead to s decreases in the time-averaged lift in one flapping cycle, whether it became extremely large or small. The main influencing factor to the lift of the foldable flapping wing discussed in this paper is the projected area of the wing surface on the normal plane of lift. Such findings of aerodynamic lift characteristics could support our understanding of real biological flight, provide essential data for the design of future FWAVs, and also promote important lift optimization of foldable flapping wing designs.

The near fling effect, similar to previous works, was also revealed in the flow field in this research. However, the induced force from these effects was not the main contributing factor to the lift. It should be noticed that this research has employed a rigid wing model which neglects the flexible effect of the wing, this might reduce the lift contributed by the near fling or clap and fling effect. Experiment tests or fluid–structure interaction analyses are needed to determine the contribution of the flexibility to the span foldable flapping wing.