Similitude for the Dynamic Characteristics of Dual-Rotor System with Bolted Joints

Abstract

The dual-rotor system has been widely used in aero-engines and has the characteristics of large axial size, the interaction between the high-pressure rotor and low-pressure rotor, and stiffness nonlinearity of bolted joints. However, the testing of a full-scale dual-rotor system is expensive and time-consuming. In this paper, the scaling relationships for the dual-rotor system with bolted joints are proposed for predicting the responses of full-scale structure, which are developed by generalized and fundamental equations of substructures (shaft, disk, and bolted joints). Different materials between prototype and model are considered in the derived scaling relationships. Moreover, the effects of bolted joints on the dual-rotor system are analyzed to demonstrate the necessity for considering bolted joints in the similitude procedure. Furthermore, the dynamic characteristics for different working conditions (low-pressure rotor excitation, high-pressure rotor excitation, two frequency excitations, and counter-rotation) are predicted by the scaled model made of a relatively cheap material. The results show that the critical speeds, vibration responses, and frequency components can be predicted with good accuracy, even though the scaled model is made of different materials.

1. Introduction

Dual-rotor systems are widely used in large rotating machines, such as aero-engines [1,2] and gas turbines [3,4]. The dual-rotor system of an aero-engine is composed of several components with different materials to reduce its weight. These components are usually connected by bolted joints, which change the local stiffness and affect the dynamics of the rotor system [5,6]. However, prototype testing is usually expensive and time-consuming. Thus, similitude design can be used to overcome this issue and reduce costs and difficulties.

Many scholars have studied the application of beams, plates, and cylindrical shells. For beams, Asl et al. studied the scaling laws for fundamental frequency [7] and transverse deflection [8] of I-beams. Kasivitamnuay and Singhatanadgid [9] predicted the displacement of a static-loaded beam by using the scaled model. Subsequently, they [10] investigated the similitude problem related to the static displacement of a cracked beam. As for the similitude of plates, Coutinho et al. [11] developed the scaling laws for beam-plates and predicted the transverse displacement of full-size structures. Yazdi studied the scaling laws for free vibration [12] and flutter speed [13] of plates. Frostig and Simitses [14] investigated the similitude of sandwich panels and predicted the wrinkling and global buckling through scaled models. Singhatanadgid and Songkhla [15] derived the scaling laws for natural frequencies of rectangular plates with various boundary conditions, then the effectiveness is demonstrated by experimental test. Franco et al. predicted the vibration velocity [16] of a flexural plate with a high modal overlap factor, and then the vibration responses under a turbulent boundary layer excitation [17] were put into similitude. Mazzariol and Alves [18] established the scaling laws of plates under impact load. In addition, Petrone et al. [19] conducted a similitude investigation of stiffened cylinders, and the natural frequency and forced response were considered in the prediction procedure. Rezaeepazhand et al. designed the scaled models for predicting the free vibration [20] and buckling under compressive loads [21] of cylinders. Ungbhakorn and Wattanasakulpong [22] proposed a similitude procedure for buckling and free vibration of cylindrical shells. Aiming at coupled structures, De Rosa and Franco predicted the vibration responses of assemblies of plates [23], coupling beams and plates [24], and long and short wavelength coupling structures [25]. You et al. [26] presented a scaling procedure for wing boxes and put the deflection and modal behavior into similitude. Song et al. [27] investigated the similitude of machine tools, and then the modal shape was predicted by the scaled model. However, the similitude studies of rotor systems are relatively rare. The complete scaling laws for dynamic characteristics of rotor systems were presented by Wu [28]. Li et al. [29] developed the scaling laws for critical speeds of rotor systems. Furthermore, similitude studies of dual-rotor systems considering the nonlinearity of bolted joints are not found.

During the past decades, many studies have been conducted on similitude methods. Dimensional analysis (DA) and Similitude Theory Applied to Governing Equations (STAGE) are widely used [27]. DA is simple to use and useful for those systems without a set of governing equations, such as complex or new systems [30]. The drawbacks of DA are that (1) great effort and experience are required for obtaining scaling laws and (2) this method is not suitable for partial similitude since the geometric parameters can not be scaled in different scales. STAGE obtains scaling laws directly through the governing equations or its analytical solutions and has been adopted by many scholars. For instance, Rezaeepazhand et al. investigated the similitude of laminated plates [31] and composite beam-plates [32] based on STAGE. Singhatanadgid et al. [15] developed the scaling laws for plates through the governing equations. However, STAGE needs the governing equation and a lot of work of derivation. Then energy method (EM) was presented by Ungbhakorn and Singhatanadgid [33], which considered the scaling laws of boundary conditions, but the work of derivation was also required. De Rosa et al. [34] proposed a similitude method named SAMSARA, which was applied to the similitude issue of acoustic structures, such as flexural plates [35]. In recent years, sensitivity analysis (SA) [36] was used to obtain scaling laws. However, the scaling laws established by SA lack physical meaning and may ignore important phenomena [30].

Although many meaningful works on similitude have been completed, the following issues still exist: (i) most similitude studies focused on the linear systems or simple structures, but similitude studies of dual-rotor systems considering the nonlinearity of bolted joints are not found; (ii) STAGE and EM need the governing equation and a lot of work of derivation. In addition, SA lacks physical meaning and may overlook important phenomena.

In the paper, the dynamic model of a dual-rotor system with bolted joints is developed and the effects of bolted joints on the dual-rotor system are investigated in Section 2. The scaling laws considering different materials are derived by the generalized and fundamental equations of shaft, disk, and bolted joints in Section 3. In Section 4, aiming at the working conditions and structural characteristics of dual-rotor systems, the similitude for dynamic characteristics in the cases of low-pressure (LP) rotor excitation, high-pressure (HP) rotor excitation, two frequency excitations, and counter-rotation are discussed. The conclusions are listed in Section 5.

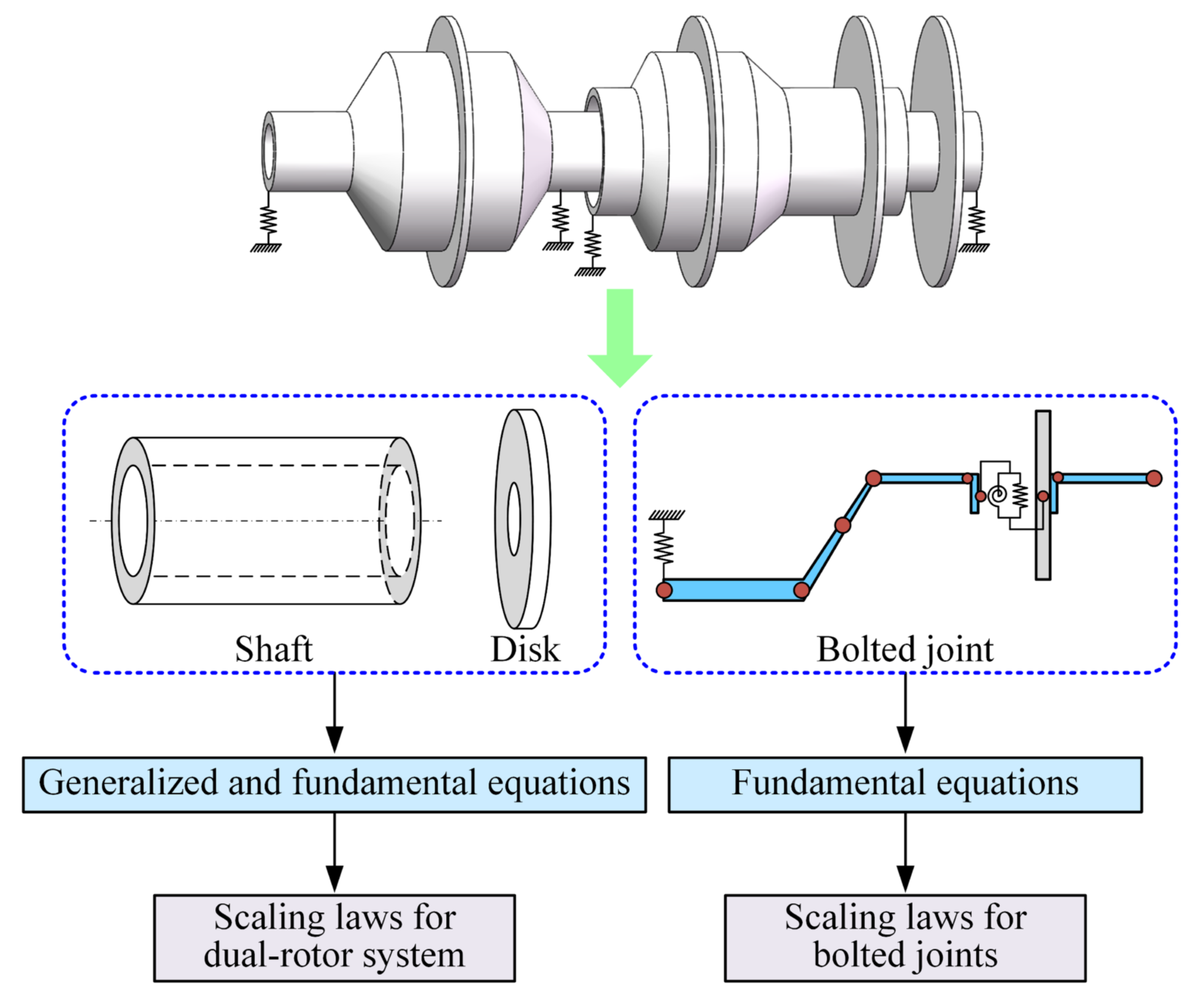
2. Dynamic Model of the Dual-Rotor System with Bolted Joints

As shown in Figure 1, a dual-rotor system consisting of a high-pressure (HP) rotor, a low-pressure (LP) rotor, bolted joint structures and elastic supports is developed. The dual-rotor system includes five supports and is modeled by the finite element method. Support 5 denotes the intershaft bearing, which couples the vibration responses of the HP rotor and LP rotor. The parameters of the dual-rotor system are listed in Appendix A. There are two bolted joints in the model, which are used to connect the LP rotor and the HP rotor, respectively.

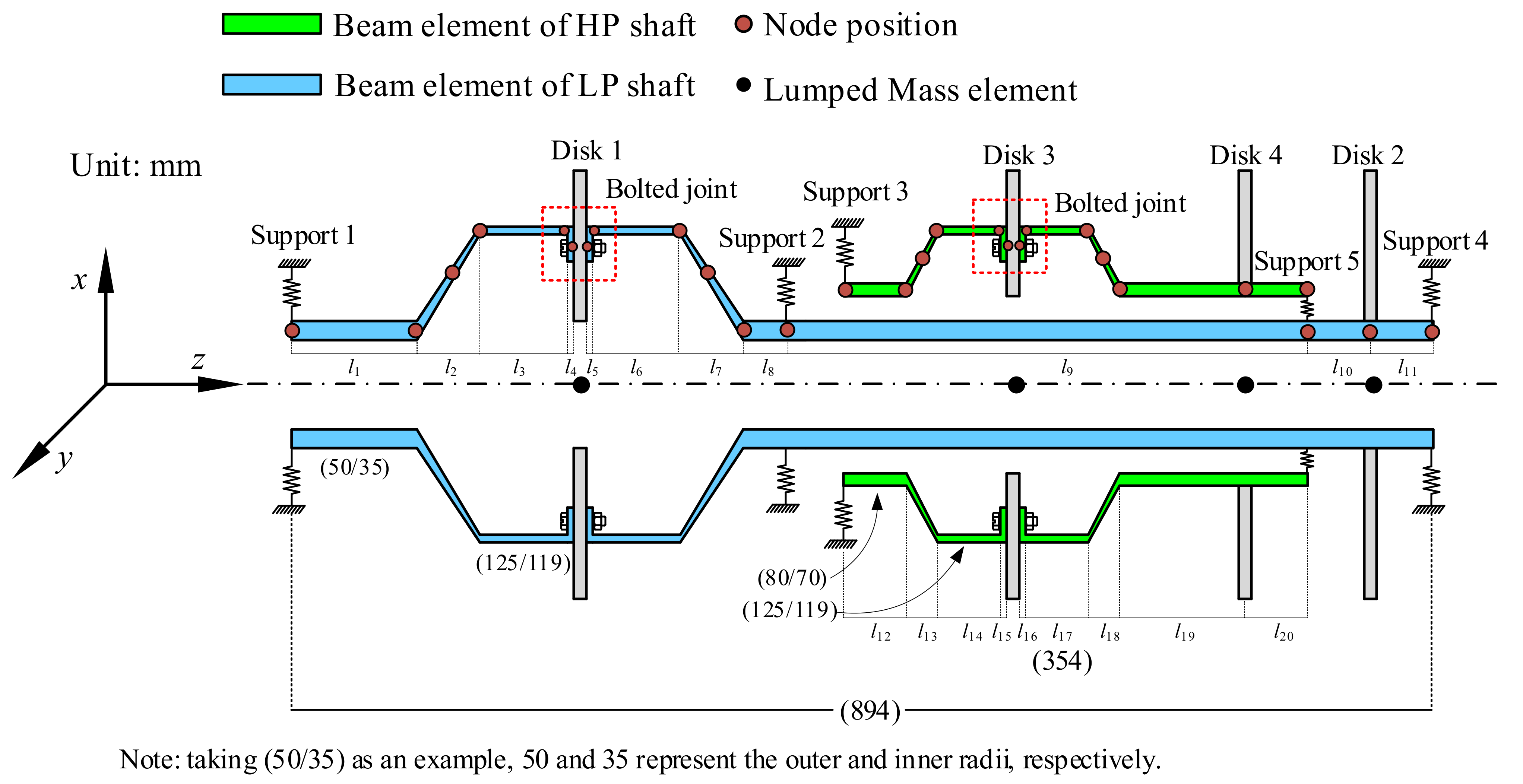
2.1. Dual-Rotor System Model

The dual-rotor system is modeled by Timoshenko beam elements. The finite element model includes 24 beam elements. The disks and bearings are simplified as lumped mass elements and spring-damping elements, respectively. The bolted joint is a noncontinuous structure; thus, the beam elements near the bolted joint structures are connected by the joint elements.

The generalized displacement vectors of the LP rotor can be expressed as follows:

$${\mathit{u}}_{\mathrm{L}}={\left[x_1,y_1,{\theta }_{x1},{\theta }_{y1},\cdots ,x_{15},y_{15},{\theta }_{x15},{\theta }_{y15}\right]}^{\mathrm{T}}$$

(1)

where x₁, y₁,⋯, x₁₅, y₁₅ are translations of nodes 1−15, and θ_x1, θ_y1,⋯, θ_x15, θ_y15 are rotations of nodes 1−15.

The LP rotor can be described in matrix form as follows:

$${\mathit{M}}_{\mathrm{L}}{\ddot{\mathit{u}}}_{\mathrm{L}}+\left({\mathit{C}}_{\mathrm{L}}-{\mathit{\Omega }}_{\mathrm{L}}{\mathit{G}}_{\mathrm{L}}\right){\dot{\mathit{u}}}_{\mathrm{L}}+{\mathit{K}}_{\mathrm{L}}{\mathit{u}}_{\mathrm{L}}={\mathit{Q}}_{\mathrm{L}}$$

(2)

where Ω is the rotating speed. M, C, G, and K are the mass, damping, gyroscopic, and stiffness matrices. The subscript L represents the index of the LP rotor. Rayleigh damping is applied, and the damping matrix is given as C_L = αM_L + βK_L.

The generalized force vectors of the LP rotor can be described as

$${\mathit{Q}}_{\mathrm{L}}={\left[\begin{array}{l}0,0,0,0,\cdots ,m_1{e}_1{\Omega }_{\mathrm{L}}^2\cos \left({\Omega }_{\mathrm{L}}t\right),m_1{e}_1{\Omega }_{\mathrm{L}}^2\sin \left({\Omega }_{\mathrm{L}}t\right),0,0,\cdots ,\\ m_2{e}_2{\Omega }_{\mathrm{L}}^2\cos \left({\Omega }_{\mathrm{L}}t\right),m_2{e}_2{\Omega }_{\mathrm{L}}^2\sin \left({\Omega }_{\mathrm{L}}t\right),0,0,\cdots ,0,0,0,0\end{array}\right]}^{\mathrm{T}}$$

(3)

where m and e denote the mass and eccentricity, and the subscript represents the number of disks.

The generalized displacement vectors of the HP rotor are described as follows:

$${\mathit{u}}_{\mathrm{H}}={\left[x_{16},y_{16},{\theta }_{x16},{\theta }_{y16},\cdots ,x_{28},y_{28},{\theta }_{x28},{\theta }_{y28}\right]}^{\mathrm{T}}$$

(4)

Accordingly, the motion equation of the HP rotor can be obtained:

$${\mathit{M}}_{\mathrm{H}}{\ddot{\mathit{u}}}_{\mathrm{H}}+\left({\mathit{C}}_{\mathrm{H}}-{\mathit{\Omega }}_{\mathrm{H}}{\mathit{G}}_{\mathrm{H}}\right){\dot{\mathit{u}}}_{\mathrm{H}}+{\mathit{K}}_{\mathrm{H}}{\mathit{u}}_{\mathrm{H}}={\mathit{Q}}_{\mathrm{H}}$$

(5)

where the subscript H denotes the index of the HP rotor.

The force of the HP rotor is expressed as follows:

$${\mathit{Q}}_{\mathrm{H}}={\left[\begin{array}{l}0,0,0,0,\cdots ,m_3{e}_3{\Omega }_{\mathrm{H}}^2\cos \left({\Omega }_{\mathrm{H}}t\right),m_3{e}_3{\Omega }_{\mathrm{H}}^2\sin \left({\Omega }_{\mathrm{H}}t\right),0,0,\cdots ,\\ m_4{e}_4{\Omega }_{\mathrm{H}}^2\cos \left({\Omega }_{\mathrm{H}}t\right),m_4{e}_4{\Omega }_{\mathrm{H}}^2\sin \left({\Omega }_{\mathrm{H}}t\right),0,0,\cdots ,0,0,0,0\end{array}\right]}^{\mathrm{T}}$$

(6)

Consider the eccentricity of all disks, and the eccentricity is 0.5 mm. Then, the motion equation of the dual-rotor system is further written as follows:

$$\left[\begin{array}{cc}{\mathit{M}}_{\mathrm{L}}& \\ & {\mathit{M}}_{\mathrm{H}}\end{array}\right]\left[\begin{array}{c}{\ddot{\mathit{u}}}_{\mathrm{L}}\\ {\ddot{\mathit{u}}}_{\mathrm{H}}\end{array}\right]+\left[\begin{array}{cc}{\mathit{C}}_{\mathrm{L}}-{\mathsf{\Omega }}_{\mathrm{L}}{\mathit{G}}_{\mathrm{L}}& \\ & {\mathit{C}}_{\mathrm{H}}-{\mathsf{\Omega }}_{\mathrm{H}}{\mathit{G}}_{\mathrm{H}}\end{array}\right]\left[\begin{array}{c}{\dot{\mathit{u}}}_{\mathrm{L}}\\ {\dot{\mathit{u}}}_{\mathrm{H}}\end{array}\right]+\left[\begin{array}{cc}{\mathit{K}}_{\mathrm{L}}& \\ & {\mathit{K}}_{\mathrm{H}}\end{array}\right]\left[\begin{array}{c}{\mathit{u}}_{\mathrm{L}}\\ {\mathit{u}}_{\mathrm{H}}\end{array}\right]=\left[\begin{array}{c}{\mathit{Q}}_{\mathrm{L}}\\ {\mathit{Q}}_{\mathrm{H}}\end{array}\right]$$

(7)

Next, the stiffness of supports needs to be added to the stiffness matric of dual-rotor system, where the positions are K(1,1), K(2,2), K(45,45), K(46,46), K(57,57), K(58,58), K(61,61), and K(62,62). Damping is added in the same way. The stiffness of the intershaft bearing is added as follows:

$$\begin{array}{ll}& \hspace{1em}4k-3\hspace{1em}4k-2\hspace{1em}4l-3\hspace{1em}4l-2\\ {\mathit{K}}_{\mathrm{in}}=& \left[\begin{array}{cccc}{k}_{\mathrm{in},xx}& k_{\mathrm{in},xy}& -k_{\mathrm{in},xx}& -k_{\mathrm{in},xy}\\ k_{\mathrm{in},yx}& k_{\mathrm{in},yy}& -k_{\mathrm{in},yx}& -k_{\mathrm{in},yy}\\ -k_{\mathrm{in},xx}& -k_{\mathrm{in},xy}& k_{\mathrm{in},xx}& k_{\mathrm{in},xy}\\ -k_{\mathrm{in},yx}& -k_{\mathrm{in},yy}& k_{\mathrm{in},yx}& k_{\mathrm{in},yy}\end{array}\right] \begin{array}{c}4k-3\\ 4k-2\\ 4l-3\\ 4l-2\end{array}\end{array}$$

(8)

where k = 13 and l = 28.

2.2. Bolted Joint Model

The bolted joint is considered as a joint element with two nodes. One of the nodes is located at the end of the flange near the disk, and the other node is located at the position of the disk, as shown in Figure 2. The generalized displacement vectors and stiffness matrix are expressed as follows:

$$q_{\mathrm{J}}={\left[x_{\mathrm{J}},y_{\mathrm{J}},{\theta }_{x\mathrm{J}},{\theta }_{y\mathrm{J}},x_{\mathrm{J}+1},y_{\mathrm{J}+1},{\theta }_{x\left(\mathrm{J}+1\right)},{\theta }_{y\left(\mathrm{J}+1\right)}\right]}^{\mathrm{T}}$$

(9)

$${\mathit{K}}_{\mathrm{J}}=\left[\begin{array}{cccccccc}k& & & & & & & \\ 0& k& & & & & & \\ 0& 0& k_{\theta }& & & \mathrm{sym}& & \\ 0& 0& 0& k_{\theta }& & & & \\ -k& 0& 0& 0& k& & & \\ 0& -k& 0& 0& 0& k& & \\ 0& 0& -k_{\theta }& 0& 0& 0& k_{\theta }& \\ 0& 0& 0& -k_{\theta }& 0& 0& 0& k_{\theta }\end{array}\right]$$

(10)

In terms of [6,37,38], the stiffness of the bolted joint has piecewise linear characteristics, and the critical point occurs when the external force is equal to the effect of preload applied on the bolts. The first and second stages of piecewise linear characteristics are depicted in Figure 3a,b, respectively.

The stiffness characteristics of bolted joint in [37] are expressed as

$$k_{\theta }=\left\{\begin{array}{l}{k}_{\theta 1}, \left|\varphi \right|\le {\varphi }_0\\ k_{\theta 2}, \left|\varphi \right|>{\varphi }_0\end{array}\right.$$

(11)

where ϕ is the relative rotation angle between the flange and disk and is shown in Figure 3. ϕ₀ is the critical rotation angle when the external force is equal to the effect of preload applied on the bolts. k_θ1 and k_θ2 are the stiffness at the first and second stages, respectively. ϕ can be obtained as follows:

$$\varphi =\sqrt{{\left({\theta }_{x\mathrm{J}}-{\theta }_{x\left(\mathrm{J}+1\right)}\right)}^2+{\left({\theta }_{y\mathrm{J}}-{\theta }_{y\left(\mathrm{J}+1\right)}\right)}^2}$$

(12)

where the subscripts J and J + 1 represent the left and right nodes of the joint element, respectively. The stiffness and critical rotation angle can be calculated according to [37] and are listed in

Table 1. Stiffness and critical rotation angle of bolted joints.

Parameter	Value
kθ1 (N∙m/rad)	3.16 × 107
kθ2 (N∙m/rad)	2.70 × 106
ϕ0 (rad)	1.42 × 10−5

. More detailed formulas can be found in [37].

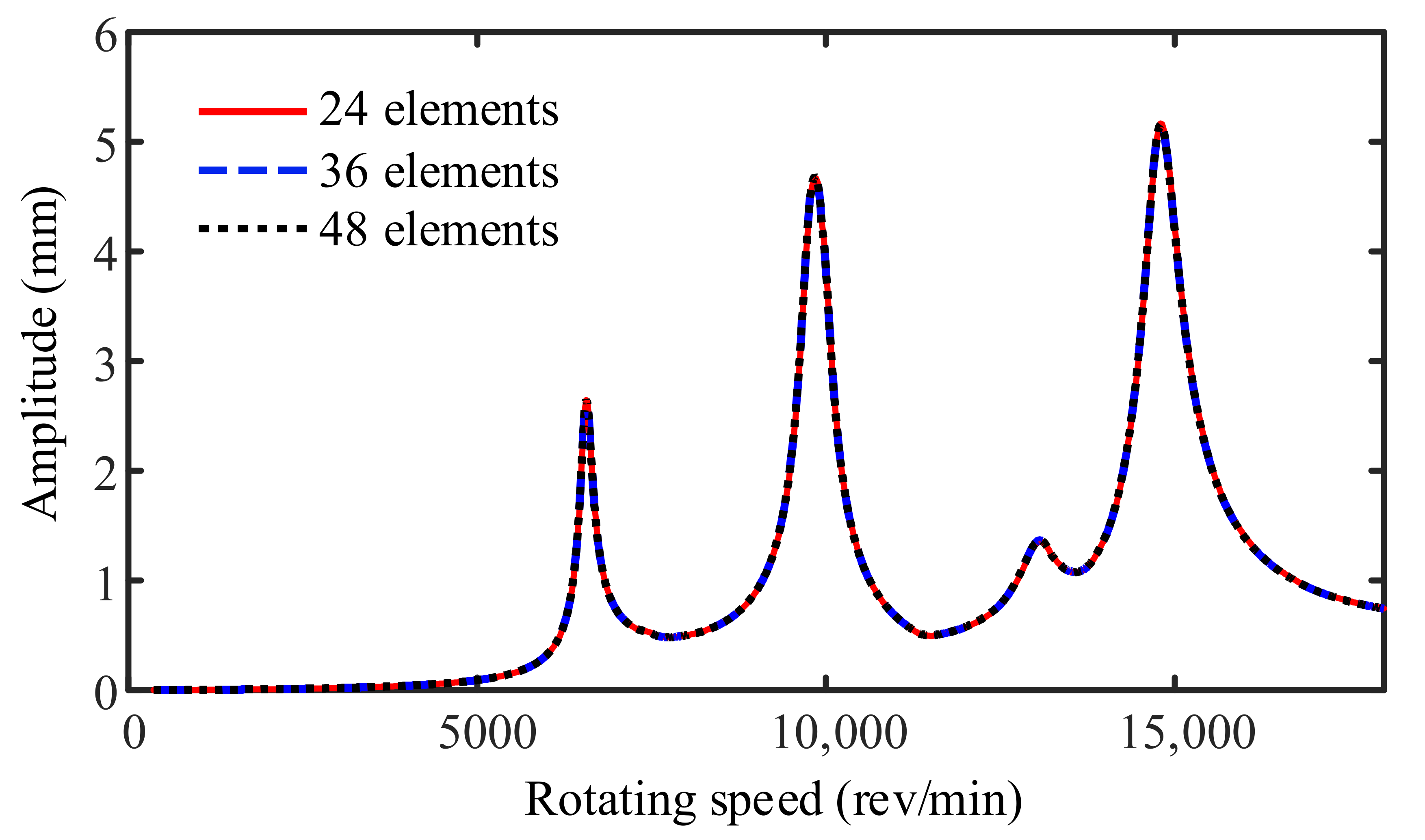
2.3. Convergence Analysis

In this section, the influence of node number on the vibration responses is assessed. The element number is chosen as 24, 36, and 48. The amplitude frequency responses for 24, 36, and 48 elements are shown in Figure 4, and the spectrum cascades under different rotating speeds are illustrated in Figure 5. It is found that the amplitude frequency responses and spectrum cascades for the cases of 24, 36, and 48 elements are almost identical. The vibration responses have already converged. Therefore, the case of 24 elements is used in the following analysis.

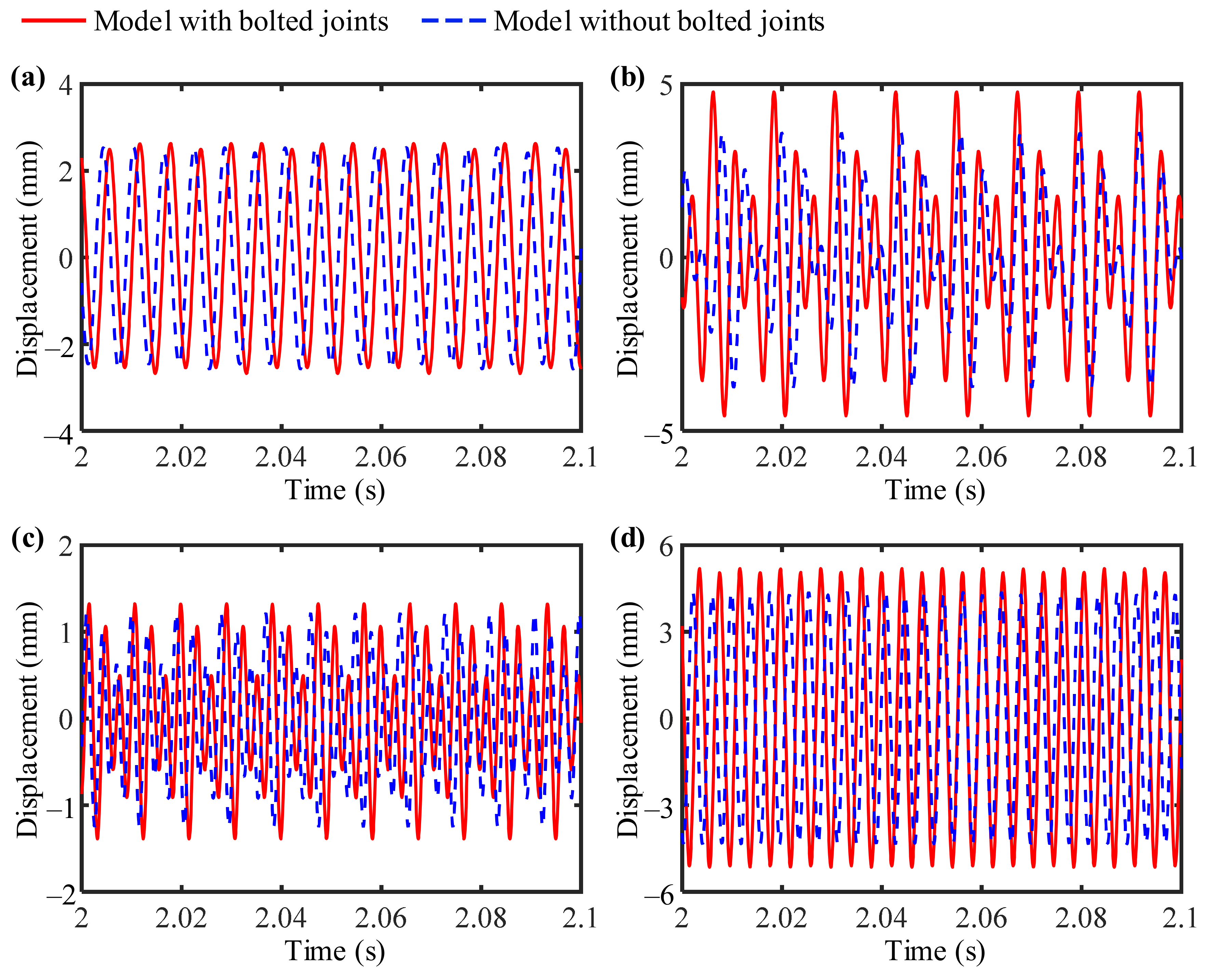
2.4. Comparison of the Dual-Rotor System with and without Bolted Joints

The bolted joint changes the local stiffness of the rotor system subjected to a heavy load, which further influences the vibration behaviors of the rotor system [5,6]. Thus, the section aims at investigating the effect of bolted joints and further verifying the bolted joint model.

The dual-rotor system models with and without bolted joints are established to compare the vibration characteristics of these two models. The configurations of the models with and without bolted joints are the same except for the parameters of the bolted joints. Note that the positions of bolted joints are continuous structures in the model without bolted joints. The dynamic equations of these two models are obtained by the Newmark method. The ratio of rotational speed (R = Ω_H/Ω_L) is 1.5 [39] in this paper. The amplitude frequency responses of disk 1 of the two models are presented in Figure 6. The time responses at resonance of these two models are shown in Figure 7. It can be seen that the critical speeds of the model with bolted joints are less than those of the model without bolted joints. Thus, the bolted joints reduce the critical speed of the rotor system since they change the local stiffness, which is consistent with the laws in [37]. In addition, the spectrum cascades under different rotating speeds of these two models are shown in Figure 8. Only the fundamental frequencies of LP and HP rotors are prominent in the spectrum cascades of the model without bolted joints (see Figure 8b). As a contrast, for the model with bolted joints, the frequency multiplication of LP and HP rotors (2f_L, 3f_L, 4f_L, 2f_H, 3f_H, and 4f_H) has occurred, which is consistent with the results of [40]. Thus, the bolted joint model is considered verified, and the bolted joints must be considered in developing scaling relationships due to the significant influence on the rotor system.

3. Scaling Relationships for the Dual-Rotor System with Bolted Joints

In terms of STAGE and EM, the governing equation or a certain work of derivation is required, which is not available for the complex structure. Aiming at these issues, the scaling relationships are obtained by generalized and fundamental equations, rather than the governing equation of the whole structure. The schematic diagram is shown in Figure 9. Furthermore, developing scaling relationships from generalized and fundamental equations reduces the calculation effort. As for the dual-rotor system, nonlinearity is introduced by bolted joints. The parameters of the bolt joints need to be put into similitude appropriately to obtain exact results.

3.1. Scaling Relationships for Dual-Rotor System

The generalized translational equation of rigid disks is expressed as

$${\rho }_{\mathrm{d}}\pi l_{\mathrm{d}}{r}_{\mathrm{d}}^2\frac{{\mathrm{d}}^2{x}_{\mathrm{d}}}{\mathrm{d}{t}^2}={\rho }_{\mathrm{d}}\pi l_{\mathrm{d}}{r}_{\mathrm{d}}^{2}e{\Omega }^2$$

(13)

where ρ_d, l_d, and r_d are the density, thickness, and radius of the disks.

The generalized translational equation of a shaft element can be written as

$${\rho }_{\mathrm{s}}\pi l_{\mathrm{s}}{r}_{\mathrm{s}}^2\frac{{\mathrm{d}}^2{x}_{\mathrm{s}}}{\mathrm{d}{t}^2}+c_{\mathrm{s}}\frac{\mathrm{d}{x}_{\mathrm{s}}}{\mathrm{d}t}+k_{\mathrm{s}}{x}_{\mathrm{s}}={\rho }_{\mathrm{s}}\pi l_{\mathrm{s}}{r}_{\mathrm{s}}^{2}g$$

(14)

Where ρ_s, l_s, r_s, c_s, k_s, and x_s are the density, length, radius, damping, stiffness, and displacement in the x-direction of a shaft element.

The internal bending moment of the shaft can be written as

$$M=EI\frac{{\mathrm{d}}^2{x}_{\mathrm{s}}}{\mathrm{d}{z}^2}$$

(15)

where E and I are Young’s modulus and area moment of inertia, respectively.

The internal bending moment M can be also expressed as

$$M=k_{\mathrm{s}}{x}_{\mathrm{s}}{l}_s$$

(16)

Substituting Equations (15) and (16) into Equation (14) yields

$${\rho }_{\mathrm{s}}\pi l_{\mathrm{s}}{r}_{\mathrm{s}}^2\frac{{\mathrm{d}}^2{x}_{\mathrm{s}}}{\mathrm{d}{t}^2}+c_{\mathrm{s}}\frac{\mathrm{d}{x}_{\mathrm{s}}}{\mathrm{d}t}+\frac{EI}{l_s}\frac{{\mathrm{d}}^{2}x}{\mathrm{d}{z}^2}={\rho }_{\mathrm{s}}\pi r_{\mathrm{s}}^2$$

(17)

According to the continuity of displacements,

$$x_{\mathrm{d}}=x_{\mathrm{s}}$$

(18)

Herein, the scaling factor λ is the prototype parameter divided by the model parameter. A parameter is written in the subscript of scaling factor λ, which indicates the scaling factor of the parameter. Then, applying similitude theory to Equations (13) and (17) yields

$${\lambda }_{\rho }{\lambda }_l{\lambda }_r^2\frac{{\lambda }_x}{{\lambda }_t^2}={\lambda }_{\rho }{\lambda }_l{\lambda }_r^2{\lambda }_e{\lambda }_{\mathsf{\Omega }}^2={\lambda }_c\frac{{\lambda }_x}{{\lambda }_t}=\frac{{\lambda }_E{\lambda }_r^4{\lambda }_x}{{\lambda }_l^3}={\lambda }_{\rho }{\lambda }_r^2$$

(19)

Here, the geometric parameters of the dual-rotor system are scaled by the same ratio; i.e., λ_r = λ_l. The following scaling relationships are obtained by Equation (19) and λ_Ω = 1/λ_t:

$${\lambda }_k={\lambda }_E{\lambda }_l$$

(20)

$${\lambda }_c={\lambda }_l^2\sqrt{{\lambda }_E{\lambda }_{\rho }}$$

(21)

$${\lambda }_{\omega }={\lambda }_{\mathsf{\Omega }}=\frac{1}{{\lambda }_t}=\frac{1}{{\lambda }_l}\sqrt{\frac{{\lambda }_E}{{\lambda }_{\rho }}}$$

(22)

$${\lambda }_x={\lambda }_e=\frac{{\lambda }_l{\lambda }_{\rho }}{{\lambda }_E}$$

(23)

According to dimensional analysis [30], the parameters with the same unit can be scaled by the same scaling factor. Thus, the scaling factors of supports’ stiffness and damping are equal to those of shaft; i.e., λ_k and λ_c represent the scaling factors for the stiffness and damping of both shaft and supports.

The forces on the intershaft support can be expressed as follows:

$$\left\{\begin{array}{l}{k}_{\mathrm{in}}\left(x_{\mathrm{L}}-x_{\mathrm{H}}\right)=Q\\ Q=EI\frac{{\partial }^3{x}_{\mathrm{L}}}{\partial z^3}\end{array}\right.$$

(24)

where k_in is the stiffness of the intershaft support, and x_L and x_H are the displacements of LP and HP rotors, respectively. Q is the shear force.

Applying similitude theory to Equation (24) yields

$${\lambda }_{k_{\mathrm{in}}}{\lambda }_{x_{\mathrm{L}}}={\lambda }_E{\lambda }_{x_{\mathrm{L}}}\frac{{\lambda }_r^4}{{\lambda }_l^3}$$

(25)

$${\lambda }_{k_{\mathrm{in}}}{\lambda }_{x_{\mathrm{L}}}={\lambda }_{k_{\mathrm{in}}}{\lambda }_{x_{\mathrm{H}}}$$

(26)

In terms of Equations (25) and (26), the following scaling relationships can be obtained:

$${\lambda }_{k_{\mathrm{in}}}={\lambda }_E\frac{{\lambda }_r^4}{{\lambda }_l^3}$$

(27)

$${\lambda }_{x_{\mathrm{L}}}={\lambda }_{x_{\mathrm{H}}}$$

(28)

It is found from Equation (27) that the scaling factor of intershaft support stiffness λ_kin is equal to that of the shaft stiffness λ_k. According to Equation (28), the scaling factors of displacements can be represented by scaling factor λ_x.

3.2. Scaling Relationships for Bolted Joints

In this section, the scaling relationships related to the bolt joint parameters and rotor system parameters are developed. Nonlinearity is introduced by the piecewise linear stiffness of bolted joints. Therefore, the stiffness of bolted joints needs to be put into similitude first.

The piecewise linear stiffness is the angular stiffness; thus, the angular stiffness can be expressed as

$$k_{\theta }=\frac{M}{\varphi }$$

(29)

where k_θ is the piecewise linear stiffness, which includes k_θ1 and k_θ2. ϕ is the deflection angle of the bolted joints, which is dimensionless.

According to Equations (15), (23), and (29), the scaling factor for the piecewise linear stiffness can be obtained:

$${\lambda }_{k_{\theta }}={\lambda }_l^3{\lambda }_{\rho }$$

(30)

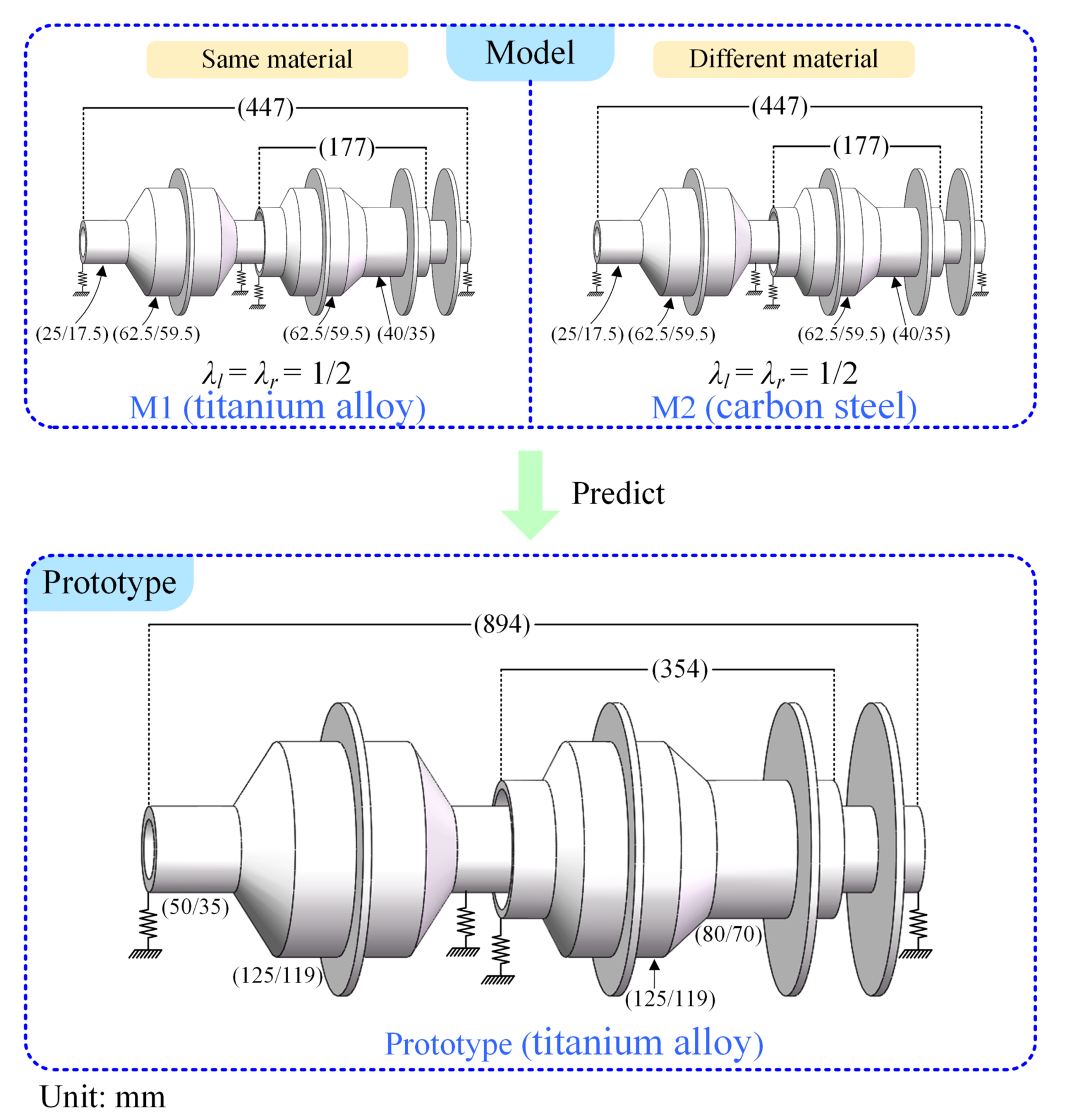
4. Verification of the Scaling Relationships

Two scaled models are studied and are named M1 and M2. The scaling factors of the models are listed in

Table 2. The scaling factors of the models M1 and M2.

Model	λl (λr)	λρ	λE	λc	λk	λω (λΩ)	λt	λx (λe)	λkθ
M1	0.5	1	1	0.25	0.5	2	0.5	0.5	0.125
M2	0.5	1.8	2	0.47	1	2.11	0.47	0.45	0.23

. Columns 2–4 of Table 2 are artificially determined scaling factors. The other scaling factors in Table 2 are obtained according to Equations (20)–(23) and (30). The parameters of the prototype are listed in

Table A1. Dimensions of the dual-rotor system.

Parameter (m)	Value	Parameter (m)	Value
			rLi	rLo	rRi	rRo
Length of shaft element l1	0.100	Radii of shaft element l1	0.035	0.050	0.035	0.050
Length of shaft element l2	0.050	Radii of shaft element l2	0.035	0.050	0.119	0.125
Length of shaft element l3, l6	0.070	Radii of shaft element l3, l6	0.119	0.125	0.119	0.125
Length of shaft element l4, l5	0.002	Radii of shaft element l4, l5	0.105	0.125	0.105	0.125
Length of shaft element l7	0.050	Radii of shaft element l7	0.119	0.125	0.035	0.050
Length of shaft element l8, l10, l11	0.050	Radii of shaft element l8, l10, l11	0.035	0.050	0.035	0.050
Length of shaft element l9	0.400	Radii of shaft element l9	0.035	0.050	0.035	0.050
Length of shaft element l12, l20	0.050	Radii of shaft element l12, l20	0.070	0.080	0.070	0.080
Length of shaft element l13	0.025	Radii of shaft element l13	0.070	0.080	0.119	0.125
Length of shaft element l14, l17	0.050	Radii of shaft element l14, l17	0.119	0.125	0.119	0.125
Length of shaft element l15, l16	0.002	Radii of shaft element l15, l16	0.105	0.125	0.105	0.125
Length of shaft element l18	0.025	Radii of shaft element l18	0.119	0.125	0.070	0.080
Length of shaft element l19	0.100	Radii of shaft element l19	0.070	0.080	0.070	0.080

and

Table A2. Physical parameters of the dual-rotor system.

Parameter (m)	Value	Parameter (m)	Value
Mass of disks 1 and 2 (kg)	7.22	Moment of inertia of disks 1 and 2 (kg.m2)	0.11
Mass of disk 3 (kg)	6.56	Moment of inertia of disk 3 (kg.m2)	0.11
Mass of disk 4 (kg)	6.15	Moment of inertia of disk 4 (kg.m2)	0.11
Support stiffness k1, k2, k3, k4 (N/m)	2 × 107	Support damping c1, c2, c3, c4, c5 (N/m)	500
Support stiffness kin (N/m)	4 × 107	Density (kg/m3)	4350
Elastic modulus (GPa)	105	Poisson ratio	0.26

, and the prototype is made of titanium alloy. The model M1 is a complete similitude model and the material is consistent with the prototype (titanium alloy). Furthermore, the material of M2 is carbon steel (density: 7850 kg/m³, Young’s modulus: 210 GPa, Poisson ratio: 0.26), which is different from M1. The purpose of M2 is to demonstrate the effectiveness of the proposed scaling relationships for the problem of different materials (between prototype and model). Note that M2 further reduces the costs of model testing since it allows for replacing the prototype with a model made of cheaper material. The sketches of the models and the prototype are presented in Figure 10.

Aiming at the working condition and structural characteristics of dual-rotor systems, LP rotor excitation (Section 4.1), HP rotor excitation (Section 4.2), two frequency excitations (Section 4.3), and counter-rotation (Section 4.4) are considered in predicting the vibration characteristics.

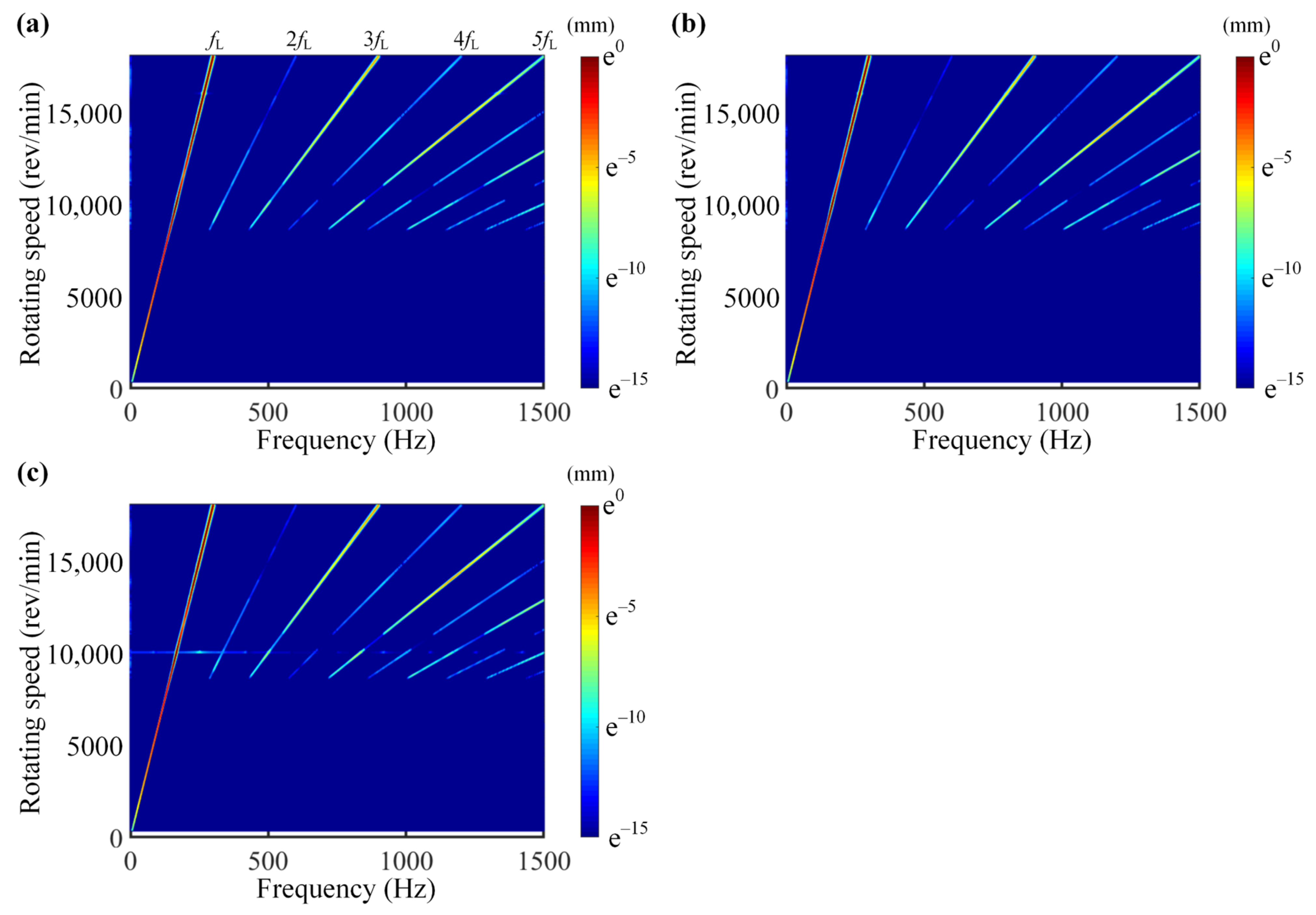
4.1. Case 1: LP Rotor Excitation

LP rotor excitation represents that disk 1 and disk 2 have mass eccentricities. Figure 8 presents the vibration responses of disk 1 of the prototype, the prediction as well as unscaled results. The aim is to predict the responses of the prototype (red line in Figure 11) by using the responses of models (dotted line in Figure 8). The dotted lines labeled as “unscaled” are the responses of models M1 and M2. The abscissa of the model’s response is multiplied by λ_ω (see Table 2), and the ordinate is multiplied by λ_x. Then, the prediction results (circles and points in Figure 11) can be obtained and are compared with the results of the prototype. It can be seen from Figure 11 that the unscaled results differ greatly from the results of the prototype. After prediction, the amplitude frequency responses (AFRs) of the prototype and predicted results overlap perfectly. It is worth noting that M2 is beneficial to further reduce the test cost since it is not only reduced in size but also relatively cheap in material (compared to the prototype).

Figure 12 presents the spectrum cascades under different rotating speeds of the prototype and corresponding predicted results for models M1 and M2. The frequency components of the prototype are made up of the frequency multiplication, such as 2f_L, 3f_L, 4f_L, and 5f_L, which is consistent with the laws in [40]. The results of models M1 and M2 are consistent with those of the prototype. This indicates that both the models M1 and M2 can accurately reproduce the frequency components of the prototype, even though M2 is made of relatively cheap material.

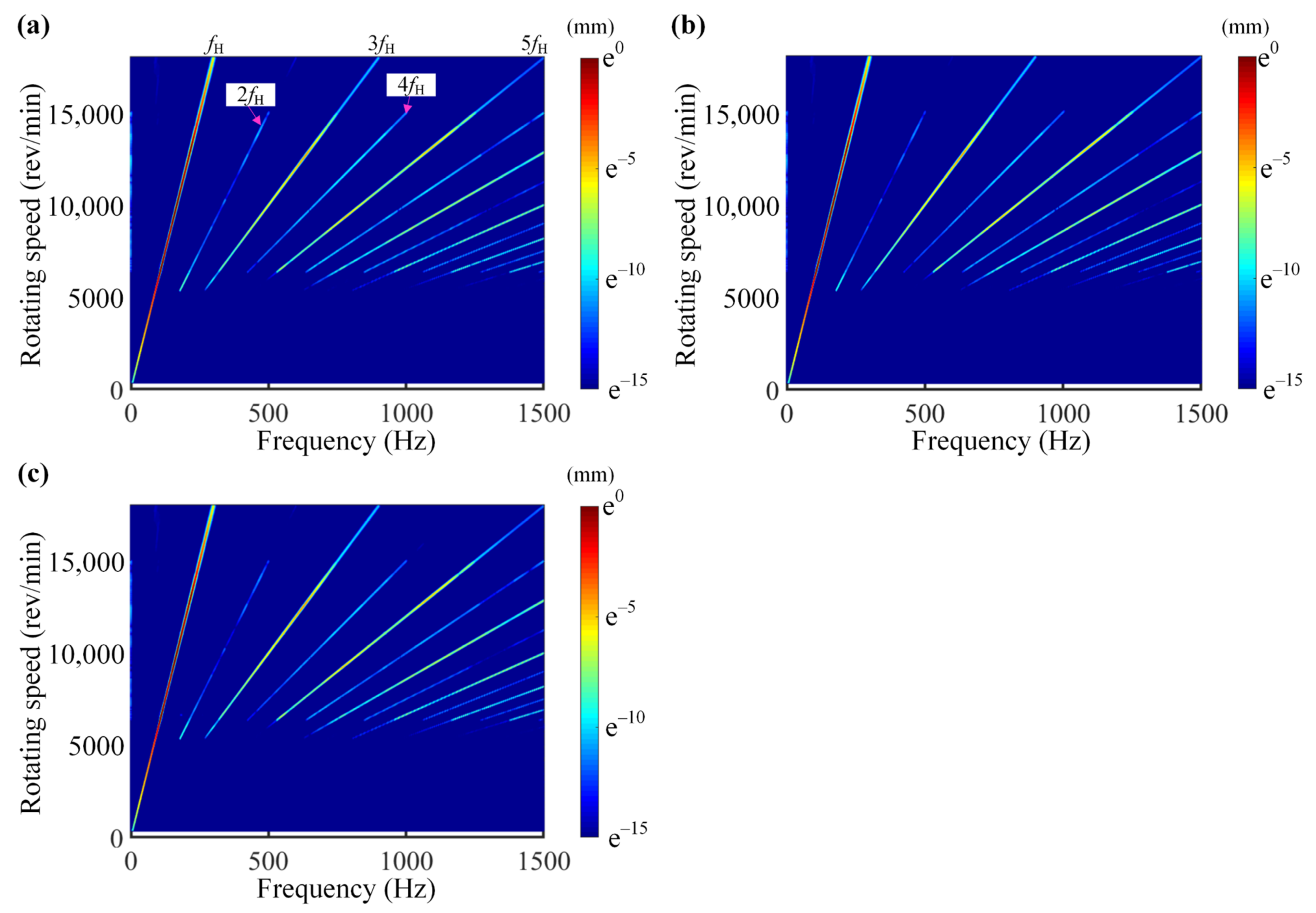
4.2. Case 2: HP Rotor Excitation

HP rotor excitation means that disk 3 and disk 4 have mass eccentricities. Figure 13 and Figure 14 show the predicted results for the amplitude frequency responses and spectrum cascades, respectively. It is found that the vibration responses of the prototype can also be predicted accurately by the models M1 and M2, where different materials between the prototype and models are considered in the similitude procedure. In addition, the frequency components (2f_L, 3f_L, 4f_L, and 5f_L) of the prototype can also be predicted by the two models under HP rotor excitation.

4.3. Case 3: Two Frequency Excitations

Two frequency excitations mean that all disks of LP and HP rotors have mass eccentricities. Figure 15 and Figure 16 describe the predicted results of the amplitude frequency responses and frequency components in the case of two frequency excitations. The vibration responses of M1 and M2 agree well with those of the prototype. Besides, the frequency components of the two models match perfectly those of the prototype.

4.4. Case 4: Counter-Rotation

Co- and counter-rotation indicate that the LP rotor and HP rotor rotate in the same and opposite directions, respectively. Cases 1–3 are investigated in the case of corotation; thus, counter-rotation is considered. The case of two frequency excitations is taken as an example in this section. The predictions for amplitude frequency responses and spectrum cascades are shown in Figure 17 and Figure 18. The predicted results show exact agreement between the models M1 and M2 and the prototype. Thus, the accuracy and effectiveness of proposed scaling relationships are considered verified.

5. Conclusions

This paper presents the scaling relationships for predicting the dynamic characteristics of a dual-rotor system with bolted joints, where different materials between the prototype and models are taken into account. The effects of bolted joints on the dual-rotor system are investigated, and the results indicate that bolted joints should be considered in the similitude procedure. The scaling relationships are obtained by generalized and fundamental equations and are used to predict the critical speeds, vibration responses, and frequency components under different working conditions. The main findings are summarized as follows:

• The scaling relationships are developed by generalized and fundamental equations of substructures (shaft, disk, and bolted joint). The scaling factors of geometric dimensions, support parameters, critical speed, and vibration displacement are derived and can be used to design scaled models. However, as for STAGE and EM, the governing equations of the whole structure are required when deriving scaling relationships. Thus, the scaling relationships developed in this paper can reduce the calculation effort and provide the possibility for the application of complex structures.
• The effect of bolted joints on the dual-rotor system is considered. It is found that the bolted joints reduce the critical speed and lead to the emergence of frequency multiplication. Thus, the bolted joints need to be considered in similitude analysis. Aiming at this issue, the scaling relationships derived in this paper take the nonlinear stiffness of bolted joint into account for the first time.
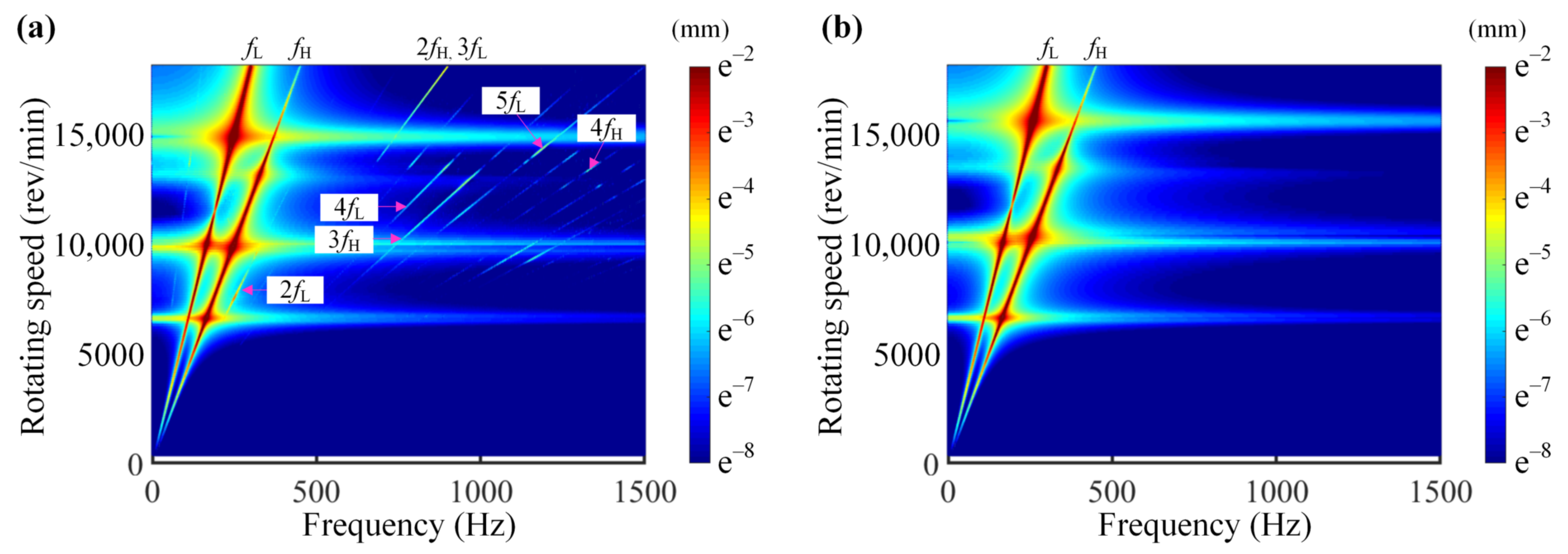
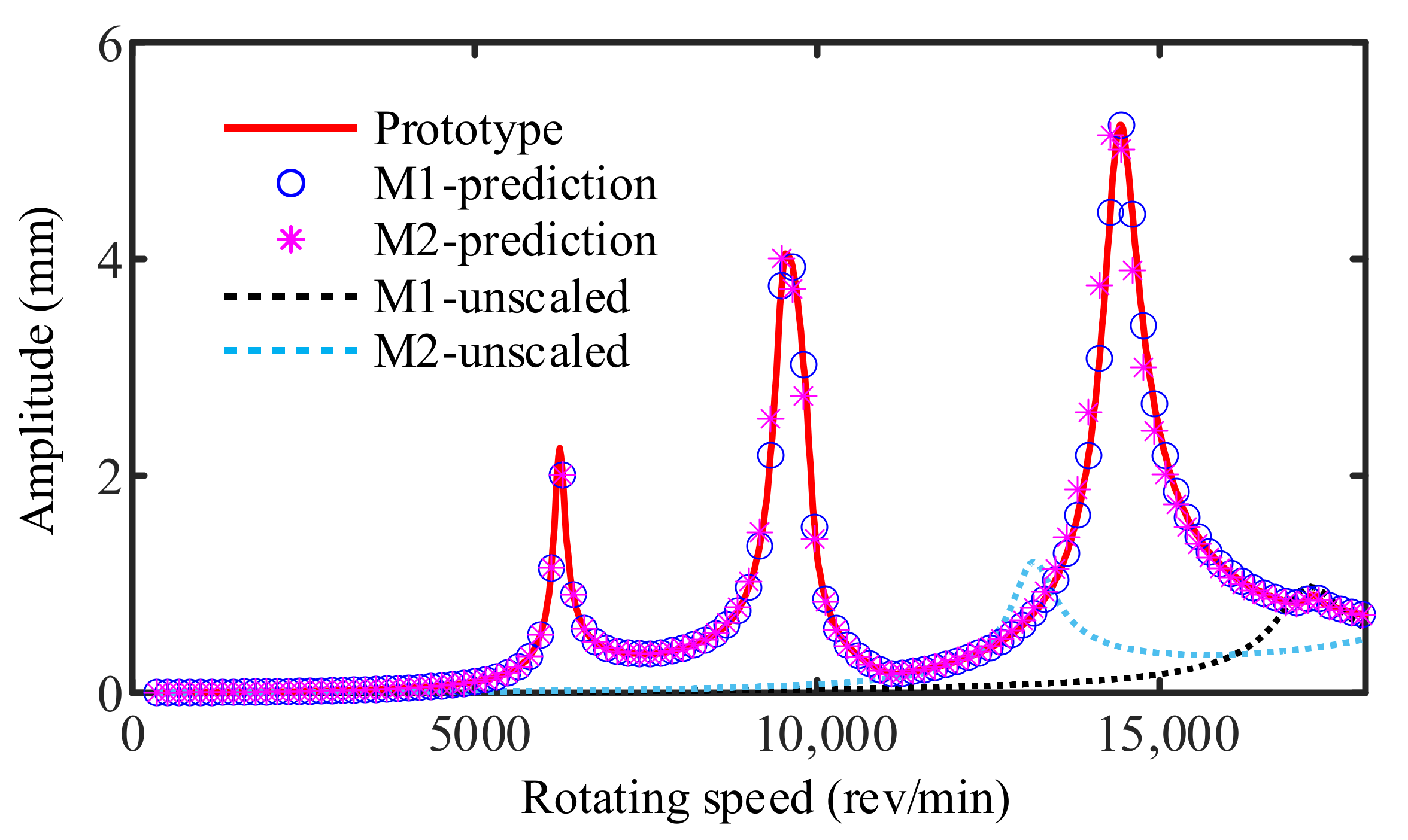
• For the case of LP rotor excitation or HP rotor excitation, only the resonance and frequency components of the LP rotor or HP rotor can be found. As for two frequency excitations, both the resonance and frequency components of LP and HP rotors can be observed. Besides, the critical speeds decrease slightly under the case of counter-rotation. The predicted results of scaled models are compared with the results of the prototype in these cases. The results for all working conditions show that the derived scaling relationships can accurately predict the critical speeds, vibration responses, and frequency components of the prototype, even though different materials and the nonlinearity introduced by bolted joints are considered.

In our future work, the case of the shaft, disk, and bearings being made of different materials will be investigated and more emphasis will be placed on the experimental verification.