Topology Identification of Time-Scales Complex Networks
Abstract
:1. Introduction
- We discuss the topology identification of complex networks on the theory of time scales, which makes the proposed criteria more general. These criteria not only applies to the continuous cases, but also to the discrete cases with arbitrary time step, and even to the intermittent cases;
- To overcome the identification failure caused by the inner synchronization of complex network, we improve the synchronization-based method on time scales by constructing a chaotic auxiliary network;
- An impulsive control method is developed ensuring that the outer synchronization is between the original network and the auxiliary network. Impulsive control criteria are offered on time scales.
2. Preliminaries
3. A Complex Network Model and Its Topology Identification
4. Modified Topology Identification Based on Impulsive Synchronization
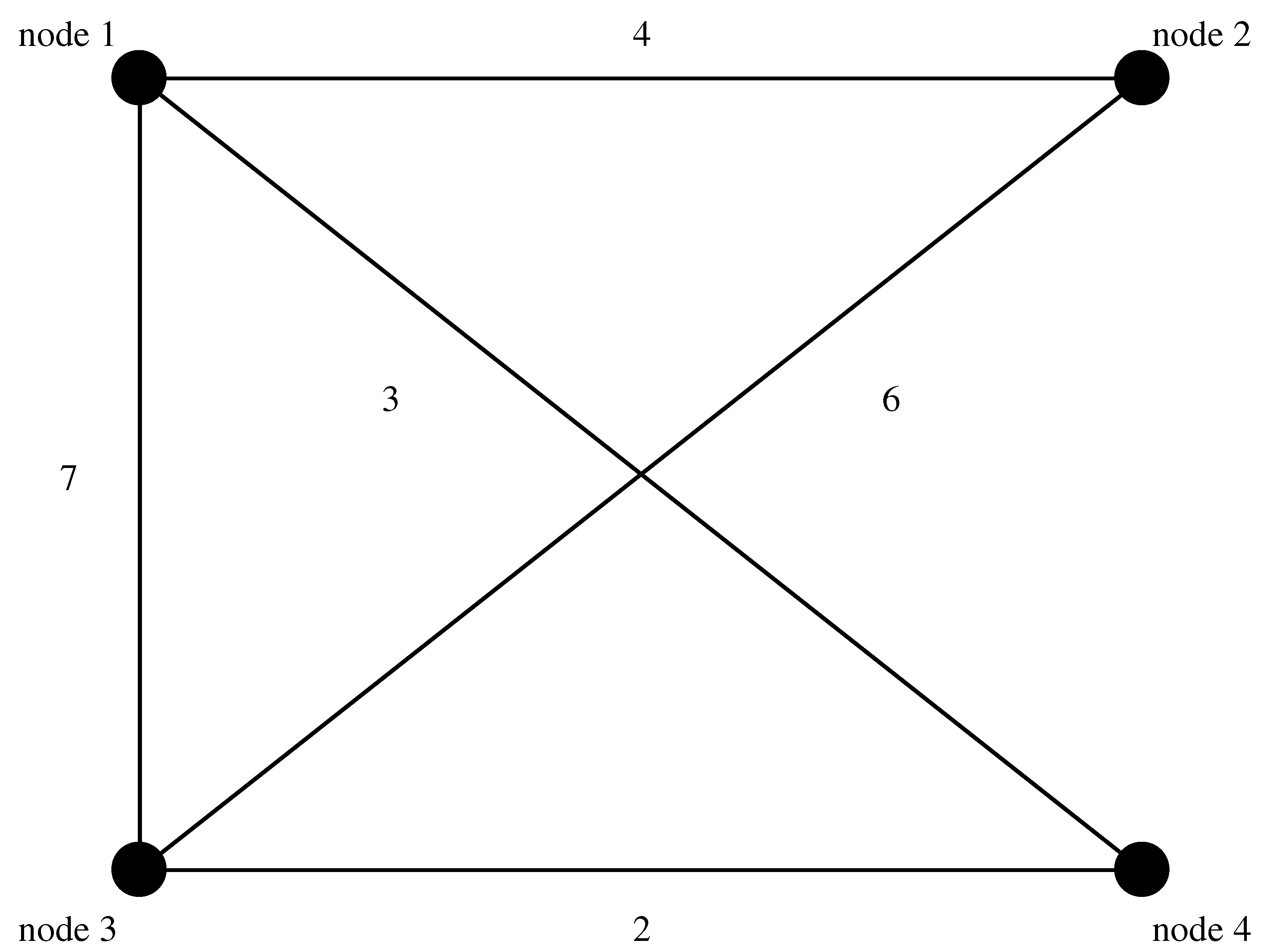
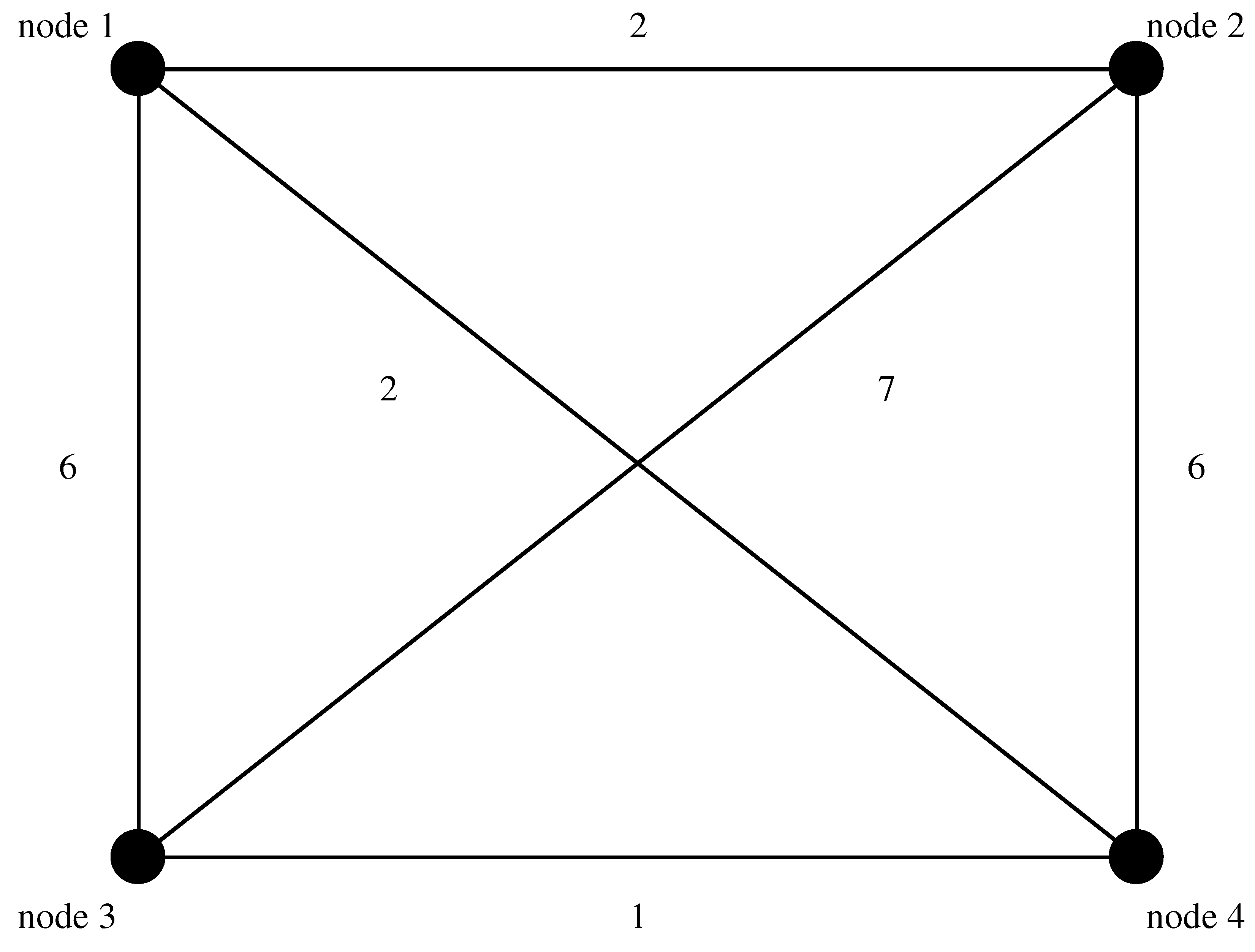
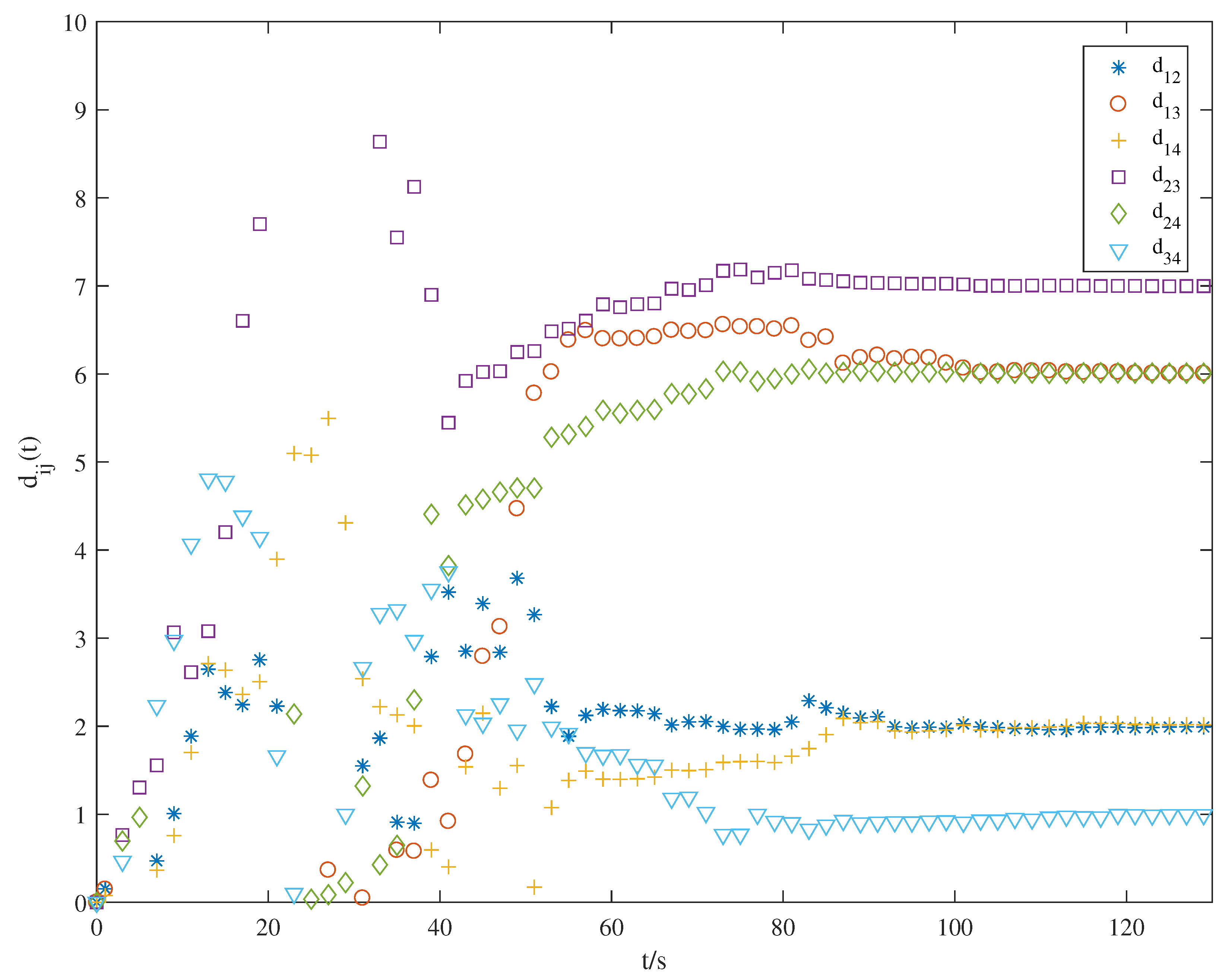
5. Numerical Examples
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Boccaletti, S.; Latora, V.; Moreno, Y.; Chavez, M.; Hwang, D.U. Complex networks: Structure and dynamics. Phys. Rep. 2006, 424, 175–308. [Google Scholar] [CrossRef]
- Schöll, E. Synchronization patterns and chimera states in complex networks: Interplay of topology and dynamics. Eur. Phys. J. Spec. Top. 2016, 225, 891–919. [Google Scholar] [CrossRef]
- Lü, L.; Chen, D.; Ren, X.L.; Zhang, Q.M.; Zhang, Y.C.; Zhou, T. Vital nodes identification in complex networks. Phys. Rep. 2016, 650, 1–63. [Google Scholar] [CrossRef] [Green Version]
- Zañudo, J.G.T.; Yang, G.; Albert, R. Structure-based control of complex networks with nonlinear dynamics. Proc. Natl. Acad. Sci. USA 2017, 114, 7234–7239. [Google Scholar] [CrossRef] [Green Version]
- Zhao, J.; Li, Q.; Lu, J.A.; Jiang, Z.P. Topology identification of complex dynamical networks. Chaos Interdiscip. J. Nonlinear Sci. 2010, 20, 023119. [Google Scholar] [CrossRef] [Green Version]
- Peng, H.; Li, L.; Kurths, J.; Li, S.; Yang, Y. Topology identification of complex network via chaotic ant swarm algorithm. Math. Probl. Eng. 2013, 2013, 401983. [Google Scholar] [CrossRef]
- Chen, J.; Lu, J.; Zhou, J. Topology identification of complex networks from noisy time series using ROC curve analysis. Nonlinear Dyn. 2014, 75, 761–768. [Google Scholar] [CrossRef]
- Yu, D.; Righero, M.; Kocarev, L. Estimating topology of networks. Phys. Rev. Lett. 2006, 97, 188701. [Google Scholar] [CrossRef] [Green Version]
- Zhou, J.; Lu, J.A. Topology identification of weighted complex dynamical networks. Phys. A Stat. Mech. Appl. 2007, 386, 481–491. [Google Scholar] [CrossRef]
- Zhao, X.; Zhou, J.; Zhu, S.; Ma, C.; Lu, J.A. Topology identification of multiplex delayed networks. IEEE Trans. Circuits Syst. II Express Briefs 2019, 67, 290–294. [Google Scholar] [CrossRef]
- Hai, X.; Yu, Y. Topology Identification of Fractional Complex Networks with An Auxiliary Network. IFAC-PapersOnLine 2020, 53, 3675–3682. [Google Scholar] [CrossRef]
- Liu, H.; Lu, J.; Lu, J. Topology identification of an uncertain general complex dynamical network. In Proceedings of the 2008 IEEE International Symposium on Circuits and Systems, Seattle, WA, USA, 18–21 May 2008; pp. 109–112. [Google Scholar]
- Zheng, Y.; Wu, X.; He, G.; Wang, W. Topology identification of fractional-order complex dynamical networks based on auxiliary-system approach. Chaos Interdiscip. J. Nonlinear Sci. 2021, 31, 043125. [Google Scholar] [CrossRef] [PubMed]
- Si, G.; Sun, Z.; Zhang, H.; Zhang, Y. Parameter estimation and topology identification of uncertain fractional order complex networks. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 5158–5171. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, X.; Lü, J.; Lu, J.a.; D’Souza, R.M. Topology identification in two-layer complex dynamical networks. IEEE Trans. Netw. Sci. Eng. 2018, 7, 538–548. [Google Scholar] [CrossRef]
- Xu, Y.; Zhou, W.; Fang, J. Topology identification of the modified complex dynamical network with non-delayed and delayed coupling. Nonlinear Dyn. 2012, 68, 195–205. [Google Scholar] [CrossRef]
- Tu, C.; Cheng, Y.; Chen, K. Estimating the varying topology of discrete-time dynamical networks with noise. Cent. Eur. J. Phys. 2013, 11, 1045–1055. [Google Scholar] [CrossRef]
- Zhao, J.; Aziz-Alaoui, M.; Bertelle, C.; Corson, N. Sinusoidal disturbance induced topology identification of Hindmarsh-Rose neural networks. Sci. China Inf. Sci. 2016, 59, 1–9. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, X.Y.; Lin, X.H. Topology identification and module–phase synchronization of neural network with time delay. IEEE Trans. Syst. Man Cybern. Syst. 2016, 47, 885–892. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, J.; Sun, W. Adaptive outer synchronization and topology identification between two complex dynamical networks with time-varying delay and disturbance. IMA J. Math. Control Inf. 2019, 36, 949–961. [Google Scholar] [CrossRef]
- Zhang, S.; Wu, X.; Lu, J.A.; Feng, H.; Lu, J. Topology identification of complex dynamical networks based on generalized outer synchronization. In Proceedings of the 33rd Chinese Control Conference, Nanjing, China, 28–30 July 2014; pp. 2763–2767. [Google Scholar]
- Che, Y.; Li, R.; Han, C.; Cui, S.; Wang, J.; Wei, X.; Deng, B. Topology identification of uncertain nonlinearly coupled complex networks with delays based on anticipatory synchronization. Chaos Interdiscip. J. Nonlinear Sci. 2013, 23, 013127. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.; Zhou, W.; Sun, W.; Pan, L. Topology identification and adaptive synchronization of uncertain complex networks with non-derivative and derivative coupling. J. Frankl. Inst. 2010, 347, 1566–1576. [Google Scholar] [CrossRef]
- Yu, D. Estimating the topology of complex dynamical networks by steady state control: Generality and limitation. Automatica 2010, 46, 2035–2040. [Google Scholar] [CrossRef]
- Zhang, Q.; Luo, J.; Wan, L. Parameter identification and synchronization of uncertain general complex networks via adaptive-impulsive control. Nonlinear Dyn. 2013, 71, 353–359. [Google Scholar] [CrossRef]
- Zhu, S.; Zhou, J.; Lu, J.a. Identifying partial topology of complex dynamical networks via a pinning mechanism. Chaos Interdiscip. J. Nonlinear Sci. 2018, 28, 043108. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.; Lu, J.a.; Chi, K.T. Synchronization: An obstacle to identification of network topology. IEEE Trans. Circuits Syst. II Express Briefs 2009, 56, 310–314. [Google Scholar] [CrossRef]
- Zhu, S.; Zhou, J.; Chen, G.; Lu, J.A. A new method for topology identification of complex dynamical networks. IEEE Trans. Cybern. 2019, 51, 2224–2231. [Google Scholar] [CrossRef]
- Liu, H.; Li, Y.; Li, Z.; Lü, J.; Lu, J.A. Topology Identification of Multilink Complex Dynamical Networks via Adaptive Observers Incorporating Chaotic Exosignals. IEEE Trans. Cybern. 2021. [Google Scholar] [CrossRef]
- Cheng, Q.; Cao, J. Global synchronization of complex networks with discrete time delays on time scales. Discret. Dyn. Nat. Soc. 2011, 2011, 287670. [Google Scholar] [CrossRef]
- Chen, X.; Song, Q. Global stability of complex-valued neural networks with both leakage time delay and discrete time delay on time scales. Neurocomputing 2013, 121, 254–264. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, K. Synchronization of linear dynamical networks on time scales: Pinning control via delayed impulses. Automatica 2016, 72, 147–152. [Google Scholar] [CrossRef]
- Ogulenko, A. Asymptotical properties of social network dynamics on time scales. J. Comput. Appl. Math. 2017, 319, 413–422. [Google Scholar] [CrossRef] [Green Version]
- Huang, Z.; Cao, J.; Raffoul, Y.N. Hilger-type impulsive differential inequality and its application to impulsive synchronization of delayed complex networks on time scales. Sci. China Inf. Sci. 2018, 61, 1–3. [Google Scholar] [CrossRef]
- Wang, B.; Zhang, Y.; Zhang, B. Exponential synchronization of nonlinear complex networks via intermittent pinning control on time scales. Nonlinear Anal. Hybrid Syst. 2020, 37, 100903. [Google Scholar] [CrossRef]
- Ali, M.S.; Yogambigai, J. Synchronization of complex dynamical networks with hybrid coupling delays on time scales by handling multitude Kronecker product terms. Appl. Math. Comput. 2016, 291, 244–258. [Google Scholar]
- Xiao, Q.; Lewis, F.L.; Zeng, Z. Event-based time-interval pinning control for complex networks on time scales and applications. IEEE Trans. Ind. Electron. 2018, 65, 8797–8808. [Google Scholar] [CrossRef]
- Pei, Y.; Bohner, M.; Pi, D. Impulsive synchronization of time-scales complex networks with time-varying topology. Commun. Nonlinear Sci. Numer. Simul. 2020, 80, 104981. [Google Scholar] [CrossRef]
- Cheng, Q.; Cao, J. Synchronization of complex dynamical networks with discrete time delays on time scales. Neurocomputing 2015, 151, 729–736. [Google Scholar] [CrossRef]
- Bohner, M.; Peterson, A. Dynamic Equations on Time Scales: An Introduction with Applications; Birkhäuser Boston, Inc.: Boston, MA, USA, 2001; 358p. [Google Scholar] [CrossRef] [Green Version]
- Bohner, M.; Peterson, A.C. Advances in Dynamic Equations on Time Scales; Springer Science & Business Media: Berlin, Germany, 2002. [Google Scholar]
- Boyd, S.; El Ghaoui, L.; Feron, E.; Balakrishnan, V. Linear Matrix Inequalities in System and Control Theory; SIAM: Philadelphia, PA, USA, 1994. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pei, Y.; Chen, C.; Pi, D. Topology Identification of Time-Scales Complex Networks. Mathematics 2022, 10, 1755. https://doi.org/10.3390/math10101755
Pei Y, Chen C, Pi D. Topology Identification of Time-Scales Complex Networks. Mathematics. 2022; 10(10):1755. https://doi.org/10.3390/math10101755
Chicago/Turabian StylePei, Yong, Churong Chen, and Dechang Pi. 2022. "Topology Identification of Time-Scales Complex Networks" Mathematics 10, no. 10: 1755. https://doi.org/10.3390/math10101755
APA StylePei, Y., Chen, C., & Pi, D. (2022). Topology Identification of Time-Scales Complex Networks. Mathematics, 10(10), 1755. https://doi.org/10.3390/math10101755





