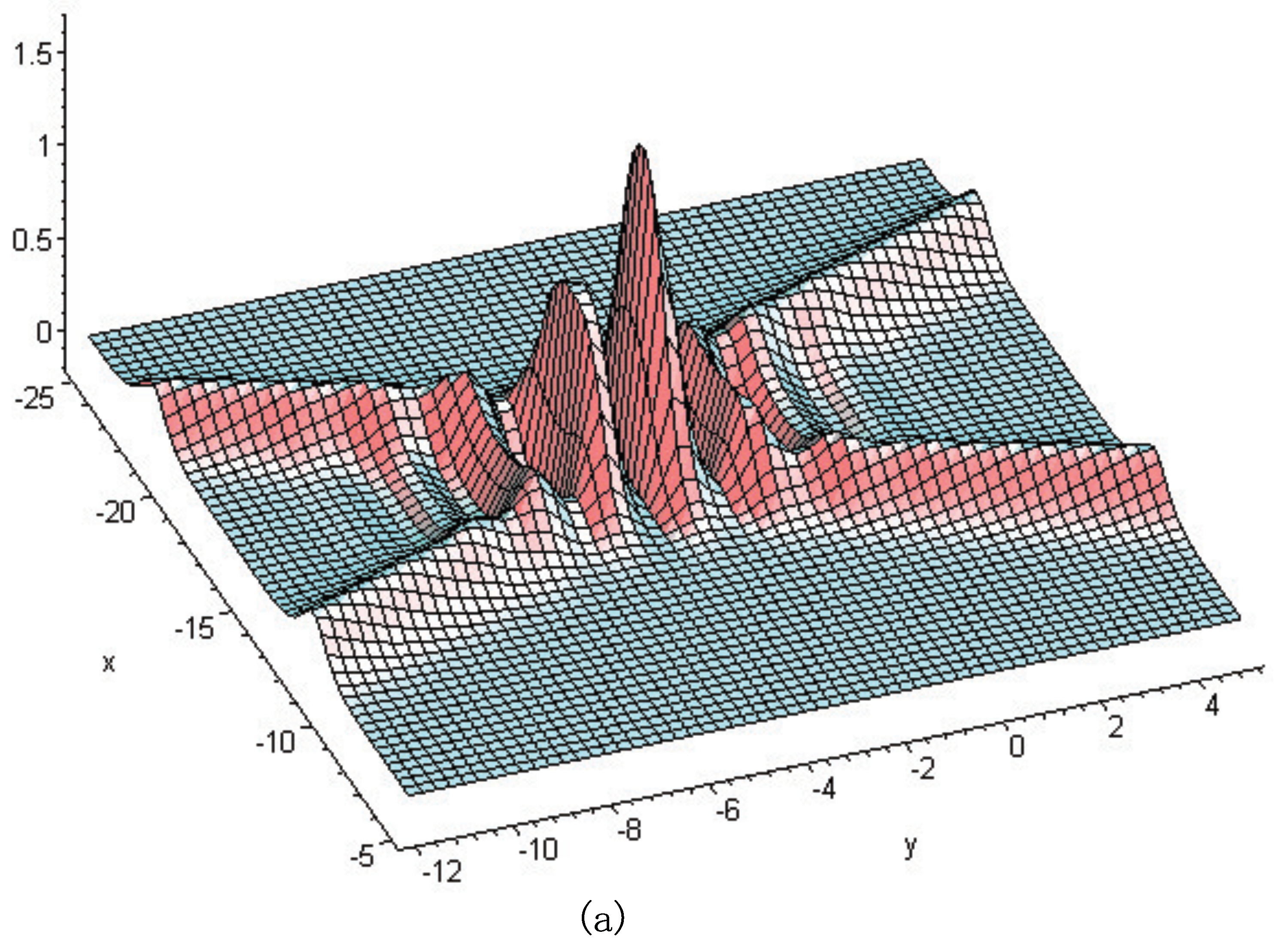
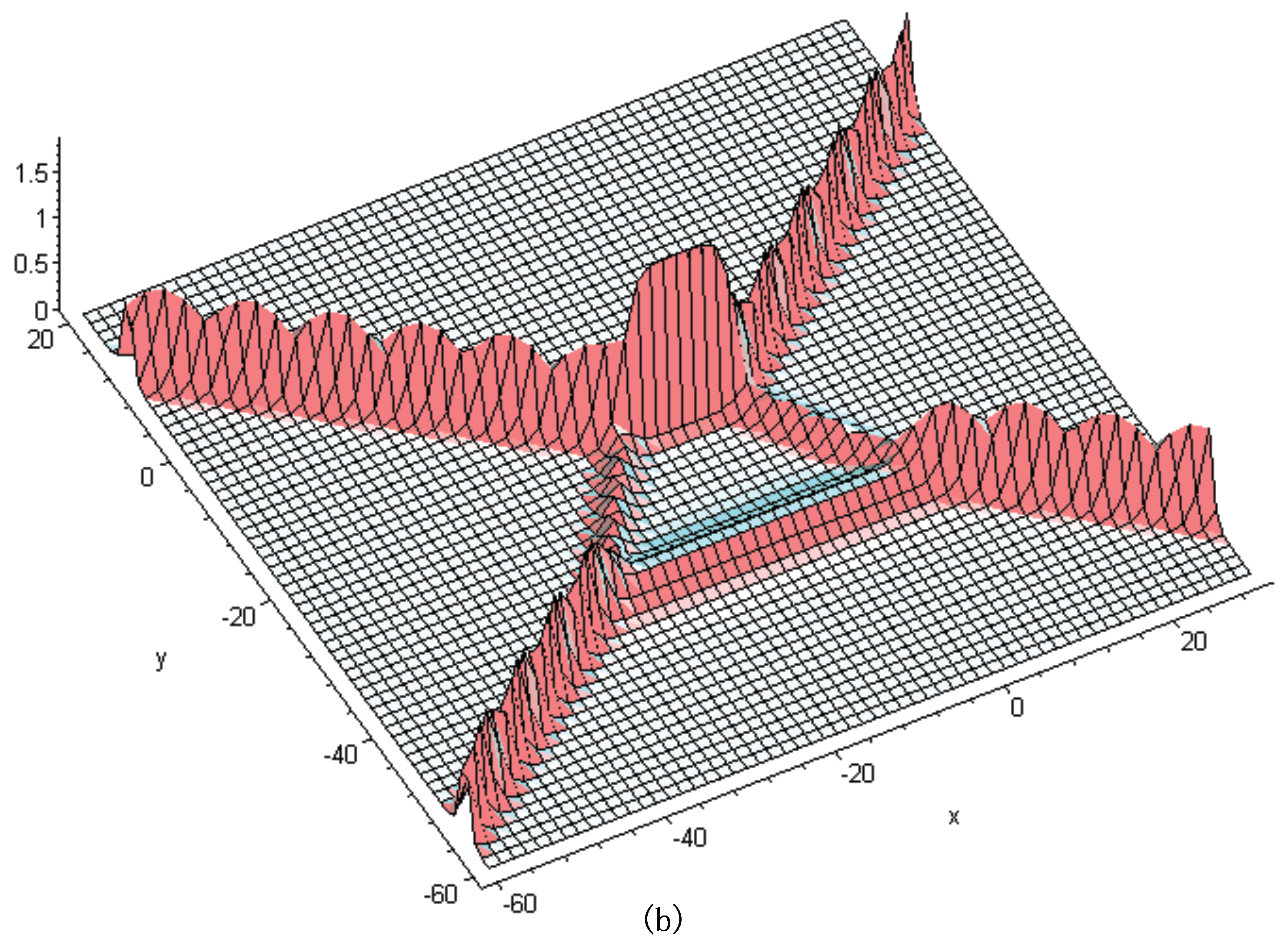
New Localized Structure for (2+1) Dimensional Boussinesq-Kadomtsev-Petviashvili Equation

Abstract

In this work, we use a variable separation approach to construct some novel exact solutions of a (2+1)-dimensional Boussinesq-Kadomtsev-Petviashvili equation. Thanks to two variable-separated arbitrary functions, some new soliton excitations and localized structures are obtained. It is observed that large amplitude waves are generated in the process of interaction between two solitons.

1. Introduction

The soliton concept has appeared in various nonlinear partial differential equations (PDEs). It results from interplay between the nonlinear and linear dispersive effects and propagates stably without distortion of shape with particle-like properties [1]. In an integrable system, solitary waves collide elastically and retain their identity after collisions [2,3,4,5,6]. However, the collision may be highly complex in non-integrable system [7]. Its application can be found in many areas of physics, including nonlinear optics and plasma physics [8,9,10]. There are many powerful methods to construct exact solutions of nonlinear evolution equations, for example, the inverse scattering transform [10], Hirota’s bilinear operators [11], the Jacobi elliptic function expansion [12], variable separation approach [13], etc. Exact solutions of nonlinear evolution equations have been used to study various collision scenarios in a large array of physical systems, such as transmission, reflection, annihilation, trapping, and creation of solitary waves. In particular, thanks to the arbitrary functions in the solutions, a variable separation approach initiated by Professor Lou [13] has been used to develop various kinds of interesting local structures, including multi-dromion solutions driven by multiple straight line ghost solitons, dromion solutions with oscillated tails, ring soliton solutions, standing and moving breather-like structures, chaotic dromions, resonant dromion and solitoff solutions, and foldon interactions [13,14,15,16,17].

In this work, we will study the (2+1)-dimensional Boussinesq-Kadomtsev-Petviashvili (B-KP) hierarchy (KP hierarchy of B-type) [18].

$$\begin{array}{c}{\displaystyle w_t+w_{xxx}+w_{yyy}+6{(uw)}_x+6{(vw)}_y=0,}\hfill \end{array}$$

(1)

$$\begin{array}{c}{\displaystyle u_y=w_x,v_x=w_y.}\hfill \end{array}$$

(2)

This equation is integrable and is related to a Clifford algebra generated by two neutral fermion fields. Many researchers has used different methods to search for explicit solutions of Boussinesq-Kadomtsev-Petviashvili equation. By using some exact solutions of the auxiliary ordinary differential equation, Ma et al. have constructed its exact complex [19]. The authors in [20] have studied its various exact solutions by using the bifurcation theory of dynamical systems. Zhang and Cheng’s groups have also constructed its exact solutions by the $\frac{G^{{}^{\prime }}}{G}$-expansion method and the first integral method [21,22]. Recently, Akinyemi et al. have obtained numerous exact solutions of generalized (B-KP)-like equations with four different forms by using sub-equation method [23]. We also notice that some exact solutions of Kadomtsev–Petviashvili equation of B-type with the other version were also studied extensively in [24,25,26]. The aim of this paper is to study Boussinesq-Kadomtsev-Petviashvili equation by variable separation approach to obtain new soliton excitations.

This paper is organized as follows: In Section 2, we obtain a variable separation solution of the Boussinesq-Kadomtsev-Petviashvili equation with the aid of a variable separation approach. In Section 3, various soliton excitations are constructed using the arbitrariness of the functions of p and q in a variable separation solution. In Section 4, a simple conclusion is presented.

2. Variable Separation Approach for Boussinesq-Kadomtsev-Petviashvili Equation

For details of the variable separation approach, the readers are directed to reference [13]. In this section, we will use the variable separation approach to construct solutions of the Boussinesq-Kadomtsev-Petviashvili equation. To this aim, we need to take the following transformation:

$$\begin{array}{c}{\displaystyle w={(lnf)}_{xy},}\hfill \\ u={(lnf)}_{xx}+u_0(x,t),\hfill \\ v={(lnf)}_{yy}+v_0(y,t),\hfill \end{array}$$

(3)

where $u_0(x,t)$ and $v_0(y,t)$ are two arbitrary functions. In fact, $\{w=0,u_0(x,t),v=v_0(y,t)\}$ is a solution of the Boussinesq-Kadomtsev-Petviashvili equation. Substituting (3) into (1) leads to a trilinear form

$$\begin{array}{c}{\displaystyle f_{xyt}{f}^2-f_{x^{4}y}{f}^2-f_{y^{4}x}{f}^2+f_{x^4}{f}_{y}f+f_x{f}_{y^4}f-6f_{xxy}{f}_x^2-6f_{xyy}{f}_y^2}\hfill \\ +4f_{x^{3}y}{f}_{x}f-f_{xy}{f}_{t}f-f_{xt}{f}_{y}f-f_x{f}_{yt}f-2f_{xy}{f}_{xxx}f+4f_{x{y}^3}{f}_{y}f\hfill \\ -2f_{xy}{f}_{y^3}f+2f_x{f}_y{f}_t+6f_{xy}{f}_x{f}_{xx}-2f_{x^3}{f}_y{f}_x+6f_{xy}{f}_y{f}_{yy}-2f_x{f}_y{f}_{y^3}\hfill \\ +6f(f_x{f}_y-f_{xy}f)(u_{0x}+v_{0y})+v_0(6f_x{f}_{yy}f+12f_{xy}{f}_{y}f-12f_x{f}_y^2-6f_{xyy}{f}^2)\hfill \\ +u_0(6f_y{f}_{xx}f+12f_{xy}{f}_{x}f-12f_y{f}_x^2-6f_{xxy}{f}^2)=0.\hfill \end{array}$$

(4)

According to variable separation approach, we utilize the assumption in [13]

$$\begin{array}{c}{\displaystyle f(x,y,t)=a_0+a_{1}p\left(x,t\right)+a_{2}q\left(y,t\right)+a_{3}p\left(x,t\right)q\left(y,t\right).}\hfill \end{array}$$

(5)

Substituting ansatz (5) into (4), we have

$$\begin{array}{c}{\displaystyle f[p_{xt}{q}_y-6u_0{q}_y{p}_{xx}-q_y{p}_{xxxx}-6p_x(v_{0y}{q}_y+q_y{u}_{0x})+p_x(q_{yt}-6v_0{q}_{yy}-q_{yyyy})]}\hfill \\ +2q_y{p}_x[(a_2+a_{3}p)(6q_y{v}_0+q_{yyy}-q_t)+(a_1+a_{3}q)(6u_0{p}_x+p_{xxx}-p_t)]=0.\hfill \end{array}$$

(6)

With the arbitrariness of the functions $v_0$ and $w_0$, we directly obtain

$$\begin{array}{ccc}& & {\displaystyle u_0\left(x,t\right)=\frac{6c_1(t)\left(a_2+a_{3}p\right)+p_t-p_{xxx}}{6p_x},}\hfill \end{array}$$

(7)

$$\begin{array}{ccc}& & {\displaystyle v_0\left(y,t\right)=\frac{-6c_1(t)\left(a_1+a_{3}q\right)+q_t-q_{yyy}}{6q_y},}\hfill \end{array}$$

(8)

then $p(x,t)$, $q(y,t)$ and $c_1(t)$ become two arbitrary functions. Finally, the variable separation solution of (2+1)-D B-KP equation is derived as

$$\begin{array}{ccc}& & {\displaystyle w=\frac{p_x{q}_y(a_3{a}_0-a_1{a}_2)}{{(a_0+a_{1}p+a_{2}q+a_{3}pq)}^2},}\hfill \end{array}$$

(9)

$$\begin{array}{ccc}& & {\displaystyle u=\frac{-{(q{a}_3+a_1)}^2{p}_x^2+(a_1+a_{3}q)f{p}_{xx}}{{(a_0+a_{1}p+a_{2}q+a_{3}pq)}^2}+\frac{6c_1(t)\left(a_2+a_{3}p\right)+p_t-p_{xxx}}{6p_x},}\hfill \end{array}$$

(10)

$$\begin{array}{ccc}& & {\displaystyle v=\frac{-{(p{a}_3+a_2)}^2{q}_y^2+(a_2+a_{3}p)f{q}_{yy}}{{(a_0+a_{1}p+a_{2}q+a_{3}pq)}^2}+\frac{-6c_1(t)\left(a_1+a_{3}q\right)+q_t-q_{yyy}}{6q_y}.}\hfill \end{array}$$

(11)

Now, one could use Equation (9), called a “universal” formula in [27], to construct a coherent structure of a (2+1) dimensional B-KP equation. Due to the arbitrariness of the functions of $p,q$ and $c(t)$, we will construct some novel localized structures of (2+1) dimensional B-KP equation in the next section.

3. Soliton Excitations

(i) Two soliton interactions affected by a periodic perturbation. If the arbitrary functions $p(x,t)$ and $q(y,t)$ are simply selected as cosh function together with periodic cosine function with the following form

$$\begin{array}{c}{\displaystyle p=2cosh\left(kx+k^{3}t\right),q=16cosh\left(l_{1}y+{l_1}^{3}t\right)+cos(l_{2}y+l_2^{3}t),}\hfill \end{array}$$

(12)

where $k,l_1$ and $l_2$ are some arbitrary real numbers. Figure 1a shows the two solitons produces breathing effect at the position of the two soliton collision resulting from periodic cosine function; however, the two solitons preserve the initial shape after the collision due to soliton stability.

Figure 1. The snapshot of two soliton interaction at the time $t=6$. (a). The arbitrary function in Equation (3) for v is taken as Equation (12) for $l_1=1,l_2=4,k=1$, at $t=6$. (b). Under the condition Equation (13) for $l_1=1,l_2=-2,k=1.4,{\xi }_0=1,{\xi }_1=2,{\xi }_2=3$, respectively.

(ii) Two soliton interplay with complex dynamic structure. If the arbitrary functions $p(x,t)$ and $q(y,t)$ are simply selected as a cosh function in the following form

$$\begin{array}{c}{\displaystyle p=cosh\left(kx+k^{3}t+{\xi }_0\right),q=cosh\left(l_{1}y+{l_1}^{3}t+{\xi }_1\right)+cosh\left(l_{2}y+{l_2}^{2}t+{\xi }_2\right),}\hfill \end{array}$$

(13)

where $k, l_1, l_2, {\xi }_0, {\xi }_1$ and ${\xi }_2$ are some arbitrary real numbers. It is remarked that ${\xi }_0, {\xi }_1$ and ${\xi }_2$ are shifting parameters and they have no influence for the dynamics of soliton excitations. In this case, from Figure 1b, after the two soliton head-on collision, they pass though a comparable complex process.

4. Conclusions

In this work, we have obtained two kinds of variable separation solutions of (2+1)-dimensional Boussinesq-Kadomtsev-Petviashvili equation by a variable separation approach. By choosing combination of cosh function and periodic cosine function, two different soliton excitation scenes are observed graphically. In case (i), at the moment of collision, we found that breathing behavior is generated and the collision process is more long than the ordinary iteration between two solitons. In case (ii), two solitons interact in the long range and resonant. In both cases, we see that large amplitude waves are generated. Recently, the large amplitude wave phenomenon is a hot topic in the field of the rogue wave community [28]. We will study this topic in the future by a variable separation approach.