A Fractional Order Model to Study the Effectiveness of Government Measures and Public Behaviours in COVID-19 Pandemic
Abstract
:1. Introduction
- Finding analytical solutions is difficult for Caputo differential systems.
- There are many concepts, such as bifurcation theory, parametric optimisation, persistence, etc., that have not yet been developed for Caputo fractional order systems.
- The numerical algorithms for delayed systems and stochastic fractional systems have not yet been developed.
2. Model Construction
3. Basic Nonlinear Analysis
3.1. Case 1: Model without Control
- Disease-free equilibrium:
- Endemic equilibrium:
- and
- and
- and.
3.2. Case 2: Model with Effects of Governmental Action and Additional Control
4. Sensitivity Analysis
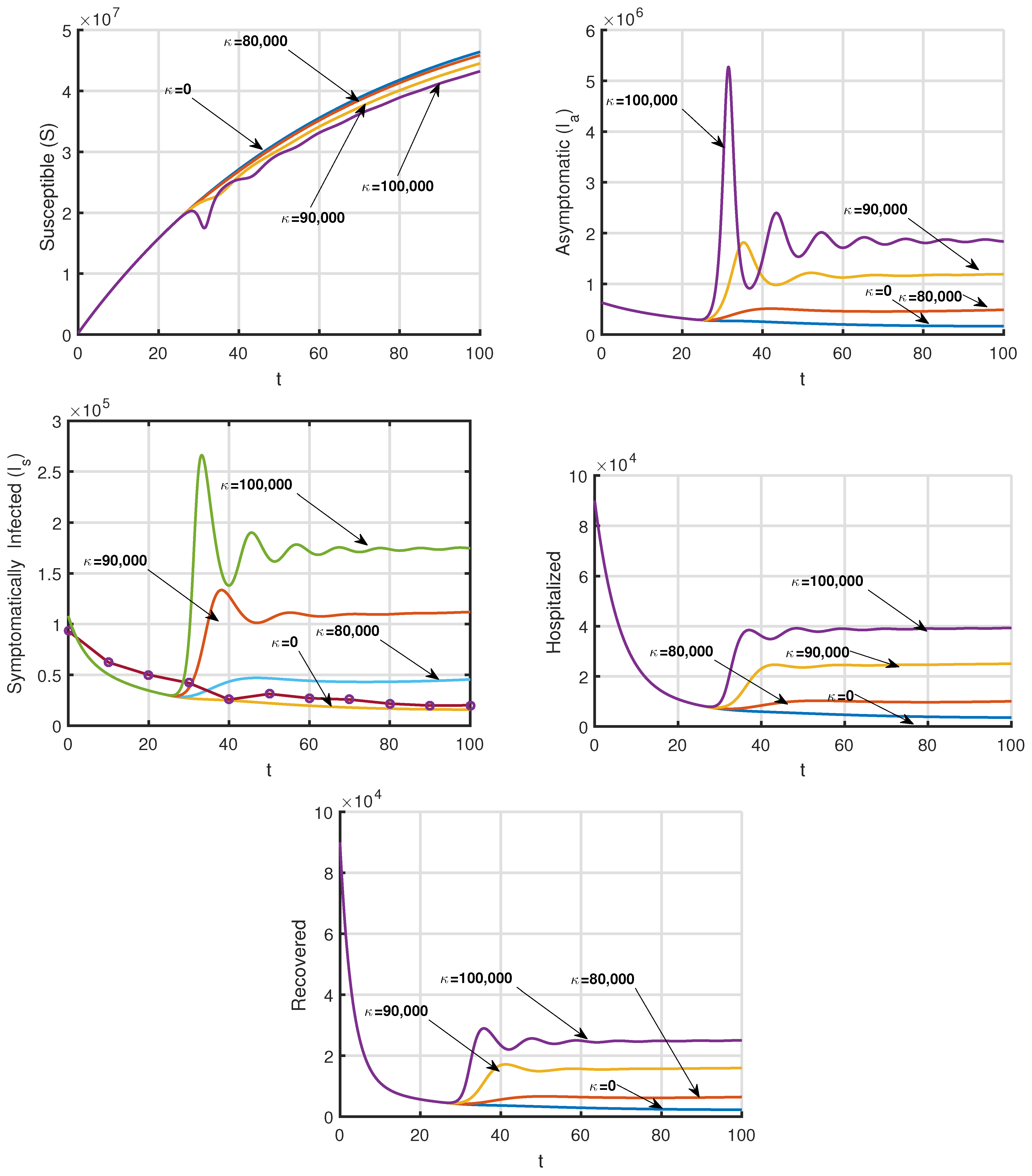
5. Numerical Simulations
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- WHO COVID-19 Dashboard; World Health Organization: Geneva, Switzerland, 2020. Available online: https://covid19.who.int/ (accessed on 23 June 2022).
- Islam, K.; Ali, S.; Akanda, S.Z.R.; Rahman, S.; Kamruzzaman, A.H.M. COVID-19 Pandemic and Level of Responses in Bangladesh. Int. J. Rare Dis. Disord. 2020, 3, 19. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Petras, I. Fractional-Order Nonlinear Systems: Modeling Aanlysis and Simulation; Higher Education Press: Beijing, China, 2011. [Google Scholar]
- Du, M.; Wang, Z.; Hu, H. Measuring memory with the order of fractional derivative. Sci. Rep. 2013, 3, 3431. [Google Scholar] [CrossRef] [PubMed]
- Podlubny, I. Geometric and Physical Interpretation of Fractional Integration and Fractional Differentiation. Fract. Calc. Appl. Anal. 2002, 5, 367–386. [Google Scholar]
- Sardar, T.; Rana, S.; Bhattacharya, S.; Al-Khaled, K.; Chattopadhyay, J. A generic model for a single strain mosquito-transmitted disease with memory on the host and the vector. Math. Biosci. 2015, 263, 18–36. [Google Scholar] [CrossRef] [PubMed]
- Das, M.; Maiti, A.; Samanta, G.P. Stability analysis of a prey-predator fractional order model incorporating prey refuge. Ecol. Genet. Genom. 2018, 7-8, 33–46. [Google Scholar] [CrossRef]
- Das, M.; Samanta, G.P. A prey-predator fractional order model with fear effect and group defense. Int. J. Dyn. Control. 2020, 9, 334–349. [Google Scholar] [CrossRef]
- Das, M.; Samanta, G.P. A delayed fractional order food chain model with fear effect and prey refuge. Math. Comput. Simul. 2020, 178, 218–245. [Google Scholar] [CrossRef]
- Das, M.; Samanta, G.P. Optimal Control of Fractional Order COVID-19 Epidemic Spreading in Japan and India 2020. Biophys. Rev. Lett. 2020, 15, 207–236. [Google Scholar] [CrossRef]
- Das, M.; Samanta, G.P. Stability analysis of a fractional ordered COVID-19 model. Comput. Math. Biophys. 2021, 9, 22–45. [Google Scholar] [CrossRef]
- Das, M.; Samanta, G.P.; De la Sen, M. A Fractional Ordered COVID-19 Model Incorporating Comorbidity and Vaccination. Mathematics 2021, 9, 2806. [Google Scholar] [CrossRef]
- Khan, M.A.; Atangana, A. Modeling the dynamics of novel coronavirus (2019-nCov) with fractional derivative. Alex. Eng. J. 2020, 59, 2379–2389. [Google Scholar] [CrossRef]
- Liu, Z.; Magal, P.; Seyd, O.; Webb, G. A COVID-19 epidemic model with latency period. Infect. Dis. Model. 2020, 5, 323–337. [Google Scholar] [CrossRef] [PubMed]
- Shaikh, A.S.; Shaikh, I.N.; Nisar, K.S. A mathematical model of COVID-19 using fractional derivative: Outbreak in India with dynamics of transmission and control. Adv. Differ. Equ. 2020, 373, 1–19. [Google Scholar] [CrossRef] [PubMed]
- Xie, Y.; Wang, Z.; Meng, B.; Xia Huang, X. Dynamical analysis for a fractional-order prey–predator model with Holling III type functional response and discontinuous harvest. Appl. Math. Lett. 2020, 106, 106342. [Google Scholar] [CrossRef]
- Wang, X.; Wang, Z.; Xia, J. Stability and bifurcation control of a delayed fractional-order eco-epidemiological model with incommensurate orders. J. Frankl. Inst. 2019, 356, 8278–8295. [Google Scholar] [CrossRef]
- Shen, M.; Peng, Z.; Xiao, Y.; Zhang, L. Modelling the epidemic trend of the 2019 novel coronavirus outbreak in China. bioRxiv 2020. [Google Scholar] [CrossRef]
- Wikipedia Contributors. Statistics of the COVID-19 Pandemic in Bangladesh. In Wikipedia, The Free Encyclopedia. Retrieved 19:19. 12 June 2022. Available online: https://en.wikipedia.org/wiki/Statistics_of_the_COVID-19_pandemic_in_Bangladesh (accessed on 16 June 2022).
- Kwuimy, C.A.K.; Nazari, F.; Jiao, X.; Rohani, P.; Nataraj, C. Nonlinear dynamic analysis of an epidemiological model for COVID-19 including public behavior and government action. Nonlinear Dyn. 2020, 101, 1545–1559. [Google Scholar] [CrossRef] [PubMed]
- Van den Driessche, P.; Watmough, J. Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math. Biosci. 2002, 180, 29–48. [Google Scholar] [CrossRef]
- Gelf, I.M.; Kapranov, M.M.; Zelevinsky, A.V. Discriminants, Resultants, and Multidimensional Determinants; Birkhäuser: Boston, MA, USA, 1994; ISBN 978-0-8176-3660-9. [Google Scholar]
- Ahmed, E.; El-Sayed, A.M.A.; El-Saka, H. On some Routh–Hurwitz conditions for fractional order differential equations and their applications in Lorenz, Rössler, Chua and Chen systems. Phys. Lett. A 2006, 358, 1–4. [Google Scholar] [CrossRef]
- Arriola, L.; Hyman, J. Sensitivity Analysis for Uncertainty Quantification in Mathematical Models. In Mathematical and Statistical Estimation Approaches in Epidemiology. Chowell, G., Hyman, J.M., Bettencourt, L.M.A., Castillo-Chavez, C., Eds.; Springer: Dordrecht, The Netherland, 2009. [Google Scholar] [CrossRef]
- Garrappa, R. On linear stability of predictor-corrector algorithms for fractional differential Equations. Int. J. Comput. Math. 2010, 87, 2281–2290. [Google Scholar] [CrossRef]
- COVID-19 Coronavirus Pandemic. 2022. Available online: https://www.worldometers.info/coronavirus/country/italy (accessed on 3 September 2020).
- World-Population. Bangladesh. Available online: https://www.worldometers.info/world-population/bangladesh-population (accessed on 26 June 2022).
- Garrappa, R. Predictor-Corrector PECE Method for Fractional Differential Equations. MATLAB Central File Exchange. Available online: https://www.mathworks.com/matlabcentral/fileexchange/32918-predictor-corrector-pece-method-for-fractional-differential-equations (accessed on 16 August 2022).

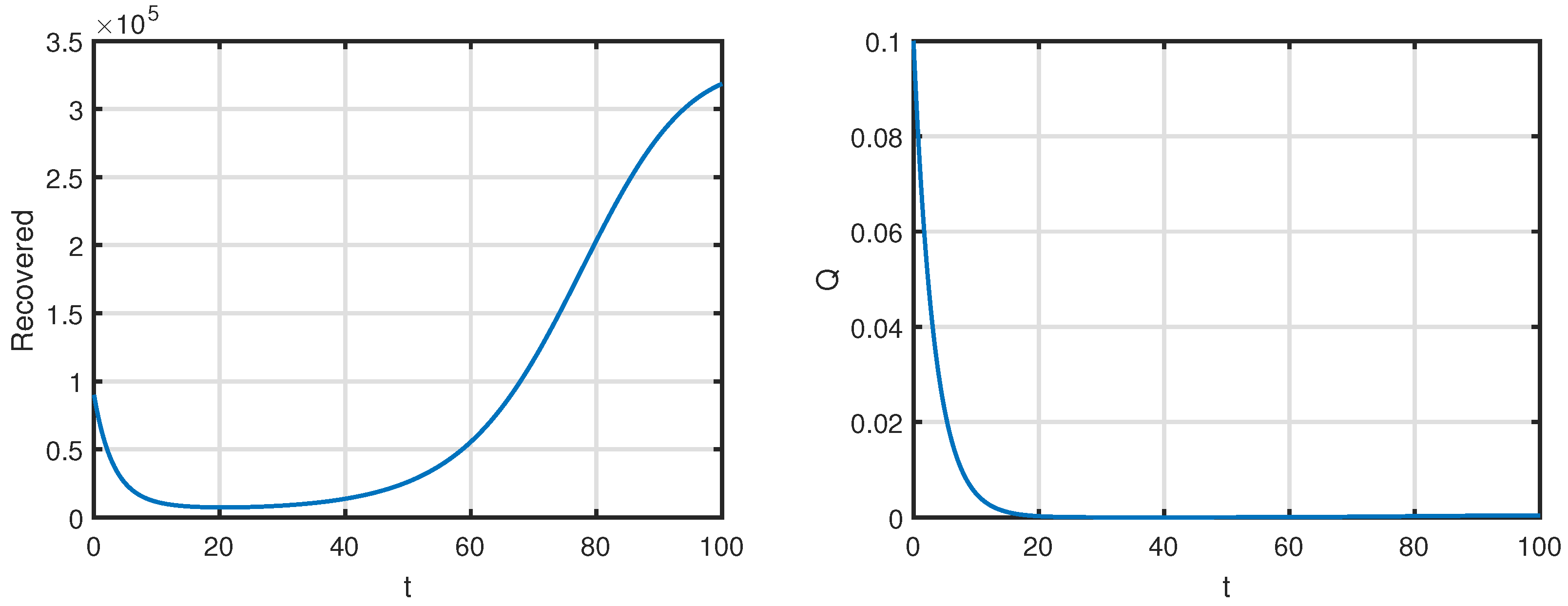
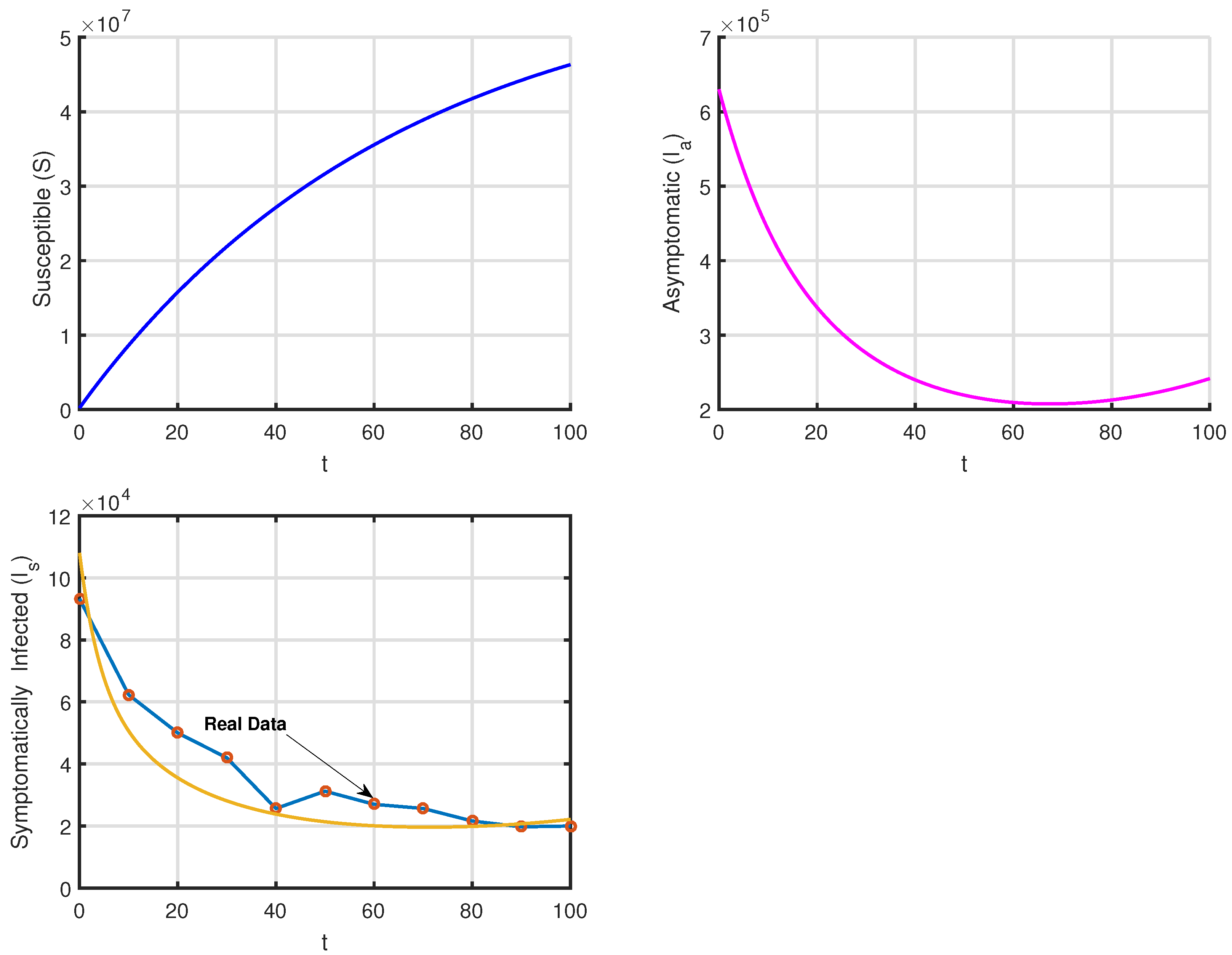

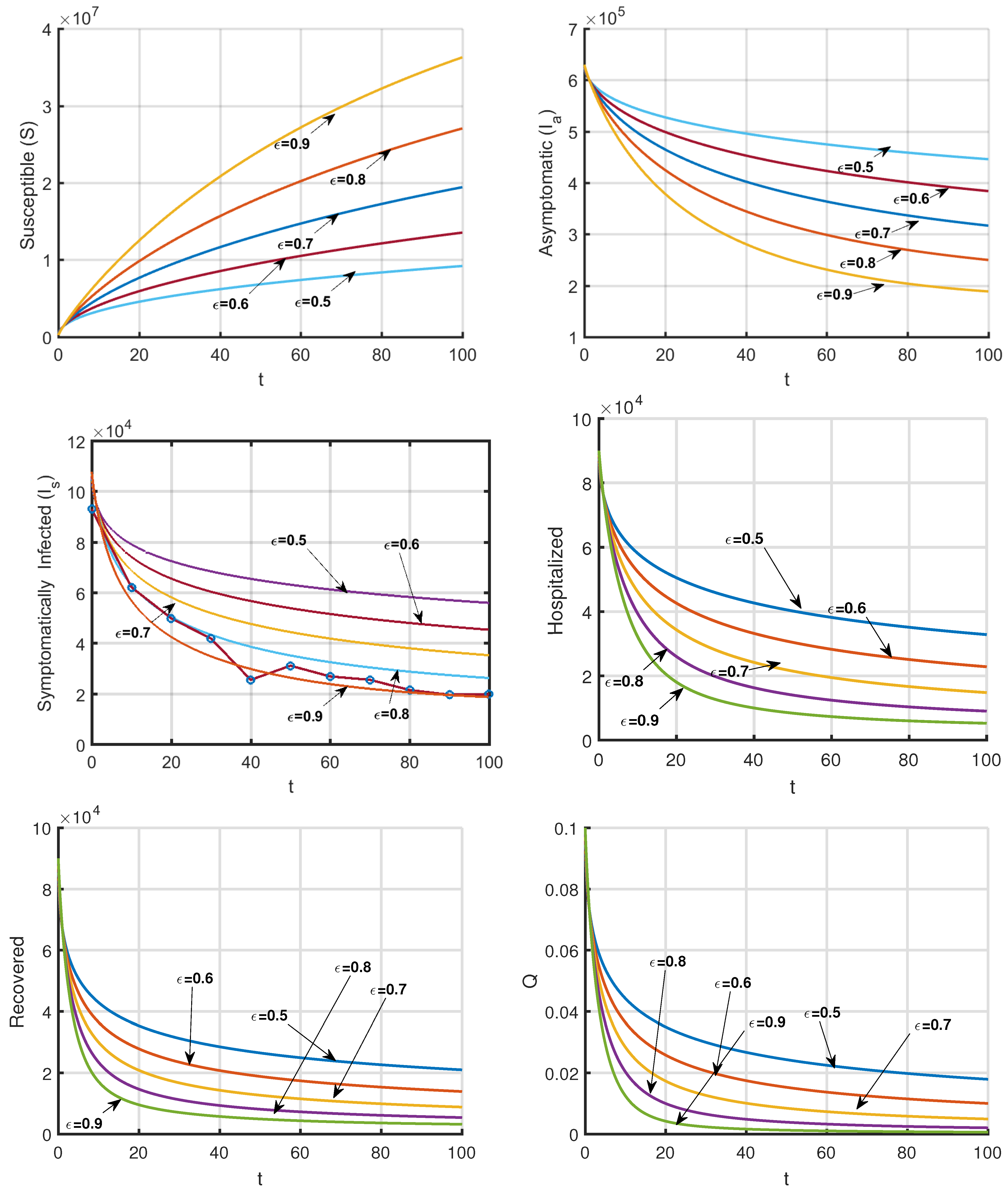
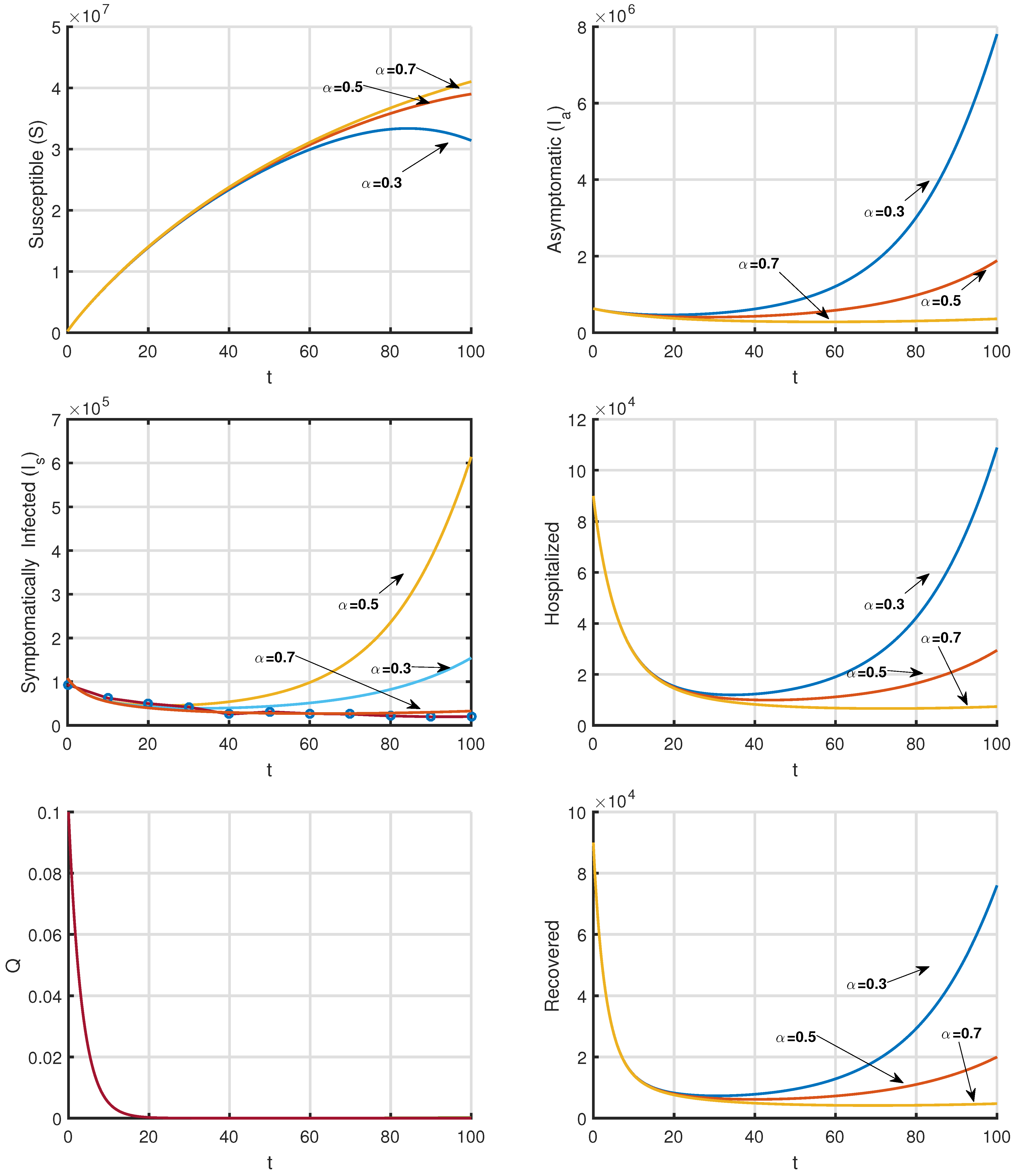
| Parameter | Interpretation | Values (Range) | Reference |
|---|---|---|---|
| recruitment rate of the human population | 0.001 | [20] | |
| rate of infection per unit of time by the symptomatic infected | 0.35 (0.005–0.34) | [21] | |
| reduction factor of infected population by the class compared to class | 0.32 (0.005–0.34) | [21] | |
| rate at which asymptomatic becomes symptomatic | 0.025 (0.02–0.1) | [21] | |
| rate at which the symptomatic infected individuals are hospitalised | 0.07 | Assumed | |
| rate of recovery of the symptomatic infected individuals | 0.14 | Assumed | |
| rate of mortality of symptomatic infected individuals | 0.05 (0.05–0.1) | [20] | |
| rate of retreat from recovered class to susceptible class | 0.1 | [21] | |
| rate of mortality of hospitalised individuals | 0.07 | [20] | |
| rate of transfer of hospitalised individuals to recovered class | 0.05 | Assumed | |
| order of fractional derivative | 0.95 (0–1) | Assumed |
| Parameters | Sensitivity Index |
|---|---|
| +1 | |
| −0.5502 | |
| −0.0127 | |
| −0.0141 | |
| +0.0748 | |
| +0.9252 |
| Day | Active Cases |
|---|---|
| 1 March 2022 | 93,206 |
| 11 March 2022 | 62,302 |
| 21 March 2022 | 50,030 |
| 31 March 2022 | 42,010 |
| 10 April 2022 | 25,650 |
| 20 April 2022 | 31,241 |
| 31 April 2022 | 27,005 |
| 10 May 2022 | 25,665 |
| 20 May 2022 | 21,616 |
| 30 May 2022 | 19,739 |
| 10 May 2022 | 20,011 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Das, M.; Samanta, G.; De la Sen, M. A Fractional Order Model to Study the Effectiveness of Government Measures and Public Behaviours in COVID-19 Pandemic. Mathematics 2022, 10, 3020. https://doi.org/10.3390/math10163020
Das M, Samanta G, De la Sen M. A Fractional Order Model to Study the Effectiveness of Government Measures and Public Behaviours in COVID-19 Pandemic. Mathematics. 2022; 10(16):3020. https://doi.org/10.3390/math10163020
Chicago/Turabian StyleDas, Meghadri, Guruprasad Samanta, and Manuel De la Sen. 2022. "A Fractional Order Model to Study the Effectiveness of Government Measures and Public Behaviours in COVID-19 Pandemic" Mathematics 10, no. 16: 3020. https://doi.org/10.3390/math10163020
APA StyleDas, M., Samanta, G., & De la Sen, M. (2022). A Fractional Order Model to Study the Effectiveness of Government Measures and Public Behaviours in COVID-19 Pandemic. Mathematics, 10(16), 3020. https://doi.org/10.3390/math10163020








