Self-Similar Solutions of a Gravitating Dark Fluid
Abstract
:1. Introduction
2. The Model
- (i)
- means the EoS for ordinary non-relativistic ‘matter’ (e.g., cold dust);
- (ii)
- means ultra-relativistic ‘radiation’ (including neutrinos) and, in the very early universe, other particles that later become non-relativistic;
- (iii)
- is the simplest case and describes the expanding universe, hypothetical phantom energy would cause Big Rip;
- (iv)
- means quintessence as hypothetical fluid;
- (v)
- is responsible for the flatness of the Big Bang;
- (vi)
- A scalar field can be viewed as a sort of perfect fluid with EoS ofwhere is the time derivative of , and is the potential energy. A free scalar field has and the one with vanishing kinetic energy is equivalent to . Any EoS in between but not crossing the barrier is known as the Phantom Divide Line (PDL) [27] and is achievable,which makes scalar fields useful models for any phenomena in cosmology.
3. Analytic Solution with the Sedov–Ansatz
- The linear algebraic equation system which contains the exponents is overdetermined, and leads to a contradiction. In this case, the system has inherently no physically self-similar power-law decaying or exploding solutions. Such systems are very rare; however, damped wave equations like the telegraph equations are like this.
- All exponents have given numerical values. In that case, the analysis of the solutions is simple. The derived coupled nonlinear ODE system can be analyzed, sometimes it can be decoupled and in best cases all variables can be written down in analytic formulas.
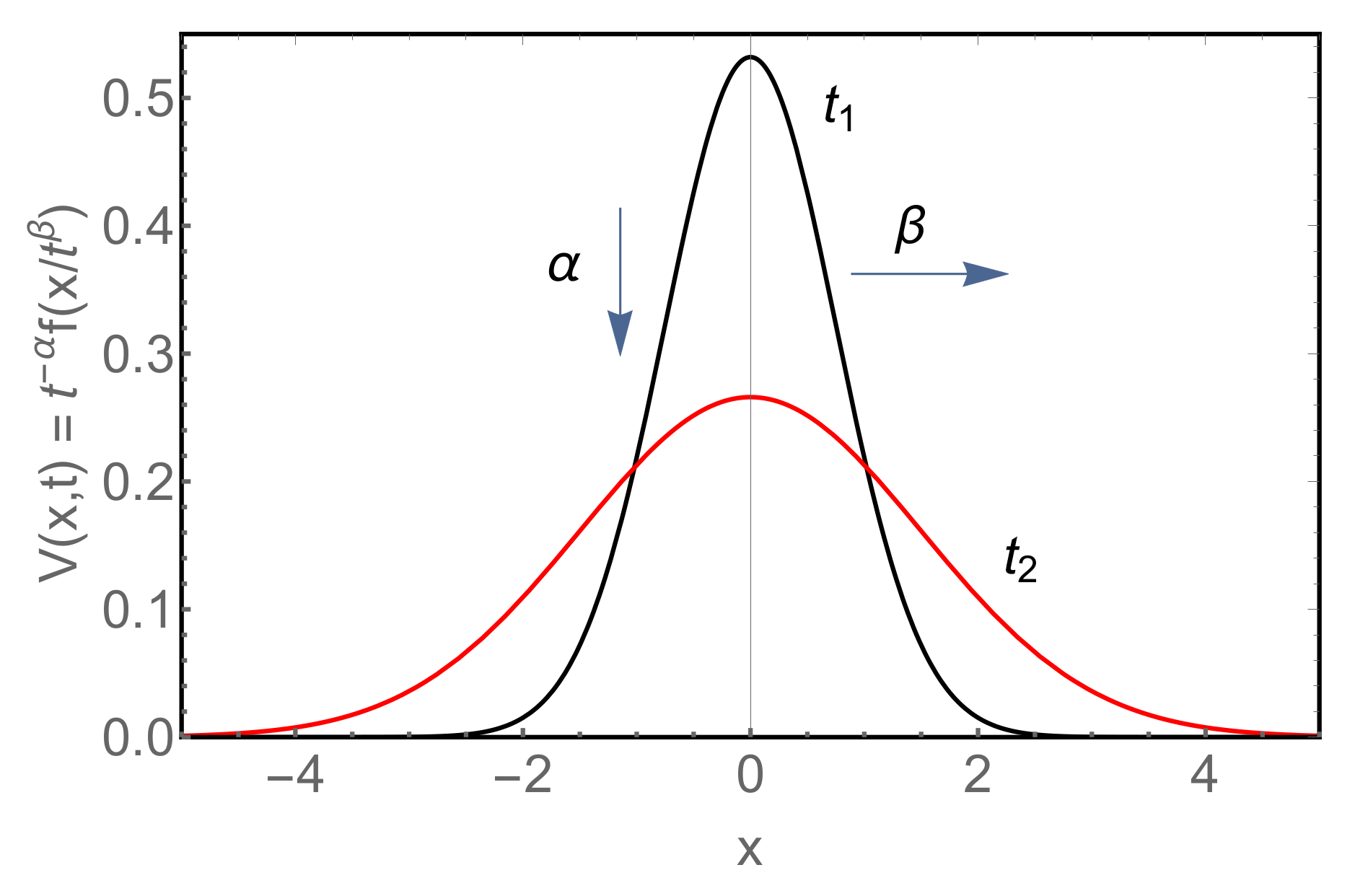
- The given linear algebraic equation system is under-determined, leaving usually one or two self-similar exponents completely free, this means extra free parameters in the obtained ODE system. Such systems have a very rich mathematical structure. The derived free exponent can have either negative or positive sign. Negative values in most cases result in power-law divergent or exploding solutions on the other side; positive exponents usually mean power-law decaying solutions, which are very desirable for dissipative systems.
4. Results
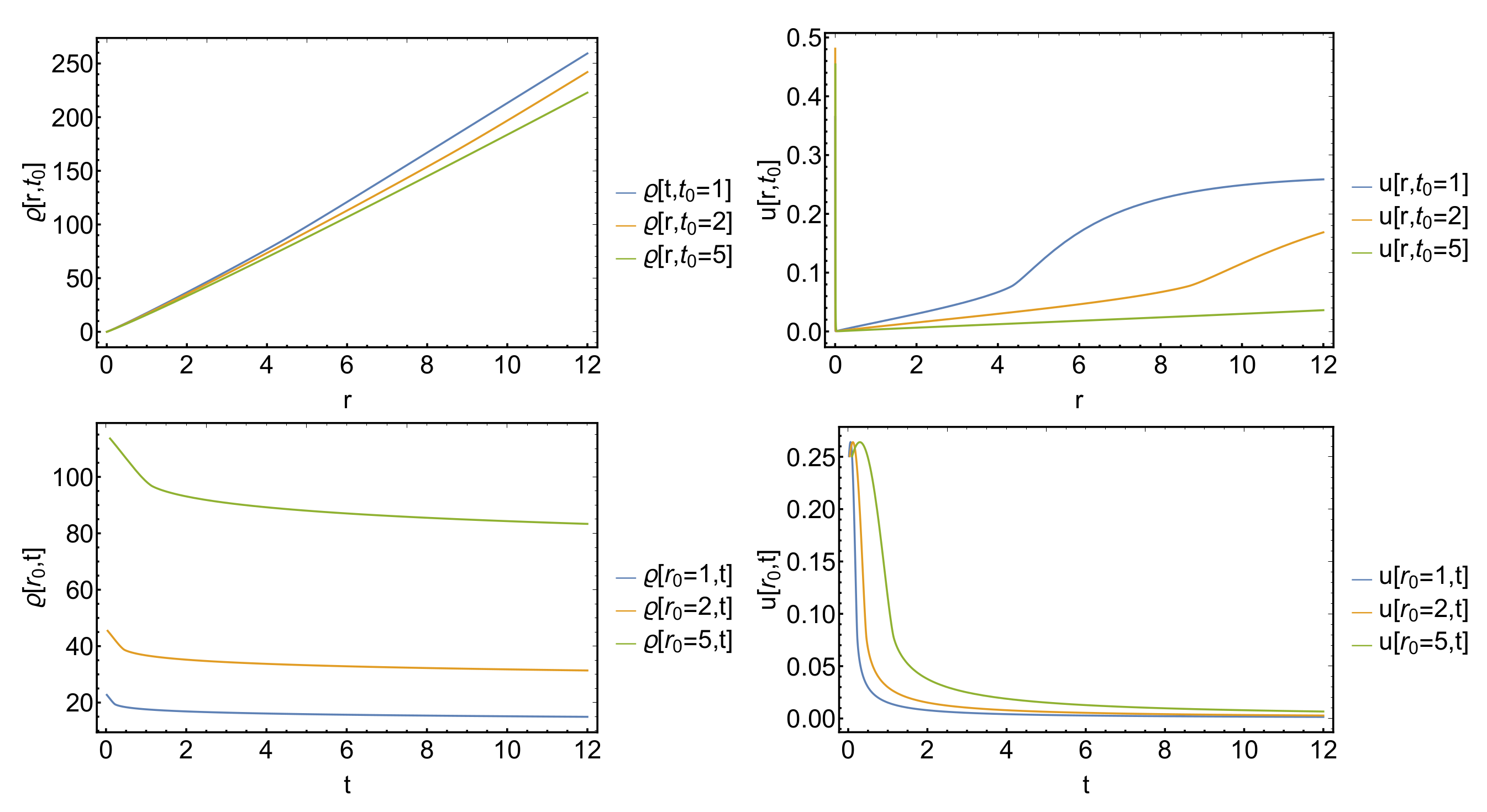
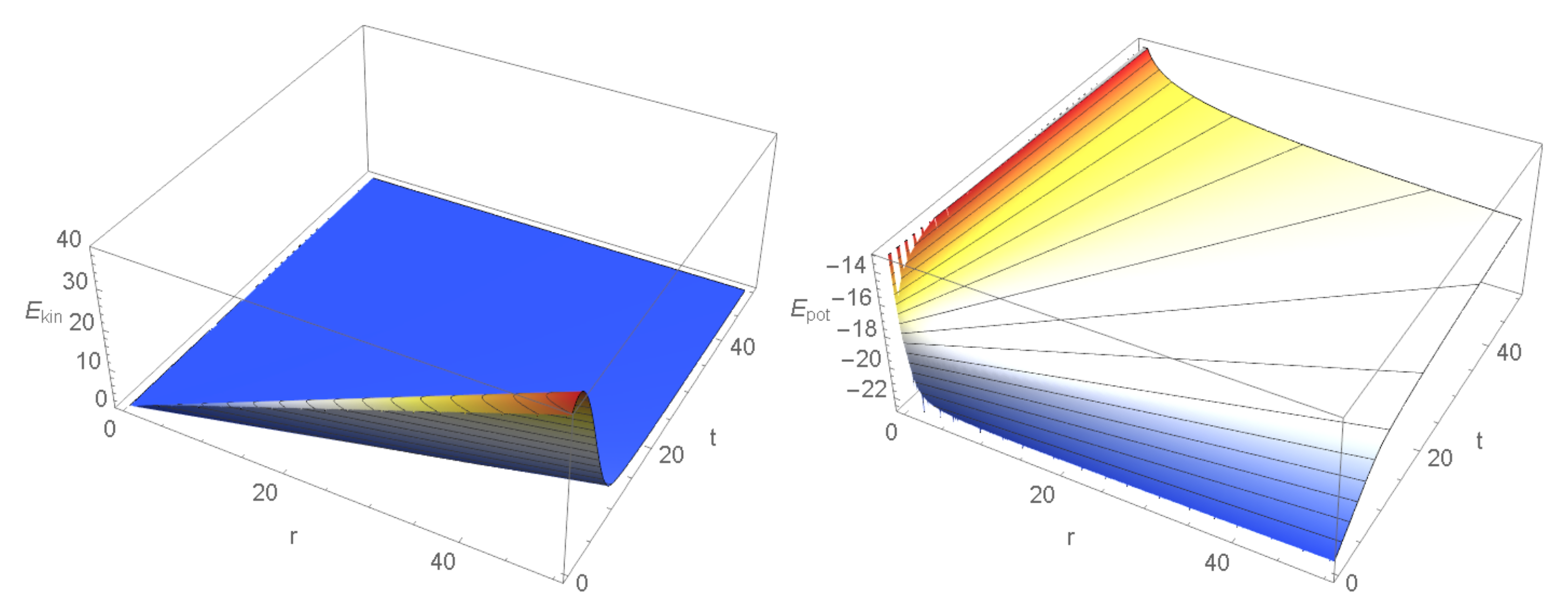
- case is seen in the first line panels with setting the kinetic-to-potential energy ratio to −50. Both physical quantities changed radically. The feature that the center of the fluid gains more and more kinetic and potential energy density is much more suppressed; on the other side, at small times, the kinetic energy density of the total fluid enhances. The larger the radius, the larger the energy density gain. Note that the values of the maximum linearly enhance with time. One may say that this phenomenon is a kind of “delayed” acceleration and deceleration of the fluid due to the enhanced potential energy density. This effect is not visible for larger initial velocities, where the initial kinetic energy densities dominate the potential energy. In this scenario, the strength of the two energy density terms lies almost in the same range. The figure of the total energy density function is drastically different to the former case, since it becomes negative everywhere. The effect of the kinetic energy density for small time values at any radius is clearly seen as a "bump" which lifts the potential energy density. The same statements are valid also for the panel of the third line panels, where the dynamics for a small positive initial velocity case, is presented.
- case describes the dynamics of a fluid with zero initial velocity (middle panels). Here, the effect of the potential energy density can be studied in a non-perturbed way. The “bump” of the kinetic energy color density for small times at an all fluid radius—a short acceleration and deceleration—of the fluid is originated by the gravitational potential of the fluid itself.
5. Discussion
6. Summary and Outlook
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Density | |
| u | Radial velocity |
| p | Pressure field |
| w | Strength of the EoS |
| Adiabatic speed of sound | |
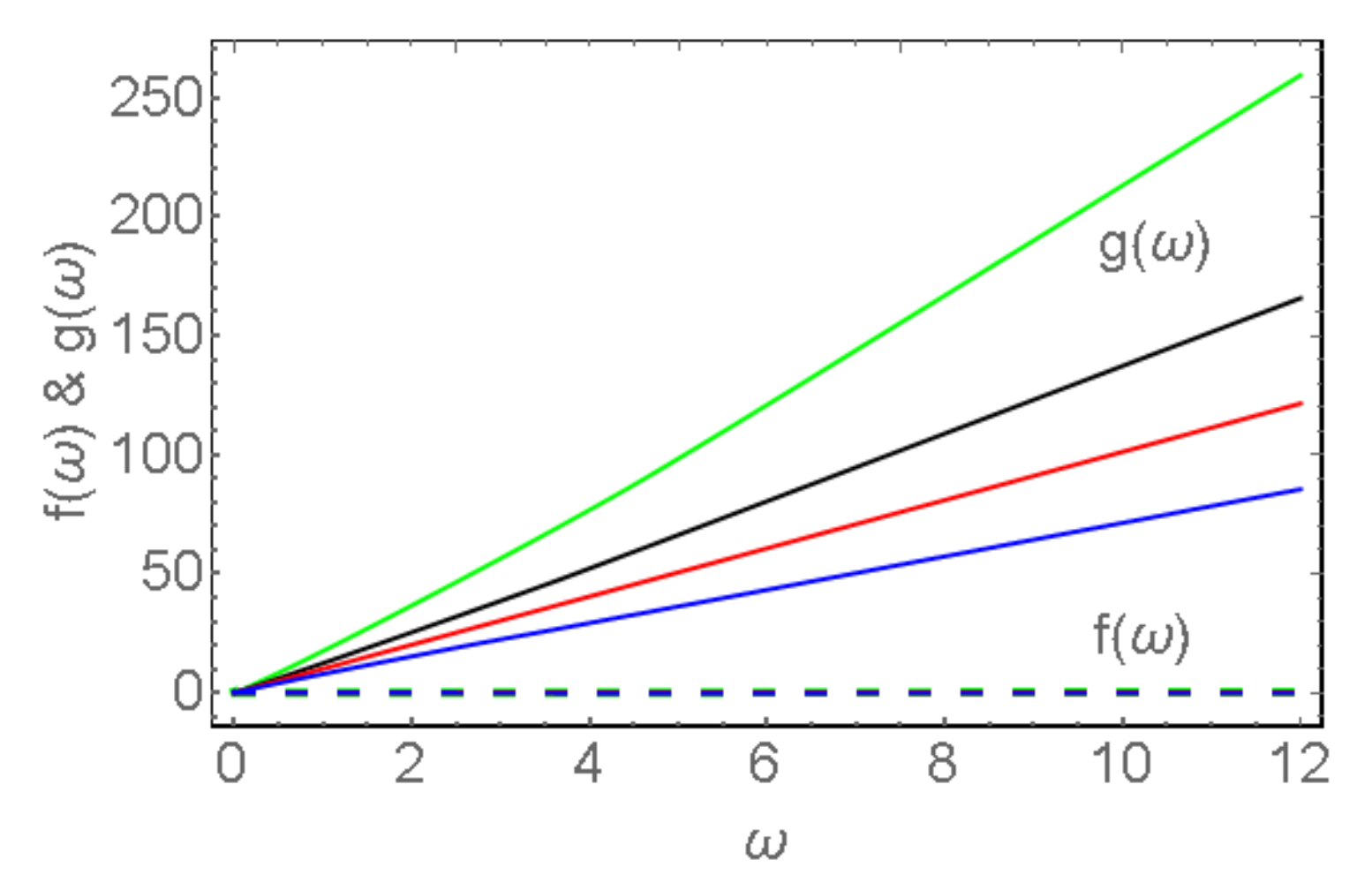
| f | Shape function of the radial velocity |
| g | Shape function of the density |
| The argument of the shape functions | |
| Kinetic energy | |
| Potential energy |
References
- Brandt, C.F.C.; Lin, L.M.; da Rocha, J.F.V.; Wang, A.Z. Gravitational collapse of spherically symmetric perfect fluid with kinematic selfsimilarity. Int. J. Mod. Phys. D 2002, 11, 155–186. [Google Scholar] [CrossRef]
- Brandt, C.F.C.; Chan, R.; da Silva, M.F.A.; da Rocha, J.F.; Villas, J.F. Dressing a Naked Singularity: An Example. Int. J. Mod. Phys. D 2010, 19, 317–328. [Google Scholar] [CrossRef]
- Heydari-Fard, M.; Sepangi, H.R. Can local bulk effects explain the galactic dark matter? J. Cosmol. Astropart. Phys. 2008, 2008, 12. [Google Scholar] [CrossRef]
- Worrakitpoonpon, T. Spherical symmetry breaking in cold gravitational collapse of isolated systems. Mon. Not. R. Astron. Soc. 2014, 446, 1335–1346. Available online: https://academic.oup.com/mnras/article-pdf/446/2/1335/9380591/stu2159.pdf (accessed on 13 July 2022). [CrossRef]
- Sedov, L.I. Similarity and Dimensional Methods in Mechanics; CRC Press: Boca Raton, FL, USA, 1993. [Google Scholar]
- Barna, I.F. Self-Similar Solutions of Three-Dimensional Navier–Stokes Equation. Commun. Theor. Phys. 2011, 56, 745. [Google Scholar] [CrossRef]
- Barna, I.F.; Mátyás, L. Analytic solutions for the three-dimensional compressible Navier–Stokes equation. Fluid Dyn. Res. 2014, 46, 055508. [Google Scholar] [CrossRef]
- Barna, I.F.; Pocsai, M.A.; Mátyás, L. Analytic Solutions of the Madelung Equation. J. Gen. Lie Theory Appl. 2017, 11, 271. [Google Scholar] [CrossRef]
- Tabensky, R.; Taub, A.H. Plane symmetric self-gravitating fluids with pressure equal to energy density. Commun. Math. Phys. 1973, 29, 61–77. [Google Scholar] [CrossRef]
- Christodoulou, D. Self-gravitating relativistic fluids: A two-phase model. Arch. Ration. Mech. Anal. 1995, 130, 343–400. [Google Scholar] [CrossRef]
- Ducomet, B.; Feireisl, E.; Petzeltová, H.; Straškraba, I. Global in time weak solutions for compressible barotropic self-gravitating fluids. Discret. Contin. Dyn. Syst. 2004, 11, 113–130. [Google Scholar] [CrossRef]
- Ahmad, S.; Jami, A.R.; Mughal, M.Z. Stability of anisotropic self-gravitating fluids. Mod. Phys. Lett. A 2018, 33, 1850095. [Google Scholar] [CrossRef]
- Amodio, P.; Giordano, D.; Iavernaro, F.; Labianca, A.; Lazzo, M.; Mazzia, F.; Pisani, L. Mathematical aspects relative to the fluid statics of a self-gravitating perfect-gas isothermal sphere. Eur. J. Mech. B Fluids 2019, 78, 62. [Google Scholar] [CrossRef]
- Yuen, M. Analytically periodic solutions to the three-dimensional Euler–Poisson equations of gaseous stars with a negative cosmological constant. Class. Quantum Gravity 2009, 26, 235011. [Google Scholar] [CrossRef]
- Gratton, J. Fundamentals of Cosmic Physics; Gordon and Breach: New York, NY, USA, 2012; Volume 15. [Google Scholar]
- Fedi, M. Physical vacuum as a dilatant fluid yields exact solutions to Pioneer anomaly and Mercury’s perihelion precession. Can. J. Phys. 2019, 97, 417–420. [Google Scholar] [CrossRef]
- Deruelle, N.; Uzan, J.P. Relativity in Modern Physics; Oxford University Press: Oxford, UK, 2018. [Google Scholar]
- Valev, D. Estimations of total mass and energy of the universe. Phys. Int. 2014, 5, 15–20. [Google Scholar] [CrossRef]
- Peacock, J.A.; Cole, S.; Norberg, P.; Baugh, C.M.; Bland-Hawthorn, J.; Bridges, T.; Cannon, R.D.; Colless, M.; Collins, C.; Couch, W.; et al. A measurement of the cosmological mass density from clustering in the 2dF Galaxy Redshift Survey. Nature 2001, 410, 169–173. [Google Scholar] [CrossRef]
- Hinshaw, G.; Weiland, J.L.; Hill, R.S.; Odegard, N.; Larson, D.; Bennett, C.L.; Dunkley, J.; Gold, B.; Greason, M.R.; Jarosik, N.; et al. Five-Year Wilkinson Microwave Anisotropy Probe Observations: Data Processing, Sky Maps, and Basic Results. Astrophys. J. Suppl. Ser. 2009, 180, 225–245. [Google Scholar] [CrossRef]
- Freese, K.; Rindler-Daller, T.; Spolyar, D.; Valluri, M. Dark stars: A review. Rep. Prog. Phys. 2016, 79, 066902. [Google Scholar] [CrossRef]
- Rácz, G.; Szapudi, I.; Csabai, I.; Dobos, L. The anisotropy of the power spectrum in periodic cosmological simulations. Mon. Not. R. Astron. Soc. 2021, 503, 5638–5645. Available online: https://academic.oup.com/mnras/article-pdf/503/4/5638/37057174/stab874.pdf (accessed on 13 July 2022). [CrossRef]
- Emden, R. Gaskugeln: Anwendungen der Mechanischen Wärmetheorie auf Kosmologische und Meteorologische Probleme; B. G. Teubner: Leipzig, Germany; Berlin, Germany, 1907. [Google Scholar]
- Horedt, G.P. Polytropes: Applications in Astrophysics and Related Fields; Kluwer Academic Publishers: Dordrecht, The Netherlands; Boston, MA, USA, 2004. [Google Scholar] [CrossRef]
- Perković, D.; Štefančić, H. Dark sector unifications: Dark matter-phantom energy, dark matter-constant w dark energy, dark matter-dark energy-dark matter. Phys. Lett. B 2019, 797, 134806. [Google Scholar] [CrossRef]
- Hogan, J. Welcome to the dark side. Nature 2007, 448, 240–245. [Google Scholar] [CrossRef] [PubMed]
- Vikman, A. Can dark energy evolve to the phantom? Phys. Rev. D 2005, 71, 023515. [Google Scholar] [CrossRef]
- Yousaf, Z.; Khlopov, M.Y.; Bhatti, M.Z.; Naseer, T. Influence of modification of gravity on the complexity factor of static spherical structures. Mon. Not. R. Astron. Soc. 2020, 495, 4334–4346. Available online: https://academic.oup.com/mnras/article-pdf/495/4/4334/33372154/staa1470.pdf (accessed on 13 July 2022). [CrossRef]
- Arbey, A. Is it possible to consider Dark Energy and Dark Matter as a same and unique Dark Fluid? arXiv 2005. [Google Scholar] [CrossRef]
- Arbey, A. Dark fluid: A complex scalar field to unify dark energy and dark matter. Phys. Rev. D 2006, 74, 043516. [Google Scholar] [CrossRef]
- Farnes, J.S. A unifying theory of dark energy and dark matter: Negative masses and matter creation within a modified framework. Astron. Astrophys. 2018, 620, A92. [Google Scholar] [CrossRef]
- Arbey, A.; Mahmoudi, F. One-loop quantum corrections to cosmological scalar field potentials. Phys. Rev. D 2007, 75, 063513. [Google Scholar] [CrossRef]
- Frieman, J.A.; Turner, M.S.; Huterer, D. Dark Energy and the Accelerating Universe. Annu. Rev. Astron. Astrophys. 2008, 46, 385–432. [Google Scholar] [CrossRef]
- Barenblatt, G.I.; Stein, N.; van Dyke, M. Similarity, Self-Similarity, and Intermediate Asymptotics; Consultants Bureau: New York, NY, USA, 1979. [Google Scholar]
- Zel’dovich, Y.B.; Raizer, Y.P.; Hayes, W.D.; Probstein, R.F.; Landshoff, R. (Eds.) Physics of Shock Waves and High-Temperature Hydrodynamic Phenomena; Academic Press: New York, NY, USA, 1967. [Google Scholar] [CrossRef]
- Barna, I.F.; Kersner, R. Heat conduction: A telegraph-type model with self-similar behavior of solutions. J. Phys. Math. Theor. 2010, 43, 375210. [Google Scholar] [CrossRef]
- Barna, I.F. Self-similar shock wave solutions of the nonlinear Maxwell equations. Laser Phys. 2014, 24, 086002. [Google Scholar] [CrossRef]
- Barna, I.F. Self-Similar Analysis of Various Navier–Stokes Equations in Two or Three Dimensions. In Handbook on Navier–Stokes Equations: Theory and Applied Analysis; Campos, D., Ed.; Physics Research and Technology, Nova Publishers: Hauppauge, NY, USA, 2017; Chapter 16; pp. 275–304. [Google Scholar]
- Barna, I.F.; László, M. Self-Similar and Traveling-Wave Analysis of the Madelung Equation. In Understanding the Schrödinger Equation: Some Non[Linear] Perspectives; Simpao, V., Little, H.C., Eds.; Mathematics Research Developments, Nova Publishers: Hauppauge, NY, USA, 2020; Chapter 6; pp. 181–224. [Google Scholar]
- Hubble, E. A relation between distance and radial velocity among extra-galactic nebulae. Proc. Natl. Acad. Sci. USA 1929, 15, 168–173. Available online: https://www.pnas.org/content/15/3/168.full.pdf (accessed on 13 July 2022). [CrossRef]
- Freedman, W.L.; Madore, B.F.; Gibson, B.K.; Ferrarese, L.; Kelson, D.D.; Sakai, S.; Mould, J.R.; Kennicutt, R.C., Jr.; Ford, H.C.; Graham, J.A.; et al. Final Results from the Hubble Space Telescope Key Project to Measure the Hubble Constant. Astrophys. J. 2001, 553, 47–72. [Google Scholar] [CrossRef]
- Jarosik, N.; Bennett, C.L.; Dunkley, J.; Gold, B.; Greason, M.R.; Halpern, M.; Hill, R.S.; Hinshaw, G.; Kogut, A.; Komatsu, E.; et al. Seven-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Sky Maps, Systematic Errors, and Basic Results. Astrophys. J. Suppl. Ser. 2011, 192, 14. [Google Scholar] [CrossRef]
- Planck Collaboration; Ade, P.A.R.; Aghanim, N.; Alves, M.I.R.; Armitage-Caplan, C.; Arnaud, M.; Ashdown, M.; Atrio-Barandela, F.; Aumont, J.; Aussel, H.; et al. Planck 2013 results. I. Overview of products and scientific results. Astron. Astrophys. 2014, 571, A1. [Google Scholar] [CrossRef]
- The LIGO Scientific Collaboration and The Virgo Collaboration; The 1M2H Collaboration; The Dark Energy Camera GW-EM Collaboration and the DES Collaboration; The DLT40 Collaboration; The Las Cumbres Observatory Collaboration; The VINROUGE Collaboration and The MASTER Collaboration. A gravitational-wave standard siren measurement of the Hubble constant. Nature 2017, 551, 85–88. [Google Scholar] [CrossRef]
- Kovács, A.; Beck, R.; Szapudi, I.; Csabai, I.; Rácz, G.; Dobos, L. A common explanation of the Hubble tension and anomalous cold spots in the CMB. Mon. Not. R. Astron. Soc. 2020, 499, 320–333. Available online: https://academic.oup.com/mnras/article-pdf/499/1/320/33852113/staa2631.pdf (accessed on 13 July 2022). [CrossRef]
- Harko, T.; Lobo, F.S.N. Two-fluid dark matter models. Phys. Rev. D 2011, 83, 124051. [Google Scholar] [CrossRef]
- Barna, I.F.; Mátyás, L. Analytic Solutions of a Two-Fluid Hydrodynamic Model. Math. Model. Anal. 2021, 26, 582–590. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barna, I.F.; Pocsai, M.A.; Barnaföldi, G.G. Self-Similar Solutions of a Gravitating Dark Fluid. Mathematics 2022, 10, 3220. https://doi.org/10.3390/math10183220
Barna IF, Pocsai MA, Barnaföldi GG. Self-Similar Solutions of a Gravitating Dark Fluid. Mathematics. 2022; 10(18):3220. https://doi.org/10.3390/math10183220
Chicago/Turabian StyleBarna, Imre Ferenc, Mihály András Pocsai, and Gergely Gábor Barnaföldi. 2022. "Self-Similar Solutions of a Gravitating Dark Fluid" Mathematics 10, no. 18: 3220. https://doi.org/10.3390/math10183220
APA StyleBarna, I. F., Pocsai, M. A., & Barnaföldi, G. G. (2022). Self-Similar Solutions of a Gravitating Dark Fluid. Mathematics, 10(18), 3220. https://doi.org/10.3390/math10183220









