Consensus Control of Leaderless and Leader-Following Coupled PDE-ODEs Modeled Multi-Agent Systems
Abstract
:1. Introduction
2. Problem Formulation
3. Consensus Control of the Leaderless PDE-ODEMAS
4. Consensus Control of the Leader-Following PDE-ODEMAS
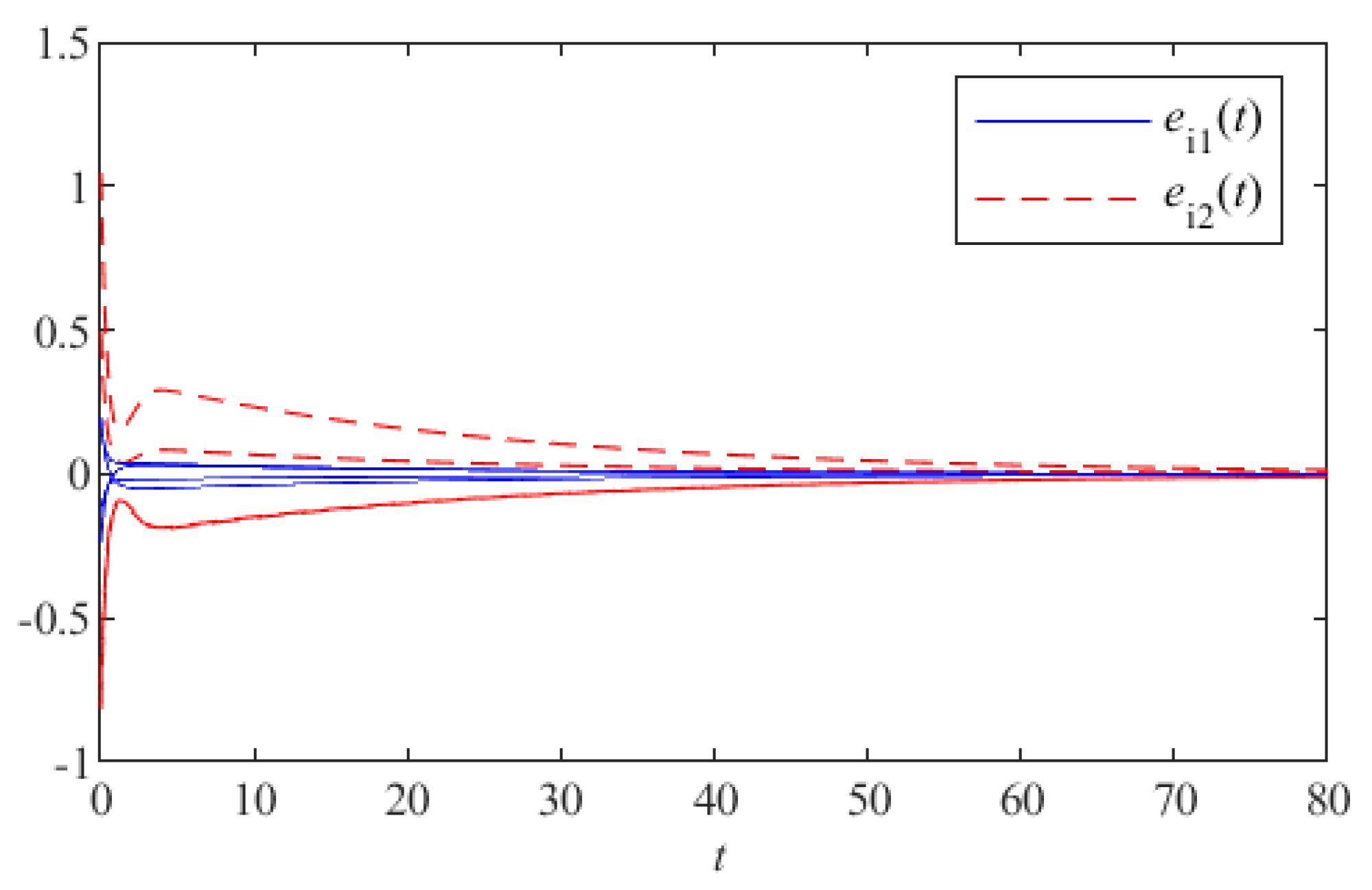
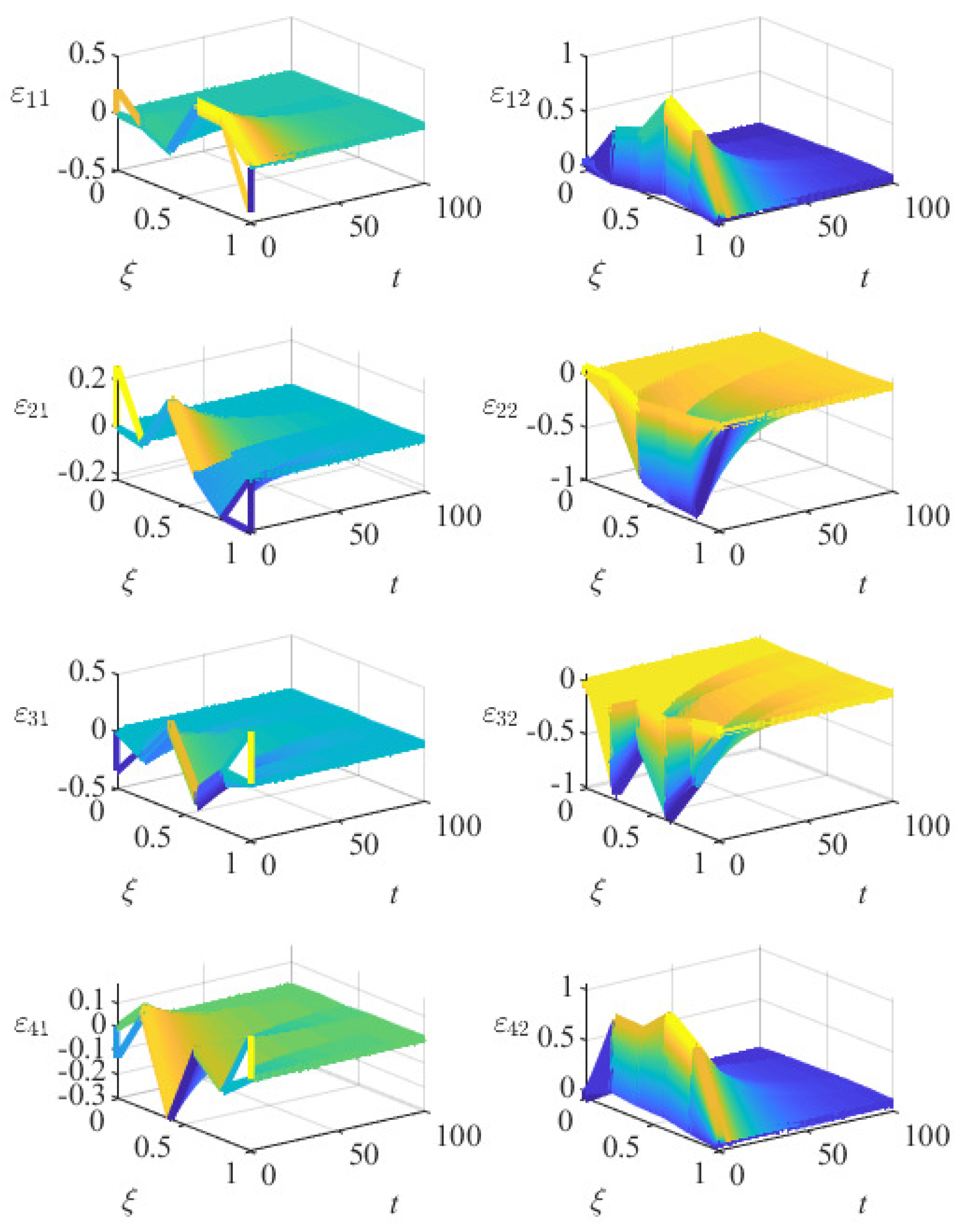
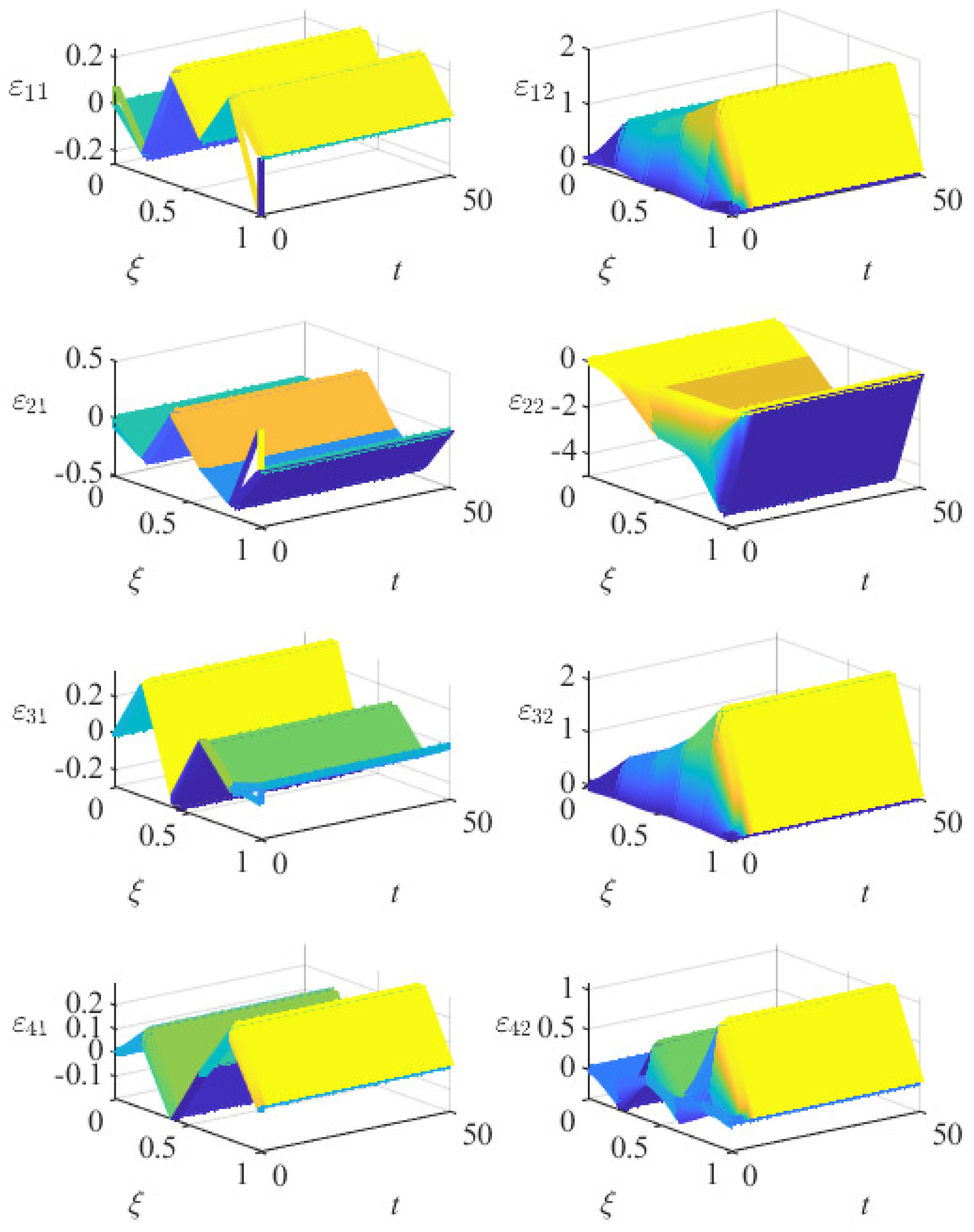
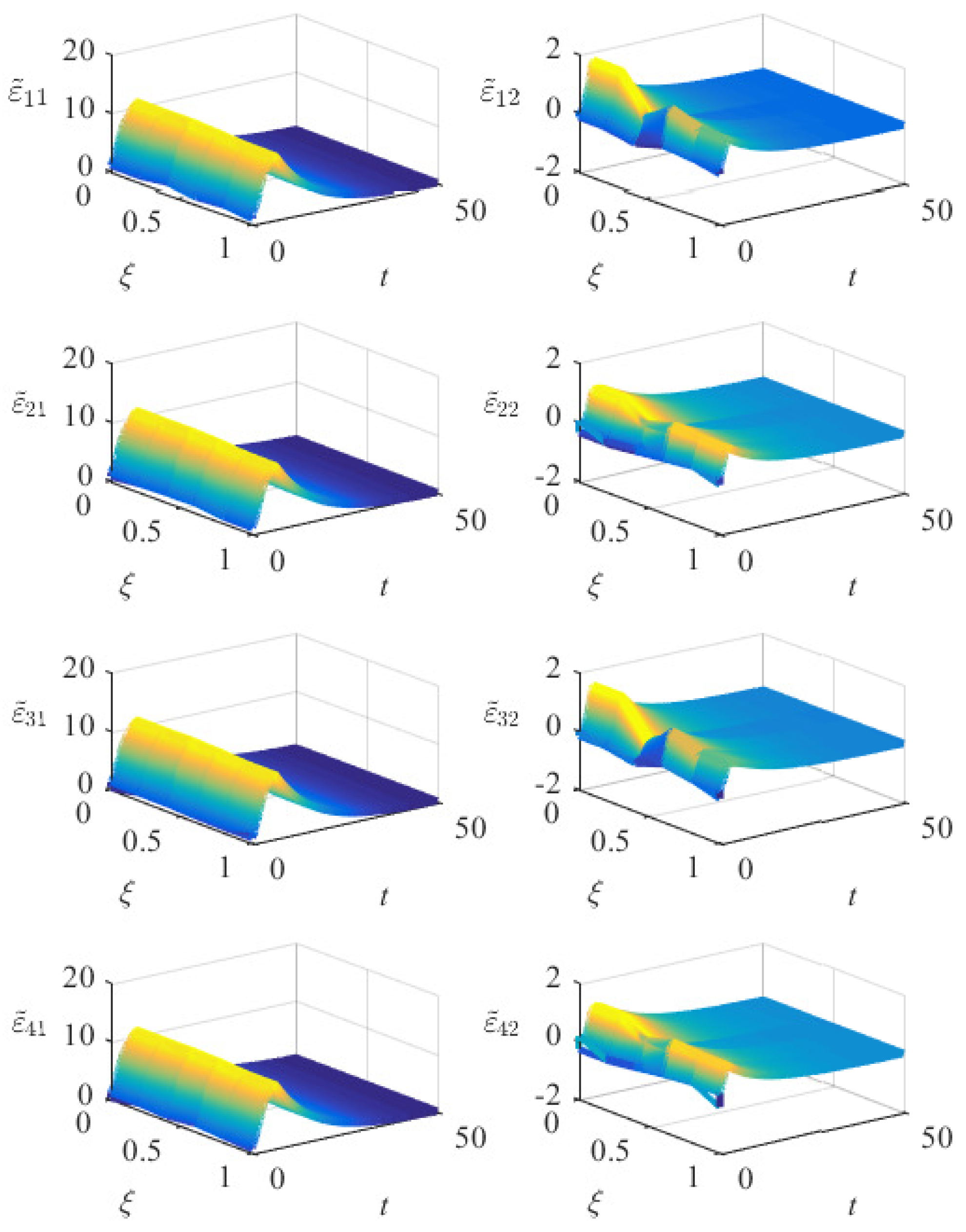
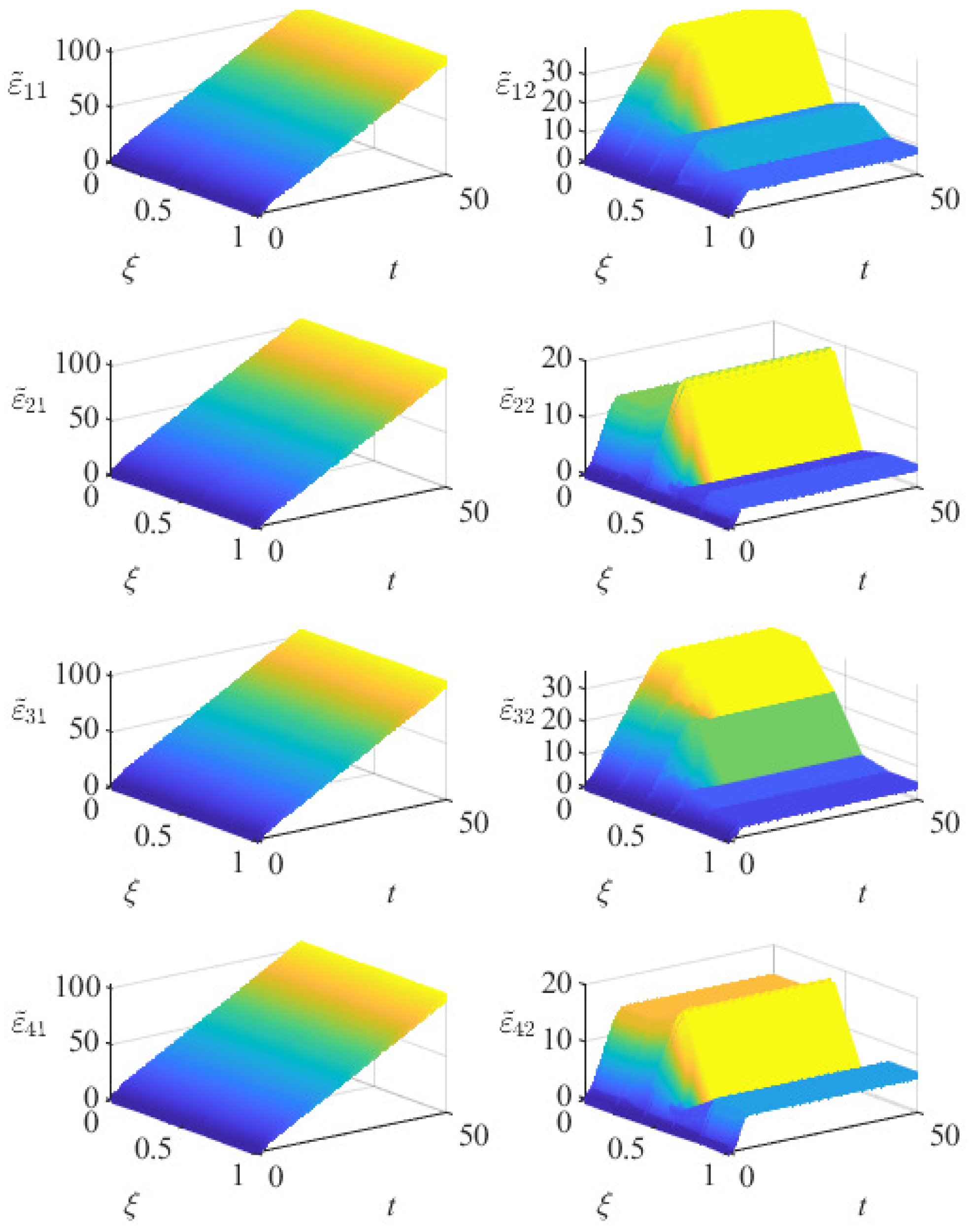
5. Numerical Simulation
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mo, L.; Yuan, X.; Yu, Y. Neuro-adaptive leaderless consensus of fractional-order multi-agent systems. Neurocomputing 2019, 339, 17–25. [Google Scholar] [CrossRef]
- Benediktsson, J.A.; Swain, P.H. Consensus theoretic classification methods. IEEE Trans. Syst. Man Cybern. 1992, 22, 688–704. [Google Scholar] [CrossRef]
- Yang, Y.; Ji, Z.; Tian, L.; Ma, H.; Qi, Q. Bipartite consensus of edge dynamics on coopetition multi-agent systems. Sci. China Inf. Sci. 2019, 62, 229201. [Google Scholar]
- Tian, B.; Lu, H.; Zuo, Z.; Yang, W. Fixed-time leader–follower output feedback consensus for second-order multiagent systems. IEEE Trans. Cybern. 2018, 49, 1545–1550. [Google Scholar] [CrossRef]
- Ning, B.; Han, Q.L. Prescribed finite-time consensus tracking for multiagent systems with nonholonomic chained-form dynamics. IEEE Trans. Autom. Control 2018, 64, 1686–1693. [Google Scholar] [CrossRef]
- Proskurnikov, A.V.; Matveev, A.S.; Cao, M. Opinion dynamics in social networks with hostile camps: Consensus vs. polarization. IEEE Trans. Autom. Control 2015, 61, 1524–1536. [Google Scholar] [CrossRef] [Green Version]
- Du, H.; Zhu, W.; Wen, G.; Duan, Z.; Lu, J. Distributed formation control of multiple quadrotor aircraft based on nonsmooth consensus algorithms. IEEE Trans. Cybern. 2017, 49, 342–353. [Google Scholar] [CrossRef]
- Cheng, Y.; Jia, R.; Du, H.; Wen, G.; Zhu, W. Robust finite-time consensus formation control for multiple nonholonomic wheeled mobile robots via output feedback. Int. J. Robust Nonlinear Control 2018, 28, 2082–2096. [Google Scholar] [CrossRef]
- Shi, S.; Xu, S.; Feng, H. Robust fixed-time consensus tracking control of high-order multiple nonholonomic systems. IEEE Trans. Syst. Man Cybern. Syst. 2019, 51, 1869–1880. [Google Scholar] [CrossRef]
- Zheng, Y.; Song, Y.; Hill, D.J.; Zhang, Y. Multiagent system based microgrid energy management via asynchronous consensus admm. IEEE Trans. Energy Convers. 2018, 33, 886–888. [Google Scholar] [CrossRef]
- Kušić, K.; Ivanjko, E.; Vrbanić, F.; Greguric, M.; Dusparic, I. Spatial-Temporal Traffic Flow Control on Motorways Using Distributed Multi-Agent Reinforcement Learning. Mathematics 2021, 9, 3081. [Google Scholar] [CrossRef]
- Nowzari, C.; Garcia, E.; Cortés, J. Event-triggered communication and control of networked systems for multi-agent consensus. Automatica 2019, 105, 1–27. [Google Scholar] [CrossRef] [Green Version]
- Zong, X.; Li, T.; Zhang, J.F. Consensus conditions of continuous-time multi-agent systems with time-delays and measurement noises. Automatica 2019, 99, 412–419. [Google Scholar] [CrossRef] [Green Version]
- Tan, X.; Cao, J.; Rutkowski, L.; Lu, G. Distributed dynamic self-triggered impulsive control for consensus networks: The case of impulse gain with normal distribution. IEEE Trans. Cybern. 2021, 51, 624–634. [Google Scholar] [CrossRef] [PubMed]
- Liu, M.; Dong, S.; Chen, G.; Liu, M.; Wu, Z. Cooperative neural-adaptive fault-tolerant output regulation for heterogeneous nonlinear uncertain multiagent systems with disturbance. Sci. China Inf. Sci. 2021, 64, 172212. [Google Scholar]
- Li, X.; Tang, Y.; Karimi, H.R. Consensus of multi-agent systems via fully distributed event-triggered control. Automatica 2020, 116, 108898. [Google Scholar] [CrossRef]
- Li, X.; Yu, Z.; Li, Z.; Wu, N. Group consensus via pinning control for a class of heterogeneous multi-agent systems with input constraints. Inf. Sci. 2021, 542, 247–262. [Google Scholar] [CrossRef]
- De la Sen, M.; Alonso-Quesada, S. On finite-time consensus objectives in time-varying interconnected discrete linear dynamic systems under internal and external delays. Adv. Mech. Eng. 2018, 10, 1687814018784840. [Google Scholar] [CrossRef]
- Olfati-Saber, R.; Fax, J.A.; Murray, R.M. Consensus and cooperation in networked multi-agent systems. Proc. IEEE 2007, 95, 215–233. [Google Scholar] [CrossRef] [Green Version]
- Aman, B.; Ciobanu, G. Knowledge dynamics and behavioural equivalences in multi-agent systems. Mathematics 2021, 9, 2869. [Google Scholar] [CrossRef]
- Wang, J.W.; Wang, J.M. Mixed H2/H∞ sampled-data output feedback control design for a semi-linear parabolic PDE in the sense of spatial L∞ norm. Automatica 2019, 103, 282–293. [Google Scholar] [CrossRef]
- Chen, P.; Villa, U.; Ghattas, O. Taylor approximation and variance reduction for pde-constrained optimal control under uncertainty. J. Comput. Phys. 2019, 385, 163–186. [Google Scholar] [CrossRef] [Green Version]
- Chen, S.; Vazquez, R.; Krstic, M. Folding backstepping approach to parabolic PDE bilateral boundary control. IFAC-PapersOnLine 2019, 52, 76–81. [Google Scholar] [CrossRef]
- Buyukkocak, A.T.; Aksaray, D.; Yazcoglu, Y. Planning of heterogeneous multi-agent systems under signal temporal logic specifications with integral predicates. IEEE Robot. Autom. Lett. 2021, 6, 1375–1382. [Google Scholar] [CrossRef]
- Wang, G.; Huang, Y.; Wang, X. Consensus of semi-markov multi-agent systems with stochastically unmatched topologies. IET Control Theory Appl. 2021, 15, 1003–1017. [Google Scholar] [CrossRef]
- d’Andréa-Novel, B.; Coron, J.M. Exponential stabilization of an overhead crane with flexible cable via a back-stepping approach. Automatica 2000, 36, 587–593. [Google Scholar] [CrossRef]
- Hiruko, K.; Okabe, S. Controllability of hybrid PDE-ODE systems with structural instability and applications to mathematical models on intermittent hormonal therapy for prostate cancer. Math. Methods Appl. Sci. 2018, 41, 8229–8247. [Google Scholar] [CrossRef]
- Lattanzio, C.; Maurizi, A.; Piccoli, B. Moving bottlenecks in car traffic flow: A PDE-ODE coupled model. SIAM J. Math. Anal. 2011, 43, 50–67. [Google Scholar] [CrossRef]
- Wang, J.W.; Wu, H.N. Exponentially stabilizing fuzzy controller design for a nonlinear ODE-beam cascaded system and its application to flexible air-breathing hypersonic vehicle. Fuzzy Sets Syst. 2020, 385, 127–147. [Google Scholar] [CrossRef]
- Meglio, F.D.; Argomedo, F.B.; Hu, L.; Krstic, M. Stabilization of coupled linear heterodirectional hyperbolic PDE-ODE systems. Automatica 2018, 87, 281–289. [Google Scholar]
- Zhao, Y.; Gao, H.; Qiu, J. Fuzzy observer based control for nonlinear coupled hyperbolic PDE-ODE systems. IEEE Trans. Fuzzy Syst. 2019, 27, 1332–1346. [Google Scholar] [CrossRef]
- He, P. Consensus of uncertain parabolic PDE agents via adaptive unit-vector control scheme. IET Control Theory Appl. 2018, 12, 2488–2494. [Google Scholar] [CrossRef]
- Fu, Q.; Du, L.; Xu, G.; Wu, J.; Yu, P. Consensus control for multi-agent systems with distributed parameter models. Neurocomputing 2018, 25, 58–64. [Google Scholar] [CrossRef]
- Dai, X.; Wang, C.; Tian, S.; Huang, Q. Consensus control via iterative learning for distributed parameter models multi-agent systems with time-delay. J. Frankl. Inst. 2019, 356, 5240–5259. [Google Scholar] [CrossRef]
- Fu, Q.; Du, L.; Xu, G.; Wu, J. Consensus control for multi-agent systems with distributed parameter models via iterative learning algorithm. J. Frankl. Inst. 2018, 355, 4453–4472. [Google Scholar] [CrossRef]
- Lan, Y.-H.; Wu, B.; Shiy, Y.-X.; Luo, Y.-P. Iterative learning based consensus control for distributed parameter multi-agent systems with time-delay. Neurocomputing 2019, 357, 77–85. [Google Scholar] [CrossRef]
- Qi, J.; Wang, S.; Fang, J.-A.; Diagne, M. Control of multi-agent systems with input delay via PDE-based method. Automatica 2019, 106, 91–100. [Google Scholar] [CrossRef]
- Yang, C.; He, H.; Huang, T.; Zhang, A.; Qiu, J.; Cao, J.; Li, X. Consensus for non-linear multi-agent systems modelled by PDEs based on spatial boundary communication. IET Control Theory Appl. 2017, 11, 3196–3200. [Google Scholar] [CrossRef]
- Yang, C.; Huang, T.; Zhang, A.; Qiu, J.; Cao, J.; Alsaadi, F.E. Output consensus of multiagent systems based on PDEs with input constraint: A boundary control approach. IEEE Trans. Syst. Man Cybern. Syst. 2021, 51, 370–377. [Google Scholar] [CrossRef]
- Seuret, A.; Gouaisbaut, F. Jensen’s and Wirtinger’s inequalities for time-delay systems. IFAC Proc. Vol. 2013, 46, 343–348. [Google Scholar] [CrossRef] [Green Version]
- Pilloni, A.; Pisano, A.; Orlov, Y.; Usai, E. Consensus-based control for a network of diffusion PDEs with boundary local interaction. IEEE Trans. Autom. Control 2015, 61, 2708–2713. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.W.; Krstic, M. Output-feedback boundary control of a heat PDE sandwiched between two ODEs. IEEE TRansactions Autom. Control 2019, 64, 4653–4660. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ni, X.; Yi, K.; Jiang, Y.; Zhang, A.; Yang, C. Consensus Control of Leaderless and Leader-Following Coupled PDE-ODEs Modeled Multi-Agent Systems. Mathematics 2022, 10, 201. https://doi.org/10.3390/math10020201
Ni X, Yi K, Jiang Y, Zhang A, Yang C. Consensus Control of Leaderless and Leader-Following Coupled PDE-ODEs Modeled Multi-Agent Systems. Mathematics. 2022; 10(2):201. https://doi.org/10.3390/math10020201
Chicago/Turabian StyleNi, Xu, Kejia Yi, Yiming Jiang, Ancai Zhang, and Chengdong Yang. 2022. "Consensus Control of Leaderless and Leader-Following Coupled PDE-ODEs Modeled Multi-Agent Systems" Mathematics 10, no. 2: 201. https://doi.org/10.3390/math10020201
APA StyleNi, X., Yi, K., Jiang, Y., Zhang, A., & Yang, C. (2022). Consensus Control of Leaderless and Leader-Following Coupled PDE-ODEs Modeled Multi-Agent Systems. Mathematics, 10(2), 201. https://doi.org/10.3390/math10020201





