A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow
Abstract
:1. Introduction
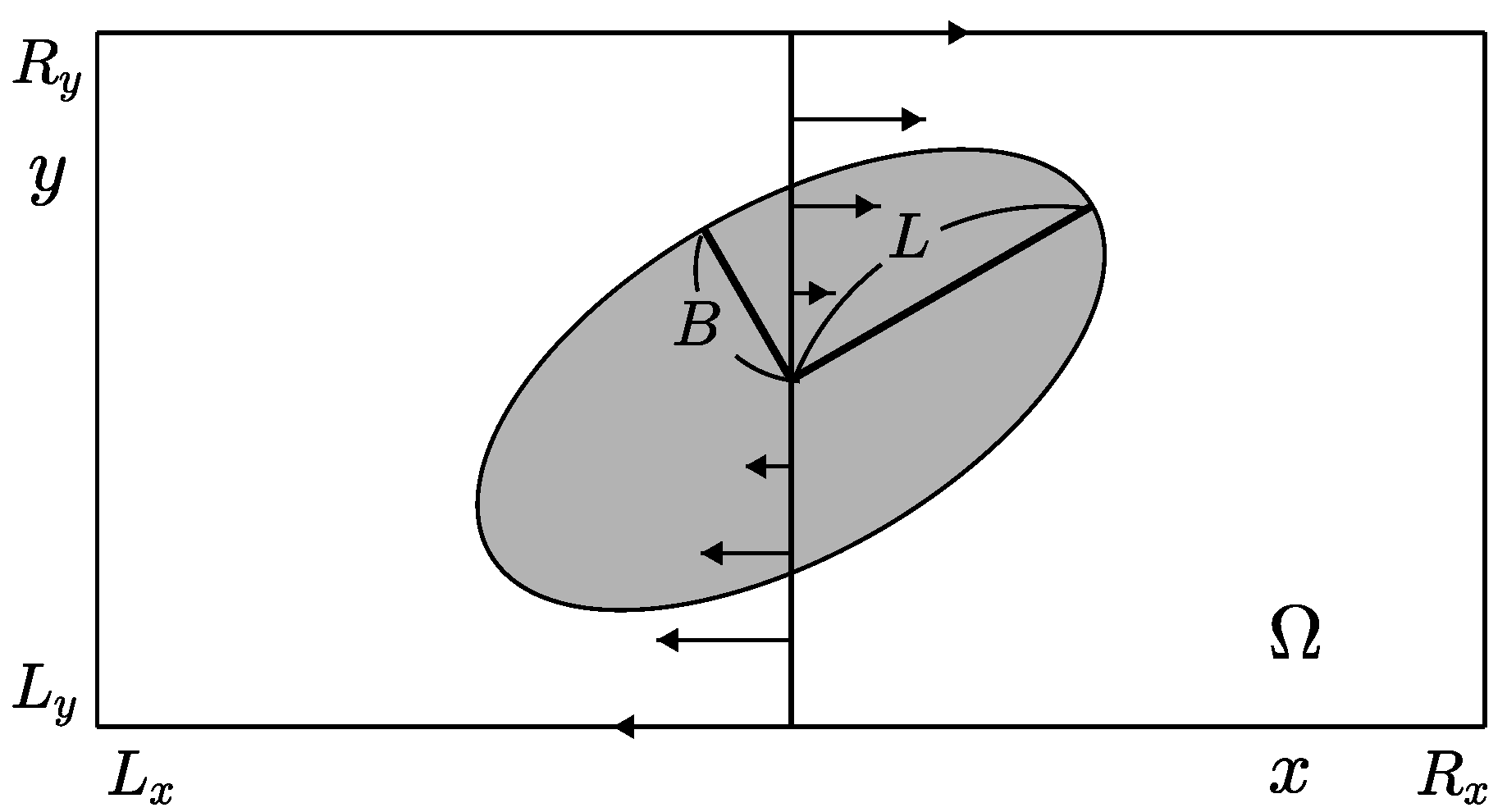
2. Governing Equations and Numerical Solution
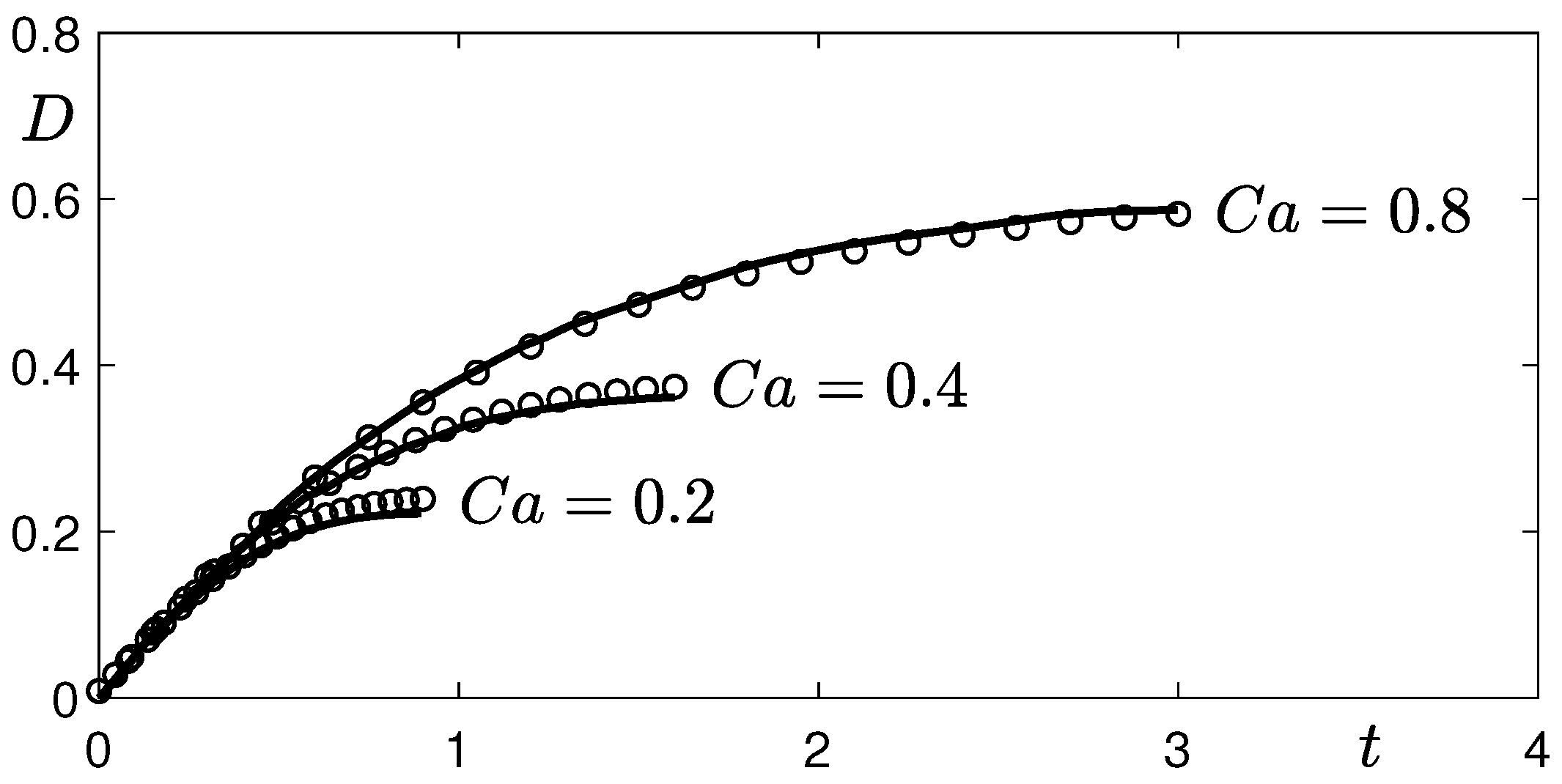
3. Numerical Experiments
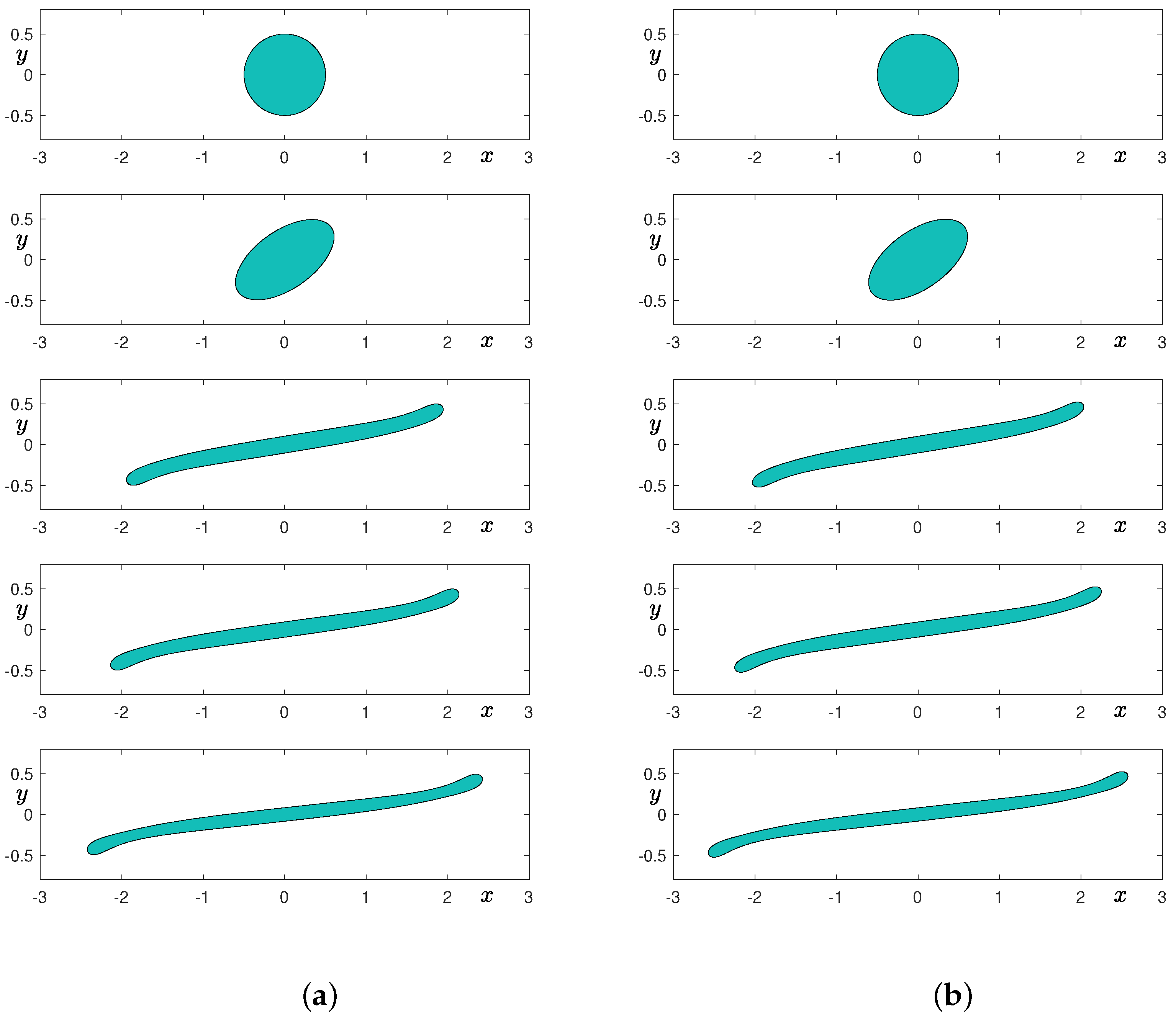
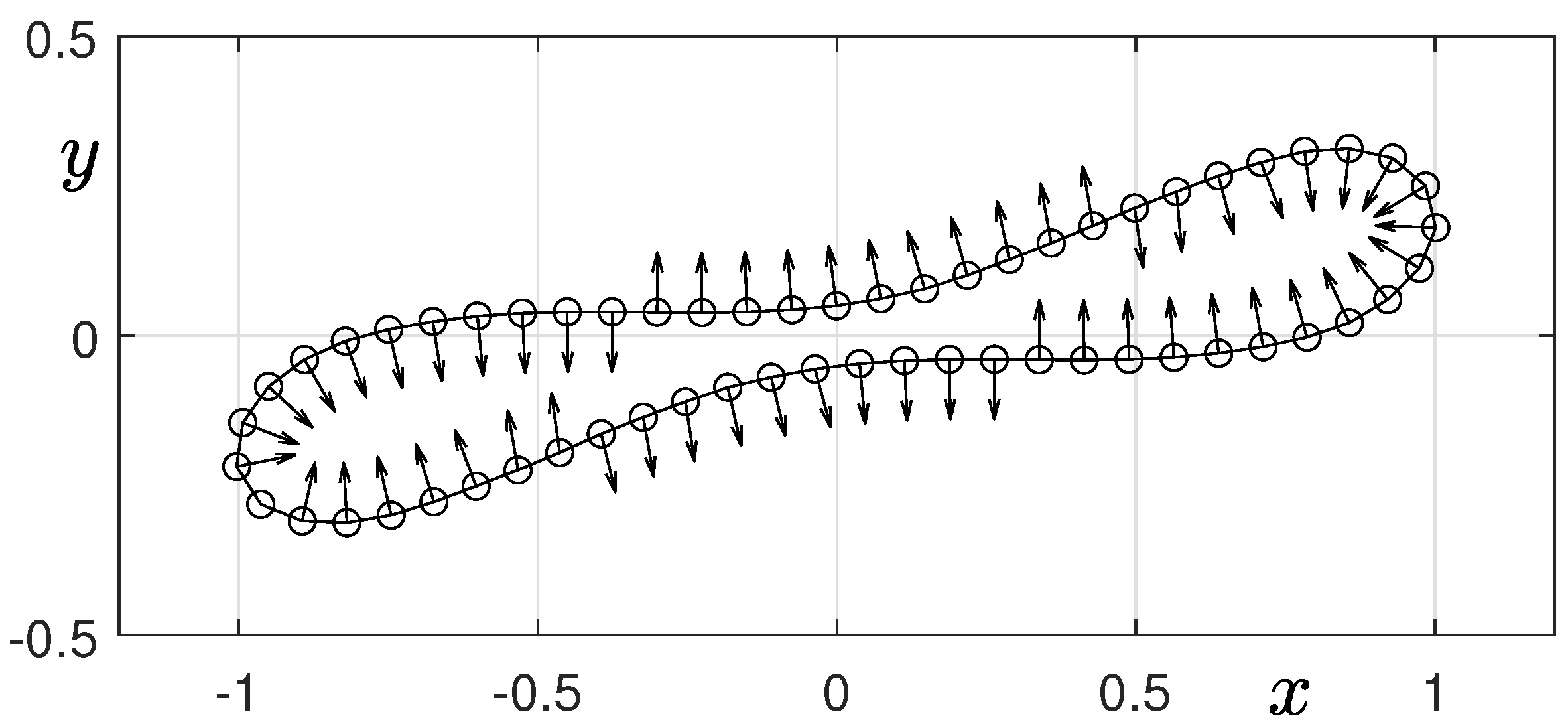
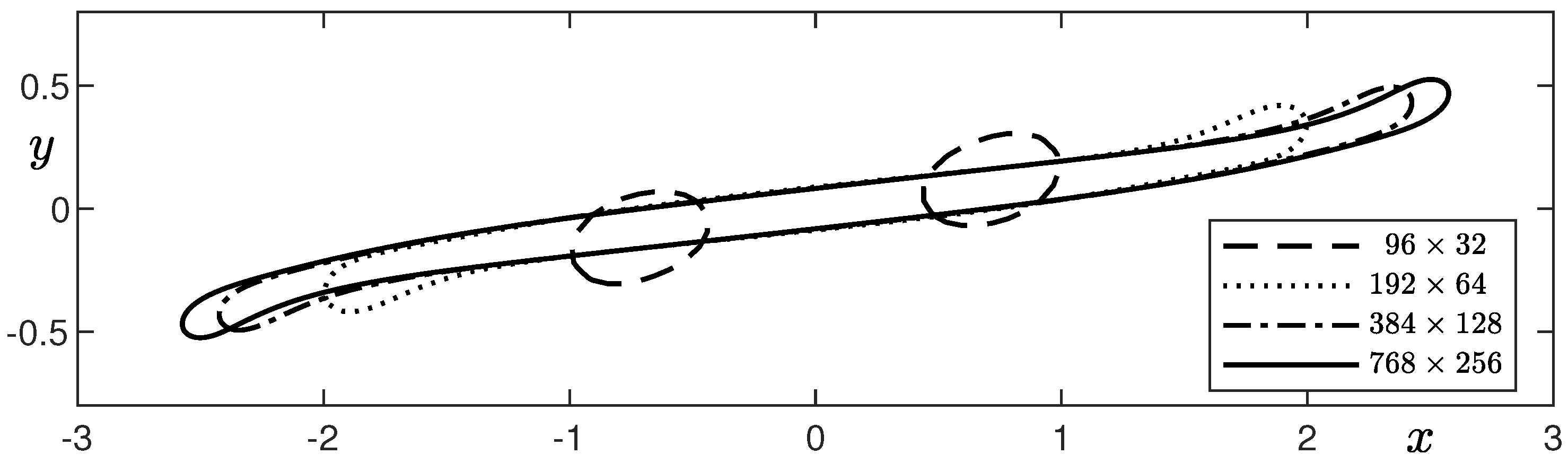
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Han, F. Cellular automata modeling of Ostwald ripening and Rayleigh instability. Materials 2018, 11, 1936. [Google Scholar] [CrossRef] [Green Version]
- Hao, X.; Zeng, Y. Simulation of Breakup Process of Polymer Jet during Melt Blowing. Fibers Polym. 2020, 21, 1222–1228. [Google Scholar] [CrossRef]
- Xie, L.; Yang, L.; Ye, H. Instability of gas-surrounded Rayleigh viscous jets: Weakly nonlinear analysis and numerical simulation. Phys. Fluids 2017, 29, 074101. [Google Scholar] [CrossRef]
- Amani, A.; Balcázar, N.; Castro, J.; Oliva, A. Numerical study of droplet deformation in shear flow using a conservative level-set method. Chem. Eng. Sci. 2019, 207, 153–171. [Google Scholar] [CrossRef]
- Komrakova, A.E.; Shardt, O.; Eskin, D.; Derksen, J.J. Lattice Boltzmann simulations of drop deformation and breakup in shear flow. Int. J. Multiph. Flow 2014, 59, 24–43. [Google Scholar] [CrossRef]
- Komrakova, A.E.; Shardt, O.; Eskin, D.; Derksen, J.J. Effects of dispersed phase viscosity on drop deformation and breakup in inertial shear flow. Chem. Eng. Sci. 2015, 126, 150–159. [Google Scholar] [CrossRef]
- Wang, N.; Liu, H.; Zhang, C. Deformation and breakup of a confined droplet in shear flows with power-law rheology. J. Rheol. 2017, 61, 741–758. [Google Scholar] [CrossRef]
- Liu, H.; Lu, Y.; Li, S.; Yu, Y.; Sahu, K.C. Deformation and breakup of a compound droplet in three-dimensional oscillatory shear flow. Int. J. Multiph. Flow 2021, 134, 103472. [Google Scholar] [CrossRef]
- Wang, Y.; Ye, Y. Energy conservation for weak solutions to the 3D Navier–Stokes–Cahn–Hilliard system. Appl. Math. Lett. 2022, 123, 107587. [Google Scholar] [CrossRef]
- Farokhirad, S.; Lee, T.; Morris, J.F. Effects of inertia and viscosity on single droplet deformation in confined shear flow. Commun. Comput. Phys. 2013, 13, 706–724. [Google Scholar] [CrossRef]
- Zhang, J.; Shu, S.; Guan, X.; Yang, N. Regime mapping of multiple breakup of droplets in shear flow by phase-field lattice Boltzmann simulation. Chem. Eng. Sci. 2021, 240, 116673. [Google Scholar] [CrossRef]
- Barai, N.; Mandal, N. Breakup modes of fluid drops in confined shear flows. Phys. Fluids 2016, 28, 073302. [Google Scholar] [CrossRef]
- Nazari, M.; Sani, H.M.; Kayhani, M.H.; Daghighi, Y. Different stages of liquid film growth in a microchannel: Two-phase Lattice Boltzmann study. Braz. J. Chem. Eng. 2018, 35, 977–994. [Google Scholar] [CrossRef] [Green Version]
- Adam, N.; Franke, F.; Aland, S. A Simple Parallel Solution Method for the Navier–Stokes Cahn–Hilliard Equations. Mathematics 2020, 8, 1224. [Google Scholar] [CrossRef]
- Bai, F.; Han, D.; He, X.M.; Yang, X. Deformation and coalescence of ferrodroplets in Rosensweig model using the phase field and modified level set approaches under uniform magnetic fields. Commun. Nonlinear Sci. Numer. Simul. 2020, 85, 105213. [Google Scholar] [CrossRef]
- Chen, Y.; Huang, Y.; Yi, N. Error analysis of a decoupled, linear and stable finite element method for Cahn–Hilliard–Navier–Stokes equations. Appl. Math. Comput. 2022, 421, 126928. [Google Scholar] [CrossRef]
- Chen, L.; Zhao, J. A novel second-order linear scheme for the Cahn–Hilliard–Navier–Stokes equations. J. Comput. Phys. 2020, 423, 109782. [Google Scholar] [CrossRef]
- Guo, Z.; Cheng, Q.; Lin, P.; Liu, C.; Lowengrub, J. Second order approximation for a quasi-incompressible Navier–Stokes Cahn–Hilliard system of two-phase flows with variable density. J. Comput. Phys. 2022, 448, 110727. [Google Scholar] [CrossRef]
- Dehghan, M.; Gharibi, Z. Numerical analysis of fully discrete energy stable weak Galerkin finite element Scheme for a coupled Cahn–Hilliard–Navier–Stokes phase-field model. Appl. Math. Comput. 2021, 410, 126487. [Google Scholar] [CrossRef]
- Li, M.; Xu, C. New efficient time-stepping schemes for the Navier–Stokes–Cahn–Hilliard equations. Comput. Fluids 2021, 231, 105174. [Google Scholar] [CrossRef]
- Liu, C.; Frank, F.; Thiele, C.; Alpak, F.O.; Berg, S.; Chapman, W.; Riviere, B. An efficient numerical algorithm for solving viscosity contrast Cahn–Hilliard–Navier–Stokes system in porous media. J. Comput. Phys. 2020, 400, 108948. [Google Scholar] [CrossRef]
- Sohaib, M.; Shah, A. Fully decoupled pressure projection scheme for the numerical solution of diffuse interface model of two-phase flow. Commun. Nonlinear Sci. Numer. Simul. 2022, 112, 106547. [Google Scholar] [CrossRef]
- Choi, J.W.; Lee, H.G.; Jeong, D.; Kim, J.S. An unconditionally gradient stable numerical method for solving the Allen–Cahn equation. Phys. A 2009, 388, 1791–1803. [Google Scholar] [CrossRef]
- Brackbill, J.U.; Kothe, D.B.; Zemach, C. A continuum method for modeling surface tension. J. Comput. Phys. 1992, 100, 335–354. [Google Scholar] [CrossRef]
- Kim, J.S. A continuous surface tension force formulation for diffuse-interface models. J. Comput. Phys. 2005, 204, 784–804. [Google Scholar] [CrossRef]
- Kim, J.S. Phase-Field Models for Multi-Component Fluid Flows. Comm. Comput. Phys. 2012, 12, 613–661. [Google Scholar] [CrossRef]
- Chorin, A.J. Numerical Solution of the Navier–Stokes Equations. Math. Comput. 1968, 22, 745–762. [Google Scholar] [CrossRef]
- Trottenberg, U.; Oosterlee, C.W.; Schüller, A. Multigrid; Academic Press: New York, NY, USA, 2001. [Google Scholar]
- Lee, C.; Jeong, D.; Yang, J.; Kim, J. Nonlinear multigrid implementation for the two-dimensional Cahn–Hilliard equation. Mathematics 2020, 8, 97. [Google Scholar] [CrossRef] [Green Version]
- Yoon, S.; Jeong, D.; Lee, C.; Kim, H.; Kim, S.; Lee, H.G.; Kim, J.S. Fourier-spectral method for the phase-field equations. Mathematics 2020, 8, 1385. [Google Scholar] [CrossRef]
- Chen, Y.; Liang, Y.; Chen, M. The deformation and breakup of a droplet under the combined influence of electric field and shear flow. Fluid Dyn. Res. 2021, 53, 065504. [Google Scholar] [CrossRef]
- Sheth, K.S.; Pozrikidis, C. Effects of inertia on the deformation of liquid drops in simple shear flow. Comput. Fluids 1995, 24, 101–119. [Google Scholar] [CrossRef]
- Li, Y.; Wang, J. Unconditional convergence analysis of stabilized FEM-SAV method for Cahn–Hilliard equation. Appl. Math. Comput. 2022, 419, 126880. [Google Scholar] [CrossRef]
- Lee, D.; Kim, J.S. Comparison study of the conservative Allen–Cahn and the Cahn–Hilliard equations. Math. Comput. Simul. 2016, 119, 35–56. [Google Scholar] [CrossRef]
- Cui, C.; Liu, J.; Mo, Y.; Zhai, S. An effective operator splitting scheme for two-dimensional conservative nonlocal Allen–Cahn equation. Appl. Math. Lett. 2022, 130, 108016. [Google Scholar] [CrossRef]
- Barosan, I.; Anderson, P.D.; Meijer, H.E.H. Application of mortar elements to diffuse-interface methods. Comput. Fluids 2006, 35, 1384–1399. [Google Scholar] [CrossRef]
- Liu, P.; Ouyang, Z.; Chen, C.; Yang, X. A novel fully-decoupled, linear, and unconditionally energy-stable scheme of the conserved Allen–Cahn phase-field model of a two-phase incompressible flow system with variable density and viscosity. Commun. Nonlinear Sci. Numer. Simul. 2022, 107, 106120. [Google Scholar] [CrossRef]
- Kwak, S.; Yang, J.; Kim, J.S. A conservative Allen–Cahn equation with a curvature-dependent Lagrange multiplier. Appl. Math. Lett. 2022, 126, 107838. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Li, Y.; Kim, J. A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow. Mathematics 2022, 10, 4092. https://doi.org/10.3390/math10214092
Yang J, Li Y, Kim J. A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow. Mathematics. 2022; 10(21):4092. https://doi.org/10.3390/math10214092
Chicago/Turabian StyleYang, Junxiang, Yibao Li, and Junseok Kim. 2022. "A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow" Mathematics 10, no. 21: 4092. https://doi.org/10.3390/math10214092
APA StyleYang, J., Li, Y., & Kim, J. (2022). A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow. Mathematics, 10(21), 4092. https://doi.org/10.3390/math10214092






