Design and Motion Characteristics of Active–Passive Composite Suspension Actuator
Abstract
1. Introduction
2. Numerical Simulation of Flow Field
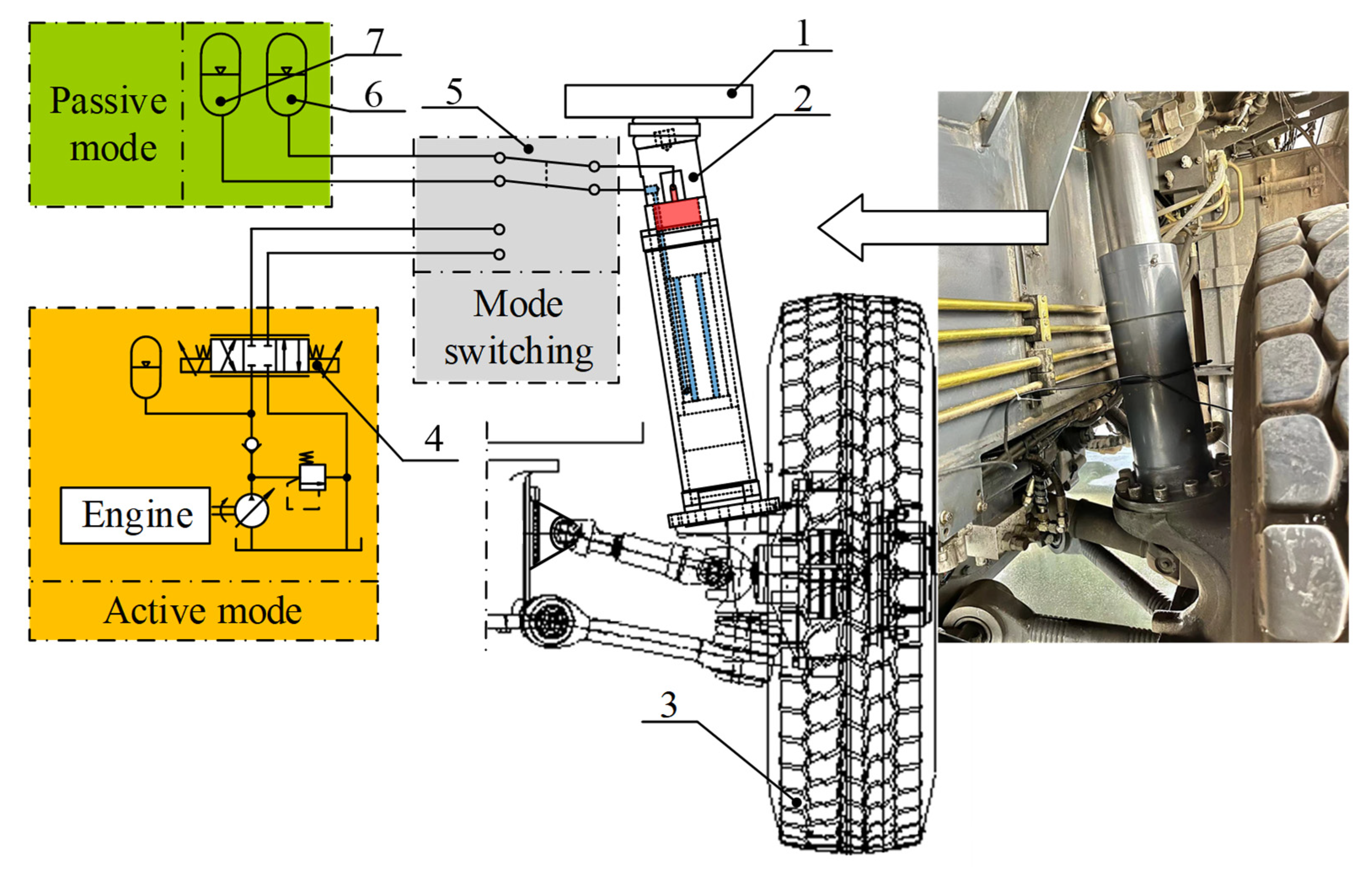
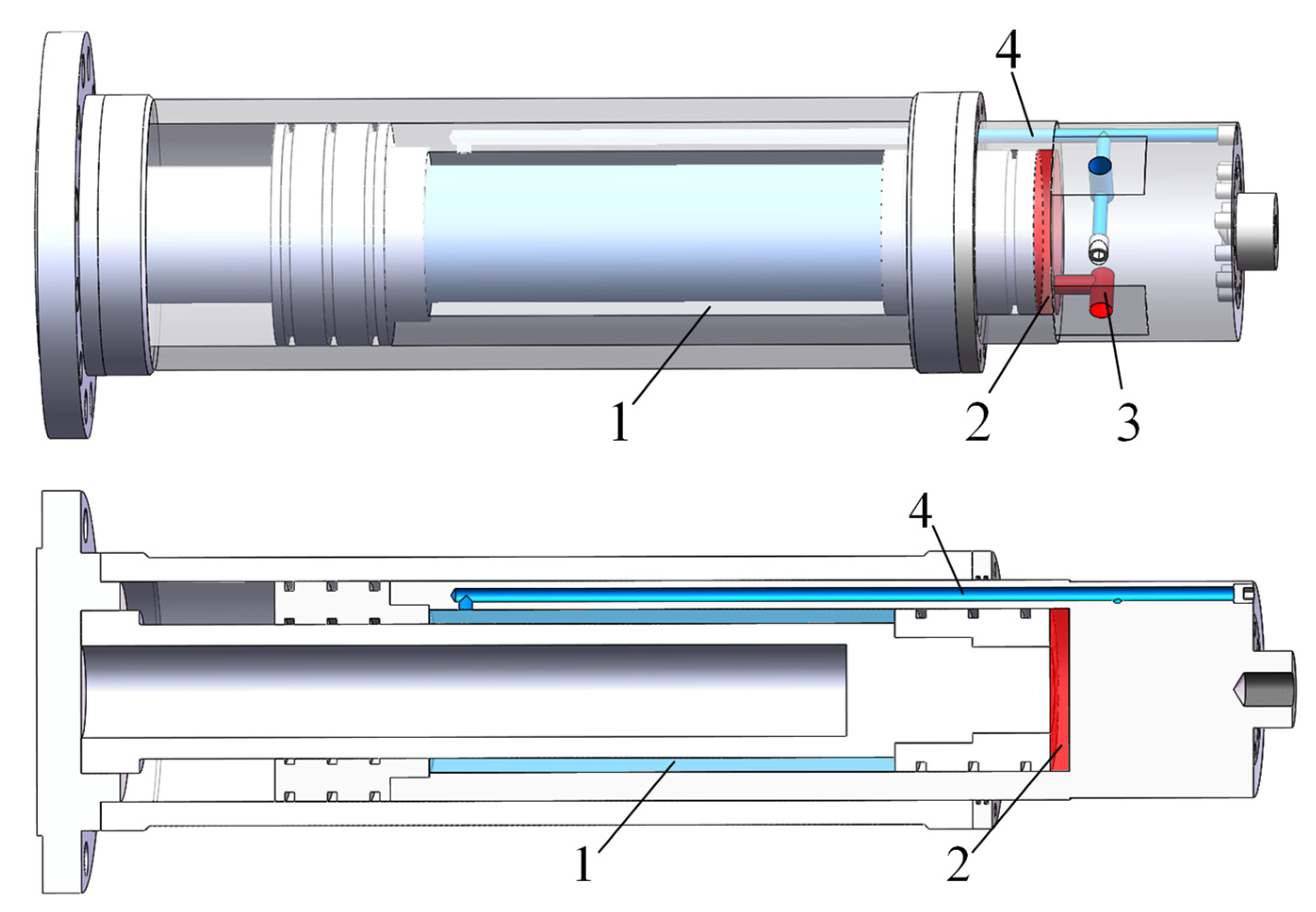
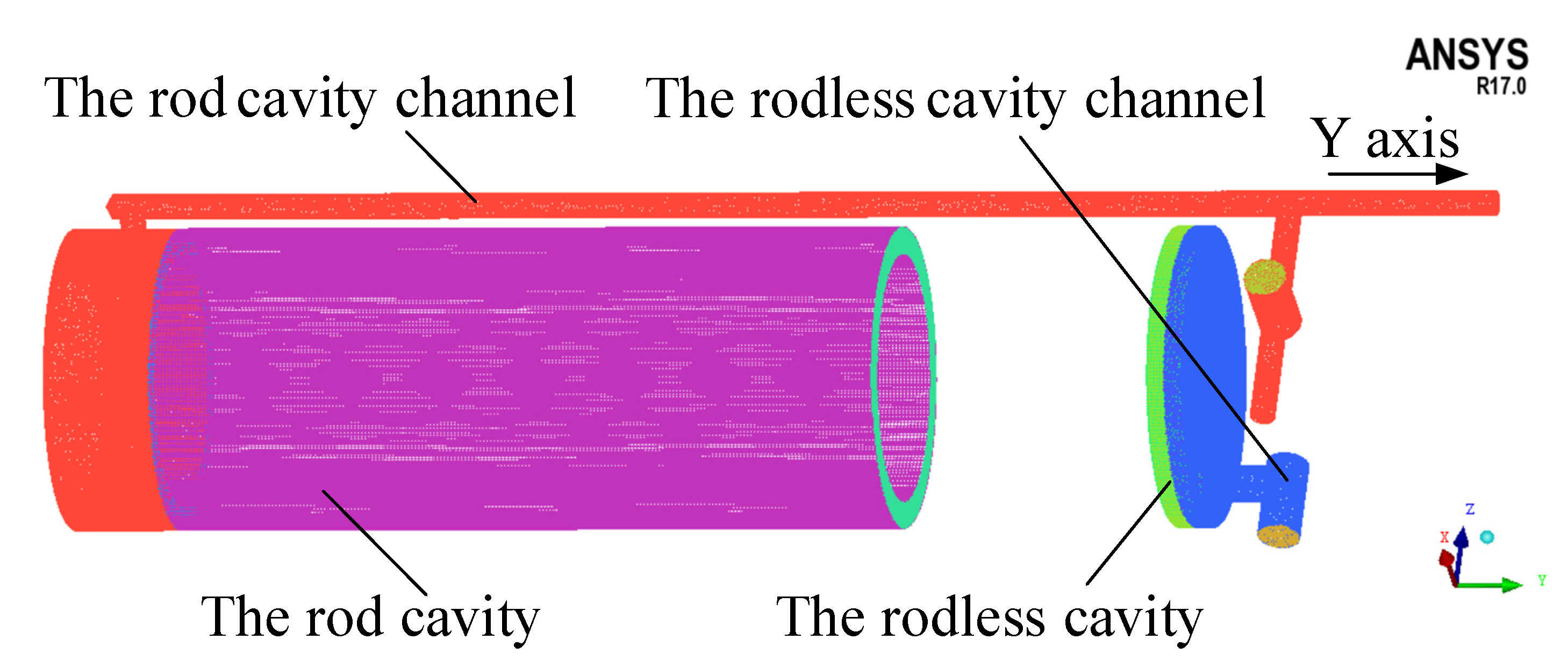
2.1. Actuator Structure and Geometric Modelling
2.2. Flow Field Simulation
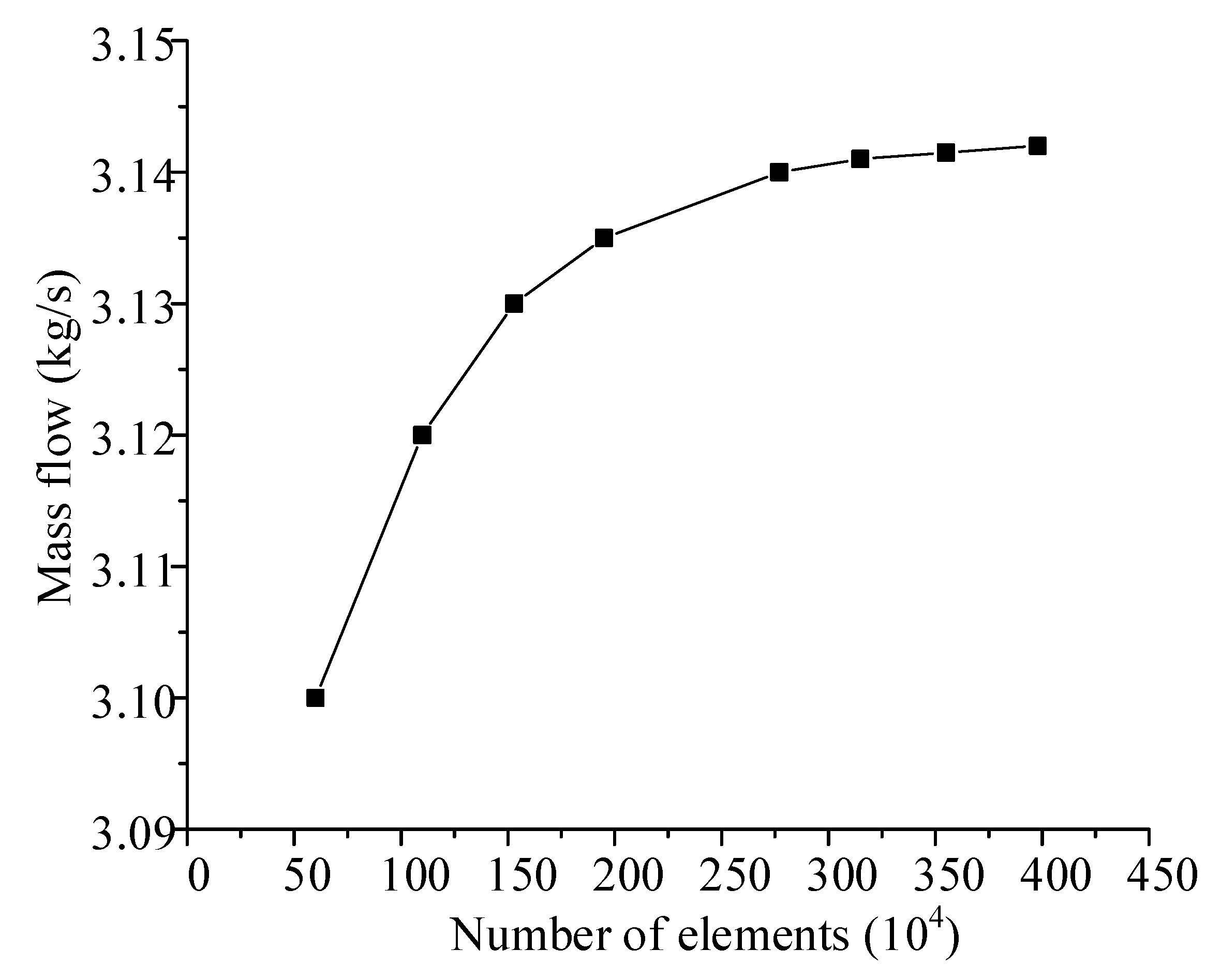
2.2.1. Meshing
2.2.2. Fluid Mechanical Governing Equation
2.2.3. Parameter Setting
3. Simulation Results and Analysis
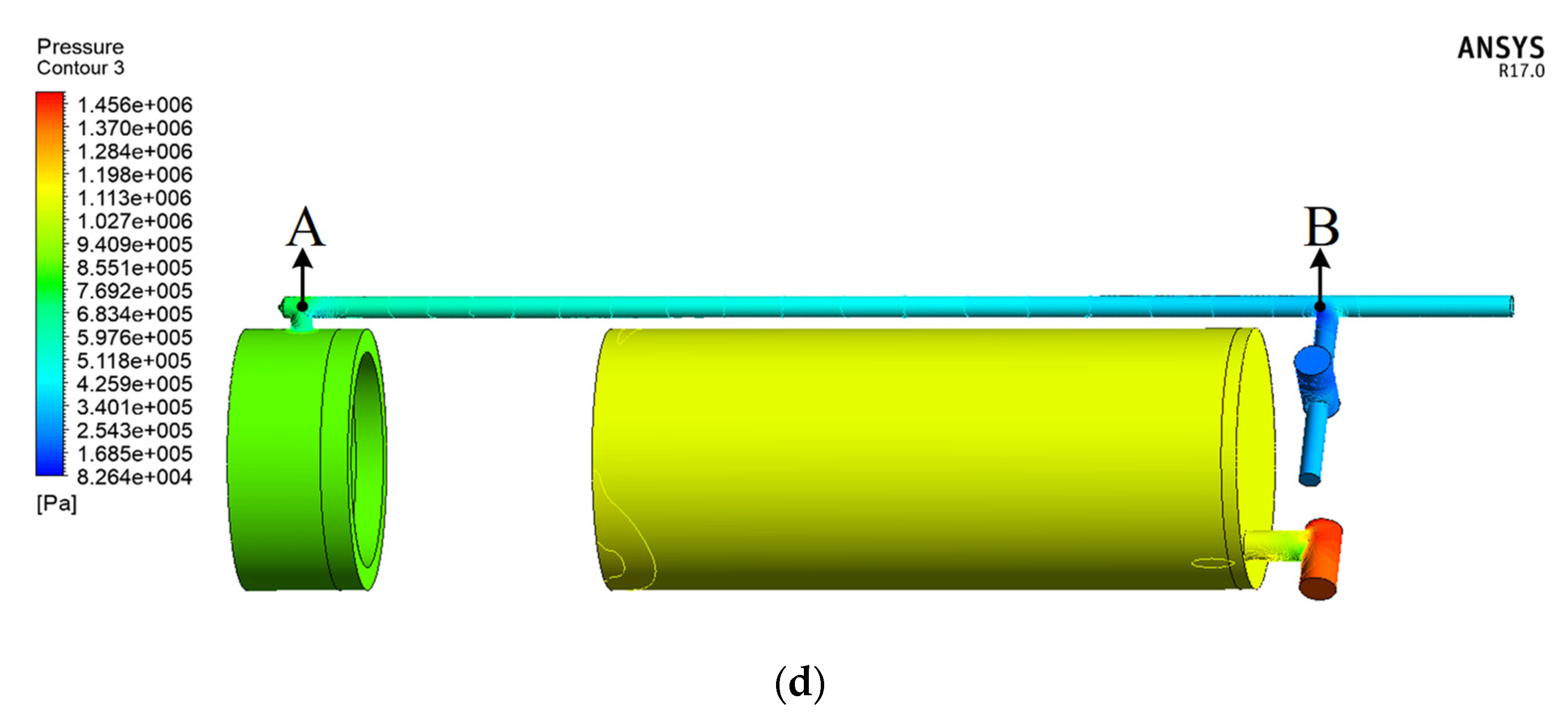
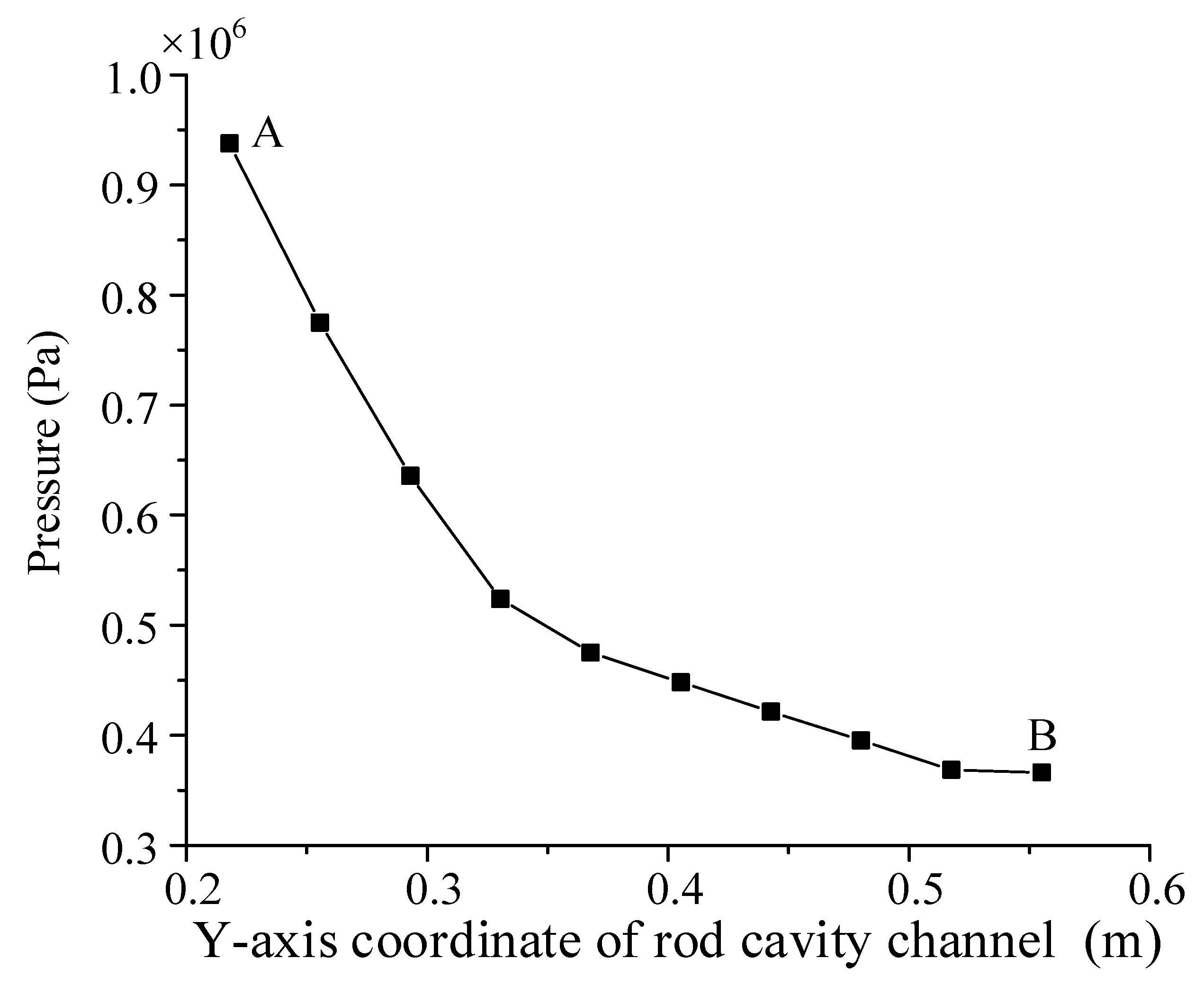
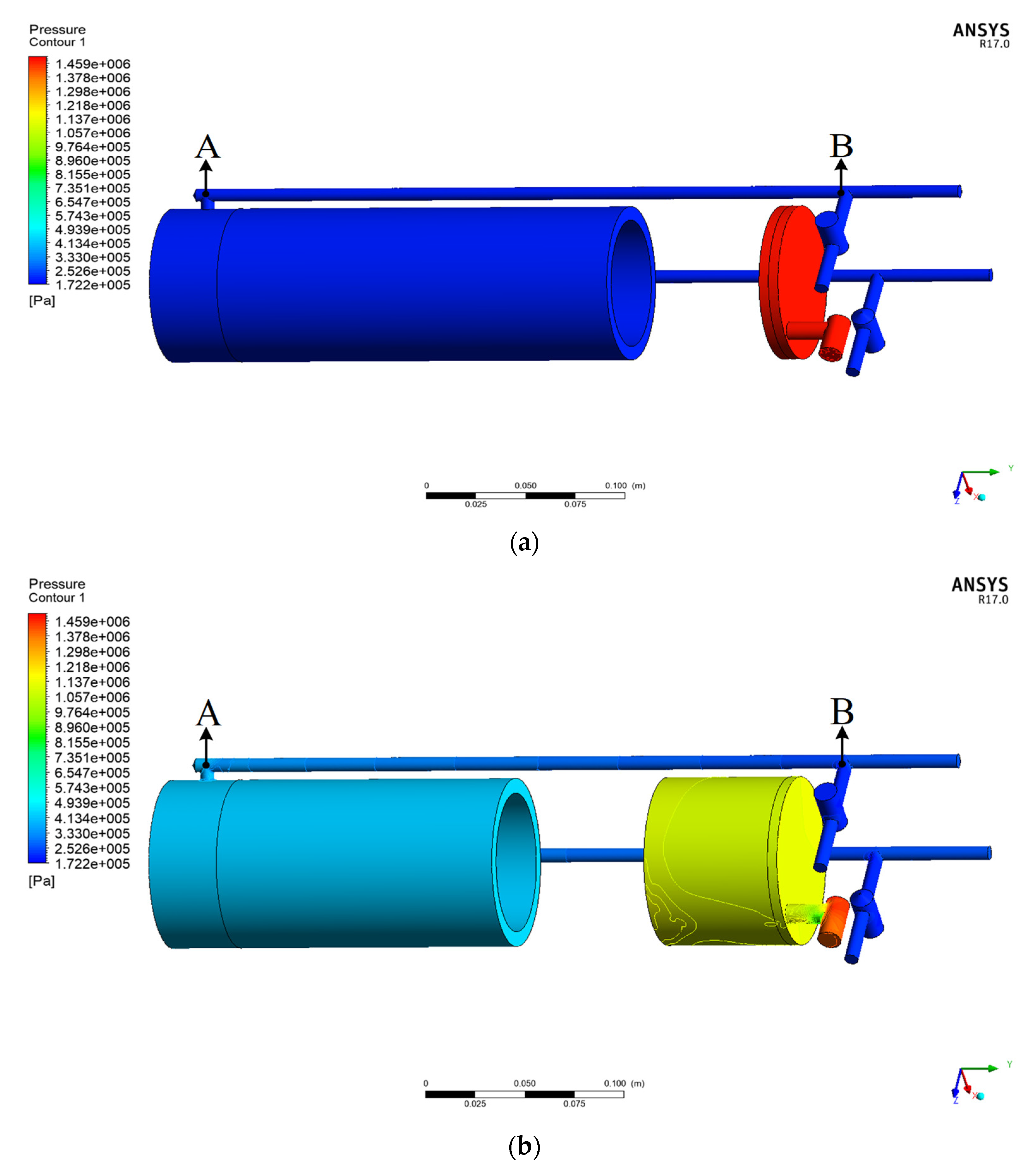
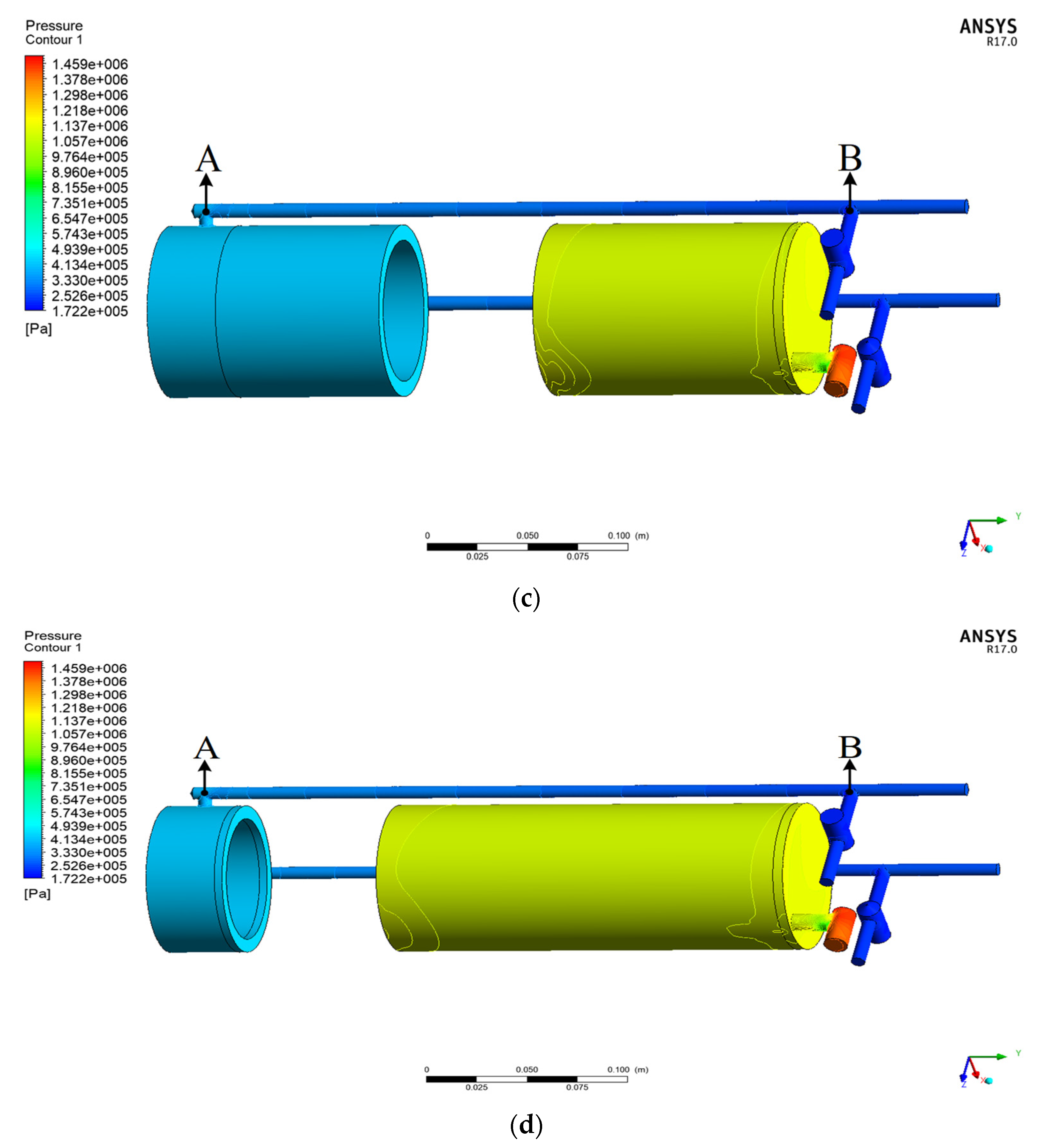
3.1. Actuator Flow Field Pressure Contour
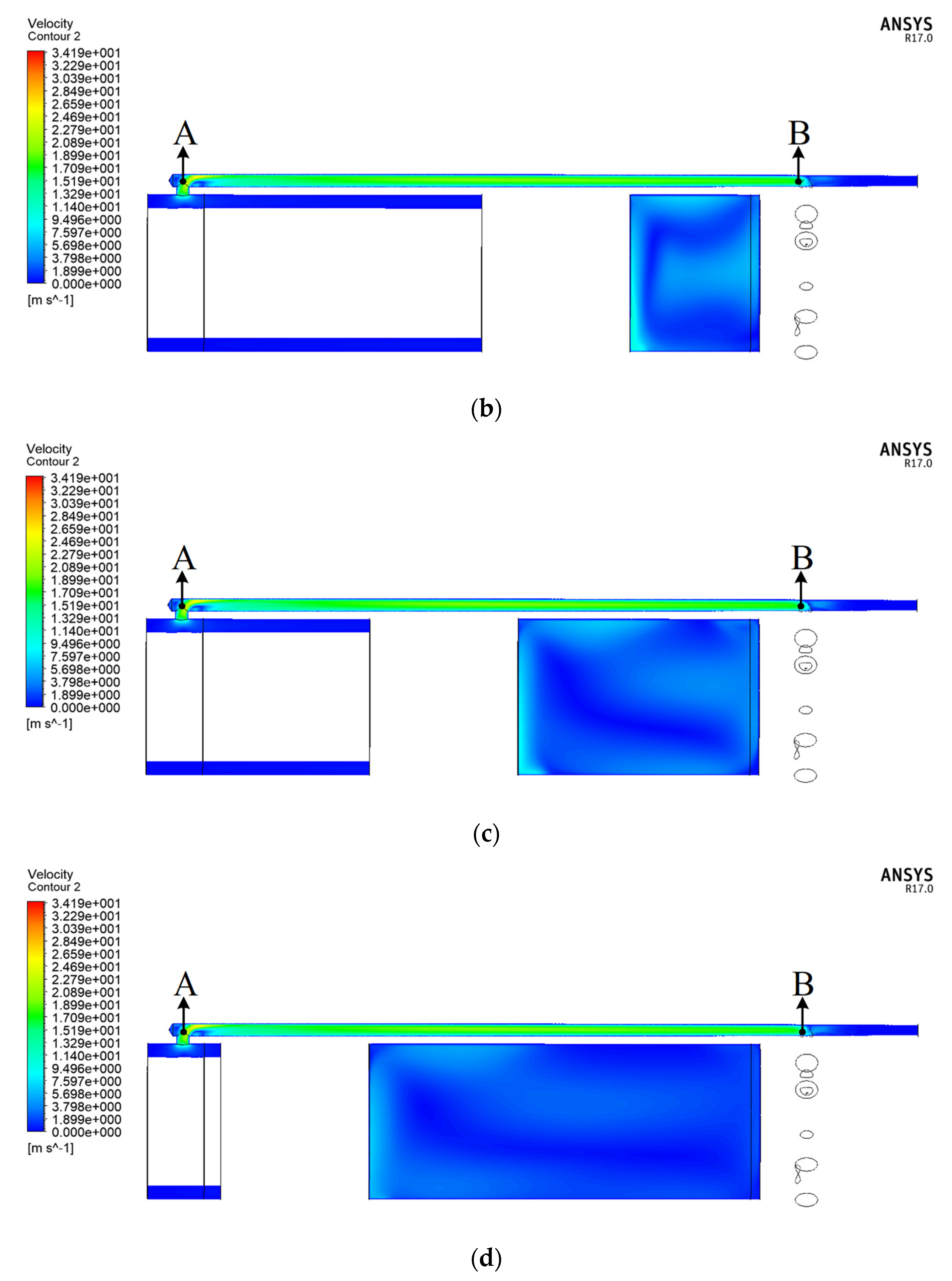
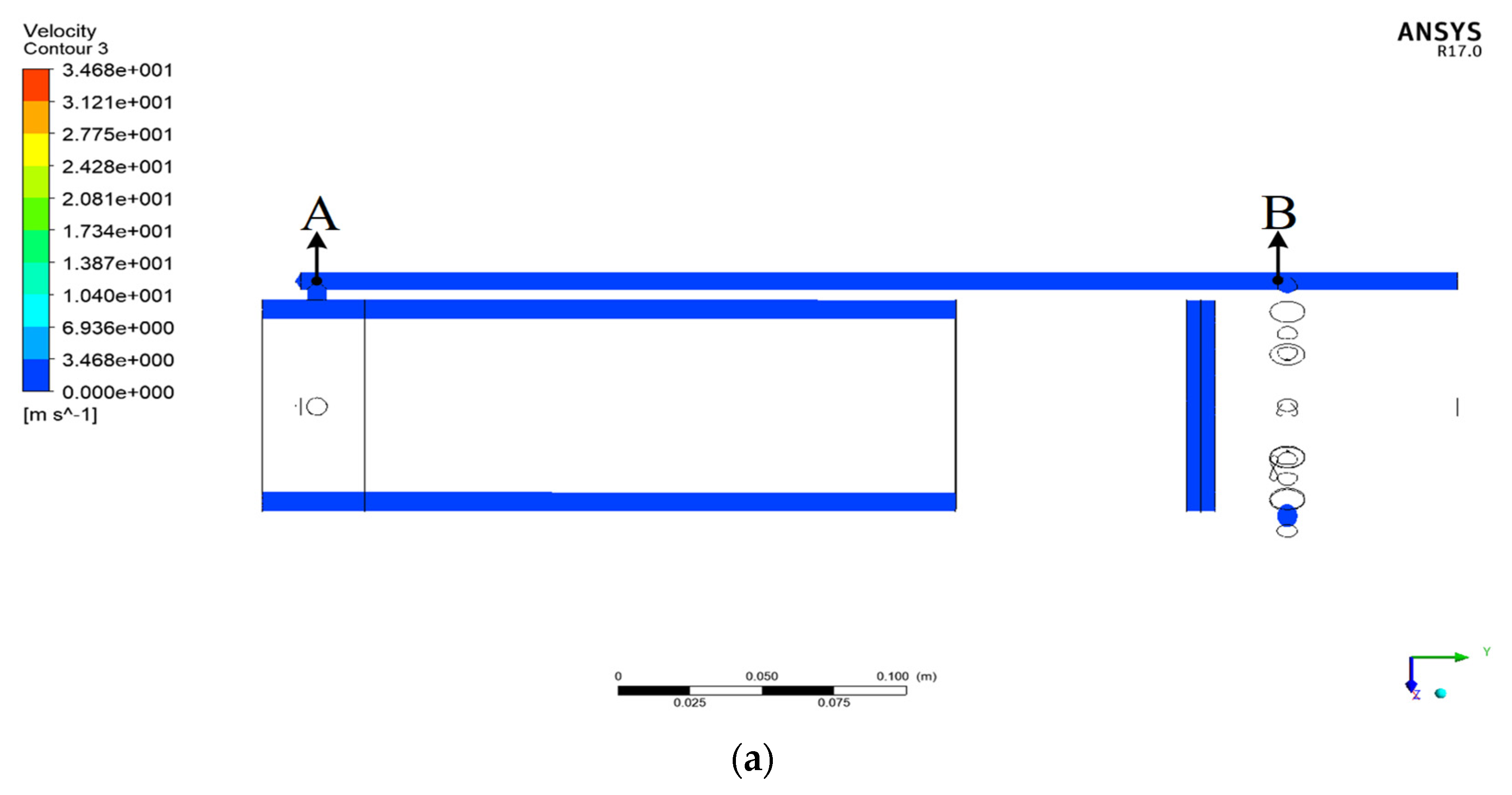
3.2. Actuator Flow Field Velocity Contour
4. Structure Design and Flow Field Simulation of the APCSA
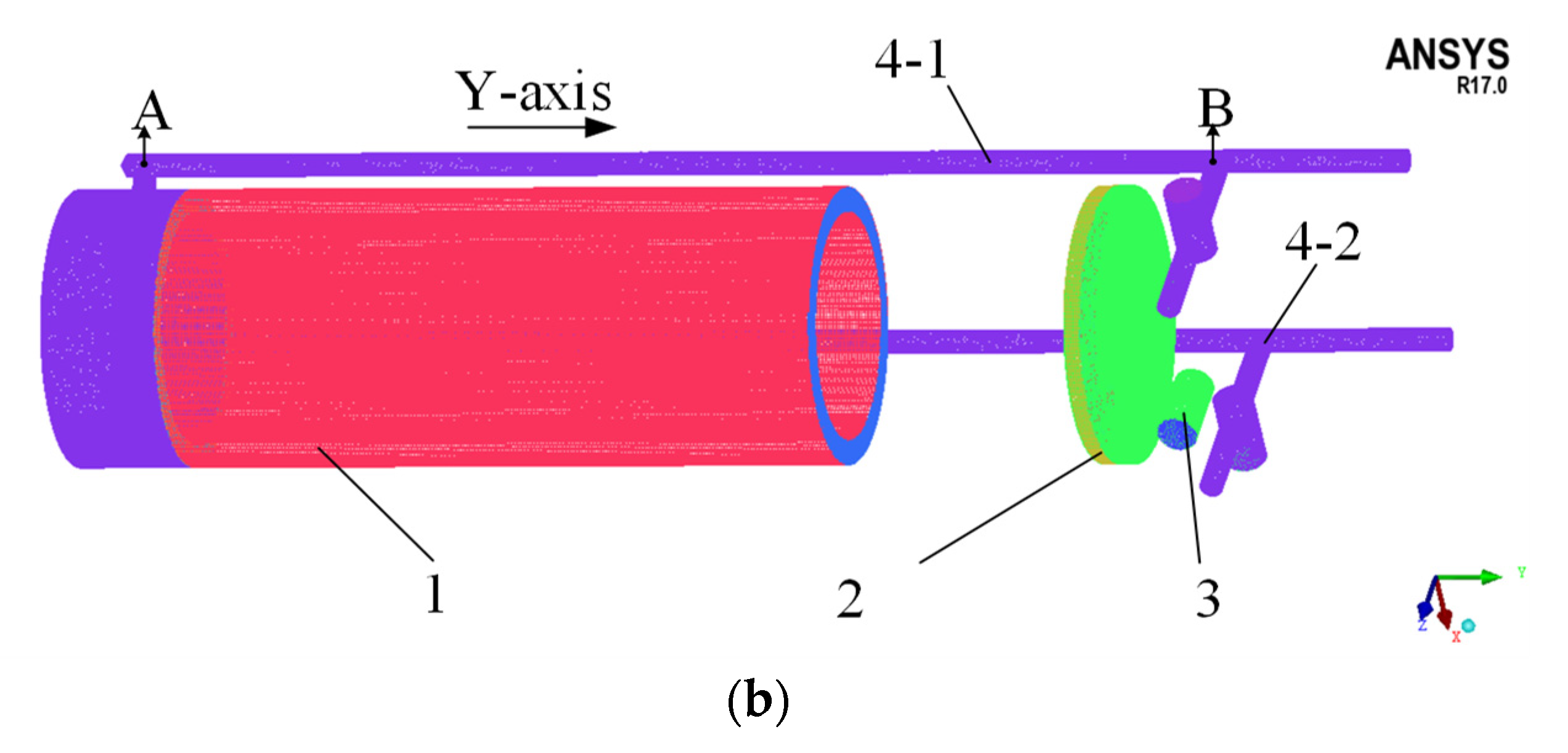
4.1. Structural Design of the APCSA
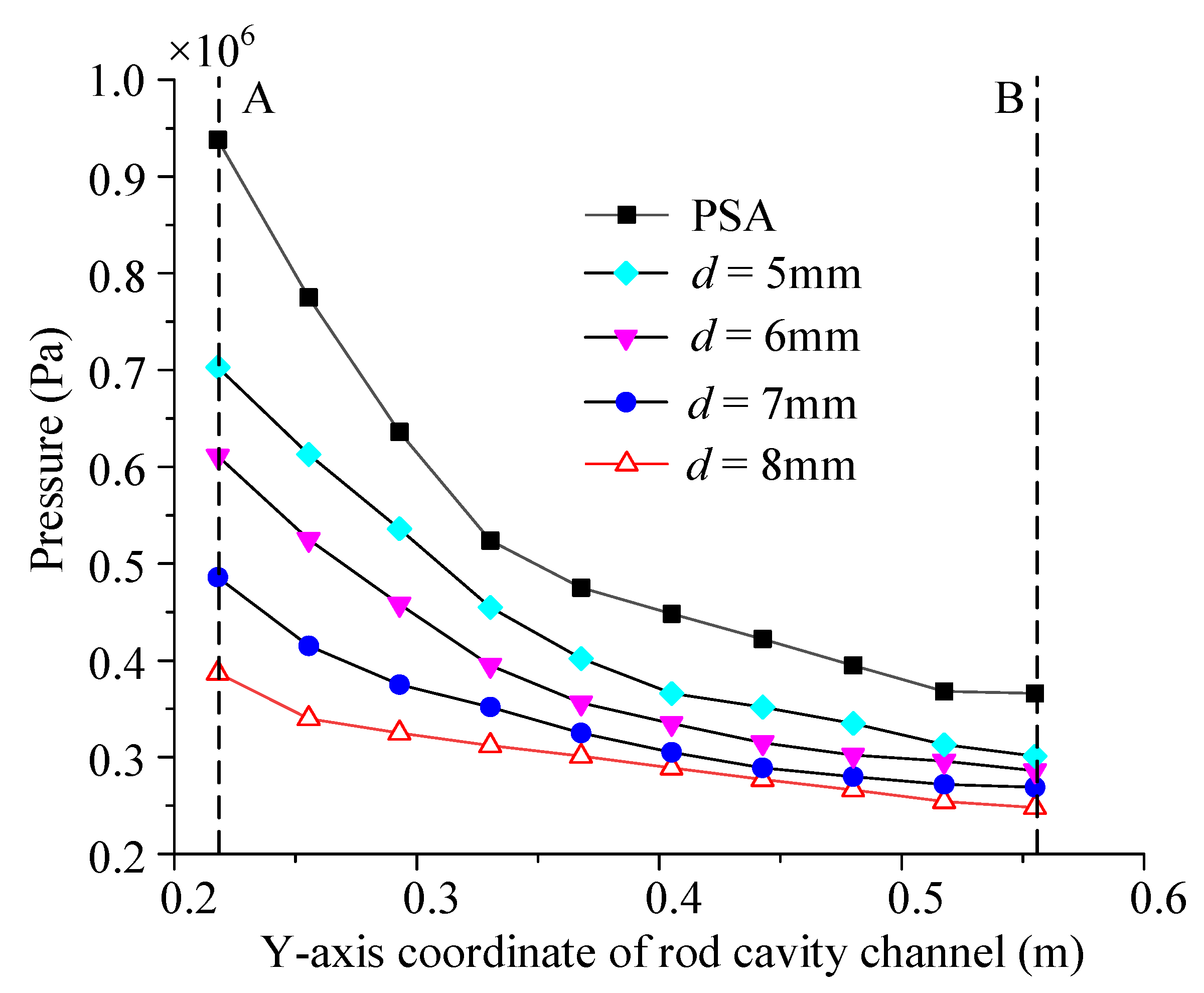
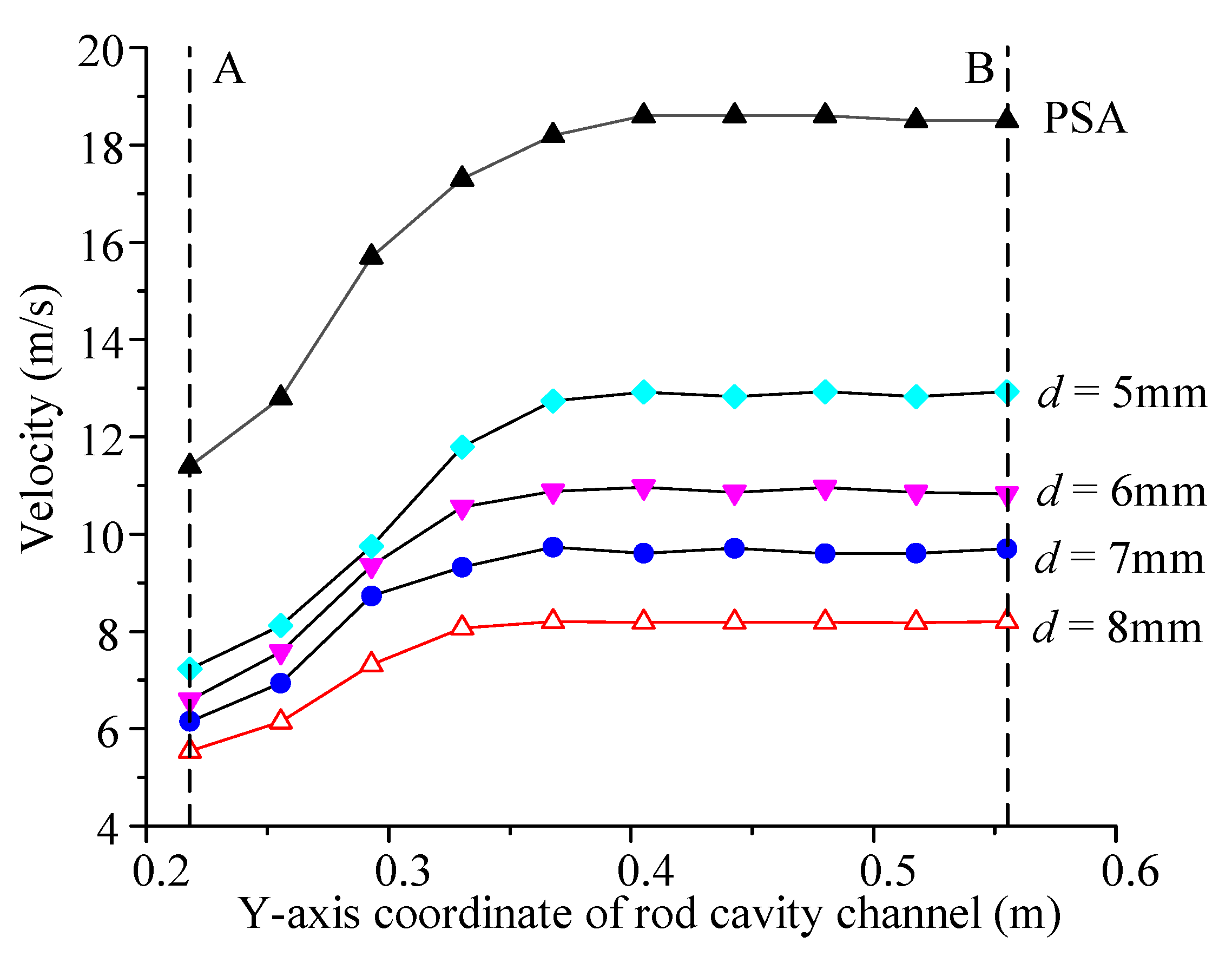
4.2. Simulation Results (d = 8 mm)
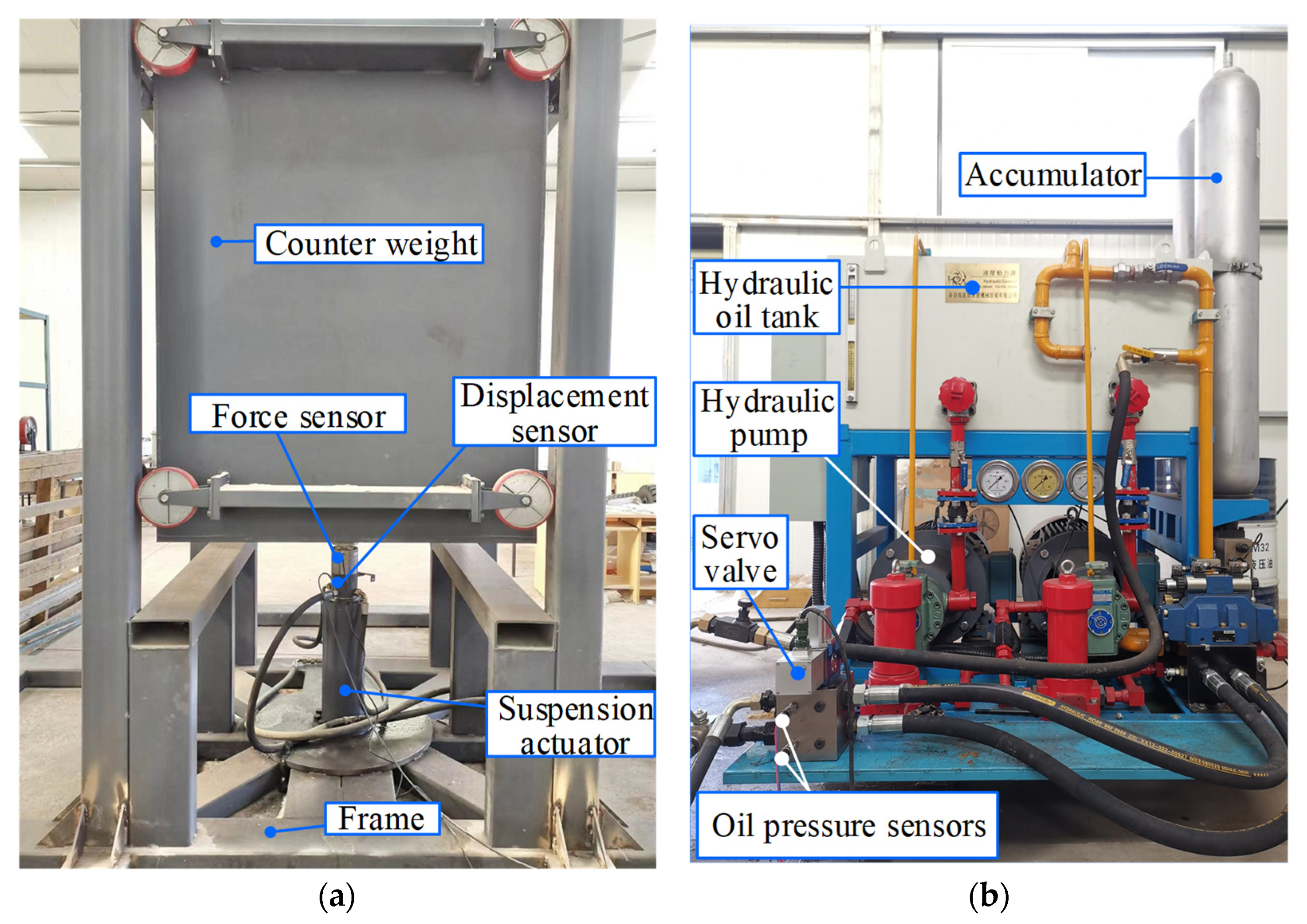
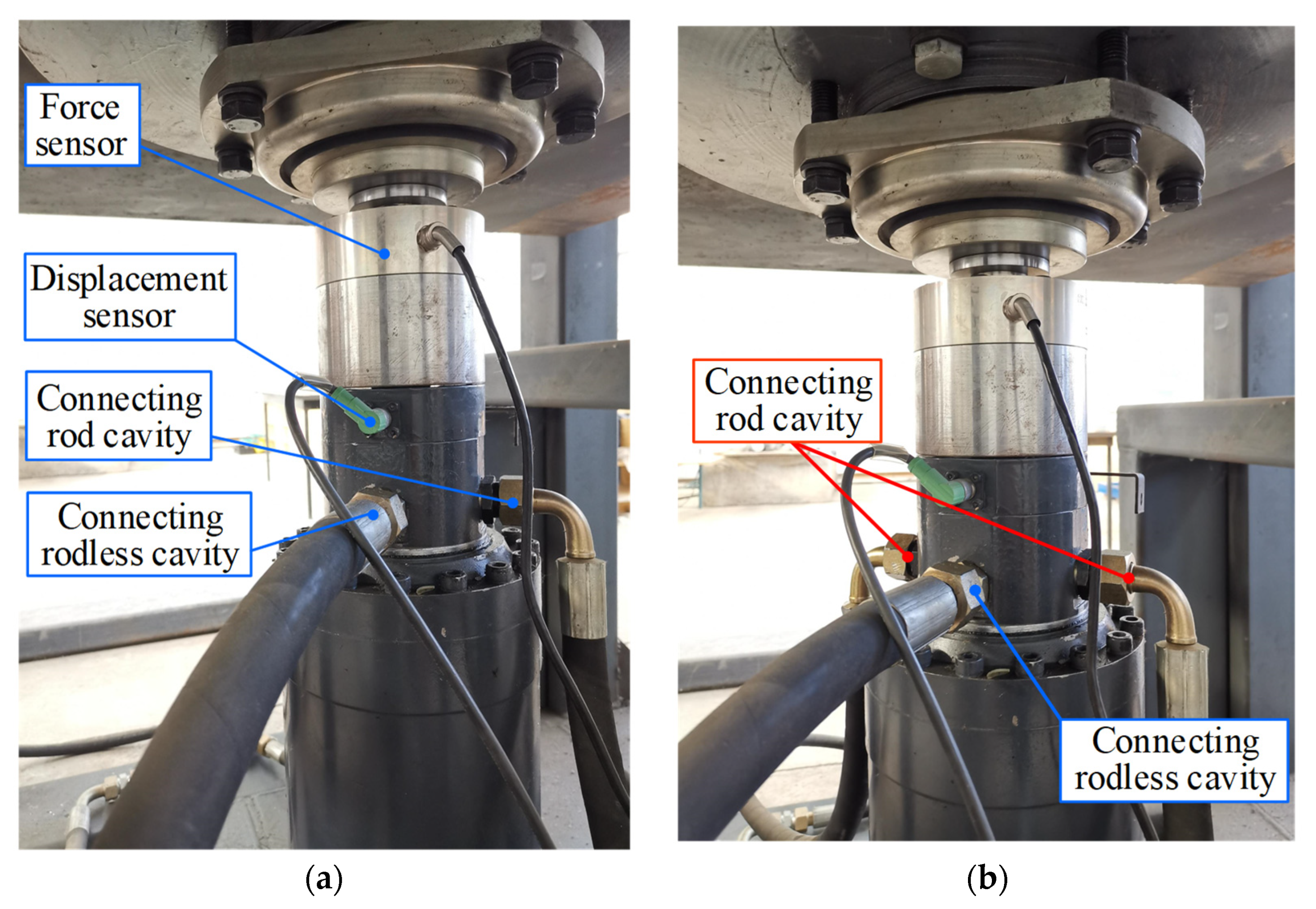
5. Experiment
6. Conclusions
- (1)
- Combined with CFD theory and the dynamic mesh technique, a simulation study on a typical PSA is carried out to redesign the actuator structure and overcome the structural barriers between passive and active suspension actuators. Hence, a type of APCSA can be obtained to meet the needs of switching between active mode and passive mode.
- (2)
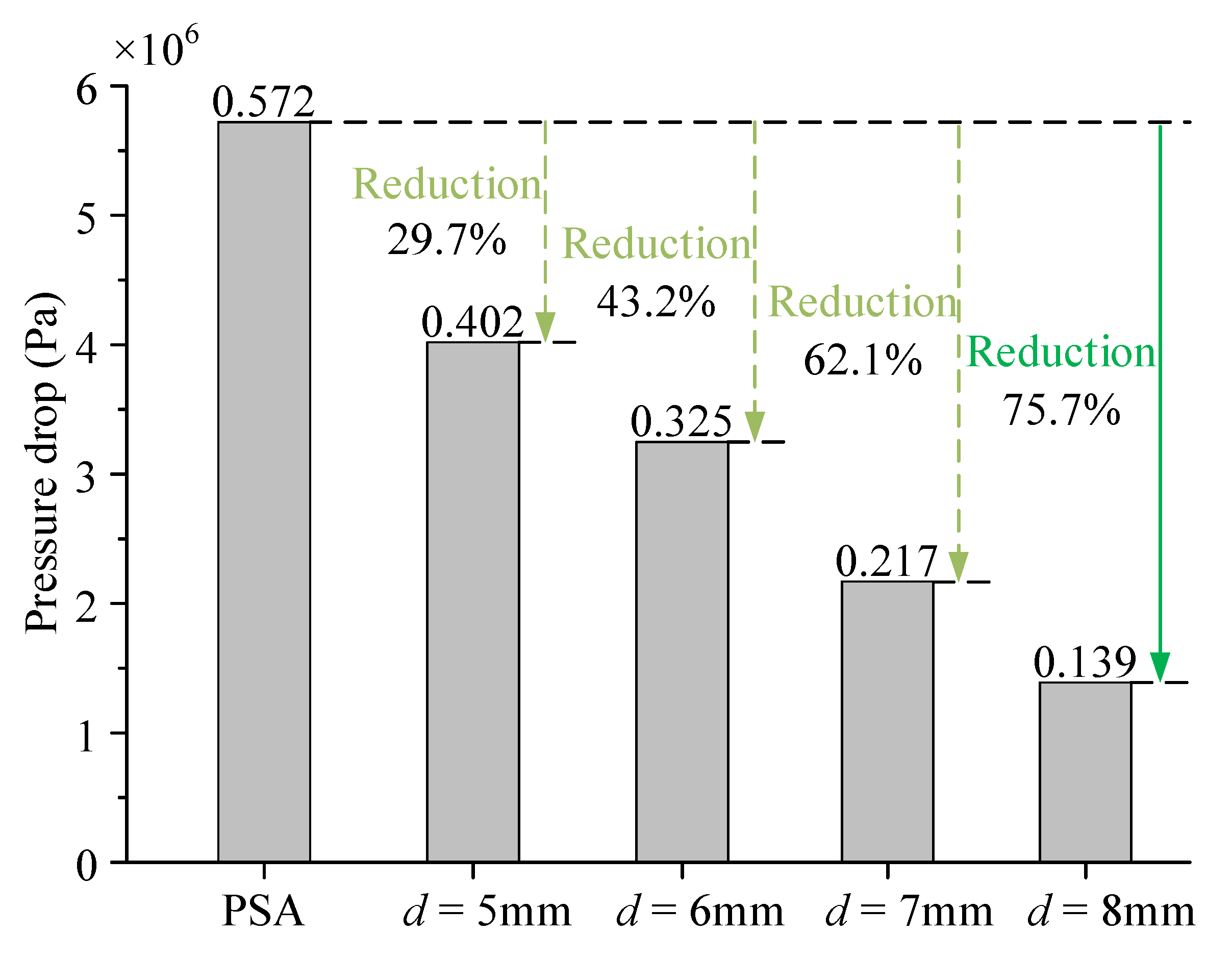
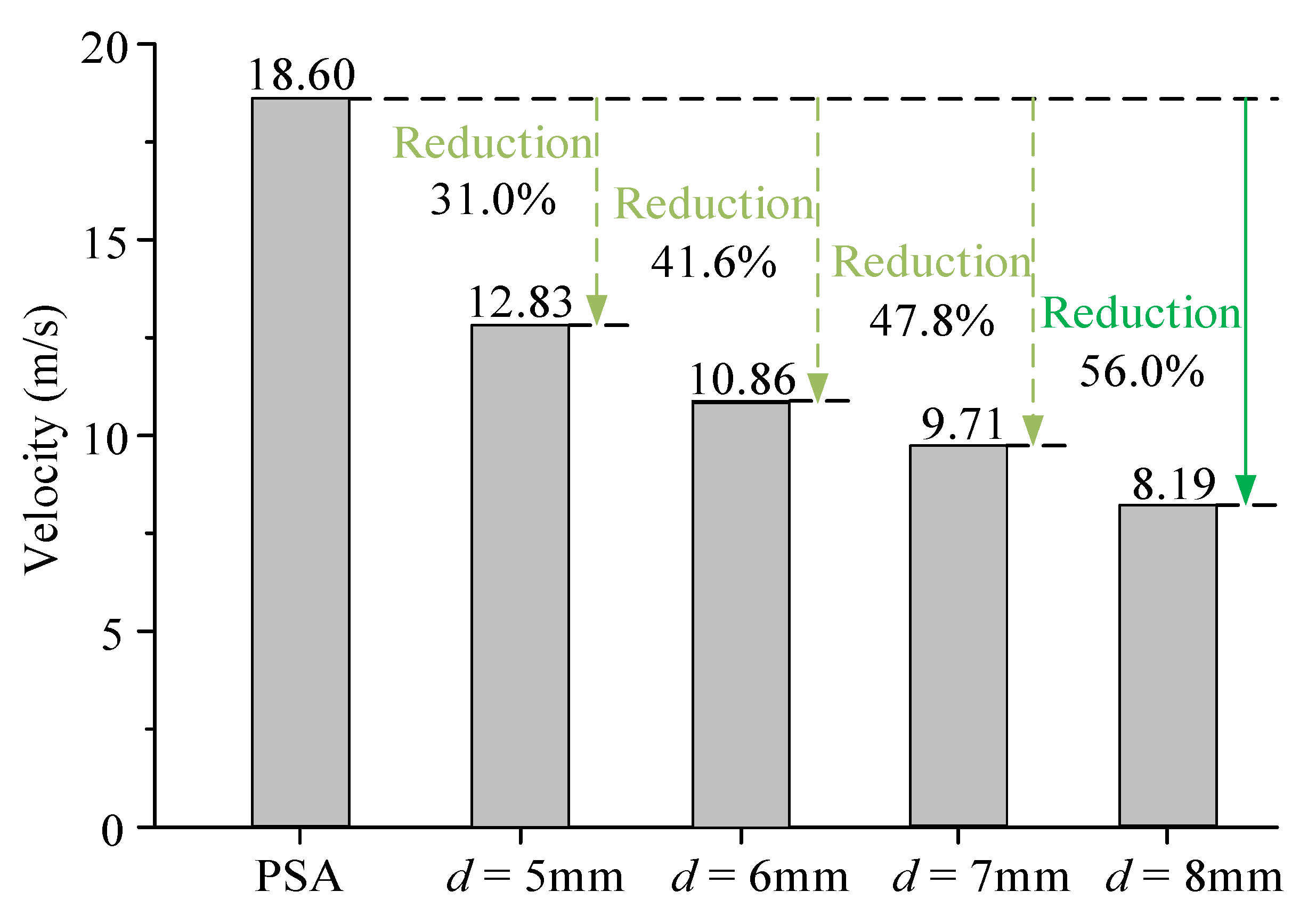
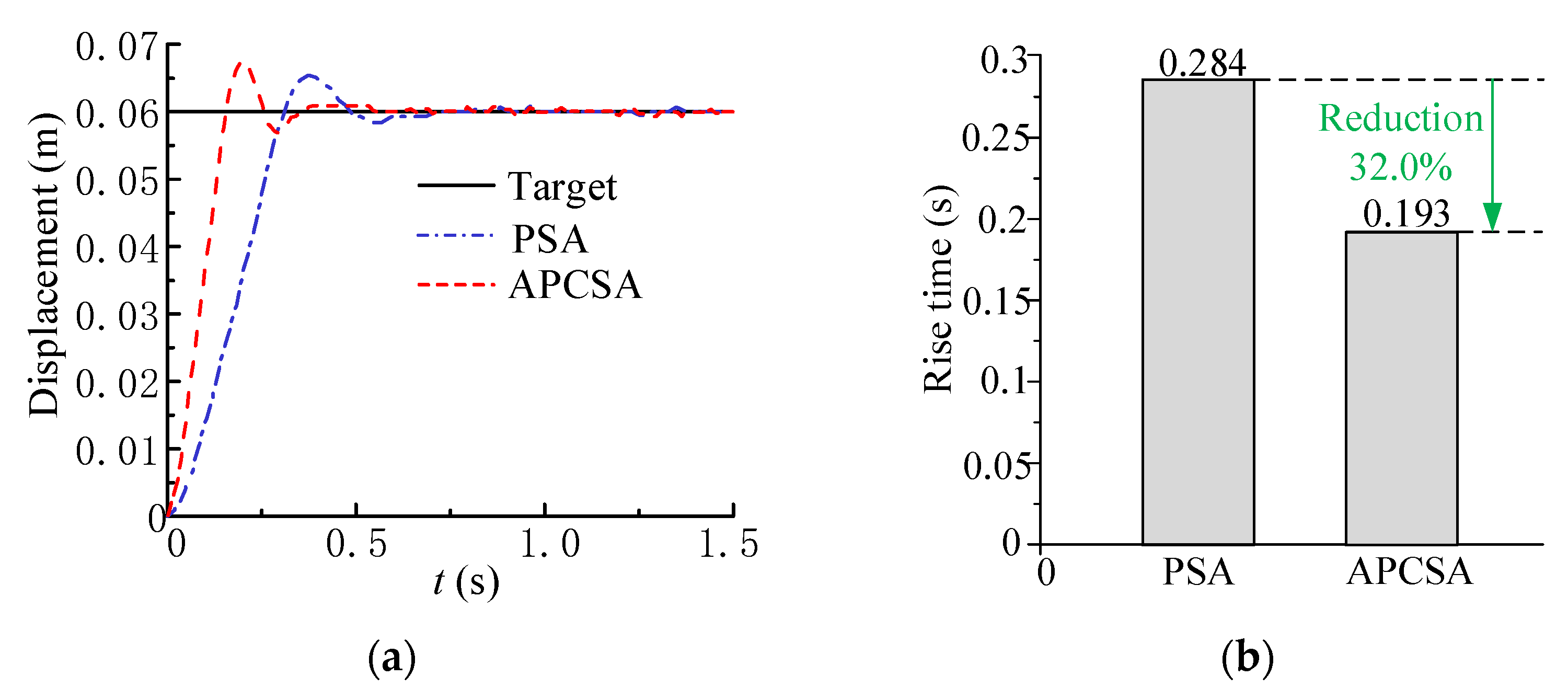
- The flow field simulation results indicate that after diameter selection, the pressure loss of the rod cavity channel of the PSA is 0.572 MPa, and the maximum flow speed is 18.63 m/s. Meanwhile, the pressure loss of the rod cavity channel of the APCSA is 0.139 MPa, and the maximum flow speed is 8.19 m/s. Thus, the pressure loss is reduced by 75.7%, and the maximum flow speed is reduced by 56.0%. The effect of the structural redesign is obvious.
- (3)
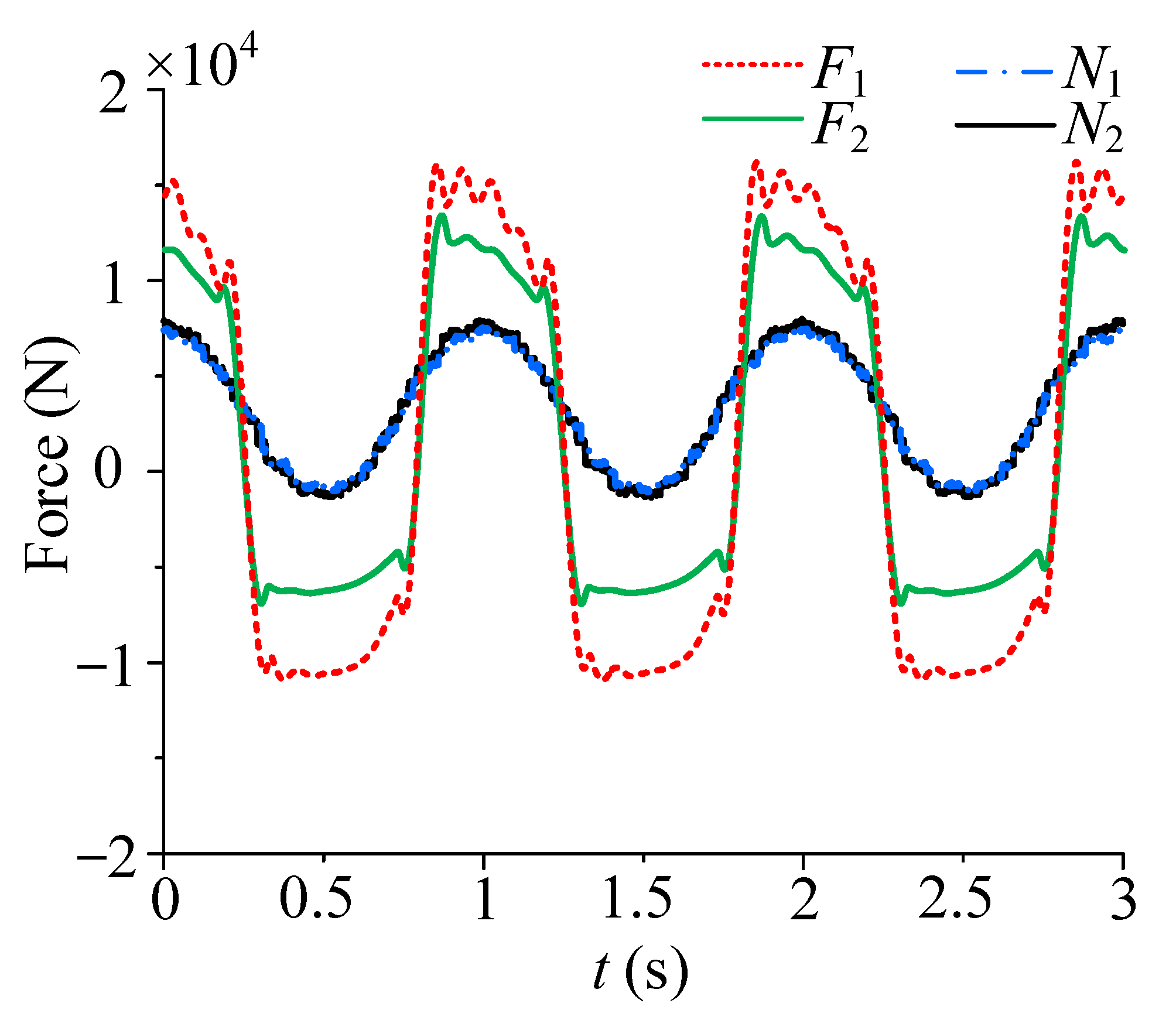
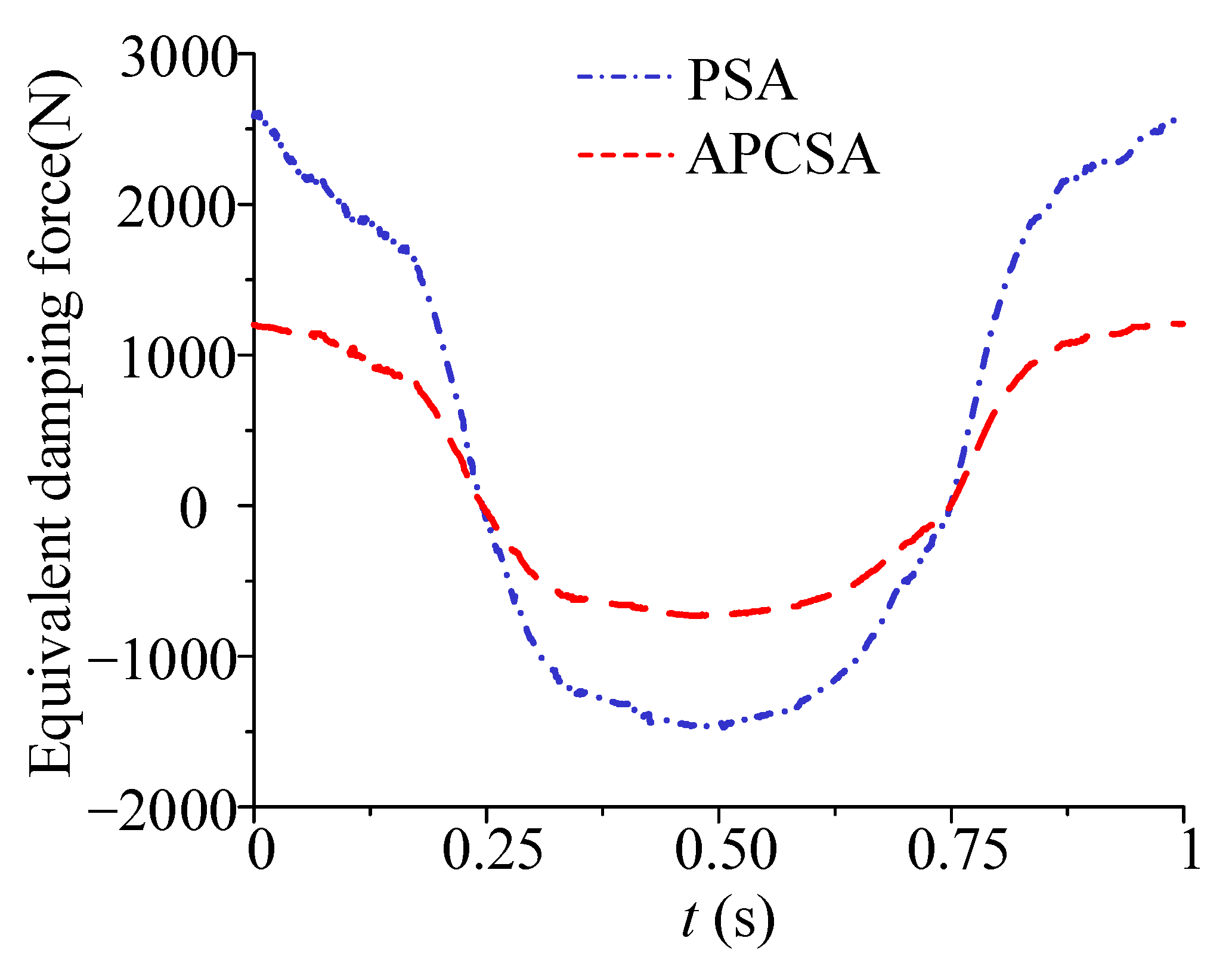
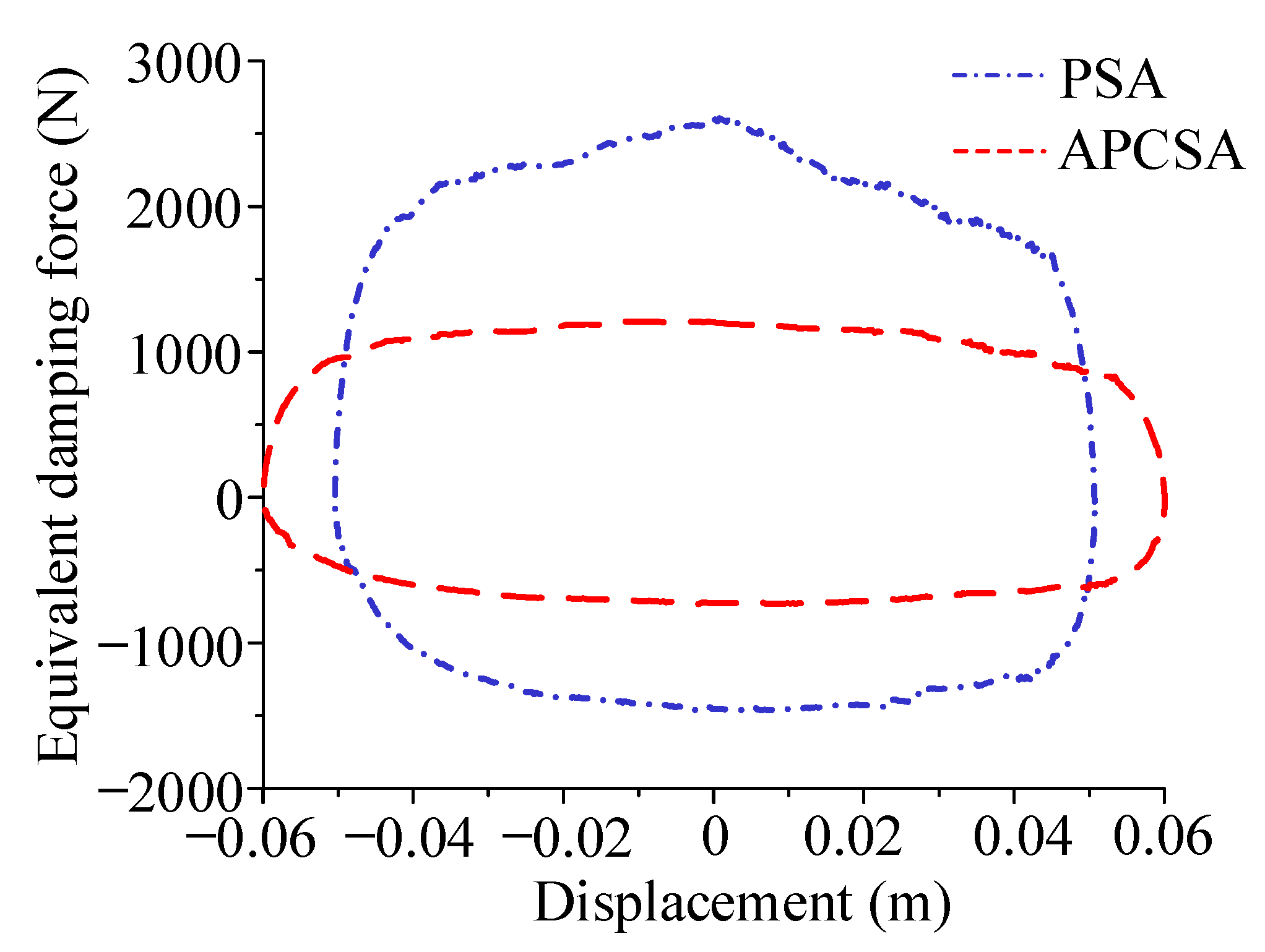
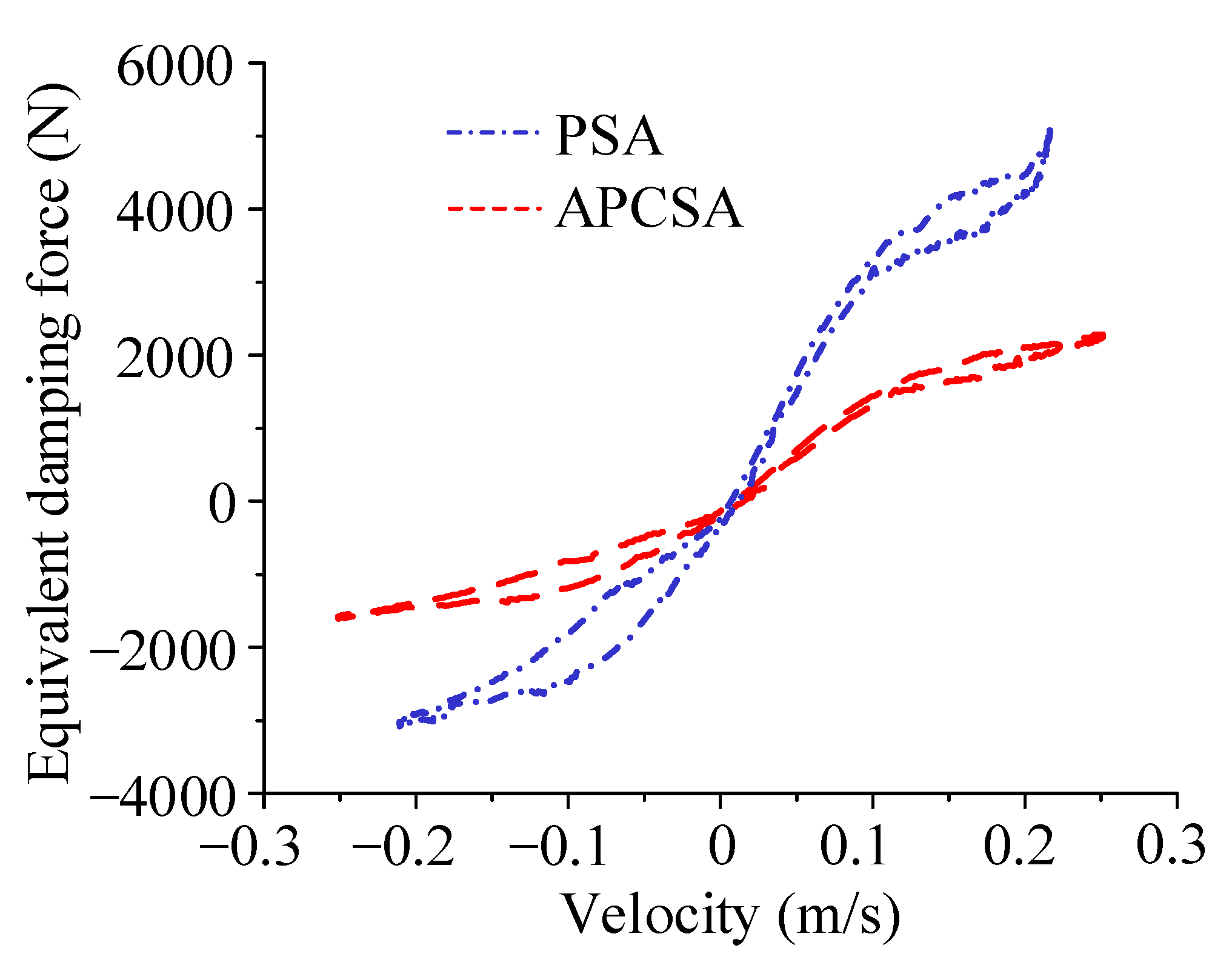
- In the step and sinusoidal response experiments, the response velocity and displacement following performance of the APCSA are significantly improved compared with the PSA. The maximum restoring damping force/compression damping force of the APCSA is 2428.98 N/−1470.29 N, which is 53.5%/50.4% lower than that of the PSA. The dynamic response ability of the actuator is improved. The APCSA will assist the active suspension system of the whole vehicle to realize various advanced control algorithms.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhou, C.; Liu, X.H.; Xu, F.X. Intervention criterion and control strategy of active front steering system for emergency rescue vehicle. Mech. Syst. Signal Process. 2021, 148, 107160. [Google Scholar] [CrossRef]
- Xue, J.; Tu, Q.; Pan, M.; Lai, X.; Zhou, C. An improved energy management strategy for 24t heavy-duty hybrid emergency rescue vehicle with dual-motor torque increasing. IEEE Access 2020, 9, 5920–5932. [Google Scholar] [CrossRef]
- Li, P.; Yan, J.; Tu, Q.; Pan, M.; Xue, J. A novel energy management strategy for series hybrid electric rescue vehicle. Math. Probl. Eng. 2018, 2018, 1–15. [Google Scholar] [CrossRef]
- Xu, F.; Liu, X.; Chen, W.; Zhou, C. Dynamic switch control of steering modes for four wheel independent steering rescue vehicle. IEEE Access 2019, 7, 135595–135605. [Google Scholar] [CrossRef]
- Jeong, K.; Choi, S.B.; Choi, H. Sensor fault detection and isolation using a support vector machine for vehicle suspension systems. IEEE T. Veh. Technol. 2020, 69, 3852–3863. [Google Scholar] [CrossRef]
- Abdi, B.; Mirzaei, M.; Gharamaleki, R.M. A new approach to optimal control of nonlinear vehicle suspension system with input constraint. J. Vib. Control 2018, 24, 3307–3320. [Google Scholar] [CrossRef]
- Jing, X.J.; Pan, H.H.; Sun, W.C. Robust finite-time tracking control for nonlinear suspension systems via disturbance compensation. Mech. Syst. Signal Process. 2017, 88, 49–61. [Google Scholar]
- Shah, D.; Santos, M.M.D.; Chaoui, H.; Justo, J.F. Event-triggered non-switching networked sliding mode control for active suspension system with random actuation network delay. IEEE T. Intell. Transp. early access. 2021. [Google Scholar] [CrossRef]
- Bai, R.; Guo, D. Sliding-mode control of the active suspension system with the dynamics of a hydraulic actuator. Complexity 2018, 2018, 5907208. [Google Scholar] [CrossRef]
- Liu, Y.J.; Zeng, Q.; Tong, S.; Chen, C.; Liu, L. Actuator failure compensation-based adaptive control of active suspension systems with prescribed performance. IEEE T. Ind. Electron. 2020, 67, 7044–7053. [Google Scholar] [CrossRef]
- Attia, T.; Vamvoudakis, K.G.; Kochersberger, K.; Bird, J.; Furukawa, T. Simultaneous dynamic system estimation and optimal control of vehicle active suspension. Vehicle Syst. Dyn. 2019, 57, 1467–1493. [Google Scholar] [CrossRef]
- Ramalingam, M.; Thirumurugan, M.A.; Kumar, T.A.; Jebaseelan, D.D.; Jebaraj, C. Response characteristics of car seat suspension using intelligent control policies under small and large bump excitations. Int. J. Dyn. Control 2020, 8, 545–557. [Google Scholar] [CrossRef]
- Enders, E.; Burkhard, G.; Munzinger, N. Analysis of the influence of suspension actuator limitations on ride comfort in passenger cars using model predictive control. Actuators 2020, 9, 77. [Google Scholar] [CrossRef]
- Han, S.Y.; Zhang, C.H.; Tang, G.Y. Approximation optimal vibration for networked nonlinear vehicle active suspension with actuator time delay. Asian J. Control 2017, 19, 983–995. [Google Scholar] [CrossRef]
- Li, H.; Gao, H.; Liu, H.; Liu, M. Fault-tolerant h control for active suspension vehicle systems with actuator faults. Part I J. Syst. Control. Eng. 2011, 226, 348–363. [Google Scholar] [CrossRef]
- Tang, X.; Duan, X.; Gao, H.; Li, X.; Shi, X. CFD investigations of transient cavitation flows in pipeline based on weakly-compressible model. Water 2020, 12, 448. [Google Scholar] [CrossRef]
- Liu, C.; Li, X.; Li, A.; Cui, Z.; Li, Y. Cavitation onset caused by a dynamic pressure wave in liquid pipelines. Ultrason. Sonochem. 2020, 68, 105225. [Google Scholar] [CrossRef]
- Abdulkarim, A.H.; Ates, A.; Altinisik, K.; Canli, E. Internal flow analysis of a porous burner via CFD. Int. J. Numer. Method Heat 2019, 29, 2666–2683. [Google Scholar] [CrossRef]
- Hsu, C.H.; Chen, J.L.; Yuan, S.C.; Kung, K.Y. CFD simulations on the rotor dynamics of a horizontal axis wind turbine activated from stationary. Appl. Mech. 2021, 2, 147–158. [Google Scholar] [CrossRef]
- Rezaeiha, A.; Montazeri, H.; Blocken, B. CFD analysis of dynamic stall on vertical axis wind turbines using Scale-Adaptive Simulation (SAS): Comparison against URANS and hybrid RANS/LES. Energ. Convers. Manag. 2019, 196, 1282–1298. [Google Scholar] [CrossRef]
- Yang, X.; Zhou, H.; Wu, H. CFD modelling of biomass ash deposition under multiple operation conditions using a 2D mass-conserving dynamic mesh approach. Fuel 2022, 316, 123250. [Google Scholar] [CrossRef]
- Mlakar, D.; Winter, M.; Stadlbauer, P.; Seidel, H. Subdivision-specialized linear algebra kernels for static and dynamic mesh connectivity on the GPU. Comput. Graph. Forum 2020, 39, 335–349. [Google Scholar] [CrossRef]
- Zhu, Q.; Zhang, Y.; Zhu, D. Study on dynamic characteristics of the bladder fluid pulsation attenuator based on dynamic mesh technology. J. Mech. Sci. Technol. 2019, 33, 1159–1168. [Google Scholar] [CrossRef]
- Salinas, P.; Regnier, G.; Jacquemyn, C.; Pain, C.C.; Jackson, M.D. Dynamic mesh optimisation for geothermal reservoir modelling. Geothermics 2021, 94, 102089. [Google Scholar] [CrossRef]
- Zheng, Z.; Yang, W.; Yu, P.; Cai, Y.; Zhou, H.; Boon, S.K. Simulating growth of ash deposit in boiler heat exchanger tube based on CFD dynamic mesh technique. Fuel 2020, 259, 1–16. [Google Scholar] [CrossRef]
- Abdalla, M.O.; Nagarajan, T. A computational study of the actuation speed of the hydraulic cylinder under different ports’ sizes and configurations. J. Eng. Sci. Technol. 2015, 10, 160–173. [Google Scholar]
- Behrens, B.A.; Hübner, S.; Krimm, R.; Wager, C.; Vucetic, M.; Cahyono, T. Development of a hydraulic actuator to superimpose oscillation in metal-forming presses. Key Eng. Mater. 2011, 473, 217–222. [Google Scholar] [CrossRef]
- Lai, Q.; Liang, L.; Li, J.; Wu, S.; Liu, J. Modeling and analysis on cushion characteristics of fast and high-flow-rate hydraulic cylinder. Math. Probl. Eng. 2016, 2016, 1–17. [Google Scholar] [CrossRef]
- Li, S.B.; Liu, Y.L. Numerical simulation on flow field of the rotary cylinder’s spiral flow. Appl. Mech. Mater. 2011, 63, 365–368. [Google Scholar] [CrossRef]






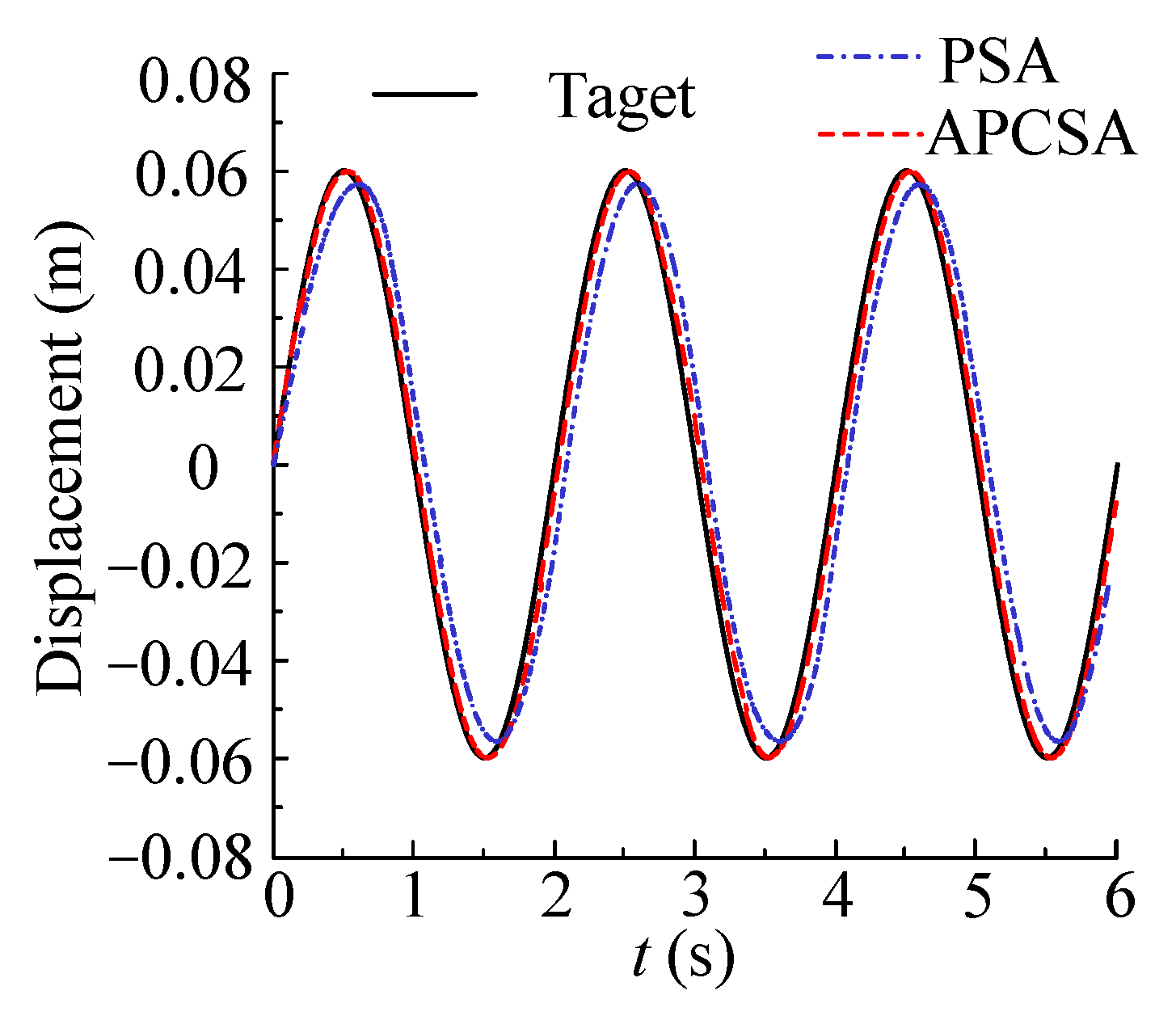

| Comparison | Time Delay (s) | Amplitude Attenuation Ratio (%) | ||
|---|---|---|---|---|
| 0.5 Hz | 1 Hz | 0.5 Hz | 1 Hz | |
| PSA | 0.10 | 0.13 | 5.5 | 10.8 |
| APCSA | 0.04 | 0.05 | 0.3 | 0.5 |
| Performance improvement | 60.0% | 61.5% | 94.5% | 95.4% |
| Maximum Damping Force | PSA | APCSA | Reduction |
|---|---|---|---|
| Restoring | 2608.16 N | 1209.88 N | 53.6% |
| Compression | −1472.51 N | −735.35 N | 50.1% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.; Gong, M.; Zhao, D.; Zhang, W.; Liu, W.; Zhang, Y. Design and Motion Characteristics of Active–Passive Composite Suspension Actuator. Mathematics 2022, 10, 4303. https://doi.org/10.3390/math10224303
Chen H, Gong M, Zhao D, Zhang W, Liu W, Zhang Y. Design and Motion Characteristics of Active–Passive Composite Suspension Actuator. Mathematics. 2022; 10(22):4303. https://doi.org/10.3390/math10224303
Chicago/Turabian StyleChen, Hao, Mingde Gong, Dingxuan Zhao, Wei Zhang, Wenbin Liu, and Yue Zhang. 2022. "Design and Motion Characteristics of Active–Passive Composite Suspension Actuator" Mathematics 10, no. 22: 4303. https://doi.org/10.3390/math10224303
APA StyleChen, H., Gong, M., Zhao, D., Zhang, W., Liu, W., & Zhang, Y. (2022). Design and Motion Characteristics of Active–Passive Composite Suspension Actuator. Mathematics, 10(22), 4303. https://doi.org/10.3390/math10224303






