Some New Generalizations of Integral Inequalities for Harmonical cr-(h₁,h₂)-Godunova–Levin Functions and Applications

Abstract

The interval analysis is famous for its ability to deal with uncertain data. This method is useful for addressing models with data that contain inaccuracies. Different concepts are used to handle data uncertainty in an interval analysis, including a pseudo-order relation, inclusion relation, and center–radius (cr)-order relation. This study aims to establish a connection between inequalities and a cr-order relation. In this article, we developed the Hermite–Hadamard ($\mathcal{H}.\mathcal{H}$) and Jensen-type inequalities using the notion of harmonical $(h_1,h_2)$-Godunova–Levin (GL) functions via a cr-order relation which is very novel in the literature. These new definitions have allowed us to identify many classical and novel special cases that illustrate our main findings. It is possible to unify a large number of well-known convex functions using the principle of this type of convexity. Furthermore, for the sake of checking the validity of our main findings, some nontrivial examples are given.

1. Introduction

There are many domains where the convexity of functions is used, including game theory, variational science, mathematical programming theory, economics, optimal control theory, etc. During the 1960s, a new field of mathematics called convex analysis began to emerge. Over the last 20 years, many authors have used some related notions of convexity and generalized different inequalities, such as h-convex functions, see Refs. [1,2,3,4]; log convex functions, see Refs. [5,6,7]; and coordinated convex functions, see Refs. [8,9]. The concept of convexity is integral to optimization concepts, which are used throughout operations research, economics, control theory, decision making, and management. Different convex functions have been used by several authors to expand and generalize integral inequalities; see Refs. [10,11,12,13,14,15,16].

It has always been a challenge in a numerical analysis to calculate errors. A lot of attention has been paid to the interval analysis as a new tool to solve uncertainty problems due to its ability to reduce calculation errors and render calculations meaningless. An interval analysis falls under the set-valued analysis, the philosophy of mathematics and topology that centers on sets. As opposed to point variables, it deals with interval variables, and the computation results are expressed as intervals, so it eliminates errors that cause misleading conclusions. First of all, an interval analysis was applied by Moore [17], in 1966, to an automatic error analysis to handle the uncertainty in data. The result was an improvement in the calculation performance, and the work attracted a great deal of attention from scholars. With their ability to be expressed as uncertain variables, they are useful in a variety of applications, such as computer graphics [18], an automatic error analysis [19], a decision analysis [20], etc. For readers interested in the interval analysis, there are many excellent applications and results available in different fields of mathematics; see Refs. [21,22,23,24,25,26].

A generalized convexity mapping, on the other hand, has the capability of tackling a variety of problems in both a nonlinear and pure analysis. Recently, several related classes of convexity have been used to construct well-known inequalities, including Jensen, Simpson, Opial, Ostrowski, Bullen, and the famous Hermite–Hadamard which are extended in the context of interval-valued functions ($\mathcal{IVFS}$). It was Chalco-Cano [27] who used a derivative of the Hukuhara type to establish interval-based inequalities for the Ostrowski type. Costa developed Opial-type inequalities for $\mathcal{IVFS}$ in [28]. Among the inequalities, Beckenbach and Roman-Flores proposed the Minkowski inequalities for $\mathcal{IVFS}$ in [29]. The literature review revealed that most authors examined inequalities using an inclusion relation like in 2018. Zhao et al. developed these inequalities for the h-convex $\mathcal{IVFS}$ and harmonic h-convex $\mathcal{IVFS}$; see Refs. [30,31]. As a step forward, the following authors utilized $(h_1,h_2)$-convex functions as well as harmonical $(h_1,h_2)$-convex functions to develop these inequalities; see Refs. [32,33]. Accordingly, Afzal et al. [34,35] developed the following results based on interval-valued $(h_1,h_2)$-$\mathbf{GL}$ functions using the inclusion relation.

Theorem 1 (See [35]). 

Let $\Psi :[q,r]\to {R_I}^{+}.$ Consider $h_1,h_2:(0,1)\to R^{+}$ and $H\left(\frac{1}{2},\frac{1}{2}\right)=h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)\ne 0.$ If $\Psi \in$ SGHX($(h_1,h_2)$, [q,r],${R_I}^{+})$ and $\Psi \in I{R}_{[q,r]}$, then

$$\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{2}\Psi \left(\frac{2qr}{q+r}\right)\supseteq \frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho \supseteq [\Psi \left(q\right)+\Psi \left(r\right)]{\int }_0^1\frac{de}{H(e,1-e)}.$$

(1)

In addition, a Jensen-type inequality was also developed by using the inclusion relation.

Theorem 2 (See [35]). 

Let $d_i\in R^{+}$, $z_i\in [q,r]$, $\Psi :[q,r]\to {R_I}^{+}$. Consider h is a super multiplicative function such that $h\ne 0$ and $\Psi \in$ SGHX($h_1,h_2$),[q,r],${R_I}^{+}).$ Then, this holds

$$\Psi \left(\frac{1}{\frac{1}{D_k}{\sum }_{i=1}^k{d}_i{z}_i}\right)\supseteq \sum _{i=1}^k\left[\frac{\Psi \left(z_i\right)}{H\left(\frac{d_i}{D_k},\frac{D_{k-1}}{D_k}\right)}\right].$$

(2)

Bhunia and his co-author defined the center–radius order in 2014 using the radius and midpoint of the interval; see Ref. [36]. In 2022, Afzal et al. and Wei Liu and his co-authors developed the following results by using the term of the center–radius-order relation for the cr-h-convex, harmonically cr-h-convex, and cr-h-$\mathbf{GL}$ functions; see Refs. [37,38,39]. Our literature review revealed that the majority of these inequalities were derived from a pseudo-order relation and inclusion relation. The main advantage of the center–radius-order relation pertaining to $\mathbf{GL}$ functions is that the inequality term derived by using these notions is more precise, and the validity of the argument can be justified with interesting examples of illustrated theorems. Due to this, it is essential to understand how a total order relation can be utilized to examine the convexity and inequality. Additional observations show that interval differences between endpoints in examples are much closer than in these old partial order relations.

The significance of this study is that it introduces the notion of harmonical $(h_1,h_2)$-$\mathbf{GL}$ functions connected to a total order relation, which is extremely new in the literature. The article provides a new way to investigate inequalities by incorporating cr-interval-valued functions. Comparatively to the pseudo-order relation, the inclusion relation and the interval of the center–radius order can be calculated by the midpoint and center of the interval, such as $q_c=\frac{\underline{q}+\overline{q}}{2}$ and $q_r=\frac{\overline{q}-\underline{q}}{2}$, respectively, where t = $[\overline{q},\underline{q}]$.

Inspired by Refs. [35,36,37,38,39], in this study, a new class of convexity based on the cr-order is presented, called harmonically cr-$(h_1,h_2)$-$\mathbf{GL}$ functions. With the help of these novel notions, we are in a position to construct new $\mathcal{H}.\mathcal{H}$ inequalities, and eventually, the Jensen inequality is established. A number of examples are included in the study in order to support the conclusions drawn.

Lastly, the article is designed as follows: Some basic background is provided in Section 2. The main findings are described in Section 3, Section 4 and Section 5. Section 6 explores a brief conclusion.

2. Preliminaries

The paper uses some terms without defining them; see Refs. [30,38]. The pack of intervals is denoted by $R_I$ of R, while an interval pack with all positive values would be represented as follows: $R_I^{+}$. For $\varrho \in R$, the scalar multiplication and addition are defined as

$$q+r=[\underline{q},\overline{q}]+[\underline{r},\overline{r}]=[\underline{q}+\underline{r},\overline{q}+\overline{r}]$$

$$\varrho q=\varrho .[\underline{q},\overline{q}]=\left\{\begin{array}{c}[\varrho \underline{q},\varrho \overline{q}],if\varrho >0,\hfill \\ \left\{0\right\},if\varrho =0,\hfill \\ \varrho \overline{q},\varrho \underline{q}],if\varrho <0,\hfill \end{array}\right.$$

respectively. Let $q=[\underline{q},\overline{q}]\in R_I$, $q_c=\frac{\underline{q}+\overline{q}}{2}$ is called center of interval q, and $q_r=\frac{\overline{q}-\underline{q}}{2}$ is said to be radius of interval q. This is the center–radius (cr) form of interval q

$$q=\left(\frac{\underline{q}+\overline{q}}{2},\frac{\overline{q}-\underline{q}}{2}\right)=(t_c,t_r).$$

Definition 1 (See [37]). 

Consider $q=[\underline{q},\overline{q}]=(q_c,q_r)$, $r=[\underline{r},\overline{r}]=(r_c,r_r)\in R_I$, then cr-order relation is defined as

$$q{⪯}_{cr}r\iff \left\{\begin{array}{c}{q}_c<r_c,if{q}_c\ne r_c\hfill \\ \\ q_c\le r_c,if{q}_c=r_c\hfill \end{array}\right.$$

Riemann integrable (in short $IR$) for $\mathcal{IVFS}$ using cr-order can be presented as follows.

Theorem 3 (See [37]). 

Let $\Psi :[q,r]\to R_I$ be $\mathcal{IVF}$ given by $\Psi \left(\varrho \right)=[\underline{\Psi }\left(\varrho \right),\overline{\Psi }\left(\varrho \right)]$ for each $\varrho \in [q,r]$ and $\underline{\Psi },\overline{\Psi }$ are IR over interval $[q,r]$. Then, we would call Ψ as $IR$ over interval $[q,r]$, and

$${\int }_q^r\Psi \left(\varrho \right)d\varrho =\left[{\int }_q^r\underline{\Psi }\left(\varrho \right)d\varrho ,{\int }_q^r\overline{\Psi }\left(\varrho \right)d\varrho \right].$$

Riemann integrables ($IR$) $\mathcal{IVFS}$ over the interval $[q,r]$ can be presented as $I{R}_{[q,r]}$.

Theorem 4 (See [37]). 

Let $\Psi ,\psi :[q,r]\to R_I^{+}$ given by $\Psi =[\underline{\Psi },\overline{\Psi }]$, and $\psi =[\underline{\psi },\overline{\psi }].$ If $\Psi ,\psi \in {IR}_{[q,r]}$, and $\Psi \left(\varrho \right){⪯}_{cr}\psi \left(\varrho \right)$$\forall \varrho \in [q,r],$ then

$${\int }_q^r\Psi \left(\varrho \right)d\varrho {⪯}_{cr}{\int }_q^r\psi \left(\varrho \right)d\varrho .$$

We will now provide an illustration with some interesting examples to support the above theorem.

Example 1. 

Consider $\Psi =[z,2z]$ and $\zeta =[z^2,z^2+2]$, ∀$z\in [0,1]$

$${\psi }_{\mathcal{R}}=1,{\Psi }_{\mathcal{R}}=\frac{z}{2},{\psi }_{\mathcal{C}}=z^2+1\mathrm{and}{\Psi }_{\mathcal{C}}=\frac{3z}{2}.$$

By Definition 1, we have $\Psi \left(z\right){⪯}_{cr}\psi \left(z\right)$, ∀$z\in [0,1]$.

We have,

$${\int }_0^1[z^2,z^2+2]dz=\left[\frac{1}{3},\frac{7}{3}\right]$$

and

$${\int }_0^1[z,2z]dz=\left[\frac{1}{2},1\right]$$

From Theorem 4 (see Figure 1 and Figure 2), we have

$${\int }_0^1\Psi \left(z\right)dz{⪯}_{cr}{\int }_0^1\psi \left(z\right)dz.$$

Definition 2 ([38]). 

Let $h:[0,1]\to R^{+}.$ Thus, we say $\Psi :[q,r]\to R^{+}$ is known harmonically h-convex function, or that $\Psi \in SHX(h,[q,r],R^{+})$, if ∀$q_1,r_1\in [q,r]$ and $\varrho \in [0,1],$ we have

$$\Psi \left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right)\le h\left(\varrho \right)\Psi \left(q_1\right)+h(1-\varrho )\Psi \left(r_1\right).$$

(3)

If in (3) ≤ altered with ≥, it is called harmonically h-concave function or $\Psi \in SHV$$(h,[q,r],R^{+}).$

Definition 3 ([35]). 

Let $h:(0,1)\to R^{+}.$ Thus, we say $\Psi :[q,r]\to R^{+}$ is known as harmonically h-$\mathbf{GL}$ function, or that $\Psi \in SGHX(h,[q,r],R^{+})$, if ∀$q_1,r_1\in [q,r]$ and $\varrho \in (0,1),$ we have

$$\Psi \left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right)\le \frac{\Psi \left(q_1\right)}{h\left(\varrho \right)}+\frac{\Psi \left(r_1\right)}{h(1-\varrho )}.$$

(4)

If in (4) ≤ altered with ≥, it is called harmonically h-$\mathbf{GL}$ concave function or $\Psi \in SGHV$$(h,[q,r],R^{+}).$

Now, let us look at the $\mathcal{IVF}$ concept with respect to cr-h-convexity.

Definition 4 (See [35,39]). 

Consider $h_1,h_2:(0,1)\to R^{+}.$ Thus, $\Psi =[\underline{\Psi },\overline{\Psi }]:[q,r]\to R_I^{+}$ is called harmonically cr-$(h_1,h_2)$-$\mathbf{GL}$ convex function, or that $\Psi \in SGHX(cr$-$(h_1,h_2),[q,r],R_I^{+})$, if ∀$q_1,r_1\in [q,r]$ and $\varrho \in (0,1),$ we have

$$\Psi \left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right){⪯}_{cr}\frac{\Psi \left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{\Psi \left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)}.$$

(5)

If in (5) ${⪯}_{cr}$ altered with ${⪰}_{cr}$, it is called harmonically cr-$(h_1,h_2)$-$\mathbf{GL}$ concave function or $\Psi \in SGHV($cr-h$,[q,r],R_I^{+}).$ The pack of all harmonical cr-$(h_1,h_2)$-$\mathbf{GL}$-convex functions can be represented by $\Psi \in SGHX(cr$-$(h_1,h_2),[q,r],R_I^{+})$.

Remark 1. 

• If $h_1=h_2=1$, Definition 4 incorporates harmonic cr-P-function.
• If $h_1\left(\varrho \right)=\frac{1}{h_1\left(\varrho \right)}$, $h_2=1$, Definition 4 incorporates harmonic cr-h-convex function.
• If $h_1\left(\varrho \right)=h\left(\varrho \right)$, $h_2=1$, Definition 4 incorporates harmonic cr-h-$\mathbf{GL}$ function.
• If $h_1\left(\varrho \right)=\frac{1}{{\varrho }^s}$, $h_2=1$, Definition 4 incorporates harmonic cr-s-convex function.
• If $h_1\left(\varrho \right)={\varrho }^s$, $h_2\left(\varrho \right)=1$, Definition 4 incorporates harmonic cr-s-$\mathbf{GL}$ function.

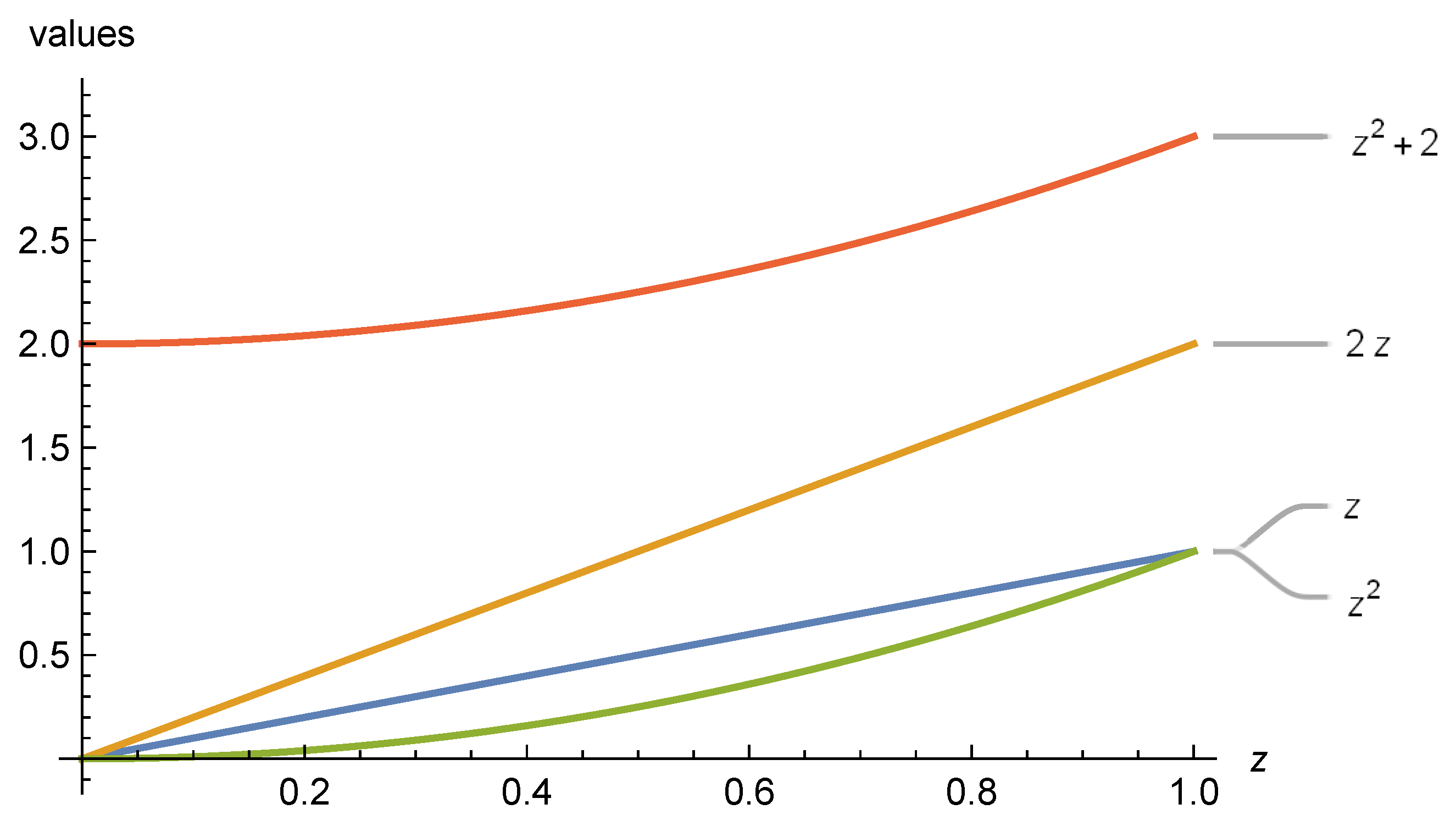
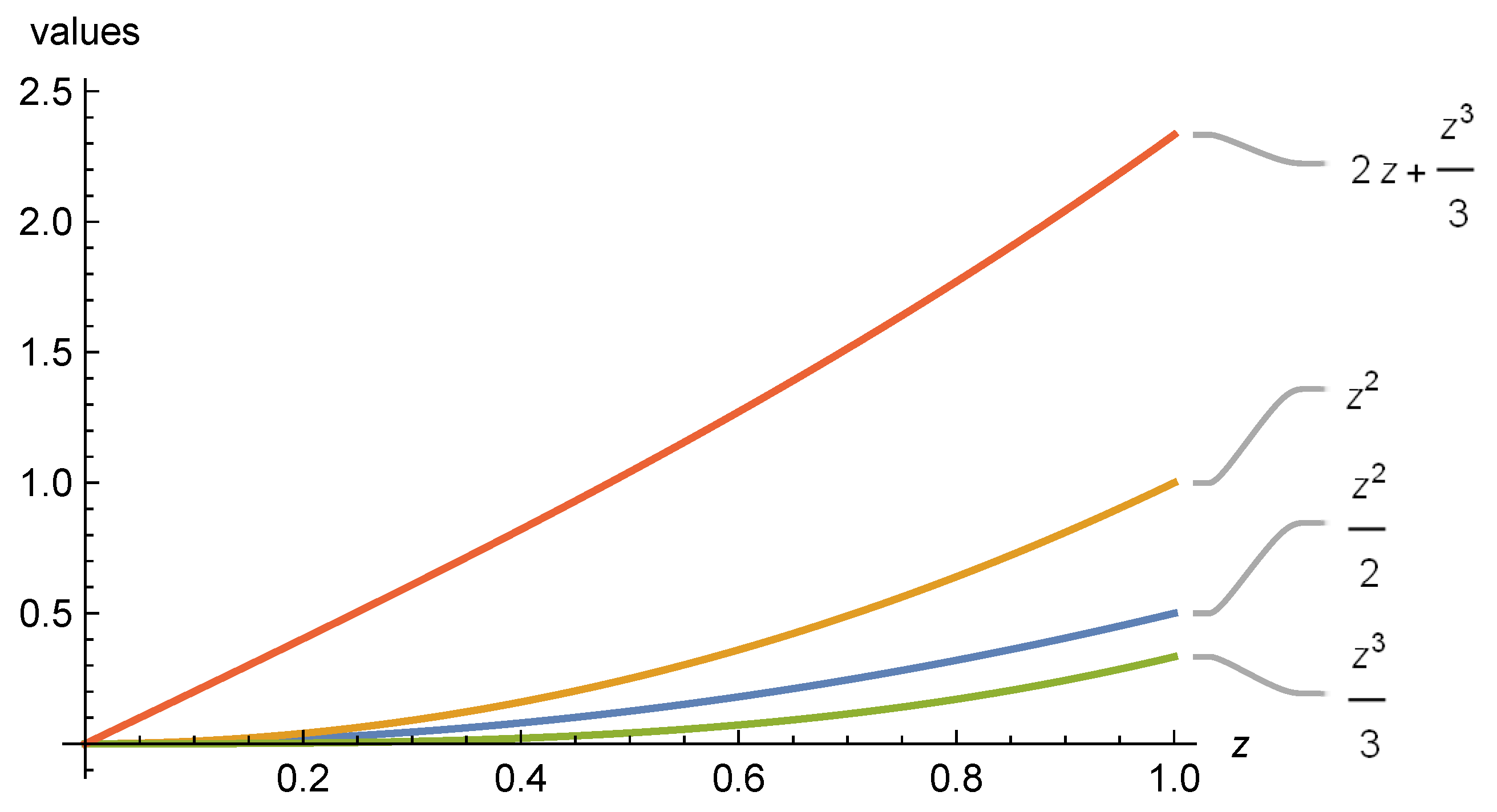
3. Main Results

Proposition 1. 

Let $\Psi :[q,r]\to R_I$ given by $[\underline{\Psi },\overline{\Psi }]=\left({\Psi }_c,{\Psi }_r\right).$ If ${\Psi }_c$ and ${\Psi }_r$ are harmonically $(h_1,h_2)$-$\mathbf{GL}$ over $[q,r]$, then Ψ is said to be harmonically cr-$(h_1,h_2)$-$\mathbf{GL}$ function over $[q,r].$

Proof. 

Because ${\Psi }_c$ and ${\Psi }_r$ are harmonically cr-$(h_1,h_2)$-$\mathbf{GL}$ over $[q,r]$, then for each $\varrho \in (0,1)$ and for all $q_1,r_1\in [q,r]$, we have

$${\Psi }_c\left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right){⪯}_{cr}\frac{{\Psi }_c\left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{{\Psi }_c\left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)},$$

and

$${\Psi }_r\left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right){⪯}_{cr}\frac{{\Psi }_r\left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{{\Psi }_r\left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)},$$

Now, if

$${\Psi }_c\left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right)\ne \frac{{\Psi }_c\left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{{\Psi }_c\left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)},$$

then for each $\varrho \in (0,1)$ and for all $q_1,r_1\in [q,r]$,

$${\Psi }_c\left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right)<\frac{{\Psi }_c\left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{{\Psi }_c\left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)},$$

Accordingly,

$${\Psi }_c\left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right){⪯}_{cr}\frac{{\Psi }_c\left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{{\Psi }_c\left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)}.$$

Apart from that, for each $\varrho \in (0,1)$ and ∀$q_1,r_1\in [q,r]$,

$$\begin{array}{cc}& {\Psi }_r\left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right)\le \frac{{\Psi }_r\left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{{\Psi }_r\left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)}\hfill \\ \hfill & \Rightarrow \Psi \left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right){⪯}_{cr}\frac{\Psi \left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{\Psi \left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)}.\hfill \end{array}$$

Based on the foregoing and Equation (5), this can be stated as follows:

$$\Psi \left(\frac{q_1{r}_1}{\varrho q_1+(1-\varrho )r_1}\right){⪯}_{cr}\frac{\Psi \left(q_1\right)}{h_1\left(\varrho \right)h_2(1-\varrho )}+\frac{\Psi \left(r_1\right)}{h_1(1-\varrho )h_2\left(\varrho \right)}$$

for each $\varrho \in (0,1)$ and for all $q_1,r_1\in [q,r].$

This completes the proof. □

4. Hermite–Hadamard-Type Inequality

The $\mathcal{H}.\mathcal{H}$ inequalities for harmonically cr-$(h_1,h_2)$-$\mathbf{GL}$ functions were developed in this section.

Theorem 5. 

Define $h_1,h_2:(0,1)\to R^{+}$ and $H\left(\frac{1}{2},\frac{1}{2}\right)\ne 0.$ Let $\Psi :[q,r]\to {R_I}^{+}$, if $\Psi \in SGHX(cr$-$(h_1,h_2),[q,r],{R_I}^{+})$ and $\Psi \in I{R}_{[q,r]}$, we have

$$\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{2}\Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho {⪯}_{cr}[\Psi \left(q\right)+\Psi \left(r\right)]{\int }_0^1\frac{de}{H(e,1-e)}.$$

(6)

Proof. 

Because $\Psi \in SGHX(cr$-$(h_1,h_2),[q,r],{R_I}^{+})$, we have

$$\begin{array}{c}\hfill \left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]\Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\Psi \left(\frac{qr}{eq+(1-e)r}\right)+\Psi \left(\frac{qr}{(1-e)q+er}\right).\end{array}$$

With an integration over $(0,1)$, we have

$$\begin{array}{cc}& \left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]\Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\left[{\int }_0^1\Psi \left(\frac{qr}{eq+(1-e)r}\right)de+{\int }_0^1\Psi \left(\frac{qr}{(1-e)q+er}\right)de\right]\hfill \\ \hfill & =\left[{\int }_0^1\underline{\Psi }\left(\frac{qr}{eq+(1-e)r}\right)de+{\int }_0^1\underline{\Psi }\left(\frac{qr}{(1-e)q+er}\right)de,\right.\hfill \\ \hfill & \left.{\int }_0^1\overline{\Psi }\left(\frac{qr}{eq+(1-e)r}\right)de+{\int }_0^1\overline{\Psi }\left(\frac{qr}{(1-e)q+er}\right)de\right]\hfill \\ \hfill & =\left[\frac{2qr}{r-q}{\int }_q^r\frac{\underline{\Psi }\left(\varrho \right)}{{\varrho }^2}d\varrho ,\frac{2qr}{r-q}{\int }_q^r\frac{\overline{\Psi }\left(\varrho \right)}{{\varrho }^2}d\varrho \right]\hfill \\ \hfill & =\frac{2qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho .\hfill \end{array}$$

(7)

From Definition 4, we have

$$\Psi \left(\frac{qr}{eq+(1-e)r}\right){⪯}_{cr}\frac{\Psi \left(q\right)}{h_1\left(e\right)h_2(1-e)}+\frac{\Psi \left(r\right)}{h_1(1-e)h_2\left(e\right)}$$

With an integration over (0,1), we have

$${\int }_0^1\Psi \left(\frac{qr}{eq+(1-e)r}\right)de{⪯}_{cr}\Psi \left(q\right){\int }_0^1\frac{de}{h_1\left(e\right)h_2(1-e)}+\Psi \left(r\right){\int }_0^1\frac{de}{h_1(1-e)h_2\left(e\right)}$$

Because at $e=\frac{1}{2}$, $h_1\left(e\right)h_2(1-e)=h_1(1-e)h_2\left(e\right)=H(e,1-e)$, we have

$$\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho {⪯}_{cr}\left[\Psi \left(q\right)+\Psi \left(r\right)\right]{\int }_0^1\frac{de}{H(e,1-e)}$$

(8)

Adding (7) and (8), the results are obtained as expected:

$$\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{2}\Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho {⪯}_{cr}[\Psi \left(q\right)+\Psi \left(r\right)]{\int }_0^1\frac{de}{H(e,1-e)}.$$

□

Remark 2. 

• If $h_1\left(e\right)=h_2\left(e\right)=1$, Theorem 5 becomes the result for harmonically cr-P-function:

  $$\frac{1}{2}\Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho {⪯}_{cr}[\Psi \left(q\right)+\Psi \left(r\right)].$$
• If $h_1\left(e\right)=\frac{1}{e}$, $h_2\left(e\right)$, Theorem 5 becomes the result for harmonically cr-convex function:

  $$\Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho {⪯}_{cr}\frac{[\Psi (q)+\Psi (r\left)\right]}{2}.$$
• If $h_1\left(e\right)=\frac{1}{{\left(e\right)}^s}$, $h_2\left(e\right)=1$, Theorem 5 becomes the result for harmonically cr-s-convex function:

  $$2^{s-1}\Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho {⪯}_{cr}\frac{[\Psi (q)+\Psi (r\left)\right]}{s+1}.$$

Example 2. 

Let $[q,r]=[1,2]$, $h_1\left(e\right)=\frac{1}{e}$, $h_2\left(e\right)=\frac{1}{4}$, ∀$e\in (0,1).$$\Psi :[q,r]\to {R_I}^{+}$ is defined as

$$\Psi \left(\varrho \right)=\left[{\varrho }^2,2{\varrho }^2+1\right]$$

where

$$\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{2}\Psi \left(\frac{2qr}{q+r}\right)=\frac{1}{4}\Psi \left(\frac{4}{3}\right)=\left[\frac{16}{36},\frac{41}{36}\right],$$

$$\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho =2\left[{\int }_1^2{\varrho }^{2}d\varrho ,{\int }_1^2(2{\varrho }^2+1)d\varrho \right]=\left[\frac{14}{3},\frac{34}{3}\right],$$

$$\left[\Psi \left(q\right)+\Psi \left(r\right)\right]{\int }_0^1\frac{de}{H(e,1-e)}=\left[10,24\right].$$

As a result,

$$\left[\frac{16}{36},\frac{41}{36}\right]{⪯}_{cr}\left[\frac{14}{3},\frac{34}{3}\right]{⪯}_{cr}\left[10,24\right].$$

The above theorem is therefore proved.

Example 3. 

Let $[q,r]=[1,2]$, $h_1\left(e\right)=\frac{1}{e}$, $h_2\left(e\right)=1$, ∀$e\in (0,1).$$\Psi :[q,r]\to {R_I}^{+}$ is defined as

$$\Psi \left(\varrho \right)=\left[\frac{-1}{{\varrho }^4}+3,\frac{1}{{\varrho }^4}+4\right]$$

where

$$\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{2}\Psi \left(\frac{2qr}{q+r}\right)=\Psi \left(\frac{4}{3}\right)=\left[\frac{687}{256},\frac{1105}{256}\right],$$

$$\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho =2\left[{\int }_1^2\left(\frac{3{\varrho }^4-1}{{\varrho }^6}\right)d\varrho ,{\int }_1^2\left(\frac{4{\varrho }^4+1}{{\varrho }^6}\right)d\varrho \right]=\left[\frac{418}{160},\frac{702}{160}\right],$$

$$\left[\Psi \left(q\right)+\Psi \left(r\right)\right]{\int }_0^1\frac{de}{H(e,1-e)}=\left[\frac{79}{32},\frac{145}{32}\right].$$

As a result,

$$\left[\frac{687}{256},\frac{1105}{256}\right]{⪯}_{cr}\left[\frac{418}{160},\frac{702}{160}\right]{⪯}_{cr}\left[\frac{79}{32},\frac{145}{32}\right].$$

The above theorem is therefore proved.

Theorem 6. 

Define $h_1,h_2:(0,1)\to R^{+}$ and $\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]\ne 0.$ Let $\Psi :[q,r]\to {R_I}^{+}$, if $\Psi \in SGHX($cr-h$,[q,r],{R_I}^{+})$ and $\Psi \in I{R}_{[q,r]}$, we have

$$\frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{4}\Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}{△}_1{⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho {⪯}_{cr}{△}_2$$

$${⪯}_{cr}\left\{\left[\Psi \left(q\right)+\Psi \left(r\right)\right]\left[\frac{1}{2}+\frac{1}{H\left(\frac{1}{2},\frac{1}{2}\right)}\right]\right\}{\int }_0^1\frac{de}{H(e,1-e)},$$

where

$${△}_1=\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{4}\left[\Psi \left(\frac{4qr}{3q+r}\right)+\Psi \left(\frac{4qr}{3r+q}\right)\right],$$

$${△}_2=\left[\Psi \left(\frac{2qr}{q+r}\right)+\frac{\Psi \left(q\right)+\Psi \left(r\right)}{2}\right]{\int }_0^1\frac{de}{H(e,1-e)}.$$

Proof. 

Consider $\left[q,\frac{q+r}{2}\right]$, we have

$$\Psi \left(\frac{4qr}{q+3r}\right){⪯}_{cr}\frac{\Psi \left(\frac{q\frac{2qr}{q+r}}{eq+(1-e)\frac{2qr}{q+r}}\right)}{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}+\frac{\Psi \left(\frac{q\frac{2qr}{q+r}}{(1-e)q+e\frac{2qr}{q+r}}\right)}{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}$$

With an integration over $(0,1)$, we have

$$\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{4}\Psi \left(\frac{4qr}{r+3q}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_q^{\frac{2qr}{q+r}}\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho .$$

(9)

Similarly for interval $\left[\frac{q+r}{2},r\right]$, we have

$$\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{4}\Psi \left(\frac{4qr}{q+3r}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_{\frac{2qr}{q+r}}^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho .$$

(10)

Adding (9) and (10), the results are obtained as expected

$${△}_1=\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{4}\left[\Psi \left(\frac{4qr}{r+3q}\right)+\Psi \left(\frac{4qr}{q+3r}\right)\right]{⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho .$$

Now,

$$\begin{array}{cc}& \frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{4}\Psi \left(\frac{2qr}{q+r}\right)\hfill \\ \hfill & =\frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{4}\Psi \left(\frac{1}{2}\left(\frac{4qr}{3r+q}\right)+\frac{1}{2}\left(\frac{4qr}{3q+r}\right)\right)\hfill \\ \hfill & {⪯}_{cr}\frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{4}\left[\frac{\Psi \left(\frac{4qr}{r+3q}\right)}{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}+\frac{\Psi \left(\frac{4qr}{3r+q}\right)}{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}\right]\hfill \\ \hfill & =\frac{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}{4}\left[\Psi \left(\frac{4qr}{r+3q}\right)+\Psi \left(\frac{4qr}{3r+q}\right)\right]\hfill \\ \hfill & ={△}_1\hfill \\ \hfill & {⪯}_{cr}\frac{qr}{r-q}{\int }_u^t\frac{\Psi \left(\varrho \right)}{{\varrho }^2}d\varrho \hfill \\ \hfill & {⪯}_{cr}\frac{1}{2}\left[\Psi \left(q\right)+\Psi \left(r\right)+2\Psi \left(\frac{2qr}{q+r}\right)\right]{\int }_0^1\frac{de}{H(e,1-e)}\hfill \\ \hfill & ={△}_2\hfill \\ \hfill & {⪯}_{cr}\left[\frac{\Psi \left(q\right)+\Psi \left(r\right)}{2}+\frac{\Psi \left(q\right)}{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}+\frac{\Psi \left(r\right)}{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}\right]{\int }_0^1\frac{de}{H(e,1-e)}\hfill \\ \hfill & {⪯}_{cr}\left[\frac{\Psi \left(q\right)+\Psi \left(r\right)}{2}+\frac{1}{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}\left[\Psi \left(q\right)+\Psi \left(r\right)\right]\right]{\int }_0^1\frac{de}{H(e,1-e)}\hfill \\ \hfill & {⪯}_{cr}\left\{\left[\Psi \left(q\right)+\Psi \left(r\right)\right]\left[\frac{1}{2}+\frac{1}{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}\right]\right\}{\int }_0^1\frac{de}{H(e,1-e)}.\hfill \end{array}$$

□

Example 4. 

Thanks to Example 3, we have

$$\begin{array}{cc}\hfill \frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{4}\Psi \left(\frac{2qr}{q+r}\right)& =\Psi \left(\frac{4}{3}\right)=\left[\frac{687}{256},\frac{1105}{256}\right],\hfill \\ \hfill {△}_1& =\frac{1}{2}\left[\Psi \left(\frac{8}{5}\right)+\Psi \left(\frac{8}{7}\right)\right]=\left[\frac{10775}{4096},\frac{17897}{4096}\right],\hfill \\ \hfill {△}_2& =\left[\frac{\Psi \left(1\right)+\Psi \left(2\right)}{2}+\Psi \left(\frac{4}{3}\right)\right]{\int }_0^1\frac{de}{H(e,1-e)},\hfill \\ \hfill & =\left[\frac{1319}{512},\frac{2265}{512}\right].\hfill \end{array}$$

and

$$\left\{\left[\Psi \left(q\right)+\Psi \left(r\right)\right]\left[\frac{1}{2}+\frac{1}{H\left(\frac{1}{2},\frac{1}{2}\right)}\right]\right\}{\int }_0^1\frac{de}{H(e,1-e)}=\left[\frac{79}{32},\frac{145}{32}\right].$$

Thus, we obtain

$$\left[\frac{687}{256},\frac{1105}{256}\right]{⪯}_{cr}\left[\frac{10775}{4096},\frac{17897}{4096}\right]{⪯}_{cr}\left[\frac{418}{160},\frac{702}{160}\right]{⪯}_{cr}\left[\frac{1319}{512},\frac{2265}{512}\right]{⪯}_{cr}\left[\frac{79}{32},\frac{145}{32}\right].$$

As a consequence, the theorem above is proved.

Theorem 7. 

Let $\Psi ,\psi :[q,r]\to {R_I}^{+},h_1,h_2:(0,1)\to R^{+}$ where $h_1,h_2\ne 0$. If $\Psi \in SGHX(cr$ -$h_1,[q,r],{R_I}^{+})$, $\psi \in SGHX(cr$-$h_2,[q,r],{R_I}^{+})$ and $\Psi ,\psi \in I{R}_{[v,w]}$, then we have

$$\begin{array}{cc}\hfill \frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)\psi \left(\varrho \right)}{{\varrho }^2}d\varrho & {⪯}_{cr}M(q,r){\int }_0^1\frac{1}{H^2(e,1-e)}de\hfill \\ \hfill & +N(q,r){\int }_0^1\frac{1}{H(e,e)H(1-e,1-e)}de.\hfill \end{array}$$

where

$$M(q,r)=\Psi \left(q\right)\psi \left(q\right)+\Psi \left(r\right)\psi \left(r\right),N(q,r)=\Psi \left(q\right)\psi \left(r\right)+\Psi \left(r\right)\psi \left(q\right).$$

Proof. 

Consider $\Psi \in SGHX(cr$-$h_1,[q,r],{R_I}^{+})$, $\psi \in SGHX(cr$-$h_2,[q,r],{R_I}^{+})$, then we have

$$\Psi \left(\frac{qr}{qe+(1-e)r}\right){⪯}_{cr}\frac{\Psi \left(q\right)}{h_1\left(e\right)h_2(1-e)}+\frac{\Psi \left(r\right)}{h_1(1-e)h_2\left(e\right)},$$

$$\psi \left(\frac{qr}{qe+(1-e)r}\right){⪯}_{cr}\frac{\psi \left(q\right)}{h_1\left(e\right)h_2(1-e)}+\frac{\psi \left(r\right)}{h_1(1-e)h_2\left(e\right)}.$$

Then,

$$\begin{array}{cc}& \Psi \left(\frac{qr}{qe+(1-e)r}\right)\psi \left(\frac{qr}{qe+(1-e)r}\right)\hfill \\ \hfill & {⪯}_{cr}\frac{\Psi \left(q\right)\psi \left(q\right)}{H^2(e,1-e)}+\frac{\Psi \left(r\right)\psi \left(r\right)}{H^2(1-e,e)}+\frac{\Psi \left(q\right)\psi \left(r\right)+\Psi \left(r\right)\psi \left(q\right)}{H(e,e)H(1-e,1-e)}.\hfill \end{array}$$

(11)

With an integration over (0,1), we have

$$\begin{array}{cc}& {\int }_0^1\Psi \left(\frac{qr}{qe+(1-e)r}\right)\psi \left(\frac{qr}{qe+(1-e)r}\right)de\hfill \\ \hfill & =\left[{\int }_0^1\underline{\Psi }\left(\frac{qr}{qe+(1-e)r}\right)\underline{\psi }\left(\frac{qr}{qe+(1-e)r}\right)de,\right.\hfill \\ \hfill & \left.{\int }_0^1\overline{\Psi }\left(\frac{qr}{qe+(1-e)r}\right)\overline{\psi }\left(\frac{qr}{qe+(1-e)r}\right)de\right]\hfill \\ \hfill & =\left[\frac{qr}{r-q}{\int }_q^r\frac{\underline{\Psi }\left(\varrho \right)\underline{\psi }\left(\varrho \right)}{{\varrho }^2}d\varrho ,\frac{qr}{r-q}{\int }_q^r\frac{\overline{\Psi }\left(\varrho \right)\overline{\psi }\left(\varrho \right)}{{\varrho }^2}d\varrho \right]=\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)\psi \left(\varrho \right)}{{\varrho }^2}d\varrho \hfill \\ \hfill & {⪯}_{cr}{\int }_0^1\frac{\left[\Psi \left(q\right)\psi \left(q\right)+\Psi \left(r\right)\psi \left(r\right)\right]}{H^2(e,1-e)}de+{\int }_0^1\frac{\left[\Psi \left(q\right)\psi \left(r\right)+\Psi \left(r\right)\psi \left(q\right)\right]}{H(e,e)H(1-e,1-e)}de\hfill \end{array}$$

It follows that

$$\begin{array}{cc}\hfill \frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)\psi \left(\varrho \right)}{{\varrho }^2}d\varrho & {⪯}_{cr}M(q,r){\int }_0^1\frac{1}{H^2(e,1-e)}de\hfill \\ \hfill & +N(q,r){\int }_0^1\frac{1}{H(e,e)H(1-e,1-e)}de.\hfill \end{array}$$

The theorem is proved. □

Example 5. 

Let $[q,r]=[1,2]$, $h_1\left(e\right)=\frac{1}{e}$, $h_2\left(e\right)=1$, ∀$e\in (0,1)$. $\Psi ,\psi :[q,r]\to {R_I}^{+}$ be defined as

$$\Psi \left(\varrho \right)=\left[\frac{-1}{{\varrho }^4}+2,\frac{1}{{\varrho }^4}+3\right],\psi \left(\varrho \right)=\left[\frac{-1}{\varrho }+1,\frac{1}{\varrho }+2\right].$$

Then, we have

$$\begin{array}{cc}\hfill \frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)\psi \left(\varrho \right)}{{\varrho }^2}d\varrho & =\left[\frac{282}{640},\frac{5986}{640}\right],\hfill \\ \hfill M(q,r){\int }_0^1\frac{1}{H^2(e,1-e)}de& =M(1,2){\int }_0^1{e}^{2}de=\left[\frac{31}{96},\frac{629}{96}\right],\hfill \end{array}$$

and

$$N(q,r){\int }_0^1\frac{1}{H(e,e)H(1-e,1-e)}de=N(1,2){\int }_0^1(e-e^2)de=\left[\frac{1}{12},\frac{307}{96}\right].$$

It follows that

$$\left[\frac{282}{640},\frac{5986}{640}\right]{⪯}_{cr}\left[\frac{31}{96},\frac{629}{96}\right]+\left[\frac{1}{12},\frac{307}{96}\right]=\left[\frac{13}{32},\frac{39}{4}\right].$$

This proves the above theorem.

Theorem 8. 

Define $\Psi ,\psi :[q,r]\to {R_I}^{+},h_1,h_2:(0,1)\to R^{+}$ where $h_1,h_2\ne 0$. If $\Psi \in SGHX(cr$-$h_1,[q,r],{R_I}^{+})$, $\psi \in SGHX(cr$-$h_2,[q,r],{R_I}^{+})$ and $\Psi ,\psi \in I{R}_{[v,w]}$, then we have

$$\begin{array}{cc}& \frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{2}\Psi \left(\frac{2qr}{q+r}\right)\psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)\psi \left(\varrho \right)}{{\varrho }^2}d\varrho \hfill \\ \hfill & +M(q,r){\int }_0^1\frac{de}{H(e,e)H(1-e,1-e)}+N(q,r){\int }_0^1\frac{de}{H^2(e,1-e)}.\hfill \end{array}$$

Proof. 

Because $\Psi \in SGHX(cr$-$h_1,[q,r],{R_I}^{+})$, $\psi \in SGHX(cr$-$h_2,[q,r],{R_I}^{+})$, we have

$$\begin{array}{cc}& \Psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{\Psi \left(\frac{qr}{qe+(1-e)r}\right)}{H\left(\frac{1}{2},\frac{1}{2}\right)}+\frac{\Psi \left(\frac{qr}{q(1-e)+er}\right)}{H\left(\frac{1}{2},\frac{1}{2}\right)},\hfill \\ \hfill & \psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{\psi \left(\frac{qr}{qe+(1-e)r}\right)}{H\left(\frac{1}{2},\frac{1}{2}\right)}+\frac{\psi \left(\frac{qr}{q(1-e)+er}\right)}{H\left(\frac{1}{2},\frac{1}{2}\right)}.\hfill \end{array}$$

Then,

$$\begin{array}{cc}\hfill & \Psi \left(\frac{2qr}{q+r}\right)\psi \left(\frac{2qr}{q+r}\right)\hfill \\ \hfill & {⪯}_{cr}\frac{1}{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}\left[\Psi \left(\frac{qr}{qe+(1-e)r}\right)\psi \left(\frac{qr}{qe+(1-e)r}\right)+\Psi \left(\frac{qr}{q(1-e)+er}\right)\psi \left(\frac{qr}{q(1-e)+er}\right)\right]\hfill \\ \hfill & +\frac{1}{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}\left[\Psi \left(\frac{qr}{qe+(1-e)r}\right)\psi \left(\frac{qr}{q(1-e)+er}\right)+\Psi \left(\frac{qr}{q(1-e)+er}\right)\psi \left(\frac{qr}{qe+(1-e)r}\right)\right]\hfill \\ \hfill & {⪯}_{cr}\frac{1}{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}\left[\Psi \left(\frac{qr}{qe+(1-e)r}\right)\psi \left(\frac{qr}{qe+(1-e)r}\right)+\Psi \left(\frac{qr}{q(1-e)+er}\right)\psi \left(\frac{qr}{q(1-e)+er}\right)\right]\hfill \\ \hfill & +\frac{1}{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}\left[\left(\frac{\Psi \left(q\right)}{H(e,1-e)}+\frac{\Psi \left(r\right)}{H(1-e,e)}\right)\left(\frac{\psi \left(q\right)}{H(1-e,e)}+\frac{\psi \left(r\right)}{H(e,1-e)}\right)\right.\hfill \\ \hfill & +\left.\left(\frac{\Psi \left(q\right)}{H(1-e,e)}+\frac{\Psi \left(r\right)}{H(e,1-e)}\right)\left(\frac{\psi \left(q\right)}{H(e,1-e)}+\frac{\psi \left(r\right)}{H(1-e,e)}\right)\right]\hfill \\ \hfill & {⪯}_{cr}\frac{1}{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}\left[\Psi \left(\frac{qr}{qe+(1-e)r}\right)\psi \left(\frac{qr}{qe+(1-e)r}\right)+\Psi \left(\frac{qr}{q(1-e)+er}\right)\psi \left(\frac{qr}{q(1-e)+er}\right)\right]\hfill \\ \hfill & +\frac{1}{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}\left[\left(\frac{2}{H(e,e)H(1-e,1-e)}\right)M(q,r)+\left(\frac{1}{H^2(e,1-e)}+\frac{1}{H^2(1-e,e)}\right)N(q,r)\right].\hfill \end{array}$$

With an integration over $(0,1)$, we have

$$\begin{array}{cc}\hfill {\int }_0^1\Psi \left(\frac{2qr}{q+r}\right)\psi \left(\frac{2qr}{q+r}\right)de& =\left[{\int }_0^1\underline{\Psi }\left(\frac{2qr}{q+r}\right)\underline{\psi }\left(\frac{2qr}{q+r}\right)de,{\int }_0^1\overline{\Psi }\left(\frac{2qr}{q+r}\right)\overline{\psi }\left(\frac{2qr}{q+r}\right)de\right]\hfill \\ \hfill & =\Psi \left(\frac{2qr}{q+r}\right)\psi \left(\frac{2qr}{q+r}\right)de\hfill \\ \hfill & {⪯}_{cr}\frac{2}{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}\left[\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)\psi \left(\varrho \right)}{{\varrho }^2}d\varrho \right]\hfill \\ \hfill & +\frac{2}{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}\left[M(q,r){\int }_0^1\frac{de}{H(e,e)H(1-e,1-e)}\right.\hfill \\ \hfill & \left.+N(q,r){\int }_0^1\frac{de}{H^2(e,1-e)}\right].\hfill \end{array}$$

Multiply by $\frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{2}$ the above equation, and we obtain the desired result

$$\begin{array}{cc}& \frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{2}\Psi \left(\frac{2qr}{q+r}\right)\psi \left(\frac{2qr}{q+r}\right){⪯}_{cr}\frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)\psi \left(\varrho \right)}{{\varrho }^2}d\varrho \hfill \\ \hfill & +M(q,r){\int }_0^1\frac{de}{H(e,e)H(1-e,1-e)}+N(q,r){\int }_0^1\frac{de}{H^2(e,1-e)}.\hfill \end{array}$$

□

Example 6. 

Let $[q,r]=[1,2]$, $h_1\left(e\right)=\frac{1}{e}$, $h_2\left(e\right)=1$, ∀$x\in (0,1)$. $\Psi ,\psi :[q,r]\to {R_I}^{+}$ be defined as

$$\Psi \left(\varrho \right)=\left[\frac{-1}{{\varrho }^4}+2,\frac{1}{{\varrho }^4}+3\right],\psi \left(\varrho \right)=\left[\frac{-1}{\varrho }+1,\frac{1}{\varrho }+2\right].$$

Then,

$$\begin{array}{cc}\hfill \frac{{\left[H\left(\frac{1}{2},\frac{1}{2}\right)\right]}^2}{2}\Psi \left(\frac{2qr}{q+r}\right)\psi \left(\frac{2qr}{q+r}\right)& =2\Psi \left(\frac{4}{3}\right)\psi \left(\frac{4}{3}\right)=\left[\frac{431}{512},\frac{9339}{512}\right],\hfill \\ \hfill \frac{qr}{r-q}{\int }_q^r\frac{\Psi \left(\varrho \right)\psi \left(\varrho \right)}{{\varrho }^2}d\varrho & =\left[\frac{282}{640},\frac{5986}{640}\right],\hfill \\ \hfill M(q,r){\int }_0^1\frac{de}{H(e,e)H(1-e,1-e)}& =M(1,2){\int }_0^1(e-e^2)de=\left[\frac{31}{192},\frac{629}{192}\right]\hfill \end{array}$$

and

$$N(q,r){\int }_0^1\frac{de}{H^2(e,1-e)}=N(1,2){\int }_0^1{e}^{2}de=\left[\frac{1}{6},\frac{307}{48}\right].$$

It follows that

$$\left[\frac{431}{512},\frac{9339}{512}\right]{⪯}_{cr}\left[\frac{282}{640},\frac{5986}{640}\right]+\left[\frac{31}{192},\frac{629}{192}\right]+\left[\frac{1}{6},\frac{307}{48}\right]=\left[\frac{123}{160},\frac{761}{40}\right].$$

This proves the above theorem.

5. Jensen-Type Inequality

Theorem 9. 

Let $d_i\in {\mathcal{R}}^{+}$, $z_i\in [q,r]$. If $h_1,h_2$ functions that are both non-negative and supermultiplicative and if $\Psi \in SGHX(cr$-$(h_1,h_2),[q,r],{{\mathcal{R}}_{\mathcal{I}}}^{+})$, then the inequality becomes:

$$\Psi \left(\frac{1}{\frac{1}{D_k}{\sum }_{i=1}^k{d}_i{z}_i}\right){⪯}_{cr}\sum _{i=1}^k\left[\frac{\Psi \left(z_i\right)}{H\left(\frac{d_i}{D_k},\frac{D_{k-1}}{D_k}\right)}\right],$$

(12)

where $D_k={\sum }_{i=1}^k{d}_i$

Proof. 

When $k=2$, then (12) holds. Suppose that (12) is also valid for $k-1$, then

$$\begin{array}{cc}& \Psi \left(\frac{1}{\frac{1}{D_k}{\sum }_{i=1}^k{d}_i{z}_i}\right)=\Psi \left(\frac{1}{\frac{d_k}{D_k}{v}_k+{\sum }_{i=1}^{k-1}\frac{d_i}{D_k}{z}_i}\right)\hfill \\ \hfill & {⪯}_{cr}\frac{\Psi \left(j_k\right)}{h_1\left(\frac{d_k}{D_k}\right)h_2\left(\frac{D_{k-1}}{D_k}\right)}+\frac{\Psi \left({\sum }_{i=1}^{k-1}\frac{d_i}{D_k}{z}_i\right)}{h_1\left(\frac{D_{k-1}}{D_k}\right)h_2\left(\frac{d_k}{D_k}\right)}\hfill \\ \hfill & {⪯}_{cr}\frac{\Psi \left(j_k\right)}{h_1\left(\frac{d_k}{D_k}\right)h_2\left(\frac{D_{k-1}}{D_k}\right)}+\frac{1}{h_1\left(\frac{D_{k-1}}{D_k}\right)h_2\left(\frac{d_k}{D_k}\right)}\sum _{i=1}^{k-1}\left[\frac{\Psi \left(z_i\right)}{H\left(\frac{d_i}{D_k},\frac{D_{k-2}}{D_{k-1}}\right)}\right]\hfill \\ \hfill & {⪯}_{cr}\frac{\Psi \left(j_k\right)}{h_1\left(\frac{d_k}{D_k}\right)h_2\left(\frac{D_{k-1}}{D_k}\right)}+\sum _{i=1}^{k-1}\left[\frac{\Psi \left(z_i\right)}{H\left(\frac{d_i}{D_k},\frac{D_{k-2}}{U_{k-1}}\right)}\right]\hfill \\ \hfill & {⪯}_{cr}\sum _{i=1}^k\left[\frac{\Psi \left(z_i\right)}{H\left(\frac{d_i}{D_k},\frac{D_{k-1}}{D_k}\right)}\right].\hfill \end{array}$$

It follows from mathematical induction that the conclusion is correct. □

Remark 3. 

• If $h_1\left(e\right)=1$, $h_2\left(e\right)=1$ in this case, Theorem 9 incorporates output for harmonically cr- P-function:

  $$\Psi \left(\frac{1}{\frac{1}{D_k}{\sum }_{i=1}^k{d}_i{z}_i}\right){⪯}_{cr}\sum _{i=1}^k\Psi \left(z_i\right).$$
• If $h_1\left(e\right)=\frac{1}{e}$, $h_2=1$ in this case, Theorem 9 incorporates output for harmonically cr-convex function:

  $$\Psi \left(\frac{1}{\frac{1}{D_k}{\sum }_{i=1}^k{d}_i{z}_i}\right){⪯}_{cr}\sum _{i=1}^k\frac{d_i}{D_k}\Psi \left(z_i\right).$$
• If $h_1\left(e\right)=\frac{1}{{\left(e\right)}^s}$, $h_2\left(e\right)=1$ in this case, Theorem 9 incorporates output for harmonical cr-s-convex function:

  $$\Psi \left(\frac{1}{\frac{1}{D_k}{\sum }_{i=1}^k{d}_i{z}_i}\right){⪯}_{cr}\sum _{i=1}^k{\left(\frac{d_i}{D_k}\right)}^s\Psi \left(z_i\right).$$

6. Conclusions

In this study, we present a harmonically cr-$(h_1,h_2)$-$\mathbf{GL}$ concept for $\mathcal{IVFS}$. This concept was used to develop the $\mathcal{H}.\mathcal{H}$ and Jensen-type inequalities using a cr-order relation. This study generalizes some recent results developed by Afzal et al. [35,39] and the following authors, Refs. [37,38]. Furthermore, for the sake of checking the validity of our main findings, some nontrivial examples are given. It is interesting to investigate how equivalent inequalities are determined for different types of convexity and by using different integral operators in the future. Due to the extensive use of integral operators in engineering technology, such as different types of mathematical modeling, and the fact that various integral operators are suitable for different types of practical problems, our study of interval integral operator-type integral inequalities will broaden their practical applications. This concept influences the development of a new direction in convex optimization theory. The concept will likely be beneficial for other researchers working across a variety of scientific fields.