Generalized Moment Method for Smoluchowski Coagulation Equation and Mass Conservation Property
Abstract
:1. Introduction
2. Flux Form of Smoluchowski Coagulation Equation
- (a1)
- (a2)
- (a3)
- (b1)
- (b2)
- (b3)
- (b4)
- (b5)
- (1) holds for .
3. Generalized Moment Method
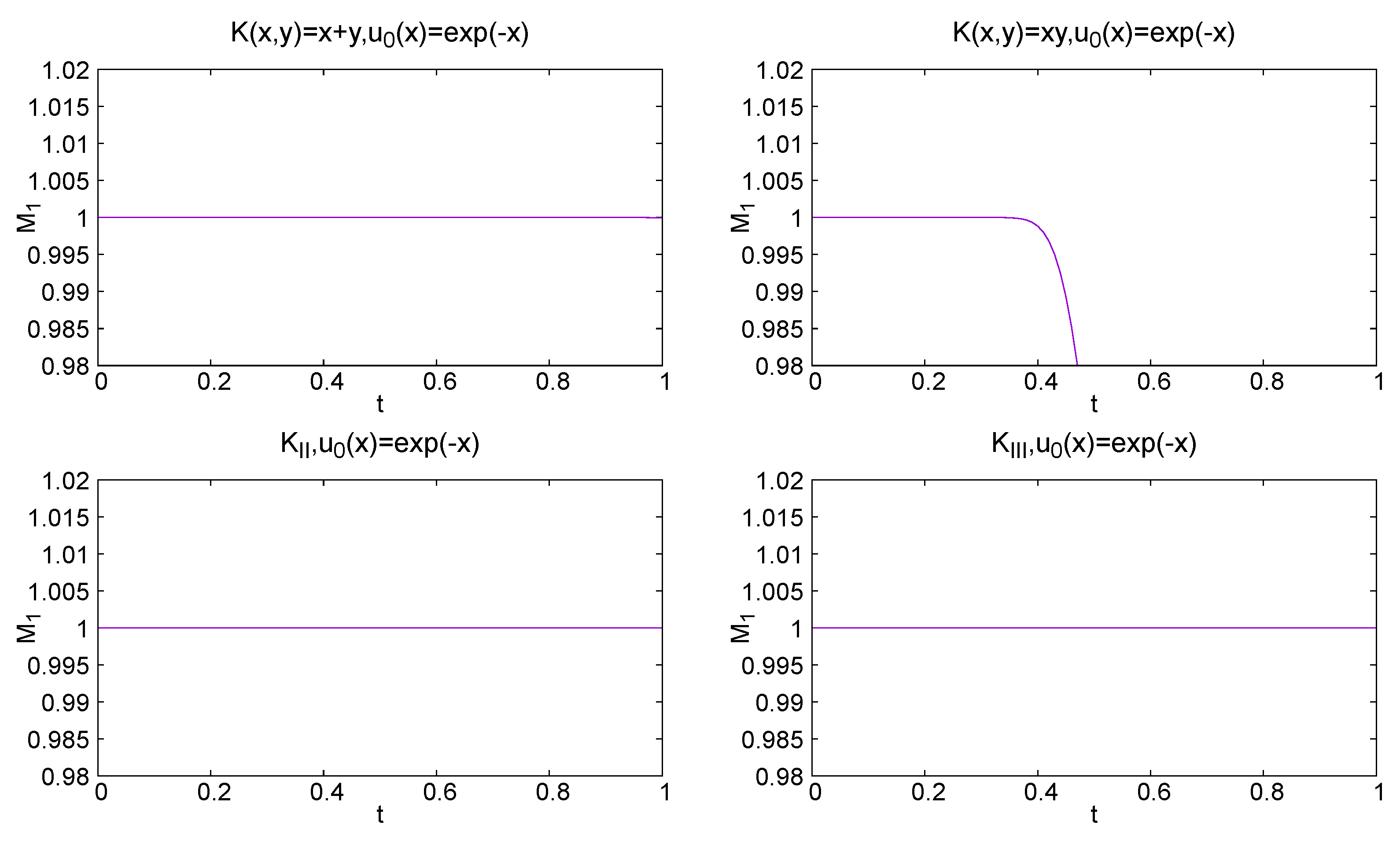
4. Mass Conservation Theorem
- (c1)
- (c2)
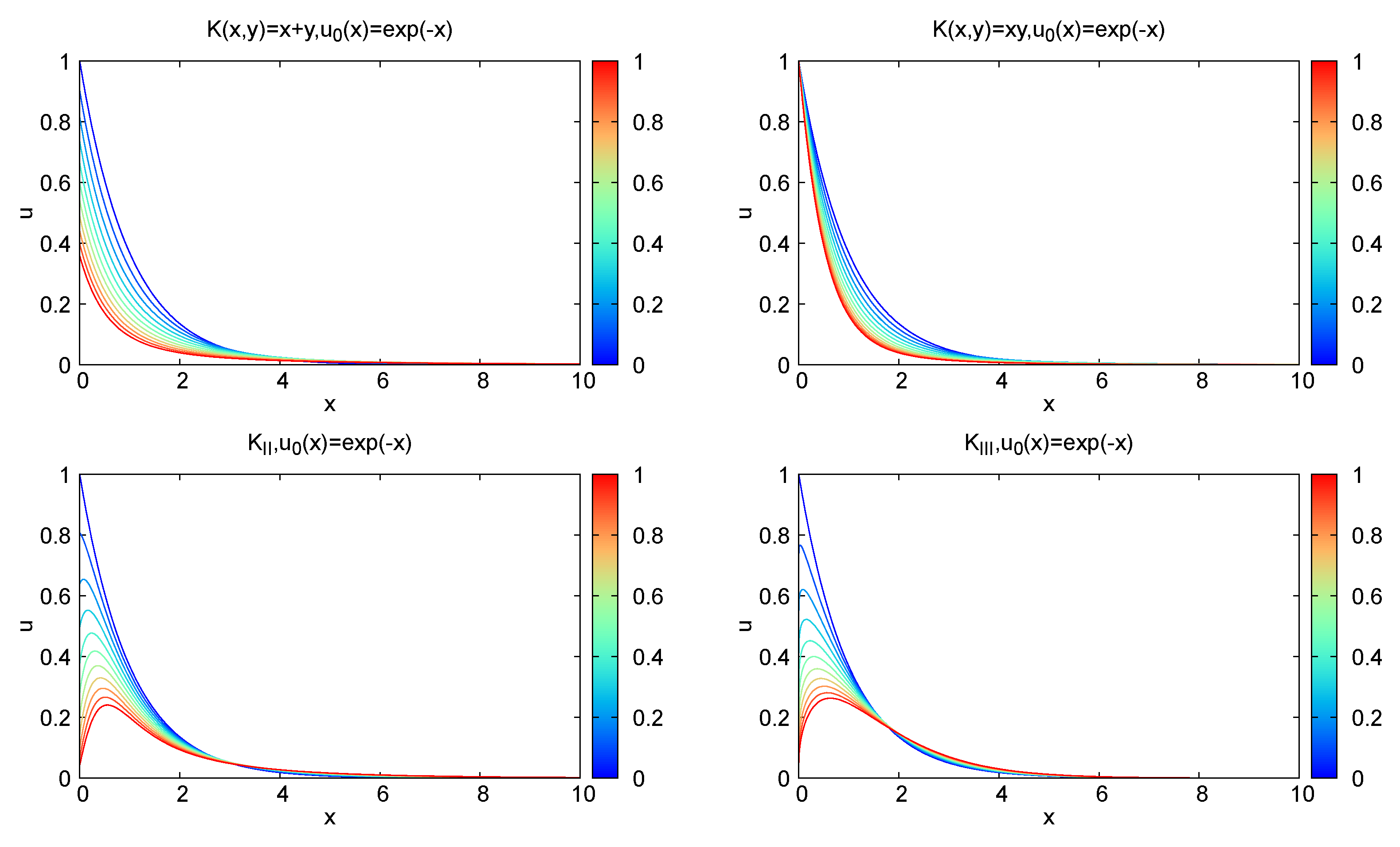
5. Examples of Mass-Conserving Kernels
5.1. Polynomial Growth Kernel I
5.2. Polynomial Growth Kernel II
5.3. Exponential Growth Kernel
5.4. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Derivation of Flux
Appendix B. A Simple Proof of Gelation Phenomena
Appendix C. Supplementary Lemma
Appendix D. Convexity and Superadditivity
- b is called convex on if
- b is called superadditive on if
References
- Pesmazoglou, I.; Kempf, A.; Navarro-Martinez, S. Stochastic modelling of particle aggregation. Int. J. Multiph. Flow 2016, 80, 118–130. [Google Scholar] [CrossRef]
- Ernst, M.; Ziff, R.M.; Hendriks, E. Coagulation processes with a phase transition. J. Colloid Interface Sci. 1984, 97, 266–277. [Google Scholar] [CrossRef]
- Ziff, R.M. Kinetics of polymerization. J. Stat. Phys. 1980, 23, 241–263. [Google Scholar] [CrossRef]
- Drake, R.L. A general mathematical survey of the coagulation equation. Int. Rev. Aerosol Phys. Chem. 1972, 3, 201–376. [Google Scholar]
- Seinfeld, J.H. ES and T books: Atmospheric chemistry and physics of air pollution. Environ. Sci. Technol. 1986, 20, 863. [Google Scholar] [CrossRef] [PubMed]
- Kumar, J.; Warnecke, G.; Peglow, M.; Heinrich, S. Comparison of numerical methods for solving population balance equations incorporating aggregation and breakage. Powder Technol. 2009, 189, 218–229. [Google Scholar] [CrossRef]
- Zidar, M.; Kuzman, D.; Ravnik, M. Characterisation of protein aggregation with the Smoluchowski coagulation approach for use in biopharmaceuticals. Soft Matter 2018, 14, 6001–6012. [Google Scholar] [CrossRef]
- Tavaré, S. Line-of-descent and genealogical processes, and their applications in population genetics models. Theor. Popul. Biol. 1984, 26, 119–164. [Google Scholar] [CrossRef]
- Family, F.; Landau, D.P. Kinetics of Aggregation and Gelation; Elsevier: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Silk, J.; White, S.D. The development of structure in the expanding universe. Astrophys. J. 1978, 223, L59–L62. [Google Scholar] [CrossRef]
- Banakar, Z.; Tavana, M.; Huff, B.; Di Caprio, D. A bank merger predictive model using the Smoluchowski stochastic coagulation equation and reverse engineering. Int. J. Bank Mark. 2018, 36, 634–662. [Google Scholar] [CrossRef]
- Smoluchowski, M. Drei vorträge über diffusion, brownsche molekularbewegung und koagulation von kolloidteilchen. Pisma Marian. Smoluchowskiego 1927, 2, 530–594. [Google Scholar]
- Müller, H. Zur allgemeinen Theorie ser raschen Koagulation. Kolloidchem. Beih. 1928, 27, 223–250. [Google Scholar] [CrossRef]
- Smit, D.; Hounslow, M.; Paterson, W. Aggregation and gelation—I. Analytical solutions for CST and batch operation. Chem. Eng. Sci. 1994, 49, 1025–1035. [Google Scholar] [CrossRef]
- Aldous, D.J. Deterministic and stochastic models for coalescence (aggregation and coagulation): A review of the mean-field theory for probabilists. Bernoulli 1999, 5, 3–48. [Google Scholar] [CrossRef]
- Estrada, P.R.; Cuzzi, J.N. Solving the coagulation equation by the moments method. Astrophys. J. 2008, 682, 515–526. [Google Scholar] [CrossRef]
- Menon, G.; Pego, R.L. Approach to self-similarity in Smoluchowski’s coagulation equations. Commun. Pure Appl. Math. A J. Issued Courant Inst. Math. Sci. 2004, 57, 1197–1232. [Google Scholar] [CrossRef]
- Van Dongen, P.; Ernst, M. Scaling solutions of Smoluchowski’s coagulation equation. J. Stat. Phys. 1988, 50, 295–329. [Google Scholar] [CrossRef]
- Kruis, F.E.; Maisels, A.; Fissan, H. Direct simulation Monte Carlo method for particle coagulation and aggregation. Am. Inst. Chem. Eng. J. 2000, 46, 1735–1742. [Google Scholar] [CrossRef]
- Mahoney, A.W.; Ramkrishna, D. Efficient solution of population balance equations with discontinuities by finite elements. Chem. Eng. Sci. 2002, 57, 1107–1119. [Google Scholar] [CrossRef]
- Filbet, F.; Laurençot, P. Numerical simulation of the Smoluchowski coagulation equation. SIAM J. Sci. Comput. 2004, 25, 2004–2028. [Google Scholar] [CrossRef]
- Sadri, K.; Ebrahimi, H. New Method for Homogeneous Smoluchowski Coagulation Equation. J. Appl. Comput. Math. 2015, 4, 2. [Google Scholar]
- Makino, J.; Fukushige, T.; Funato, Y.; Kokubo, E. On the mass distribution of planetesimals in the early runaway stage. New Astron. 1998, 3, 411–417. [Google Scholar] [CrossRef]
- Tanaka, H.; Inaba, S.; Nakazawa, K. Steady-state size distribution for the self-similar collision cascade. Icarus 1996, 123, 450–455. [Google Scholar] [CrossRef]
- McLeod, J. On an infinite set of non-linear differential equations. Q. J. Math. 1962, 13, 119–128. [Google Scholar] [CrossRef]
- Escobedo, M.; Mischler, S.; Perthame, B. Gelation in coagulation and fragmentation models. Commun. Math. Phys. 2002, 231, 157–188. [Google Scholar] [CrossRef]
- Laurençot, P. On a class of continuous coagulation-fragmentation equations. J. Differ. Equ. 2000, 167, 245–274. [Google Scholar] [CrossRef]
- Escobedo, M.; Mischler, S.; Ricard, M.R. On self-similarity and stationary problem for fragmentation and coagulation models. Ann. l’Inst. Henri Poincaré C Anal. Non Linéaire 2005, 22, 99–125. [Google Scholar] [CrossRef]
- Escobedo, M.; Mischler, S. Dust and self-similarity for the Smoluchowski coagulation equation. Ann. l’Inst. Henri Poincaré C Anal. Non Linéaire 2006, 23, 331–362. [Google Scholar] [CrossRef]
- Norris, J.R. Smoluchowski’s coagulation equation: Uniqueness, nonuniqueness and a hydrodynamic limit for the stochastic coalescent. Ann. Appl. Probab. 1999, 9, 78–109. [Google Scholar] [CrossRef]
- Pego, R.L. Dynamics and scaling in models of coarsening and coagulation. In Proceedings of the Pan-American Advanced Studies Institute (VIII America’s Conference on Differential Equations), Mexico City, Mexico, 15–17 October 2009. [Google Scholar]
- Evans, L.C.; Gariepy, R.F. Measure Theory and Fine Properties of Functions; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Islam, M.S.; Kimura, M.; Miyata, H. Generalized Moment Method for Smoluchowski Coagulation Equation and Mass Conservation Property. Mathematics 2023, 11, 2770. https://doi.org/10.3390/math11122770
Islam MS, Kimura M, Miyata H. Generalized Moment Method for Smoluchowski Coagulation Equation and Mass Conservation Property. Mathematics. 2023; 11(12):2770. https://doi.org/10.3390/math11122770
Chicago/Turabian StyleIslam, Md. Sahidul, Masato Kimura, and Hisanori Miyata. 2023. "Generalized Moment Method for Smoluchowski Coagulation Equation and Mass Conservation Property" Mathematics 11, no. 12: 2770. https://doi.org/10.3390/math11122770
APA StyleIslam, M. S., Kimura, M., & Miyata, H. (2023). Generalized Moment Method for Smoluchowski Coagulation Equation and Mass Conservation Property. Mathematics, 11(12), 2770. https://doi.org/10.3390/math11122770






