Stochastic Strike-Slip Fault as Earthquake Source Model
Abstract
:1. Introduction
2. Mathematical Formulation
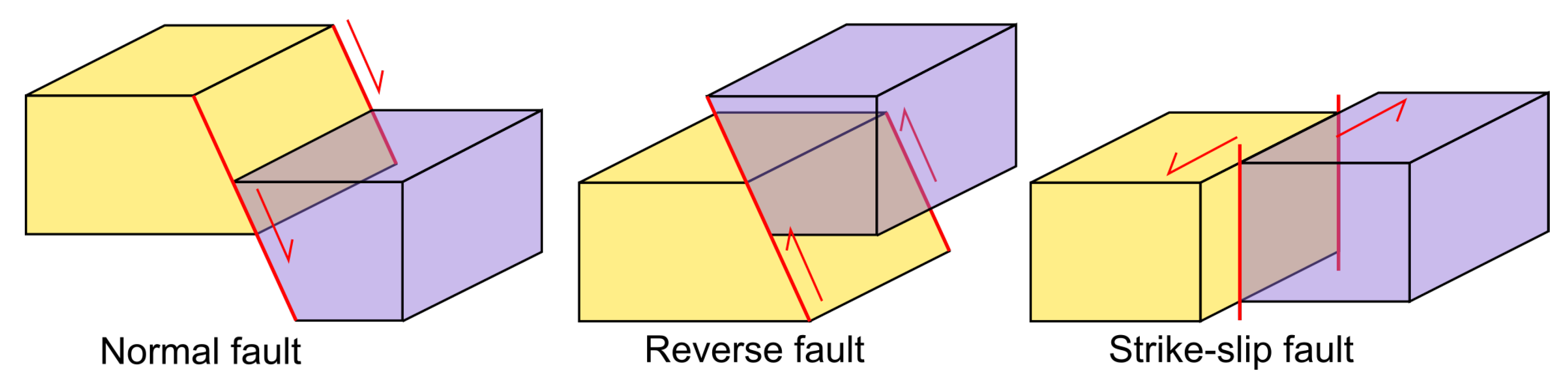
2.1. Earthquake Fault Model
2.2. Stress–Strain State of the Earth’s Crust
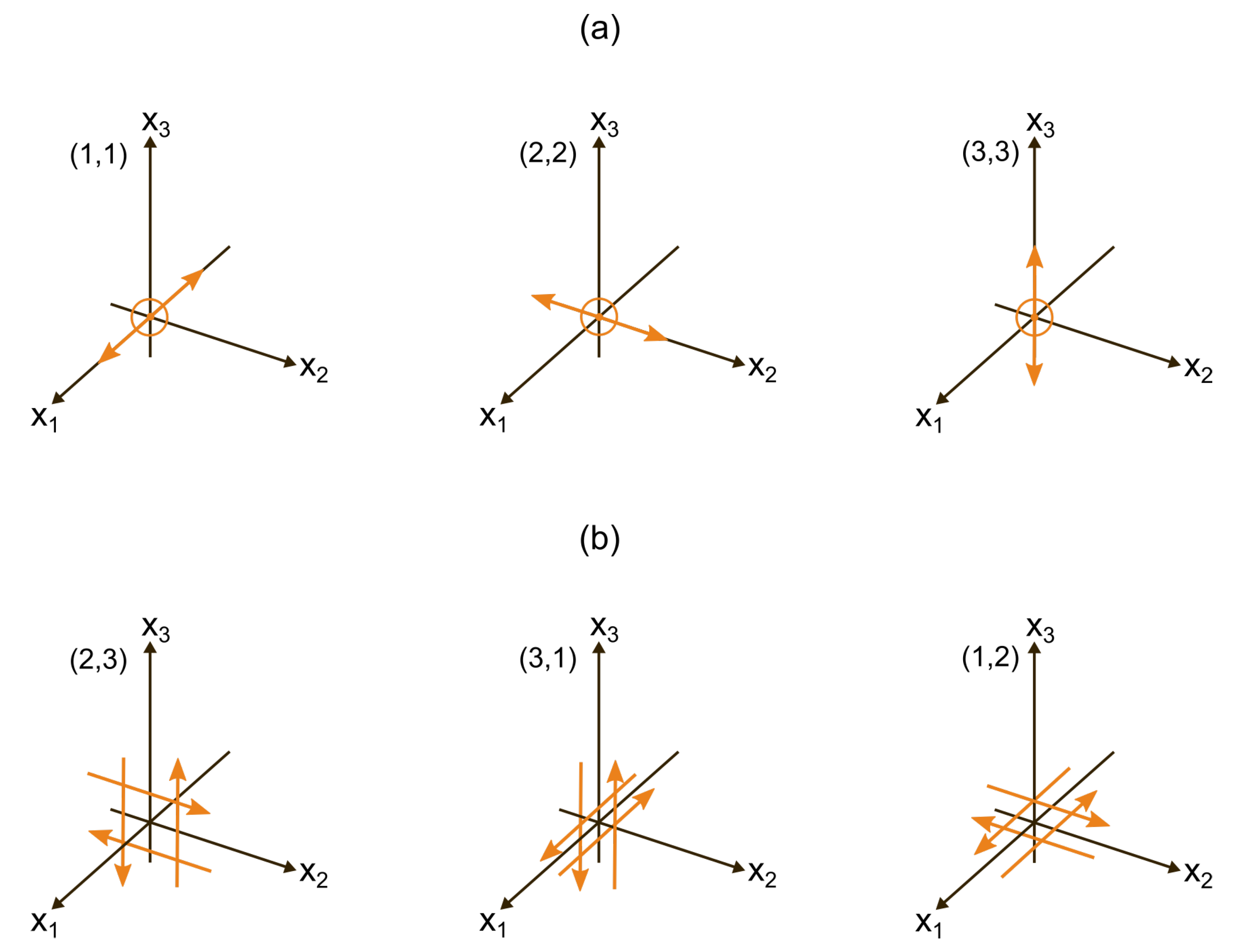
2.3. Point Source: Mindlin Solutions
2.4. Distributed Source
2.5. Dislocation with a Constant Displacement Vector along a Finite Rectangular Surface
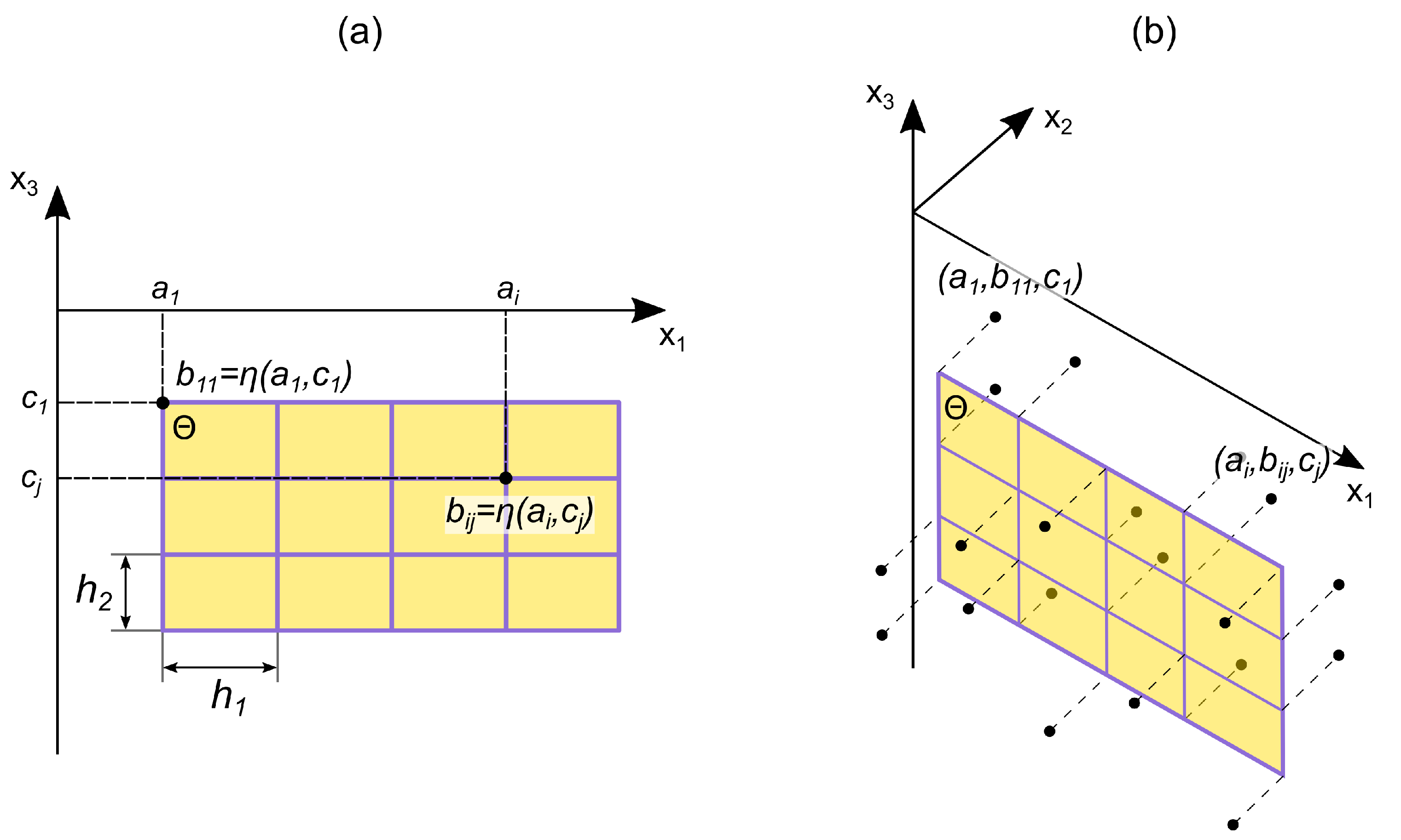
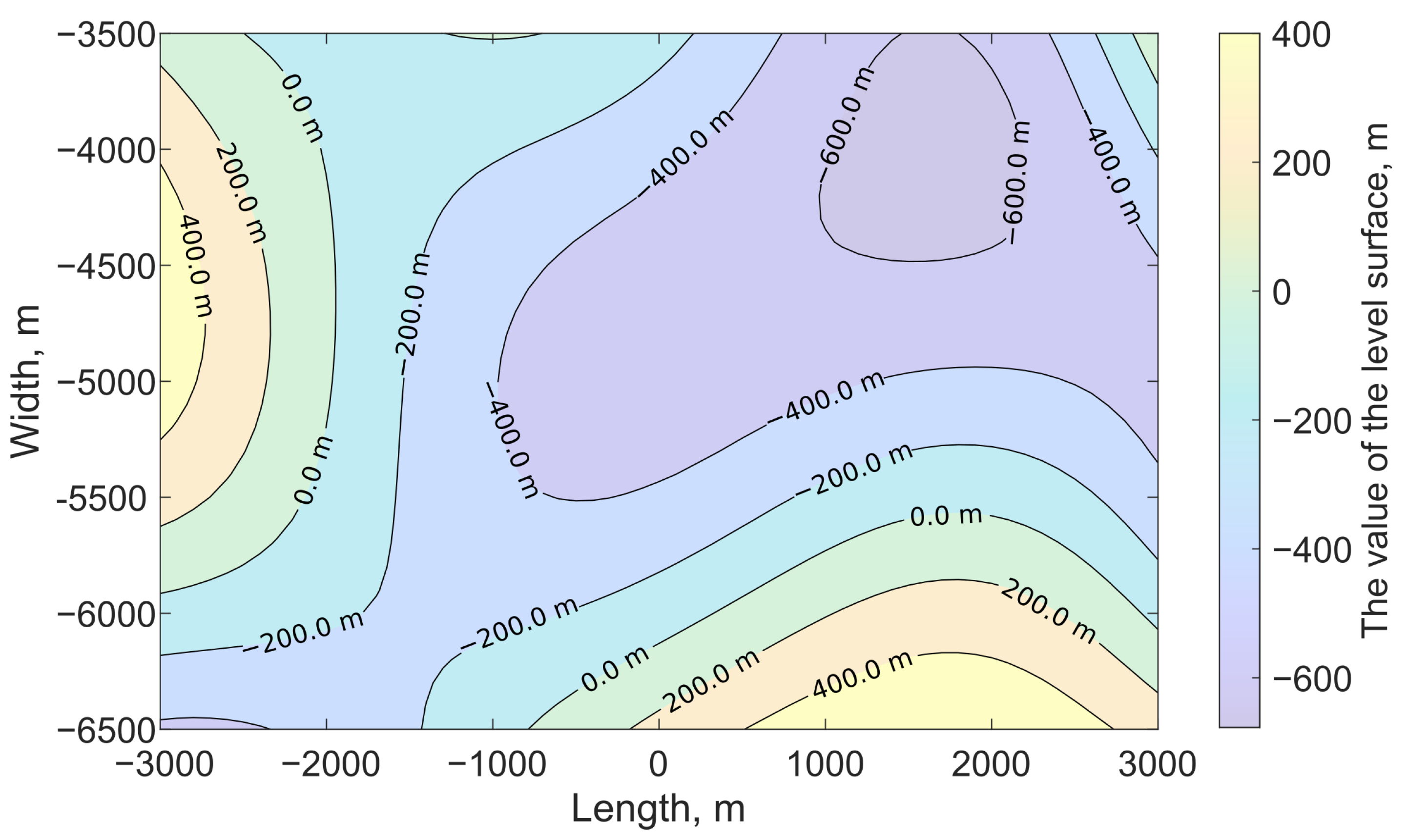
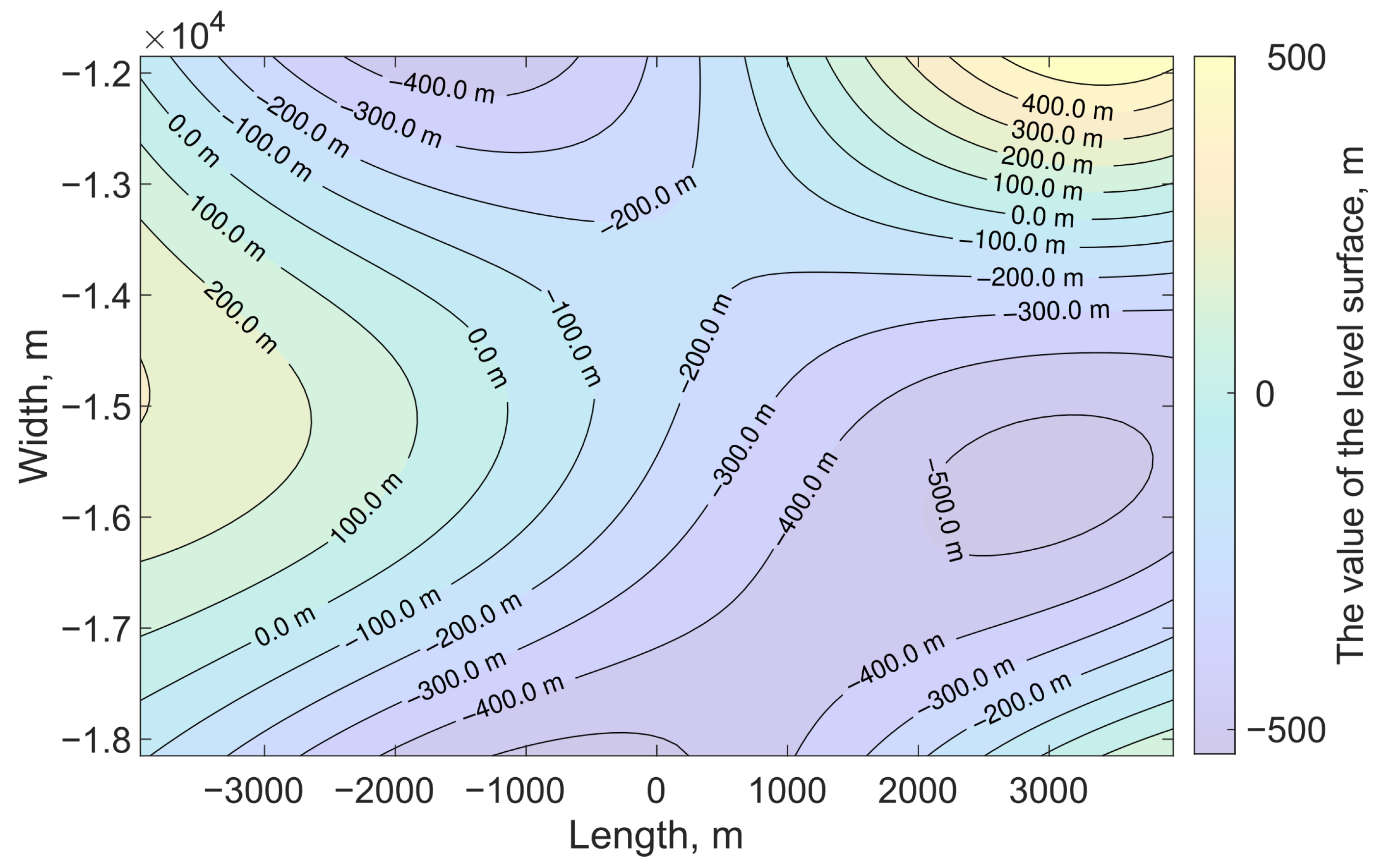
2.6. Stochastic Geometry of the Fault Surface
- We build a two-dimensional grid on the plane with horizontal and vertical steps and , respectively (Figure 4a).
- We define a random function . For simplicity, we assume that is characterized by a single distribution function for all values of the arguments . Function at each grid node () determines the value . These values are the stochastic deformation components of the original plane (Figure 4b).
- The resulting fault surface is determined by the two-dimensional Lagrange interpolation polynomial constructed from the points as follows:where and are functions defined in terms of polynomials and :
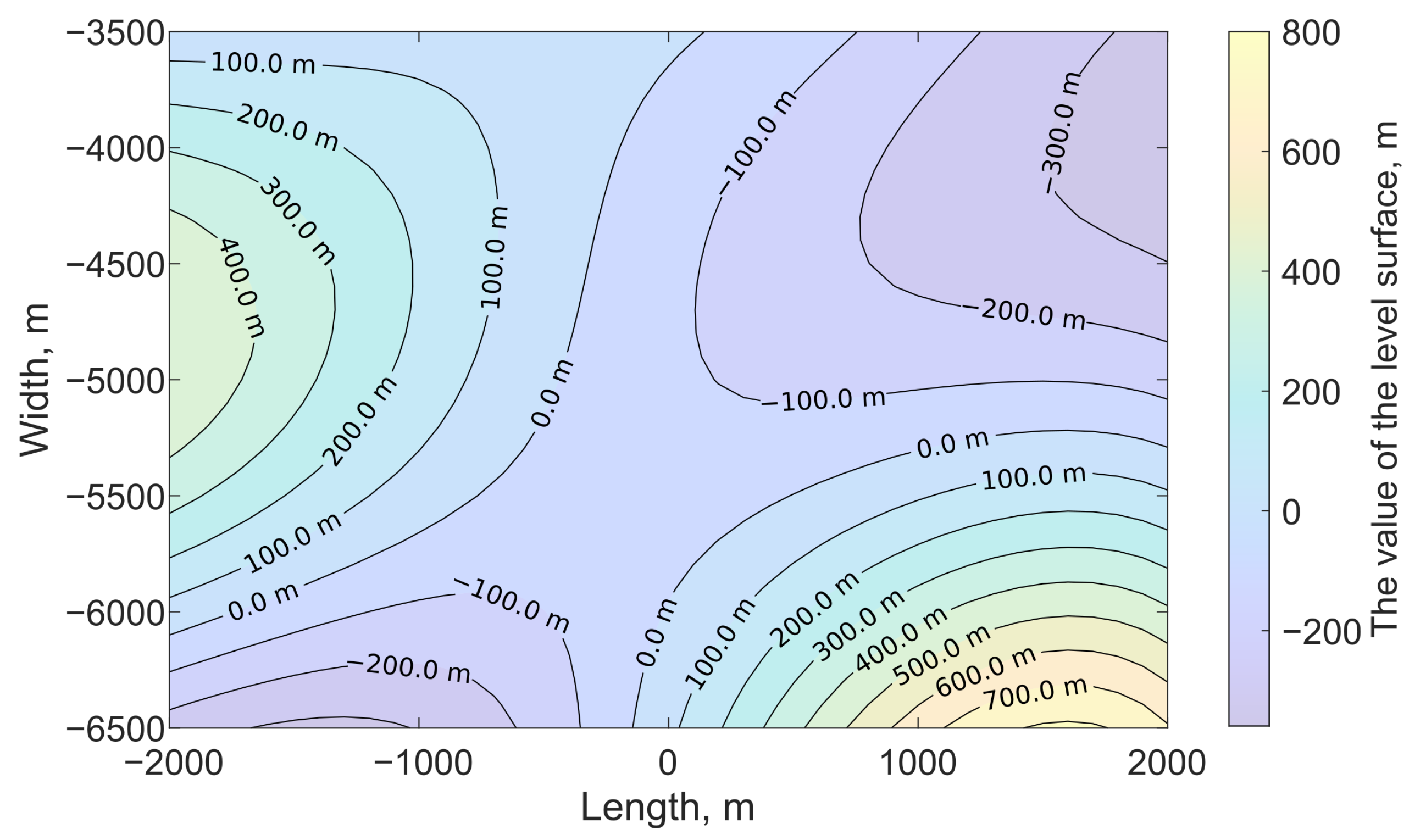
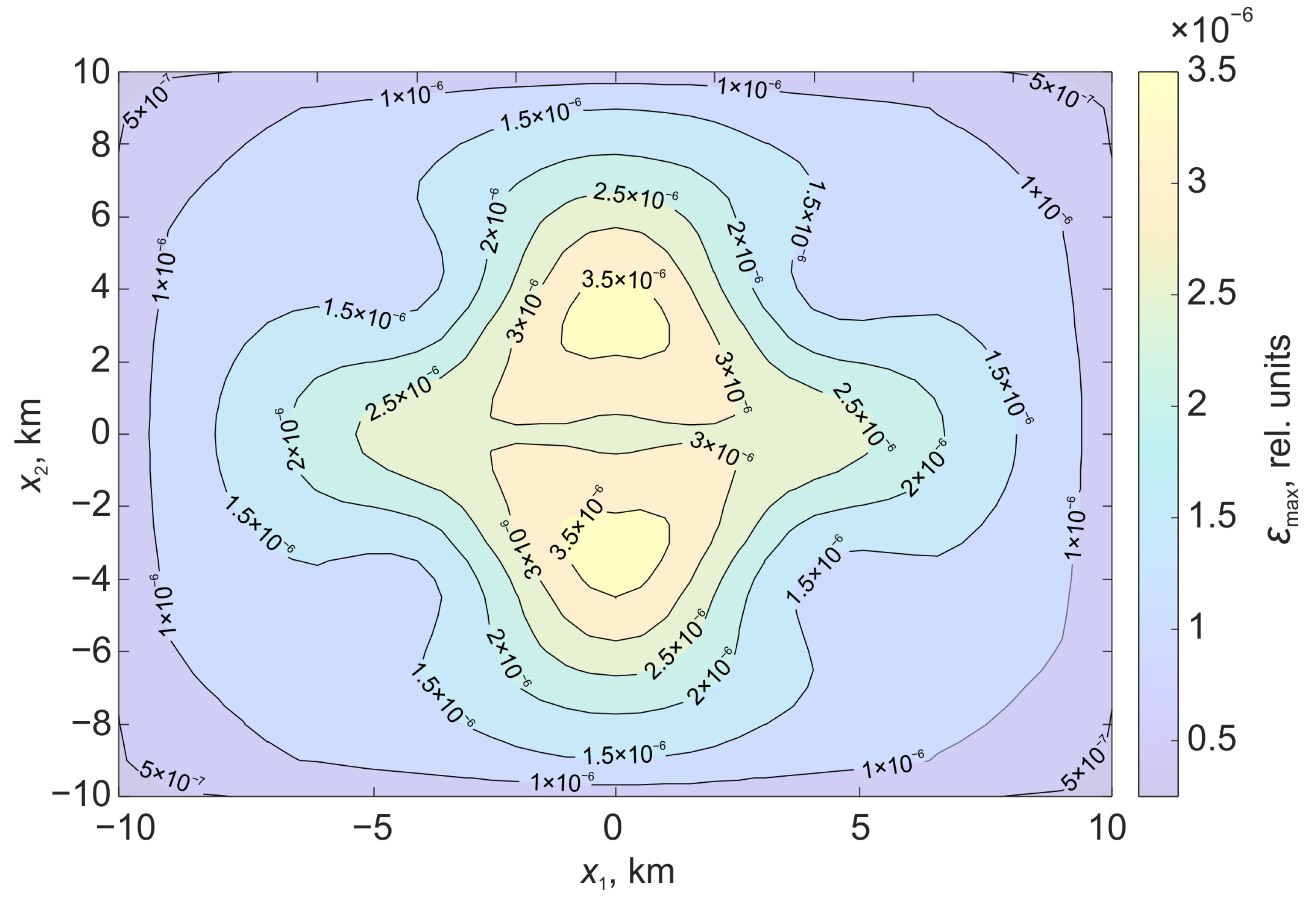
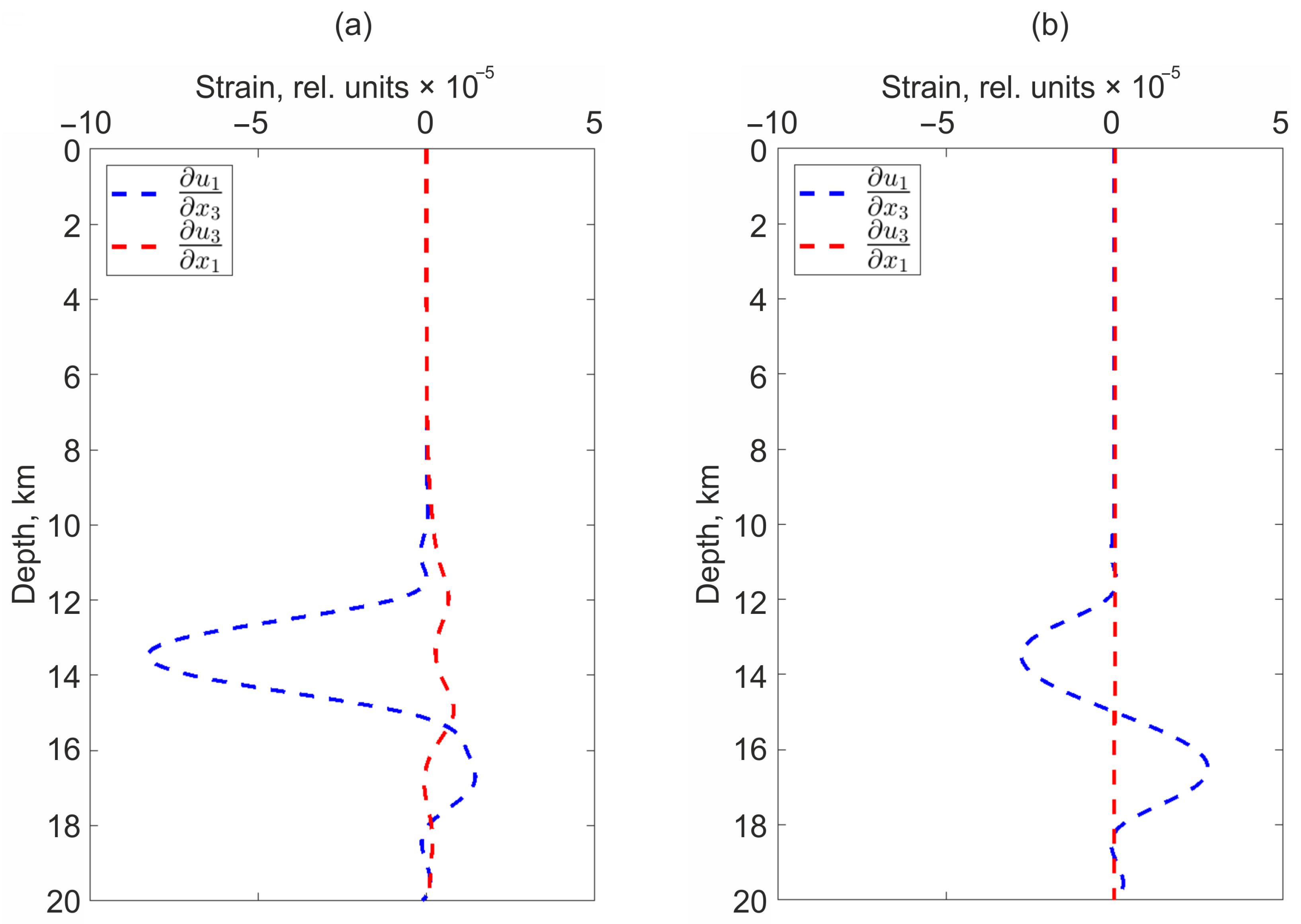
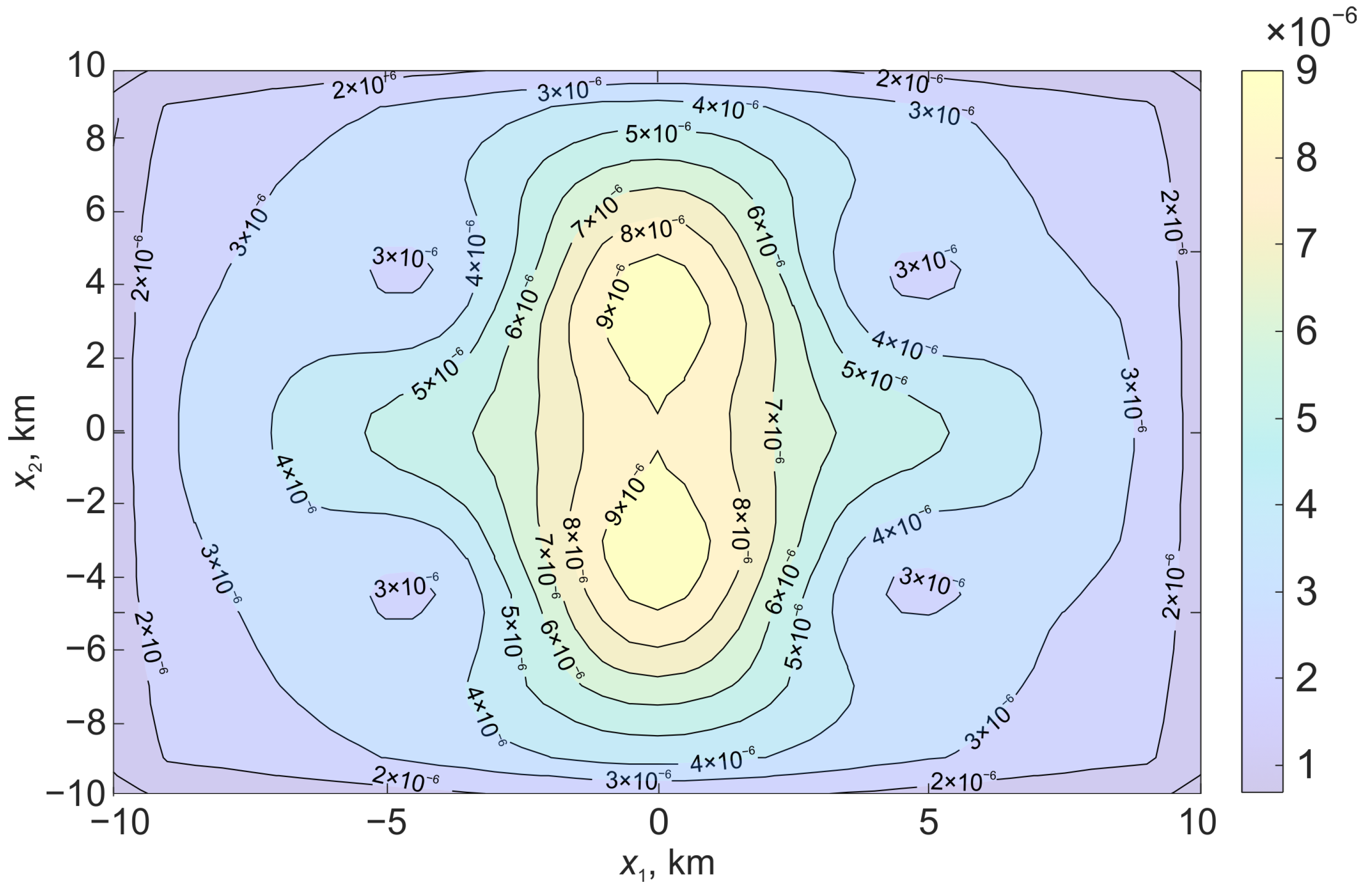
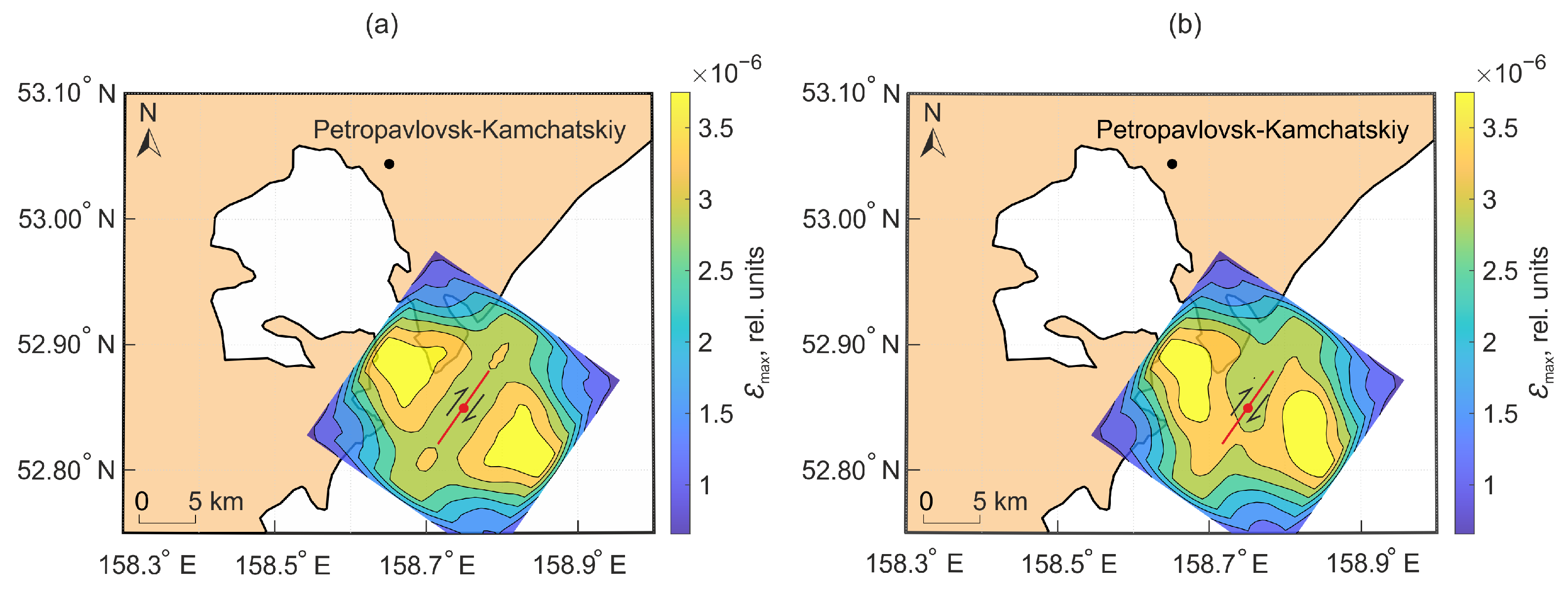
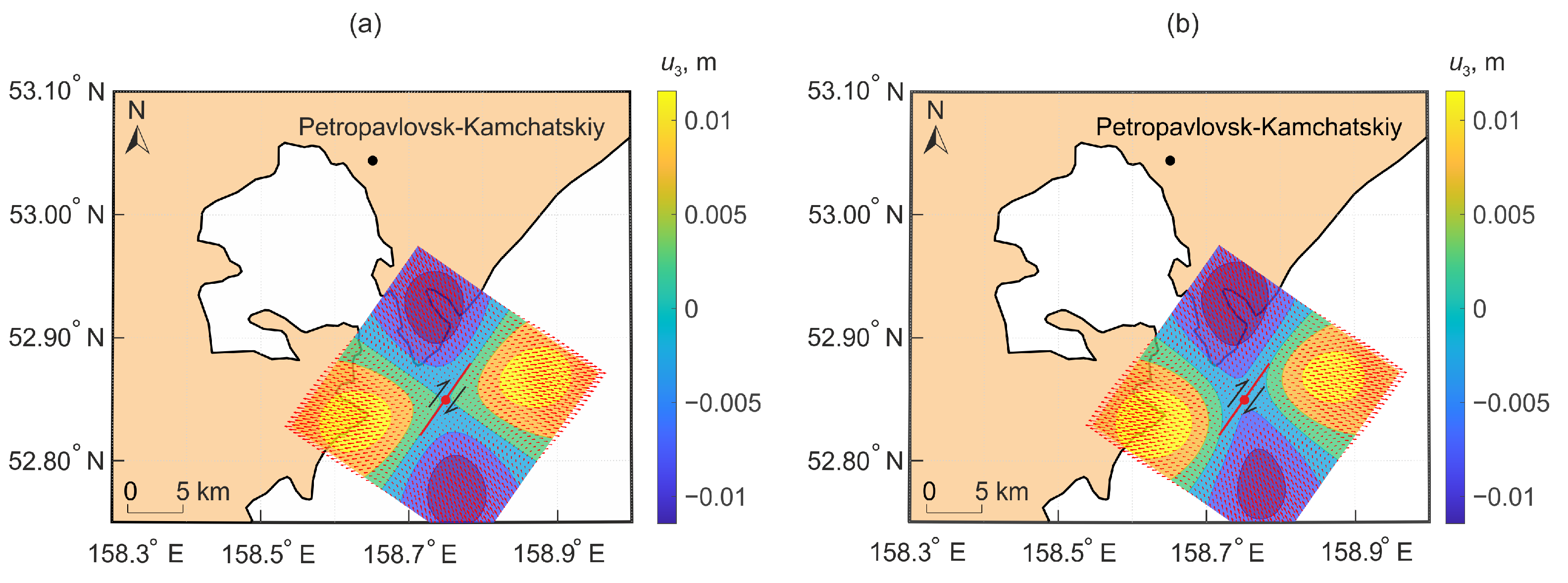
3. Numerical Results
3.1. Computational Experiment No. 1
3.2. Computational Experiment No. 2
3.3. Computational Experiment No. 3
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. List of Symbols
| Displacement vector, m | |
| Body forces, N | |
| Lame coefficient, N/m2 | |
| Cauchy stress tensor, Pa | |
| Deformation tensor | |
| Fault surface | |
| Rectangular flat fault surface | |
| Local coordinate system of fault surface, m | |
| Kronecker delta | |
| The unit vector of the normal to the fault surface | |
| Two-dimensional Lagrange interpolation polynomial | |
| Subfunctions of Lagrange interpolation polynomial | |
| Random function on fault surface, m | |
| Maximum shear stresses, Pa | |
| Deformations corresponding to maximum shear stresses | |
| Poisson ration | |
| E | Young’s modulus, Pa |
Appendix B. Values bij of Computational Experiments (in Meters)
| −151.575662 | −167.2501849 | 86.02206964 | 350.6734393 | −182.1638785 |
| 303.8948632 | −245.7829534 | 482.6726622 | 30.68377715 | 108.2363553 |
| −59.72560829 | −160.705628 | 14.25973541 | 54.62442853 | 112.2277064 |
| 588.2951885 | 653.4678239 | −114.36393 | −148.4616588 | −166.7357272 |
| −175.1326393 | 176.655831 | −290.0019462 | −346.9933307 | 2.728991464 |
| −51.77557155 | 276.9250111 | 233.9080196 | −175.8669899 | −765.4548334 |
| 239.6292914 | −427.8632821 | 486.255464 | 126.8461625 | 229.7749523 |
| −436.943723 | −220.3490067 | −215.757756 | −13.15225722 | 380.5576059 |
| 96.11786833 | 255.8154879 | −518.2024406 | −132.5533594 | −670.5679676 |
| −609.451099 | 554.4508372 | −380.6856921 | −604.8762498 | −2.250633035 |
| −124.1006191 | −237.508935 | 170.5411082 | −242.9299812 | −292.624739 |
| −154.8061296 | −557.7516502 | 125.7641039 | 248.990626 | 288.8774588 |
| −101.6265996 | −88.81333909 | 300.1738999 | 17.92761169 | −200.1037148 |
| 130.7396886 | −519.9234898 | −30.36251049 | −399.3414365 | 310.1585568 |
| 337.0176471 | −204.7378636 | −454.7613229 | 270.9554677 | 64.18209765 |
| 25.63449076 | 239.316592 | −295.6241063 | −100.4050599 | −3.649509249 |
References
- Aki, K.; Richards, P. Quantitative Seismology, 2nd ed.; University Science Books: Cambridge, UK, 2002. [Google Scholar]
- Sholz, C. The Mechanics of Earthquakes and Faulting, 3rd ed.; Cambridge University Press: Cambridge, UK, 2019. [Google Scholar]
- Steketee, J. On Volterra’s dislocations in a semi-infinity elastic medium. Can. J. Phys. 1958, 36, 192–205. [Google Scholar] [CrossRef]
- Kundu, P.; Sarkar, S.; Mondal, D. Creeping effect across a buried, inclined, finite strike-slip fault in an elastic-layer overlying an elastic half-space. GEM—Int. J. Geomath. 2021, 12, 1–33. [Google Scholar] [CrossRef]
- Liu, T.; Fu, G.; She, Y.; Zhao, C. Co-seismic internal deformations in a spherical layered earth model. Geophys. J. Int. 2020, 221, 1515–1531. [Google Scholar] [CrossRef]
- Mondal, D.; Debnath, P. An application of fractional calculus to geophysics: Effect of a strike-slip fault on displacement, stresses and strains in a fractional order Maxwell type visco-elastic half-space. Int. J. Appl. Math. 2021, 34, 873–888. [Google Scholar] [CrossRef]
- Press, F. Displacements, strains, and tilts at teleseismic distances. J. Geophys. Res. 1965, 70, 2395–2412. [Google Scholar] [CrossRef]
- Saltykov, V.A.; Kugaenko, Y.A. Development of near-surface dilatancy zones as a possible cause for seismic emission anomalies before strong earthquakes. Russ. J. Pac. Geol. 2012, 6, 86–95. [Google Scholar] [CrossRef]
- Okada, Y. Internal deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Am. 1992, 82, 1018–1040. [Google Scholar] [CrossRef]
- Gusev, A. Statistics of the values of the normalized movement at the points of the fault-the focus of the earthquake. Phys. Earth 2011, 3, 24–33. (In Russian) [Google Scholar]
- Lekshmy, P.; Raghukanth, S. Stochastic earthquake source model for ground motion simulation. Earthq. Eng. Eng. Vib. 2019, 18, 1–34. [Google Scholar] [CrossRef]
- Zhang, G.; Wang, Z.; Sang, W.; Zhou, B.; Wang, Z.; Yao, G.; Bi, J. Research on Dynamic Deformation Laws of Super High-Rise Buildings and Visualization Based on GB-RAR and LiDAR Technology. Remote Sens. 2023, 15, 3651. [Google Scholar] [CrossRef]
- Ding, Y.; Xu, Y.; Ding, S. A Stochastic Earthquake Ground Motion Database and Its Application in Seismic Analysis of an RC Frame-Shear Wall Structure. Buildings 2023, 13, 1637. [Google Scholar] [CrossRef]
- Small, D.T.; Melgar, D. Geodetic coupling models as constraints on stochastic earthquake ruptures: An example application to PTHA in Cascadia. J. Geophys. Res. Solid Earth 2021, 126, e2020JB021149. [Google Scholar] [CrossRef]
- Gusev, A. Doubly stochastic earthquake source model: “Omega-Square” spectrum and low high-frequency directivity revealed by numerical experiments. Pure Appl. Geophys. 2014, 171, 2581–2599. [Google Scholar] [CrossRef]
- Peng, W.; Huang, X.; Wang, Z. Focal Mechanism and Regional Fault Activity Analysis of 2022 Luding Strong Earthquake Constraint by InSAR and Its Inversion. Remote Sens. 2023, 15, 3753. [Google Scholar] [CrossRef]
- Zhao, J.-J.; Chen, Q.; Yang, Y.-H.; Xu, Q. Coseismic Faulting Model and Post-Seismic Surface Motion of the 2023 Turkey–Syria Earthquake Doublet Revealed by InSAR and GPS Measurements. Remote Sens. 2023, 15, 3327. [Google Scholar] [CrossRef]
- Yu, S.; Su, X. A Crustal Deformation Pattern on the Northeastern Margin of the Tibetan Plateau Derived from GPS Observations. Remote Sens. 2023, 15, 2905. [Google Scholar] [CrossRef]
- Rebetsky, Y.L. Regularities of crustal faulting and tectonophysical indicators of fault metastability. Geodyn. Tectonophys. 2018, 9, 629–652. [Google Scholar] [CrossRef]
- Molotnikov, V.; Molotnikova, A. Theory of Elasticity and Plasticity; Springer International Publishing: Cham, Switzerland, 2021. [Google Scholar]
- Mindlin, R. Force at a point in the interior of a Semi-Infinite solid. J. Appl. Phys. 1936, 195, 195–292. [Google Scholar] [CrossRef]
- Mindlin, R.; Cheng, D. Nuclei of Strain in the Semi-Infinite Solid. J. Appl. Phys. 1950, 21, 926–930. [Google Scholar] [CrossRef]
- Pan, P.-Z.; Miao, S.; Wu, Z.; Feng, X.-T.; Shao, C. Laboratory observation of spalling process induced by tangential stress concentration in hard rock tunnel. Int. J. Geomech. 2020, 20, 04020011. [Google Scholar] [CrossRef]
- Wang, C.; Wang, S. Modified Generalized Maximum Tangential Stress Criterion for Simulation of Crack Propagation and Its Application in Discontinuous Deformation Analysis. Eng. Fract. Mech. 2022, 259, 108159. [Google Scholar] [CrossRef]
- Xiao, P.; Li, D.; Zhu, Q. Strain Energy Release and Deep Rock Failure Due to Excavation in Pre-Stressed Rock. Minerals 2022, 12, 488. [Google Scholar] [CrossRef]
- Clenshaw, C.W.; Curtis, A.R. A method for numerical integration on an automatic computer. Numer. Math. 1960, 2, 197–205. [Google Scholar] [CrossRef]
- Gusev, A.A.; Melnikova, V.N. Relations between magnitudes: Global and Kamchatka data. J. Volcanol. Seismol. 1990, 6, 55–63. (In Russian) [Google Scholar]
- Kozhurin, A.; Acocella, V.; Kyle, P.R.; Lagmay, F.M.; Melekestsev, I.V.; Ponomareva, V.; Rust, D.; Tibaldi, A.; Tunesi, A.; Crazzato, C.; et al. Trenching studies of active faults in Kamchatka, eastern Russia: Palaeoseismic, tectonic and hazard implications. Tectonophysics 2006, 417, 285–304. [Google Scholar] [CrossRef]
- Kropotkin, P.N. Tectonic Stresses in the Earth’s Crust. Geotectonics 1996, 30, 85–96. [Google Scholar]
- Nazarov, L.A.; Nazarova, L.A.; Kozlova, M.P. Method of interpretation of the geodetic data for the estimation of parameters of an imminent seismic event focus. Interexpo GEO-Sib. 2011, 2, 49–53. (In Russian) [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gapeev, M.; Solodchuk, A.; Parovik, R. Stochastic Strike-Slip Fault as Earthquake Source Model. Mathematics 2023, 11, 3932. https://doi.org/10.3390/math11183932
Gapeev M, Solodchuk A, Parovik R. Stochastic Strike-Slip Fault as Earthquake Source Model. Mathematics. 2023; 11(18):3932. https://doi.org/10.3390/math11183932
Chicago/Turabian StyleGapeev, Maksim, Alexandra Solodchuk, and Roman Parovik. 2023. "Stochastic Strike-Slip Fault as Earthquake Source Model" Mathematics 11, no. 18: 3932. https://doi.org/10.3390/math11183932
APA StyleGapeev, M., Solodchuk, A., & Parovik, R. (2023). Stochastic Strike-Slip Fault as Earthquake Source Model. Mathematics, 11(18), 3932. https://doi.org/10.3390/math11183932







