Revisiting Long-Time Dynamics of Earth’s Angular Rotation Depending on Quasiperiodic Solar Activity
Abstract
:1. Introduction: The Dependence of Earth’s Angular Rotation Dynamics on Earth’s Heat Flux Thermobalance via Quasiperiodic Solar Activity
2. Methodology
2.1. Estimation of Thermobalance of Earth’s Heat Fluxes via Quasiperiodic SolarActivity
- -
- (2) Surface of Earth reradiates all the energy fluxes to outer space according to the Stefan-Boltzmann law [21]:
- -
- (3) The additional losses for evaporation of water from the surface of the ocean [20] should also be taken into account:
- -
- (4) We should additionally take into account the losses for the convection according to the NASA Earth Observatory report [20] as given below
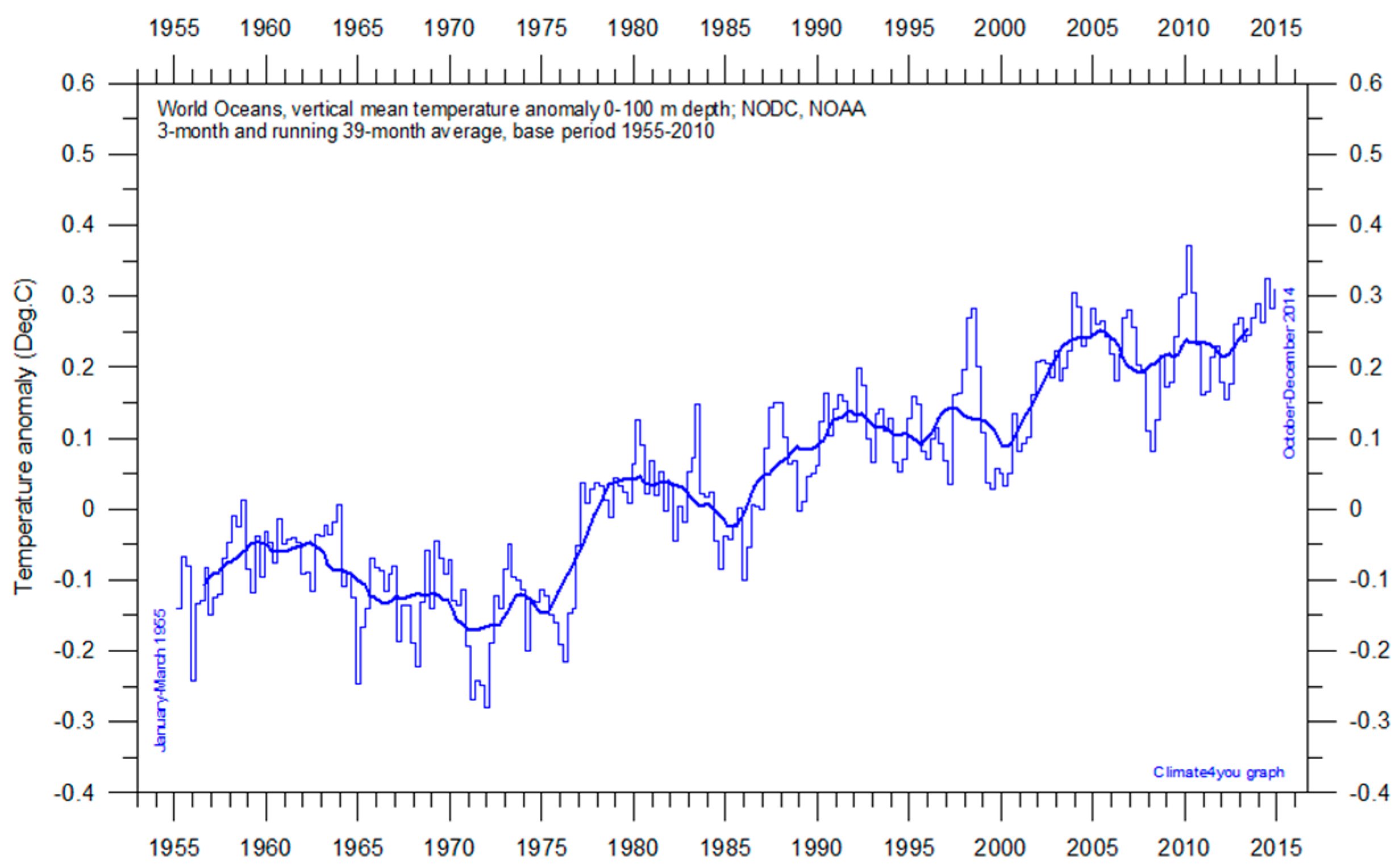
2.2. Quasiperiodic Dynamics of Earth Surface Mean Temperature
→ F out (1) + F out (2) + F out (3) = 0.48 F ext
→ F out (1) + F out (2) + F out (3) = 0.48 F ext
→ F out (1) + F out (2) + F out (3) ≅ 0.48 F ext
3. Results and Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zotov, L.; Bizouard, C.; Shum, C.K. A possible interrelation between Earth rotation and climatic variability at decadal time-scale. Geod. Geodyn. 2016, 7, 216–222. [Google Scholar] [CrossRef]
- Ambrosino, F.; Thinová, L.; Briestenský, M.; Sabbarese, C. Anomalies identification of Earth’s rotation rate time series (2012–2017) for possible correlation with strong earthquakes occurrence. Geod. Geodyn. 2019, 10, 455–459. [Google Scholar] [CrossRef]
- Witze, A. Has Earth’s inner core stopped its strange spin? Nature 2023, 614, 19. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Song, X. Differential rotation of the Earth’s inner core changes over decades and has come to near-halt. Nat. Geosci. 2023, 16, 113–114. [Google Scholar] [CrossRef]
- Moritz, H.; Mueller, I.I. Earth Rotation: Theory and Observation; The Ungar Publishing Company, New York: New York, NY, USA, 1987. [Google Scholar]
- Efroimsky, M. On the theory of canonical perturbations and its application to Earth rotation. arXiv 2005, arXiv:astro-ph/0409282. [Google Scholar]
- Efroimsky, M.; Escapa, A. The theory of canonical perturbations applied to attitude dynamics and to the Earth rotation. Osculating and nonosculating Andoyer variables. Celest. Mech. Dyn. Astron. 2007, 98, 251–283. [Google Scholar] [CrossRef]
- Zharov, V.E. Connection of the earth’s rotation with the atmosphericangular momentum and the strongest earthquakes. Astron. Astrophys. Trans. 1996, 9, 317–327. [Google Scholar] [CrossRef]
- Kinoshita, H. Theory of the rotation of the rigid Earth. Celest. Mech. 1977, 15, 277–326. [Google Scholar] [CrossRef]
- Kinoshita, H.; Souchay, J. The theory of the nutation for the rigid-Earth model at the second order. Celest. Mech. Dyn. Astron. 1990, 48, 187–265. [Google Scholar] [CrossRef]
- Tisserand, F. Traité de Mécanique Céleste; Gauthier-Villars: Paris, France, 1889. [Google Scholar]
- Peale, S.J. Rotation of solid bodies in the solar system. Rev. Geophys. Space Phy. 1973, 11, 767–793. [Google Scholar] [CrossRef]
- Woolard, E.W. Theory of the rotation of the Earth around its centre of mass. Astron. Papers Amer. Ephemer. 1953, 15, 1. [Google Scholar]
- Kubo, Y. Solution to the rotation of the elastic Earth by method of rigid dynamics. Celes. Mech. Dyn. Astron. 1991, 50, 165–187. [Google Scholar] [CrossRef]
- Levin, B.W.; Sasorova, E.V.; Steblov, G.M.; Domanski, A.V.; Prytkov, A.S.; Tsyba, E.N. Variations of the Earth’s rotation rate and cyclic processes in geodynamics. Geod. Geodyn. 2017, 8, 206–212. [Google Scholar] [CrossRef]
- Ershkov, S.V.; Leshchenko, D. Revisiting dynamics of Sun center relative to barycenter of Solar system or Can we move towards stars using Solar self-resulting photo-gravitational force? J. Space Saf. Eng. 2022, 9, 160–164. [Google Scholar] [CrossRef]
- Burša, M.; Fialová, V. Parameters of the Earth’s tri-axial level ellipsoid. Stud. Geophys. Et Geod. 1993, 37, 1–13. [Google Scholar] [CrossRef]
- Massoud, M. §2.1 Blackbody radiation. In Engineering Thermofluids: Thermodynamics, Fluid Mechanics, and Heat Transfer; Springer: Berlin/Heidelberg, Germany, 2005; p. 568. [Google Scholar]
- Kopp, G.; Lean, J.L. A new, lower value of total solar irradiance: Evidence and climate significance. Geophys. Res. Lett. 2011, 38. [Google Scholar] [CrossRef]
- Environmental Encyclopedia, 3rd ed.; Thompson Gale: Farmington Hills, MI, USA, 2003. Available online: http://earthobservatory.nasa.gov/Features/EnergyBalance (accessed on 1 April 2023).
- Cole, G.H.A.; Woolfson, M.M. Planetary Science: The Science of Planets Around Stars, 1st ed.; Institute of Physics Publishing: Bristol, UK, 2002; Volume 36–37, pp. 380–382. [Google Scholar]
- Milankovitch, M. Handbuch der Klimatologie 1. In Mathematische Klimalehre und Astronomische Theorie der Klimaschwankungen; Gebrüder Borntraeger: Stuttgart, Germany, 1930. [Google Scholar]
- Ershkov, S.V.; Shamin, R.V. A Riccati-type solution of 3D Euler equations for incompressible flow. J. King Saud Univ.-Sci. 2020, 32, 125–130. [Google Scholar] [CrossRef]
- Ershkov, S.V. Forbidden Zones for Circular Regular Orbits of the Moons in Solar System, R3BP. J. Astrophys. Astron. 2017, 38, 1–4. [Google Scholar] [CrossRef]
- Legrand, J.P.; Le Goff, M.; Mazaudier, C.; Schröder, W. Solar and auroral activities during the seventeenth century. Acta Geod. Geophys. Hung. 1992, 27, 251–282. [Google Scholar]
- NASA’s Physical Oceanography Distributed Active Archive Center (PO.DAAC). Provider of Historic and Near Real Time SST Data from 14 Satellites, from 1981 through Yesterday. Available online: https://podaac.jpl.nasa.gov/DATA_CATALOG/sst.html (accessed on 1 April 2023).
- Ershkov, S.; Leshchenko, D. Inelastic Collision Influencing the Rotational Dynamics of a Non-Rigid Asteroid (of Rubble Pile Type). Mathematics 2023, 11, 1491. [Google Scholar] [CrossRef]
- Heller, R.; Leconte, J.; Barnes, R. Tidal obliquity evolution of potentially habitable planets. arXiv 2011, arXiv:1101.2156. [Google Scholar] [CrossRef]
- Christianto, V.; Smarandache, F. A Thousand Words: How Shannon Entropy Perspective Provides Link between Exponential Data Growth, Average Temperature of the Earth and Declining Earth Magnetic Field. Bull. Pure Appl. Sci.-Geol. 2019, 38F, 225–231. [Google Scholar] [CrossRef]
- Gross, R.S. Earth rotation variations—Long period. In Treatise on Geophysics; Schubert, G., Ed.; Elsevier: Amsterdam, The Netherlands, 2007; Volume 3, pp. 239–294. [Google Scholar] [CrossRef]
- Lambeck, K.; Casenave, A. Long term variations in the length of day and climatic change. Geophys. J. R. Astr. Soc. 1976, 46, 555–573. [Google Scholar] [CrossRef]
- Lambeck, K. The Earth’s Variable Rotation; Cambridge University Press: Cambridge, UK, 1980; 350p. [Google Scholar]
- Ershkov, S.; Leshchenko, D.; Prosviryakov, E.Y. A novel type of ER3BP introducing Milankovitch cycles or seasonal irradiation processes influencing onto orbit of planet. Arch. Appl. Mech. 2023, 93, 813–822. [Google Scholar] [CrossRef]
- Ershkov, S.; Leshchenko, D.; Aboeulmagd, E. About influence of differential rotation in convection zone of gaseous or fluid giant planet (Uranus) onto the parameters of orbits of satellites. Eur. Phys. J. Plus 2021, 136, 387. [Google Scholar] [CrossRef]
- Ershkov, S.V. Revolving scheme for solving a cascade of Abel equations in dynamics of planar satellite rotation. Theor. Appl. Mech. Lett. 2017, 7, 175–178. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ershkov, S.; Leshchenko, D.; Prosviryakov, E. Revisiting Long-Time Dynamics of Earth’s Angular Rotation Depending on Quasiperiodic Solar Activity. Mathematics 2023, 11, 2117. https://doi.org/10.3390/math11092117
Ershkov S, Leshchenko D, Prosviryakov E. Revisiting Long-Time Dynamics of Earth’s Angular Rotation Depending on Quasiperiodic Solar Activity. Mathematics. 2023; 11(9):2117. https://doi.org/10.3390/math11092117
Chicago/Turabian StyleErshkov, Sergey, Dmytro Leshchenko, and Evgeniy Prosviryakov. 2023. "Revisiting Long-Time Dynamics of Earth’s Angular Rotation Depending on Quasiperiodic Solar Activity" Mathematics 11, no. 9: 2117. https://doi.org/10.3390/math11092117
APA StyleErshkov, S., Leshchenko, D., & Prosviryakov, E. (2023). Revisiting Long-Time Dynamics of Earth’s Angular Rotation Depending on Quasiperiodic Solar Activity. Mathematics, 11(9), 2117. https://doi.org/10.3390/math11092117







