Parameterizations of Delaunay Surfaces from Scratch
Abstract
:1. Introduction
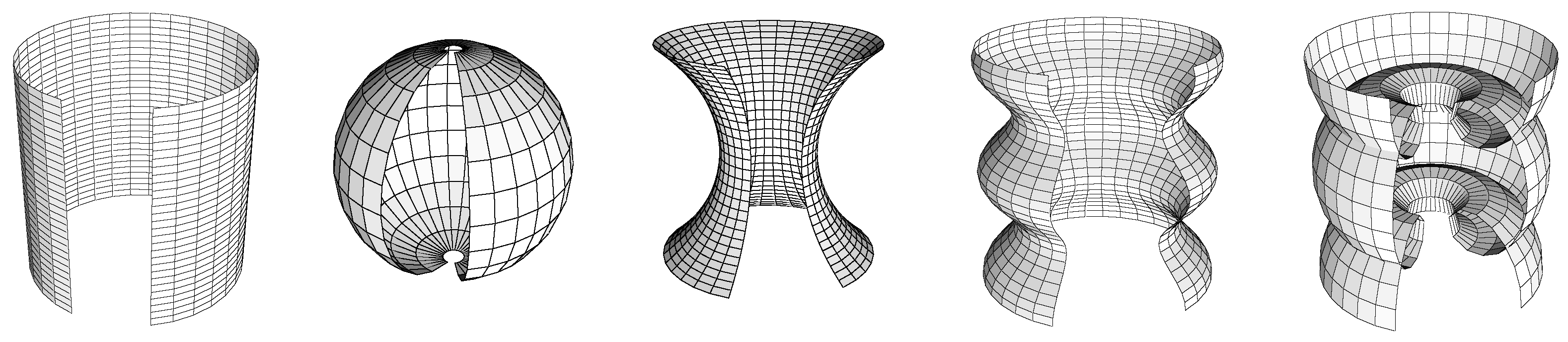
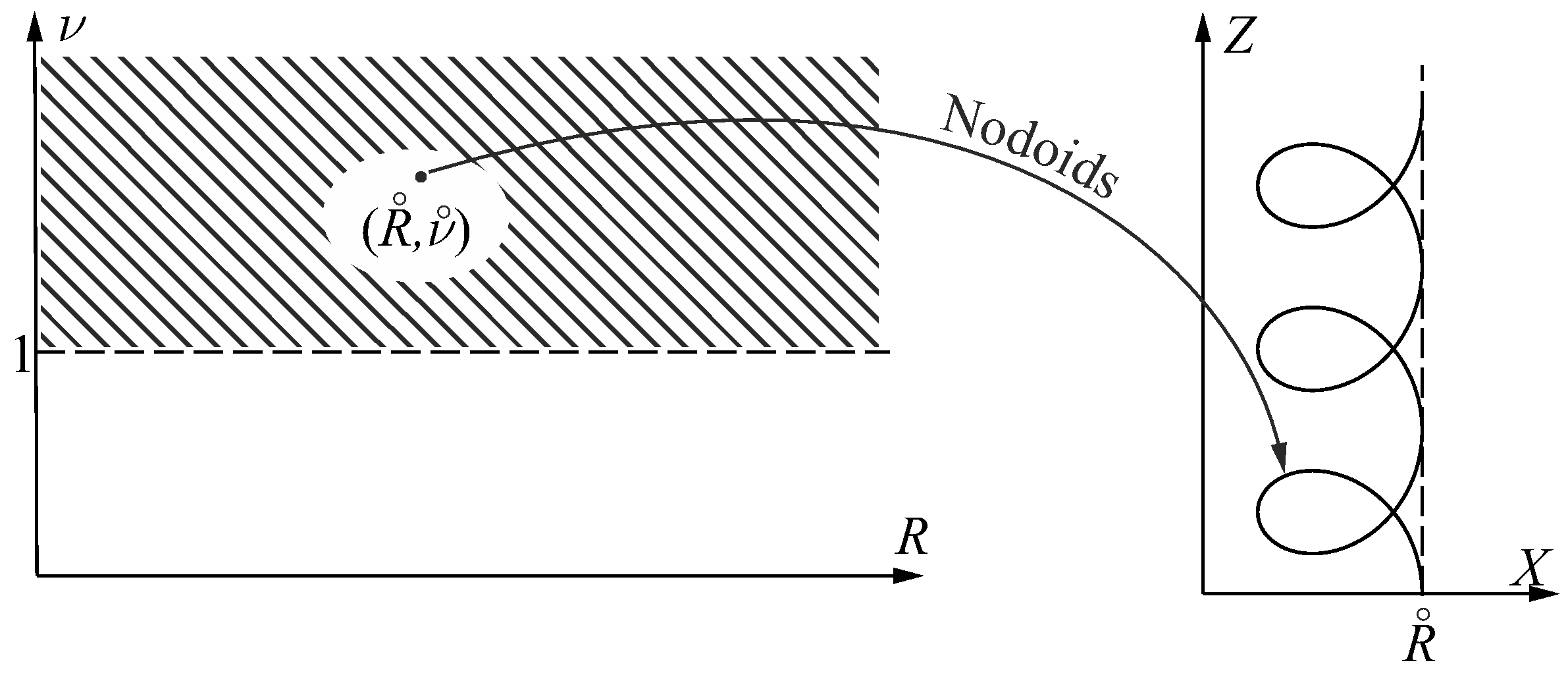
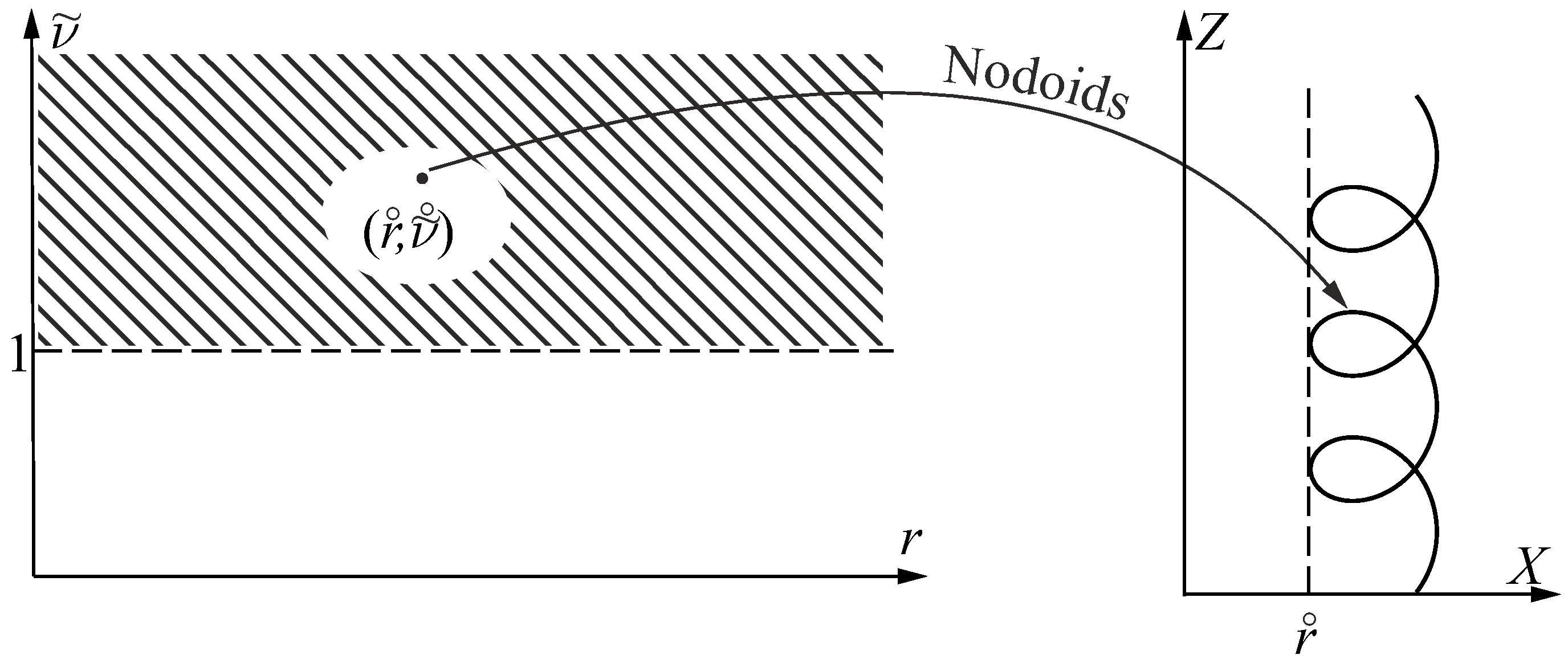
2. The New Parameterizations
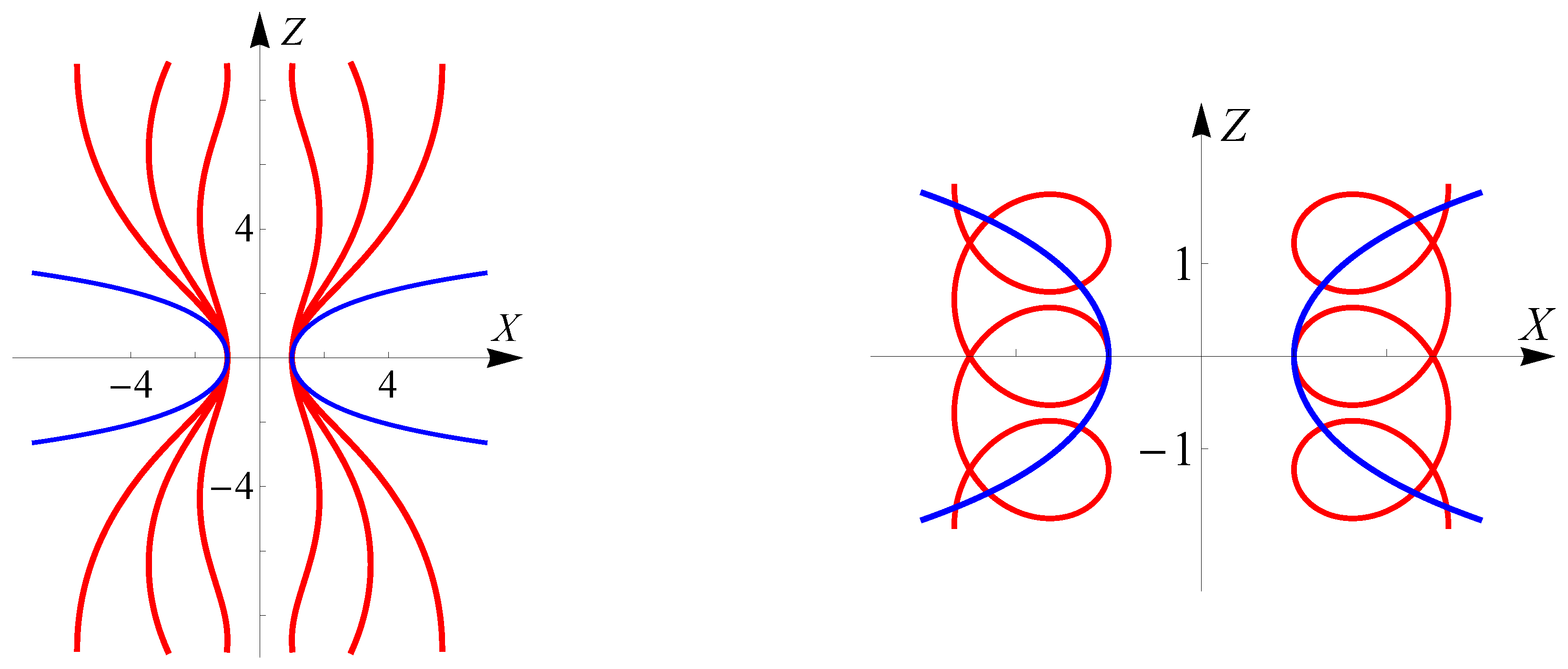
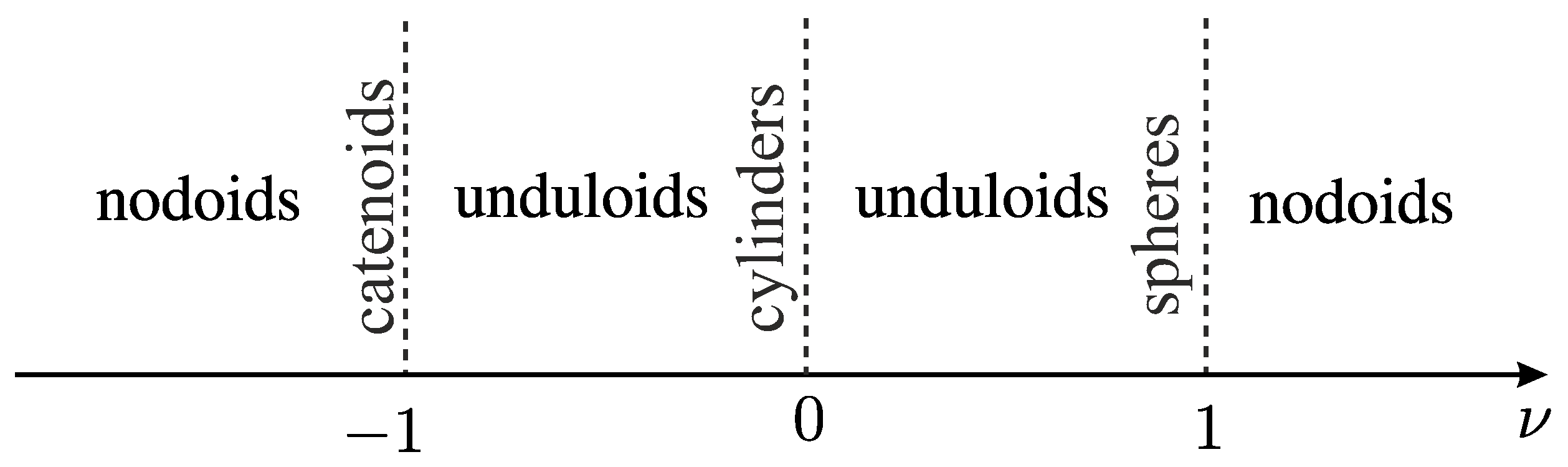
3. Geometry of Delaunay Surfaces
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- 1841 Ch. Delaunay [5] (p. 313)Here and —are three arbitrary real constants. The symmetry axis of the generated surfaces is and their mean curvatures are
- 1886 A. Lane [26] (p. 133)Introducingin which a and b are semi-axes of the ellipse rolling on the line, he presents the respective roulette in the formDelaunay or Sturm are not mentioned in the paper at all.
- 1911 G. Loria [27] (p. 127)The symmetry axis of the surfaces is , a and e are real parameters and s is the natural parameter along the curve. Thenis the equation of the profile curve in which e is the eccentricity of the rolling curve.Loria gives credit about this result to the relatively obscure paper published in 1868 by Spitzer [28], in which he does not mention either Delaunay or Sturm.However on the same page Loria presents also the natural equation of Delaunay curves in the formThe natural equation is listed here for later comparison.
- 1969 M. Hady [29] (pp. 380–381)The profile curve with symmetry axis is parameterized by the formulasin which and C are arbitrary real constants.Neither Delaunay, Sturm or some of the above mentioned people were cited in the paper.
- 1980 K. Kenmotsu [30] (p. 150)The profile curve of CMC surfaces with symmetry axis is presented in the formin which s is the arc-length parameter, H denotes the fixed mean curvature and B is another real constant.An updated version of this work along nice pictures can be found in the book [31].
- 2002 I. Mladenov [32] (pp. 22–24)The derived parameterization of the Delaunay surfaces is of the formin which have the meaning used up to now, , and ± signs refer to unduloids/nodoids.
- 2002 I. Mladenov [33] (p. 329)The symmetry axis in this case is , and denote the complete elliptic integrals of the first, respectively second kind [11,12,13,14]. The parameterization is provided by the formulasThe coefficients of the first and the second fundamental forms for this parameterization are found to beand applying the formula from (42) one immediately obtains that
- 2003 I. Mladenov and J. Oprea [15] (p. 220)The parameterization derived in this paper has the formwhile the coefficients of the first and the second fundamental forms were found to be
- 2004 I. Mladenov [34] (pp. 164–166)This paper presents the derivation of the conformal parameterizations for unduloidsand respectively, nodoidsAn alternative approach for finding such parameterizations can be seen in [35].
- 2008 I. Mladenov, M. Hadzhilazova, P. Djondjorov and V. Vassilev [36] (p. 278)The profile curve which symmetry axis is taken to be , is parameterized via the natural parameter s in the form . Relying on the intrinsic equation of the Delaunay curveswhere is the curvature of the profile curve which explicit form is found to beand this leads toHere and are real parameters with specific ranges and the other ingredients are given by the expressions
- 2014 E. Bendito, M. Bowick and A. Medina [37] (pp. 33–35)If the symmetry axis is , roulettes of the ellipses were found to be described by the formulaswhile that ones of hyperbolas are given by
- 2014 B. Athukorallage, T. Paragoda and M. Toda [38] (pp. 6–10)The parameterization of undulary with symmetry axis is cast into the formand that one corresponding to undulary is given by the formulas
- 2014 V. Pulov, M. Hadzhilazova and I. Mladenov [39] (p. 222)In this paper the reader will find the parameterization of the most interesting cases of Delaunay surfaces, namely—unduloids and nodoids, in terms of the complete set of the Weierstrassian elliptic functions, i.e., Weierstrass p-function , and . Assuming that the -axis coincides with the axis of revolution and the generating curves of the surfaces lie in the -plane, the parameterization of the undularies (upper signs) and nodaries (lower signs) have the formwhereThe above functions are built with the so-called invariants of the Weierstrassian functions given by the expressionsin which and are arbitrary real constants. Here u denotes the argument of which produces the value for , , , and , etc. The mean curvature of the generated unduloids, respectively nodoids, is
- 2017 M. Koiso and B. Palmer [40] (p. 14)Relying on variational arguments the authors have concluded that the surface of revolution with symmetry axis will have CMC if its coordinates are given by the formulasin which H denotes the mean curvature and F is the so-called flux parameter of the surface under consideration.
References
- Klimek, G.; Klimek, M. Discovering Curves and Surfaces with MAPLE; Springer: New York, NY, USA, 1997. [Google Scholar]
- Gray, A. Modern Differential Geometry of Curves and Surfaces with Mathematica®, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1998. [Google Scholar]
- Rovenski, V. Geometry of Curves and Surfaces with Maple; Birkhäuser: New York, NY, USA, 2000. [Google Scholar]
- Oprea, J. Differential Geometry and Its Applications, 2nd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2003. [Google Scholar]
- Delaunay, C. Sur la surface de révolution dont la courbure moyenne est constante. J. Math. Pures Appl. 1841, 6, 309–314. [Google Scholar]
- Sturm, M. Note, à l’occasion de l’article précédent. J. Math. Pures Appl. 1841, 6, 315–320. [Google Scholar]
- Eells, J. The surfaces of Delaunay. Math. Intell. 1987, 9, 53–57. [Google Scholar] [CrossRef]
- Krivoshapko, S.; Ivanov, V. Encyclopedia of Analytical Surfaces; Springer: New York, NY, USA, 2015. [Google Scholar]
- Whittaker, E.; Watson, G. A Course of Modern Analysis, 4th ed.; Cambridge University Press: Cambridge, UK, 1927. [Google Scholar]
- Mladenov, I.; Hadzhilazova, M. The Many Faces of Elastica; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Byrd, P.; Friedman, M. Handbook of Elliptic Integrals for Engineers and Scientists, 2nd ed.; Springer: New York, NY, USA, 1971. [Google Scholar]
- Janhke, E.; Emde, F.; Lösch, F. Tafeln Höherer Funktionen; Teubner Verlag: Stuttgart, Germany, 1960. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. (Eds.) Handbook of Mathematical Functions; Dover: New York, NY, USA, 1972. [Google Scholar]
- Olver, F.; Lozier, D.; Boisvert, R.; Clark, C. The NIST Handbook of Mathematical Functions; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Mladenov, I.; Oprea, J. Unduloids and their closed geodesics. Geom. Integr. Quant. 2003, 4, 206–234. [Google Scholar]
- Pulov, V.; Mladenov, I.M. Rotating liquid drops and Delaunay surfaces. J. Geom. Symmetry Phys. 2019, 54, 55–78. [Google Scholar] [CrossRef]
- Thompson, D.W. On Growth and Form; University Press: Cambridge, UK, 1917; Available online: https://www.gutenberg.org/files/55264/55264-h/55264-h.htm#fig63 (accessed on 5 March 2024).
- Isenberg, C. The Science of Soap Films and Soap Bubbles; Dover: New York, NY, USA, 1992. [Google Scholar]
- Oprea, J. The Mathematics of Soap Films: Explorations with Maple®; AMS: Providence, RI, USA, 2000. [Google Scholar]
- Plateau, J. Statique Expérimetal et Théorique des Liquides Soumis Aux Seules Forces Moléculares, 2 Volumes; Gauthier-Villars: Paris, France, 1873. [Google Scholar]
- Deuling, H.; Helfrich, W. A theoretical explanation for the myelin shapes of red blood cells. Blood Cells 1977, 73, 713–720. [Google Scholar]
- Bar-Ziv, R.; Moses, E. Instability and “pearling” states produced in tubular membranes by competition of curvature and tension. Phys. Rev. Lett. 1994, 73, 1392–1395. [Google Scholar] [CrossRef]
- Perera, B.; Paragoda, T.; Dharmasena, D. A survey of Delaunay surfaces with applications in capillary surfaces. J. Geom. Symmetry Phys. 2022, 64, 51–65. [Google Scholar] [CrossRef]
- Yoneda, M. Tension at the surface of sea-urchin egg: A critical examination of Cole’s Eexpariment. J. Exp. Biol. 1964, 41, 893–906. [Google Scholar] [CrossRef]
- Hadzhilazova, M.; Mladenov, I. Membrane approach to balloons and some related surfaces. Geom. Integr. Quant. 2006, 7, 176–186. [Google Scholar]
- Lane, A. Note on a Roulette. Am. J. Math. 1886, VIII, 132–137. [Google Scholar] [CrossRef]
- Loria, G. Spezielle Algebraische Transzendent Ebene Kurven: Theorie und Geschichte; Teubner Verlag: Leipzig, Germany, 1911. [Google Scholar]
- Spitzer, S. Merkwürdige Eigenschaft derjenigen Curve, welche von Brennpunkte einer Ellipse beschrieben wird, wenn diese auf einer Geraden rollt. Arch. Math. Phys. 1868, VIII, 235–238. [Google Scholar]
- Hady, M. Eine chrakterstische Eigenschaft der Sphäre as rotationsfläche. Math. Nachrichten 1969, 42, 379–385. [Google Scholar] [CrossRef]
- Kenmotsu, K. Surfaces of revolution with prescribed mean curvature. Tôhoku Math. J. 1980, 32, 147–153. [Google Scholar] [CrossRef]
- Kenmotsu, K. Surfaces with Constant Mean Curvature (Translated by Katsuhiro Molriya); AMS: Providence, RI, USA, 2003. [Google Scholar]
- Mladenov, I.M. Delaunay surfaces revisited. C. R. Acad. Sci. 2002, 55, 19–24. [Google Scholar]
- Mladenov, I.M. New solutions of the shape equation. Eur. Phys. J. B 2002, 29, 327–330. [Google Scholar] [CrossRef]
- Mladenov, I.M. Conformal immersions of Delaunay surfaces and their duals. Geom. Integr. Quant. 2004, 5, 158–168. [Google Scholar]
- Bracken, P. Delaunay surfaces expressed in terms of a Cartan moving frame. J. Appl. Anal. 2020, 26, 153–160. [Google Scholar] [CrossRef]
- Mladenov, I.; Hadzhilazova, M.; Djondjorov, P.; Vassilev, V. On the intrinsic equation behind the Delaunay surfaces. AIP Conf. Proc. 2008, 1079, 274–280. [Google Scholar]
- Bendito, E.; Bowick, M.; Medina, A. A natural parameterization of the roulettes of the conics generating the Delaunay surfaces. J. Geom. Symmetry Phys. 2014, 33, 27–45. [Google Scholar]
- Athukorallage, B.; Paragoda, T.; Toda, M. Roulettes of conics, Delaunay surfaces and applications. Surv. Math. Math. Sci. 2014, 4, 1–23. [Google Scholar]
- Pulov, V.; Hadzhilazova, M.; Mladenov, I.M. Delaunay surfaces in terms of Weierstrassian functions. In Proceedings of the International Conference on Integrability, Recursion Operators and Soliton Interactions, Sofia, Bulgaria, 29–31 August 2012; Aneva, B., Grahovski, G., Ivanov, R., Mladenov, D., Eds.; Avangard Prima: Sofia, Bulgaria, 2014; pp. 218–224. [Google Scholar]
- Koiso, M.; Palmer, B. Higher order variations of constant mean curvature surfaces. Calc. Var. 2017, 56, 159. [Google Scholar] [CrossRef]

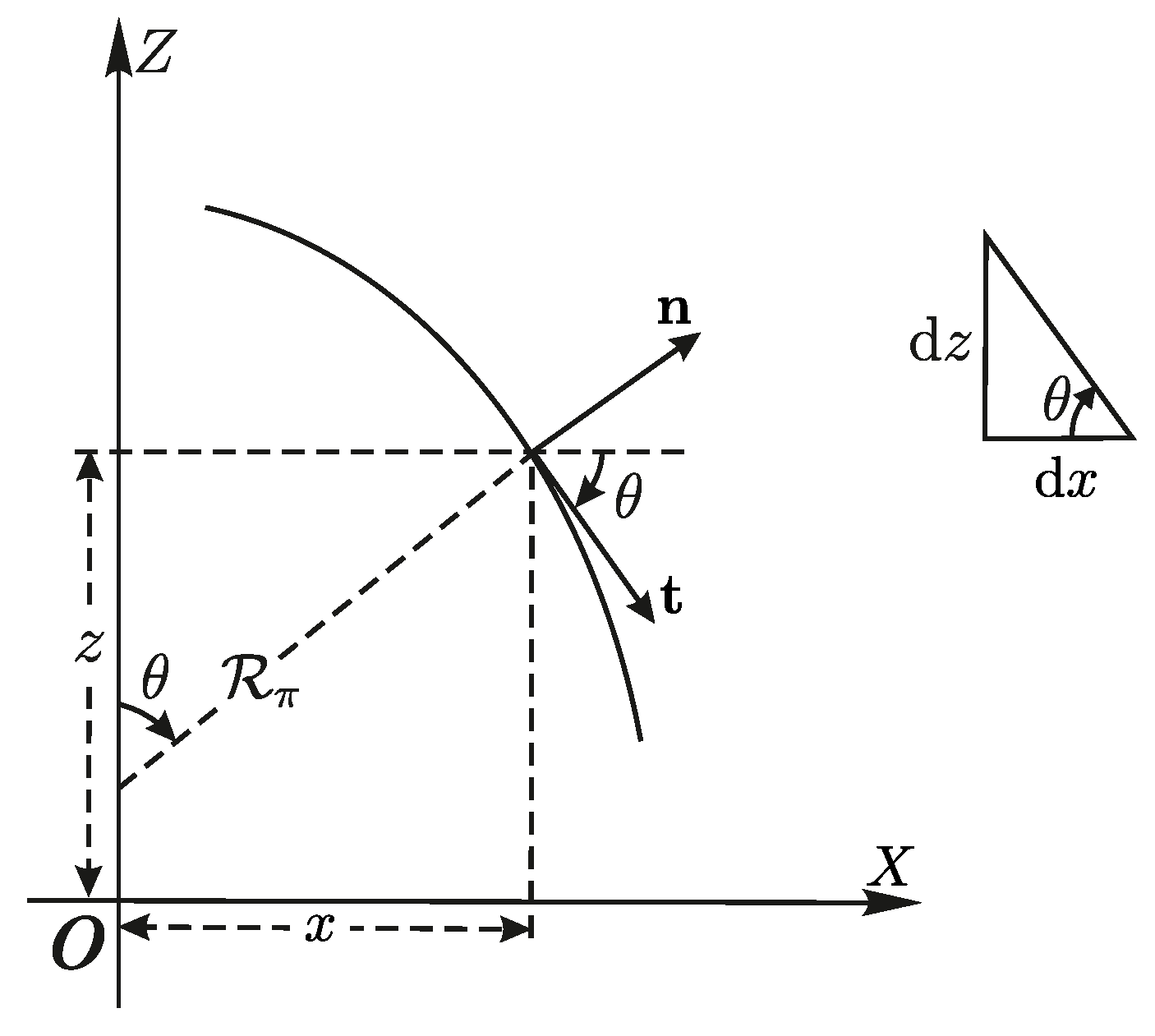




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mladenova, C.D.; Mladenov, I.M. Parameterizations of Delaunay Surfaces from Scratch. Mathematics 2024, 12, 1570. https://doi.org/10.3390/math12101570
Mladenova CD, Mladenov IM. Parameterizations of Delaunay Surfaces from Scratch. Mathematics. 2024; 12(10):1570. https://doi.org/10.3390/math12101570
Chicago/Turabian StyleMladenova, Clementina D., and Ivaïlo M. Mladenov. 2024. "Parameterizations of Delaunay Surfaces from Scratch" Mathematics 12, no. 10: 1570. https://doi.org/10.3390/math12101570
APA StyleMladenova, C. D., & Mladenov, I. M. (2024). Parameterizations of Delaunay Surfaces from Scratch. Mathematics, 12(10), 1570. https://doi.org/10.3390/math12101570





