The Connection between Der(${\mathbf{U}}_{\mathbf{q}}^{\mathbf{+}}\mathbf{\left(}\mathbf{g}\mathbf{\right)}$) and Der(${\mathbf{U}}_{\mathbf{r}\mathbf{,}\mathbf{s}}^{\mathbf{+}}\mathbf{\left(}\mathbf{g}\mathbf{\right)}$)

Abstract

To facilitate the parallel development of the structures and properties of two-parameter quantum groups with those of one-parameter quantum groups, this paper primarily elucidates the interrelations and distinctions between the derivation algebras of these two types of quantum groups. Additionally, we also proposed a method for deriving the derivation algebra of one-parameter quantum groups from known two-parameter quantum group derivations, and vice versa.

1. Introduction

In 1985, Drinfel’d introduced the concept of quantum groups while investigating the quantum Yang–Baxter equation [1]. Over the past three decades, scholars have extensively explored and demonstrated the significant influence of quantum group theory across diverse domains of mathematics and physics. In 1990, Kulish introduced a two-parameter quantum group utilizing the constant solution of the Yang–Baxter equation.

Benkart and Witherspoon studied the two-parameter quantum groups $U_{r,s}\left(g{l}_n\right)$ and $U_{r,s}\left(s{l}_n\right)$ through down–up algebra [2,3]. Bergeron, Gao and Hu extended this analysis to two-parameter quantum orthogonal and symplectic groups [4,5]. Subsequently, Hu and Shi investigated the two-parameter quantum group for exceptional types $G_2$ [6]; Hu and Wang further developed the $B_n$-type [7].

In particular, Hu and Pei presented a simplified definition for a class of two-parameter quantum groups $U_{r,s}$, demonstrating that the two-parameter quantum groups $U_{r,s}\left(\mathfrak{g}\right)$ can be obtained from the one-parameter quantum group $U_{q,q^{-1}}\left(\mathfrak{g}\right)$ by twisting the multiplication via an explicit Hopf 2-cocycle $\sigma$ [8,9].

In 2015, building on Lusztig’s work, Fan and Li constructed another version of the two-parameter quantum group using simple perverse sheaves [10]. This geometric construction provides a new representation of the generators and generation relationships of two-parameter quantum group $U_{v,t}$.

In the study of quantum groups, derivations may be used to describe some deformation or evolution of quantum groups, or to reveal relationships between quantum groups and other algebraic structures. In this paper, our main focus is to explore the connection between derivative algebras of one-parameter quantum groups and derivation algebras of two-parameter quantum groups, where one-parameter and two-parameter quantum groups correspond to simple Lie algebras of the same finite type. Unlike previous studies of derivation algebra of a quantum group, a key contribution of this study is the establishment of the structure of derivation algebra of an iterated Ore extension, so that through this structure, we can obtain the derivation algebra of any quantum group, thus building a bridge for understanding the relationship between the derivative algebras of single-parameter quantum groups and two-parameter quantum groups.

In Section 4, we initially derive the corresponding one-parameter quantum group derivation algebra from the derivation algebra of the two-parameter quantum group. This process is relatively straight forward. Conversely, constructing a two-parameter quantum group from a one-parameter quantum group is complex, thus making it challenging to construct the derivation algebra of a two-parameter quantum group from that of a one-parameter quantum group. Finally, we employ the method proposed by Fan and Li to construct two-parameter quantum groups from one-parameter quantum groups. Building upon this approach, we derive the derivation algebra of a two-parameter quantum group from that of a one-parameter quantum group.

2. Preliminaries

Before delving into the main topic of this article, we will first review some pertinent preparatory knowledge to ensure that readers are equipped for subsequent discussions. In this section, we fix the notations that will be used throughout this paper. For any $n,k,v>0$, let

$$\begin{array}{cccc}\hfill & {\left(n\right)}_v={\displaystyle \frac{v^n-1}{v-1}},\hfill & \hfill & {\left[n\right]}_v={\displaystyle \frac{v^n-v^{-n}}{v-v^{-1}}}\hfill \\ & {\left(n\right)}_v!={\left(1\right)}_v\cdots {\left(n\right)}_v,\hfill & \hfill & {\left[n\right]}_v!={\left[1\right]}_v\cdots {\left[n\right]}_v\hfill \\ \hfill & {\left(\begin{array}{c}n\\ k\end{array}\right)}_v={\displaystyle \frac{{\left(n\right)}_v!}{{\left(k\right)}_v!{(n-k)}_v!}},\hfill & \hfill & {\left[\begin{array}{c}n\\ k\end{array}\right]}_v={\displaystyle \frac{{\left[n\right]}_v!}{{\left[k\right]}_v!{[n-k]}_v!}}\hfill \end{array}$$

and ${\left[0\right]}_v!=1$, ${\left(0\right)}_v!=1$.

2.1. The Algebras $U_q\left(\mathfrak{g}\right)$ and $U_{r,s}\left(\mathfrak{g}\right)$

This subsection introduces the definitions of one-parameter quantum groups and two-parameter quantum groups, which will serve as the foundation for our subsequent research and analysis.

Let $\mathfrak{g}$ be a finite-dimensional simple Lie algebra over a field $\mathbb{C}$ and $A={\left(a_{ij}\right)}_{n\times n}$ be an associated Cartan matrix, there exists a diagonal matrix $D=\mathrm{diag}(d_1,\cdots ,d_n)$ such that $d_i\in \mathbb{Z}\backslash \left\{0\right\}$ and $DA={\left(DA\right)}^T$. Let $q\in {\mathbb{C}}^{*}$ be such that q is not a unit root.

Definition 1.

The one-parameter quantum group $U_q\left(\mathfrak{g}\right)$ is a unital associative algebra over $\mathbb{C}$ by generators $E_i$, $F_i$, $K_i^{\pm 1}$$(1\le i\le n)$, and relations

$$\begin{array}{cc}\hfill \left(\mathrm{A}1\right)& K_i{K}_i^{-1}=K_i^{-1}{K}_i=1,K_i{K}_j=K_j{K}_i\hfill \\ \hfill \left(\mathrm{A}2\right)& K_i{E}_i{K}_i^{-1}=q^{d_i{a}_{ij}}{E}_j,K_i{F}_i{K}_i^{-1}=q^{d_i{a}_{ij}}{F}_j\hfill \\ \hfill \left(\mathrm{A}3\right)& E_i{F}_j-F_j{E}_i=\delta {\displaystyle \frac{K_i^2-K_i^{-2}}{q^{2d_i}-q^{-2d_i}}}\hfill & \hfill & \\ \hfill \left(\mathrm{A}4\right)& \sum _{v=0}^{1-a_{ij}}{(-1)}^v{\left[\begin{array}{c}1-a_{ij}\\ v\end{array}\right]}_{q^{2d_i}}{E}_i^{1-a_{ij}-v}{E}_j{E}_i^v=0(i\ne j)\hfill \\ \hfill \left(\mathrm{A}5\right)& \sum _{v=0}^{1-a_{ij}}{(-1)}^v{\left[\begin{array}{c}1-a_{ij}\\ v\end{array}\right]}_{q^{2d_i}}{F}_i^{1-a_{ij}-v}{F}_j{F}_i^v=0(i\ne j)\hfill \end{array}$$

The above content described the generators and generating relations for one-parameter quantum groups. Next, let us review the generators and generating relations for two-parameter quantum groups.

Let $\mathrm{\Pi }=\left\{{\alpha }_i\right|i\in I\}$ be the set of simple roots, $\mathsf{\Phi }$ be the set of roots and ${\mathsf{\Phi }}^{+}$ positive roots. Let $\mathbb{Q}(r,s)$ be the rational functions field in two variables $r,s$ over $\mathbb{Q}$. Let $r_i=r^{d_i}$, $s_i=s^{d_i}$ for $i\in I$ and $⟨-,-⟩$ be the Euler bilinear form on $\mathbb{Z}$ defined by

$$⟨i,j⟩:=⟨{\alpha }_i,{\alpha }_j⟩=\left\{\begin{array}{cc}{d}_i{a}_{ij}\hfill & i<j\hfill \\ d_i\hfill & i=j\hfill \\ 0\hfill & i>j\hfill \end{array}\right.$$

(1)

Definition 2.

The two-parameter quantum group $U_{r,s}\left(\mathfrak{g}\right)$ is a unital associative algebra over $\mathbb{Q}(r,s)$ generated by $e_i$, $f_i$, ${\omega }_i^{\pm 1}$, ${{\omega }_i^{\prime }}^{\pm 1}$$(1\le i\le n)$, subject to the relations:

$$\begin{array}{cc}\hfill \left(\mathrm{B}1\right)& {\omega }_i^{\pm 1}{\omega }_j^{\pm 1}={\omega }_j^{\pm 1}{\omega }_i^{\pm 1},{{\omega }_i^{\prime }}^{\pm 1}{{\omega }_j^{\prime }}^{\pm 1}={{\omega }_j^{\prime }}^{\pm 1}{{\omega }_i^{\prime }}^{\pm 1}\hfill \\ \hfill & {\omega }_i^{\pm 1}{{\omega }_j^{\prime }}^{\pm 1}={{\omega }_j^{\prime }}^{\pm 1}{{\omega }_i}^{\pm 1},{{\omega }_i}^{\pm 1}{{\omega }_i}^{\mp 1}={{\omega }_i^{\prime }}^{\pm 1}{{\omega }_i^{\prime }}^{\mp 1}\hfill \\ \hfill \left(\mathrm{B}2\right)& {\omega }_i{e}_j{\omega }_i^{-1}=r^{⟨j,i⟩}{s}^{-⟨i,j⟩}{e}_j,{\omega }_i^{\prime }{e}_j{{\omega }_i^{\prime }}^{-1}=r^{-⟨i,j⟩}{s}^{⟨j,i⟩}{e}_j\hfill \\ \hfill & {\omega }_i{f}_j{\omega }_i^{-1}=r^{-⟨j,i⟩}{s}^{⟨i,j⟩}{f}_j,{\omega }_i^{\prime }{f}_j{{\omega }_i^{\prime }}^{-1}=r^{⟨i,j⟩}{s}^{-⟨j,i⟩}{f}_j\hfill \\ \hfill \left(\mathrm{B}3\right)& e_i{f}_j-f_j{e}_i={\delta }_{i,j}{\displaystyle \frac{{\omega }_i-{\omega }_i^{\prime }}{v_i-v_i^{-1}}}\hfill \\ \hfill \left(\mathrm{B}4\right)& \sum _{k=0}^{1-a_{ij}}{(-1)}^k{\left(\begin{array}{c}1-a_{ij}\\ k\end{array}\right)}_{r_i{s}_i^{-1}}{c}_{ij}^{\left(k\right)}{e}_i^{1-a_{ij}-k}{e}_j{e}_i^k=0i\ne j\hfill \\ \hfill \left(\mathrm{B}5\right)& \sum _{k=0}^{1-a_{ij}}{(-1)}^k{\left(\begin{array}{c}1-a_{ij}\\ k\end{array}\right)}_{r_i{s}_i^{-1}}{c}_{ij}^{\left(k\right)}{f}_i^k{f}_j{f}_i^{1-a_{ij}-k}=0i\ne j\hfill \end{array}$$

Let $U_q^{+}\left(\mathfrak{g}\right)$ (resp., $U_q^{-}\left(\mathfrak{g}\right)$) be the subalgebra of $U_q\left(\mathfrak{g}\right)$ generated by the elements $E_i$ (resp., $F_i$) for $1\le i\le n$, and $U_q^0\left(\mathfrak{g}\right)$ is subalgebra of $U_q\left(\mathfrak{g}\right)$ generated by $K_i^{\pm 1}$ for $1\le i\le n$. Moreover, we know

$$U_q\left(\mathfrak{g}\right)\cong U_q^{+}\left(\mathfrak{g}\right)\otimes U_q^0\left(\mathfrak{g}\right)\otimes U_q^{-}\left(\mathfrak{g}\right)$$

Similary, Let $U_{r,s}^{+}\left(\mathfrak{g}\right)$ (resp., $U_{r,s}^{-}\left(\mathfrak{g}\right)$) be the subalgebra of $U_{r,s}\left(\mathfrak{g}\right)$ generated by the elements $e_i$ (resp., $f_i$) for $1\le i\le n$, and $U_{r,s}^0$ the subalgebra of $U_{r,s}\left(\mathfrak{g}\right)$ generated by ${\omega }_i^{\pm 1}$, ${{\omega }_i^{\prime }}^{\pm 1}$ for $1\le i\le n$, we have

$$U_{r,s}\left(\mathfrak{g}\right)\cong U_{r,s}^{+}\left(\mathfrak{g}\right)\otimes U_{r,s}^0\left(\mathfrak{g}\right)\otimes U_{r,s}^{-}\left(\mathfrak{g}\right)$$

In this paper, our primary focus is on the derivation algebras of the positive parts of both one-parameter and two-parameter quantum groups.

2.2. Iterated Ore Extension

This subsection will furnish the definitions and properties of iterative Ore extensions, crucial for the subsequent discussion regarding the derivation algebra of quantum groups.

Let R be a ring. An Ore extension $R[x,\sigma ,\delta ]$ is a ring with elements of the form $f\left(x\right)={\sum }_{i=0}^n{a}_i{x}^i$, $a_i\in R$ and multiplication satisfying $xr=\sigma \left(r\right)x+\delta \left(r\right)$ for all $r\in R$, where $\sigma$ is an endomorphism of R and $\delta$ is a $\sigma$-derivation of R, i.e.,

$$\delta \left(r_1{r}_2\right)=\sigma \left(r_1\right)\delta \left(r_2\right)+\delta \left(r_1\right)r_2,forall{r}_1,r_2\in R$$

Definition 3.

An iterated Ore extension $R[x_1,{\sigma }_1,{\delta }_1]\cdots [x_n,{\sigma }_n,{\delta }_n]$ is an Ore extension where for all $j\ge 1$, ${\sigma }_j$ and ${\delta }_j$ are a ring endomorphism and a ${\sigma }_j$-derivation of $R_{(j-1)}:=R[x_1,{\sigma }_1,{\delta }_1]$⋯$[x_{j-1},{\sigma }_{j-1},{\delta }_{j-1}]$. Elements in this extension have the form ${\sum }_{i=0}^n{a}_i{x}_1^{i_1}\cdots x_n^{i_n}$, $a_i\in R$.

Let us review the concept of quantum root vectors in quantum groups and the exchange relationship.

Let $\mathfrak{g}$ be a finite dimensional simple Lie algebra, ${\mathsf{\Phi }}^{+}$ be a positive root system of the complex simple Lie algebra of $\mathfrak{g}$ and $|{\mathsf{\Phi }}^{+}|=n$. The following lemma holds.

Lemma 1

([11]). For each of the trees in Figure 1, the set of words determined by the Lyndon paths in the tree is the complete set of standard Lyndon words for the corresponding finite-dimensional simple Lie algebra.

Figure 1. The tree given standard Lyndon paths. The root of each tree is the leftmost vertex.

Assuming that ${\beta }_i,{\beta }_j\in {\mathsf{\Phi }}^{+}$, ${\mathcal{X}}_i$ and ${\mathcal{X}}_j$ are the quantum root vectors corresponding to ${\beta }_i$ and ${\beta }_j$, respectively. We have that $U_q^{+}\left(\mathfrak{g}\right)$ consists of quantum root vectors ${\mathcal{X}}_1,\cdots ,{\mathcal{X}}_n$ and the set of products $\{{\mathcal{X}}_1^{a_1}\cdots {\mathcal{X}}_n^{a_n}|a_i\in \mathbb{Z}\}$ forms a basis for $U_q^{+}\left(\mathfrak{g}\right)$. If i and j are two integers such that $1\le i<j\le n$, then we can obtain

$$\begin{array}{c}\hfill {\mathcal{X}}_i{\mathcal{X}}_j-q^{(i,j)}{\mathcal{X}}_j{\mathcal{X}}_i=\sum _{k=(k_{i+1},\cdots ,k_{j-1})}{c}_k{\mathcal{X}}_{i+1}^{k_{i+1}}\cdots {\mathcal{X}}_{j-1}^{k_{j-1}}\end{array}$$

(2)

where $c_k\in \mathbb{C}$, $q^{(i,j)}:=q^{({\beta }_i,{\beta }_j)}$, and $({\beta }_i,{\beta }_j)$ is the inner product of ${\beta }_i$ and ${\beta }_j$. Similarly, $X_i$ and $X_j$ are the quantum root vectors corresponding to ${\beta }_i$ and ${\beta }_j$, respectively. The two-parameter quantum group $U_{r,s}^{+}\left(\mathfrak{g}\right)$ contains a set of quantum root vector and the products $\{X_1^{a_1}\cdots X_n^{a_n}|a_i\in \mathbb{Z}\}$ form a basis of $U_{r,s}^{+}\left(\mathfrak{g}\right)$. If i and j are two integers such that $1\le i<j\le l$, then we can obtain

$$\begin{array}{c}\hfill X_i{X}_j-({\omega }_i^{\prime },{\omega }_j)X_j{X}_i=\sum _{k=(k_{i+1},\cdots ,k_{j-1})}{e}_k{X}_{i+1}^{k_{i+1}}\cdots X_{j-1}^{k_{j-1}}\end{array}$$

(3)

where $e_k\in \mathbb{C}$, $({\omega }_i^{\prime },{\omega }_j)=r^{⟨{\beta }_i,{\beta }_j⟩}{s}^{-⟨{\beta }_j,{\beta }_i⟩}$.

Based on the Definition 3, in conjunction with Equations (2) and (3), it is evident that the following lemma is valid.

Lemma 2.

$U_q^{+}\left(\mathfrak{g}\right)$ and $U_{r,s}^{+}\left(\mathfrak{g}\right)$ are two skew polynomial rings, which can be expressed as

$$\begin{array}{cc}\hfill U_q^{+}\left(\mathfrak{g}\right)& =\mathbb{C}\left[{\mathcal{X}}_1\right][{\mathcal{X}}_2;{\sigma }_2,{\delta }_2]\cdots [{\mathcal{X}}_l;{\sigma }_l,{\delta }_l]\hfill \\ \hfill U_{r,s}^{+}\left(\mathfrak{g}\right)& =\mathbb{C}\left[X_1\right][X_2;{\sigma }_2^{\prime },{\delta }_2^{\prime }]\cdots [X_l;{\sigma }_l^{\prime },{\delta }_l^{\prime }]\hfill \end{array}$$

where the ${\sigma }_j$’s are $\mathbb{C}$-linear automorphisms and the ${\delta }_j$’s are $\mathbb{C}$-linear ${\sigma }_j$-derivations such that for $1\le i<j\le l$, ${\sigma }_j\left({\mathcal{X}}_i\right)=q^{(i,j)}{\mathcal{X}}_i$ and ${\delta }_j\left({\mathcal{X}}_i\right)={\sum }_{k=(k_{i+1},\cdots ,k_{j-1})}{c}_k{\mathcal{X}}_{i+1}^{k_{i+1}}\cdots {\mathcal{X}}_{j-1}^{k_{j-1}}$; for $1\le i<j\le l$, ${\sigma }_j^{\prime }\left(X_i\right)=⟨{\omega }_i^{\prime },{\omega }_j⟩X_i$ and ${\delta }_j^{\prime }\left(X_i\right)={\sum }_{k=(k_{i+1},\cdots ,k_{j-1})}{e}_k{X}_{i+1}^{k_{i+1}}\cdots X_{j-1}^{k_{j-1}}$.

In [9], Hu and Pei offer a more straightforward definition for a class of two-parameter quantum groups, denoted as $U_{r,s}\left(\mathfrak{g}\right)$, associated with semisimple Lie algebras. They do so by employing the Euler form and further elucidate the connection between two-parameter quantum groups and the one-parameter Drinfel’d–Jimbo quantum group. More specifically, In the subsequent lemma, they detail the method of deriving a one-parameter quantum group from a two-parameter one by adjusting the parameters.

Lemma 3.

Let $r=q$, $s=q^{-1}$. Then, $U_{q,q^{-1}}\left(\mathfrak{g}\right)$ modulo is the Hopf ideal generated by ${\omega }_i^{\prime }-{\omega }_i^{-1}$$(i\in [1,n\left]\right)$ is isomorphic to the standard one-parameter quantum group $U_q\left(\mathfrak{g}\right)$.

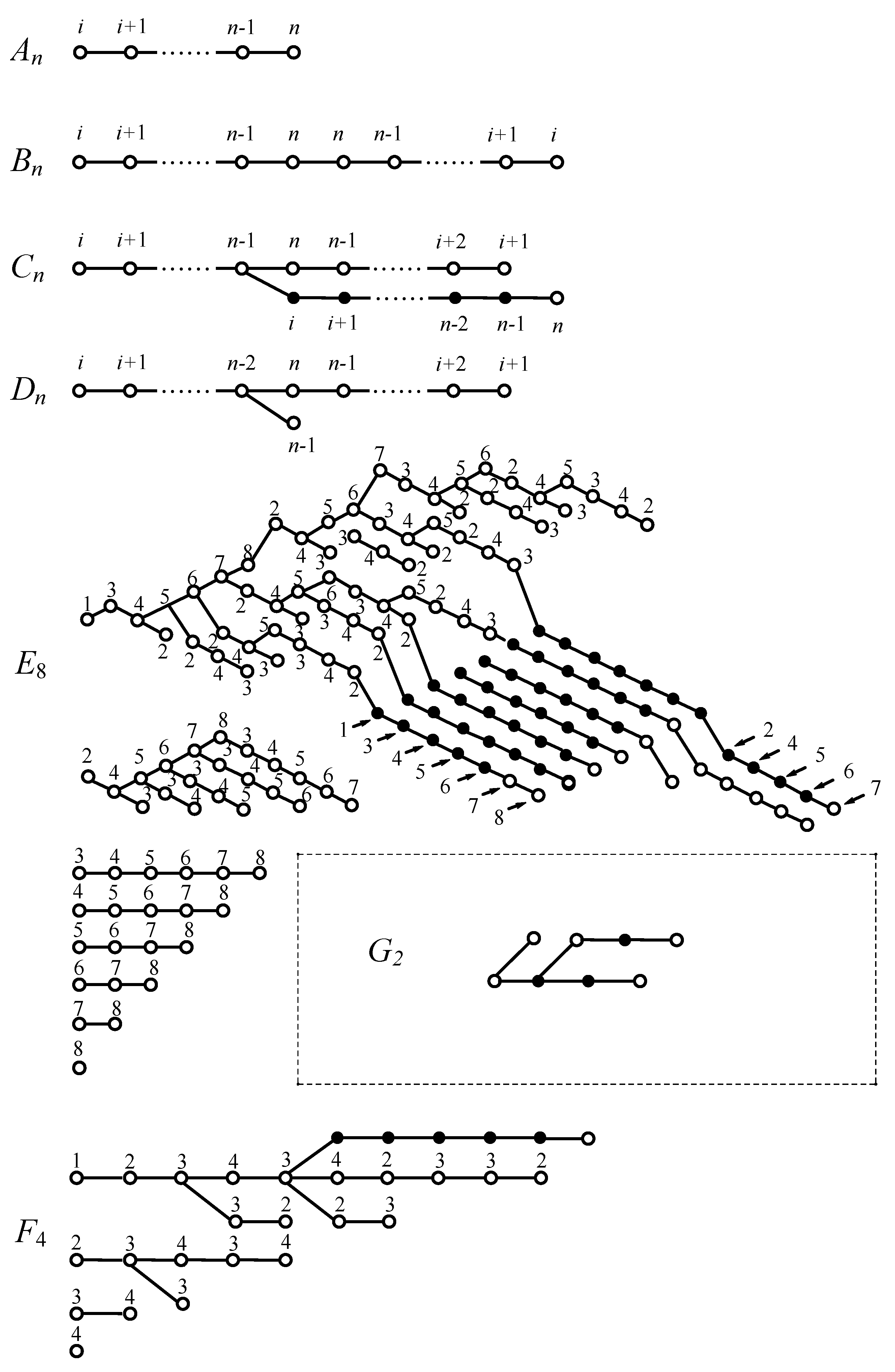
3. Derivations of Iterated Ore Extension

Drawing on the foundational principles outlined in Section 2, it is clear that both one-parameter quantum groups and two-parameter quantum groups can be characterized as iterated Ore extensions. As a result, the central objective of this section is to clarify the structural underpinnings of the derivative algebra associated with iterative Ore extensions. This elucidation forms a crucial basis for delving deeper into the relationships between the derivation algebra of one-parameter quantum groups and two-parameter quantum groups.

Definition 4

([12]). Let R be an algebra over the field $\mathbb{F}$, and δ be a linear transformation over R. If δ satisfies the following conditions:

$$\begin{array}{c}\hfill \delta (r+s)=\delta \left(r\right)+\delta \left(s\right),\forall r,s\in R,\\ \hfill \delta \left(rs\right)=\delta \left(r\right)s+r\delta \left(s\right).\forall r,s\in R,\end{array}$$

then δ is called a derivation of R.

Example 1.

Let R be a polynomial ring over the complex field $\mathbb{C}$, δ be a mapping over R, and satisfying the following:

$$\delta \left(\sum _i{a}_i{X}^i\right)=\sum _{i}i{a}_i{X}^{i-1},$$

so δ is a derivative on R.

Let $R_n:=\mathbb{C}\left[X_1\right][X_2;{\sigma }_2,{\delta }_2]\cdots [X_n;{\sigma }_n,{\delta }_n]$ be a special type of iterated Ore extension, for $1\le j<i\le n$,

$$\begin{array}{c}\hfill X_j{X}_i-\lambda (X_j,X_i)X_i{X}_j=P(X_j,X_i),\end{array}$$

(4)

where $P(X_j,X_i)={\sum }_{\overline{k}=k_{i+1},\cdots ,k_{j-1}}{c}_{\overline{k}}{X}_{i+1}^{k_{i+1}}\cdots X_{j-1}^{k_{j-1}}$. It is worth noting that $U_{r,s}^{+}\left(\mathfrak{g}\right)$ and $U_q^{+}\left(\mathfrak{g}\right)$ are all iterated Ore extensions of this type.

We give a mapping $a_r$, which is mapped from $R_n$ to $\mathbb{C}$ and satisfies $a_{X_i^k+X_j^l}(X_i^k+X_j^l)$ = $2^{k-1}{a}_{X_i}\left(X_i\right)+2^{l-1}{a}_{X_j}\left(X_j\right)$

Theorem 1.

If $R_n$ is a special type of iterated Ore extension, then the derivation of $R_n$ can be uniquely written in the following form:

$$D\left(X_i\right)=a_{X_i}\left(X_i\right)X_i,$$

for some $r\in R_n$, and where $a_{X_i}\left(X_i\right)\in \mathbb{C}$ and

$$\begin{array}{c}\hfill a_{X_i}\left(X_i\right)+a_{X_j}\left(X_j\right)=a_{P(X_j,X_i)}\left(P(X_j,X_i)\right).\end{array}$$

(5)

Proof. 

Let D be the derivation of $R_n$; we have

$$\begin{array}{c}\hfill D\left(y\right)=\sum _r{a}_r\left(y\right)r,\end{array}$$

(6)

where $a_r$ is the mapping from $R_n$ to $\mathbb{C}$. In fact, we know $X_j{X}_i-\lambda (X_j,X_i)X_i{X}_j=P(X_j,X_i)$ for $P(X_j,X_i)={\sum }_{\overline{k}=k_{i+1},\cdots ,k_{j-1}}$$c_{\overline{k}}{X}_{i+1}^{k_{i+1}}\cdots X_{j-1}^{k_{j-1}}$. Based on the definition of derivations, there is

$$\begin{array}{cc}\hfill D\left(X_j{X}_i\right)& =D\left(X_j\right)X_i+X_{j}D\left(X_i\right)\hfill \\ \hfill & =\sum _r{a}_r\left(X_j\right)r{X}_i+\sum _r{a}_r\left(X_i\right)\lambda (X_j,r)r{X}_j+\sum _r{a}_r\left(X_i\right)P(X_j,r).\hfill \end{array}$$

(7)

Since D is a bilinear mapping; thus, we have

$$\begin{array}{cc}\hfill D\left(X_j{X}_i\right)=& \lambda (X_j,X_i)D\left(X_i{X}_j\right)+D\left(P(X_j,X_i)\right)\hfill \\ \hfill =& \lambda (X_j,X_i)\left(\sum _r{a}_r\left(X_i\right)r{X}_j+\sum _r{a}_r\left(X_j\right)\lambda (X_i,r)r{X}_i+\sum _r{a}_r\left(X_j\right)P(X_i,r)\right)\hfill \\ \hfill & +\sum _r{a}_r\left(P(X_j,X_i)\right)r,\hfill \end{array}$$

(8)

then $P(X_j,X_i)$ does not include $X_j{X}_i$, so that

$$\begin{array}{cc}\hfill \sum _r{a}_r\left(X_i\right)\lambda (X_j,r)r{X}_j& =\sum _r{a}_r\left(X_i\right)\lambda (X_j,X_i)r{X}_j,\hfill \end{array}$$

(9)

$$\begin{array}{cc}\hfill \sum _r{a}_r\left(X_j\right)r{X}_i& =\sum _r{a}_r\left(X_j\right)\lambda (X_j,X_i)\lambda (X_i,r)r{X}_i,\hfill \end{array}$$

(10)

$$\begin{array}{cc}\hfill \sum _r{a}_r\left(X_i\right)P(X_j,r)& =\sum _r{a}_r\left(X_j\right)\lambda (X_j,X_i)P(X_i,r))+\sum _r{a}_r\left(P(X_j,X_i)\right)r.\hfill \end{array}$$

(11)

From this, by comparing coefficients of (9), when $r=X_i$, there is $a_{X_i}\left(X_i\right)\lambda (X_j,X_i)X_i{X}_j=a_{X_i}\left(X_i\right)\lambda (X_j,X_i)X_i{X}_j$, and when $r\ne X_i$, there is $a_r\left(X_i\right)=0$. Similarly, in Equation (10), when $r=X_j$, $a_{X_j}\left(X_j\right)X_j{X}_i=a_{X_j}\left(X_j\right)\lambda (X_j,X_i)\lambda (X_i,X_j)X_j{X}_i$ holds, and when $r\ne X_j$, $a_r\left(X_j\right)=0$.

In Equation (11), there is $a_{X_i}\left(X_i\right)P(X_j,X_i)=a_{X_j}\left(X_j\right)\lambda (X_j,X_i)P(X_i,X_j)+$$a_{P(X_j,X_i)}P(X_j,X_i)$. Since $P(X_i,X_j)=-\lambda {(X_j,X_i)}^{-1}P(X_j,X_i)$, we obtain

$$\begin{array}{c}\hfill a_{X_i}\left(X_i\right)=-a_{X_j}\left(X_j\right)+a_{P(X_i,X_j)}\left(P(X_i,X_j)\right).\end{array}$$

Under the above results, we further discuss the action of mapping $a_{P(X_i,X_j)}\left(P(X_i,X_j)\right)$. We can characterize $a_{P(X_i,X_j)}\left(P(X_i,X_j)\right)$ in two situations.

We first suppose that $a_{P(X_i,X_j)}\left(P(X_i,X_j)\right)$ contains only one generator and its exponent is 1, i.e., $X_j{X}_i=\lambda (X_j,X_i)X_i{X}_j+c_k{X}_k$ for $1\le i<k<j\le n$. Therefore, we would have

$$\begin{array}{c}\hfill a_{X_i}\left(X_i\right)=-a_{X_j}\left(X_j\right)+a_{X_k}\left(X_k\right).\end{array}$$

Next, we will prove by using mathematical induction that $D\left(X_i^k\right)=2^{k-1}{a}_{X_i}\left(X_i\right)X_i^k$.

$$\begin{array}{cc}\hfill D\left(X_i^2\right)& =D\left(X_i\right)X_i+X_{i}D\left(X_i\right)\hfill \\ \hfill & =2a_{X_i}\left(X_i\right)X_i^2.\hfill \end{array}$$

Suppose that $D\left(X_i^{k-1}\right)=2^{k-2}{a}_{X_i}\left(X_i\right)X_i^{k-1}$ for $k\in \left[\right[1,n\left]\right]$, then

$$\begin{array}{cc}\hfill D\left(X_i^k\right)& =D\left(X_i^{k-1}\right)X_i+X_{i}D\left(X_i^{k-1}\right)\hfill \\ \hfill & =2^{k-2}{a}_{X_i}\left(X_i\right)X_i^{k-1}{X}_i+X_{i}2a_{X_i}\left(X_i\right)X_i^{k-2}\hfill \\ \hfill & =2^{k-1}{a}_{X_i}\left(X_i\right)X_i^k,\hfill \end{array}$$

it can be concluded that $a_{X_i^k}\left(X_i^k\right)=2^{k-1}{a}_{X_i}\left(X_i\right)$. We thus obtain

$$\begin{array}{c}\hfill D(\sum _{\overline{k}}{c}_{\overline{k}}{X}_{i+1}^{k_{i+1}}\cdots X_{j-1}^{k_{j-1}})=\sum _{\overline{k}}{c}_{\overline{k}}\left(2^{k_{i+1}-1}{a}_{X_{i+1}}\left(X_{i+1}\right)+\cdots +2^{k_{j-1}-1}{a}_{X_{j-1}}\left(X_{j-1}\right)\right)X_{i+1}^{k_{i+1}}\cdots X_{j-1}^{k_{j-1}},\end{array}$$

where $\overline{k}=k_{i+1},\cdots ,k_{j-1}$. The proof is complete. □

4. The Relationship between $\mathbf{Der}\mathbf{\left(}{\mathbf{U}}_{\mathbf{q}}^{\mathbf{+}}\mathbf{\left(}\mathfrak{g}\mathbf{\right)}\mathbf{\right)}$ and $\mathbf{Der}\mathbf{\left(}{\mathbf{U}}_{\mathbf{r}\mathbf{,}\mathbf{s}}^{\mathbf{+}}\mathbf{\left(}\mathfrak{g}\mathbf{\right)}\mathbf{\right)}$

In this section, we delve into the structure of the derivative algebra of one-parameter quantum groups and two-parameter quantum groups. This exploration is aimed at inspiring further research on the relationship between the derivations of these two types of quantum groups.

4.1. Derivations of Quantum Groups

As $U_q^{+}\left(\mathfrak{g}\right)$ and $U_{r,s}^{+}\left(\mathfrak{g}\right)$ are iterated Ore extensions, this means that the derivations of $U_q^{+}\left(\mathfrak{g}\right)$ or $U_{r,s}^{+}\left(\mathfrak{g}\right)$ have the following structure: $D\left(x_i\right)=a_i{x}_i$, where $x_i$ is the quantum root vector of $U_q^{+}\left(\mathfrak{g}\right)$ or $U_{r,s}^{+}\left(\mathfrak{g}\right)$.

For the convenience of subsequent operations, we first provide a proposition.

Proposition 1.

Let $Z\left(U_{r,s}^{+}\left(\mathfrak{g}\right)\right)$ be the center of $U_{r,s}^{+}\left(\mathfrak{g}\right)$ and D be a derivation of $U_{r,s}^{+}\left(\mathfrak{g}\right)$; then, for all $\mu \in Z\left(U_{r,s}^{+}\left(\mathfrak{g}\right)\right)$, $\mu D$ is a derivation of $U_{r,s}^{+}\left(\mathfrak{g}\right)$.

Proof. 

Since $\mu \in Z\left(U_{r,s}^{+}\left(\mathfrak{g}\right)\right)$, we have

$$\begin{array}{cc}\hfill \mu D\left(X_j{X}_i\right)& =\mu D\left(X_j\right)X_i+X_j\mu D\left(X_i\right)\hfill \\ \hfill & =\mu a_j{X}_j{X}_i+X_j\mu a_i^{\left(k\right)}{X}_i\hfill \\ \hfill & =\mu (a_j+a_i)X_j{X}_i.\hfill \end{array}$$

This is precisely the assertion of the proposition. □

Theorem 2.

Let $U_{r,s}^{+}\left(\mathfrak{g}\right)$ be the positive part of the two-parameter quantum group $U_{r,s}\left(\mathfrak{g}\right)$ corresponding to $\mathfrak{g}$; then, each derivation D of $U_{r,s}^{+}\left(\mathfrak{g}\right)$ must be written in the following form:

$$\begin{array}{c}\hfill D\left(X_i\right)=a{d}_{\xi }\left(X_i\right)+\sum _k{\mu }_k{a}_{X_i}^{\left(k\right)}\left(X_i\right)X_i,\end{array}$$

(12)

where $\xi \in U_{r,s}^{+}\left(\mathfrak{g}\right)$, ${\mu }_k\in Z\left(U_{r,s}^{+}\left(\mathfrak{g}\right)\right)$, $a_{X_i}^{\left(k\right)}\left(X_i\right)\in \mathbb{C}$, $k=1,\cdots ,p(p\le n)$ and the following equation holds:

$$a_{X_i}^{\left(k\right)}\left(X_i\right)+a_{X_j}^{\left(k\right)}\left(X_j\right)=a_{P(X_j,X_i)}^{\left(k\right)}\left(P(X_j,X_i)\right)$$

Proof. 

Based on Lemma 2, the positive part $U_{r,s}^{+}\left(\mathfrak{g}\right)$ of the two-parameter quantum group $U_{r,s}\left(\mathfrak{g}\right)$ constitutes an iterated Ore extension. Let $X_1,\cdots ,X_n$ be the quantum root vectors of $U_{r,s}^{+}\left(\mathfrak{g}\right)$ and $D_k,k=1,\cdots ,p(p\le n)$ represent the derivation of $U_{r,s}^{+}\left(\mathfrak{g}\right)$; we have

$$D_k\left(X_i\right)=a_{X_i}^{\left(k\right)}\left(X_i\right)X_i$$

and

$$a_{X_i}^{\left(k\right)}\left(X_i\right)+a_{X_j}^{\left(k\right)}\left(X_j\right)=a_{P(X_j,X_i)}^{\left(k\right)}\left(P(X_j,X_i)\right).$$

By consolidating Proposition 1, we can conclude that

$$\begin{array}{cc}\hfill \mu D_k\left(X_i{X}_j\right)& =\mu D_k\left(X_i\right)X_j+\mu X_i{D}_k\left(X_j\right)\hfill \\ \hfill & =\mu D_k\left(X_i\right)X_j+X_i\mu D_k\left(X_j\right)\hfill \end{array}$$

Therefore, we can conclude that any outer derivation of $U_{r,s}^{+}\left(\mathfrak{g}\right)$ can be expressed in the form of ${\displaystyle \sum _k}{\mu }_k{a}_{X_i}^{\left(k\right)}\left(X_i\right)X_i$. It can be seen from this that the theorem proof is completed. □

For convenience, in subsequent studies, we will denote $a_{X_i}^{\left(k\right)}\left(X_i\right)$ by the symbol $a_{ki}$.

Theorem 3.

Let D be the derivation of a two-parameter quantum group $U_{r,s}^{+}\left(\mathfrak{g}\right)$ as shown in Equation (21); then, the derivation of the one-parameter quantum group $U_q^{+}\left(\mathfrak{g}\right)$ corresponding to $U_{r,s}^{+}\left(\mathfrak{g}\right)$ can be uniquely written in the following form:

$$\begin{array}{c}\hfill D^{\prime }\left({\mathcal{X}}_i\right)=a{d}_{{\xi }^{\prime }}\left({\mathcal{X}}_i\right)+\sum _i{c}_k^{\prime }{a}_{kj}{\mathcal{X}}_j\end{array}$$

(13)

where ${\xi }^{\prime }\in U_q^{+}\left(\mathfrak{g}\right)$, $c_k{s}^{\prime }\in Z\left(U_q^{+}\left(\mathfrak{g}\right)\right)$.

Proof. 

Let $D_k(k=1,\cdots ,p)$ be the derivations of $U_{r,s}^{+}\left(\mathfrak{g}\right)$ and

$$D_k\left(X_j\right)=a_{kj}{X}_j.$$

According to Lemma 3, when $r=q$ and $s=q^{-1}$, there is $U_{q,q^{-1}}^{+}\left(\mathfrak{g}\right)\cong U_q^{+}\left(\mathfrak{g}\right)$. Let

$$\psi :U_{q,q^{-1}}^{+}\left(\mathfrak{g}\right)\to U_q^{+}\left(\mathfrak{g}\right)$$

be a mapping from $U_{q,q^{-1}}^{+}\left(\mathfrak{g}\right)$ to $U_q^{+}\left(\mathfrak{g}\right)$; so, we have

$$\psi D_k\left(X_j\right)=a_{kj}{\mathcal{X}}_j.$$

Let $D_k^{\prime }$ be a map on $U_q^{+}\left(\mathfrak{g}\right)$ and $D_k^{\prime }\left({\mathcal{X}}_j\right)=a_{kj}{\mathcal{X}}_j=\psi D_k\left(X_j\right)$; then, we prove that $D_k^{\prime }$$(k=1,\cdots ,p)$ are derivations of $U_q^{+}\left(\mathfrak{g}\right)$. According to the definition of $D_k^{\prime }$, we can obtain

$$\begin{array}{cc}\hfill D_k^{\prime }\left({\mathcal{X}}_j{\mathcal{X}}_i\right)& =\psi \left(D_k\left(X_j{X}_i\right)\right)=a_{kj}{\mathcal{X}}_j{\mathcal{X}}_i+a_{ki}{\mathcal{X}}_j{\mathcal{X}}_i\hfill \\ \hfill & =D_k^{\prime }\left({\mathcal{X}}_j\right){\mathcal{X}}_i+{\mathcal{X}}_j{D}_k^{\prime }\left({\mathcal{X}}_i\right)\hfill \end{array}$$

From the above results, we can know that $D_i^{\prime }$$(i=1,\cdots ,p)$ are the derivations of $U_q^{+}\left(\mathfrak{g}\right)$. According to Proposition 1, if $\mu \in Z\left(U_q^{+}\left(\mathfrak{g}\right)\right)$, then $\mu D_k^{\prime }$ is also a derivation of $U_q^{+}\left(\mathfrak{g}\right)$. Therefore, we obtain that all outer derivations of $U_q^{+}\left(\mathfrak{g}\right)$ have the form of ${\displaystyle \sum _i}{c}_i^{\prime }{a}_{ij}{\mathcal{X}}_j$, where $c_i^{\prime }\in Z\left(U_q^{+}\left(\mathfrak{g}\right)\right)$. Therefore, all derivations of $U_q^{+}\left(\mathfrak{g}\right)$ can be uniquely written in the form of Equation (22). □

Example 2.

Suppose $\mathfrak{g}$ is a finite-dimensional complex simple Lie algebra of $B_2$-type, the Cartan matrix for the $B_2$-type Lie algebra is

$$B:=\left[\begin{array}{cc}2& -1\\ -2& 2\end{array}\right]$$

Moreover, it can be deduced that $U_{r,s}^{+}\left(B_2\right)$ is a $\mathbb{C}$-algebra generated by generators $e_1$ and $e_2$, satisfying the following relationship:

$$\begin{array}{cc}\hfill & e_1^2{e}_2-(r^2+s^2)e_1{e}_2{e}_2+r^2{s}^2{e}_2{e}_1=0,\hfill \\ \hfill & e_1{e}_2^3-(r^2+rs+s^2)e_2{e}_1{e}_2^2+rs(r^2+rs+s^2)e_2^2{e}_1{e}_2-r^3{s}^3{e}_2^3{e}_1=0.\hfill \end{array}$$

Based on the standard Lyndon basis presented in Figure 1, we can obtain an ordering of the positive roots of $B_2$: ${\alpha }_1,{\alpha }_1+{\alpha }_2,{\alpha }_1+2{\alpha }_2,{\alpha }_2$, which corresponds to the quantum root vectors in $U_{r,s}^{+}\left(B_2\right)$:

$$X_1=e_1,X_2=e_1{e}_2-r^2{e}_2{e}_1,X_3=e_2{X}_2-s^{-2}{X}_2{e}_2,X_4=e_2,$$

and there is an exchange relationship:

$$\begin{array}{cc}\hfill & X_1{X}_2=s^2{X}_2{X}_1,\hfill \\ \hfill & X_1{X}_3=r^2{s}^2{X}_3{X}_1,\hfill \\ \hfill & X_2{X}_3=rs{X}_3{X}_2,\hfill \\ \hfill & X_1{X}_4=r^2{X}_4{X}_1+X_2,\hfill \\ \hfill & X_2{X}_4=s^2{X}_4{X}_2-s^2{X}_3,\hfill \\ \hfill & X_4{X}_3=r^{-1}{s}^{-1}{X}_3{X}_4.\hfill \end{array}$$

In the above exchange relationship, when $i=1,j=4$, we have $P(X_i,X_j)$ is $X_2$ and $i=2,j=4$, we have $P(X_i,X_j)$ is $X_3$; in other cases, $P(X_i,X_j)=0$. Therefore, assuming that D is a derivation of $U_{r,s}^{+}\left(B_2\right)$, there is $D\left(X_i\right)=a_{X_i}\left(X_i\right)X_i$, $a_{X_i}\left(X_i\right)\in \mathbb{C}$, $i\in \left[\right[1,4\left]\right]$ and

$$\begin{array}{c}\hfill a_{X_1}\left(X_1\right)+a_{X_4}\left(X_4\right)=a_{X_2}\left(X_2\right)\end{array}$$

(14)

$$\begin{array}{c}\hfill a_{X_2}\left(X_2\right)+a_{X_4}\left(X_4\right)=a_{X_3}\left(X_3\right)\end{array}$$

(15)

The fundamental solution system can be derived by solving Equations (14) and (15): ${\xi }_1={(1,1,1,0)}^T$ and ${\xi }_2={(0,1,2,1)}^T$. We introduce the following notations: $ai,a{i}^{\prime }$, with $1\le i\le 4$:

$$\begin{array}{cccccccc}\hfill & a_1=1,\hfill & \hfill & a_2=1,\hfill & \hfill & a_3=1,\hfill & \hfill & a_4=0,\hfill \\ \hfill & a_1^{\prime }=0,\hfill & \hfill & a_2^{\prime }=1,\hfill & \hfill & a_3^{\prime }=2,\hfill & \hfill & a_4^{\prime }=1.\hfill \end{array}$$

Let $D_1$ and $D_2$ be derivations corresponding to two fundamental solution systems; therefore, there are

$$\begin{array}{cccccccc}\hfill & D_1\left(X_1\right)=X_1,\hfill & \hfill & D_1\left(X_2\right)=X_2,\hfill & \hfill & D_1\left(X_3\right)=X_3,\hfill & \hfill & D_1\left(X_4\right)=0,\hfill \\ \hfill & D_2\left(X_1\right)=0,\hfill & \hfill & D_2\left(X_2\right)=X_2,\hfill & \hfill & D_2\left(X_3\right)=2X_3,\hfill & \hfill & D_2\left(X_4\right)=X_4.\hfill \end{array}$$

Then, each derivation Dof $U_{r,s}^{+}\left(B_2\right)$ can be written in the following form:

$$\begin{array}{c}\hfill D={\mathrm{ad}}_x+{\mu }_1{D}_1+{\mu }_2{D}_2\end{array}$$

(16)

where ${\mu }_1,{\mu }_2\in Z\left(U_{r,s}^{+}\left(B_2\right)\right)$.

According to Theorem 3, there exist two mappings $D_1^{\prime }$ and $D_2^{\prime }$ on $U_q^{+}\left(B_2\right)$, such that

$$\begin{array}{cccccccc}\hfill & D_1^{\prime }\left({\mathcal{X}}_1\right)={\mathcal{X}}_1,\hfill & \hfill & D_1^{\prime }\left({\mathcal{X}}_2\right)={\mathcal{X}}_2,\hfill & \hfill & D_1^{\prime }\left({\mathcal{X}}_3\right)={\mathcal{X}}_3,\hfill & \hfill & D_1^{\prime }\left({\mathcal{X}}_4\right)=0,\hfill \\ \hfill & D_2^{\prime }\left({\mathcal{X}}_1\right)=0,\hfill & \hfill & D_2^{\prime }\left({\mathcal{X}}_2\right)={\mathcal{X}}_2,\hfill & \hfill & D_2^{\prime }\left({\mathcal{X}}_3\right)=2{\mathcal{X}}_3,\hfill & \hfill & D_2^{\prime }\left({\mathcal{X}}_4\right)={\mathcal{X}}_4.\hfill \end{array}$$

Therefore, the derivation $D^{\prime }$ of $U_q^{+}\left(B_2\right)$ can be written as

$$\begin{array}{c}\hfill D^{\prime }\left({\mathcal{X}}_i\right)={\mathit{ad}}_g\left({\mathcal{X}}_i\right)+a_i{\zeta }_1{\mathcal{X}}_i+a_i^{\prime }{\zeta }_2{\mathcal{X}}_i\end{array}$$

(17)

where $g\in U_q^{+}\left(B_2\right)$ and ${\zeta }_i\in Z\left(U_v^{+}\left(B_2\right)\right)$.

Next, we need to verify that every derivation of $U_q^{+}\left(B_2\right)$ can be expressed as shown in Equation (17). Suppose $D^{″}$ is any derivation of $U_q^{+}\left(B_2\right)$.

By combining Theorem 1 and applying $D^{″}$ on ${\mathcal{X}}_1{\mathcal{X}}_4$ and ${\mathcal{X}}_2{\mathcal{X}}_4$, respectively, it can be determined that

$$\begin{array}{cc}\hfill & D^{″}\left({\mathcal{X}}_4{\mathcal{X}}_1\right)=D^{″}\left(q^{-2}{\mathcal{X}}_1{\mathcal{X}}_4\right)-D^{″}\left(q^{-2}{\mathcal{X}}_2\right)\hfill \end{array}$$

(18)

$$\begin{array}{cc}\hfill & D^{″}\left({\mathcal{X}}_4{\mathcal{X}}_2\right)=D^{″}\left(q^2{\mathcal{X}}_2{\mathcal{X}}_4\right)+D^{″}\left({\mathcal{X}}_3\right)\hfill \end{array}$$

(19)

We can obtain the fundamental solution system by solving Equations (18) and (19): ${\xi }_1={(1,1,1,0)}^T$ and ${\xi }_2={(0,1,2,1)}^T$.

Let $D_1^{″}$ and $D_2^{″}$ be the derivatives corresponding to the fundamental solution system; therefore,

$$\begin{array}{cccccccc}\hfill & D_1^{″}\left({\mathcal{X}}_1\right)={\mathcal{X}}_1,\hfill & \hfill & D_1^{″}\left({\mathcal{X}}_2\right)={\mathcal{X}}_2,\hfill & \hfill & D_1^{″}\left({\mathcal{X}}_3\right)={\mathcal{X}}_3,\hfill & \hfill & D_1^{″}\left({\mathcal{X}}_4\right)=0,\hfill \\ \hfill & D_2^{″}\left({\mathcal{X}}_1\right)=0,\hfill & \hfill & D_2^{″}\left({\mathcal{X}}_2\right)={\mathcal{X}}_2,\hfill & \hfill & D_2^{″}\left({\mathcal{X}}_3\right)=2{\mathcal{X}}_3,\hfill & \hfill & D_2^{″}\left({\mathcal{X}}_4\right)={\mathcal{X}}_4.\hfill \end{array}$$

Clearly, $D_1^{″}=D_1^{\prime }$ and $D_2^{\prime \prime }=D_2^{\prime }$, so it can be concluded that the derivations of $U_q^{+}\left(B_2\right)$ can be expressed as

$$D^{″}={\mathrm{ad}}_g+{\zeta }_1{D}_1^{″}+{\zeta }_2{D}_2^{″}=D^{\prime }$$

where $g\in U_q^{+}\left(B_2\right)$ and ${\zeta }_q,{\zeta }_2\in Z\left(U_q^{+}\left(B2\right)\right)$.

4.2. Obtain Der$\left(U_{v,t}^{+}\left(\mathfrak{g}\right)\right)$ from Der$\left(U_v^{+}\left(\mathfrak{g}\right)\right)$

It is worth noting that while it is relatively easy to derive a one-parameter quantum group from a two-parameter quantum group, the reverse process of obtaining a two-parameter quantum group from a one-parameter quantum group is usually more challenging. Therefore, in order to facilitate future research, this section begins with a review of the definition and key properties of the two-parameter quantum group introduced by Fan and Li.

Let I be a finite set, and fix a matrix $\mathsf{\Omega }={\left({\mathsf{\Omega }}_{ij}\right)}_{i,j\in I}$ satisfying the following conditions:

(i)

${\mathsf{\Omega }}_{ii}\in {\mathbb{Z}}_{>0}$, ${\mathsf{\Omega }}_{ij}\in {\mathbb{Z}}_{\le 0}$ for all $i\ne j\in I$;

(ii)

${\displaystyle \frac{{\mathsf{\Omega }}_{ij}+{\mathsf{\Omega }}_{ji}}{{\mathsf{\Omega }}_{ii}}}\in {\mathbb{Z}}_{\le 0}$ for all $i\ne j\in I$;

(iii)

The greatest common divisor of all ${\mathsf{\Omega }}_{ii}$ is equal to 1.

Connect matrix $\mathsf{\Omega }$ with three bilinear forms on ${\mathbb{Z}}^I$:

$$\begin{array}{cc}\hfill & ⟨i,j⟩={\mathsf{\Omega }}_{ij},\forall i,j\in I.\hfill \\ \hfill & [i,j]=2{\delta }_{ij}{\mathsf{\Omega }}_{ii}-{\mathsf{\Omega }}_{ij},\forall i,j\in I.\hfill \\ \hfill & i·j=⟨i,j⟩+⟨j,i⟩,\forall i,j\in I.\hfill \end{array}$$

(20)

Definition 5.

Let $\mathsf{\Omega }={\left({\mathsf{\Omega }}_{ij}\right)}_{i,j\in I}$ be the matrix as described above. The two-parameter quantum group $U_{v,t}$ associated to Ω is an associative $Q(v,t)$-algebra with 1 generated by symbols $E_i$, $F_i$, $K_i^{\pm 1}$, ${K_i^{\prime }}^{\pm 1}$, $\forall \in I$ and subject to the following relations:

$$\begin{array}{cc}\hfill \left(\mathrm{R}1\right)& K_i^{\pm 1}{K}_j^{\pm 1}=K_j^{\pm 1}{K}_i^{\pm 1},{K_i^{\prime }}^{\pm 1}{K_j^{\prime }}^{\pm 1}={K_j^{\prime }}^{\pm 1}{K_i^{\prime }}^{\pm 1},\hfill \\ \hfill & {K_i}^{\pm 1}{K_j^{\prime }}^{\pm 1}={K_j^{\prime }}^{\pm 1}{K_i}^{\pm 1},{K_i}^{\pm 1}{K_i}^{\mp 1}=1={K_i^{\prime }}^{\pm 1}{K_i^{\prime }}^{\mp 1},\hfill \\ \hfill \left(\mathrm{R}2\right)& K_i{E}_j{K}_i^{-1}=v^{i·j}{t}^{⟨i,j⟩-⟨j,i⟩}{E}_j,K_i^{\prime }{E}_j{K_i^{\prime }}^{-1}=v^{-i·j}{t}^{⟨i,j⟩-⟨j,i⟩}{E}_j,\hfill \\ \hfill & K_i{F}_j{K}_i^{-1}=v^{-i·j}{t}^{⟨j,i⟩-⟨i,j⟩}{F}_j,K_i^{\prime }{F}_j{K_i^{\prime }}^{-1}=v^{i·j}{t}^{⟨j,i⟩-⟨i,j⟩}{F}_j,\hfill \\ \hfill \left(\mathrm{R}3\right)& E_i{F}_j-F_j{E}_i={\delta }_{ij}{\displaystyle \frac{K_i-K_i^{\prime }}{v_i-v_i^{-1}}},\hfill \\ \hfill \left(\mathrm{R}4\right)& \sum _{p+p^{\prime }=1-2{\displaystyle \frac{i·j}{i·i}}}{(-1)}^p{t_i}^{-p\left(p^{\prime }-2{\displaystyle \frac{⟨i,j⟩}{i·i}}+2{\displaystyle \frac{⟨j,i⟩}{i·i}}\right)}{E}_i^{\left(p^{\prime }\right)}{E}_j{E}_i^{\left(p\right)}=0,ifi\ne j,\hfill \\ \hfill & \sum _{p+p^{\prime }=1-2{\displaystyle \frac{i·j}{i·i}}}{(-1)}^p{t_i}^{-p\left(p^{\prime }-2{\displaystyle \frac{⟨i,j⟩}{i·i}}+2{\displaystyle \frac{⟨j,i⟩}{i·i}}\right)}{F}_i^{\left(p\right)}{F}_j{F}_i^{\left(p^{\prime }\right)}=0,ifi\ne j,\hfill \end{array}$$

where $E_i^{\left(p\right)}={\displaystyle \frac{E_i^p}{{\left[p\right]!}_{v_i,t_i}}}$.

In reference [10], the author compared the algebraic structure $U_{v,t}$ with the two-parameter quantum group defined in Definition 2 and concluded that when $v={\left(r{s}^{-1}\right)}^{{\displaystyle \frac{1}{2}}}$ and $t={\left(rs\right)}^{-{\displaystyle \frac{1}{2}}}$, the Serre relations of the algebraic $U_{v,t}$ are entirely consistent with those given in Definition 2. Therefore, our primary focus will be on studying how to derive the derivation algebra of $U_{v,t}^{+}\left(\mathfrak{g}\right)$ from that of $U_v^{+}\left(\mathfrak{g}\right)$.

We denote by $U_v^{+}\left(\mathfrak{g}\right)$ the positive part of the quantum group $U=U_v\left(\mathfrak{g}\right)$ over $\mathbb{Q}\left(v\right)$; it is the free $\mathbb{Q}\left(v\right)$ - algebra. Let ${\mathsf{\Phi }}^{+}$ be a positive root system of the complex simple Lie algebra of $\mathfrak{g}$, and $|{\mathsf{\Phi }}^{+}|=n$. Let ${\mathcal{X}}_1,\cdots ,{\mathcal{X}}_n$ be the quantum root vector of $U_v^{+}\left(\mathfrak{g}\right)$ and satisfy the exchange relation (2).

Theorem 4.

Let $U_v^{+}\left(\mathfrak{g}\right)$ be the positive part of the one-parameter quantum group $U_v\left(\mathfrak{g}\right)$ corresponding to $\mathfrak{g}$; then, each derivation D of $U_v^{+}\left(\mathfrak{g}\right)$ must be written in the following form:

$$\begin{array}{c}\hfill D\left(X_i\right)=a{d}_{\xi }\left(X_i\right)+\sum _k{\mu }_k{a}_{X_i}^{\left(k\right)}\left(X_i\right)X_i,\end{array}$$

(21)

where $\xi \in U_v^{+}\left(\mathfrak{g}\right)$, ${\mu }_k\in Z\left(U_v^{+}\left(\mathfrak{g}\right)\right)$, $a_{X_i}^{\left(k\right)}\left(X_i\right)\in \mathbb{C}$, and $k=1,\cdots ,p(p\le n)$, and the following equation holds:

$$a_{X_i}^{\left(k\right)}\left(X_i\right)+a_{X_j}^{\left(k\right)}\left(X_j\right)=a_{P(X_j,X_i)}^{\left(k\right)}\left(P(X_j,X_i)\right).$$

Proof. 

The proof for Theorem 4 mirrors that of Theorem 2; thus, we will not present a detailed proof. □

Theorem 5.

If $D_i(i=1,\cdots ,n)$ are the derivations of $U_v^{+}\left(\mathfrak{g}\right)$ and $D_i\left({\mathcal{X}}_j\right)=a_{ij}{\mathcal{X}}_j$, then there exists a mapping $D_i^{\prime }(i=1,\cdots ,n)$, where $D_i^{\prime }\left(X_j\right)=a_{ij}{X}_j$ are the derivations of $U_{v,t}^{+}\left(\mathfrak{g}\right)$, and each derivation of $U_{v,t}^{+}\left(\mathfrak{g}\right)$ can be uniquely written in the following form:

$$\begin{array}{c}\hfill D^{\prime }\left(X_i\right)=a{d}_{{\xi }^{\prime }}\left(X_i\right)+\sum _i{c}_i^{\prime }{a}_{ij}{X}_j,\end{array}$$

(22)

where ${\xi }^{\prime }\in U_{v,t}^{+}\left(\mathfrak{g}\right)$, $c_i^{\prime }\in Z\left(U_{v,t}^{+}\left(\mathfrak{g}\right)\right)$.

Proof. 

Let $U_{v,t}=U_v{\otimes }_{\mathbb{Q}\left(v\right)}\mathbb{Q}(v,t)$; according to Theorem 4 in reference [10], the bialgebra structure of $U_v$ can be naturally extended to $U_{v,t}$. We define a new multiplication “∘” on $U_{v,t}$ by

$$x\circ y=t^{\left[\right|x|,|y\left|\right]}xy,$$

for any homogeneous elements $x,y\in U_{v,t}$, where $[,]$ is defined in (20).

Since ${\mathcal{X}}_1,\cdots ,{\mathcal{X}}_n$ are quantum root vectors of $U_v^{+}\left(\mathfrak{g}\right)$ and satisfy Equation (2), the following formula holds for the algebra $U_{v,t}^{+}\left(\mathfrak{g}\right)$:

$$\begin{array}{cc}\hfill & t^{-\left[\right|{\mathcal{X}}_i|,|{\mathcal{X}}_j\left|\right]}{\mathcal{X}}_i\circ {\mathcal{X}}_j-v^{(i,j)}{t}^{-\left[\right|{\mathcal{X}}_j|,|{\mathcal{X}}_i\left|\right]}{\mathcal{X}}_j\circ {\mathcal{X}}_i\hfill \\ \hfill =& \sum _{k=(k_{i+1},\cdots ,k_{j-1})}{c}_k{t}^{-\left[\stackrel{k_{i+1}}{\overbrace{|{\mathcal{X}}_{i+1}|,\cdots ,|{\mathcal{X}}_{i+1}|}},\cdots ,\stackrel{k_{j-1}}{\overbrace{|{\mathcal{X}}_{j-1}|,\cdots ,|{\mathcal{X}}_{j-1}|}}\right]}{\mathcal{X}}_{i+1}^{k_{i+1}}\circ \cdots \circ {\mathcal{X}}_{j-1}^{k_{j-1}}.\hfill \end{array}$$

(23)

If $D^{\prime }$ is the derivation of $U_{v,t}^{+}\left(\mathfrak{g}\right)$, then we have

$$\begin{array}{cc}\hfill D^{\prime }({\mathcal{X}}_i\circ {\mathcal{X}}_j)& =D^{\prime }\left(t^{\left[\right|{\mathcal{X}}_i|,|{\mathcal{X}}_j\left|\right]}{\mathcal{X}}_i{\mathcal{X}}_j\right)\hfill \\ \hfill & =t^{\left[\right|X_i|,|X_j\left|\right]}{D}^{\prime }\left({\mathcal{X}}_i{\mathcal{X}}_j\right).\hfill \end{array}$$

Therefore, we can conclude that if $D^{\prime }$ is a derivation of $U_{v,t}^{+}\left(\mathfrak{g}\right)$, then $D^{\prime }$ acting on $U_v^{+}\left(\mathfrak{g}\right)$ must be a derivation of $U_v^{+}\left(\mathfrak{g}\right)$.

According to the given assumption, $D_i,i=1,\cdots ,n$ represents the derivation of $U_v^{+}\left(\mathfrak{g}\right)$, with $D_i\left({\mathcal{X}}_j\right)=a_{ij}{\mathcal{X}}_j$. Referring to Proposition 4, we can determine that $\mu D_i^{\prime }$ are the derivation of $U_{v,t}^{+}\left(\mathfrak{g}\right)$, where $\mu \in Z\left(U_{v,t}^{+}\left(\mathfrak{g}\right)\right)$, $D_i^{\prime }\left({\mathcal{X}}_j\right)=a_{ij}{\mathcal{X}}_j$, Based on this, we can conclude that the theorem is valid. □

Example 3.

Suppose $\mathfrak{g}$ is a finite-dimensional complex simple Lie algebra of $B_2$-type. Moreover, it can be deduced that $U_v^{+}\left(B_2\right)$ is a $\mathbb{C}$-algebra generated by generators $E_1$ and $E_2$, and satisfies the following relationship:

$$\begin{array}{cc}\hfill & E_1^2{E}_2-(v^2+v^{-2})E_1{E}_2{E}_1+E_2{E}_1^2=0,\hfill \\ \hfill & E_1{E}_2^3-(v^2+1+v^{-2})E_2{E}_1{E}_2^2+(v^2+1+v^{-2})E_2^2{E}_1{E}_2-E_2^3{E}_1=0.\hfill \end{array}$$

On the other hand, ${\alpha }_1,{\alpha }_1+{\alpha }_2,{\alpha }_1+2{\alpha }_2,{\alpha }_2$ correspond to the quantum root vectors ${\mathcal{X}}_1$, ${\mathcal{X}}_2$, ${\mathcal{X}}_3$, ${\mathcal{X}}_4$ in $U_v^{+}\left(B_2\right)$:

$${\mathcal{X}}_1=E_1,{\mathcal{X}}_2=E_1{E}_2-v^2{E}_2{E}_2,{\mathcal{X}}_3=E_2{\mathcal{X}}_2-v^2{\mathcal{X}}_2{E}_2,{\mathcal{X}}_4=E_2.$$

And there is an exchange relationship:

$$\begin{array}{cccccc}\hfill & {\mathcal{X}}_2{\mathcal{X}}_3={\mathcal{X}}_3{\mathcal{X}}_1,\hfill & \hfill & {\mathcal{X}}_1{\mathcal{X}}_3={\mathcal{X}}_3{\mathcal{X}}_1,\hfill & \hfill & {\mathcal{X}}_1{\mathcal{X}}_2=v^{-2}{\mathcal{X}}_2{\mathcal{X}}_1,\hfill \\ \hfill & {\mathcal{X}}_4{\mathcal{X}}_3={\mathcal{X}}_3{\mathcal{X}}_4,\hfill & \hfill & {\mathcal{X}}_4{\mathcal{X}}_2=v^2{\mathcal{X}}_2{\mathcal{X}}_4+{\mathcal{X}}_3,\hfill & \hfill & {\mathcal{X}}_4{\mathcal{X}}_1=v^{-2}{\mathcal{X}}_1{\mathcal{X}}_4-v^{-2}{\mathcal{X}}_2.\hfill \end{array}$$

Suppose D is a derivation of $U_v^{+}\left(B_2\right)$. Since $U_v^{+}\left(B_2\right)$ is an iterated Ore extension, there is $D\left({\mathcal{X}}_i\right)=a_{{\mathcal{X}}_i}\left({\mathcal{X}}_i\right){\mathcal{X}}_i$, $a_{{\mathcal{X}}_i}\left({\mathcal{X}}_i\right)\in \mathbb{C}$. Therefore, we have

$$\begin{array}{c}\hfill a_{{\mathcal{X}}_1}\left({\mathcal{X}}_1\right)+a_{{\mathcal{X}}_4}\left({\mathcal{X}}_4\right)=a_{{\mathcal{X}}_2}\left({\mathcal{X}}_2\right),\end{array}$$

(24)

$$\begin{array}{c}\hfill a_{{\mathcal{X}}_2}\left({\mathcal{X}}_2\right)+a_{{\mathcal{X}}_4}\left({\mathcal{X}}_4\right)=a_{{\mathcal{X}}_3}\left({\mathcal{X}}_3\right).\end{array}$$

(25)

The fundamental solution system can be derived by solving Equations (24) and (27): ${\xi }_1={(1,1,1,0)}^T$ and ${\xi }_2={(0,1,2,1)}^T$. Let $D_1$ and $D_2$ be derivations corresponding to two fundamental solution systems; therefore, there are

$$\begin{array}{cccccccc}\hfill & D_1\left({\mathcal{X}}_1\right)={\mathcal{X}}_1,\hfill & \hfill & D_1\left({\mathcal{X}}_2\right)={\mathcal{X}}_2,\hfill & \hfill & D_1\left({\mathcal{X}}_3\right)={\mathcal{X}}_3,\hfill & \hfill & D_1\left({\mathcal{X}}_4\right)=0,\hfill \\ \hfill & D_2\left({\mathcal{X}}_1\right)=0,\hfill & \hfill & D_2\left({\mathcal{X}}_2\right)={\mathcal{X}}_2,\hfill & \hfill & D_2\left({\mathcal{X}}_3\right)=2{\mathcal{X}}_3,\hfill & \hfill & D_2\left({\mathcal{X}}_4\right)={\mathcal{X}}_4.\hfill \end{array}$$

Then, each derivation D of $U_v^{+}\left(B_2\right)$ can be written in the following form:

$$\begin{array}{c}\hfill D={\mathit{ad}}_x+{\mu }_1{D}_1+{\mu }_2{D}_2\end{array}$$

(26)

where ${\mu }_1,{\mu }_2\in Z\left(U_v^{+}\left(B_2\right)\right)$. Let $U_{v,t}\left(B_2\right)=U_v\left(B_2\right){\otimes }_{\mathbb{Q}\left(v\right)}\mathbb{Q}(v,t)$; the bialgebra structure of $U_v\left(B_2\right)$ can be naturally extended to $U_{v,t}\left(B_2\right)$. According to Theorem 5, there exist two mappings $D_1^{\prime }$ and $D_2^{\prime }$ on $U_{v,t}^{+}\left(B_2\right)$, such that

$$\begin{array}{cccccccc}\hfill & D_1^{\prime }\left({\mathcal{X}}_1\right)={\mathcal{X}}_1,\hfill & \hfill & D_1^{\prime }\left({\mathcal{X}}_2\right)={\mathcal{X}}_2,\hfill & \hfill & D_1^{\prime }\left({\mathcal{X}}_3\right)={\mathcal{X}}_3,\hfill & \hfill & D_1^{\prime }\left({\mathcal{X}}_4\right)=0,\hfill \\ \hfill & D_2^{\prime }\left({\mathcal{X}}_1\right)=0,\hfill & \hfill & D_2^{\prime }\left({\mathcal{X}}_2\right)={\mathcal{X}}_2,\hfill & \hfill & D_2^{\prime }\left({\mathcal{X}}_3\right)=2{\mathcal{X}}_3,\hfill & \hfill & D_2^{\prime }\left({\mathcal{X}}_4\right)={\mathcal{X}}_4.\hfill \end{array}$$

Therefore, the derivation $D^{\prime }$ of $U_{v,t}^{+}\left(B_2\right)$ can be written as

$$\begin{array}{c}\hfill D^{\prime }\left({\mathcal{X}}_i\right)={\mathit{ad}}_g\left({\mathcal{X}}_i\right)+{\zeta }_1{D}_1^{\prime }\left({\mathcal{X}}_i\right)+{\zeta }_2{D}_2^{\prime }\left({\mathcal{X}}_i\right)\end{array}$$

(27)

where $g\in U_{v,t}^{+}\left(B_2\right)$ and ${\zeta }_i\in Z\left(U_{v,t}^{+}\left(B_2\right)\right)$.

Similar to the conclusion in Example 2, we can verify that each derivation of $U_{v,t}^{+}\left(B_2\right)$ can be represented as Equation (27).

5. Conclusions

In conclusion, this paper gives the derivation algebraic structure of a special class of iterated Ore extension, and discusses the relationship between derivation of one-parameter quantum groups and derivation of two-parameter quantum groups. Our results provide a new perspective for understanding the derivation algebra of quantum groups. Despite the important progress made in this paper, the application of derivation algebras to broader quantum groups needs to be further explored. For example, the derivation has a close relationship with the automorphism group of quantum groups; this relationship will help us gain a deeper understanding of quantum groups and their intrinsic properties of algebraic structures.