Singularity, Observability, and Independence: Unveiling Lorenz’s Cryptographic Potential

Abstract

The key findings of this study include a detailed examination of the Lorenz system’s observability, revealing that it maintains high observability compared to other chaotic systems, thus supporting its potential use in cryptographic applications. We also investigated the singularity manifolds, identifying regions where observability might be compromised, but overall demonstrating that the system remains reliable across various states. Additionally, statistical tests confirm that the Lorenz system exhibits strong statistical independence in its outputs, further validating its suitability for encryption purposes. These findings collectively underscore the Lorenz system’s potential to enhance cryptographic security and contribute significantly to the field of secure communications. By providing a thorough analysis of its key properties, this study positions the Lorenz system as a promising candidate for advanced encryption technologies.

1. Introduction

Throughout history, the necessity of securely communicating sensitive information has been paramount [1]. This need spans various domains such as military operations, diplomatic exchanges, and personal matters, where ensuring that only the intended recipient comprehends the message has always been crucial [2]. Cryptography emerged to fulfill this critical requirement, enabling parties to protect the confidentiality of their information, even when potential interceptors attempt to decode it [3,4]. Over recent decades, the evolution of cryptographic methods has been profound, with confidentiality remaining a cornerstone of the field [5,6]. The developments within cryptography have profoundly impacted digital security and information protection.

Nonlinear dynamical chaotic systems are intensely studied due to their complex and unpredictable behaviors [7]. These systems, when implemented on FPGA platforms, offer practical advantages that bridge theoretical models with real-world applications [8]. In particular, advancements in chaotic dynamical systems have significant implications for various research areas such as robotics, communication, and cryptography [3]. The integration of chaos theory with cryptography has emerged as a promising approach to developing more secure and efficient cryptographic algorithms.

Jones and Smith (2023) discuss recent advancements in chaotic systems applied to cryptography, highlighting several innovative approaches [9]. Li and Zhang (2024) provide an overview of hyperchaotic systems and their implications for secure communications, presenting new methodologies [10]. Wang and Zhou (2023) offer a comprehensive review of fractional-order chaotic systems and their applications in various fields, including cryptography [11]. Lee and Kim (2024) explore optimization techniques for chaotic maps, focusing on their application in high-performance image encryption [12]. Patel and Kumar (2023) highlight recent advances in cryptographic algorithms based on chaotic systems, providing insights into current trends and future directions [13].

Recent research in chaos-based encryption has highlighted several advanced techniques and applications. For instance, cross-channel color image encryption using 2D hyperchaotic hybrid maps of optimization test functions [14] and PSO-based image encryption schemes utilizing modular integrated logistic exponential maps [15] represent notable advances in the field. Additionally, exploiting newly designed fractional-order 3D Lorenz chaotic systems and 2D discrete polynomial hyper-chaotic maps has demonstrated high-performance capabilities in multi-image encryption [16]. Moreover, image encryption algorithms based on plane-level image filtering and discrete logarithmic transforms [17] showcase innovative approaches to enhancing encryption techniques.

Despite these advancements, not all dynamical systems exhibit desirable cryptographic properties [18,19], and a universal solution applicable across all contexts remains elusive. This paper seeks to address this challenge by extending the results shown in [20] and evaluating the properties of the Lorenz chaotic system from three distinct perspectives: observability [21,22,23], singularity [24], and statistical independence [25,26].

The original contribution of this paper lies in its comprehensive analysis, which combines typically separate investigations of observability, singularity, and statistical independence to provide a unified and robust perspective on the use of dynamical chaotic maps in cryptography. This integrated approach not only deepens the understanding of chaotic systems but also enhances their reliability in cryptographic applications. Furthermore, this study examines and compares several discrete chaotic systems alongside the continuous Lorenz chaotic system, offering valuable insights from the perspectives of singularity, observability, and statistical independence.

Section 2 provides an overview of the current state-of-the-art findings that form the foundation of this research. This section reviews essential concepts and recent advancements that have guided this study. Section 3 delves into observability, singularity, and statistical independence, presenting new experimental data and analyses for the Lorenz system. The main findings and potential future research directions are discussed in Section 4, highlighting the implications of the results and suggesting areas for further research.

2. Theoretical Background

This section provides a comprehensive overview of the theoretical concepts and state-of-the-art findings that underpin the research presented in this paper. We start by introducing the foundational theories relevant to chaotic systems and their applications in cryptography. This background sets the stage for the subsequent analysis by reviewing essential concepts, recent advancements, and key literature that have guided our study. The aim is to ensure that readers have a solid understanding of the theoretical framework before diving into the experimental results and analysis.

The theoretical concepts described below (observability, singularity and statistical independence) are mainly carried over from [20] and their description is not changed in a significant way. What is different in this paper is the chaotic system which is used and a comparison and correlation with the previous results is made.

A dynamical system can be succinctly described as a framework governing the evolution of states over time. One notable example is the Lorenz system, encapsulated by a trio of differential equations (continuous form—(1) and discrete form—(2); with $\Delta t=1/F_s$). This set of equations, credited to Edward Lorenz, an American meteorologist, was developed to model variations in wind speed and temperature. Lorenz achieved this by formulating the three differential equations outlined below. Computational experiments later confirmed that these equations led to a highly sensitive system, where minuscule differences in initial conditions resulted in drastically divergent outcomes over time. The solutions generated by this chaotic system consistently evolve around a geometric structure known as a strange attractor fractal [24]. For chaotic behavior, typical parameter values are $a=10$, $b=8/3$, and $c=28$ [20,27]; the variables x, y, and z represent the state variables of the Lorenz system.

$$\left\{\begin{array}{c}{\displaystyle \frac{dx}{dt}}=a·(y-x)\hfill \\ {\displaystyle \frac{dy}{dt}}=x·(c-z)-y\hfill \\ {\displaystyle \frac{dz}{dt}}=x·y-b·z\hfill \end{array}\right.$$

(1)

$$\left\{\begin{array}{c}{\displaystyle \frac{x_{k+1}-x_k}{\Delta t}}=a·(y_k-x_k)\hfill \\ {\displaystyle \frac{y_{k+1}-y_k}{\Delta t}}=x_k·(c-z_k)-y_k\hfill \\ {\displaystyle \frac{z_{k+1}-z_k}{\Delta t}}=x_k·y_k-b·z_k\hfill \end{array}\right.$$

(2)

The subsequent section elaborates on the core theoretical concepts employed in this paper. Observability refers to the ability to infer the states or the temporal evolution of the input/output of a system within any finite, non-zero time frame based on the system’s past behavior. Essentially, observability permits the monitoring and examination of a system’s internal states solely by accessing its input/output data. For an n-dimensional chaotic system, observability implies that by utilizing a sequence of values generated by one of the system’s state variables, one can reconstruct the system’s phase space [28]. It is important to note that the discussion of observability assumes that the system parameters are known. Extensive discussions on the observability of multi-dimensional systems can be found in [29,30].

The concept of the singularity manifold in dynamical systems denoted as $S_{\overline{O}}$, can be approached from various perspectives and is intricately linked to observability. The region of space where the system loses its observability for a particular state variable defines the singularity manifold for that variable, indicating that the system is unobservable in that region. In cryptography, the full observability of a system is critically important, making the determination of singularity manifolds a key aspect of our investigation in this paper [31].

The methodology for assessing statistical independence is outlined below. Vlad et al. [25] introduced a test procedure to determine if two random variables are statistically independent. This procedure combines a Pearson-like test with a visual assessment. The same research group further refined their approach [26] by innovatively applying the chi-square test [32] to evaluate whether two experimental datasets align with the Gaussian bivariate probability distribution.

In this paper, we will examine the statistical independence of two datasets, denoted as X and Y, which are derived from the solution space of the Lorenz system. According to the method described in [25], these datasets X and Y will be transformed into two new datasets, U and V, which are expected to follow a Gaussian distribution. The refined chi-square test described in [26] will then be used to assess the statistical independence of U and V by checking if they adhere to the standard normal bivariate probability distribution given by (3). If U and V satisfy this distribution, it implies that the original datasets X and Y are statistically independent.

$$p(u,v)={\displaystyle \frac{1}{2\pi }}{e}^{-{\displaystyle \frac{u^2+v^2}{2}}}$$

(3)

The datasets under evaluation are $(u_1,u_2,\dots ,u_N)$ and $(v_1,v_2,\dots ,v_N)$, each of which should follow an i.i.d. statistical model, meaning the data points are from independent and identically distributed random variables, and both datasets should exhibit a standard Gaussian distribution (mean = 0; variance = 1). Each variable in the dataset is assumed to be independently and identically distributed (i.i.d.), adhering to a standard statistical model. This assumption is crucial for the validity of the subsequent analyses and results.

The hypotheses for the spatial chi-square test are as follows:

• $H_0$—null hypothesis: The datasets (U and V) conform to the bivariate normal distribution described in (3), indicating that U and V (and hence X and Y) are statistically independent.
• $H_1$—alternative hypothesis: The datasets (U and V) do not adhere to the bivariate normal distribution described in (3), suggesting that U and V (and hence X and Y) are not statistically independent.

3. Experimental Results

This section presents the experimental results obtained from our investigations into the Lorenz chaotic system. The purpose of this section is to provide a detailed analysis of the data collected through various experiments designed to evaluate the system’s properties in the context of cryptographic applications.

We begin by discussing the methodology used for the experiments, followed by a presentation of the results for each aspect studied: observability, singularity, and statistical independence. The results are analyzed in detail to highlight key findings and their implications for enhancing cryptographic algorithms based on chaotic systems.

Finally, this section concludes with a discussion on the impact of the experimental findings and their relevance to the overall research goals outlined in the introduction.

3.1. Observability

The procedure used to calculate the observability coefficient for the Lorenz chaotic system is described next and is stemming from [21,31]:

• Write the fluency matrix $F_{ij}$ by replacing each (non)constant element of the Jacobian matrix with ($\overline{1}$) 1, and zero otherwise.
  The Jacobian for the Lorenz system is calculated considering the equations from (1) and their partial derivatives with respect to the state variables $x_i$ (for the Lorenz system, the state variables are represented by x, y, and z). The result is shown in (4), which is the Jacobian of the Lorenz system.

  $$J=\left[\begin{array}{ccc}-a& a& 0\\ c-z& -1& -x\\ y& x& -b\end{array}\right]$$

  (4)
  The fluency matrix is presented in (5):

  $$F_{ij}=\left[\begin{array}{ccc}1& 1& 0\\ \overline{1}& 1& \overline{1}\\ \overline{1}& \overline{1}& 1\end{array}\right]$$

  (5)
• The next step is to choose a state variable to reconstruct the dynamics of the Lorenz system. One should also define the column array $C_{1,i}$, with 1 on the ith position and 0 elsewhere: $c_{1,1}=\left[\begin{array}{c}1\\ 0\\ 0\end{array}\right]$ for x $c_{1,2}=\left[\begin{array}{c}0\\ 1\\ 0\end{array}\right]$ for y and $c_{1,3}=\left[\begin{array}{c}0\\ 0\\ 1\end{array}\right]$ for z.
• Matrix $H_{1,i}$ is an auxiliary variable required in the algorithm, calculated by multiplying each line from the fluency matrix (5) with the corresponding element from array $c_{1,i}$ and replacing element $F_{ii}$ with a ·.
  $H_{1,1}=\left[\begin{array}{ccc}·& 1& 0\\ 0& 0& 0\\ 0& 0& 0\end{array}\right]$ for x; $H_{1,2}=\left[\begin{array}{ccc}0& 0& 0\\ \overline{1}& ·& \overline{1}\\ 0& 0& 0\end{array}\right]$ for y; $H_{1,3}=\left[\begin{array}{ccc}0& 0& 0\\ 0& 0& 0\\ \overline{1}& \overline{1}& ·\end{array}\right]$ for z.
• Next, variable $p_{1,i}$ will be used to count the number of linear elements from $H_{1,i}$ and $q_{1,i}$ is storing the number of non-linear elements from $H_{1,i}$, for each state variable $x_i$.

  $$\left\{\begin{array}{c}{p}_{1,1}=1\hfill \\ q_{1,1}=0\hfill \end{array}\right.\mathrm{for}x;\left\{\begin{array}{c}{p}_{1,2}=0\hfill \\ q_{1,2}=2\hfill \end{array}\right.\mathrm{for}y\mathrm{and}\left\{\begin{array}{c}{p}_{1,3}=0\hfill \\ q_{1,3}=2\hfill \end{array}\right.\mathrm{for}z.$$
• Going further, the · elements from matrices $H_{1,i}$ are replaced with their corresponding element from the fluency matrix, and the resulting matrix is transposed:
  $H_{1,1}=\left[\begin{array}{ccc}1& 1& 0\\ 0& 0& 0\\ 0& 0& 0\end{array}\right]$→$H_{11}^T=\left[\begin{array}{ccc}1& 0& 0\\ 1& 0& 0\\ 0& 0& 0\end{array}\right]$ for x
  $H_{1,2}=\left[\begin{array}{ccc}0& 0& 0\\ \overline{1}& 1& \overline{1}\\ 0& 0& 0\end{array}\right]$→$H_{12}^T=\left[\begin{array}{ccc}0& \overline{1}& 0\\ 0& 1& 0\\ 0& \overline{1}& 0\end{array}\right]$ for y
  $H_{1,3}=\left[\begin{array}{ccc}0& 0& 0\\ 0& 0& 0\\ \overline{1}& \overline{1}& 1\end{array}\right]$→$H_{13}^T=\left[\begin{array}{ccc}0& 0& \overline{1}\\ 0& 0& \overline{1}\\ 0& 0& 1\end{array}\right]$ for z
• Arrays $c_{2,i}$ are computed by summing the elements of the lines of the previously defined matrices $H_{1,i}^T$ (1 and $\overline{1}$ are considered as 1):
  $c_{2,1}=\left[\begin{array}{c}1\\ 1\\ 0\end{array}\right]$ for x $c_{2,2}=\left[\begin{array}{c}1\\ 1\\ 1\end{array}\right]$ for y and $c_{2,3}=\left[\begin{array}{c}1\\ 1\\ 1\end{array}\right]$ for z.
• One of the final steps of the procedure used to calculate the observability coefficients for the Lorenz system consists in calculating the $H_{2,i}$ matrices. They are created by replacing any non-zero element from $H_{1,i}^T$ with a · and the zero elements with the corresponding terms from the fluency matrix multiplied by the corresponding element from array $c_{2,i}$
  $H_{21}=\left[\begin{array}{ccc}·& 1& 0\\ ·& 1& \overline{1}\\ 0& 0& 0\end{array}\right]$ for x, $H_{22}=\left[\begin{array}{ccc}1& ·& 0\\ \overline{1}& ·& \overline{1}\\ \overline{1}& ·& 1\end{array}\right]$ for y and, $H_{23}=\left[\begin{array}{ccc}1& 1& ·\\ \overline{1}& 1& ·\\ \overline{1}& \overline{1}& ·\end{array}\right]$ for z
• Similarly to $p_{1,i}$, the quantities $p_{2,i}$ will count the number of linear elements from $H_{2,i}$ and $q_{2,i}$ the number of non-linear elements from $H_{2,i}$, for each state variable.

  $$\left\{\begin{array}{c}{p}_{2,1}=2\hfill \\ q_{2,1}=1\hfill \end{array}\right.\mathrm{for}x\left\{\begin{array}{c}{p}_{2,2}=2\hfill \\ q_{2,2}=3\hfill \end{array}\right.\mathrm{for}y\left\{\begin{array}{c}{p}_{2,3}=3\hfill \\ q_{2,3}=3\hfill \end{array}\right.\mathrm{for}z.$$
• The observability coefficient for each state variable, ${\eta }_i$, is computed based on the below formulas:

  $${\eta }_i={\displaystyle \frac{1}{2}}·\left[{\displaystyle \frac{p_{i,1}}{p_{i,1}+q_{i,1}}}+{\displaystyle \frac{q_{i,1}}{{(p_{i,1}+q_{i,1})}^3}}+{\displaystyle \frac{p_{i,2}}{p_{i,2}+q_{i,2}}}+{\displaystyle \frac{q_{i,2}}{{(p_{i,2}+q_{i,2})}^2}}\right]$$

  (6)

  where $p_i+q_i=1+q_i$ for $p_i=0$.
  The observability coefficients for the Lorenz system are as follows:

  $$\left\{\begin{array}{c}{\eta }_1=0.88\mathrm{for}x\hfill \\ {\eta }_2=0.27\mathrm{for}y\hfill \\ {\eta }_3=0.32\mathrm{for}z.\hfill \end{array}\right.$$

The results of the observability analysis for the Lorenz system are combined with the ones from [20] in

Table 1. Observability coefficients per state variable and chaotic system analyzed.

Chaotic System	${\mathit{\eta }}_1$ Observability Coefficient	${\mathit{\eta }}_2$ Observability Coefficient	${\mathit{\eta }}_3$ Observability Coefficient
Clifford (discrete)	0.54	0.54	Not Applicable (NA)
Ikeda (discrete)	0.17	0.17	NA
Tinkerbell (discrete)	0.44	0.88	NA
Lorenz (continuous)	0.88	0.27	0.32

. This presents data related to the observability characteristics of the chaotic systems under study. The table is designed to provide detailed insights into how different chaotic systems or configurations perform in terms of observability, which is a crucial property for evaluating their suitability for cryptographic applications.

The observability coefficient results for the Lorenz system (specifically for variables y, z) look promising and comparable with the best results obtained for the discrete systems analyzed in [20] (Ikea, Clifford and Tinkerbell). As mentioned in that paper, the observability characteristics of a chaotic system represent only part of the big picture, so we need to continue the analysis with the singularity manifolds.

3.2. Singularity

Another critical concept closely related to observability is singularity, which will be explored in the following sections. A singularity manifold refers to the region where the system loses its observability. In cryptographic applications, it is crucial for the system to be non-singular, which implies that the system remains entirely observable from the selected state variable, denoted as $S_{\overline{O}}=\varnothing$.

The methodology for identifying singularity regions in the Lorenz chaotic system, along with the results obtained, is described next. The connection between the current and subsequent iterations in the phase space for the state variable $x_i$ (x, y, and z) is given by ${\Phi }_i$ (7):

$${\Phi }_i:\left\{\begin{array}{c}A=s=x_i\hfill \\ B=s^{+}={x_i}^{+}\hfill \\ C=s^{++}={x_i}^{++}\hfill \end{array}\right.$$

(7)

Here, ${\Phi }_i$ represents the transformation function that maps the state variable $x_i$ from its current state s to the next states $s^{+}$ and $s^{++}$. Determining singularity regions is vital to ensure that the system maintains observability across all states, thereby ensuring its robustness and security in cryptographic contexts.

Variables A, B, and C correspond to iterations k, $k+1$, and $k+2$, respectively. The observability matrix $O_i$, corresponding to the state variable $x_i$ of a non-linear dynamical system, is equal to the Jacobian of the map ${\Phi }_i$. The matrix $O_i$ is given by (8):

$$O_i=\left(\begin{array}{ccc}{\displaystyle \frac{\delta A}{\delta x_1}}& {\displaystyle \frac{\delta A}{\delta x_2}}& {\displaystyle \frac{\delta A}{\delta x_3}}\\ {\displaystyle \frac{\delta B}{\delta x_1}}& {\displaystyle \frac{\delta B}{\delta x_2}}& {\displaystyle \frac{\delta B}{\delta x_3}}\\ {\displaystyle \frac{\delta C}{\delta x_1}}& {\displaystyle \frac{\delta C}{\delta x_2}}& {\displaystyle \frac{\delta C}{\delta x_3}}\end{array}\right)$$

(8)

The chaotic system is fully observable if $\det \left(O_i\right)\ne 0$ for all iterations. This condition ensures that the map ${\Phi }_i$ is invertible and that the system can always be represented in an iterative form as shown in (9):

$$\left\{\begin{array}{c}{A}^{+}=B\hfill \\ B^{+}=C\hfill \\ C^{+}=F_i(A,B,C)\hfill \end{array}\right.$$

(9)

where the function $F_i$ does not have singularities, and the index i denotes the state variable. If the determinant of $O_i$ is zero, the system is not fully observable. The singularity manifold for the state variable $x_i$ is mathematically described by:

$$S_{{\overline{O}}_i}=\{(x_1,x_2,x_3)\in {\mathbb{R}}^3\mid \det \left(O_i\right)=0\}$$

(10)

For the Lorenz system, the singularity manifolds for the three state variables are calculated below.

$${\Phi }_1:\left\{\begin{array}{c}A=s=x\hfill \\ B=s^{+}=x^{+}=\sigma ·(y-x)C=-{\sigma }^2·(y-x)+\sigma ·(x·(\rho -z)-y)\hfill \end{array}\right.$$

(11)

$$O_1=\left(\begin{array}{ccc}1& 0& 0\\ \sigma & \sigma & 0\\ {\sigma }^2+\sigma ·(\rho -z)& -{\sigma }^2-\sigma & -\sigma ·x\end{array}\right)$$

(12)

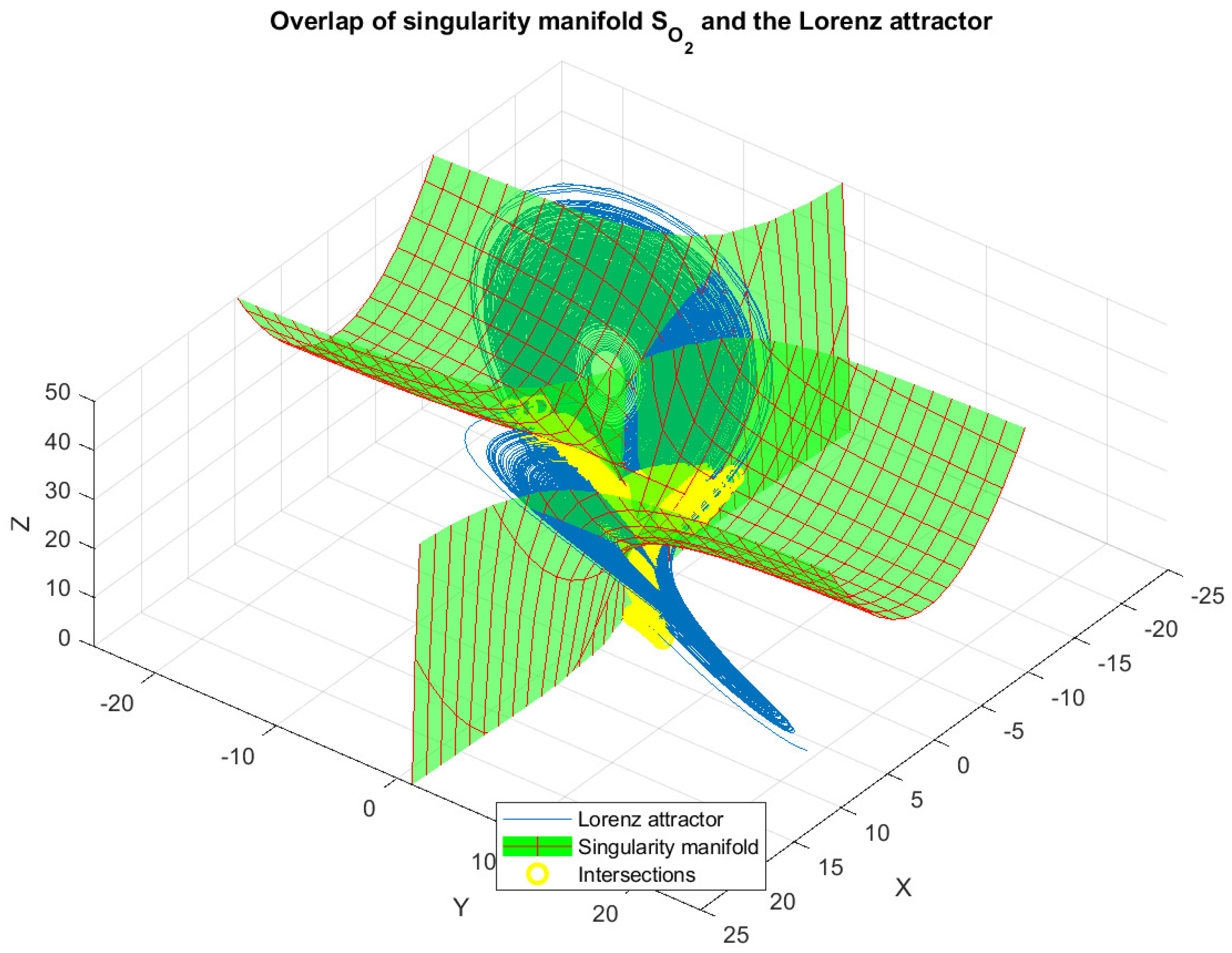
The singularity manifold for variable x, resulting from $det\left(O_1\right)=0$, translates to x = 0 and can be visualized in Figure 1. We used a similar approach as in [20] and considered the overlap of the Lorenz attractor with a band of $\pm 1$ around x = 0 (similar to the discrete systems from the previous paper, where a $\pm 0.1$ band was considered, in both cases, the values correspond to approx. 10% of the system’s support).

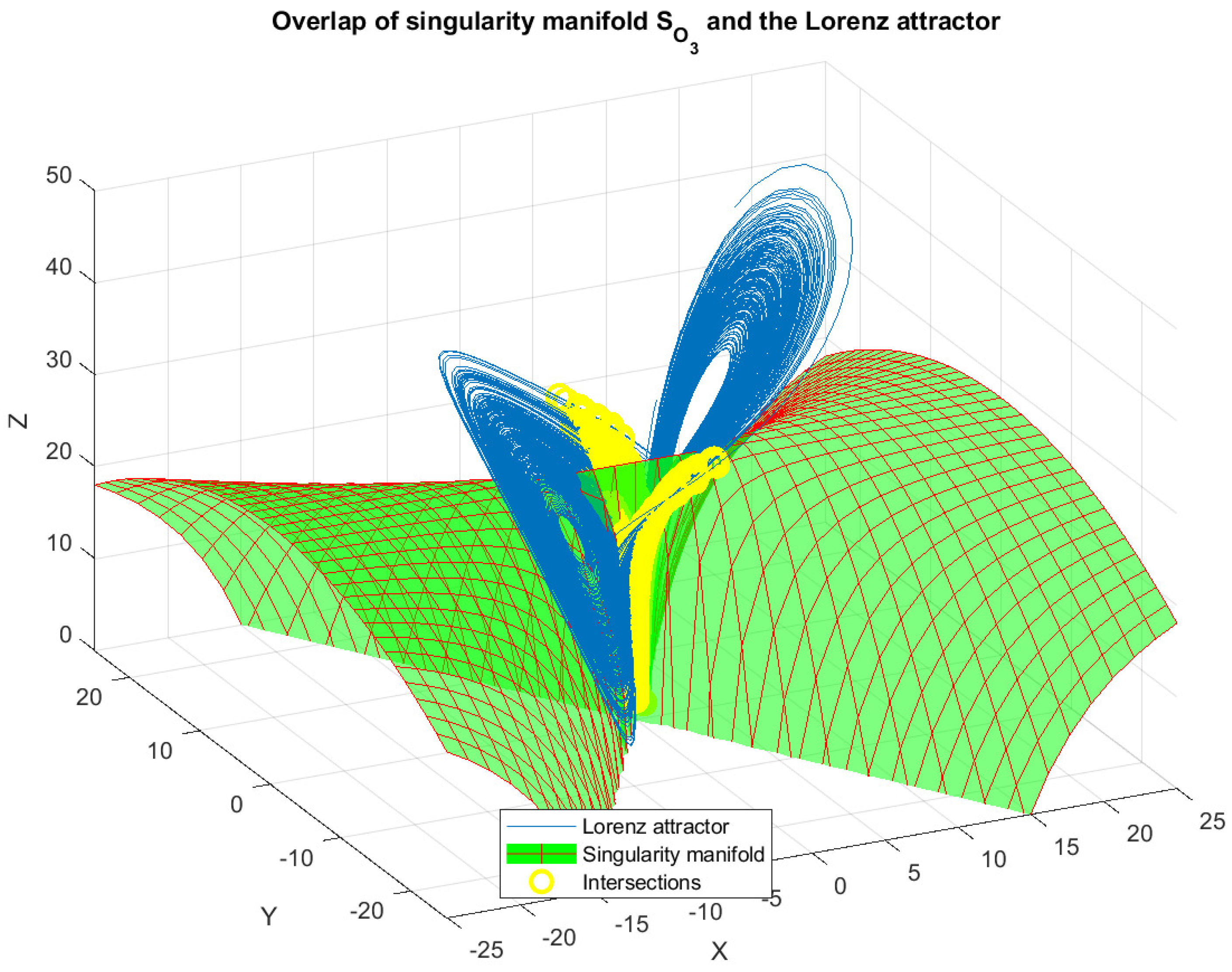
The singularity manifolds for the remaining two state variables can be calculated similarly as for x and the results are aligned with the ones obtained in [24].

Equations (13) and (14) mathematically describe the singularity areas for y and z and Figure 2 and Figure 3 depict them.

$$\begin{array}{cc}\hfill S_{{\overline{O}}_2}& =\{(x,y,z)\in {\mathbb{R}}^3|det\left(O_2\right)=\hfill \\ \hfill & =\rho -{\displaystyle \frac{\rho ·\beta ·x}{\sigma ·y}}+{\displaystyle \frac{2·x^2}{y}}=0\}\hfill \end{array}$$

(13)

$$\begin{array}{cc}\hfill S_{{\overline{O}}_3}& =\{(x,y,z)\in {\mathbb{R}}^3|det\left(O_3\right)=\hfill \\ \hfill & =\rho -\sigma {\displaystyle \frac{y^2}{x^2}}=0\}\hfill \end{array}$$

(14)

The singularity results for the Lorenz system and the reference chaotic discrete systems from [20] are combined in

Table 2. Overlap percentage between attractor and singularity areas for each chaotic system analyzed.

Chaotic System	Overlap Attractor and ${\mathit{S}}_{{\overline{\mathit{O}}}_1}$	Overlap Attractor and ${\mathit{S}}_{{\overline{\mathit{O}}}_2}$	Overlap Attractor and ${\mathit{S}}_{{\overline{\mathit{O}}}_3}$
Clifford (discrete)	12.26%	22.02	NA%
Ikeda (discrete)	0%	0.41	NA%
Tinkerbell (discrete)	2.84%	4.83	NA%
Lorenz (continuous)	11.84%	3.04	3.50%

.

From a singularity point of view, the Lorenz system has a mixed performance, being somehow in between the best and the worst systems analyzed (note: a small percentage of overlap means that the system is more observable than a bigger percentage). This conclusion could have been drawn from the observability coefficient values calculated above. The conclusion from [20] stands true for continuous chaotic systems as well: a high observability coefficient for a specific state variable translates into a high percentage of overlap between the singularity region and the attractor of the respective chaotic system.

3.3. Statistical Independence

The statistical independence for the Lorenz system was studied in detail in [27] and was based on work from [25,26,33].

The primary objective of this investigation was to determine the independence distance/time of the Lorenz chaotic system and assess the impact of sampling frequency on this independence. To achieve this, we followed a structured methodology.

Firstly, we generated 10,000 random initial conditions (x, y, and z) and solved the Lorenz equations for each set within a 0–100 s time frame, using various time steps. For instance, with a sampling frequency ($F_S$) of 100 Hz, solutions were calculated at $t=0.01\mathrm{s}$. This resulted in 10,000 spatial trajectories aligning with the Lorenz attractor.

We then applied the spatial Chi-Square independence test using data beyond the transient time. The variables X and Y were selected from the coordinates of $s_n$ and $s_{n+d}$, respectively, with d representing the independence distance. This process was conducted for each of the three dimensions (x, y, and z), and the smallest d where all tests passed was identified as the independence distance.

Transient time, the period before the system stabilizes was evaluated and found to be around one second. Figure 4 shows that after one second, the probability density functions stabilized. Hence, we evaluated statistical independence after this transient period.

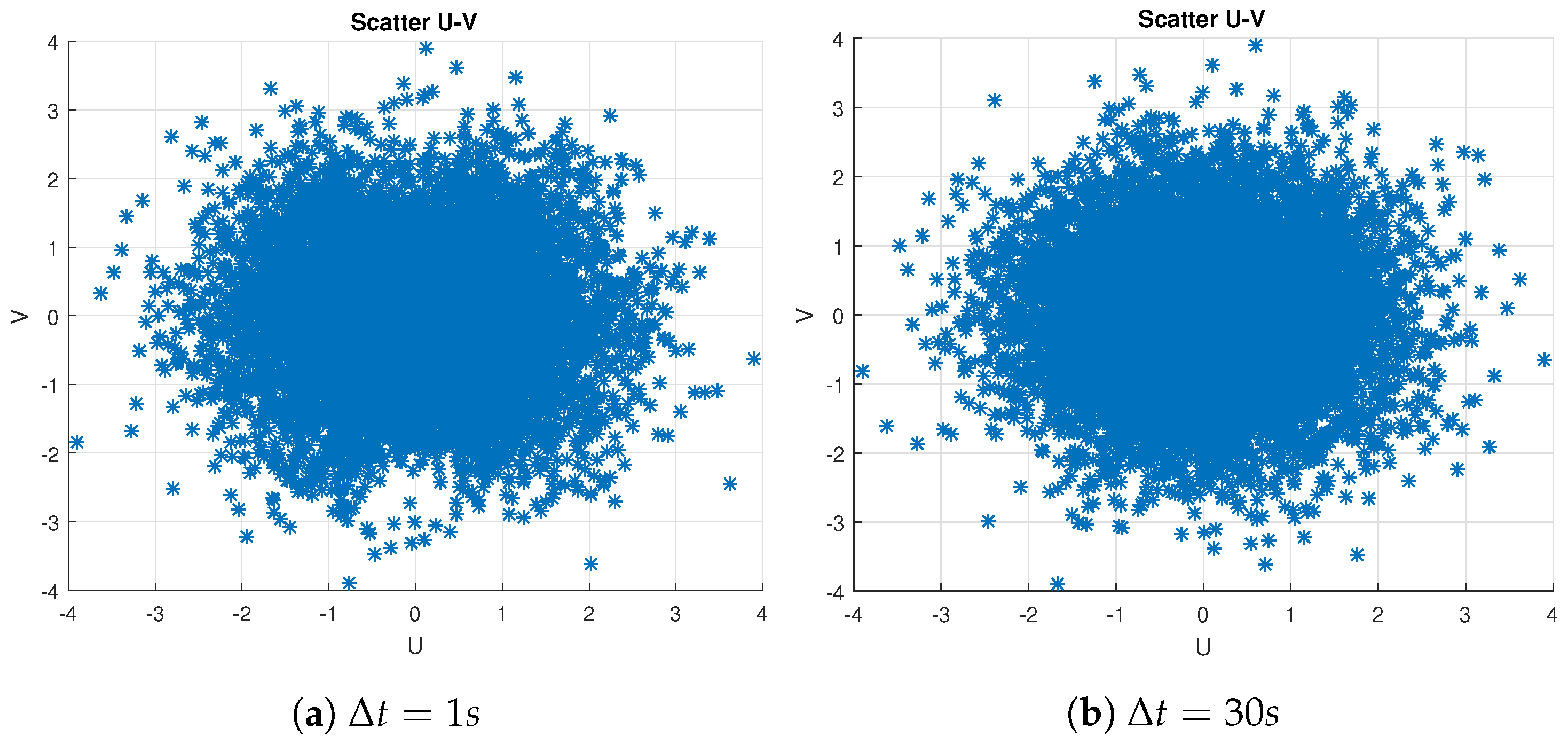
Data were collected at two seconds and subsequently in steps of $1/F_S$ until independence was reached according to the spatial Chi Square test. It was observed that the independence time was approximately three seconds regardless of the sampling frequency (10 Hz or 100 Hz). Figure 5a,b illustrate this, showing the independence test for the y dimension at different intervals. The scatter diagrams demonstrate the test’s failure at 0.5 s and success at 30 s.

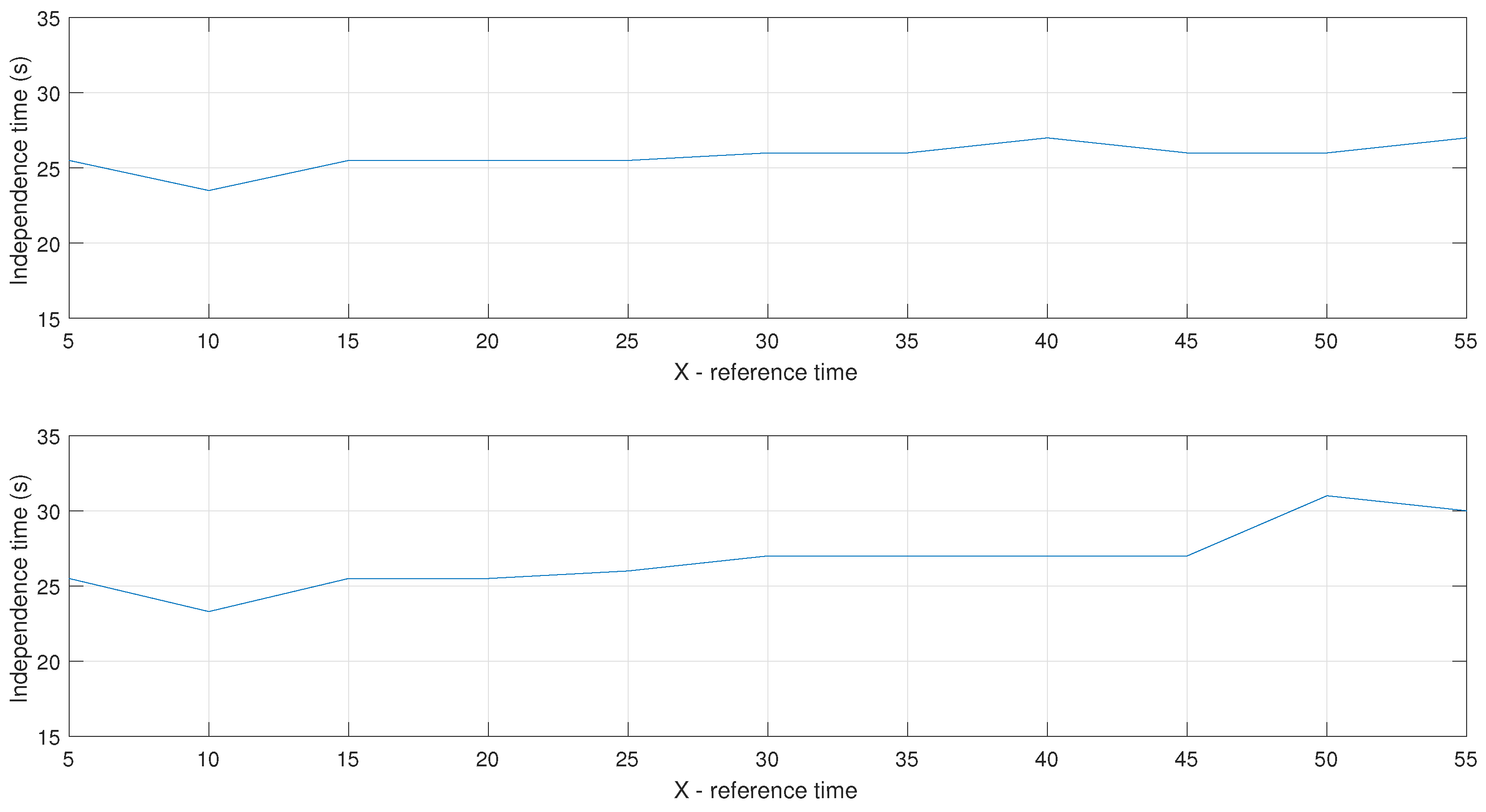
Figure 6 depicts the independence time based on the start sampling moment. Results showed minimal variation for $F_S=100\mathrm{Hz}$ and slightly more for $F_S=10\mathrm{Hz}$, yet they aligned closely. Empirical evidence indicates that the independence time for the Lorenz chaotic system is consistent across different sampling frequencies, with approximately 30 s ensuring statistical independence.

In summary, the independence time of the Lorenz system is unaffected by the sampling frequency, and a time difference of about 30 s ensures statistical independence.

4. Conclusions

The concluding section summarizes the primary findings of the research and reflects on their significance. We provide a concise overview of the study’s contributions, highlighting the enhanced understanding of chaotic systems and their application in cryptography. The conclusions also address potential future research directions, suggesting areas where additional studies could expand upon the insights gained from this work. This summary encapsulates the impact of our research and offers guidance for future investigations in the field.

This paper evaluates the Lorenz chaotic system from three perspectives: observability, singularity, and statistical independence, extending previous research in this field.

The Lorenz system, characterized by its sensitivity to initial conditions and chaotic behavior, was analyzed for its cryptographic properties. Observability was assessed by reconstructing the system’s phase space using its state variables. The observability coefficients for the Lorenz system were found to be comparable to the best results from other discrete chaotic systems like Clifford, Ikeda, and Tinkerbell, indicating its potential utility in cryptographic applications.

Singularity analysis was conducted to ensure the system remains observable across all states, which is crucial for maintaining robustness and security. The singularity manifolds, where observability is lost, were identified, highlighting the importance of choosing the appropriate state variables for cryptographic use.

Statistical independence was tested using the chi-square method to determine if the transformed datasets followed a Gaussian distribution, implying independence. The results confirmed that the original datasets derived from the Lorenz system were statistically independent, reinforcing their suitability for cryptographic purposes.

Overall, the holistic approach combining observability, singularity, and statistical independence provides a robust framework for evaluating the Lorenz system and other chaotic systems for cryptographic applications. Future research should explore further integration of these analyses to enhance the reliability and security of cryptographic methods based on chaotic systems.