Improving Risk Assessment Model for Cyber Security Using Robust Aggregation Operators for Bipolar Complex Fuzzy Soft Inference Systems

Abstract

Improving a risk assessment technique for the problem of cyber security is required to modify the technique’s capability to identify, evaluate, assess, and mitigate potential cyber threats and ambiguities. The major theme of this paper is to find the best strategy to improve and refine the cyber security risk assessment model. For this, we compute some operational laws for bipolar complex fuzzy soft (BCFS) sets and then propose the BCFS weighted averaging (BCFSWA) operator, BCFS ordered weighted averaging (BCFSOWA) operator, BCFS weighted geometric (BCFSWG) operator, and BCFS ordered weighted geometric (BCFSOWG) operator. Furthermore, we give their properties, such as idempotency, monotonicity, and boundedness. Additionally, we improve the risk assessment technique for the cyber security model based on the proposed operators. We illustrate the technique of multi-attribute decision-making (MADM) problems for the derived operators based on BCFS information. Finally, we compare our ranking results with those of some existing operators for evaluating and addressing the supremacy, validity, and efficiency of these operators under BCFS information.

1. Introduction

The application of cyber security plays a critical and valuable role in the field of artificial intelligence and machine learning, and so a risk assessment model for cyber security is very important. However, improving the risk assessment technique for cyber security becomes an awkward and challenging task. Many applications have been presented by different scholars based on the risk assessment model for cyber security under the consideration of the classical set theory. But, in the case of the classical set theory, we may encounter problems because crisp sets are limited with only the two values of zero and one. During the decision-making procedure, we may lose a lot of information due to these limited options with crisp sets. To solve this kind of problem, Zadeh [1] initiated the fuzzy sets (FSs) theory by extending the range of sets to be between zero and one. FSs have various applications, with uncertainty arising from partial belongingness, such as in [2,3,4,5]. The implementation of bipolar FSs (BFSs) is necessary because, in the presence of the positive truth grade only, it is sometimes awkward and ambiguous to handle some problems. In many situations, we may face a problem that contains a positive truth grade as well as a negative truth grade; therefore, a positive truth grade alone is not able to cope with unreliable and complicated information in genuine life problems. For this, Zhang [6] initiated the theory of BFSs, which covered the positive truth grade and negative truth grade in two different directions, where the range of the positive truth grade is [0, 1], and the range of the negative truth grade is [−1, 0]. Thus, FSs are the dominant case of BFSs because of some limitations in FSs, and so BFSs are used to improve FSs. Because of their advantages, researchers have applied BFSs in different fields, such as in [7,8,9].

Complexity and ambiguity are a part of every decision-making procedure because, in many situations, a lot of experts need to face two-dimensional information problems. Since the theory of FSs and BFSs only deals with one-dimensional information, FSs and BFSs are not able to cope with unreliable and vague information. Therefore, Ramot et al. [10] initiated complex FSs (CFSs); the positive truth grade in CFSs is constructed in the shape of complex numbers whose real and imaginary parts are defined from any universal set to unit interval. Moreover, the positive complex-valued truth grade is not able to deal with vague and unreliable data because, in many problems, the negative truth grades are also involved, and so the theory of CFSs has not worked properly or effectively. Therefore, Mahmood and Ur Rehman [11] initiated the power theory of bipolar CFSs (BCFSs). The positive and negative complex-valued truth grades are the parts of the BCFSs where the range of the positive (real and imaginary) truth grade is [0, 1] and the range of negative (real and imaginary) truth grade is [−1, 0]. BCFSs are wider than the prevailing ideas of FSs, BFSs, and CFSs, so they can cope with unreliable and vague information. After the proposal of BCFSs, scholars have utilized them in many fields, such as in [12,13].

The soft set (SS), which is a modified version of FSs, was initiated by Molodtsov [14] in 1999. The idea of SSs is defined from the set of parameters to the power set of a universal set. If we put any parameter to a function, then the resultant value of the parameter will be the subset of the universal set. Further, Roy and Maji [15] derived fuzzy SSs (FSSs), where FSSs are the combination of FSs and SSs. Moreover, Abdullah et al. [16] presented bipolar FSSs (BFSSs). BFSSs are mixtures of BFSs and SSs and are very reliable and dominant sets, which can cope with unreliable or vague information in real-life problems. Additionally, complex FSSs (CFSSs) were considered by Thirunavukarasu et al. [17]. Furthermore, Mahmood et al. [18] derived bipolar CFSSs (BCFSSs) with applications in decision-making problems. We observe that many researchers have developed different kinds of operators on FSs, BFSs, CFSs, BCFSs, SSs, and BFSSs, for instance, aggregation operators (AOs) for FSs [19], geometric and power operators for CFSs [20,21], AOs for BCFSs [22,23], AOs for SSs [24], robust AOs for BFSSs [25], and Aczel–Alsina power AOs for BFSs [26].

From the above analysis, we observe that BCFSSs are very reliable and dominant because of their structure, in which the FSs, BFSs, SSs, CFSs, BCFSs, FSSs, CFSSs, and BFSSs are the special cases of BCFSSs. Although Mahmood et al. [18] initiated these BCFSSs, up until now, there have been no AOs for BCFSSs with application in a risk assessment for cyber security. Since improving a risk assessment technique for cyber security is important and requires modification of the technique’s capability to identify, evaluate, assess, and mitigate potential cyber threats and ambiguities, we advance the study by improving a risk assessment model for the problem of cyber security based on the proposed AOs for BCFSSs. Motivated by the above-mentioned works, the major contributions of this paper are listed as follows:

(1)

To compute some algebraic operational laws for BCFSSs.

(2)

To propose BCFSWA, BCFSOWA, BCFSWG, and BCFSOWG operators with their properties, such as idempotency, monotonicity, and boundedness.

(3)

To improve the risk assessment technique for the cyber security model based on the proposed BCFSWA, BCFSOWA, BCFSWG, and BCFSOWG operators.

(4)

To illustrate the technique of MADM problems for these derived operators based on BCFS information.

(5)

To compare our ranking results with those of existing operators for evaluating or addressing the supremacy and validity of the proposed operators.

This paper is arranged as follows. In Section 2, we review the theory of BCFSSs, and after this, we provide some operational laws such as algebraic laws, score values, and accuracy values for BCFSSs. In Section 3, we compute some operational laws for BCFSSs. Furthermore, we consider the BCFSWA operator, BCFSOWA operator, BCFSWG operator, and BCFSOWG operator, and then give their properties, such as idempotency, monotonicity, and boundedness. In Section 4, we improve the risk assessment technique for the cyber security model based on the proposed operators. For this, we illustrate the technique of MADM problems for the derived operators based on BCFS information. In Section 5, we compare our ranking results with the ranking results of existing operators for evaluating or addressing the supremacy and validity of the proposed operators. Some concluding remarks are stated in Section 6.

2. Preliminaries

In this section, we review the theory of bipolar complex fuzzy soft (BCFS) sets (BCFSSs), and after that, we derive some operational laws such as algebraic laws, score value, and accuracy value for BCFSSs. Further, we list all symbols used in this paper with their meanings, as shown in

Table 1. Representation of symbols with their meanings.

Symbols	Meanings	Symbols	Meanings	Symbols	Meanings
$\mathbb{Y}$	A universal set	${\mathbb{U}}^I$	The imaginary part of truth grade	$SC\left({\mathbb{B}}_{BC}^{ij}\right)$	Score value of ${\mathbb{B}}_{BC}^{ij}$
$\mathbb{A}$	A set of parameters	${\mathbb{V}}^R$	The real part of falsity grade	$AC\left({\mathbb{B}}_{BC}^{ij}\right)$	Accuracy value of ${\mathbb{B}}_{BC}^{ij}$
$\mathbb{E}$	A subset of parameters	${\mathbb{V}}^I$	The imaginary part of falsity grade	${\mathbb{S}}_{sc}$	A scale number
${\mathbb{U}}^R$	The real part of truth grade	${\mathbb{y}}_i$	An element of the universal set $\mathbb{Y}$	${\mathbb{S}}_{\mathsf{\Omega }}^j$ and ${\mathbb{S}}_{\mathsf{\Psi }}^i$	Weights
$z=\sqrt{-1}$	The complex number i	${\mathbb{B}}_{BC}^{ij}$	A BCFSN	$e_i$	A parameter $\in \mathbb{E}$

.

Definition 1.

(Ref. [18]). Let $\mathbb{Y}$ be a universal set and let$\mathbb{A}$ be a parameter set with$\mathbb{E}\subset \mathbb{A}$. A bipolar complex fuzzy set (BCFS)${\mathbb{B}}_{BCFS}$ of$$ is defined as the following:

$${\mathbb{B}}_{BCFS}=\left\{\left({\mathbb{y}}_i,{\mathbb{U}}^R\left({\mathbb{y}}_i\right)+z{\mathbb{U}}^I\left({\mathbb{y}}_i\right),{\mathbb{V}}^R\left({\mathbb{y}}_i\right)+z{\mathbb{V}}^I\left({\mathbb{y}}_i\right)\right):{\mathbb{y}}_i\in \mathbb{Y},z=\sqrt{-1}\right\}.$$

Further, the pair$\left({\mathbb{B}}_{BCFSS},\mathbb{E}\right)$is called a BCFSS over$\mathbb{Y}$for$\mathbb{E}\subset \mathbb{A},$where${\mathbb{B}}_{BCFSS}: \mathbb{E}\to BCFS\left(\mathbb{Y}\right),$and$$represents the collection of BCFSs of$\mathbb{Y}$.

Notice that${\mathbb{U}}^R,{\mathbb{U}}^I:\mathbb{Y}\to \left[0,1\right]$and${\mathbb{V}}^R,{\mathbb{V}}^I:\mathbb{Y}\to \left[-1,0\right]$are called supporting truth grade and supporting against truth grade with${\mathbb{U}}^R\left(\mathbb{y}\right),{\mathbb{U}}^I\left(\mathbb{y}\right)\in \left[0,1\right]$and${\mathbb{V}}^R\left(\mathbb{y}\right),{\mathbb{V}}^I\left(\mathbb{y}\right)\in \left[-1,0\right]$. Additionally, the simple shape of a BCFS number (BCFSN) is described as${\mathbb{B}}_{BC}^{ij}=\left({\mathbb{U}}_{ij}^R+z{\mathbb{U}}_{ij}^I,{\mathbb{V}}_{ij}^R+z{\mathbb{V}}_{ij}^I\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$.

We next give the newly defined operators $\oplus$ and $\otimes$ for any two BCFSNs ${\mathbb{B}}_{BC}^{11}$ and ${\mathbb{B}}_{BC}^{12}$ and also the score and accuracy values for any BCFSN ${\mathbb{B}}_{BC}^{ij}$ as follows.

Definition 2.

Let$\mathbb{Y}$be a universal set. Then, for any two BCFSNs${\mathbb{B}}_{BC}^{11}$and${\mathbb{B}}_{BC}^{12}$, we define the operators$\oplus$and$\otimes$and the scale product and power as follows:

$${\mathbb{B}}_{BC}^{11}\oplus {\mathbb{B}}_{BC}^{12}=\left(\left({\mathbb{U}}_{11}^R+{\mathbb{U}}_{12}^R-{\mathbb{U}}_{11}^R{\mathbb{U}}_{12}^R\right)+z\left(\left({\mathbb{U}}_{11}^I+{\mathbb{U}}_{12}^I-{\mathbb{U}}_{11}^I{\mathbb{U}}_{12}^I\right)\right),-\left|{\mathbb{V}}_{11}^R\right|\left|{\mathbb{V}}_{12}^R\right|+z\left(-\left|{\mathbb{V}}_{11}^I\right|\left|{\mathbb{V}}_{12}^I\right|\right)\right);$$

$${\mathbb{B}}_{BC}^{11}\otimes {\mathbb{B}}_{BC}^{12}=\left({\mathbb{U}}_{11}^R{\mathbb{U}}_{12}^R+z\left({\mathbb{U}}_{11}^I{\mathbb{U}}_{12}^I\right),\left({\mathbb{V}}_{11}^R+{\mathbb{V}}_{12}^R-{\mathbb{V}}_{11}^R{\mathbb{V}}_{12}^R\right)+z\left({\mathbb{V}}_{11}^I+{\mathbb{V}}_{12}^I-{\mathbb{V}}_{11}^I{\mathbb{V}}_{12}^I\right)\right);$$

$${\mathbb{S}}_{sc}{\mathbb{B}}_{BC}^{11}=\left(\left(1-{\left(1-{\mathbb{U}}_{11}^R\right)}^{{\mathbb{S}}_{sc}}\right)+z\left(1-{\left(1-{\mathbb{U}}_{11}^I\right)}^{{\mathbb{S}}_{sc}}\right),\left(-{\left|{\mathbb{V}}_{11}^R\right|}^{{\mathbb{S}}_{sc}}\right)+z\left(-{\left|{\mathbb{V}}_{11}^I\right|}^{{\mathbb{S}}_{sc}}\right)\right);$$

$${\left({\mathbb{B}}_{BC}^{11}\right)}^{{\mathbb{S}}_{sc}}=\left({\left({\mathbb{U}}_{11}^R\right)}^{{\mathbb{S}}_{sc}}+z{\left({\mathbb{U}}_{11}^I\right)}^{{\mathbb{S}}_{sc}},\left(-1+{\left|1+{\mathbb{V}}_{11}^R\right|}^{{\mathbb{S}}_{sc}}\right)+z\left(-1+{\left|1+{\mathbb{V}}_{11}^I\right|}^{{\mathbb{S}}_{sc}}\right)\right).$$

Definition 3.

Let$\mathbb{Y}$be a universal set. The score and accuracy values for any BCFSN${\mathbb{B}}_{BC}^{ij}$are defined as the following:

$$SC\left({\mathbb{B}}_{BC}^{ij}\right)=\frac{1}{2}\left({\mathbb{U}}_{ij}^R+{\mathbb{V}}_{ij}^R+{\mathbb{U}}_{ij}^I+{\mathbb{V}}_{ij}^I\right)\in \left[-1,1\right]$$

$$AC\left({\mathbb{B}}_{BC}^{ij}\right)=\frac{1}{2}\left({\mathbb{U}}_{ij}^R-{\mathbb{V}}_{ij}^R+{\mathbb{U}}_{ij}^I-{\mathbb{V}}_{ij}^I\right)\in \left[0,1\right]$$

For the above information, we have the following properties. If$SC\left({\mathbb{B}}_{BC}^{11}\right)>SC\left({\mathbb{B}}_{BC}^{12}\right)$, then${\mathbb{B}}_{BC}^{11}>{\mathbb{B}}_{BC}^{12}$, and if$AC\left({\mathbb{B}}_{BC}^{11}\right)>AC\left({\mathbb{B}}_{BC}^{12}\right)$, then${\mathbb{B}}_{BC}^{11}>{\mathbb{B}}_{BC}^{12}.$

3. Robust Aggregation Operators for BCFSSs

In this section, we initiate the concept of the BCFSWA operator, BCFSOWA operator, BCFSWG operator, and BCFSOGA operator and then discuss their properties.

Definition 4.

Let${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$be BCFSNs. The BCFSWA operator for${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$is defined as the following:

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)={\oplus }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{ij}\right)$$

Notice that${\mathbb{S}}_{\Omega }^j, {\mathbb{S}}_{\Psi }^i\in \left[0,1\right]$represent weight vectors with the condition of${\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\Psi }^i=1$and${\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\Omega }^j=1.$

Theorem 1.

Let${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$be BCFSNs. Then, the aggregating value of the BCFSWA operator for${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$is again a BCFSN and can be written as follows:

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)=\left(\begin{array}{c}\left(1-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right).$$

Proof. 

To prove the above result, we use the method of mathematical induction. For this, we have to use $\daleth =1$ and ${\mathbb{S}}_{\mathsf{\Psi }}^i=1$, thus

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{1\mathcal{g}}\right)={\oplus }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\mathbb{B}}_{BC}^{1j}\right)=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^1}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^1}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^1}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^1}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$={\oplus }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^1{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{ij}\right)=BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right).$$

If we use $\mathcal{g}=1$ and ${\mathbb{S}}_{\mathsf{\Omega }}^j=1$, thus

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth 1}\right)=\left({\oplus }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{i1}\right)=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)\end{array}\right)$$

$$=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^1}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^1}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^1}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^1}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$={\oplus }_{j=1}^1{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{ij}\right)=BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)$$

We have proven that $\daleth =\mathcal{g}=1$. Further, if we assume that for $\mathcal{g}={\mathcal{ơ}}_1+1,\daleth ={\mathcal{ơ}}_2$ and $\mathcal{g}={\mathcal{ơ}}_1,\daleth ={\mathcal{ơ}}_2+1$, we can obtain the following results:

$${\oplus }_{j=1}^{{\mathcal{ơ}}_1+1}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^{{\mathcal{ơ}}_2}{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{ij}\right)=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

and

$${\oplus }_{j=1}^{{\mathcal{ơ}}_1}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^{{\mathcal{ơ}}_2+1}{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{ij}\right)=\left(\begin{array}{c}\left(1-{\displaystyle {{\displaystyle \prod }}_{j=1}^{{\mathcal{ơ}}_1}}{\left({\displaystyle {{\displaystyle \prod }}_{i=1}^{{\mathcal{ơ}}_2+1}}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {{\displaystyle \prod }}_{j=1}^{{\mathcal{ơ}}_1}}{\left({\displaystyle {{\displaystyle \prod }}_{i=1}^{{\mathcal{ơ}}_2+1}}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {{\displaystyle \prod }}_{j=1}^{{\mathcal{ơ}}_1}}{\left({\displaystyle {{\displaystyle \prod }}_{i=1}^{{\mathcal{ơ}}_2+1}}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {{\displaystyle \prod }}_{j=1}^{{\mathcal{ơ}}_1}}{\left({\displaystyle {{\displaystyle \prod }}_{i=1}^{{\mathcal{ơ}}_2+1}}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

Then, we prove that the above theory also holds for $\mathcal{g}={\mathcal{ơ}}_1+1$ and $\daleth ={\mathcal{ơ}}_2+1$:

$${\oplus }_{j=1}^{{\mathcal{ơ}}_1+1}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^{{\mathcal{ơ}}_2+1}{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{ij}\right)={\oplus }_{j=1}^{{\mathcal{ơ}}_1+1}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^{{\mathcal{ơ}}_2}{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{ij}\oplus {\mathbb{S}}_{\mathsf{\Psi }}^{{\mathcal{ơ}}_2+1}{\mathbb{B}}_{BC}^{\left({\mathcal{ơ}}_2+1\right)j}\right)$$

$$={\oplus }_{j=1}^{{\mathcal{ơ}}_1+1}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^{{\mathcal{ơ}}_2}{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{ij}\right){\oplus }_{j=1}^{{\mathcal{ơ}}_1+1}{\mathbb{S}}_{\mathsf{\Omega }}^j\oplus {\mathbb{S}}_{\mathsf{\Psi }}^{{\mathcal{ơ}}_2+1}{\mathbb{B}}_{BC}^{\left({\mathcal{ơ}}_2+1\right)j}$$

$$\begin{array}{c}=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\\ \oplus \left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\left(1-{\mathbb{U}}_{\left({\mathcal{ơ}}_2+1\right)j}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{\left({\mathcal{ơ}}_2+1\right)}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\left(1-{\mathbb{U}}_{\left({\mathcal{ơ}}_2+1\right)j}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{\left({\mathcal{ơ}}_2+1\right)}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\left(\left|{\mathbb{V}}_{\left({\mathcal{ơ}}_2+1\right)j}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{\left({\mathcal{ơ}}_2+1\right)}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\left(\left|{\mathbb{V}}_{\left({\mathcal{ơ}}_2+1\right)j}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{\left({\mathcal{ơ}}_2+1\right)}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)\end{array}$$

$$=\left(\begin{array}{c}\left(1-{\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

Hence, the proposed theory holds for values of $\mathcal{g}={\mathcal{ơ}}_1+1$ and $\daleth ={\mathcal{ơ}}_2+1.$

□

We next simplify the above information with the help of a simple example for which the data are shown in

Table 2. BCFS decision matrix for expert one.

${\mathbb{B}}_{\mathit{B}\mathit{C}}^1$	${\mathit{e}}_1$	${\mathit{e}}_2$	${\mathit{e}}_3$	${\mathit{e}}_4$
${\mathbb{B}}_{AT}^1$	$\left(\begin{array}{c}0.8+z\left(0.7\right),\\ -0.2+z\left(-0.3\right)\end{array}\right)$	$\left(\begin{array}{c}0.81+z\left(0.71\right),\\ -0.21+z\left(-0.31\right)\end{array}\right)$	$\left(\begin{array}{c}0.82+z\left(0.72\right),\\ -0.22+z\left(-0.32\right)\end{array}\right)$	$\left(\begin{array}{c}0.83+z\left(0.73\right),\\ -0.23+z\left(-0.33\right)\end{array}\right)$

.

Thus, by using the proposed theory, we obtain the following results:

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},{\mathbb{B}}_{BC}^{13},{\mathbb{B}}_{BC}^{14}\right)=\left(0.8034+z\left(0.4583\right),-0.3513+z\left(-0.3085\right)\right).$$

Property 1

(Idempotency). If ${\mathbb{B}}_{BC}^{ij}={\mathbb{B}}_{BC}=\left({\mathbb{U}}^R+z{\mathbb{U}}^I,{\mathbb{V}}^R+z{\mathbb{V}}^I\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$, then the idempotency property holds for BCFSWA, as

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)={\mathbb{B}}_{BC}$$

Proof. 

We know that ${\mathbb{B}}_{BC}^{ij}={\mathbb{B}}_{BC}=\left({\mathbb{U}}^R+z{\mathbb{U}}^I,{\mathbb{V}}^R+z{\mathbb{V}}^I\right)$; thus

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$=\left(\begin{array}{c}\left(1-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$=\left(\begin{array}{c}\left(1-{\left({\left(1-{\mathbb{U}}^R\right)}^{{\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\left({\left(1-{\mathbb{U}}^I\right)}^{{\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\left({\left(\left|{\mathbb{V}}^R\right|\right)}^{{\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\left({\left(\left|{\mathbb{V}}^I\right|\right)}^{{\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$=\left(\begin{array}{c}\left(1-\left(1-{\mathbb{U}}^R\right)\right)+z\left(1-\left(1-{\mathbb{U}}^I\right)\right),\\ \left(-\left(\left|{\mathbb{V}}^R\right|\right)\right)+z\left(-\left(\left|{\mathbb{V}}^I\right|\right)\right)\end{array}\right)$$

$$=\left({\mathbb{U}}^R+z{\mathbb{U}}^I,{\mathbb{V}}^R+z{\mathbb{V}}^I\right)={\mathbb{B}}_{BC}.$$

□

Property 2

(Monotonicity). If ${\mathbb{B}}_{BC}^{ij}\le {{\mathbb{B}}^{\#}}_{BC}^{ij}$, then

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le BCFSWA\left({{\mathbb{B}}^{\#}}_{BC}^{11},{\mathbb{B}}_{BC}^{\#12},\dots ,{{\mathbb{B}}^{\#}}_{BC}^{\daleth \mathcal{g}}\right)$$

Proof. 

Consider that ${\mathbb{B}}_{BC}^{ij}\le {\mathbb{B}}_{BC}^{{\#}^{ij}}$; thus, ${\mathbb{U}}_{ij}^R\le {\mathbb{U}}_{ij}^{{\#}^R},{\mathbb{U}}_{ij}^I\le {\mathbb{U}}_{ij}^{{\#}^I}$ and ${\mathbb{V}}_{ij}^R\le {\mathbb{V}}_{ij}^{{\#}^R},{\mathbb{V}}_{ij}^I\le {\mathbb{V}}_{ij}^{{\#}^I}$. Then, we simplify the positive truth grade:

$${\mathbb{U}}_{R_{ij}}\le {\mathbb{U}}_{R_{ij}}^{\#}\Rightarrow 1-{\mathbb{U}}_{ij}^R\ge 1-{\mathbb{U}}_{ij}^{{\#}^R}$$

$$\Rightarrow {\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\ge {\left(1-{\mathbb{U}}_{ij}^{{\#}^R}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}$$

$$\Rightarrow {\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\ge {\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^{{\#}^R}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}$$

$$\Rightarrow {\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\ge {\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^{{\#}^R}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

$$\Rightarrow {\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\ge {\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^{{\#}^R}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

$$\Rightarrow -{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\ge -{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^{{\#}^R}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

$$\Rightarrow 1-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\ge 1-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^{{\#}^R}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

Further, we know that

$${\mathbb{U}}_{I_{ij}}\le {\mathbb{U}}_{I_{ij}}^{\#}\Rightarrow 1-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\ge 1-{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(1-{\mathbb{U}}_{ij}^{{\#}^I}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

Moreover, we evaluate it for negative truth grades:

$${\mathbb{V}}_{R_{ij}}\le {\mathbb{V}}_{R_{ij}}^{\#}\Rightarrow \left|{\mathbb{V}}_{ij}^R\right|\ge \left|{\mathbb{V}}_{ij}^{{\#}^R}\right|$$

$$\Rightarrow {\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\ge {\left(\left|{\mathbb{V}}_{ij}^{{\#}^R}\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}$$

$$\Rightarrow {\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\ge {\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^{{\#}^R}\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}$$

$$\Rightarrow {\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\ge {\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^{{\#}^R}\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

$$\Rightarrow {\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\ge {\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^{{\#}^R}\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

$$\Rightarrow -{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\le -{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^{{\#}^R}\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

Further, we know that ${\mathbb{V}}_{ij}^I\le {\mathbb{V}}_{ij}^{{\#}^I}\Rightarrow -{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\le -{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|{\mathbb{V}}_{ij}^{{\#}^I}\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$. Thus, according to the definition of the score value $SC\left({\mathbb{B}}_{BC}^{ij}\right)$, we can easily derive the following inequality: $BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le BCFSWA\left({\mathbb{B}}_{BC}^{{\#}^{11}},{\mathbb{B}}_{BC}^{{\#}^{12}},\dots ,{\mathbb{B}}_{BC}^{{\#}^{\daleth \mathcal{g}}}\right).$ Thus, the proof is completed.

□

Property 3

(Boundedness). When ${\mathbb{B}}_{BC}^{-}=\left(\underset{i}{min} \underset{j}{min}{\mathbb{U}}_{ij}^R+z\left(\underset{i}{min} \underset{j}{min}{\mathbb{U}}_{ij}^I\right),\underset{i}{max} \underset{j}{max}{\mathbb{V}}_{ij}^R+z\left(\underset{i}{max} \underset{j}{max}{\mathbb{V}}_{ij}^I\right)\right)$ and${\mathbb{B}}_{BC}^{+}=\left(\underset{i}{max} \underset{j}{max}{\mathbb{U}}_{ij}^R+z\left(\underset{i}{max} \underset{j}{max}{\mathbb{U}}_{ij}^I\right),\underset{i}{min} \underset{j}{min}{\mathbb{V}}_{ij}^R+z\left(\underset{i}{min} \underset{j}{min}{\mathbb{V}}_{ij}^I\right)\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$, we know that

$${\mathbb{B}}_{BC}^{-}\le BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le {\mathbb{B}}_{BC}^{+}$$

Proof. 

Consider that ${\mathbb{B}}_{BC}^{-}=\left(\underset{i}{\min } \underset{j}{\min }{\mathbb{U}}_{ij}^R+z\left(\underset{i}{\min } \underset{j}{\min }{\mathbb{U}}_{ij}^I\right),\underset{i}{\max } \underset{j}{\max }{\mathbb{V}}_{ij}^R+z\left(\underset{i}{\max } \underset{j}{\max }{\mathbb{V}}_{ij}^I\right)\right)$ and ${\mathbb{B}}_{BC}^{+}=\left(\underset{i}{\max } \underset{j}{\max }{\mathbb{U}}_{ij}^R+z\left(\underset{i}{\max } \underset{j}{\max }{\mathbb{U}}_{ij}^I\right),\underset{i}{\min } \underset{j}{\min }{\mathbb{V}}_{ij}^R+z\left(\underset{i}{\min } \underset{j}{\min }{\mathbb{V}}_{ij}^I\right)\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$. Thus, by using the information for Property 1 and Property 2, we know that

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le BCFSWA\left({\mathbb{B}}_{BC}^{+11},{\mathbb{B}}_{BC}^{+12},\dots ,{\mathbb{B}}_{BC}^{+\daleth \mathcal{g}}\right)={\mathbb{B}}_{BC}^{+}$$

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\ge BCFSWA\left({\mathbb{B}}_{BC}^{-11},{-}_{BC}^{-12},\dots ,{\mathbb{B}}_{BC}^{-\daleth \mathcal{g}}\right)={\mathbb{B}}_{BC}^{-}$$

Then, ${\mathbb{B}}_{BC}^{-}\le BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le {\mathbb{B}}_{BC}^{+}.$

□

Definition 5.

Let${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$be BCFSNs. The BCFSOWA operator for${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$is defined as the following:

$$BCFSOWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)={\oplus }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j\left({\oplus }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i{\mathbb{B}}_{BC}^{o\left(i\right)o\left(j\right)}\right)$$

Notice that${\mathbb{S}}_{\Omega }^j, {\mathbb{S}}_{\mathsf{\Psi }}^i\in \left[0,1\right]$represent weight vectors with a strong condition,${\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i=1$and${\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\Omega }^j=1$, with order$o\left(i\right),o\left(j\right)\le o\left(i-1\right),o\left(j-1\right)$.

Theorem 2.

Let${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$be BCFSNs. Then, the aggregating value of the BCFSOWA operator is again a BCFSN, as

$$BCFSOWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{o\left(i\right)o\left(j\right)}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{o\left(i\right)o\left(j\right)}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{o\left(i\right)o\left(j\right)}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{o\left(i\right)o\left(j\right)}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

Proof. 

It is similar to Theorem 1.

□

Property 4

(Idempotency). If ${\mathbb{B}}_{BC}^{ij}={\mathbb{B}}_{BC}=\left({\mathbb{U}}^R+z{\mathbb{U}}^I,{\mathbb{V}}^R+z{\mathbb{V}}^I\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$, then the idempotency property holds for BCFSOWA, as

$$BCFSOWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)={\mathbb{B}}_{BC}$$

Proof. 

It is similar to Property 1.

□

Property 5

(Monotonicity). When ${\mathbb{B}}_{BC}^{ij}\le {\mathbb{B}}_{BC}^{{\#}^{ij}}$, we know that

$$BCFSOWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le BCFSOWA\left({\mathbb{B}}_{BC}^{{\#}^{11}},{\mathbb{B}}_{BC}^{{\#}^{12}},\dots ,{\mathbb{B}}_{BC}^{{\#}^{\daleth \mathcal{g}}}\right)$$

Proof. 

It is similar to Property 2.

□

Property 6

(Boundedness). When ${\mathbb{B}}_{BC}^{-}=\left(\underset{i}{min} \underset{j}{min}{\mathbb{U}}_{ij}^R+z\left(\underset{i}{min} \underset{j}{min}{\mathbb{U}}_{ij}^I\right),\underset{i}{max} \underset{j}{max}{\mathbb{V}}_{ij}^R+z\left(\underset{i}{max} \underset{j}{max}{\mathbb{V}}_{ij}^I\right)\right)$ and${\mathbb{B}}_{BC}^{+}=\left(\underset{i}{max} \underset{j}{max}{\mathbb{U}}_{ij}^R+z\left(\underset{i}{max} \underset{j}{max}{\mathbb{U}}_{ij}^I\right),\underset{i}{min} \underset{j}{min}{\mathbb{V}}_{ij}^R+z\left(\underset{i}{min} \underset{j}{min}{\mathbb{V}}_{ij}^I\right)\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$, we know that

$${\mathbb{B}}_{BC}^{-}\le BCFSOWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le {\mathbb{B}}_{BC}^{+}$$

Proof. 

It is similar to Property 3.

□

Definition 6.

Let${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$be BCFSNs. The BCFSWG operator for${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$is defined as the following:

$$BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)={\otimes }_{j=1}^{\mathcal{g}}{\left({\otimes }_{i=1}^{\daleth }{\left({\mathbb{B}}_{BC}^{ij}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

Notice that${\mathbb{S}}_{\Omega }^j, {\mathbb{S}}_{\mathsf{\Psi }}^i\in \left[0,1\right]$represent weight vectors with a strong condition,${\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i=1$and${\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\Omega }^j=1$.

Theorem 3.

Let${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$be BCFSNs. Then, the aggregating value of BCFSWG operator is again a BCFSN and

$$BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)=\left(\begin{array}{c}\left({\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

Proof. 

Using the mathematical induction, it is easy to prove the above result. For this, we have to use $\daleth =1$ and ${\mathbb{S}}_{\mathsf{\Psi }}^i=1$; thus

$$BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{1\mathcal{g}}\right)={\otimes }_{j=1}^{\mathcal{g}}{\left({\mathbb{B}}_{BC}^{1j}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^1}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^1}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^1}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^1}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$={\otimes }_{j=1}^{\mathcal{g}}{\left({\otimes }_{i=1}^1{\left({\mathbb{B}}_{BC}^{ij}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}=BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right).$$

If we use $\mathcal{g}=1$ and ${\mathbb{S}}_{\mathsf{\Omega }}^j=1$, then

$$BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth 1}\right)=\left({\otimes }_{i=1}^{\daleth }{\left({\mathbb{B}}_{BC}^{i1}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)+z\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)\end{array}\right)$$

$$=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^1}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^1}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^1}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^1}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$={\otimes }_{j=1}^1{\left({\otimes }_{i=1}^{\daleth }{\left({\mathbb{B}}_{BC}^{ij}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}=BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)$$

We have proven that $\daleth =\mathcal{g}=1$. Further, we assume that for $\mathcal{g}={\mathcal{ơ}}_1+1,\daleth ={\mathcal{ơ}}_2$ and $\mathcal{g}={\mathcal{ơ}}_1,\daleth ={\mathcal{ơ}}_2+1$. Then, we can also obtain the following results:

$${\otimes }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\otimes }_{i=1}^{{\mathcal{ơ}}_2}{\left({\mathbb{B}}_{BC}^{ij}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

and

$${\otimes }_{j=1}^{{\mathcal{ơ}}_1}{\left({\otimes }_{i=1}^{{\mathcal{ơ}}_2+1}{\left({\mathbb{B}}_{BC}^{ij}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

Then, we prove that the above theory also holds for $\mathcal{g}={\mathcal{ơ}}_1+1$ and $\daleth ={\mathcal{ơ}}_2+1$:

$${\otimes }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\otimes }_{i=1}^{{\mathcal{ơ}}_2+1}{({\mathbb{B}}_{BC}^{ij})}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}={\otimes }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\otimes }_{i=1}^{{\mathcal{ơ}}_2}{\left({\mathbb{B}}_{BC}^{ij}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\otimes {\left({\mathbb{B}}_{BC}^{\left({\mathcal{ơ}}_2+1\right)j}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{{\mathcal{ơ}}_2+1}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

$$={\otimes }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\otimes }_{i=1}^{{\mathcal{ơ}}_2}{\left({\mathbb{B}}_{BC}^{ij}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}{\otimes }_{j=1}^{{\mathcal{ơ}}_1+1}{\left({\left({\mathbb{B}}_{BC}^{\left({\mathcal{ơ}}_2+1\right)j}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{{\mathcal{ơ}}_2+1}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

$$\begin{array}{c}=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2}}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\\ \oplus \left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\left({\mathbb{U}}_{\left({\mathcal{ơ}}_2+1\right)j}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{\left({\mathcal{ơ}}_2+1\right)}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\left({\mathbb{U}}_{\left({\mathcal{ơ}}_2+1\right)j}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{\left({\mathcal{ơ}}_2+1\right)}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\left(\left|1+{\mathbb{V}}_{\left({\mathcal{ơ}}_2+1\right)j}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{\left({\mathcal{ơ}}_2+1\right)}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\left(\left|1+{\mathbb{V}}_{\left({\mathcal{ơ}}_2+1\right)j}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^{\left({\mathcal{ơ}}_2+1\right)}}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)\end{array}$$

$$=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{{\mathcal{ơ}}_1+1}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{{\mathcal{ơ}}_2+1}}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

Hence, the proposed theory holds for values of $\mathcal{g}={\mathcal{ơ}}_1+1$ and $\daleth ={\mathcal{ơ}}_2+1$.

□

Similarly, we simplify the above information with the same data as shown in Table 2. By using the proposed theory, we obtain the following result:

$$BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},{\mathbb{B}}_{BC}^{13},{\mathbb{B}}_{BC}^{14}\right)=\left(0.7906+z\left(0.4121\right),-0.4411+z\left(-0.4608\right)\right).$$

Property 7

(Idempotency). If ${\mathbb{B}}_{BC}^{ij}={\mathbb{B}}_{BC}=\left({\mathbb{U}}^R+z{\mathbb{U}}^I,{\mathbb{V}}^R+z{\mathbb{V}}^I\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$, then the idempotency property holds for BCFSWG, as

$$BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)={\mathbb{B}}_{BC}$$

Proof. 

It is similar to Property 1.

□

Property 8

(Monotonicity). When ${\mathbb{B}}_{BC}^{ij}\le {\mathbb{B}}_{BC}^{{\#}^{ij}}$, we know that

$$BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le BCFSWG\left({\mathbb{B}}_{BC}^{{\#}^{11}},{\mathbb{B}}_{BC}^{{\#}^{12}},\dots ,{\mathbb{B}}_{BC}^{{\#}^{\daleth \mathcal{g}}}\right).$$

Proof. 

It is similar to Property 2.

□

Property 9

(Boundedness). When ${\mathbb{B}}_{BC}^{-}=\left(\underset{i}{min} \underset{j}{min}{\mathbb{U}}_{ij}^R+z\left(\underset{i}{min} \underset{j}{min}{\mathbb{U}}_{ij}^I\right),\underset{i}{max}\underset{j}{max}{\mathbb{V}}_{ij}^R+z\left(\underset{i}{max} \underset{j}{max}{\mathbb{V}}_{ij}^I\right)\right)$ and${\mathbb{B}}_{BC}^{+}=\left(\underset{i}{max} \underset{j}{max}{\mathbb{U}}_{ij}^R+z\left(\underset{i}{max} \underset{j}{max}{\mathbb{U}}_{ij}^I\right),\underset{i}{min}\underset{j}{min}{\mathbb{V}}_{ij}^R+z\left(\underset{i}{min} \underset{j}{min}{\mathbb{V}}_{ij}^I\right)\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$, we know that

$${\mathbb{B}}_{BC}^{-}\le BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le {\mathbb{B}}_{BC}^{+}.$$

Proof. 

It is similar to Property 3.

□

Definition 7.

Let${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$be BCFSN. The BCFSOWA operator for${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$is defined as the following:

$$BCFSOWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)={\otimes }_{j=1}^{\mathcal{g}}{\left({\otimes }_{i=1}^{\daleth }{\left({\mathbb{B}}_{BC}^{o\left(i\right)o\left(j\right)}\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}$$

Notice that${\mathbb{S}}_{\Omega }^j, {\mathbb{S}}_{\mathsf{\Psi }}^i\in \left[0,1\right]$represent weight vectors with a condition of${\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i=1$and${\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\Omega }^j=1$and with the order$o\left(i\right),o\left(j\right)\le o\left(i-1\right),o\left(j-1\right)$.

Theorem 4.

Let${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$be BCFSNs. Then, the aggregating value of BCFSOWA operator is again a BCFSN and

$$BCFSOWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)=\left(\begin{array}{c}\left({\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left({\mathbb{U}}_{o\left(i\right)o\left(j\right)}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left({\mathbb{U}}_{o\left(i\right)o\left(j\right)}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|1+{\mathbb{V}}_{o\left(i\right)o\left(j\right)}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle \prod }_{j=1}^{\mathcal{g}}{\left({\displaystyle \prod }_{i=1}^{\daleth }{\left(\left|1+{\mathbb{V}}_{o\left(i\right)o\left(j\right)}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

Proof. 

It is similar to Theorem 3.

□

Property 10

(Idempotency). If ${\mathbb{B}}_{BC}^{ij}={\mathbb{B}}_{BC}=\left({\mathbb{U}}^R+z{\mathbb{U}}^I,{\mathbb{V}}^R+z{\mathbb{V}}^I\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$, then the idempotency property holds for BCFSOWG, as

$$BCFSOWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)={\mathbb{B}}_{BC}.$$

Proof. 

It is similar to Property 1.

□

Property 11

(Monotonicity). If ${\mathbb{B}}_{BC}^{ij}\le {\mathbb{B}}_{BC}^{{\#}^{ij}}$, then

$$BCFSOWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le BCFSOWG\left({\mathbb{B}}_{BC}^{{\#}^{11}},{\mathbb{B}}_{BC}^{{\#}^{12}},\dots ,{\mathbb{B}}_{BC}^{{\#}^{\daleth \mathcal{g}}}\right).$$

Proof. 

It is similar to Property 2.

□

Property 12

(Boundedness). When ${\mathbb{B}}_{BC}^{-}=\left(\underset{i}{min} \underset{j}{min}{\mathbb{U}}_{ij}^R+z\left(\underset{i}{min} \underset{j}{min}{\mathbb{U}}_{ij}^I\right),\underset{i}{max} \underset{j}{max}{\mathbb{V}}_{ij}^R+z\left(\underset{i}{max} \underset{j}{max}{\mathbb{V}}_{ij}^I\right)\right)$ and${\mathbb{B}}_{BC}^{+}=\left(\underset{i}{max} \underset{j}{max}{\mathbb{U}}_{ij}^R+z\left(\underset{i}{max} \underset{j}{max}{\mathbb{U}}_{ij}^I\right),\underset{i}{min} \underset{j}{min}{\mathbb{V}}_{ij}^R+z\left(\underset{i}{min} \underset{j}{min}{\mathbb{V}}_{ij}^I\right)\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$, we know that

$${\mathbb{B}}_{BC}^{-}\le BCFSOWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)\le {\mathbb{B}}_{BC}^{+}.$$

Proof. 

It is similar to Property 3.

□

4. The MADM Method with Application to the Risk Assessment Model for Cyber Security

To evaluate the supremacy and validity of the proposed operators, we analyze the ability of the MADM technique to evaluate some genuine life problems based on the proposed operators, such as the BCFSWA and BCFSWG operators, to enhance the worth of the derived techniques.

To evaluate the MADM procedure, we require the collection of alternatives ${\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}$, and for each alternative, we have a group of attributes $A_{BC}^{11},A_{BC}^{12},\dots ,A_{BC}^{\daleth \mathcal{g}}$. Further, for every attribute, we require the collection of weight vectors, such as ${\mathbb{S}}_{\mathsf{\Omega }}^j, {\mathbb{S}}_{\mathsf{\Psi }}^i\in \left[0,1\right]$, which represent the weight vectors with a condition of ${\displaystyle \sum }_{i=1}^{\daleth }{\mathbb{S}}_{\mathsf{\Psi }}^i=1$ and ${\displaystyle \sum }_{j=1}^{\mathcal{g}}{\mathbb{S}}_{\mathsf{\Omega }}^j=1$. In general, the pair $\left({\mathbb{B}}_{BCFSS},\mathbb{E}\right)$ is called a BCFSS $,$ and ${\mathbb{B}}_{BCFSS}: \mathbb{E}\to BCFS\left(\mathbb{Y}\right),$ where $BCFS\left(\mathbb{Y}\right)$ represents the collection of BCFSs of $\mathbb{Y}$. Additionally, we calculate the matrix by including the values of BCFS numbers (BCFSNs), where ${\mathbb{U}}^R,{\mathbb{U}}^I:\mathbb{Y}\to \left[0,1\right]$ and ${\mathbb{V}}^R,{\mathbb{V}}^I:\mathbb{Y}\to \left[-1,0\right]$ are called the supporting truth grade and supporting against truth grade and BCFSNs are described as ${\mathbb{B}}_{BC}^{ij}=\left({\mathbb{U}}_{ij}^R+z{\mathbb{U}}_{ij}^I,{\mathbb{V}}_{ij}^R+z{\mathbb{V}}_{ij}^I\right),i,j=1,2,\dots ,\daleth ,\mathcal{g}$. Finally, we illustrate the procedure of the MADM technique for addressing our selected problems. Therefore, the major steps of the MADM technique are listed below:

Step 1: Calculate the matrix of BCFS values; if the matrix covers cost types of information, then we normalize the matrix:

$$N=\left\{\begin{array}{cc}\left({\mathbb{U}}_{ij}^R+z{\mathbb{U}}_{ij}^I,{\mathbb{V}}_{ij}^R+z{\mathbb{V}}_{ij}^I\right)& for benefit\\ \left(1-{\mathbb{U}}_{ij}^R+z\left(1-{\mathbb{U}}_{ij}^I\right),-1-{\mathbb{V}}_{ij}^R+z\left(-1-{\mathbb{V}}_{ij}^I\right)\right)& for cost\end{array}\right..$$

Furthermore, if the matrix contains a benefit type of information, then we are not able to evaluate the matrix.

Step 2: Calculate the aggregated values of the normalized matrix using the theory of BCFSWA and BCFSWG operators:

$$BCFSWA\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)=\left(\begin{array}{c}\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(1-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(1-{\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

$$BCFSWG\left({\mathbb{B}}_{BC}^{11},{\mathbb{B}}_{BC}^{12},\dots ,{\mathbb{B}}_{BC}^{\daleth \mathcal{g}}\right)=\left(\begin{array}{c}\left({\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left({\mathbb{U}}_{ij}^R\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left({\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left({\mathbb{U}}_{ij}^I\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right),\\ \left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|1+{\mathbb{V}}_{ij}^R\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)+z\left(-1+{\displaystyle {\displaystyle \prod }_{j=1}^{\mathcal{g}}}{\left({\displaystyle {\displaystyle \prod }_{i=1}^{\daleth }}{\left(\left|1+{\mathbb{V}}_{ij}^I\right|\right)}^{{\mathbb{S}}_{\mathsf{\Psi }}^i}\right)}^{{\mathbb{S}}_{\mathsf{\Omega }}^j}\right)\end{array}\right)$$

Step 3: Calculate the score data of each alternative:

$$SC\left({\mathbb{B}}_{BC}^{ij}\right)=\frac{1}{2}\left({\mathbb{U}}_{ij}^R+{\mathbb{V}}_{ij}^R+{\mathbb{U}}_{ij}^I+{\mathbb{V}}_{ij}^I\right)\in \left[-1,1\right]$$

Step 4: Calculate the ranking data of the alternatives according to their score information and evaluate the best optimal.

Furthermore, we simplify the above techniques with some numerical data that aim to improve the risk assessment model for cyber security under the consideration of the initiated techniques to enhance the effectiveness of the presented operators. The major influence of this application is to improve the risk assessment technique for cyber security to simplify, identify, or assess cyber threats and complications. For this reason, we select the application of cyber security in the presence of initiated operators. Here are strategies to improve and refine the problem of cyber security risk assessment techniques with the following five alternatives, ${\mathbb{B}}_{BC}^1$ to ${\mathbb{B}}_{BC}^5,$ of cyber security risks:

(1)

Threat intelligence integration “${\mathbb{B}}_{BC}^1$”.

(2)

Vulnerability assessment “${\mathbb{B}}_{BC}^2$”.

(3)

Threat modeling “${\mathbb{B}}_{BC}^3$”.

(4)

Collaboration and information sharing “${\mathbb{B}}_{BC}^4$”.

(5)

Third-party risk management “${\mathbb{B}}_{BC}^5$”.

These five points represent the collection of alternatives, and for each alternative, we have the following four attributes $, {\mathbb{B}}_{AT}^1$ to ${\mathbb{B}}_{AT}^4,$ with growth analysis, social impact, political impact, and environmental impact. We then apply four parameters, $e_1$ (internet), $e_2$ (computers), $e_3$ (computer lab), and $e_4$ (specialists). Furthermore, we use the weight vector ${\left(0.3, 0.3, 0.2, 0.2\right)}^T$ for each attribute and also use the weight vector ${\left(0.2, 0.3, 0.4, 0.1\right)}^T$ for parameters. Finally, we illustrate the procedure of the MADM technique for addressing our application problem. For the given data shown in

Table 3. BCFS decision matrix for the term one ${\mathbb{B}}_{BC}^1$.

${\mathbb{B}}_{\mathit{B}\mathit{C}}^1$	${\mathit{e}}_1$	${\mathit{e}}_2$	${\mathit{e}}_3$	${\mathit{e}}_4$
${\mathbb{B}}_{AT}^1$	$\left(\begin{array}{c}0.8+z\left(0.7\right),\\ -0.2+z\left(-0.3\right)\end{array}\right)$	$\left(\begin{array}{c}0.81+z\left(0.71\right),\\ -0.21+z\left(-0.31\right)\end{array}\right)$	$\left(\begin{array}{c}0.82+z\left(0.72\right),\\ -0.22+z\left(-0.32\right)\end{array}\right)$	$\left(\begin{array}{c}0.83+z\left(0.73\right),\\ -0.23+z\left(-0.33\right)\end{array}\right)$
${\mathbb{B}}_{AT}^2$	$\left(\begin{array}{c}0.7+z\left(0.3\right),\\ -0.3+z\left(-0.1\right)\end{array}\right)$	$\left(\begin{array}{c}0.71+z\left(0.31\right),\\ -0.31+z\left(-0.11\right)\end{array}\right)$	$\left(\begin{array}{c}0.72+z\left(0.32\right),\\ -0.32+z\left(-0.12\right)\end{array}\right)$	$\left(\begin{array}{c}0.73+z\left(0.33\right),\\ -0.33+z\left(-0.13\right)\end{array}\right)$
${\mathbb{B}}_{AT}^3$	$\left(\begin{array}{c}0.8+z\left(0.4\right),\\ -0.4+z\left(-0.5\right)\end{array}\right)$	$\left(\begin{array}{c}0.81+z\left(0.41\right),\\ -0.41+z\left(-0.51\right)\end{array}\right)$	$\left(\begin{array}{c}0.82+z\left(0.42\right),\\ -0.42+z\left(-0.52\right)\end{array}\right)$	$\left(\begin{array}{c}0.83+z\left(0.43\right),\\ -0.43+z\left(-0.53\right)\end{array}\right)$
${\mathbb{B}}_{AT}^4$	$\left(\begin{array}{c}0.9+z\left(0.3\right),\\ -0.7+z\left(-0.8\right)\end{array}\right)$	$\left(\begin{array}{c}0.91+z\left(0.31\right),\\ -0.71+z\left(-0.81\right)\end{array}\right)$	$\left(\begin{array}{c}0.92+z\left(0.32\right),\\ -0.72+z\left(-0.82\right)\end{array}\right)$	$\left(\begin{array}{c}0.93+z\left(0.33\right),\\ -0.73+z\left(-0.83\right)\end{array}\right)$

for the first alternative (i.e., ${\mathbb{B}}_{BC}^1$), we can see that the ${\mathbb{B}}_{BC}^1$ supports the assertion that (${\mathbb{B}}_{BC}^1$,$e_1$) is suitable at ${\mathbb{B}}_{AT}^1$ with 0.8 and disproves it with −0.2. However, the ${\mathbb{B}}_{BC}^1$ supports that the current status of (${\mathbb{B}}_{BC}^1$,$e_1$) is suitable at ${\mathbb{B}}_{AT}^1$ with 0.7 and disproves it with −0.3. Thus, the first ${\mathbb{B}}_{BC}^1$’s information regarding (${\mathbb{B}}_{BC}^1$,$e_1$)’s suitability at ${\mathbb{B}}_{AT}^1$ is represented as $\left(\begin{array}{c}0.8+z\left(0.7\right),\\ -0.2+z\left(-0.3\right)\end{array}\right).$ Similarly, the others have the same interpretations. Therefore, the major steps of the MADM technique are listed as follows.

Step 1: Calculate the matrix of BCFS values, as shown in Table 3,

Table 4. BCFS decision matrix for the term two ${\mathbb{B}}_{BC}^2$.

${\mathbb{B}}_{\mathit{B}\mathit{C}}^2$	${\mathit{e}}_1$	${\mathit{e}}_2$	${\mathit{e}}_3$	${\mathit{e}}_4$
${\mathbb{B}}_{AT}^1$	$\left(\begin{array}{c}0.2+z\left(0.3\right),\\ -0.4+z\left(-0.6\right)\end{array}\right)$	$\left(\begin{array}{c}0.21+z\left(0.31\right),\\ -0.41+z\left(-0.61\right)\end{array}\right)$	$\left(\begin{array}{c}0.22+z\left(0.32\right),\\ -0.42+z\left(-0.62\right)\end{array}\right)$	$\left(\begin{array}{c}0.23+z\left(0.33\right),\\ -0.43+z\left(-0.63\right)\end{array}\right)$
${\mathbb{B}}_{AT}^2$	$\left(\begin{array}{c}0.3+z\left(0.4\right),\\ -0.6+z\left(-0.7\right)\end{array}\right)$	$\left(\begin{array}{c}0.31+z\left(0.41\right),\\ -0.61+z\left(-0.71\right)\end{array}\right)$	$\left(\begin{array}{c}0.32+z\left(0.42\right),\\ -0.62+z\left(-0.72\right)\end{array}\right)$	$\left(\begin{array}{c}0.33+z\left(0.43\right),\\ -0.63+z\left(-0.73\right)\end{array}\right)$
${\mathbb{B}}_{AT}^3$	$\left(\begin{array}{c}0.5+z\left(0.6\right),\\ -0.7+z\left(-0.8\right)\end{array}\right)$	$\left(\begin{array}{c}0.51+z\left(0.61\right),\\ -0.71+z\left(-0.81\right)\end{array}\right)$	$\left(\begin{array}{c}0.52+z\left(0.62\right),\\ -0.72+z\left(-0.82\right)\end{array}\right)$	$\left(\begin{array}{c}0.53+z\left(0.63\right),\\ -0.73+z\left(-0.83\right)\end{array}\right)$
${\mathbb{B}}_{AT}^4$	$\left(\begin{array}{c}0.1+z\left(0.2\right),\\ -0.8+z\left(-0.6\right)\end{array}\right)$	$\left(\begin{array}{c}0.11+z\left(0.21\right),\\ -0.81+z\left(-0.61\right)\end{array}\right)$	$\left(\begin{array}{c}0.12+z\left(0.22\right),\\ -0.82+z\left(-0.62\right)\end{array}\right)$	$\left(\begin{array}{c}0.13+z\left(0.23\right),\\ -0.83+z\left(-0.63\right)\end{array}\right)$

,

Table 5. BCFS decision matrix for the term three ${\mathbb{B}}_{BC}^3$.

${\mathbb{B}}_{\mathit{B}\mathit{C}}^3$	${\mathit{e}}_1$	${\mathit{e}}_2$	${\mathit{e}}_3$	${\mathit{e}}_4$
${\mathbb{B}}_{AT}^1$	$\left(\begin{array}{c}0.1+z\left(0.5\right),\\ -0.3+z\left(-0.7\right)\end{array}\right)$	$\left(\begin{array}{c}0.11+z\left(0.51\right),\\ -0.31+z\left(-0.71\right)\end{array}\right)$	$\left(\begin{array}{c}0.12+z\left(0.52\right),\\ -0.32+z\left(-0.72\right)\end{array}\right)$	$\left(\begin{array}{c}0.13+z\left(0.53\right),\\ -0.33+z\left(-0.73\right)\end{array}\right)$
${\mathbb{B}}_{AT}^2$	$\left(\begin{array}{c}0.2+z\left(0.6\right),\\ -0.4+z\left(-0.8\right)\end{array}\right)$	$\left(\begin{array}{c}0.21+z\left(0.61\right),\\ -0.41+z\left(-0.81\right)\end{array}\right)$	$\left(\begin{array}{c}0.22+z\left(0.62\right),\\ -0.42+z\left(-0.82\right)\end{array}\right)$	$\left(\begin{array}{c}0.23+z\left(0.63\right),\\ -0.43+z\left(-0.83\right)\end{array}\right)$
${\mathbb{B}}_{AT}^3$	$\left(\begin{array}{c}0.3+z\left(0.7\right),\\ -0.5+z\left(-0.9\right)\end{array}\right)$	$\left(\begin{array}{c}0.31+z\left(0.71\right),\\ -0.51+z\left(-0.91\right)\end{array}\right)$	$\left(\begin{array}{c}0.32+z\left(0.72\right),\\ -0.52+z\left(-0.92\right)\end{array}\right)$	$\left(\begin{array}{c}0.33+z\left(0.73\right),\\ -0.53+z\left(-0.93\right)\end{array}\right)$
${\mathbb{B}}_{AT}^4$	$\left(\begin{array}{c}0.4+z\left(0.8\right),\\ -0.6+z\left(-0.9\right)\end{array}\right)$	$\left(\begin{array}{c}0.41+z\left(0.81\right),\\ -0.61+z\left(-0.91\right)\end{array}\right)$	$\left(\begin{array}{c}0.42+z\left(0.82\right),\\ -0.62+z\left(-0.92\right)\end{array}\right)$	$\left(\begin{array}{c}0.43+z\left(0.83\right),\\ -0.63+z\left(-0.93\right)\end{array}\right)$

,

Table 6. BCFS decision matrix for the term four ${\mathbb{B}}_{BC}^4$.

${\mathbb{B}}_{\mathit{B}\mathit{C}}^4$	${\mathit{e}}_1$	${\mathit{e}}_2$	${\mathit{e}}_3$	${\mathit{e}}_4$
${\mathbb{B}}_{AT}^1$	$\left(\begin{array}{c}0.5+z\left(0.1\right),\\ -0.3+z\left(-0.5\right)\end{array}\right)$	$\left(\begin{array}{c}0.51+z\left(0.11\right),\\ -0.31+z\left(-0.51\right)\end{array}\right)$	$\left(\begin{array}{c}0.52+z\left(0.12\right),\\ -0.32+z\left(-0.52\right)\end{array}\right)$	$\left(\begin{array}{c}0.53+z\left(0.13\right),\\ -0.33+z\left(-0.53\right)\end{array}\right)$
${\mathbb{B}}_{AT}^2$	$\left(\begin{array}{c}0.4+z\left(0.2\right),\\ -0.4+z\left(-0.2\right)\end{array}\right)$	$\left(\begin{array}{c}0.41+z\left(0.21\right),\\ -0.41+z\left(-0.21\right)\end{array}\right)$	$\left(\begin{array}{c}0.42+z\left(0.22\right),\\ -0.42+z\left(-0.22\right)\end{array}\right)$	$\left(\begin{array}{c}0.43+z\left(0.23\right),\\ -0.43+z\left(-0.23\right)\end{array}\right)$
${\mathbb{B}}_{AT}^3$	$\left(\begin{array}{c}0.3+z\left(0.3\right),\\ -0.5+z\left(-0.3\right)\end{array}\right)$	$\left(\begin{array}{c}0.31+z\left(0.31\right),\\ -0.51+z\left(-0.31\right)\end{array}\right)$	$\left(\begin{array}{c}0.32+z\left(0.32\right),\\ -0.52+z\left(-0.32\right)\end{array}\right)$	$\left(\begin{array}{c}0.33+z\left(0.33\right),\\ -0.53+z\left(-0.33\right)\end{array}\right)$
${\mathbb{B}}_{AT}^4$	$\left(\begin{array}{c}0.2+z\left(0.6\right),\\ -0.8+z\left(-0.4\right)\end{array}\right)$	$\left(\begin{array}{c}0.21+z\left(0.61\right),\\ -0.81+z\left(-0.41\right)\end{array}\right)$	$\left(\begin{array}{c}0.22+z\left(0.62\right),\\ -0.82+z\left(-0.42\right)\end{array}\right)$	$\left(\begin{array}{c}0.23+z\left(0.63\right),\\ -0.83+z\left(-0.43\right)\end{array}\right)$

and

Table 7. BCFS decision matrix for the term five ${\mathbb{B}}_{BC}^5$.

${\mathbb{B}}_{\mathit{B}\mathit{C}}^5$	${\mathit{e}}_1$	${\mathit{e}}_2$	${\mathit{e}}_3$	${\mathit{e}}_4$
${\mathbb{B}}_{AT}^1$	$\left(\begin{array}{c}0.5+z\left(0.2\right),\\ -0.7+z\left(-0.5\right)\end{array}\right)$	$\left(\begin{array}{c}0.51+z\left(0.21\right),\\ -0.71+z\left(-0.51\right)\end{array}\right)$	$\left(\begin{array}{c}0.52+z\left(0.22\right),\\ -0.72+z\left(-0.52\right)\end{array}\right)$	$\left(\begin{array}{c}0.53+z\left(0.23\right),\\ -0.73+z\left(-0.53\right)\end{array}\right)$
${\mathbb{B}}_{AT}^2$	$\left(\begin{array}{c}0.2+z\left(0.3\right),\\ -0.7+z\left(-0.1\right)\end{array}\right)$	$\left(\begin{array}{c}0.21+z\left(0.31\right),\\ -0.71+z\left(-0.11\right)\end{array}\right)$	$\left(\begin{array}{c}0.22+z\left(0.32\right),\\ -0.72+z\left(-0.12\right)\end{array}\right)$	$\left(\begin{array}{c}0.23+z\left(0.33\right),\\ -0.73+z\left(-0.13\right)\end{array}\right)$
${\mathbb{B}}_{AT}^3$	$\left(\begin{array}{c}0.3+z\left(0.4\right),\\ -0.9+z\left(-0.5\right)\end{array}\right)$	$\left(\begin{array}{c}0.31+z\left(0.41\right),\\ -0.91+z\left(-0.51\right)\end{array}\right)$	$\left(\begin{array}{c}0.32+z\left(0.42\right),\\ -0.92+z\left(-0.52\right)\end{array}\right)$	$\left(\begin{array}{c}0.33+z\left(0.43\right),\\ -0.93+z\left(-0.53\right)\end{array}\right)$
${\mathbb{B}}_{AT}^4$	$\left(\begin{array}{c}0.3+z\left(0.5\right),\\ -0.7+z\left(-0.8\right)\end{array}\right)$	$\left(\begin{array}{c}0.31+z\left(0.51\right),\\ -0.71+z\left(-0.81\right)\end{array}\right)$	$\left(\begin{array}{c}0.32+z\left(0.52\right),\\ -0.72+z\left(-0.82\right)\end{array}\right)$	$\left(\begin{array}{c}0.33+z\left(0.53\right),\\ -0.73+z\left(-0.83\right)\end{array}\right)$

, and if the matrix covers cost types of information, then we normalize the matrix:

$$N=\left\{\begin{array}{cc}\left({\mathbb{U}}_{ij}^R+z{\mathbb{U}}_{ij}^I,{\mathbb{V}}_{ij}^R+z{\mathbb{V}}_{ij}^I\right)& for benefit\\ \left(1-{\mathbb{U}}_{ij}^R+z\left(1-{\mathbb{U}}_{ij}^I\right),-1-{\mathbb{V}}_{ij}^R+z\left(-1-{\mathbb{V}}_{ij}^I\right)\right)& for cost\end{array}\right.$$

Note that the information in Table 3, Table 4, Table 5, Table 6 and Table 7 is not required to be normalized.

Step 2: Calculate the aggregated values of the normalized matrix using the BCFSWA and BCFSWG operators, as shown in

Table 8. BCFS aggregated information matrix.

	BCFSWA Operator	BCFSWG Operator
${\mathbb{B}}_{BC}^1$	$\left(\begin{array}{c}0.8034+z\left(0.4583\right),\\ -0.3513+z\left(-0.3085\right)\end{array}\right)$	$\left(\begin{array}{c}0.7906+z\left(0.4121\right),\\ -0.4411+z\left(-0.4608\right)\end{array}\right)$
${\mathbb{B}}_{BC}^2$	$\left(\begin{array}{c}0.3690+z\left(0.4721\right),\\ -0.6188+z\left(-0.7180\right)\end{array}\right)$	$\left(\begin{array}{c}0.3186+z\left(0.4280\right),\\ -0.6615+z\left(-0.7292\right)\end{array}\right)$
${\mathbb{B}}_{BC}^3$	$\left(\begin{array}{c}0.2587+z\left(0.6659\right),\\ -0.4431+z\left(-0.8391\right)\end{array}\right)$	$\left(\begin{array}{c}0.2334+z\left(0.6463\right),\\ -0.4876+z\left(-0.8545\right)\end{array}\right)$
${\mathbb{B}}_{BC}^4$	$\left(\begin{array}{c}0.3799+z\left(0.289\right),\\ -0.4558+z\left(-0.3162\right)\end{array}\right)$	$\left(\begin{array}{c}0.3611+z\left(0.2428\right),\\ -0.5236+z\left(-0.3425\right)\end{array}\right)$
${\mathbb{B}}_{BC}^5$	$\left(\begin{array}{c}0.3321+z\left(0.3596\right),\\ -0.7870+z\left(-0.3406\right)\end{array}\right)$	$\left(\begin{array}{c}0.3076+z\left(0.3399\right),\\ -0.8133+z\left(-0.4704\right)\end{array}\right)$

.

Step 3: Calculate the score data of each alternative, as shown in

Table 9. BCFS score information.

	BCFSWA Operator	BCFSWG Operator
${\mathbb{B}}_{BC}^1$	$0.3009$	$0.1504$
${\mathbb{B}}_{BC}^2$	$-0.2478$	$-0.3220$
${\mathbb{B}}_{BC}^3$	$-0.1788$	$-0.2311$
${\mathbb{B}}_{BC}^4$	$-0.0515$	$-0.1310$
${\mathbb{B}}_{BC}^5$	$-0.2179$	$-0.3181$

.

Step 4: Calculate the ranking data of the alternatives according to their score information and evaluate the best optimal, as shown in

Table 10. Representation of the ranking values.

Methods	Ranking Values	Best Optimal
BCFSWA Operator	${\mathbb{B}}_{BC}^1>{\mathbb{B}}_{BC}^4>{\mathbb{B}}_{BC}^3>{\mathbb{B}}_{BC}^5>{\mathbb{B}}_{BC}^2$	${\mathbb{B}}_{BC}^1$
BCFSWG Operator	${\mathbb{B}}_{BC}^1>{\mathbb{B}}_{BC}^4>{\mathbb{B}}_{BC}^3>{\mathbb{B}}_{BC}^5>{\mathbb{B}}_{BC}^2$	${\mathbb{B}}_{BC}^1$

.

From the ranking in Table 10, we can see that the best one is ${\mathbb{B}}_{BC}^1$ based on our proposed BCFSWA and BCFSWG operators. This means that the term $″{\mathbb{B}}_{BC}^1:$ threat intelligence integration” has the most impact on cyber security risks. In fact, according to our intuitive knowledge about the risk assessment for cyber security, “${\mathbb{B}}_{BC}^1:$ threat intelligence integration” should be the most important among factors ${\mathbb{B}}_{BC}^1~{\mathbb{B}}_{BC}^5$. The final result based on the BCFSWA and BCFSWG operators is actually reasonable. Furthermore, we make comparisons between the proposed method and some existing techniques by using the above techniques and information from the above examples. For this, we discuss the comparative analysis in the next section.

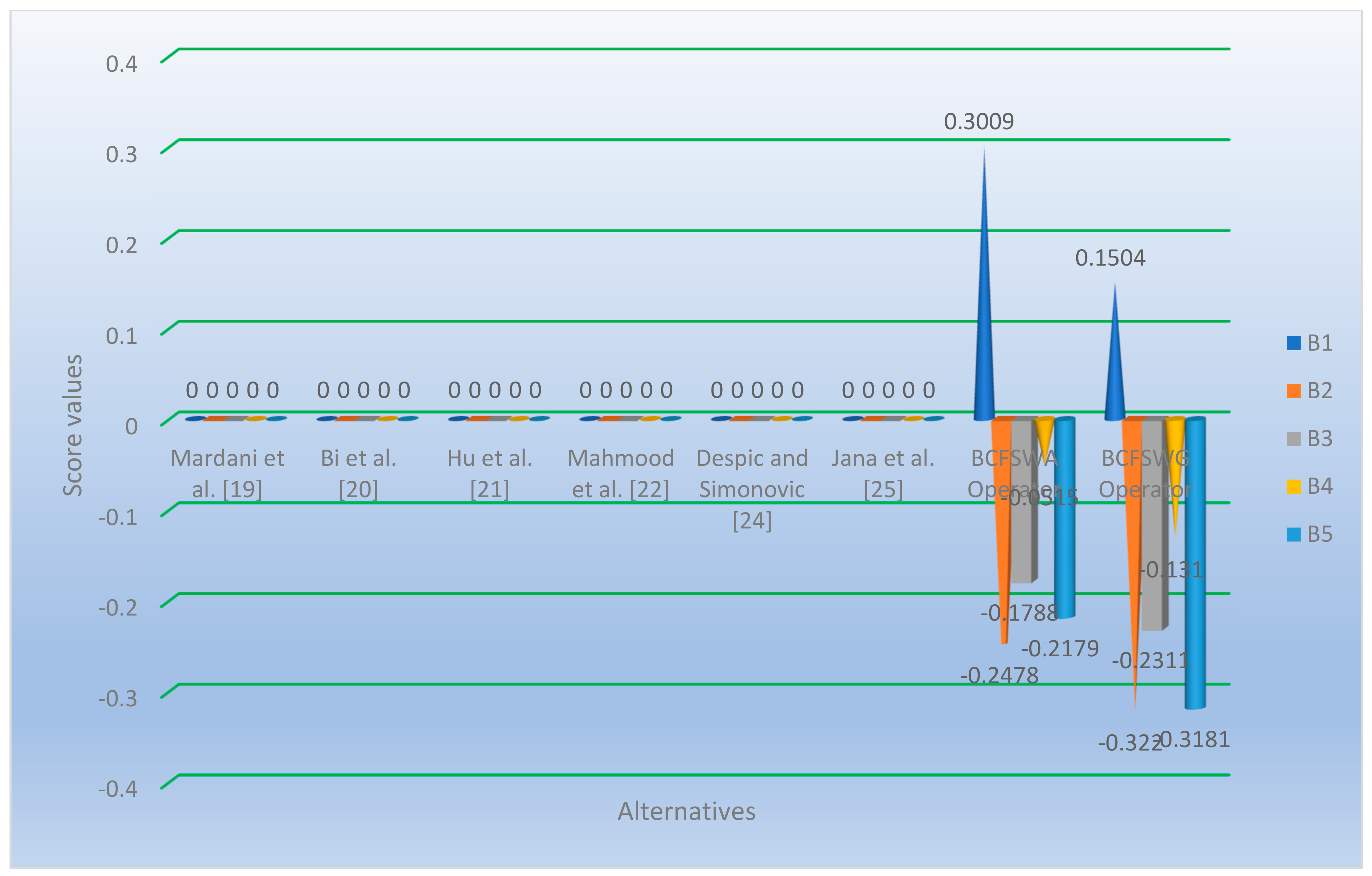
5. Comparative Analysis

In this section, we aim to evaluate and discuss the comparisons between the proposed operators with some existing operators for addressing the supremacy and dominancy of the derived techniques. For this, our major influence is to collect some existing operators based on existing information and try to evaluate our selected information with the help of these operators. We use the following existing methods: Mardani et al. [19] considered aggregation operators (AOs) for FSs; Bi et al. [20] presented the geometric operators for CFSs; Hu et al. [21] introduced the power operators for CFSs; Mahmood et al. [22] derived the AOs for BCFSs; Despic and Simonovic [24] initiated the AOs for SSs; and Jana et al. [25] introduced the robust AOs for BFSSs. Based on the information and results in Table 1, Table 2, Table 3, Table 4 and Table 5 in Section 4, the comparative analysis is listed in

Table 11. Representation of the comparative analysis.

Methods	Score Values	Ranking Values
Mardani et al. [19]	Failed	Failed
Bi et al. [20]	Failed	Failed
Hu et al. [21]	Failed	Failed
Mahmood et al. [22]	Failed	Failed
Despic and Simonovic [24]	Failed	Failed
Jana et al. [25]	Failed	Failed
BCFSWA Operator	0.3009, −0.2478, −0.1788, −0.0515, −0.2179	${\mathbb{B}}_{BC}^1>{\mathbb{B}}_{BC}^4>{\mathbb{B}}_{BC}^3>{\mathbb{B}}_{BC}^5>{\mathbb{B}}_{BC}^2$
BCFSWG Operator	0.1504, −0.322, −0.2311, −0.131, −0.3181	${\mathbb{B}}_{BC}^1>{\mathbb{B}}_{BC}^4>{\mathbb{B}}_{BC}^3>{\mathbb{B}}_{BC}^5>{\mathbb{B}}_{BC}^2$

.

From the rankings in Table 11, we observe that the best optimal is ${\mathbb{B}}_{BC}^1$ accordi to the BCFSWA and BCFSWG operators, which represents the threat intelligence integration. We also use Figure 1 to describe the geometrical interpretation of alternatives according to their score values, as shown in Table 11. We discuss the weakness of these existing techniques as follows.

(1)

Mardani et al. [19] proposed AOs for FSs, where the FS theory contains the truth grade from the unit interval. However, the proposed operators based on BCFSS information, which is an extended version of FS, demonstrate that the proposed theory of Mardani et al. [19] is a special case for the proposed operators.

(2)

Bi et al. [20] and Hu et al. [21] presented the GOs and power operators for CFSs, where the CFS theory contains the truth grade in the shape of a complex number whose real and imaginary parts are from the unit interval. In fact, the proposed operators based on BCFSS information not only contain the truth grade but also have the falsity grade, and so the proposed theory of Bi et al. [20] and Hu et al. [21] cannot handle these cases under the BCFSS environment.

(3)

Mahmood et al. [22] derived AOs for BCFSs (bipolar complex fuzzy sets) where the soft sets for parameter setting are not considered. Therefore, the proposed operators in Mahmood et al. [22] cannot handle these cases under the BCFSS environment.

(4)

Despic and Simonovic [24] initiated AOs for SSs that only contain the truth grade and no falsity grade. It means that the proposed AOs of Despic and Simonovic [24] are a special case of the proposed operators for BCFSSs.

(5)

Jana et al. [25] gave robust AOs for BFSSs (bipolar fuzzy soft sets) that can only be used in the real plane but not in a complex system, so they cannot handle these cases under the BCFSS environment.

Anyway, the existing techniques are not able to evaluate the information in Table 1, Table 2, Table 3, Table 4 and Table 5 because of many limitations and problems; the existing techniques are special cases of the proposed information. Therefore, the proposed operators based on BCFS information are reliable and dominant compared to existing techniques.

6. Conclusions

Bipolar complex fuzzy soft (BCFS) sets (BCFSSs) represent vague and unreliable information well in real-life problems. These FSs, BFSs, SSs, CFSs, BCFSs, FSSs, CFSSs, and BFSSs are the special parts of BCFSSs. After BCFSSs were considered by Mahmood et al. [9], there were still few works on aggregation operators (AOs) for BCFSSs in the literature. In this paper, we continue working on BCFSSs by giving operational laws for BCFSSs and then propose some AOs for BCFSSs. These are the BCFS weighted averaging (BCFSWA), BCFS ordered weighted averaging (BCFSOWA), BCFS weighted geometric (BCFSWG), and BCFS ordered weighted geometric (BCFSOWG) operators. We advance the theorems and properties of these proposed operators on BCFSSs. According to these proposed operators, we construct a MADM technique for evaluating some decision-making problems. We then apply it to improve the risk assessment model for cyber security based on the derived operators. We also make some comparisons of the proposed method with some existing methods in the literature to enhance the effectiveness, supremacy, and validity of these operators under BCFS information. In the future, we aim to improve the ideas of fuzzy N soft sets [27] and cubic bipolar fuzzy VIKOR [28] with the BCFSS extensions, evaluate more AOs based on these extended ideas, and then discuss more applications in AHP and TOPSIS decision making and optimization theory [29,30].