A Hybrid MCDM Approach in Third-Party Logistics (3PL) Provider Selection
Abstract
:1. Introduction
2. Literature Review
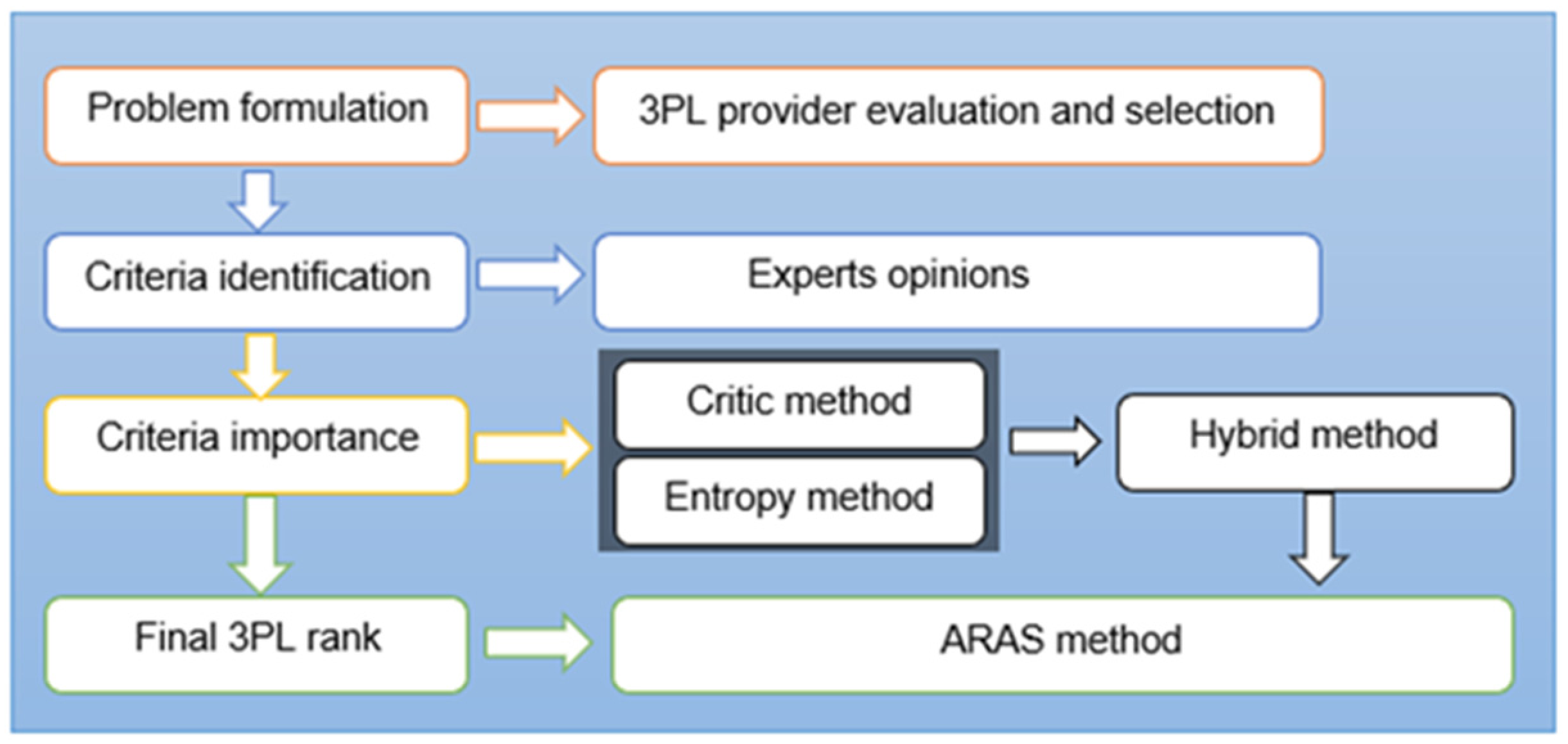
3. Methodology
3.1. CRITIC (Criteria Importance through Intercriteria Correlation) Method
3.2. Entropy Method
3.3. Hybrid Criteria Weights
3.4. The Additive Ratio Assessment (ARAS) Method
4. Application of the Hybrid–ARAS Method to 3PL Evaluation and Selection
4.1. Application of the Hybrid–ARAS Method to 3PL Evaluation and Selection Problem
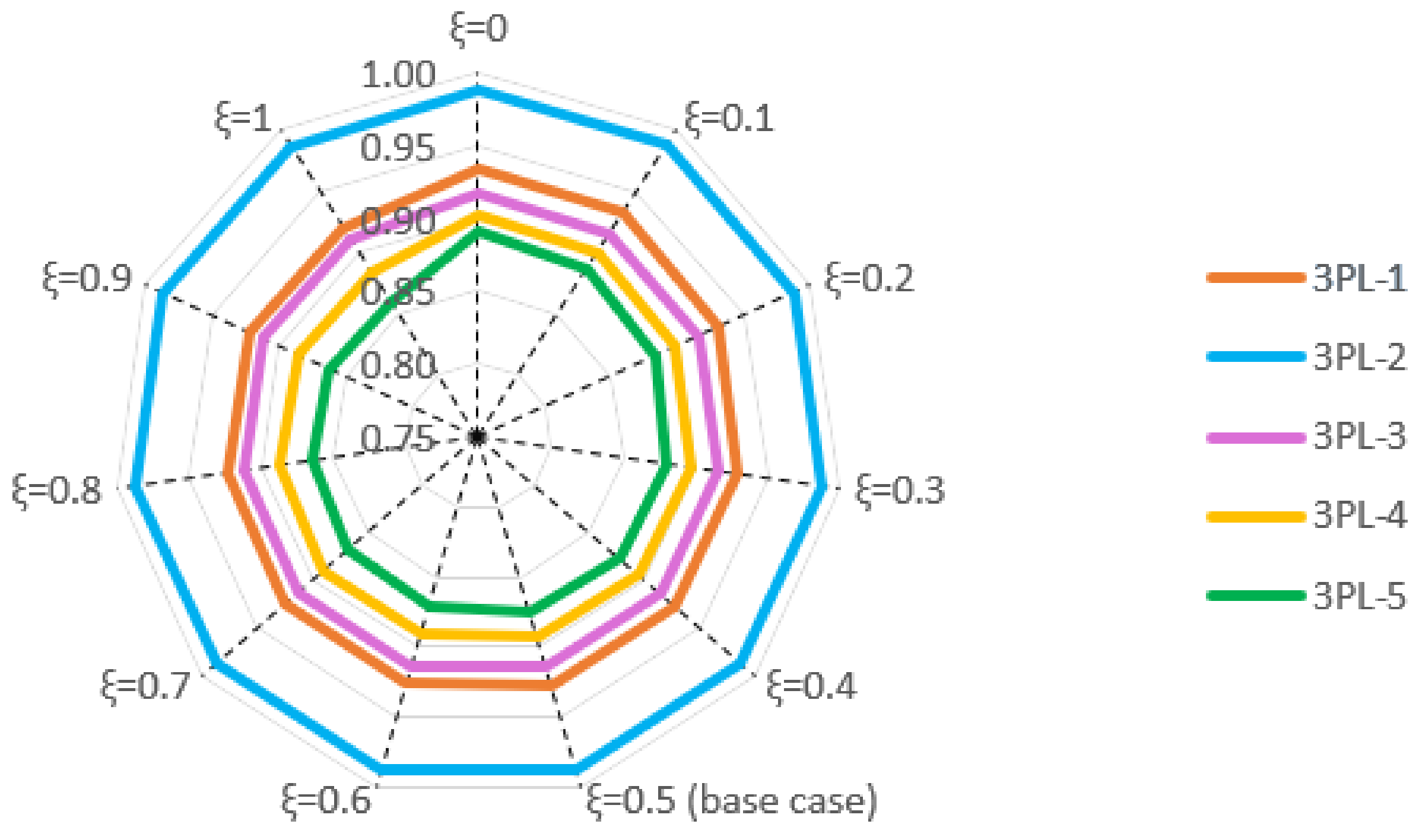
4.2. Sensitivity Analysis
4.3. Comparative Analysis
5. Managerial Insights
6. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Simić, V.; Lazarević, D.; Dobrodolac, M. Picture fuzzy WASPAS method for selecting last-mile delivery mode: A case study of Belgrade. Eur. Transp. Res. Rev. 2021, 13, 43. [Google Scholar] [CrossRef]
- Lazarević, D.; Dobrodolac, M.; Švadlenka, L.; Stanivuković, B. A model for business performance improvement: A case of the postal company. J. Bus. Econ. Manag. 2020, 21, 564–592. [Google Scholar] [CrossRef] [Green Version]
- Wang, C.-N.; Nguyen, N.-A.-T.; Dang, T.-T.; Lu, C.-M. A Compromised Decision-Making Approach to Third-Party Logistics Selection in Sustainable Supply Chain Using Fuzzy AHP and Fuzzy VIKOR Methods. Mathematics 2021, 9, 886. [Google Scholar] [CrossRef]
- Wang, C.-N.; Dang, T.-T.; Nguyen, N.-A.-T.; Le, T.-T.-H. Supporting Better Decision-Making: A Combined Grey Model and Data Envelopment Analysis for Efficiency Evaluation in E-Commerce Marketplaces. Sustainability 2020, 12, 10385. [Google Scholar] [CrossRef]
- Wang, G.; Dou, W.; Zhu, W.; Zhou, N. The effects of firm capabilities on external collaboration and performance: The moderating role of market turbulence. J. Bus. Res. 2015, 68, 1928–1936. [Google Scholar] [CrossRef]
- Soh, S. A decision model for evaluating third-party logistics providers using fuzzy analytic hierarchy process. Afr. J. Bus. Manag. 2009, 4, 339–349. [Google Scholar]
- Hsu, C.H.; Wang, F.K.; Tzeng, G.H. The best vendor selection for conducting the recycled material based on a hybrid MCDM model combining DANP with VIKOR. Resour. Conserv. Recycl. 2012, 66, 95–111. [Google Scholar] [CrossRef]
- Jovčić, S.; Průša, P.; Dobrodolac, M.; Švadlenka, L. A Proposal for a Decision-Making Tool in Third-Party Logistics (3PL) Provider Selection Based on Multi-Criteria Analysis and the Fuzzy Approach. Sustainability 2019, 11, 4236. [Google Scholar] [CrossRef] [Green Version]
- Saaty, T.L. The Analytic Hierarchy Process; McGraw-Hill: New York, NY, USA, 1980. [Google Scholar]
- Korpela, J.; Tuominen, M. A decision aid in warehouse site selection. Int. J. Prod. Econ. 1996, 45, 169–180. [Google Scholar] [CrossRef]
- Yahya, S.; Kingsman, B. Vendor rating for an entrepreneur development program: A case study using the Analytic Hierarchy Process Method. J. Oper. Res. Soc. 1999, 50, 916–930. [Google Scholar] [CrossRef]
- Akarte, M.M.; Surendra, N.V.; Ravi, B.; Rangaraj, N. Web based casting supplier evaluation using analytical hierarchy process. J. Oper. Res. Soc. 2001, 52, 511–522. [Google Scholar] [CrossRef]
- Muralidharan, C.; Anantharaman, N.; Deshmukh, S.G. A multi-criteria group decision-making model for supplier rating. J. Supply Chain Manag. 2002, 38, 22–33. [Google Scholar] [CrossRef]
- Liu, F.H.F.; Hai, H.L. The voting analytic hierarchy process method for selecting supplier. Int. J. Prod. Econ. 2005, 97, 308–317. [Google Scholar] [CrossRef]
- So, S.H.; Kim, J.J.; Cheong, K.J.; Cho, G. Evaluating the service quality of third-party logistics service providers using the analytic hierarchy process. J. Inf. Syst. Technol. Manag. 2006, 3, 261–270. [Google Scholar]
- Göl, H.; Catay, B. Third-party logistics provider selection: Insights from a Turkish automotive company. Supply Chain Manag. 2007, 12, 379–384. [Google Scholar] [CrossRef]
- Chan, F.T.S.; Chan, H.K.; Ip, R.W.L.; Lau, H.C.W. A decision support system for supplier selection in the airline industry. Proc. Inst. Mech. Eng. Part B–J. Eng. Manuf. 2007, 221, 741–758. [Google Scholar] [CrossRef]
- Hou, J.; Su, D. EJB–MVC oriented supplier selection system for mass customization. J. Manuf. Technol. Manag. 2007, 18, 54–71. [Google Scholar] [CrossRef]
- Gómez, J.C.O.; Herrera, M.F.U.; Vinasco, M.A. Modelo para la evaluación del desempeño de los proveedores utilizando AHP. Ing. Y Desarro. 2008, 23, 43–58. [Google Scholar]
- Hudymáčová, M.; Benková, M.; Pócsová, J.; Škovránek, T. Supplier selection based on multi-criterial AHP method. Acta Montan. Slovaca 2010, 15, 249. [Google Scholar]
- Asamoah, D.; Annan, J.; Nyarko, S. AHP approach for supplier evaluation and selection in a pharmaceutical manufacturing firm in Ghana. Int. J. Bus. Manag. 2012, 7, 49. [Google Scholar] [CrossRef]
- Hruška, R.; Průša, P.; Babić, D. The use of AHP method for selection of supplier. Transport 2014, 29, 195–203. [Google Scholar] [CrossRef] [Green Version]
- Jayant, A.; Singh, P. Application of AHP-VIKOR Hybrid MCDM Approach for 3pl Selection: A Case Study. Int. J. Comput. Appl. 2015, 125, 4–11. [Google Scholar]
- Tuljak-Suban, D.; Bajec, P. Integration of AHP and GTMA to Make a Reliable Decision in Complex Decision-Making Problems: Application of the Logistics Provider Selection Problem as a Case Study. Symmetry 2020, 12, 766. [Google Scholar] [CrossRef]
- Aguezzoul, A.; Pache, G. An AHP-ELECTRE I Approach for 3PL-Provider Selection. Int. J. Transp. Econ. 2020, 47, 37–50. [Google Scholar]
- Meade, L.; Sarkis, J. A conceptual model for selecting and evaluating third-party reverse logistics providers. Supply Chain Manag. Int. J. 2002, 7, 283–295. [Google Scholar] [CrossRef]
- Sarkis, J.; Talluri, S. A model for strategic supplier selection. J. Supply Chain Manag. 2002, 38, 18–28. [Google Scholar] [CrossRef]
- Bayazit, O. Use of Analytic Network Process in vendor selection decisions. Benchmarking Int. J. 2006, 13, 566–579. [Google Scholar] [CrossRef]
- Jharkharia, S.; Shankar, R. Selection of logistics service provider: An analytic Network process (ANP) approach. Omega: Int. J. Manag. Sci. 2007, 35, 274–289. [Google Scholar] [CrossRef]
- Zareinejad, M.; Javanmard, H. Evaluation and selection of a third-party reverse logistics provider using ANP and IFG-MCDM methodology. Life Sci. J. 2013, 10, 350–355. [Google Scholar]
- Chen, Z.; Yang, W. An MAGDM based on constrained FAHP and FTOPSIS and its application to supplier selection. Math. Comput. Model. 2011, 54, 2802–2815. [Google Scholar] [CrossRef]
- Zeydan, M.; Çolpan, C.; Çobanoğlu, C. A combined methodology for supplier selection and performance evaluation. Expert Syst. Appl. 2011, 38, 2741–2751. [Google Scholar] [CrossRef]
- Singh, R.; Rajput, H.; Chaturvedi, V.; Vimal, J. Supplier selection by technique of order preference by similarity to ideal solution (TOPSIS) method for automotive industry. Int. J. Adv. Technol. 2012, 2, 157–160. [Google Scholar]
- Jayant, A.; Gupta, P.; Garg, S.K.; Khan, M. TOPSIS-AHP Based Approach for Selection of Reverse Logistics Service Provider: A Case Study of Mobile Phone Industry. Procedia Eng. 2014, 97, 2147–2156. [Google Scholar] [CrossRef] [Green Version]
- Laptate, V.R. Fuzzy Modified TOPSIS for Supplier Selection Problem in Supply Chain Management. Int. J. Innov. Res. Comput. Sci. Technol. 2015, 3, 22–28. [Google Scholar]
- Aguezzoul, A.; Pires, S. 3PL performance evaluation and selection: A MCDM method. Supply Chain Forum Int. J. 2016, 17, 87–94. [Google Scholar] [CrossRef]
- Govindan, K.; Grigore, M.C.; Kannan, D. Ranking of third-party logistics provider using Fuzzy-Electre II. In Proceedings of the 40th International Conference on Computers & Industrial Engineering, Awaji, Japan, 25–28 July 2010. [Google Scholar] [CrossRef]
- Cheng, C.H. Evaluating Naval Tactical Missile System by Fuzzy AHP Based on the Grade Value of Membership Function. Eur. J. Oper. Res. 1997, 96, 343–350. [Google Scholar] [CrossRef]
- Cheng, C.H.; Yang, L.L.; Hwang, C.L. Evaluating Attack Helicopter by AHP Based on Linguistic Variable Weight. Eur. J. Oper. Res. 1999, 116, 423–435. [Google Scholar] [CrossRef]
- Ayhan, M.B. A Fuzzy-AHP approach for supplier selection problem: A case study in a Gear-motor company. Int. J. Manag. Value Supply Chain. 2013, 4, 12–23. [Google Scholar] [CrossRef]
- Kilincci, O.; Onal, S.A. Fuzzy AHP approach for supplier selection in a washing machine company. Expert Syst. Appl. 2011, 38, 9656–9664. [Google Scholar] [CrossRef]
- Shaw, K.; Shankar, R.; Yadav, S.S.; Thakur, L.S. Supplier Selection Using Fuzzy AHP and Fuzzy Multi Objective Linear programming for developing low carbon supply chain. Expert Syst. Appl. 2012, 39, 8182–8192. [Google Scholar] [CrossRef]
- Zhang, H.; Xiu, L.; Wenhuang, L.; Bing, L.; Zhang, Z. An application of the AHP in 3PL vendor selection of a 4PL system. In Proceedings of the IEEE International Conference on Systems, Man and Cybernetics (IEEE Cat. No.04CH37583), The Hague, The Netherlands, 10–13 October 2004; pp. 1255–1260. [Google Scholar]
- Zhang, Y.; Feng, Y. A selection approach of reverse logistics provider based on fuzzy AHP. In Proceedings of the Fourth International Conference on Fuzzy Systems and Knowledge Discovery (FSKD 2007), Haikou, China, 24–27 August 2007; pp. 479–482. [Google Scholar]
- Cheng, J.-H.; Chen, S.-S.; Chuang, Y.-W. An application of fuzzy-Delphi and Fuzzy AHP formulation-criteria evaluation model of fourth party logistics. WSEAS Trans. Syst. 2008, 7, 466–478. [Google Scholar]
- Arikan, F. An interactive solution approach for multiple-objective supplier selection problem with fuzzy parameters. J. Intell. Manuf. 2015, 26, 989–998. [Google Scholar] [CrossRef]
- Jagannath, R.; Pamučar, D.; Kar, S. Evaluation and selection of third-party logistics provider under sustainability perspectives: An interval valued fuzzy-rough approach. Ann. Oper. Res. 2019, 293, 669–714. [Google Scholar] [CrossRef]
- Rezaeisaray, M.; Ebrahimnejad, S.; Khalili-Damghani, K. A novel hybrid MCDM approach for outsourcing supplier selection. J. Model. Manag. 2016, 11, 536–559. [Google Scholar] [CrossRef]
- Sremac, S.; Stević, Ž.; Pamučar, D.; Arsić, M.; Matić, B. Evaluation of a Third-Party Logistics (3PL) Provider Using a Rough SWARA–WASPAS Model Based on a New Rough Dombi Aggregator. Symmetry 2018, 10, 305. [Google Scholar] [CrossRef] [Green Version]
- Zarbakhshnia, N.; Wu, Y.; Govindan, K.; Soleimani, H. A novel hybrid multiple attribute decision-making approach for outsourcing sustainable reverse logistics. J. Clean. Prod. 2019, 242, 118461. [Google Scholar] [CrossRef]
- Özcan, E.; Ahiskali, M. 3PL Service Provider Selection with a Goal Programming Model Supported with Multi-criteria Decision-Making Approaches. Gazi Univ. J. Sci. 2020, 33, 413–427. [Google Scholar] [CrossRef]
- Lai, K.; Ngai, E.W.T.; Cheng, T.C.E. Measures for evaluating supply chain performance in transport logistics. Transp. Res. Part E Logist. Transp. Rev. 2002, 38, 439–456. [Google Scholar] [CrossRef] [Green Version]
- Sheen, G.L.; Tai, C.T. A study on decision factors and third party selection criterion of logistics outsourcing: An exploratory study of direct selling industry. J. Am. Acad. Bus. 2006, 9, 331–337. [Google Scholar]
- Yeung, C.L.A. The impact of third-party logistics performance on the logistics and export performance of users: An empirical study. Marit. Econ. Logist. 2006, 8, 121–139. [Google Scholar] [CrossRef]
- Lai, K.-h. Service capability and performance of logistic service providers. Transp. Res. Part E Logist. Transp. Rev. 2004, 40, 385–399. [Google Scholar] [CrossRef]
- Sinkovics, R.R.; Roath, S.A. Strategic orientation, capabilities, and performance in manufacturer-3PL relationships. J. Bus. Logist. 2004, 25, 43–64. [Google Scholar] [CrossRef]
- Knemeyer, M.A.; Murphy, R.P. Evaluating the performance of third-party logistics arrangements: A relationship marketing perspective. J. Supply Chain Manag. 2004, 40, 35–51. [Google Scholar] [CrossRef]
- Falsini, D.; Fondi, F.; Schiraldi, M.M. A logistics provider evaluation and selection methodology based on AHP, DEA and linear programming integration. Int. J. Prod. Res. 2012, 50, 4822–4829. [Google Scholar] [CrossRef] [Green Version]
- Zhou, G.; Min, H.; Xu, C.; Cao, Z. Evaluating the comparative efficiency of Chinese third-party logistics providers using data envelopment analysis. Int. J. Phys. Distrib. Logist. Manag. 2008, 38, 262–279. [Google Scholar] [CrossRef]
- Hamdan, A.; Rogers, K.J. Evaluating the efficiency of 3PL logistics operations. Int. J. Prod. Econ. 2008, 113, 235–244. [Google Scholar] [CrossRef]
- Kumar, M.; Vrat, P.; Shankar, R. A multi-objective 3PL allocation problem for fish distribution. Int. J. Phys. Distrib. Logist. Manag. 2006, 36, 702–715. [Google Scholar] [CrossRef]
- Tsai, C.-M.; Lee, H.-S.; Gan, G.-Y. A New Fuzzy DEA Model for Solving the MCDM Problems in Supplier Selection. J. Mar. Sci. Technol. 2021, 29, 7. [Google Scholar] [CrossRef]
- Liu, G.; Fan, S.; Tu, Y.; Wang, G. Innovative Supplier Selection from Collaboration Perspective with a Hybrid MCDM Model: A Case Study Based on NEVs Manufacturer. Symmetry 2021, 13, 143. [Google Scholar] [CrossRef]
- Hoseini, S.A.; Fallahpour, A.; Wong, K.Y.; Mahdiyar, A.; Saberi, M.; Durdyev, S. Sustainable Supplier Selection in Construction Industry through Hybrid Fuzzy-Based Approaches. Sustainability 2021, 13, 1413. [Google Scholar] [CrossRef]
- Kurniawan, V.R.B.; Puspitasari, F.H. A Fuzzy BWM Method for Evaluating Supplier Selection Factors in a SME Paper Manufacturer. Int. Conf. Adv. Sci. Technol. 2021. [CrossRef]
- Bošković, S.; Radonjić-Djogatović, V.; Ralević, P.; Dobrodolac, M.; Jovčić, S. Selection of Mobile Network Operator Using the Critic-Aras Method. Int. J. Traffic Transp. Eng. 2021, 11, 17–29. [Google Scholar] [CrossRef]
- Diakoulaki, D.; Mavrotas, G.; Papayannakis, L. Determining objective weights in multiple criteria problems: The critic method. Comput. Oper. Res. 1995, 22, 763–770. [Google Scholar] [CrossRef]
- Ghorabaee, K.M.; Amiri, M.; Zavadskas, E.K.; Antuchevičienė, J. Assessment of third-party logistics providers using a CRITIC–WASPAS approach with interval type-2 fuzzy sets. Transport 2017, 32, 66–78. [Google Scholar] [CrossRef] [Green Version]
- Zhang, H. Application on the Entropy Method for Determination of Weight of Evaluating Index in Fuzzy Mathematics for Wine Quality Assessment. Adv. J. Food Sci. Technol. 2015, 7, 195–198. [Google Scholar] [CrossRef]
- Ranđelović, M.; Nedeljković, S.; Jovanović, M.; Čabarkapa, M.; Stojanović, V.; Aleksić, A.; Ranđelović, D. Use of Determination of the Importance of Criteria in Business-Friendly Certification of Cities as Sustainable Local Economic Development Planning Tool. Symmetry 2020, 12, 425. [Google Scholar] [CrossRef] [Green Version]
- Zavadskas, E.K.; Turskis, Z. A new additive ratio assessment (ARAS) method in multi-criteria decision-making. Technol. Econ. Dev. Econ. 2010, 16, 159–172. [Google Scholar] [CrossRef]
- Alinezhad, A.; Khalili, J. WASPAS Method. In New Methods and Applications in Multiple Attribute Decision Making (MADM); International Series in Operations Research & Management Science; Springer: Cham, Switzerland, 2019; pp. 93–98. [Google Scholar] [CrossRef]
- Ghorabaee, M.K.; Zavadskas, E.K.; Olfat, L.; Turskis, Z. Multi-Criteria Inventory Classification Using a New Method of Evaluation Based on Distance from Average Solution (EDAS). Informatica 2015, 26, 435–451. [Google Scholar] [CrossRef]



| Author | Method |
|---|---|
| Korpela and Touminen [10]; Yahya and Kingsman [11]; Akarte et al. [12]; Liu and Hai [14]; So et al. [15]; Göl and Çatay [16]; Chan et al. [17]; Hou and Su [18]; Gomez et al. [19]; Hudymáčová [20]; Asamoah [21]; Hruška et al. [22] | AHP |
| Sarkis and Talluri [27]; Meade and Sarkis [26]; Bayazit [28]; Jkharkharia and Shankar [29]; Zareinejad and Javanmard [30] | ANP |
| Kumar et al. [61]; Zhou et al. [59]; Hamdan and Rogers [60] | DEA |
| Govindan et al. [37] | fuzzy-ELECTRE method |
| Chen and Yang [31] | fuzzy-AHP and fuzzy-TOPSIS (integrated approach) |
| Zeydan et al. [32] | fuzzy-AHP, fuzzy-TOPSIS, and DEA |
| Singh et al. [33] | TOPSIS |
| Falsini et al. [58] | AHP, DEA, and linear programming |
| Arikan [46] | fuzzy-AHP |
| Jayant et al. [34] | AHP–TOPSIS |
| Jayant and Singh [23] | AHP–VIKOR |
| Laptate [35] | fuzzy-modified TOPSIS |
| Rezaeisaray et al. [48] | DEMATEL, FANP, and DEA |
| Aguezzoul and Pires [36] | ELECTRE |
| Cheng [39]; Cheng et al. [42]; Zhang et al. [43]; Zhang and Feng [44]; Göl and Catay [16]; Cheng et al. [45]; Soh [6]; Kilincci and Onal [41]; Shaw et al. [42]; Ayhan [40]; Arikan [46] | fuzzy-AHP and fuzzy-objective linear programming (integrated approach) |
| Lai et al. [52]; Sinkovics and Roath [56]; Knemeyer and Murphy [57]; Sheen and Tai [53]; Yeung [54]; Lai [55] | Statistical methods |
| Sremac et al. [49] | rough SWARA, rough WASPAS, rough SAW, rough EDAS, rough MABAC, rough TOPSIS |
| Zarbakhshnia et al. [50] | SWARA, COPRAS |
| Jagannath et al. [47] | interval-valued fuzzy rough approach |
| Tuljak-Suban and Bajec [24] | AHP method with the Graph Theory and Matrix Approach (GTMA) |
| Aguezzoul and Pache [25] | AHP–ELECTRE I |
| Özcan and Ahıskalı [51] | MCDM–linear programming |
| Hoseini et al. [64] | fuzzy-best-Worst method and FIS |
| Whang et al. [3] | fuzzy-AHP and fuzzy-VIKOR |
| Kurniawan and Puspitasari [65] | fuzzy-best-worst method |
| Our study | Hybrid-ARAS method |
| Price (C1) | This criterion is expressed as the price that a company pays to the 3PL provider for its service provided. It is expressed in eurocent per km. Different 3PL providers request different prices for their services. |
| Delivery service (C2) | This criterion is expressed as the percentage of goods delivered in a promised timeframe. |
| QoS from customer experience (C3) | This criterion is expressed on a scale from 1 to 10, where 10 expresses maximal quality from the customer perspective. |
| Territorial coverage of the EU (C4) | This criterion is expressed as the percentage of EU territory covered by the 3PL provider. |
| Flexibility (C5) | This criterion represents the readiness of the 3PL provider to accommodate changing customer demands and expectations. It is expressed on a scale from 1 to 10, where 10 denotes the maximal degree of flexibility. |
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | |
|---|---|---|---|---|---|
| 3PL-1 | 0.95 | 99.98 | 9 | 88 | 9 |
| 3PL-2 | 0.92 | 99.95 | 10 | 92 | 10 |
| 3PL-3 | 0.99 | 99.90 | 10 | 75 | 9 |
| 3PL-4 | 0.90 | 98.98 | 8 | 85 | 8 |
| 3PL-5 | 1.20 | 99.97 | 8 | 95 | 10 |
| Sum | 4.96 | 498.78 | 45 | 435 | 46 |
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | |
|---|---|---|---|---|---|
| 3PL-1 | 0.1915 | 0.2004 | 0.2000 | 0.2023 | 0.1957 |
| 3PL-2 | 0.1855 | 0.2004 | 0.2222 | 0.2115 | 0.2174 |
| 3PL-3 | 0.1996 | 0.2003 | 0.2222 | 0.1724 | 0.1957 |
| 3PL-4 | 0.1815 | 0.1984 | 0.1778 | 0.1954 | 0.1739 |
| 3PL-5 | 0.2419 | 0.2004 | 0.1778 | 0.2184 | 0.2174 |
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | h = 1/ln(m) | |
|---|---|---|---|---|---|---|
| 3PL-1 | −0.3165 | −0.3222 | −0.3219 | −0.3233 | −0.3192 | −0.62133 |
| 3PL-2 | −0.3125 | −0.3221 | −0.3342 | −0.3286 | −0.3318 | |
| 3PL-3 | −0.3216 | −0.3221 | −0.3342 | −0.3031 | −0.3192 | |
| 3PL-4 | −0.3097 | −0.3209 | −0.3071 | −0.3190 | −0.3042 | |
| 3PL-5 | −0.3433 | −0.3221 | −0.3071 | −0.3323 | −0.3318 | |
| Sum | −1.6037 | −1.6094 | −1.6045 | −1.6062 | −1.6061 | |
| 0.9964 | 1.0000 | 0.9969 | 0.9980 | 0.9979 | Sum = 0.0107 | |
| 0.0036 | 0.0000 | 0.0031 | 0.0020 | 0.0021 | ||
| Weights | 0.3323 | 0.0004 | 0.2871 | 0.1861 | 0.1941 | 1 |
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | |
|---|---|---|---|---|---|
| 3PL-1 | 0.95 | 99.98 | 9 | 88 | 9 |
| 3PL-2 | 0.92 | 99.95 | 10 | 92 | 10 |
| 3PL-3 | 0.99 | 99.90 | 10 | 75 | 9 |
| 3PL-4 | 0.90 | 98.98 | 8 | 85 | 8 |
| 3PL-5 | 1.20 | 99.97 | 8 | 95 | 10 |
| Sum | 4.96 | 498.78 | 45 | 435 | 46 |
| min/max | min | max | max | max | max |
| Best | 0.90 | 99.98 | 10 | 95 | 10 |
| Worst | 1.20 | 98.98 | 8 | 75 | 8 |
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | |
|---|---|---|---|---|---|
| 3PL-1 | 0.8333 | 1.0000 | 0.5000 | 0.6500 | 0.5000 |
| 3PL-2 | 0.9333 | 0.9700 | 1.0000 | 0.8500 | 1.0000 |
| 3PL-3 | 0.7000 | 0.9200 | 1.0000 | 0.0000 | 0.5000 |
| 3PL-4 | 1.0000 | 0.0000 | 0.0000 | 0.5000 | 0.0000 |
| 3PL-5 | 0.0000 | 0.9900 | 0.0000 | 1.0000 | 1.0000 |
| Standard deviation ỽj | 0.4037 | 0.4349 | 0.5000 | 0.3857 | 0.4183 |
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | |
|---|---|---|---|---|---|
| Price (EUR/km) | 1.0000 | −0.4378 | 0.3922 | −0.3934 | −0.5625 |
| Delivery service (%) | −0.4378 | 1.0000 | 0.5174 | 0.2035 | 0.8135 |
| QoS from customer experience (Scale 1–10) | 0.3922 | 0.5174 | 1.0000 | −0.4213 | 0.2988 |
| Territorial coverage of the EU (%) | −0.3934 | 0.2035 | −0.4213 | 1.0000 | 0.5811 |
| Flexibility | −0.5625 | 0.8135 | 0.2988 | 0.5811 | 1.0000 |
| Price (EUR/km) | Delivery Service (%) | QoS (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | Sum by Rows | ỽj | Hj | Wj | |
|---|---|---|---|---|---|---|---|---|---|
| Price (EUR/km) | 0.0000 | 1.4378 | 0.6078 | 1.3934 | 1.5625 | 5.0015 | 0.4037 | 2.0192 | 0.2642 |
| Delivery service (%) | 1.4378 | 0.0000 | 0.4826 | 0.7965 | 0.1865 | 2.9035 | 0.4349 | 1.2627 | 0.1652 |
| QoS from customer experience (Scale 1–10) | 0.6078 | 0.4826 | 0.0000 | 1.4213 | 0.7012 | 3.2130 | 0.5000 | 1.6065 | 0.2102 |
| Territorial coverage of the EU (%) | 1.3934 | 0.7965 | 1.4213 | 0.0000 | 0.4189 | 4.0302 | 0.3857 | 1.5544 | 0.2034 |
| Flexibility (1–10) | 1.5625 | 0.1865 | 0.7012 | 0.4189 | 0.0000 | 2.8691 | 0.4183 | 1.2002 | 0.1570 |
| 7.6430 | 1 | ||||||||
| Criteria Weights | Entropy Weights | CRITIC Weights | Hybrid Weights |
|---|---|---|---|
| Price (EUR/km) | 0.3323 | 0.2642 | 0.2983 |
| Delivery service (%) | 0.0004 | 0.1652 | 0.0828 |
| QoS from customer Experience (1–10) | 0.2871 | 0.2102 | 0.2486 |
| Territorial coverage of the EU (%) | 0.1861 | 0.2034 | 0.1947 |
| Flexibility | 0.1941 | 0.157 | 0.1755 |
| 1 | |||
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | |
|---|---|---|---|---|---|
| 0—optimal value | 0.90 | 99.98 | 10 | 95 | 10 |
| 3PL-1 | 0.95 | 99.98 | 9 | 88 | 9 |
| 3PL-2 | 0.92 | 99.95 | 10 | 92 | 10 |
| 3PL-3 | 0.99 | 99.90 | 10 | 75 | 9 |
| 3PL-4 | 0.90 | 98.98 | 8 | 85 | 8 |
| 3PL-5 | 1.20 | 99.97 | 8 | 95 | 10 |
| min/max | min | max | max | max | max |
| sum | 6.2 | 598.8 | 55.0 | 530.0 | 56.0 |
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | Territorial Coverage of the EU (%) | Flexibility (Scale 1–10) | |
|---|---|---|---|---|---|
| 0 | 0.1791 | 0.1670 | 0.1818 | 0.1792 | 0.1786 |
| 3PL-1 | 0.1696 | 0.1670 | 0.1636 | 0.1660 | 0.1607 |
| 3PL-2 | 0.1752 | 0.1669 | 0.1818 | 0.1736 | 0.1786 |
| 3PL-3 | 0.1628 | 0.1668 | 0.1818 | 0.1415 | 0.1607 |
| 3PL-4 | 0.1791 | 0.1653 | 0.1455 | 0.1604 | 0.1429 |
| 3PL-5 | 0.1343 | 0.1670 | 0.1455 | 0.1792 | 0.1786 |
| min/max | min | max | max | max | max |
| Hybrid Weights | 0.2983 | 0.0828 | 0.2487 | 0.1948 | 0.1756 |
| Price (EUR/km) | Delivery Service (%) | QoS from Customer Experience (Scale 1–10) | EU Territorial Coverage (%) | Flexibility (Scale 1–10) | S | K | Rank | |
|---|---|---|---|---|---|---|---|---|
| 0 | 0.0534 | 0.0138 | 0.0452 | 0.0349 | 0.0314 | 0.1787 | Preference | |
| 3PL-1 | 0.0506 | 0.0138 | 0.0407 | 0.0323 | 0.0282 | 0.1657 | 0.9270 | 2 |
| 3PL-2 | 0.0523 | 0.0138 | 0.0452 | 0.0338 | 0.0314 | 0.1765 | 0.9873 | 1 |
| 3PL-3 | 0.0486 | 0.0138 | 0.0452 | 0.0276 | 0.0282 | 0.1634 | 0.9141 | 3 |
| 3PL-4 | 0.0534 | 0.0137 | 0.0362 | 0.0312 | 0.0251 | 0.1596 | 0.8930 | 4 |
| 3PL-5 | 0.0401 | 0.0138 | 0.0362 | 0.0349 | 0.0314 | 0.1563 | 0.8747 | 5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jovčić, S.; Průša, P. A Hybrid MCDM Approach in Third-Party Logistics (3PL) Provider Selection. Mathematics 2021, 9, 2729. https://doi.org/10.3390/math9212729
Jovčić S, Průša P. A Hybrid MCDM Approach in Third-Party Logistics (3PL) Provider Selection. Mathematics. 2021; 9(21):2729. https://doi.org/10.3390/math9212729
Chicago/Turabian StyleJovčić, Stefan, and Petr Průša. 2021. "A Hybrid MCDM Approach in Third-Party Logistics (3PL) Provider Selection" Mathematics 9, no. 21: 2729. https://doi.org/10.3390/math9212729
APA StyleJovčić, S., & Průša, P. (2021). A Hybrid MCDM Approach in Third-Party Logistics (3PL) Provider Selection. Mathematics, 9(21), 2729. https://doi.org/10.3390/math9212729







