Cross-Hedging Portfolios in Emerging Stock Markets: Evidence for the LATIBEX Index
Abstract
:1. Introduction
2. Materials and Methods
2.1. Methodology for Hedging with Futures
2.1.1. Models for First and Second Order Moments
2.1.2. The Dynamics of the Optimum Hedge Ratio
3. Data and Results
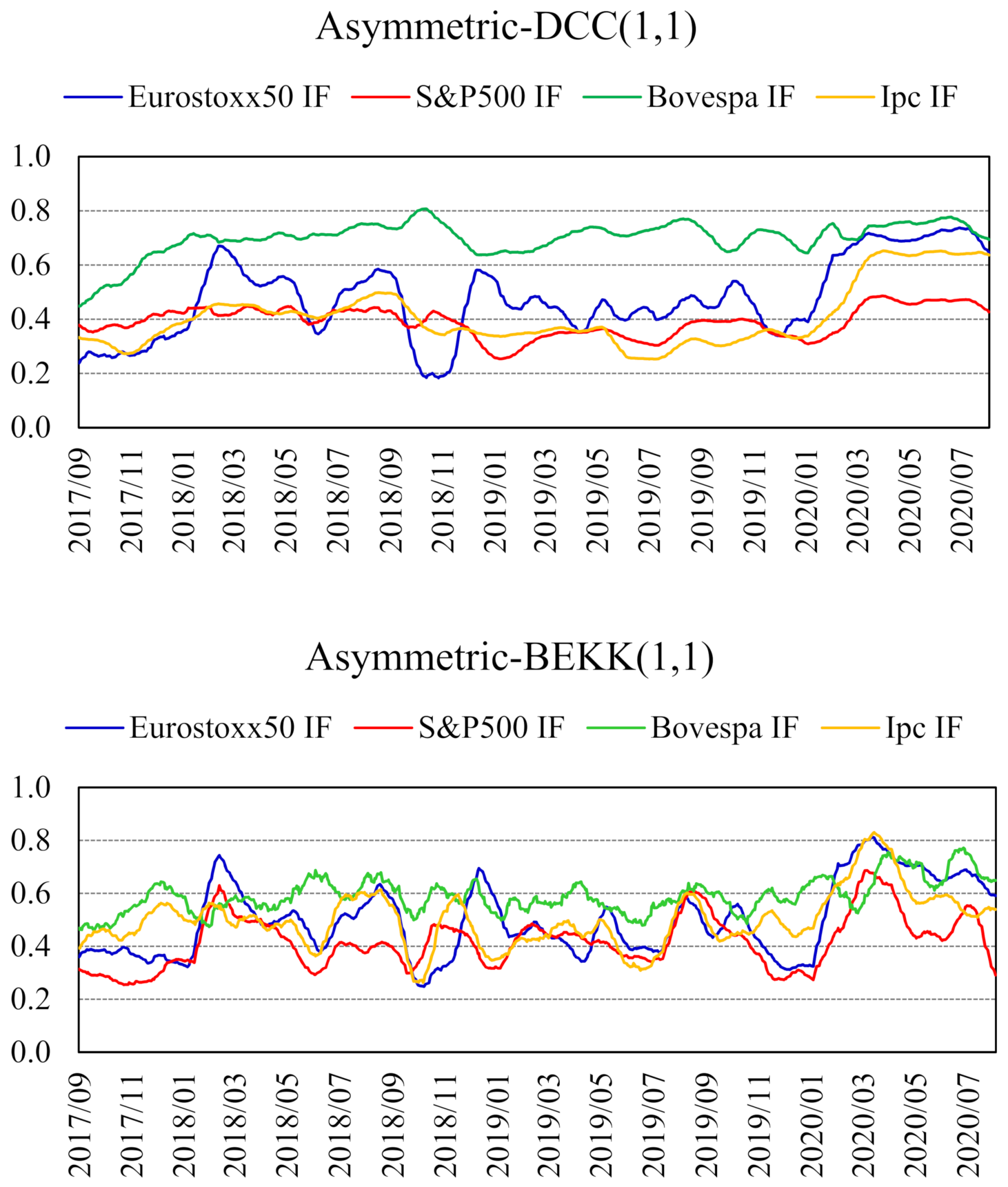
3.1. Minimum Variance Hedge Ratios
3.2. Hedging Efficiency
3.3. Further Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Multivariate Asymmetric GARCH Models for Variance–Covariance Matrices
Appendix A.1. CCC Model
Appendix A.2. DCC Model
Appendix A.3. Diagonal VEC Model (DVEC)
Appendix A.4. BEKK Model
References
- Anderson, R.; Danthine, J.P. Cross Hedging. J. Political Econ. 1981, 89, 1182–1196. [Google Scholar] [CrossRef]
- Eaker, M.; Grant, D. Cross-hedging foreign currency risk. J. Int. Money Financ. 1987, 6, 85–105. [Google Scholar] [CrossRef]
- Chen, X.; Tongurai, J. Cross-commodity hedging for illiquid futures: Evidence from China’a base metal futures market. Glob. Financ. J. 2021, 49, 100652. [Google Scholar] [CrossRef]
- Kar, S.; Khandelwal, P. Cross-hedging aviation fuel price exposures with commodity futures: Evidence from the Indian aviation industry. IIMB Manag. Rev. 2020, 32, 389–401. [Google Scholar] [CrossRef]
- Zainudin, A.D.; Mohamad, A. Cross hedging with stock index futures. Q. Rev. Econ. Financ. 2021, 82, 128–144. [Google Scholar] [CrossRef]
- Addae-Dapaah, K.; Abdullah, K. Cross Hedging Effectiveness of Real Estate Securities Exchange Traded Funds. J. Real Estate Portf. Manag. 2020, 26, 74–100. [Google Scholar] [CrossRef]
- Ederington, L. The hedging performance of the new futures markets. J. Financ. 1979, 34, 157–170. [Google Scholar] [CrossRef]
- Myers, R. Estimating time varying optimal hedge ratios on futures markets. J. Futures Mark. 1991, 11, 39–53. [Google Scholar] [CrossRef]
- Cecchetti, S.G.; Cumby, R.E.; Figlewski, S. Estimation of the optimal futures hedge. Rev. Econ. Stat. 1988, 70, 623–630. [Google Scholar] [CrossRef]
- Oliveira, F.; Maia, S.; De Jesus, D.; Besarria, C. Which information matters to market risk spreading in Brazil? Volatility transmission modelling using MGARCH-BEKK, DCC, t-Copulas. N. Am. J. Econ. Financ. 2018, 45, 83–100. [Google Scholar] [CrossRef]
- Bhhuiyan, R.A.; Rahman, M.P.; Saiti, B.; Ghani, G.B.M. Does the Malaysian Sovereign sukuk market offer portfolio diversification opportunities for global fixed-income investors? Evidence from wavelet coherence and multivariate-GARCH analyses. N. Am. J. Econ. Financ. 2019, 47, 675–687. [Google Scholar] [CrossRef]
- Baillie, R.T.; Myers, R.G. Bivariate GARCH estimation of the optimal commodity futures hedge. J. Appl. Econom. 1991, 6, 109–124. [Google Scholar] [CrossRef]
- Sukcharoen, K.; Choi, H.; Leatham, D.J. Optimal gasoline hedging strategies using futures contracts and exchange-traded funds. Appl. Econ. 2015, 47, 3482–3498. [Google Scholar] [CrossRef]
- Candila, V.; Farace, S. On the Volatility Spillover between Agricultural Commodities and Latin American Stock Markets. Risks 2018, 6, 116. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.; Lien, D. Optimal futures hedging for energy commodities: An application of the gas model. J. Futures Mark. 2020, 40, 1090–1108. [Google Scholar] [CrossRef]
- Park, T.; Switzer, L. Bivariate GARCH estimation of the optimal hedge ratios for stock index futures: A note. J. Futures Mark. 1995, 15, 61–67. [Google Scholar] [CrossRef]
- Park, T.; Switzer, L. Time-varying distributions and the optimal hedge ratios for stock index futures. Appl. Financ. Econ. 1995, 5, 131–137. [Google Scholar] [CrossRef]
- Lafuente, J.A.; Novales, A. Optimal hedging under departures from the cost-of-carry valuation: Evidence from the Spanish stock index futures market. J. Bank. Financ. 2003, 27, 1053–1078. [Google Scholar] [CrossRef] [Green Version]
- Chuang, C.-C.; Wang, Y.-H.; Yeh, T.-J.; Chuang, S.-L. Hedging effectiveness of the hedged portfolio: The expected utility maximization subject to the value-at risk approach. Appl. Econ. 2015, 47, 2040–2052. [Google Scholar] [CrossRef]
- Habiba, U.E.; Zhang, W. The dynamics of volatility spillovers between oil prices and stock market returns at the sector level and hedging strategies: Evidence from Pakistan. Environ. Sci. Pollut. Res. Int. 2020, 27, 30706–30715. [Google Scholar] [CrossRef]
- Jin, J.; Han, L.; Wu, L.; Zeng, H. The hedging effect of green bonds on carbon market risk. Int. Rev. Financ. Anal. 2020, 71, 101509. [Google Scholar] [CrossRef]
- Kroner, K.; Sultan, J. Exchange rate volatility and time varying hedge ratios. In Pacific-Basin Capital Markets Research; Rhee, S.G., Chang, R.P., Eds.; Elsevier Science Publishers: Amsterdam, The Netherlands, 1991; Volume II. [Google Scholar]
- Kroner, K.; Sultan, J. Time-varying distributions and dynamic hedging with foreign currency futures. J. Financ. Quant. Anal. 1993, 28, 535–551. [Google Scholar] [CrossRef]
- Tong, W. An examination of dynamic hedging. J. Int. Money Financ. 1996, 15, 19–35. [Google Scholar] [CrossRef]
- Brooks, C.; Chong, J. The cross-currency hedging performance of implied versus statistical forecasting models. J. Futures Mark. 2001, 21, 1043–1069. [Google Scholar] [CrossRef] [Green Version]
- Mensi, W.; Hammoudeh, S.; Rehman, M.U.; Al-Maadid, A.A.S.; Kang, S.H. Dynamic risk spillovers and portfolio risk management between precious metals and global foreign exchange markets. N. Am. J. Econ. Financ. 2020, 51, 101086. [Google Scholar] [CrossRef]
- Bollerslev, T. Modelling the coherence in short-run nominal exchange rates: A multivariate generalized ARCH model. Rev. Econ. Stat. 1990, 72, 498–505. [Google Scholar] [CrossRef]
- Engle, R.F. Dynamic conditional correlations: A simple class of multivariate GARCH. J. Bus. Econ. Stat. 2002, 20, 339–350. [Google Scholar] [CrossRef]
- Bollerslev, T.; Engle, R.F.; Wooldridge, J.M. A capital asset pricing model with time-varying covariances. J. Political Econ. 1988, 96, 116–131. [Google Scholar] [CrossRef]
- Engle, R.F.; Kroner, K.F. Multivariate Simultaneous Generalized ARCH. Econom. Theory 1995, 11, 122–150. [Google Scholar] [CrossRef]
- Engle, R.F.; Granger, C.W.J. Co-Integration and Error Correction: Representation, Estimation, and Testing. Econometrica 1987, 55, 251–276. [Google Scholar] [CrossRef]
- Lien, D. The effect of cointegration relationship on futures hedging: A note. J. Futures Mark. 1996, 16, 773–780. [Google Scholar] [CrossRef]
- Susmel, R.; Engle, R.F. Hourly volatility spillovers between international equity markets. J. Int. Money Financ. 1994, 13, 3–25. [Google Scholar] [CrossRef]
- Lai, Y.S.; Sheu, H.J. On the importance of asymmetries for dynamic hedging during the subprime crisis. Appl. Financ. Econ. 2011, 21, 801–813. [Google Scholar] [CrossRef]
- Sun, H.; Yu, B. Volatility asymmetry in functional threshold garch model. J. Time Ser. Anal. 2020, 41, 95–109. [Google Scholar] [CrossRef] [Green Version]
- Howard, C.T.; D’Antonio, L.J. Multiperiod hedging using futures: A risk minimization approach in the presence of autocorrelation. J. Futures Mark. 1991, 11, 697–710. [Google Scholar] [CrossRef]
- Lien, D.; Luo, X. Estimating multiperiod hedge ratios in cointegrated markets. J. Futures Mark. 1993, 13, 909–920. [Google Scholar] [CrossRef]
- Lien, D.; Luo, X. Multiperiod hedging in the presence of conditional heteroskedasticity. J. Futures Mark. 1994, 14, 927–955. [Google Scholar] [CrossRef]
- Levy, H.; Markowitz, H.M. Approximating expected utility by a function of mean and variance. Am. Econ. Rev. 1979, 3, 308–317. [Google Scholar]
- Anderson, R.; Danthine, J.P. Hedging and joint production: Theory and illustrations. J. Financ. 1980, 5, 487–501. [Google Scholar] [CrossRef]
- Chang, C.L.; González-Serrano, L.G.; Jiménez-Martín, J.A. Currency Hedging Strategies Using Dynamic Multivariate GARCH. Math. Comput. Simul. 2013, 94, 164–182. [Google Scholar] [CrossRef] [Green Version]
- Lai, Y.S. Evaluating the hedging performance of multivariate GARCH models. Asia Pac. Manag. Rev. 2019, 24, 86–95. [Google Scholar] [CrossRef]
- Johnson, L. The theory of hedging and speculation in commodity futures. Rev. Econ. Stud. 1960, 27, 139–151. [Google Scholar] [CrossRef]
- Stein, J.L. The simultaneous determination of spot and futures prices. Am. Econ. Rev. 1961, 5, 1012–1025. [Google Scholar]
- Alexander, C. Market Risk Analysis I: Quantitative Methods in Finance, 1st ed.; Wiley: Chichester, UK, 2008. [Google Scholar]
- Fabozzi, F.J.; Kolm, P.; Pachamanova, D.A.; Focardi, S.M. Robust Portfolio Optimization and Management, 1st ed.; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Glosten, L.; Jagannathan, R.; Runkle, D. On the relation between the expected value and the volatility of the nominal excess return on stocks. J. Financ. 1993, 48, 1779–1801. [Google Scholar] [CrossRef]


| Latibex Index | EuroStoxx 50 Index Future | S&P 500 Index Future | Bovespa Index Future | Ipc Index Future | |
|---|---|---|---|---|---|
| Observations | 4082 | 4082 | 4082 | 4082 | 4082 |
| Average | 0.007% | −0.001% | 0.026% | 0.015% | 0.010% |
| Median | 0.031% | 0.014% | 0.042% | 0.000% | 0.000% |
| Maximum | 14.391% | 12.450% | 13.197% | 17.149% | 14.626% |
| Minimum | −25.113% | −12.874% | −10.954% | −20.493% | −12.011% |
| Annual volatility | 32.898% | 25.130% | 19.502% | 37.926% | 26.639% |
| Skewness | −0.658 | −0.235 | −0.330 | −0.614 | −0.313 |
| Excess kurtosis | 9.854 | 8.556 | 16.326 | 8.254 | 7.115 |
| Jarque−Bera | 8284.5 | 5288.2 | 30,279.4 | 4951.5 | 2946.9 |
| MVHR | |||||
|---|---|---|---|---|---|
| Index Future (IF) | ECM | Asy CCC(1,1) | Asy DCC(1,1) | Asy DVEC(1,1) | Asy BEKK(1,1) |
| Average | |||||
| Eurostoxx50 IF | −0.88 | −0.90 | −0.70 | −0.73 | −0.74 |
| S&P500 IF | −0.71 | −0.90 | −0.79 | −0.71 | −0.82 |
| Bovespa IF | −0.64 | −0.56 | −0.55 | −0.55 | −0.50 |
| Ipc IF | −0.77 | −0.68 | −0.49 | −0.57 | −0.60 |
| Minimum | |||||
| Eurostoxx50 IF | −0.85 | −0.48 | −0.09 | −0.11 | 0.09 |
| S&P500 IF | −0.66 | −0.22 | −0.19 | 0.00 | −0.10 |
| Bovespa IF | −0.61 | −0.31 | −0.31 | −0.30 | 0.09 |
| Ipc IF | −0.75 | −0.32 | −0.18 | −0.02 | −0.06 |
| Maximum | |||||
| Eurostoxx50 IF | −0.89 | −1.38 | −1.32 | −1.28 | −1.24 |
| S&P500 IF | −0.74 | −1.91 | −1.74 | −1.75 | −1.65 |
| Bovespa IF | −0.65 | −0.75 | −0.79 | −0.78 | −1.20 |
| Ipc IF | −0.79 | −1.14 | −1.04 | −0.98 | −0.99 |
| Standard Deviation | |||||
| Eurostoxx50 IF | 0.01 | 0.13 | 0.20 | 0.18 | 0.18 |
| S&P500 IF | 0.02 | 0.33 | 0.33 | 0.32 | 0.26 |
| Bovespa IF | 0.01 | 0.06 | 0.09 | 0.08 | 0.24 |
| Ipc IF | 0.01 | 0.13 | 0.17 | 0.17 | 0.16 |
| (a) | |||||
| Index Future (IF) | GARCH Specifications | Asy CCC(1,1) | Asy DCC(1,1) | Asy DVEC(1,1) | Asy BEKK(1,1) |
| Eurostoxx50 IF | Asy CCC(1,1) | 1.00 | 0.05 | 0.09 | 0.02 |
| Asy DCC(1,1) | 0.67 | 1.00 | 0.90 | 0.87 | |
| Asy DVEC(1,1) | 0.62 | 0.92 | 1.00 | 0.95 | |
| Asy BEKK(1,1) | 0.41 | 0.78 | 0.81 | 1.00 | |
| S&P500 IF | Asy CCC(1,1) | 1.00 | 0.92 | 0.76 | 0.54 |
| Asy DCC(1,1) | 0.68 | 1.00 | 0.82 | 0.61 | |
| Asy DVEC(1,1) | 0.62 | 0.82 | 1.00 | 0.84 | |
| Asy BEKK(1,1) | 0.25 | 0.59 | 0.63 | 1.00 | |
| Bovespa IF | Asy CCC(1,1) | 1.00 | 0.77 | 0.76 | 0.08 |
| Asy DCC(1,1) | 0.91 | 1.00 | 0.89 | 0.17 | |
| Asy DVEC(1,1) | 0.81 | 0.93 | 1.00 | 0.20 | |
| Asy BEKK(1,1) | 0.07 | −0.01 | 0.03 | 1.00 | |
| Ipc IF | Asy CCC(1,1) | 1.00 | 0.59 | 0.59 | 0.20 |
| Asy DCC(1,1) | 0.89 | 1.00 | 0.80 | 0.55 | |
| Asy DVEC(1,1) | 0.55 | 0.70 | 1.00 | 0.73 | |
| Asy BEKK(1,1) | 0.34 | 0.52 | 0.38 | 1.00 | |
| Note: The table shows linear correlation coefficients between Minimum Variance Hedge Ratios (MVHR) from different conditional volatility models when using different stock market futures. Values above the diagonal are correlations between MVHR. Values below the diagonal are correlations between daily changes in MVHR. Sample: 783 daily forecasts between 28 August 2017 and 25 August 2020. | |||||
| (b) | |||||
| GARCH Specification | Index Future (IF) | Eurostoxx50 IF | S&P500 IF | Bovespa IF | Ipc IF |
| Asy CCC(1,1) | Eurostoxx50 IF | 1.00 | 0.66 | −0.01 | 0.43 |
| S&P500 IF | 0.54 | 1.00 | −0.03 | 0.51 | |
| Bovespa IF | 0.17 | 0.21 | 1.00 | 0.06 | |
| Ipc IF | 0.45 | 0.40 | 0.27 | 1.00 | |
| Asy DCC(1,1) | Eurostoxx50 IF | 1.00 | 0.18 | 0.40 | 0.55 |
| S&P500 IF | 0.31 | 1.00 | 0.01 | 0.39 | |
| Bovespa IF | 0.22 | 0.27 | 1.00 | 0.31 | |
| Ipc IF | 0.32 | 0.33 | 0.32 | 1.00 | |
| Asy DVEC(1,1) | Eurostoxx50 IF | 1.00 | 0.17 | 0.22 | 0.46 |
| S&P500 IF | 0.34 | 1.00 | 0.10 | 0.39 | |
| Bovespa IF | 0.20 | 0.22 | 1.00 | 0.22 | |
| Ipc IF | 0.25 | 0.22 | 0.18 | 1.00 | |
| Asy BEKK(1,1) | Eurostoxx50 IF | 1.00 | 0.09 | 0.20 | 0.46 |
| S&P500 IF | −0.09 | 1.00 | 0.00 | 0.26 | |
| Bovespa IF | −0.04 | −0.08 | 1.00 | 0.22 | |
| Ipc IF | 0.01 | 0.13 | 0.06 | 1.00 | |
| Note: The table shows linear correlation coefficients between the Minimum Variance Hedge Ratios for different stock market indices, estimated using different conditional volatility models. Values above the diagonal are correlations between MVHR values. Values below the diagonal are correlations between their daily changes. Sample: 783 daily forecasts between 28 August 2017 and 25 August 2020. | |||||
| (a) | ||||||||
| Eurostoxx50 IF | S&P500 IF | Bovespa IF | Ipc IF | |||||
| Variance of the Hedged Portfolio | Reduction from Unhedged Position | Variance of the Hedged Portfolio | Reduction from Unhedged Position | Variance of the Hedged Portfolio | Reduction from Unhedged Position | Variance of the Hedged Portfolio | Reduction from Unhedged Position | |
| Unhedged | 0.362 | 0.362 | 0.362 | 0.362 | ||||
| Naïve Hedge | 0.236 | 34.7% | 0.366 | −1.0% | 0.397 | −9.8% | 0.297 | 17.9% |
| ECM | 0.232 | 36.0% | 0.325 | 10.2% | 0.238 | 34.3% | 0.260 | 28.2% |
| Asy CCC(1,1) | 0.238 | 34.3% | 0.323 | 10.8% | 0.212 | 41.4% | 0.264 | 27.0% |
| Asy DCC(1,1) | 0.237 | 34.6% | 0.319 | 11.9% | 0.214 | 40.9% | 0.254 | 29.7% |
| Asy DVEC(1,1) | 0.239 | 33.9% | 0.320 | 11.8% | 0.213 | 41.2% | 0.262 | 27.6% |
| Asy BEKK(1,1) | 0.238 | 34.2% | 0.318 | 12.1% | 0.252 | 30.5% | 0.249 | 31.3% |
| Note: The left column in each panel shows, under the variance of the LATIBEX index, the variance of each hedged portfolio. All variances have been multiplied by 103. The right column presents the reduction in variance relative to the unhedged position. Sample: 783 daily observations between 28 August 2017 and 25 August 2020. | ||||||||
| (b) | ||||||||
| Eurostoxx50 | S&P500 IF | Bovespa IF | Ipc IF | |||||
| Annual Volatility of the Hedged Portfolio | Reduction from Unhedged Position | Annual Volatility of the Hedged Portfolio | Reduction from Unhedged Position | Annual Volatility of the Hedged Portfolio | Reduction from Unhedged Position | Annual Volatility of the Hedged Portfolio | Reduction from Unhedged Position | |
| Unhedged | 30.21% | 30.21% | 30.21% | 30.21% | ||||
| Naïve Hedge | 24.41% | 19.20% | 30.36% | −0.50% | 31.65% | −4.77% | 27.37% | 9.40% |
| ECM | 24.17% | 19.98% | 28.63% | 5.22% | 24.48% | 18.97% | 25.60% | 15.27% |
| Asy CCC(1,1) | 24.48% | 18.97% | 28.53% | 5.56% | 23.12% | 23.46% | 25.82% | 14.55% |
| Asy DCC(1,1) | 24.43% | 19.13% | 28.36% | 6.13% | 23.22% | 23.14% | 25.32% | 16.18% |
| Asy DVEC(1,1) | 24.55% | 18.72% | 28.38% | 6.07% | 23.17% | 23.31% | 25.71% | 14.90% |
| Asy BEKK(1,1) | 24.51% | 18.88% | 28.32% | 6.26% | 25.19% | 16.62% | 25.03% | 17.14% |
| Note: The left column in each panel shows, under the annualized volatility of the LATIBEX index, the volatility of each hedged portfolio. The right column presents the reduction in volatility relative to the unhedged position. Bold figures indicate the model providing the highest hedge effectiveness for each futures contract. Sample: 783 daily observations between 28 August 2017 and 25 August 2020. | ||||||||
| Index Future (IF) | CE | Unhedged | Naïve Hedge | ECM | Asy CCC(1,1) | Asy DCC(1,1) | Asy DVEC(1,1) | Asy BEKK(1,1) |
|---|---|---|---|---|---|---|---|---|
| Eurostoxx50 IF | Average | −0.031% | −0.026% | −0.026% | −0.020% | −0.023% | −0.020% | −0.018% |
| Standard Deviation | 1.90% | 1.54% | 1.52% | 1.54% | 1.54% | 1.55% | 1.54% | |
| Skewness | −0.75 | −0.57 | −0.63 | −0.71 | −0.67 | −0.60 | −0.57 | |
| Excess Kurtosis | 4.99 | 5.13 | 5.05 | 5.09 | 4.89 | 5.27 | 5.29 | |
| CE (daily) | −0.11% | −0.07% | −0.07% | −0.07% | −0.07% | −0.07% | −0.07% | |
| CE (annual) | −26.52% | −18.71% | −18.45% | −17.14% | −17.99% | −17.28% | −16.68% | |
| S&P500 IF | Average | −0.031% | −0.075% | −0.061% | −0.072% | −0.069% | −0.062% | −0.069% |
| Standard Deviation | 1.90% | 1.91% | 1.80% | 1.80% | 1.79% | 1.79% | 1.78% | |
| Skewness | −0.75 | −0.45 | −0.58 | −0.68 | −0.69 | −0.70 | −0.56 | |
| Excess Kurtosis | 4.99 | 5.68 | 4.56 | 4.69 | 4.77 | 4.80 | 4.40 | |
| CE (daily) | −0.11% | −0.15% | −0.13% | −0.14% | −0.13% | −0.13% | −0.13% | |
| CE (annual) | −26.52% | −37.64% | −32.02% | −34.62% | −33.66% | −32.13% | −33.75% | |
| Bovespa IF | Average | −0.031% | −0.003% | −0.012% | −0.015% | −0.017% | −0.024% | 0.055% |
| Standard Deviation | 1.90% | 1.99% | 1.54% | 1.46% | 1.46% | 1.46% | 1.59% | |
| Skewness | −0.75 | 0.28 | −0.59 | −0.92 | −0.93 | −0.96 | 0.96 | |
| Excess Kurtosis | 4.99 | 25.37 | 14.94 | 7.40 | 7.49 | 6.98 | 18.26 | |
| CE (daily) | −0.11% | −0.08% | −0.06% | −0.06% | −0.06% | −0.07% | 0.00% | |
| CE (annual) | −26.52% | −20.84% | −15.31% | −14.75% | −15.27% | −16.93% | 1.25% | |
| Ipc IF | Average | −0.031% | 0.034% | 0.019% | 0.007% | −0.006% | −0.009% | 0.009% |
| Standard Deviation | 1.90% | 1.72% | 1.61% | 1.63% | 1.60% | 1.62% | 1.58% | |
| Skewness | −0.75 | −0.17 | −0.36 | −0.50 | −0.59 | −0.64 | −0.49 | |
| Excess Kurtosis | 4.99 | 2.82 | 3.01 | 3.61 | 3.76 | 3.94 | 3.09 | |
| CE (daily) | −0.11% | −0.03% | −0.03% | −0.05% | −0.06% | −0.06% | −0.04% | |
| CE (annual) | −26.52% | −6.41% | −8.33% | −11.80% | −14.42% | −15.70% | −10.38% |
| Position | Index Future (IF) | Performance | Volatility (Annual) | Futures Index | Performance | Certainty Equivalent (Annual) |
|---|---|---|---|---|---|---|
| 1 | Bovespa IF | Asym CCC | 23.12% | Bovespa IF | Asym BEKK | 1.25% |
| 2 | Bovespa IF | Asym. DVEC | 23.17% | Ipc IF | Naïve Hedge | −6.41% |
| 3 | Bovespa IF | Asym DCC | 23.22% | Ipc IF | ECM | −8.33% |
| 4 | Eurostoxx50 IF | ECM | 24.17% | Ipc IF | Asym BEKK | −10.38% |
| 5 | Eurostoxx50 IF | Naïve Hedge | 24.41% | Ipc IF | Asym CCC | −11.80% |
| 6 | Eurostoxx50 IF | Asym DCC | 24.43% | Ipc IF | Asym DCC | −14.42% |
| 7 | Eurostoxx50 IF | Asym CCC | 24.48% | Bovespa IF | Asym CCC | −14.75% |
| 8 | Bovespa IF | ECM | 24.48% | Bovespa IF | Asym DCC | −15.27% |
| 9 | Eurostoxx50 IF | Asym BEKK | 24.51% | Bovespa IF | ECM | −15.31% |
| 10 | Eurostoxx50 IF | Asym DVEC | 24.55% | Ipc IF | Asym DVEC | −15.70% |
| 11 | Ipc IF | Asym BEKK | 25.03% | Eurostoxx50 IF | Asym BEKK | −16.68% |
| 12 | Bovespa IF | Asym BEKK | 25.19% | Bovespa IF | Asym DVEC | −16.93% |
| 13 | Ipc IF | Asym DCC | 25.32% | Eurostoxx50 IF | Asym CCC | −17.14% |
| 14 | Ipc IF | ECM | 25.60% | Eurostoxx50 IF | Asym DVEC | −17.28% |
| 15 | Ipc IF | Asym DVEC | 25.71% | Eurostoxx50 IF | Asym DCC | −17.99% |
| 16 | Ipc IF | Asym CCC | 25.82% | Eurostoxx50 IF | ECM | −18.45% |
| 17 | Ipc IF | Naïve Hedge | 27.37% | Eurostoxx50 IF | Naïve Hedge | −18.71% |
| 18 | S&P500 IF | Asym BEKK | 28.32% | Bovespa IF | Naïve Hedge | −20.84% |
| 19 | S&P500 IF | Asym DCC | 28.36% | Eurostoxx50 IF | Unhedged | −26.52% |
| 20 | S&P500 IF | Asym DVEC | 28.38% | S&P500 IF | Unhedged | −26.52% |
| 21 | S&P500 IF | Asym CCC | 28.53% | Bovespa IF | Unhedged | −26.52% |
| 22 | S&P500 IF | ECM | 28.63% | Ipc IF | Unhedged | −26.52% |
| 23 | Eurostoxx50 IF | Unhedged | 30.21% | S&P500 IF | ECM | −32.02% |
| 24 | S&P500 IF | Unhedged | 30.21% | S&P500 IF | Asym DVEC | −32.13% |
| 25 | Bovespa IF | Unhedged | 30.21% | S&P500 IF | Asym DCC | −33.66% |
| 26 | Ipc IF | Unhedged | 30.21% | S&P500 IF | Asym BEKK | −33.75% |
| 27 | S&P500 IF | Naïve Hedge | 30.36% | S&P500 IF | Asym CCC | −34.62% |
| 28 | Bovespa IF | Naïve Hedge | 31.65% | S&P500 IF | Naïve Hedge | −37.64% |
| (a) | ||
| B3—Brasil Bolsa Balcão | Bolsa Mexicana de Valores | |
| Domestic market capitalization (USD millions) (a) | 832,365 | 306,771 |
| Number of listed companies | 331 | 144 |
| Value of share trading (USD millions) (b) | 814,621 | 60,127 |
| Note: (a) Data for July 2020. (b) Data accumulated in the period January–July 2020. | ||
| (b) | ||
| Number of Listed Companies | Capitalization Weighting | |
| Brazil | 15 | 72.7% |
| Mexico | 5 | 26.9% |
| Argentina | 1 | 0.3% |
| Peru | 1 | 0.2% |
| Total | 22 | 100.0% |
| Note: Composition of the FTSE LATIBEX All Share index at the close of the session on 28 August 2020. | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Urtubia, P.; Novales, A.; Mora-Valencia, A. Cross-Hedging Portfolios in Emerging Stock Markets: Evidence for the LATIBEX Index. Mathematics 2021, 9, 2736. https://doi.org/10.3390/math9212736
Urtubia P, Novales A, Mora-Valencia A. Cross-Hedging Portfolios in Emerging Stock Markets: Evidence for the LATIBEX Index. Mathematics. 2021; 9(21):2736. https://doi.org/10.3390/math9212736
Chicago/Turabian StyleUrtubia, Pablo, Alfonso Novales, and Andrés Mora-Valencia. 2021. "Cross-Hedging Portfolios in Emerging Stock Markets: Evidence for the LATIBEX Index" Mathematics 9, no. 21: 2736. https://doi.org/10.3390/math9212736
APA StyleUrtubia, P., Novales, A., & Mora-Valencia, A. (2021). Cross-Hedging Portfolios in Emerging Stock Markets: Evidence for the LATIBEX Index. Mathematics, 9(21), 2736. https://doi.org/10.3390/math9212736







