The Approximate Analytic Solution of the Time-Fractional Black-Scholes Equation with a European Option Based on the Katugampola Fractional Derivative
Abstract
1. Introduction
2. Literature Review
2.1. Basic Definitions and Some Properties of Fractional Calculus
- 2.
- the Hadamard fractional integral of order
- 3.
- the Riemann–Liouville fractional derivative of order
- 4.
- the Hadamard fractional derivative of order
2.2. The Generalized Laplace Transform
- 2.
- .
2.3. Special Functions
- 1.
- is decreasing,
- 2.
- and for any
3. Methodology
3.1. Basic Idea of the GLHPM Technique
3.2. Existence and Uniqueness
3.3. Convergence Analysis and Error Estimation for GLHPM
3.4. The Approximate Analytic Solution of the Time-Fractional Black-Scholes Equation
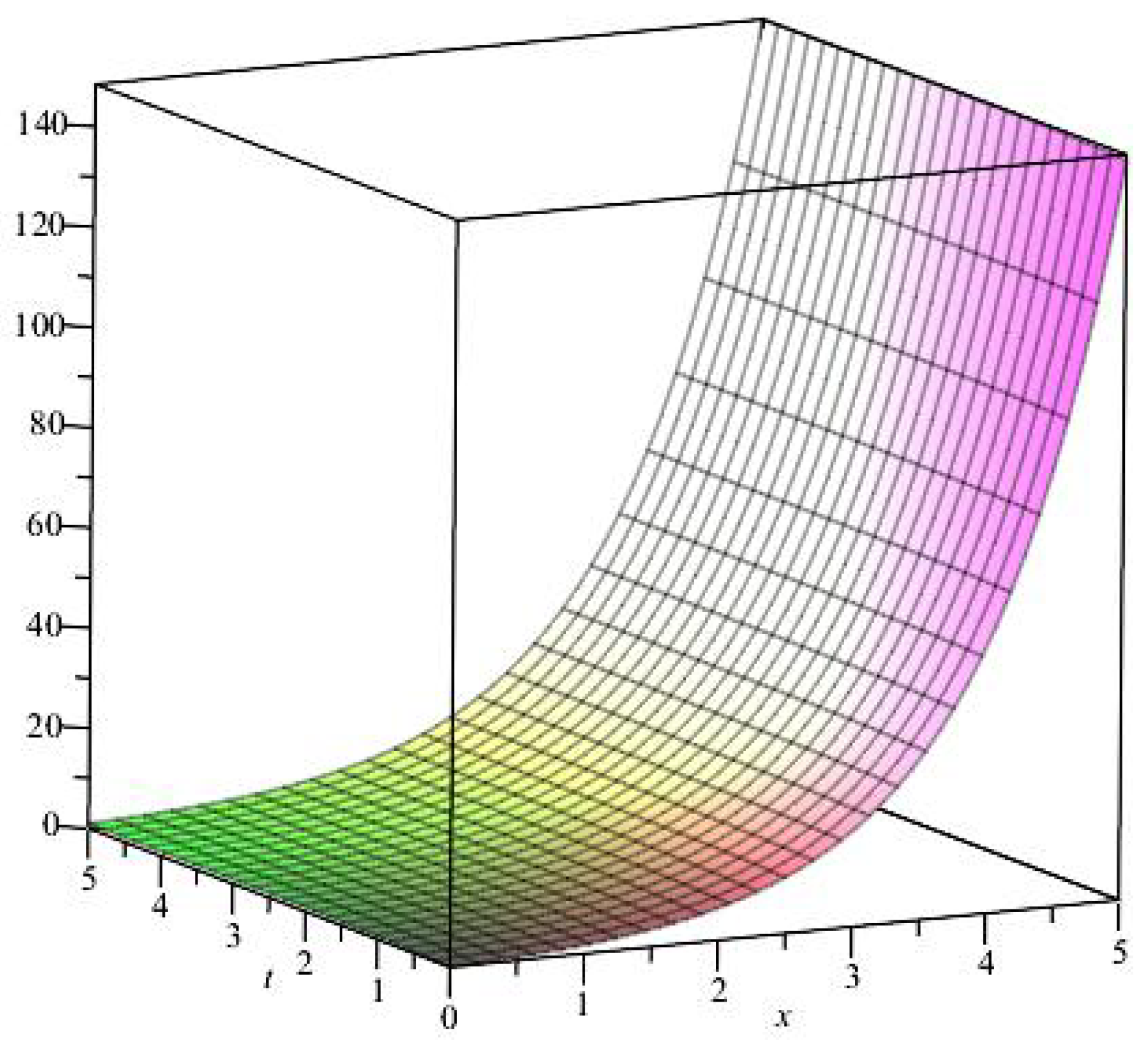
4. Numerical Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Black, F.; Scholes, M. The pricing of options and corporate liabilities. J. Polit. Econ. 1973, 81, 637–654. [Google Scholar] [CrossRef]
- Owoloko, E.A.; Okeke, M.C. Investigating the Imperfection of the B–S Model: A Case Study of an Emerging Stock Market. Br. J. Appl. Sci. Tech. 2014, 4, 4191–4200. [Google Scholar] [CrossRef]
- Mandelbrot, B. The variation of certain speculative prices. J. Bus. 1963, 36, 394–413. [Google Scholar] [CrossRef]
- Peters, E.E. Fractal structure in the capital markets. Financ. Anal. J. 1989, 45, 32–37. [Google Scholar] [CrossRef]
- Li, H.Q.; Ma, C.Q. An empirical study of long-term memory of return and volatility in Chinese stock market. J. Financ. Econ. 2005, 31, 29–37. [Google Scholar]
- Huang, T.F.; Li, B.Y.; Xiong, J.X. Test on the chaotic characteristic of Chinese futures market. Syst. Eng. 2012, 30, 43–53. [Google Scholar]
- Debnath, L. Recent applications of fractional calculus to science and engineering. Int. J. Math. Math. Sci. 2003, 2003, 753601. [Google Scholar] [CrossRef]
- He, J.H.; El-Dib, Y.O. Periodic property of the time-fractional Kundu–Mukherjee–Naskar equation. Results Phys. 2020, 19, 103345. [Google Scholar] [CrossRef]
- Noeiaghdam, S.; Dreglea, A.; He, J.; Avazzadeh, Z.; Suleman, M.; Fariborzi Araghi, M.A.; Sidorov, D.N.; Sidorov, N. Error Estimation of the Homotopy Perturbation Method to Solve Second Kind Volterra Integral Equations with Piecewise Smooth Kernels: Application of the CADNA Library. Symmetry 2003, 12, 1730. [Google Scholar] [CrossRef]
- Anjum, N.; He, J.H. Higher-order homotopy perturbation method for conservative nonlinear oscillators generally and microelectromechanical systems’ oscillators particularly. Int. J. Mod. Phys. B 2020, 34, 2050313. [Google Scholar] [CrossRef]
- Song, L. A semianalytical solution of the fractional derivative model and its application in financial market. Complexity 2018, 2018, 1872409. [Google Scholar] [CrossRef]
- Edeki, S.O.; Ugbebor, O.O.; Owoloko, E.A. Analytical solutions of the Black–Scholes pricing model for european option valuation via a projected differential transformation method. Entropy 2015, 17, 7510–7521. [Google Scholar] [CrossRef]
- Smeureanu, I.; Fanache, D. A Linear Algorithm for Black–Scholes Economic Model. Rev. Inform. Econ. 2008, 1, 150–156. [Google Scholar]
- Wilmott, P.; Howson, S.; Howison, S.; Dewynne, J. The Mathematics of Financial Derivatives: A Student Introduction; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Sawangtong, P.; Trachoo, K.; Sawangtong, W.; Wiwattanapataphee, B. The Analytical Solution for the Black-Scholes Equation with Two Assets in the Liouville-Caputo Fractional Derivative Sense. Mathematics 2018, 8, 129. [Google Scholar] [CrossRef]
- Kumar, S.; Yildirin, A.; Khan, Y.; Jafari, H.; Sayevand, K.; Wei, L. Analytical solution of fractional Black-Scholes European option pricing equations using Laplace transform. J. Frac. Cal. Appl. 2012, 2, 1–9. [Google Scholar]
- Kumar, S.; Kumar, D.; Singh, J. Numerical computation of fractional Black-Scholes equation arising in financial market. Egypt. J. Basic Appl. Sci. 2014, 1, 177–183. [Google Scholar] [CrossRef]
- Yavuz, M.; Özdemir, N. European vanilla option pricing model of fractional order without singular kernel. Fractal Fract. 2018, 2, 3. [Google Scholar] [CrossRef]
- Yavuz, M. European option pricing models described by fractional operators with classical and generalized Mittag Leffler kernels. Numer. Methods Partial. Differ. Equ. 2020. [Google Scholar] [CrossRef]
- Fall, A.N.; Ndiaye, S.N.; Sene, N. Black–Scholes option pricing equations described by the Caputo generalized fractional derivative. Chaos Solitons Fractals 2019, 125, 108–118. [Google Scholar] [CrossRef]
- Ahmad, J.; Shakee, Q.M.; Hassan, U.I.; Mohyud-Din, S.T. Analytical solution of Black-Scholes model using fractional variational iteration method. Int. J. Mod. Math. Sci. 2013, 5, 133–142. [Google Scholar]
- Blanco-Cocom, L.; Estrella, A.G.; Avila-Vales, E. Solution of the Black-Scholes equation via the Adomian decomposition method. Int. J. Appl. Math. Res. 2013, 2, 486–494. [Google Scholar]
- Sripacharasakullert, P.; Sawangtong, W.; Sawangtong, P.; Wiwattanapataphee, B. An approximate analytical solution of the fractional multi-dimensional Burgers equation by the homotopy perturbation method. Adv. Differ. Equa. 2019, 1, 1–12. [Google Scholar] [CrossRef]
- Trachoo, K.; Sawangtong, W.; Sawangtong, P. Laplace Transform Homotopy Perturbation Method for the Two Dimensional Black Scholes Model with European Call Option. Math. Comp. Appl. 2017, 1, 23. [Google Scholar] [CrossRef]
- Sawangtong, W.; Sawangtong, P. Green’s function homotopy perturbation method for the initial-boundary value problems. Adv. Differ. Equ. 2019, 1, 419. [Google Scholar] [CrossRef]
- Katugampola, U.N. A new approach to generalized fractional derivatives. arXiv 2011, arXiv:1106.0965. [Google Scholar]
- Jarad, F.; Abdeljawad, T. Generalized fractional derivatives and Laplace transform. Discret. Cont. Dyn. Syst. S 2019, 13, 709. [Google Scholar] [CrossRef]
- Sene, N.; Fall, A.N. Homotopy perturbation ρ-Laplace transform method and its application to the fractional diffusion equation and the fractional diffusion-reaction equation. Fractal Fract. 2019, 3, 14. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and applications of fractional differential equations. In North-Holland Mathematics Studies; Elsevier Science B.V.: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Garrappa, R.; Popolizio, M. Generalized exponential time differencing methods for fractional order problems. Comput. Math. Appl. 2011, 62, 876. [Google Scholar] [CrossRef]
- Ghorbani, A. Beyond Adomian polynomials: He polynomials. Chaos Solitons Fractals 2009, 39, 1486–1492. [Google Scholar] [CrossRef]

| order () | 1 | 1 | 1 | 1 | 1 |
| order () | 0.6 | 0.8 | 1 | 1.2 | 1.5 |
| rate (r) | 0.03 | 0.03 | 0.03 | 0.03 | 0.03 |
| volatility () | 0.01 | 0.01 | 0.01 | 0.01 | 0.01 |
| time () | 0.5 | 0.5 | 0.5 | 0.5 | 0.5 |
| expiration price (E) | 100 | 100 | 100 | 100 | 100 |
| asset price( S) | 100 | 100 | 100 | 100 | 100 |
| maturity (T) | 1 | 1 | 1 | 1 | 1 |
| European call option (C) | 21.32 | 3.29 | 0.49 | 0.077 | 0.0049 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ampun, S.; Sawangtong, P. The Approximate Analytic Solution of the Time-Fractional Black-Scholes Equation with a European Option Based on the Katugampola Fractional Derivative. Mathematics 2021, 9, 214. https://doi.org/10.3390/math9030214
Ampun S, Sawangtong P. The Approximate Analytic Solution of the Time-Fractional Black-Scholes Equation with a European Option Based on the Katugampola Fractional Derivative. Mathematics. 2021; 9(3):214. https://doi.org/10.3390/math9030214
Chicago/Turabian StyleAmpun, Sivaporn, and Panumart Sawangtong. 2021. "The Approximate Analytic Solution of the Time-Fractional Black-Scholes Equation with a European Option Based on the Katugampola Fractional Derivative" Mathematics 9, no. 3: 214. https://doi.org/10.3390/math9030214
APA StyleAmpun, S., & Sawangtong, P. (2021). The Approximate Analytic Solution of the Time-Fractional Black-Scholes Equation with a European Option Based on the Katugampola Fractional Derivative. Mathematics, 9(3), 214. https://doi.org/10.3390/math9030214






