1. Introduction
Various studies have shown that trainee teachers’ knowledge about education is influenced by their learning experience during their time as primary and secondary education students [
1,
2]. Therefore, these trainee teachers must necessarily go through new learning experiences that may afterwards be embedded in their teaching practice. Calderhead [
3] emphasises the need to provide opportunities for trainee teachers to grasp teaching strategies that are different from those they have encountered in the past. Such need is even greater in the case of teaching mathematics, partly due to its formal and abstract character and because of the difficulties faced by many students in this subject.
Trainee teachers frequently believe that practical activities in mathematics lessons are limited to problem solving or the development of exercises. Generally, they do not think that presenting problematic situations in which mathematics provides solutions or activities requiring processes of abstraction with a basis in specific or general situations to model daily life phenomena may also be practical mathematics activities in the classroom [
4]. All the same, taking on the role of problem-solvers helps them understand or identify the cognitive processes essential for carrying out an activity or to fathom a particular concept.
Concurrently, it is important that situations enabling understanding of the underlying rationale behind what they will be teaching in the future be presented during teacher training. As Jones [
5] claims, we must leverage teacher training so teachers-to-be acknowledge and appreciate the nature of mathematics, its function, how humankind is still creating mathematics, which is not static but dynamic, and that they can also feel the emotion of discovering such changes. The history of mathematics is an adequate resource to present students with these situations and to have them reflect on why we teach certain concepts, rules or operations while setting aside others which were successfully used in the past.
It is a fact that learning mathematics involves more than the mere accumulation of elements of knowledge. On the contrary, it requires comprehension and interrelation of such elements, something that the history of the field of study allows for. Grugnetti [
6] points out that the influence of the history of mathematics in pedagogical problems can be observed via a range of methods—for example, using old problems for students to compare their strategies with those rooted in the past. History can also be used to introduce concept arousal and “an historical and epistemological analysis allows teachers to understand why a certain concept is difficult for the student [...] and can help in the didactical approach and development” [
6] (p. 30). Considering that the history of mathematics can additionally be taken as the driving force to organise the curriculum—as proposed by Rico [
7]—it becomes a valuable resource for support at curricular, didactic, conceptual and formative levels.
Jankvist [
8] advances varied arguments pointing at the history of mathematics as a tool or resource for the actual mathematics class, which are related to how students learn mathematics. In this sense, he indicates that there are studies attempting to prove that its use enhances motivation and stimulates interest in mathematics. He asserts that it can also support learning by providing different points of view or ways to present certain concepts.
Sometimes, with the goal of taking advantage of history as a motivating element, the idea of history is included “as a means to promote mathematics in the classroom in order to humanize mathematics” [
9] (p. 3). As Radford [
10] states, doing so may result in anecdotal accounts, which are quite insubstantial considering that there are other ways to go about this, such as using problems from the past in the classroom and having students solve them.
On the other hand, Dorier and Rogers [
11] allege that an epistemological reflection on the development of ideas through the history of mathematics can enrich didactic analysis, providing essential clues about the nature of teachable knowledge and, at the same time, exploring different ways to access such knowledge.
As noted by Jahnke [
12], students learn something about their own mathematics knowledge when experimenting and reflecting on the contrast between modern concepts and their historic counterpart. According to Jahnke, a hermeneutic circle is created that complements reflection on the part of the students, bidirectionally enabling them to deepen their understanding of both history of mathematics and their own set of modern concepts.
International literature reveals many instances of history of mathematics being embedded in classroom practice at different levels [
13,
14,
15,
16,
17]. As a means of example, in Israel, trainee elementary school teachers, in general, do not specialise in mathematics. Those who wish to teach mathematics are encouraged to participate in professional development programmes to expand their knowledge on the matter. Within these courses, experiences using the history of mathematics for instruction of trainees were included, having the participants develop a more humanistic side of mathematics [
18].
In this respect, Lawrence [
19] suggests using the history of mathematics through an interdisciplinary project with trainee primary and secondary teachers that aims at approaching mathematics from an interesting and original perspective—i.e., as an element of history and cultural patrimony. In primary education, Gardner [
20] describes classroom experiences with 8- to 10-year-old students in which problems from the Rhind Papyrus were used as a basis.
In the early 1980s, Freudenthal [
21] asked: should a mathematics teacher know something about the history of mathematics? He thought about the fact that, in other disciplines, for example, medicine, their history is studied in university training. However, the history of mathematics was being removed and he pleaded for the relevance of at least giving mathematics college students the opportunity to learn about the history of this discipline. We consider that the same applies to students who are specifically trained as mathematics teachers of primary education, secondary school or A-levels.
Arcavi [
22] points out that using situations from the history of mathematics in courses with teachers or teachers-to-be stimulates discussions about mathematical ideas in which teachers rarely reinspect. This is because they tend to falsely believe that in mathematics it is not possible to figure out anything new due to the fact that mathematical ideas are closed and already consolidated. Therefore, Arcavi says that these stimuli will make teachers aware of important aspects in which they may have not thought about before.
Another aspect emphasized by Arcavi [
22] is that using the history of mathematics helps to sensitize teachers to the possible difficulties that students may experience in order to understand certain mathematical concepts. This helps teachers to put themselves in the place of their students and to become aware of the possible difficulties that are attached to some concepts.
Additionally, Krantz [
23] points out the importance of mathematics teachers using historical examples which include mathematical references in order to help students improve their understanding of these mathematical problems.
Another example of this is the study of Ozdemir, Goktepe and Kepceoglu [
24]; these authors show how history of mathematics activities related to the volume of solids, strengthened 11th grade high school students’ geometric proof skills and their spatial perceptions. Additionally, Karaduman [
25] demonstrates how the use of materials related to the history of mathematics provides an important tool for improving students’ achievements.
It is on these grounds that we have tried to introduce the history of mathematics in the classroom, fostering reflection upon specific contents. We had already introduced history in a master’s degree in teaching in secondary schools, vocational training centres and language schools (speciality in mathematics) as a way to support learning particular concepts [
17]. This paper attempts to reveal the differences between some historical or unusual multiplication methods and the modern-day one, which is used more often in Spanish mathematics lessons, considered by secondary education mathematics trainee teachers and by primary education ones. As reported by Brown [
26], specific contents, questions and examples used in class must begin with ideas that are familiar to students. Multiplication was accordingly chosen, since, as Smith [
27] claimed, the development of the idea of multiplication and the process itself is more interesting than the evolution of other, more primitive, concepts.
This study is premised on the assumption that multiplication is the operation performed on two factors and which has the goal of determining a third number, specific and unique, called the product. It is an internal binary operation that, on the set of integers, satisfies the properties of closure, commutativity, associativity, existence of an identity element and distributivity over addition [
28].
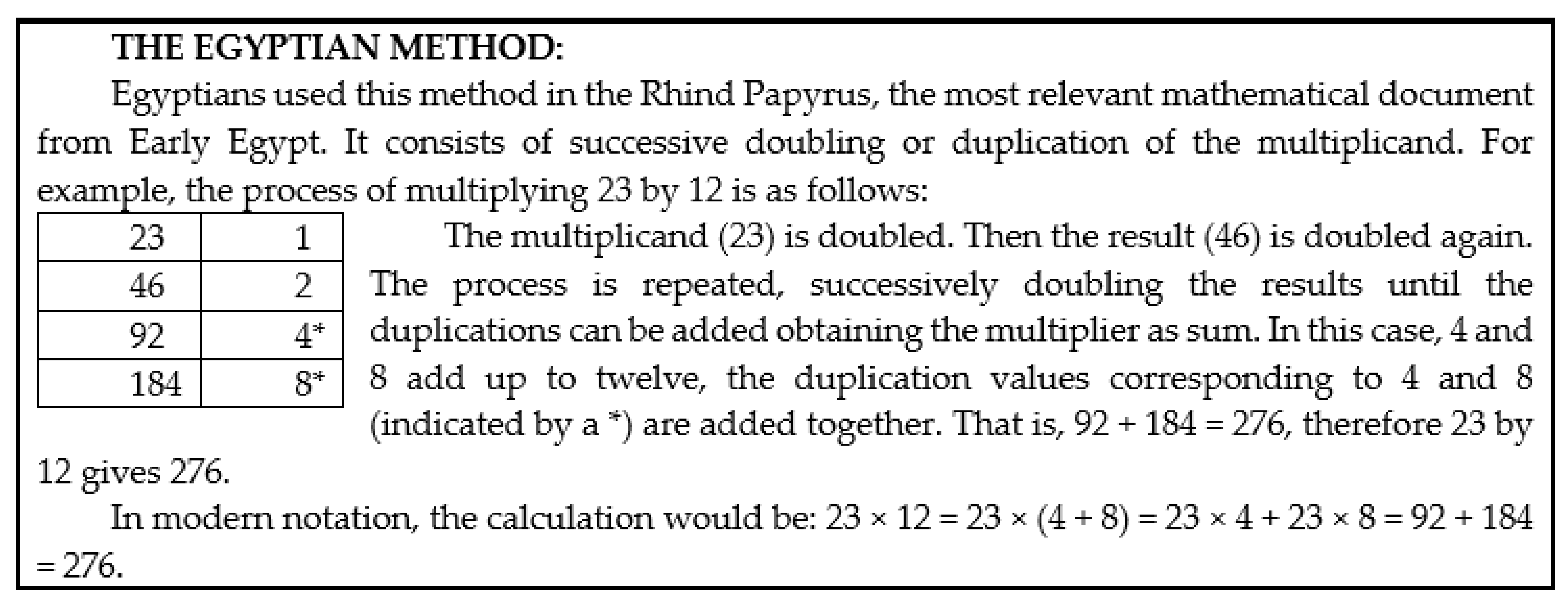
The historical approach will be brought out by the introduction of two historical or unusual multiplication methods. The Egyptian multiplication method that was used in times of Ahmes, halfway through the 16th century B.C.E., was performed through successive
duplation and
mediation [
29] (p. 36) of the factors. A more recent example of the same type of algorithm can be found in the
duplation and
mediation operations used by Russian peasants [
27].
Fauvel [
30] argued that, among all the reasons for using the history of mathematics, it enables comparison between the techniques used in antiquity and the more modern ones in order to establish the value of the latter. From this approach, the research issue arising is: can the history of mathematics be used in class for revealing differences in the value of the current multiplication method in relation to those from antiquity within teacher education courses at different levels? The question is currently of increasing interest as various methods for teaching mathematical operations have emerged over recent years. As a matter of fact, these methods have a basis in methods of calculation used in the past that were set aside in favour of the modern-day algorithm, which, being more optimal, allows the obtention of maximum efficiency from our decimal numeral system.
However, Siu [
31] points out that several unfavourable factors for the use of the history of mathematics in the classroom are related to the teachers’ beliefs regarding their students’ opinions about the use of history in the classroom. That is to say, teachers consider that their students believe that history of mathematics is not useful or is not very formal or that they do not even understand why it is used, which discourages teachers from using it. Therefore, we consider showing trainee teachers the history of mathematics and how it can be used in the classroom to be relevant.
Due to this, our study has two objectives. On the one hand, we aim to identify and classify the differences between two unusual or historical multiplication methods and a modern-day one revealed by trainee teachers. On the other hand, we aim to determine whether the established differences vary in type and percentage depending on the type of mathematical knowledge that students need to have in order to become teachers of mathematics at different levels—i.e., students of the primary education teaching degree in relation to the teaching in secondary and A-level schools, vocational training centres and language school master’s degrees.
This second objective is related to the difference in the access requisites for the primary education teaching degree and the teaching in secondary and A-level schools, vocational training centres and language school master’s degrees (speciality mathematics) in Spain.
According to Real Decreto 412/2014 [
32], students can access any university degree from different paths and, depending on these, students may have studied mathematics only in compulsory education.
In order to access the teaching in secondary and A-level schools, vocational training centres and language school master’s degrees (speciality of mathematics), ORDEN ECI/3858/2007 [
33] states that an accreditation of mastery of the competencies related to the desired specialization (in this case, mathematics) is required. This can be shown by passing a test designed for this purpose by the universities. However, all who accredited a university degree that corresponds to the chosen specialization do not need to pass this exam.
2. Materials and Methods
The mathematics course in year 1 of the primary education degree at the University of Cordoba (Spain) addresses the subject of numeral systems and operations with natural and integer numbers. During one of the practical lessons, a one-hour-long activity about unusual multiplication methods was carried out—more specifically, about the Egyptian method [
27,
29] and that of the Russian peasants [
27]. As already mentioned, these two methods rely on the knowledge of duplication and halving (
Figure 1 and
Figure 2); due to this, we believe they could be easily understandable by all the students and therefore, they could compare between them and the modern-day multiplication method they already know.
In order to work with these methods in the classroom, they were adapted to modern-day notation.
The final task of the activity included one final question requesting the differences regarding the multiplication algorithm we use currently in Spanish classrooms. This study presents the results and analysis of these question.
The same one-hour-long activity was carried out as part of a section devoted to the history of mathematics within the Disciplinary Instruction Complements for Mathematics and Computer Science module in the secondary and A-level education teaching master’s degree (speciality in mathematics). The objective of so doing was to try and find out the potentially different perceptions from primary teachers-to-be and their secondary/A-level counterparts, who already have more advanced knowledge on the matter since they, unlike the formers, need to have passed advanced mathematics courses to comply with the entrance requirements of their master’s degree.
The study population was composed of all year 1 students studying for the primary education degree and the students studying for the secondary and A-level education teaching master’s degrees who were specialising in mathematics—both degrees at the University of Cordoba for the 2015–2016 and 2016–2017 academic years. The sample comprised 158 students belonging to one line per year of the primary education degree (120 students) and the master’s degree (mathematics modality) (38 students).
This study is exploratory, qualitative and descriptive in nature [
34]. The data obtained were analysed using the content analysis technique provided by ATLAS.ti (Version 5.0) software [
35], which enabled us to establish categories of conceptual correlation. ATLAS.ti software is used as a tool for qualitative analysis—it facilitates the elaboration of semantic networks, which allows the graphic representation of the different components and the relationships that have been established between them, and therefore it has advantages for carrying out research in mathematics education [
36]. For example, Maz-Machado, Madrid, León-Mantero and Jiménez-Fanjul [
37] studied the perceptions of trainee teachers regarding the realisation of practical mathematical sessions to expand on the concepts that were taught in primary education using manipulatives; in order to do so, they used ATLAS.ti software.
To perform the analysis, the starting point was not any pre-established set of labels. On the contrary, labels emerged from the answers given by students and were consensually determined by triangulation by experts in didactics of mathematics and educational research methods of the universities of Cordoba, Pontificia de Salamanca and Salamanca. In sum, 17 labels or codes were assigned and grouped into 4 categories according to their conceptual type.
Figure 3 shows an example of how the answers led to categorisation (the answers given by the students to the questions were written in Spanish and were later translated by the researchers).
The
Figure 3 shows a primary education teacher-to-be student’s answer about the differences:
“They are very slow methods, which can be seen in the operational process in the table, so the process itself is also another difference. On the other hand, to multiply they only need to know the sum and multiply as well as divide by 2. In our method, the one we use now, we must know how to multiply by more numbers. There is also a difference in notation, since the operational process is different.”
This response was categorized by the experts as “Slower”, “They just need to multiply or divide by two” and “The have different operational processes”.
To summarize, each of the answers given by the students was defined as an analysis unit and were categorized and finally analysed.
3. Results and Discussion
Out of the 158 students who participated in the activity, only six reported to know both methods (two from the master’s degree and four from the primary education degree)—that is, 3.8% of the analysed sample. Nevertheless, none of them recalled the procedures through which the operations are performed. The rest of the students did not know the methods.
This is not unusual. For example, Madrid, Maz-Machado, León-Mantero and López-Esteban [
38] state that history of mathematics has little relevance in mathematics textbooks of the first and second years of compulsory secondary education in Spain, where it usually appears briefly as something complementary.
This situation is not exclusive for Spain. For example, Alpaslan, Işıksal and Haser [
39] considered that preservice teachers in Turkey were inadequately introduced to history of mathematics during their school education.
The analysis showed a range of differences between these two historical or unusual multiplication methods dealt with in class as compared with the modern-day one, which all the students already knew. These differences were categorised under 17 labels, which were grouped into the four big families or categories hereby listed.
Additionally, in order to clarify the relationships among the labels from each category, we included the diagrams generated by ATLAS.ti software. These diagrams present the following relations:
“is part of” indicates the inclusion relation of the labels in each category.
“is associated with” indicates that two or more labels verify the necessary and sufficient condition—i.e., one is fulfilled if and only if the other one is fulfilled.
“is cause of” indicates that a label is related to another because it is the reason why the first one is fulfilled.
- (a)
Structure of the method
It is concerned with how problematic or effective using these methods may be in relation to the modern-day one. It compiles six differences (
Figure 4): differences in notation, simpler, more complex, less practical, more intuitive and less effective.
- (b)
Procedure and application
This refers to procedures concerning duration, extension and level of difficulty/easiness in performing two-digit multiplications that are considered basic with those historical or unusual methods, compared to using the modern-day algorithm. Five differences were revealed (
Figure 5): slower, faster, more difficult, easier and longer.
- (c)
Mathematical processes
These include those processes associated with mathematical actions that are necessary to solve the multiplication concerning previous knowledge and operations that one needs to have—i.e., relative to the transference of previous knowledge required to perform it. There are four differences (
Figure 6): more operations are required as compared with the modern-day method, knowledge of all the multiplication tables is not necessary, it is only necessary to multiply or divide by two and the methods follow different operational processes.
- (d)
Other
This category comprises those differences that cannot be associated with any other difference, it includes only two differences (
Figure 7): they are entertaining, and students do not know how to explain the differences or explanation is confusing.
When a distinction between the different academic levels is made, we acquire the same categories with only slight variation as regards the differences found.
As for the structure of the method (
Table 1), differences between students in the primary education degree and those in the master’s degree are made evident. The students in the primary education degree considered differences in notation and simplicity of the methods, issues that the master’s students did not indicate. The students at the master’s level considered that the current method is simpler than the two introduced methods. This may point out that they are more aware of the potentiality of our decimal numeral system regarding fundamental arithmetic operations, as it is multiplication. In contrast, they highlight the intuitive character of these methods.
It is noteworthy that a greater percentage of the degree students than of those in the masters’ degree students believe the Egyptian and Russian methods to be less practical and less effective than the modern-day method, considering that the students in the masters’ degree had deeper mathematical training. This may be related to the fact that a greater percentage of the degree students claim that they are more complex. All the same, allegedly and probably due to their instruction, the degree students take into account more didactic aspects for stating these differences.
Generally, when the totality of students is considered for analysing “structure of the method”—i.e., the easiness or difficulty in using the modern-day method in comparison with that of the historical or unusual methods—the majority of students describe them as less practical, less effective and more complex, therefore characterising the modern-day multiplication method as optimal.
In the category of “procedure and application” (
Table 2), the percentage of students claiming that these methods are longer than the modern-day one was coincident. However, much greater were the percentages of degree students indicating differences in speed (slower), easiness (easier) and difficulty (more difficult) of completion. From this we can infer that, again, the degree students pay attention to procedural aspects for solving mathematical problems. Remarkably, the degree students also stated that the presented methods were faster than the current method, which suggests that they may lack command of the multiplication tables.
On the whole, a high percentage of the primary education students find the historical or unusual methods slower and longer than the current one. The same situation is evidenced in the group of mathematics teachers-to-be. On the contrary, 10% of the degree students believe that they are faster, while no single master’s student made such a statement. This result is in good agreement with the previously mentioned conclusion: the students completing master’s degrees are more aware that the current method to perform basic arithmetic operations is optimal.
Regarding the mathematical processes (
Table 3), the highest percentage of difference is found in the group of degree students for the difference “it is only necessary to multiply or divide by two”. This may again be due to the difficulties that students could be facing when it comes to multiplying by numbers that are higher than two, more troubling than just doubling or halving a number. The findings suggest that students value these methods for being different to the ones usually used to practice multiplications or divisions by two, stressing their didactic potential.
Smestad and Nikolantonakis [
40] considered that, for teachers, it is important to see that the multiplication tables are not necessary to carry out multiplications.
Table 3 shows how some of the teachers-to-be considered this.
The rest of the differences in the category shed relatively similar results. Considering the differences between the year 1 students in the primary education degree and the master’s students specialising in mathematics who completed degrees with more mathematics in the syllabus, it is surprising that the latter did not focus more on mathematics-related aspects. This may be due to the fact that the trend over the last few years is that many students completing master’s degrees no longer enrol after getting a degree in mathematics, or even that some of them lack knowledge about the curriculum of mathematics that must be covered during secondary education [
41].
Finally, we analysed the difference showing higher percentage of presence among the established categories. Within the “procedures” category, percentage is highest for the difference type
slower. Arguably, this difference is related as much to the aspects of structure as to the mathematical procedures. From students’ points of view, being longer makes these methods slower and more difficult (
Figure 8).
Within the category of “structure”, the difference with highest percentage of presence is
more complex. This is connected to a difference in procedures and with those regarding mathematical processes, though not with any other difference also under the “structure” tag (
Figure 9). It seems that, for the students in the sample, complexity hinges on the type and variety of operations that must be performed to obtain the product, characteristics that add difficulty to the method.
In the “mathematical processes” category, the difference with the greatest presence is
It is only necessary to multiply or divide by two, which relates with differences in the categories of structure and procedures (
Figure 10). However, contradictory correlations can be found: on the one side, some students indicate that the act of multiplying/dividing only by two makes them easier and faster than the introduced historical or unusual methods. Others claim that this is precisely what makes them longer and, hence, more difficult. These ideas are present in both the primary education and secondary education mathematics student groups.
4. Conclusions
Numerous authors report the benefits of including the history of mathematics in the classroom. Alternatively, it is generally unknown to teachers-to-be. Proof of this is that, out of the students who engaged in the activity about unusual multiplication methods, less than 4% knew any of these methods and even those who knew about them did not recall the processes they followed to perform the operations.
This is also pointed out in other studies such as Ozdemir, Goktepe and Kepceoglu [
24] or Karaduman [
25]; they concluded that teachers generally do not have the knowledge or the adequate preparation to integrate the history of mathematics in the teaching of this subject. However, as Smestad and Nikolantonakis stated [
40], it is important for teachers to be able to understand different algorithms and analysing historical algorithms can be a good way to achieve this.
The analysis of the differences noted by the students in the primary education degree and those in the master’s degree (specialisation in mathematics) reveals, by and large, many similarities. This finding is quite surprising, given the distinct mathematical instruction that is required for a primary education teachers-to-be in relation to a secondary/A-level mathematics ones. If we consider the components of the mathematical knowledge tree that Fischbein postulates [
42], formal knowledge, algorithmic knowledge and intuitive knowledge, mathematics teachers-to-be at secondary school and A-levels should have a greater formal knowledge of mathematics than primary education teachers-to-be, due to their previous studies; despite this fact, the results obtained do not seem to reflect this.
Regarding the differences between the degree and master’s degree students, those who find these methods simpler, easier and faster are remarkably among the former. Further study on the rationale that led them to such an understanding would be of interest. That is, to know whether this is due to lack of mathematical knowledge about the multiplication tables or if their answers are based on didactic aspects.
A key limitation of this study was that students were asked to voluntarily and anonymously complete the activity in order to avoid the problem of students’ answers being conditioned by any possible influence on their grades or on assessment of their knowledge. Although we believe that compliance with this premise was achieved, it hindered us from conducting interviews that would have enabled us to clarify or delve into each student’s answers on several occasions.
Furthermore, another limitation of our study is the nature of our sample; we collected the data in a Spanish university and, therefore, the results obtained in a similar study carried out in another country, region or institution could be different—for example, due to differences in the curricular contents among institutions or countries. However, we consider this would provide new opportunities for this line of research. For instance, researchers could compare the information obtained in this study with data collected from students of different degrees, in different institutions or in different geographic regions.
Ultimately, the realisation of this activity allowed students to learn about different multiplication methods and evaluate possible advantages and disadvantages as compared with the current method. At the same time, it gave students the opportunity to grasp the potential of the history of mathematics in the classroom, which may increase the chances of them using these tools with their pupils if they become teachers in the future.
Finally, we believe that the realization of this kind of practical activity will allow, as Watson and Sullivan [
43] indicate, them to revisit familiar knowledge (multiplication algorithms that they know from their primary education) but in new ways, which will favour their preparation as mathematics teachers-to-be. We can conclude that this study offers teacher trainers and mathematics teachers information about the opinions of students on one of the most important and fundamental arithmetic operations—multiplication—taking into account that, as teachers-to-be, they should not only know this mathematical structure, but also master it. The differences found between the two groups of students, which are probably due to the different knowledge of mathematics that they have, highlights the relevance of the inclusion of mathematics content in the training of mathematics teachers-to-be.