Numerical Study of Leakage and Diffusion of Underwater Oil Spill by Using Volume-of-Fluid (VOF) Technique and Remediation Strategies for Clean-Up
Abstract
:1. Introduction
2. Computational Methodologies
2.1. Governing Equations
2.2. Geometric Model and Boundary Conditions
2.3. Grid Details and Grid Mesh Independence
2.4. Model Validation
3. Results and Discussion
3.1. Effects of the Oil Spill Velocity
3.2. Effects of the Ocean Current Velocity
3.3. Effects of the Crude Oil Density
3.4. Effects of the Crude Oil Viscosity
3.5. Parameter Fitting
3.6. Remediation Suggestions on Oil Spill Treatment Measures
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, W.; Xie, P.; Li, Y.; Teng, L.; Zhu, J. Hydrodynamic characteristics and mass transfer performance of rotating packed bed for CO2 removal by chemical absorption: A review. J. Nat. Gas Sci. Eng. 2020, 79, 103373. [Google Scholar] [CrossRef]
- Zidane, A.; Firoozabadi, A. Fracture-cross-flow equilibrium in compositional two-phase reservoir simulation. SPE J. 2017, 22, 950–970. [Google Scholar] [CrossRef]
- Moreira, G.; Magalhães, H.L.F.; de Almeida Tavares, D.P.S.; de Brito Correia, B.R.; Leite, B.E.; da Costa Pereira, A.B.; de Farias Neto, S.R.; de Lima, A.G.B. Fluid Leakage in Submerged Offshore Pipeline: An Analysis of Oil Dispersion in Seawater. Open J. Fluid Dyn. 2020, 10, 95–121. [Google Scholar] [CrossRef]
- Yang, Z.; Yu, J.; Li, Z.; Chen, H.; Jiang, M.; Chen, X. Application of computational fluid dynamics simulation for submarine oil spill. Acta Oceanol. Sin. 2018, 37, 104–115. [Google Scholar] [CrossRef]
- Beyer, J.; Trannum, H.C.; Bakke, T.; Hodson, P.V.; Collier, T.K. Environmental effects of the Deepwater Horizon oil spill: A review. Mar. Pollut. Bull. 2016, 110, 28–51. [Google Scholar] [CrossRef] [Green Version]
- Wei, L.; Hu, Z.; Dong, L.; Zhao, W. A damage assessment model of oil spill accident combining historical data and satellite remote sensing information: A case study in Penglai 19-3 oil spill accident of China. Mar. Pollut. Bull. 2015, 91, 258–271. [Google Scholar] [CrossRef]
- Keramea, P.; Spanoudaki, K.; Zodiatis, G.; Gikas, G.; Sylaios, G. Oil spill modeling: A critical review on current trends, perspectives, and challenges. J. Mar. Sci. Eng. 2021, 9, 181. [Google Scholar] [CrossRef]
- Omar, M.Y.; Shehada, M.F.; Mehanna, A.K.; Elbatran, A.H.; Elmesiry, M.M. A case study of the Suez Gulf: Modelling of the oil spill behavior in the marine environment. Egypt. J. Aquat. Res. 2021, 47, 345–356. [Google Scholar] [CrossRef]
- Guo, W.; Zhang, S.; Wu, G. Quantitative oil spill risk from offshore fields in the Bohai Sea, China. Sci. Total Environ. 2019, 688, 494–504. [Google Scholar] [CrossRef]
- Ye, X.; Chen, B.; Lee, K.; Storesund, R.; Li, P.; Kang, Q.; Zhang, B. An emergency response system by dynamic simulation and enhanced particle swarm optimization and application for a marine oil spill accident. J. Clean. Prod. 2021, 297, 126591. [Google Scholar] [CrossRef]
- Zhu, H.; You, J.; Zhao, H. Underwater spreading and surface drifting of oil spilled from a submarine pipeline under the combined action of wave and current. Appl. Ocean Res. 2017, 64, 217–235. [Google Scholar] [CrossRef]
- Kyaw, W.P.; Sugiyama, M.; Takagi, Y.; Suzuki, H.; Kato, N. Numerical analysis of tsunami-triggered oil spill from industrial parks in Osaka Bay. J. Loss Prev. Process Ind. 2017, 50, 325–336. [Google Scholar] [CrossRef]
- Raznahan, M.; An, C.; Li, S.S.; Geng, X.; Boufadel, M. Multiphase CFD simulation of the nearshore spilled oil behaviors. Environ. Pollut. 2021, 288, 117730. [Google Scholar] [CrossRef]
- Zhu, H.; Lin, P.; Pan, Q. A CFD (computational fluid dynamic) simulation for oil leakage from damaged submarine pipeline. Energy 2014, 64, 887–899. [Google Scholar] [CrossRef]
- Zhu, H.; You, J.; Zhao, H. An experimental investigation of underwater spread of oil spill in a shear flow. Mar. Pollut. Bull. 2017, 116, 156–166. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Cao, X.; Liang, F. Investigation on underwater spreading characteristics and migration law of oil leakage from damaged submarine pipelines. Process Saf. Environ. Prot. 2019, 127, 329–347. [Google Scholar] [CrossRef]
- Ji, H.; Xu, M.; Huang, W.; Yang, K. The Influence of Oil leaking rate and Ocean Current Velocity on the Migration and Diffusion of Underwater Oil Spill. Sci. Rep. 2020, 10, 9226. [Google Scholar] [CrossRef] [PubMed]
- Xu, W.; Wang, S.; Liu, G.; Zhang, Q.; Hassan, M.; Lu, H. Experimental and numerical investigation on heat transfer of Therminol heat transfer fluid in an internally four-head ribbed tube. Int. J. Therm. Sci. 2017, 116, 32–44. [Google Scholar] [CrossRef]
- Xiong, J.; Cheng, R.; Lu, C.; Chai, X.; Liu, X.; Cheng, X. CFD simulation of swirling flow induced by twist vanes in a rod bundle. Nucl. Eng. Des. 2018, 338, 52–62. [Google Scholar] [CrossRef]
- Yan, T.; Qu, J.; Sun, X.; Chen, Y.; Hu, Q.; Li, W.; Zhang, H. Numerical investigation on horizontal wellbore hole cleaning with a four-lobed drill pipe using CFD-DEM method. Powder Technol. 2020, 375, 249–261. [Google Scholar] [CrossRef]
- Han, Y.; Nambi, I.M.; Prabhakar Clement, T. Environmental impacts of the Chennai oil spill accident—A case study. Sci. Total Environ. 2018, 626, 795–806. [Google Scholar] [CrossRef] [PubMed]


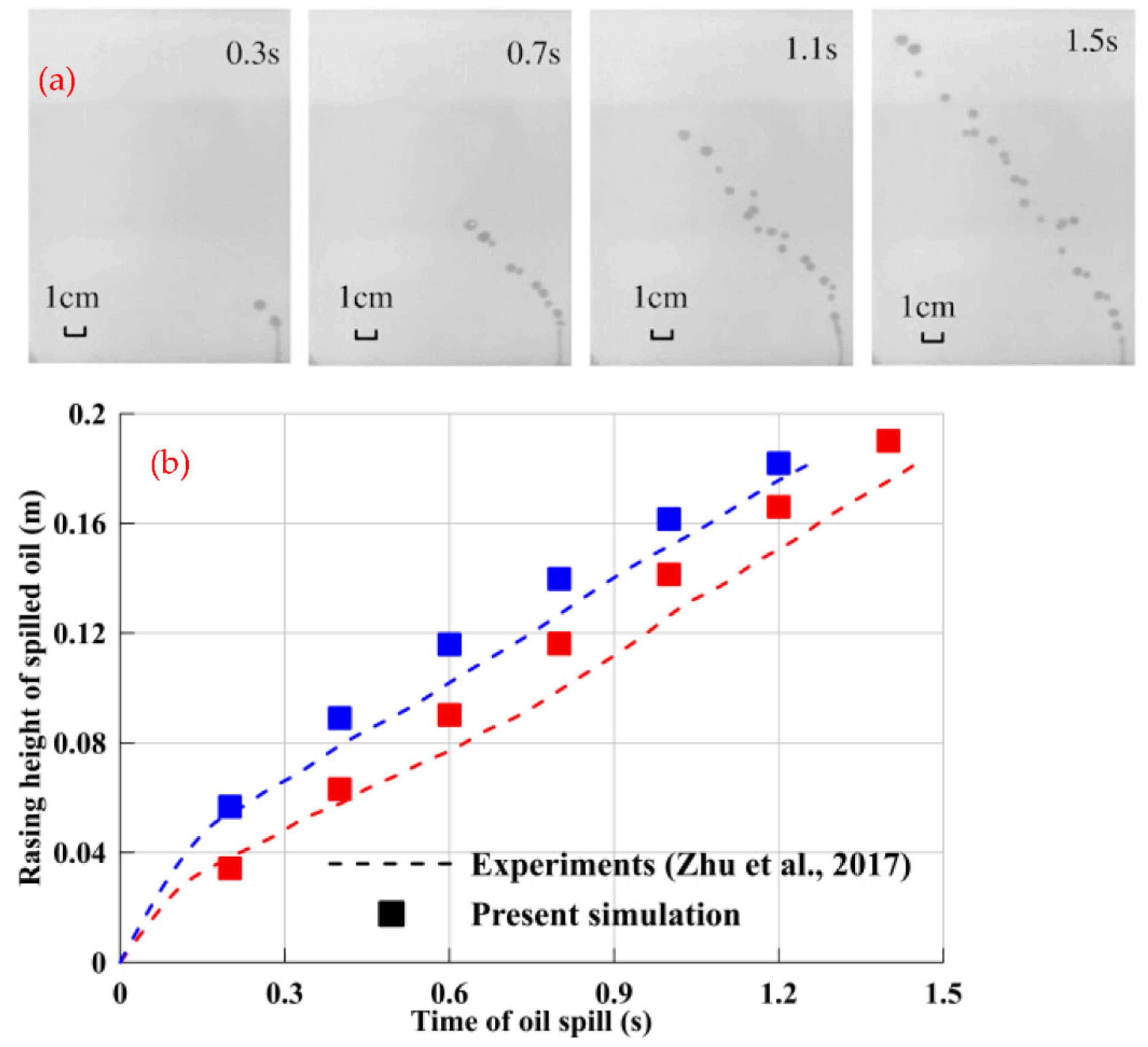
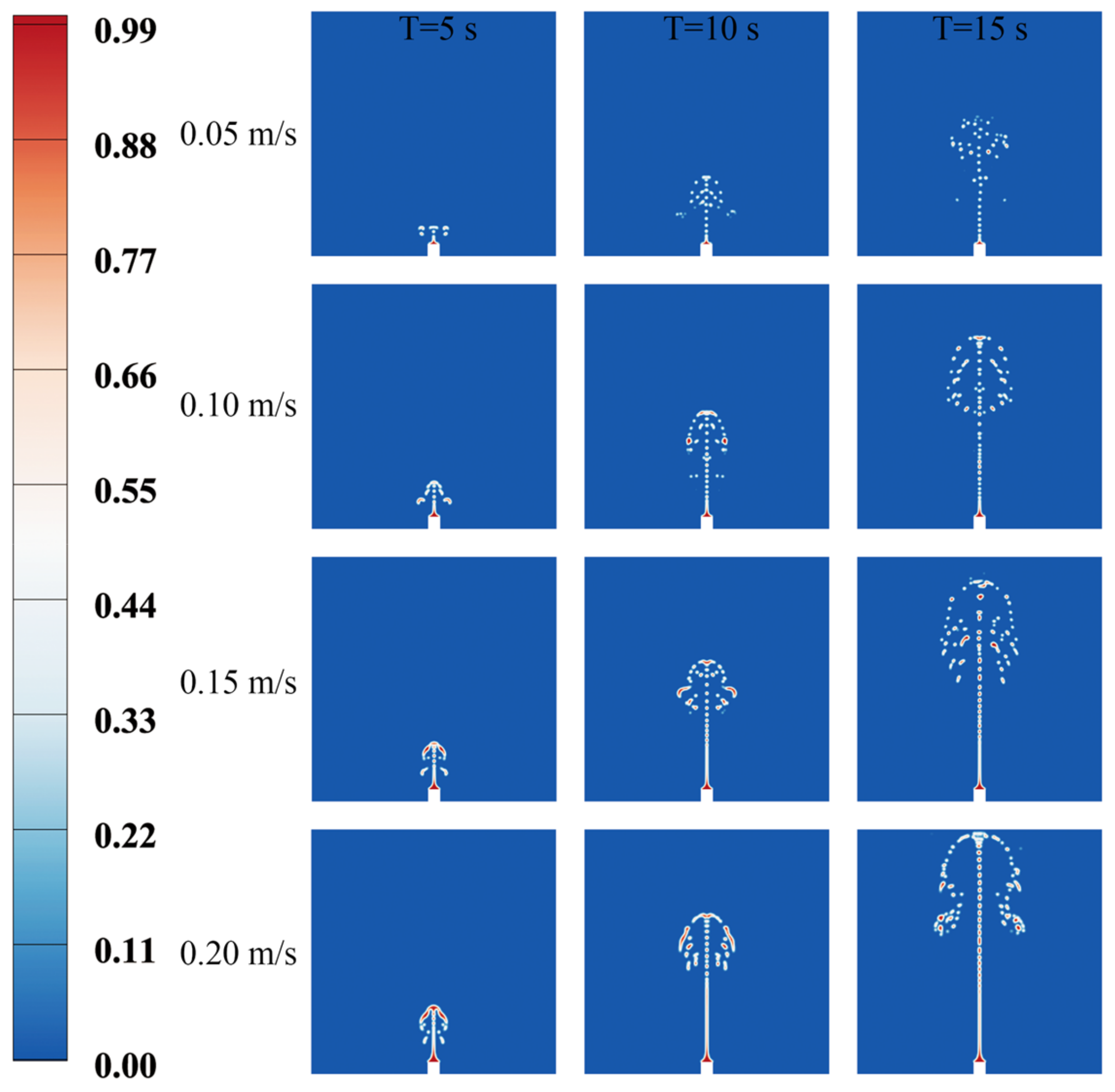
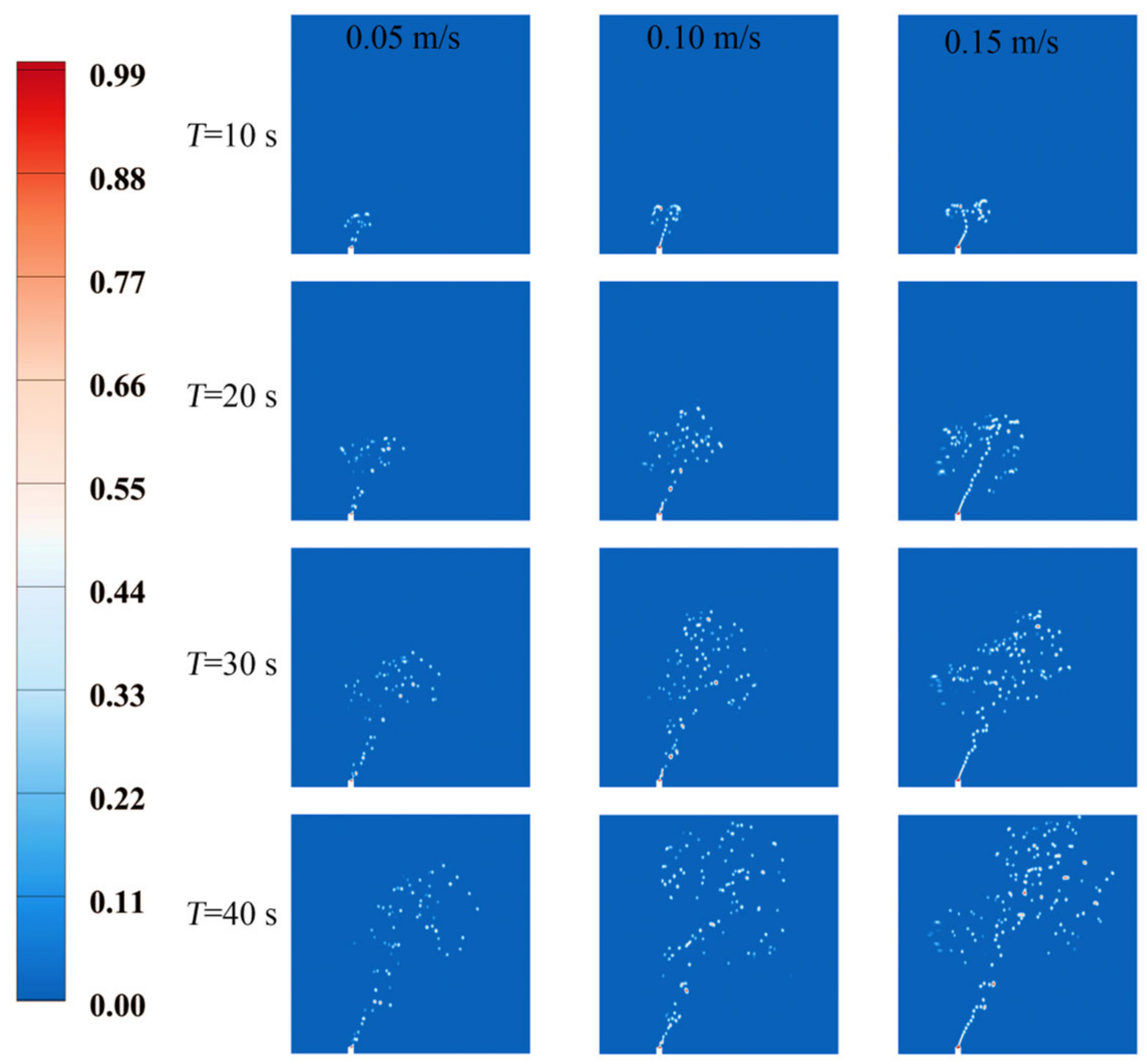
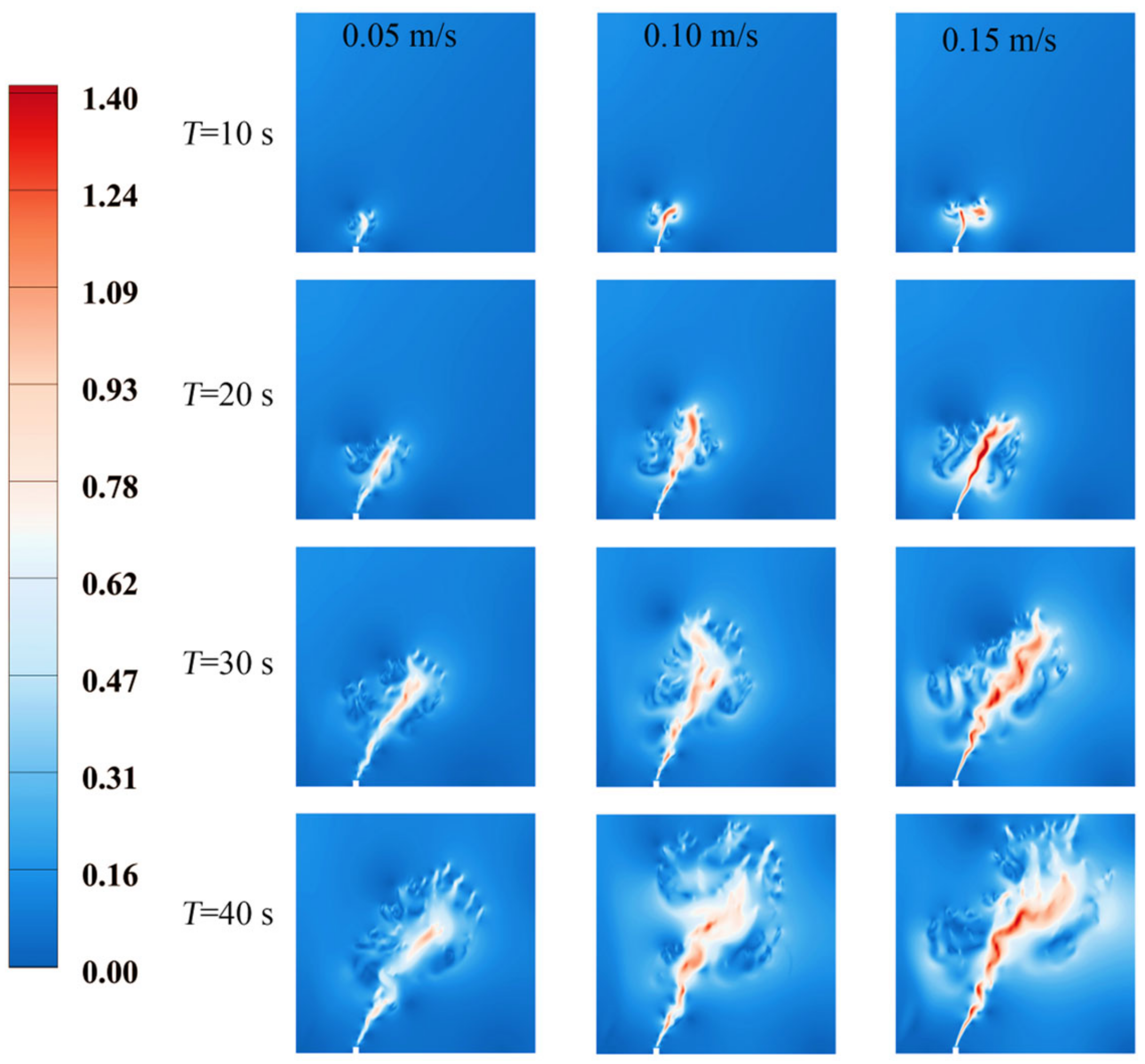
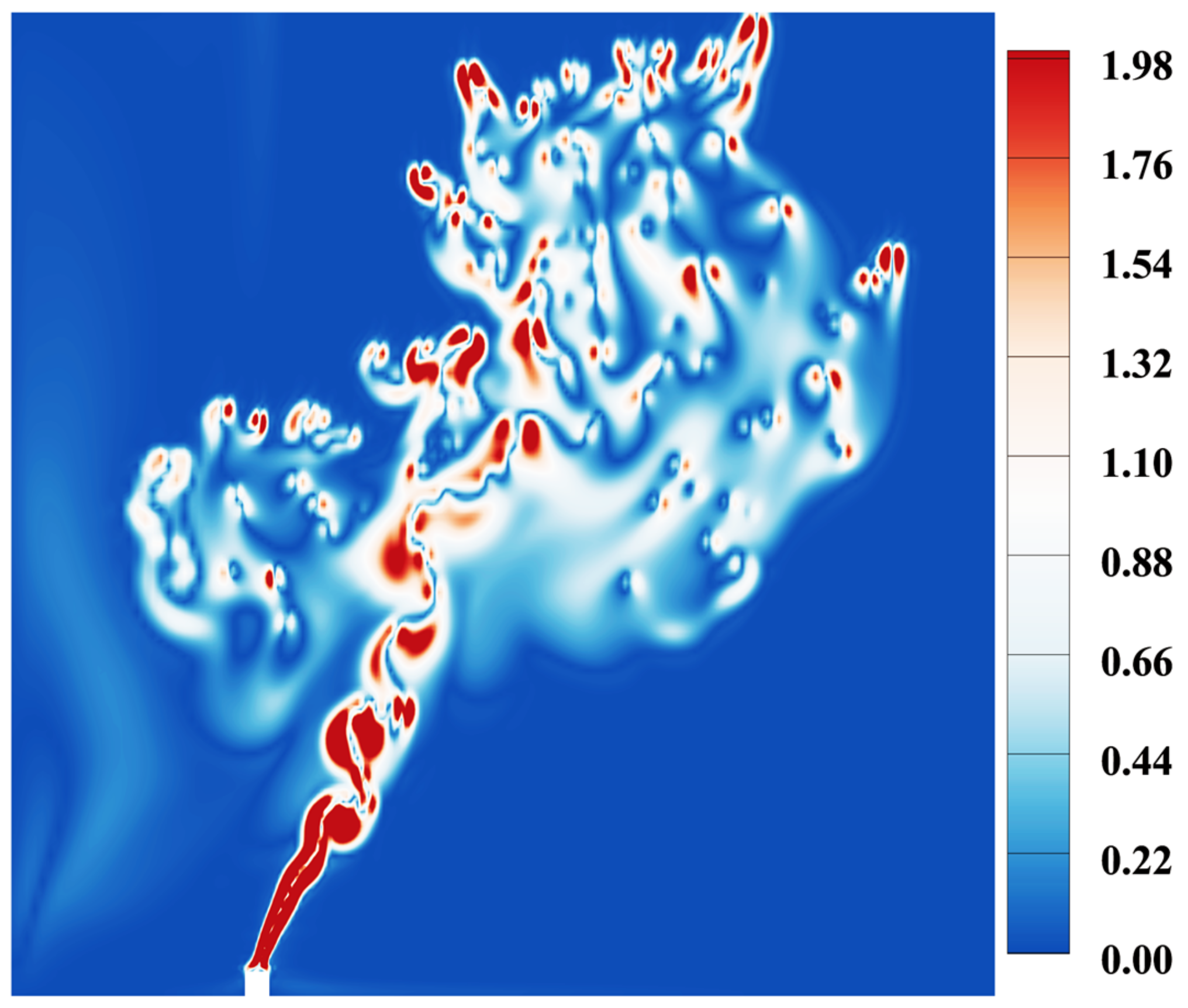
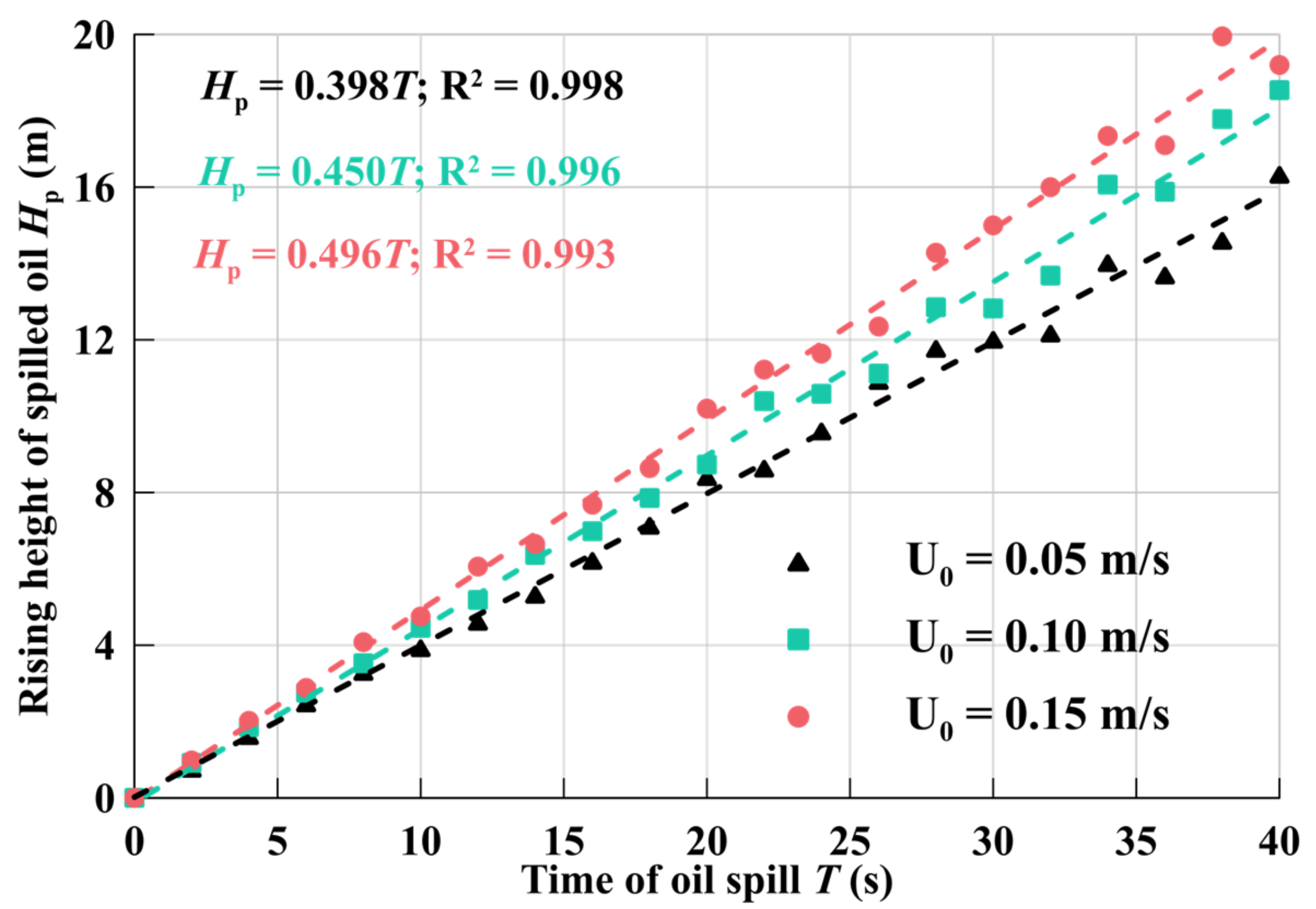





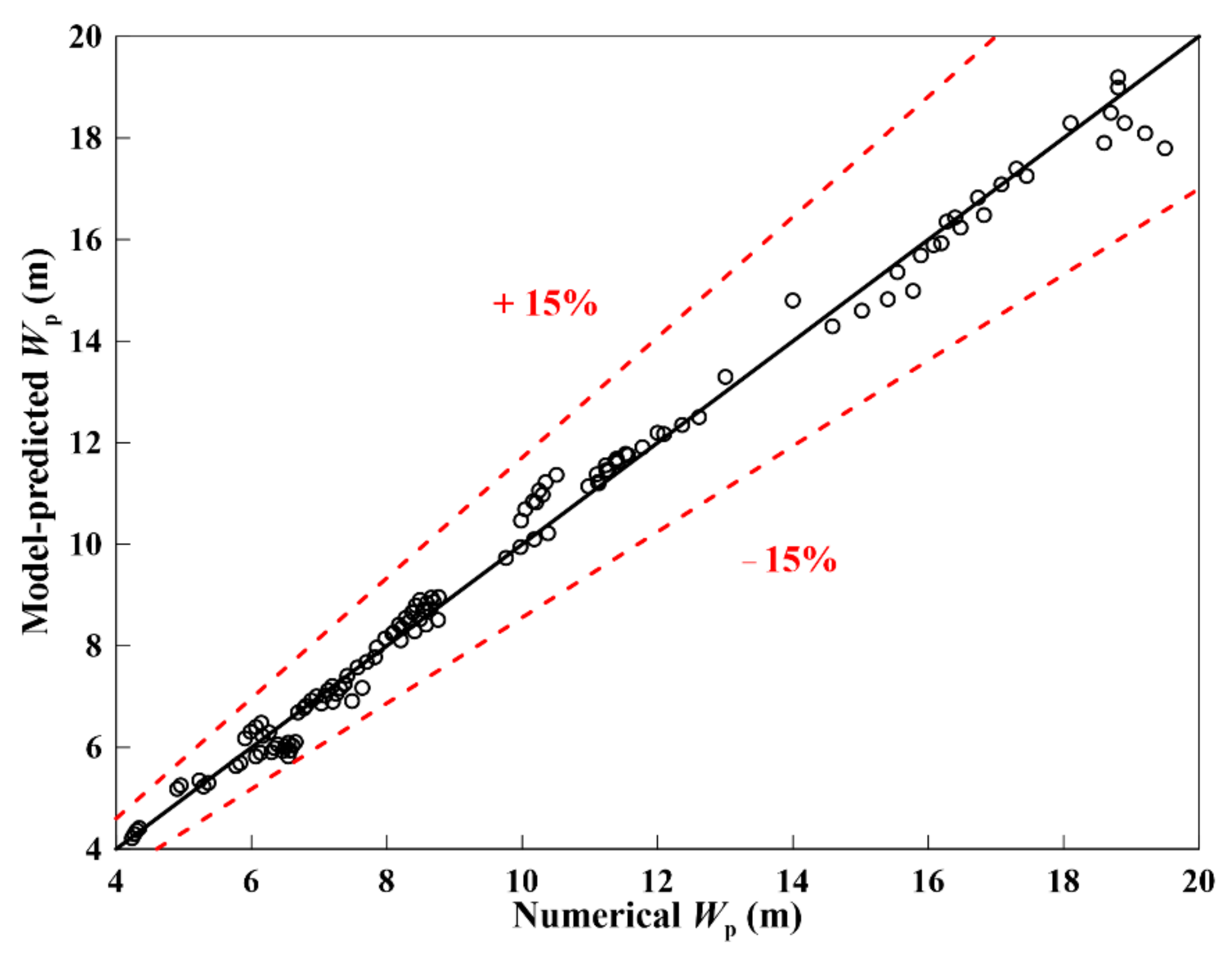
| Mesh Numbers | Rising Height of Spilled Oil (m) | Error (%) |
|---|---|---|
| 54,302 | 17.48 | / |
| 111,502 | 18.57 | 6.24% |
| 188,702 | 18.83 | 1.40% |
| 285,902 | 18.91 | 0.42% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, R.; Ding, S.; Ju, G. Numerical Study of Leakage and Diffusion of Underwater Oil Spill by Using Volume-of-Fluid (VOF) Technique and Remediation Strategies for Clean-Up. Processes 2022, 10, 2338. https://doi.org/10.3390/pr10112338
Liu R, Ding S, Ju G. Numerical Study of Leakage and Diffusion of Underwater Oil Spill by Using Volume-of-Fluid (VOF) Technique and Remediation Strategies for Clean-Up. Processes. 2022; 10(11):2338. https://doi.org/10.3390/pr10112338
Chicago/Turabian StyleLiu, Rengguang, Shidong Ding, and Guoshuai Ju. 2022. "Numerical Study of Leakage and Diffusion of Underwater Oil Spill by Using Volume-of-Fluid (VOF) Technique and Remediation Strategies for Clean-Up" Processes 10, no. 11: 2338. https://doi.org/10.3390/pr10112338
APA StyleLiu, R., Ding, S., & Ju, G. (2022). Numerical Study of Leakage and Diffusion of Underwater Oil Spill by Using Volume-of-Fluid (VOF) Technique and Remediation Strategies for Clean-Up. Processes, 10(11), 2338. https://doi.org/10.3390/pr10112338





