Theoretical Analysis of the Movement Law of Top Coal and Overburden in a Fully Mechanized Top-Coal Caving Face with a Large Mining Height
Abstract
:1. Background Introduction
2. Engineering Background
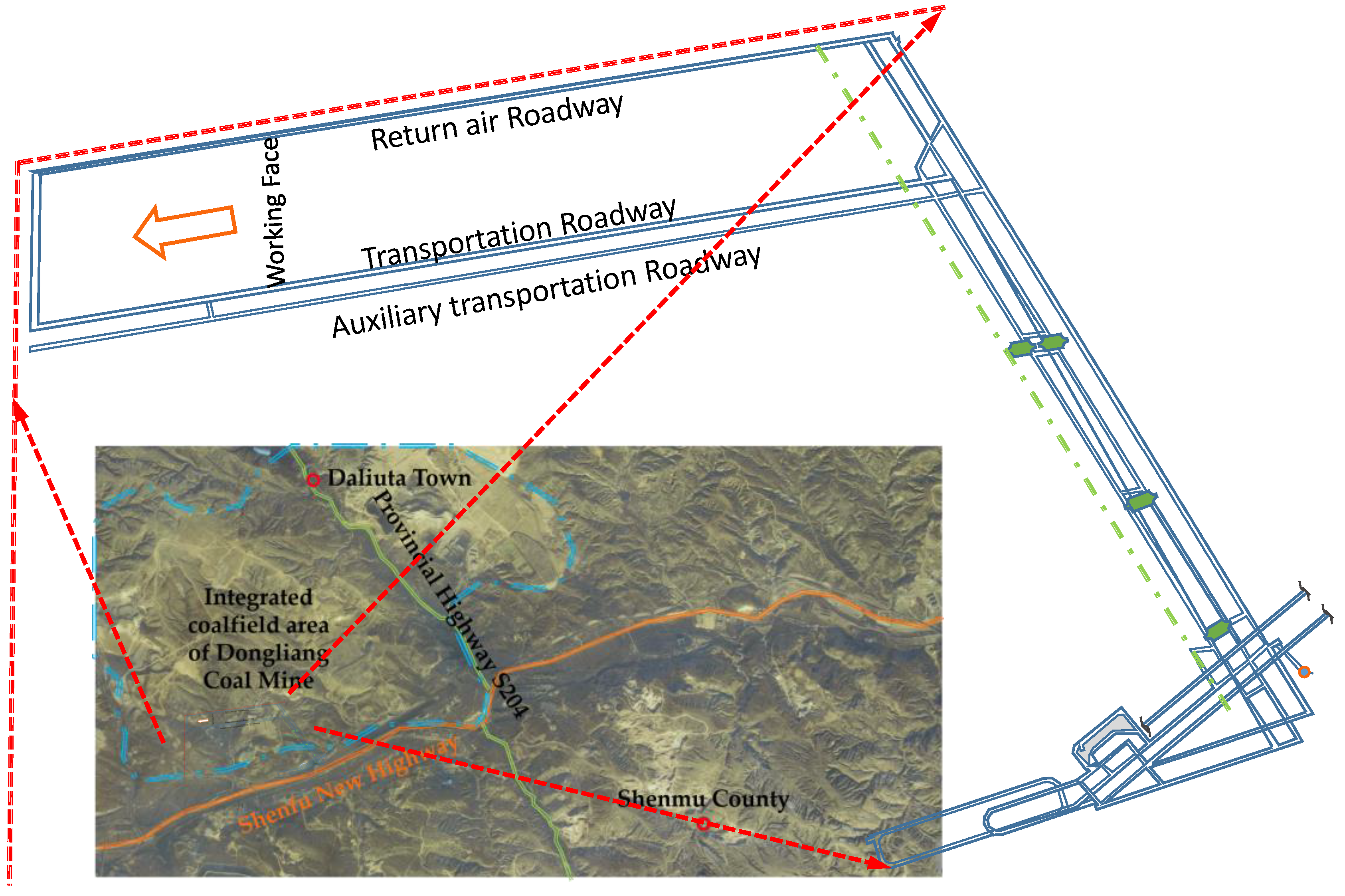
2.1. Overview of Working Face
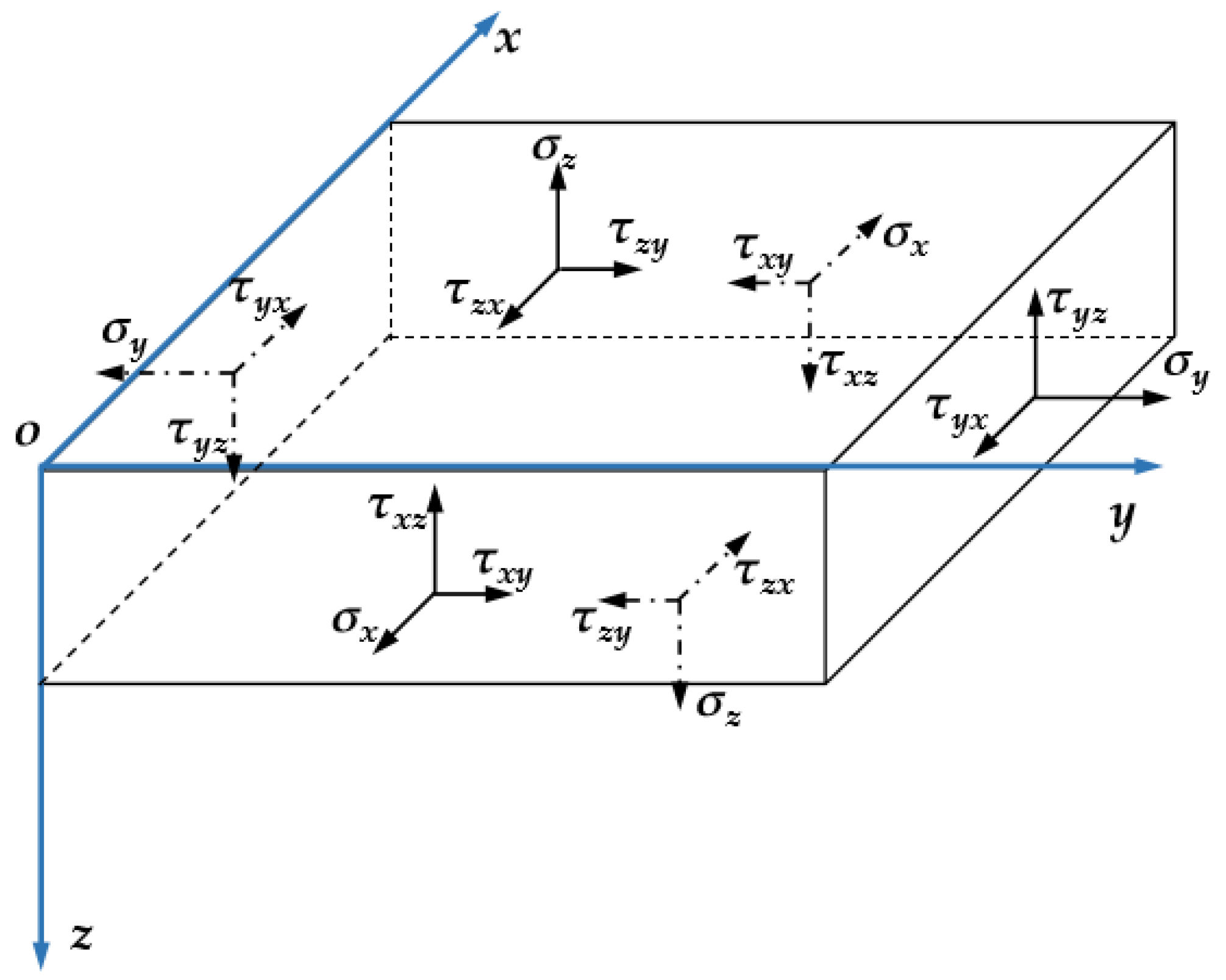
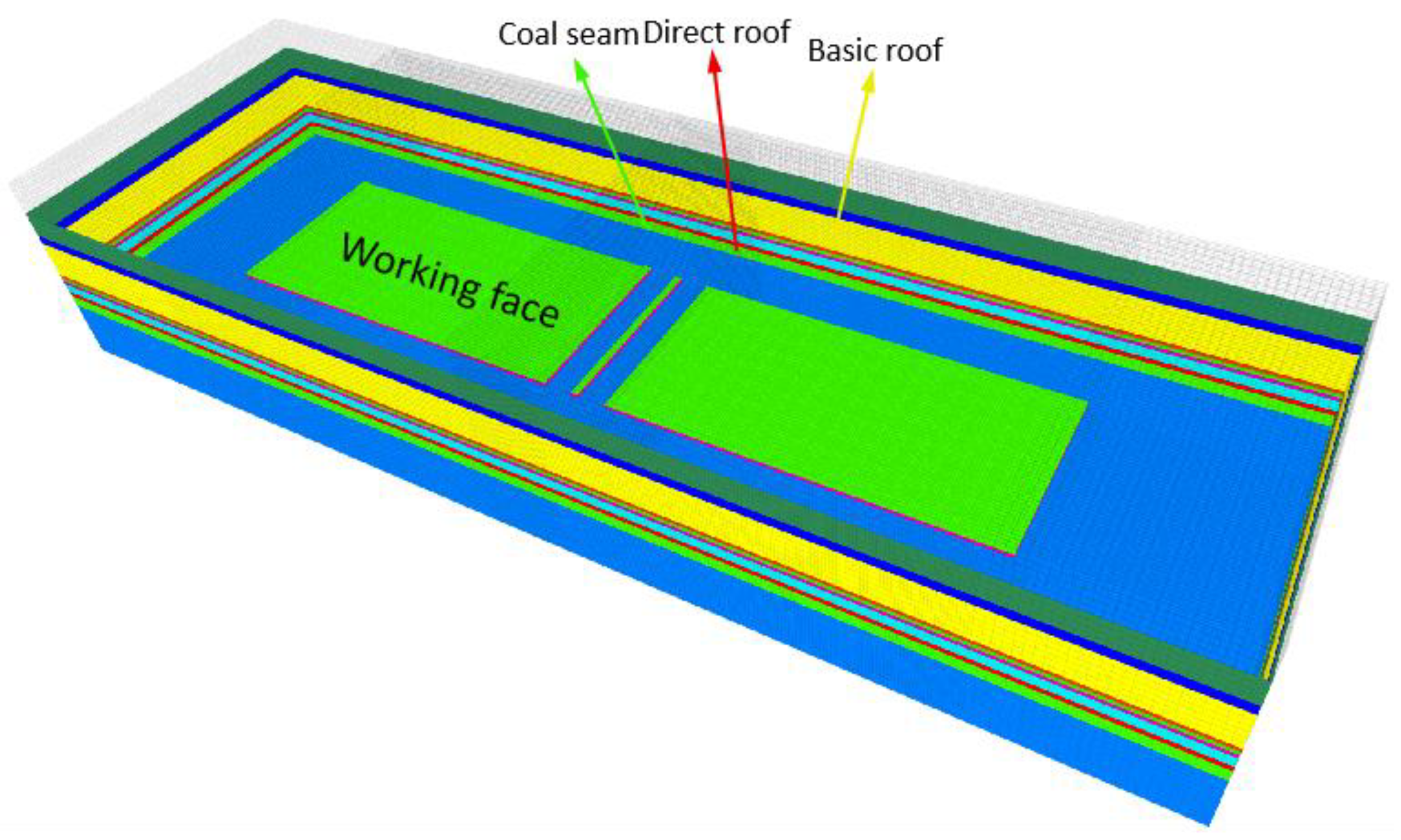
2.2. Establishment of a Mechanical Model of Overburden
3. Three Typical Characteristics of Basic Roof Movement on Direct Roofs with a Large Mining Height
3.1. Mechanical Model of a Beam Obtained by Dissecting a Medium-Thick Plate
3.2. The Boundary Conditions of the Experimental Model Are Set in the Simulation of the Excavation Results
4. Discussion and Conclusions
4.1. Discussion
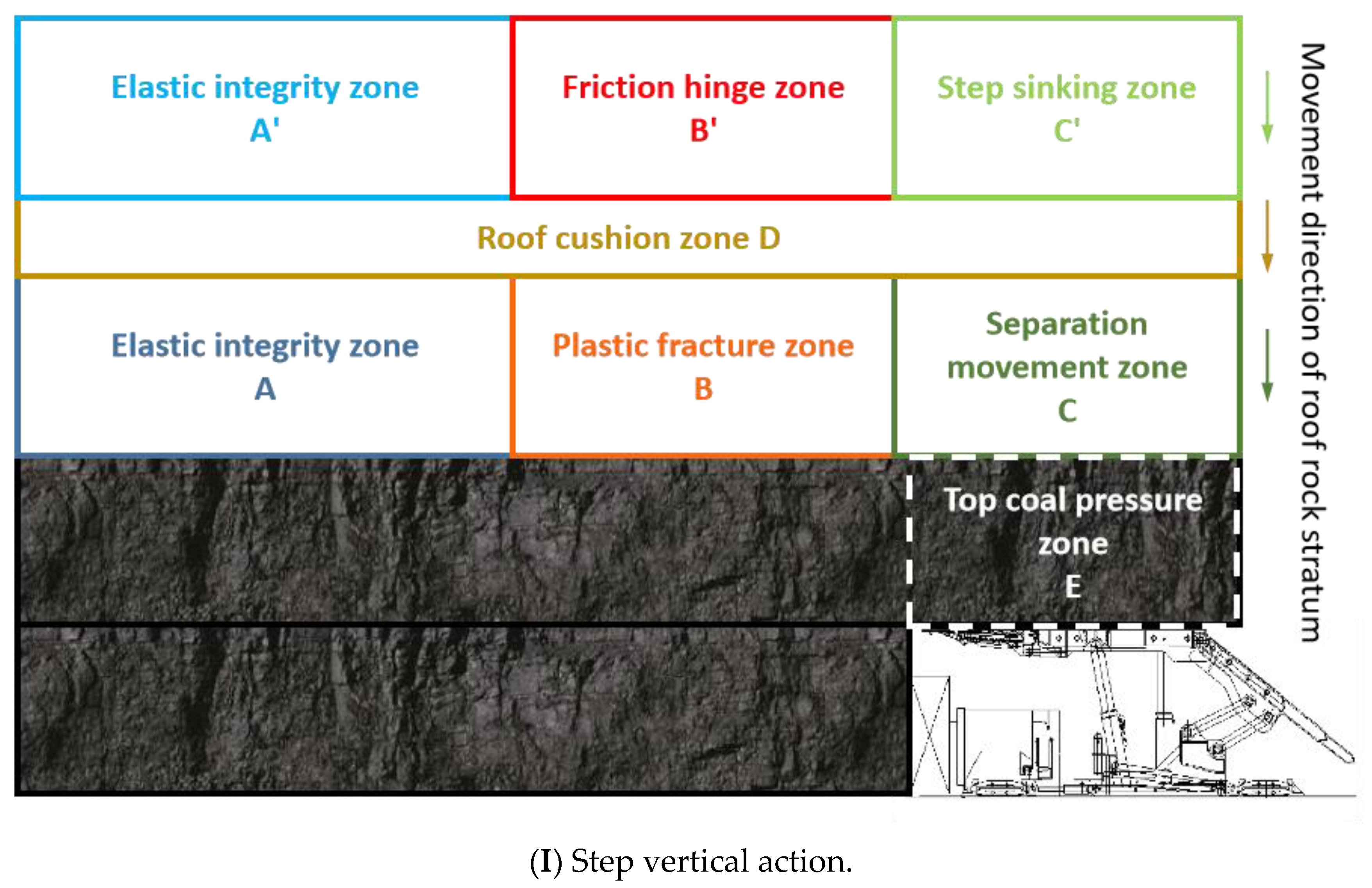
- The theoretical formula showed that when the thickness-to-span ratio of the basic roof was less than 0.5, the movement of the basic roof belonged to the long cantilever beam horizontal action mode. When the thickness-to-span ratio of the basic roof reached about 0.5, the movement of the basic roof belonged to the short cantilever beam rotation mode. When the thickness-to-span ratio of the basic roof stratum was greater than 0.5, the basic roof movement belonged to the step vertical action mode. These three different migration and fracture modes are significant for determining the state of direct roofs and top coal.
- According to the effect and recovery rate of top-coal caving, it can be judged that the bench subsidence of thick and hard basic top rock is not conducive to top-coal caving, which is somewhat different from the traditional on-site experience of weakening the support pressure by roof pre-cracking in rock pressure control. Therefore, when shortening the roof fracture step and reducing the roof pressure intensity, the thick and hard basic top rock should also be cut in the appropriate horizontal direction of the large-mining-height working face. The thickness-to-span ratio should be about 0.5 to prevent the bench vertical action from causing the top coal to accelerate toward the goaf, thereby causing a large amount of coal loss.
- Under the mining activity of the fully mechanized top-coal caving face with a large mining height, the stress state in the roof rock has a key impact on the roof cutting process. The relationship between the three-dimensional principal stresses directly determines the dominant direction of fracture development during hydraulic fracturing [20,21,22,23]. Therefore, according to the application of the theoretical formula for the ideal thickness-to-span ratio of the basic roof and the boundary conditions of the top coal obtained in this paper, the roof control parameters can be optimized. By improving the geometry of the basic roof and the way in which the rock mechanical properties act on the boundary of the top-coal roof, the purpose of improving the mine pressure and the recovery rate can be achieved.
4.2. Conclusions
- The fracture state of a basic roof with different thickness-to-span ratios has a significant influence on the recovery rate of top-coal caving.
- During coal mining, hydraulic fracturing, blasting roof breaking, ground fracturing, and other means should be used to control the thickness-to-span ratio of the basic roof at about 0.5 so as to reduce the occurrence of ground pressure and to improve the recovery rate of coal resources.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Qian, M.G.; Miao, X.X.; He, F.L. Analysis of the key blocks of the “masonry beam” structure in the stope. Acta Coal Sin. 1994, 6, 557–563. [Google Scholar]
- Miao, X.X.; Qian, M.G. The overall structure of surrounding rock in the stope and the mechanical model of masonry beams. Mine Press. Roof Manag. 1995, 3, 197. [Google Scholar]
- Qian, M.G.; Zhu, D.R.; Wang, Z.T. Fracture type of the main roof rock and its influence on the pressure on the working face. J. China Acad. Min. Technol. 1986, 2, 12–21. [Google Scholar]
- Song, Z.Q.; Liu, Y.X.; Chen, M.B.; Song, Y. Discussion on bearing pressure behavior before and after rock beam fracture and its application. J. Shandong Inst. Min. Technol. 1984, 1, 27–39. [Google Scholar] [CrossRef]
- Song, Z.Q.; Song, Y.; Jiang, Y.J.; Liu, Y.X. Methods and steps of roof control design in mining face. Coal Sci. Technol. 1989, 9, 6–63. [Google Scholar] [CrossRef]
- Bukhoino, G. Rock Pressure and Rockburst; Coal Industry Press: Beijing, China, 1985. [Google Scholar]
- Barczak, T.M.; Oyler, D.C. A Model of Shield Strata Interaction and Its Implications for Active Shield Setting Requirements Missing; US Department of the Interior, Bureau of Mines: Washington, DC, USA, 1991.
- Goodman, R.E.; Shi, G.H. Block Theory and Its Application to Rock Engineering; Prentice-Hall: Hoboken, NJ, USA, 1985. [Google Scholar]
- Xu, X.D. Study on the Mechanism of Strong Ground Pressure and Pressure Relief Control along the Goaf in Caojiatan Coal Mine’s Extra Thick Coal Seam; Jilin University: Changchun, China, 2022. [Google Scholar] [CrossRef]
- Chen, Y. Study on the Mining Floor Deformation and Failure Depth of Extra Thick Coal Seam in Datong Mining Area; China University of Mining and Technology: Beijing, China, 2021. [Google Scholar] [CrossRef]
- Dong, Z.Y.; Wang, S.M. Analysis of the impact of coal mining on groundwater resources in Yuxi River Basin in Northern Shaanxi—Taking hanglaiwan coal mining area as an example. Resour. Environ. Arid Areas 2017, 31, 185–190. [Google Scholar] [CrossRef]
- Li, H.; Tang, S.B.; Ma, L.Q.; Bai, H.B.; Kang, Z.Q.; Wu, P.F.; Miao, X.C. Study on continuous discrete coupling simulation of progressive failure of mining overburden. J. Rock Mech. Eng. 2022, 1–12. [Google Scholar] [CrossRef]
- Li, H.; Bai, H.B.; Wu, J.J.; Li, Z.Y.; Meng, Q.B.; Guo, J.Q.; Zhu, D.F.; Xiao, M.D.-P. Random damage constitutive model and its application in preventing water inrush of collapse columns. Geotech. Mech. 2018, 39, 4577–4587. [Google Scholar] [CrossRef]
- Zuo, J.P.; Yu, M.L.; Sun, Y.J.; Wu, G.S. Analysis of fracture mode transformation mechanism and mechanical model of rock layers with different thicknesses. Acta Coal Sin. 2022, 1–18. [Google Scholar] [CrossRef]
- Luo, J.; Yan, S.; Yang, T.; Mu, H.; Wei, W.; Shen, Y.; Mu, H. Mechanism of Hydraulic Fracturing Cutting Hard Basic Roof to Prevent Rockburst; Hindawi Limited: London, UK, 2021. [Google Scholar]
- Hubbert, M.K.; Willis, D. Mechanics of hydraulic fracturing. Trans. AIME 1972, 210, 153–168. [Google Scholar] [CrossRef]
- Zhang, P.; Liang, X.; Xian, C.; Liu, B.; Wang, W.; Zhang, C. Geomechanics simulation of stress regime change in hydraulic fracturing: A case study. Geomech. Geophys. Geo-Energy Geo-Resour. 2022, 8, 1–18. [Google Scholar] [CrossRef]
- Murthy, M.V.V.S.; Renji, K.; Gopalakrishnan, S. Multi-transform based spectral element to include first order shear deformation in plates. Int. J. Mech. Sci. 2015, 96–97, 110–120. [Google Scholar] [CrossRef]
- Guy, R.; Kent, M.; Russell, F. An assessment of coal pillar system stability criteria based on a mechanistic evaluation of the interaction between coal pillars and the overburden. Int. J. Min. Sci. Technol. 2017, 27, 9–15. [Google Scholar]
- Lv, K.; He, F.; Xu, X.; Zhai, W.; Qin, B. Irregular load—Fracture characteristics and stability analysis of elastic foundation basic roof structure. J. Rock Mech. Eng. 2022, 1–18. [Google Scholar] [CrossRef]
- Xu, X.; He, F.; Lv, K.; Wang, D.; Li, l.; Zhai, W. Study on reasonable roof cutting parameters of thick hard roof cutting and retaining roadway. J. Coal Ind. 2022, 1–12. [Google Scholar] [CrossRef]
- Li, Y.; Ren, Y.; Wang, N.; Jin, N.; Ou, X.; Luo, J.; Mei, C. Morphology and evolution characteristics of caving roof in goaf. J. Coal Ind. 2021, 46, 3771–3780. [Google Scholar] [CrossRef]
- Chen, D.; Wu, Y.; Xie, S.; Guo, F.; He, F.; Liu, R. Study on the initial fracture of basic roof slab at the boundary of long side goaf and elastic-plastic softening foundation. J. Coal Ind. 2022, 47, 1473–1489. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, L.; Zhang, X.; Luo, J.; Hu, B. Theoretical Analysis of the Movement Law of Top Coal and Overburden in a Fully Mechanized Top-Coal Caving Face with a Large Mining Height. Processes 2022, 10, 2596. https://doi.org/10.3390/pr10122596
Li L, Zhang X, Luo J, Hu B. Theoretical Analysis of the Movement Law of Top Coal and Overburden in a Fully Mechanized Top-Coal Caving Face with a Large Mining Height. Processes. 2022; 10(12):2596. https://doi.org/10.3390/pr10122596
Chicago/Turabian StyleLi, Li, Xiao Zhang, Jianqiao Luo, and Bin Hu. 2022. "Theoretical Analysis of the Movement Law of Top Coal and Overburden in a Fully Mechanized Top-Coal Caving Face with a Large Mining Height" Processes 10, no. 12: 2596. https://doi.org/10.3390/pr10122596
APA StyleLi, L., Zhang, X., Luo, J., & Hu, B. (2022). Theoretical Analysis of the Movement Law of Top Coal and Overburden in a Fully Mechanized Top-Coal Caving Face with a Large Mining Height. Processes, 10(12), 2596. https://doi.org/10.3390/pr10122596




