Calibration and Testing of Discrete Element Simulation Parameters for Urea Particles
Abstract
:1. Introduction
2. Materials and Methods
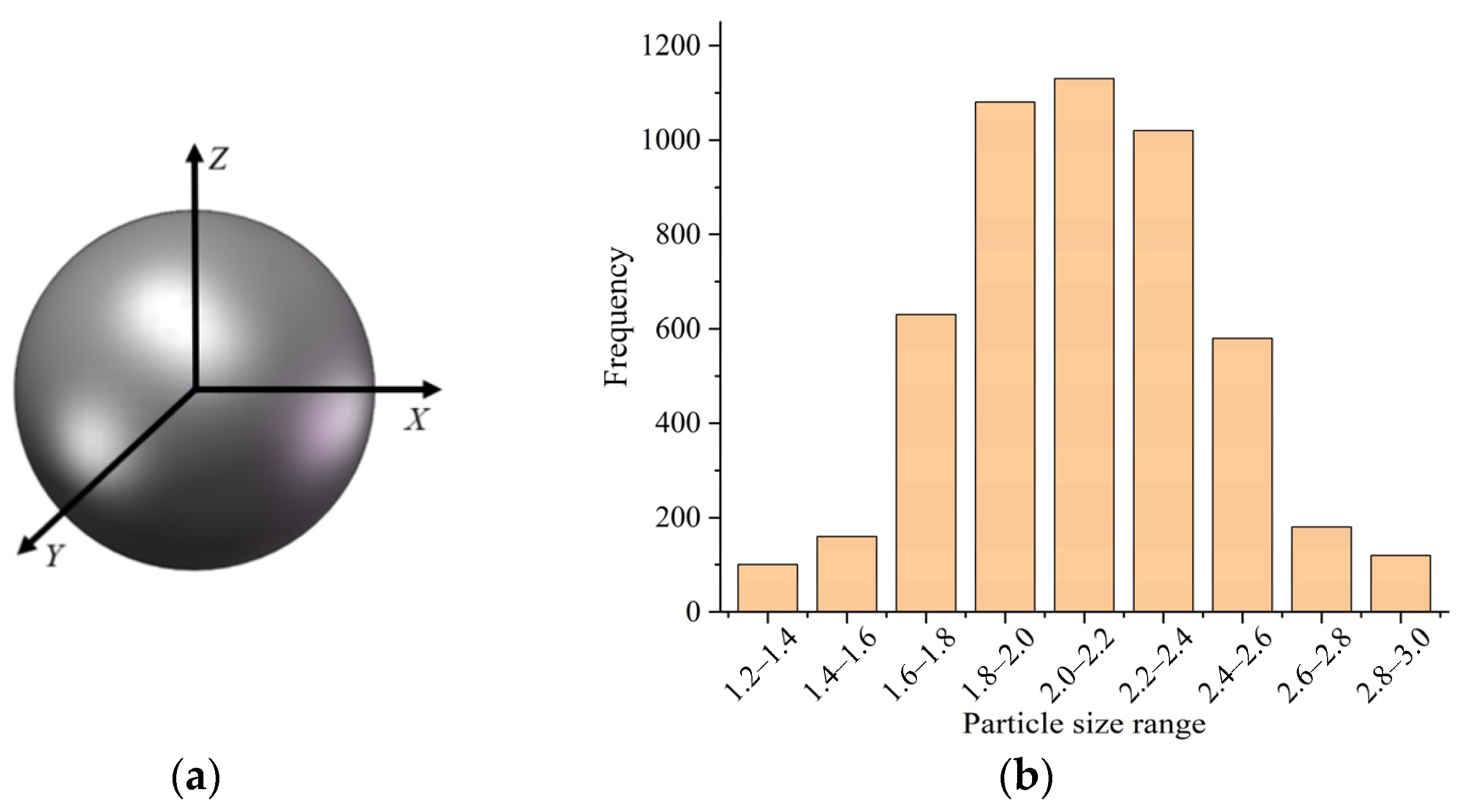
2.1. Basic Physical Properties and Geometric Model of Urea ParticlesSubsection
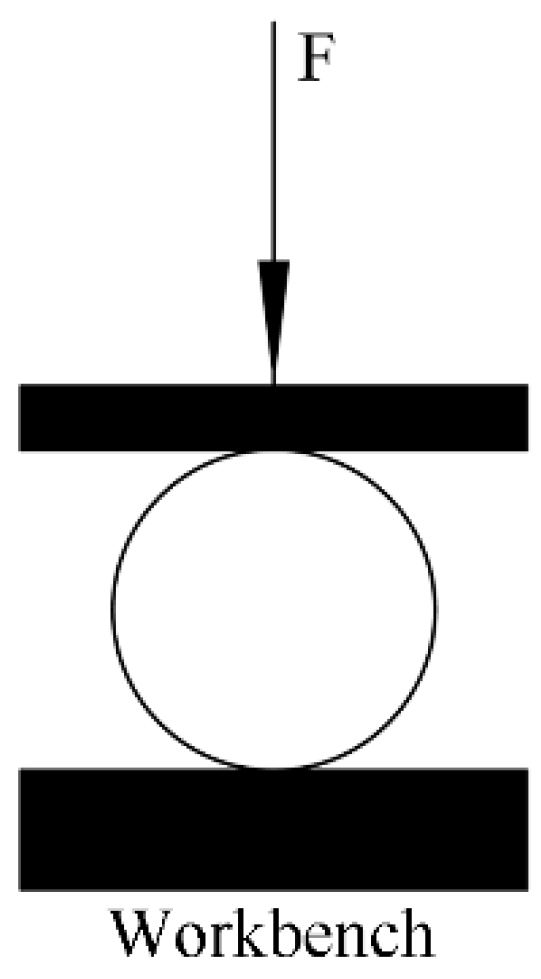
2.2. Poisson’s Ratio and Modulus of Elasticity
2.3. Restitution Coefficient
2.4. Friction Coefficient
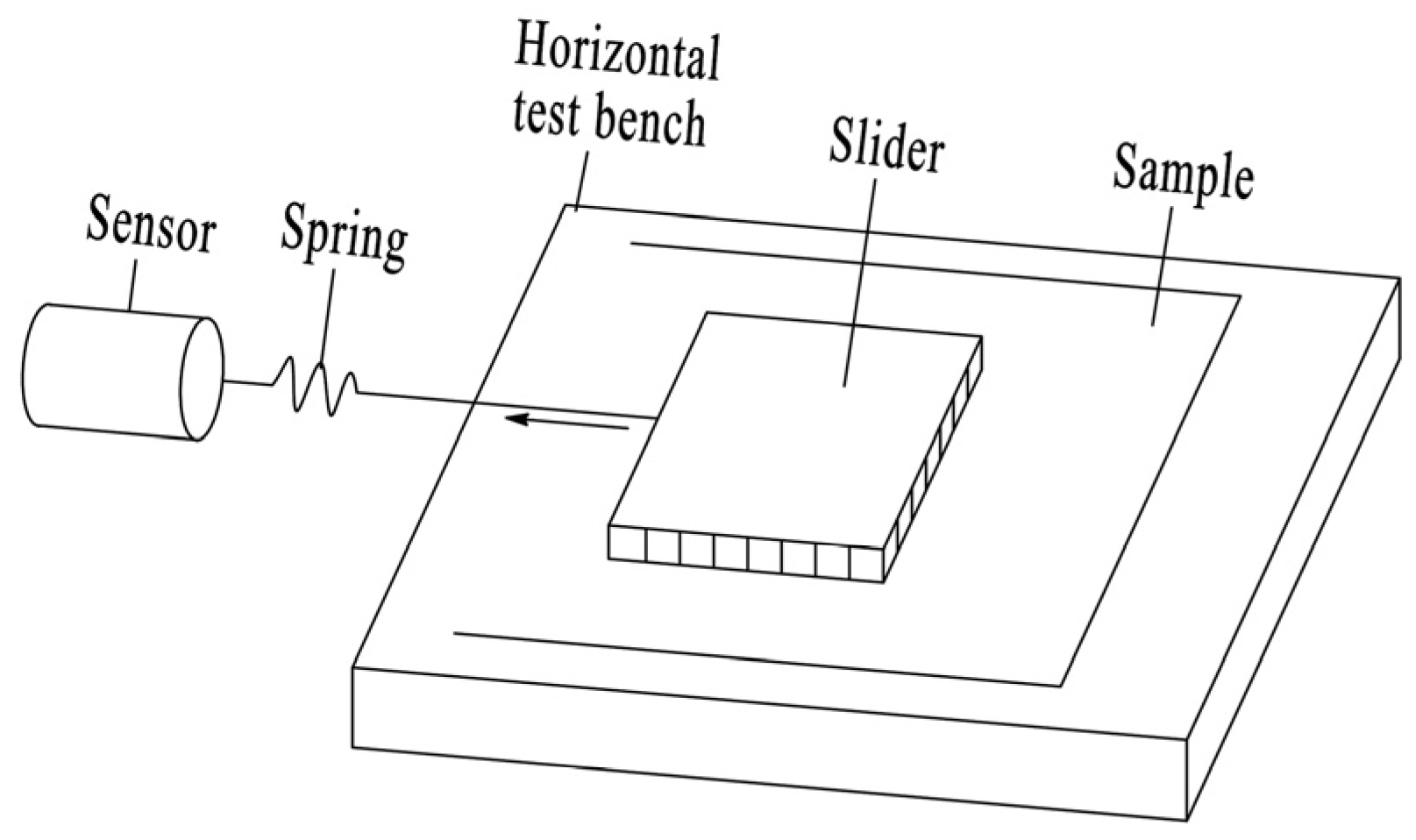
2.4.1. Static Friction Coefficient
2.4.2. Rolling Friction Coefficient
2.5. Natural Repose Angle Simulation Calibration
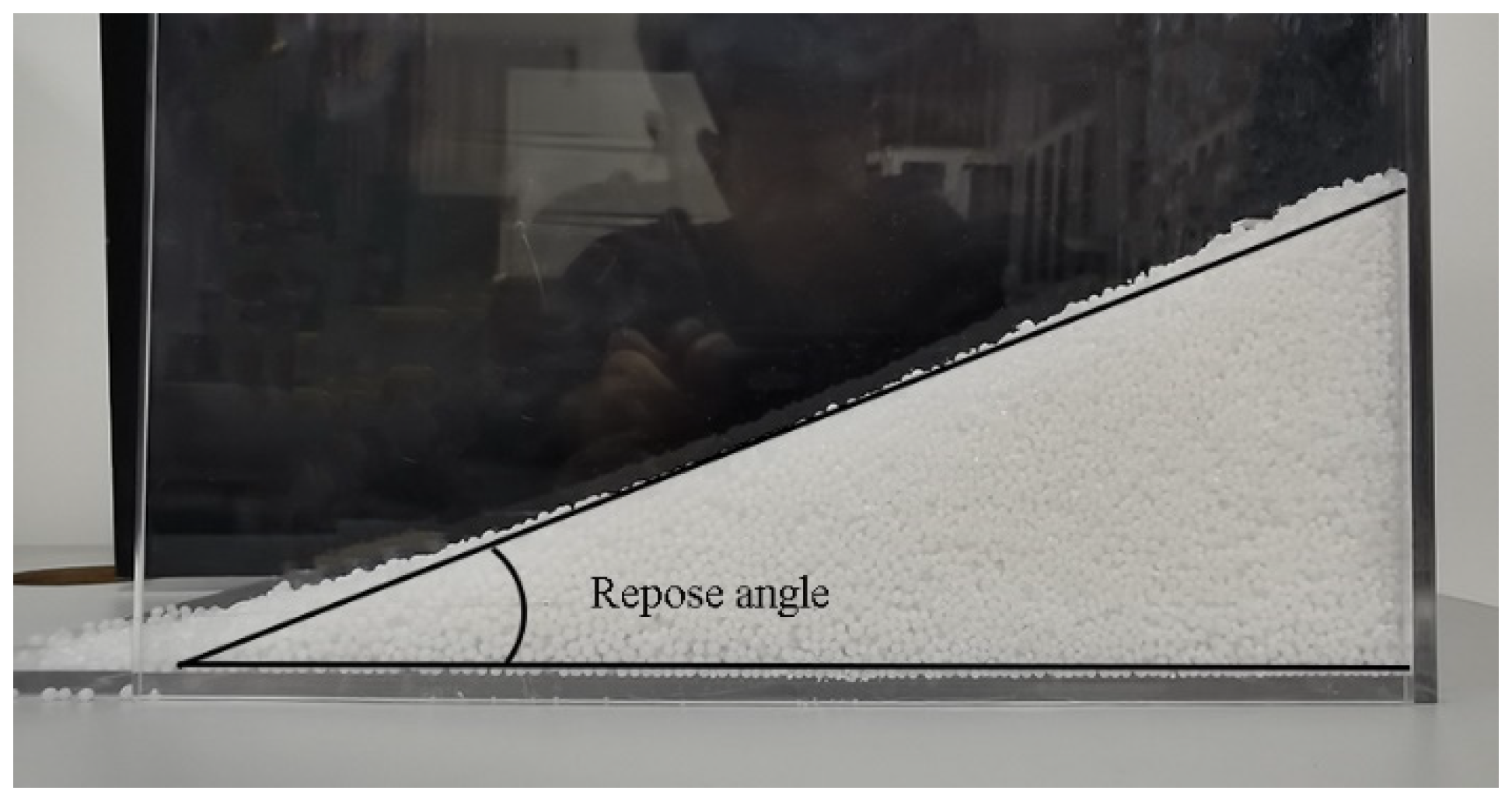
2.5.1. Physical Repose Angle Determination
2.5.2. Establishment of the Urea Particle Simulation Model and Parameter Setting
2.5.3. Urea Particle Friction Coefficient Calibration
3. Results
3.1. Repose Angle Variation Trend Analysis
3.2. Repose Angle Regression Analysis
4. Test Verification
4.1. Repose Angle Test Verification
4.2. Bulk Density Verification
4.3. Fertilization Simulation Test and Verification
4.3.1. Simulation Parameter Setting
4.3.2. Model Simulation of the Fertilizer Discharge Process and Results
4.3.3. Bench Test Verification
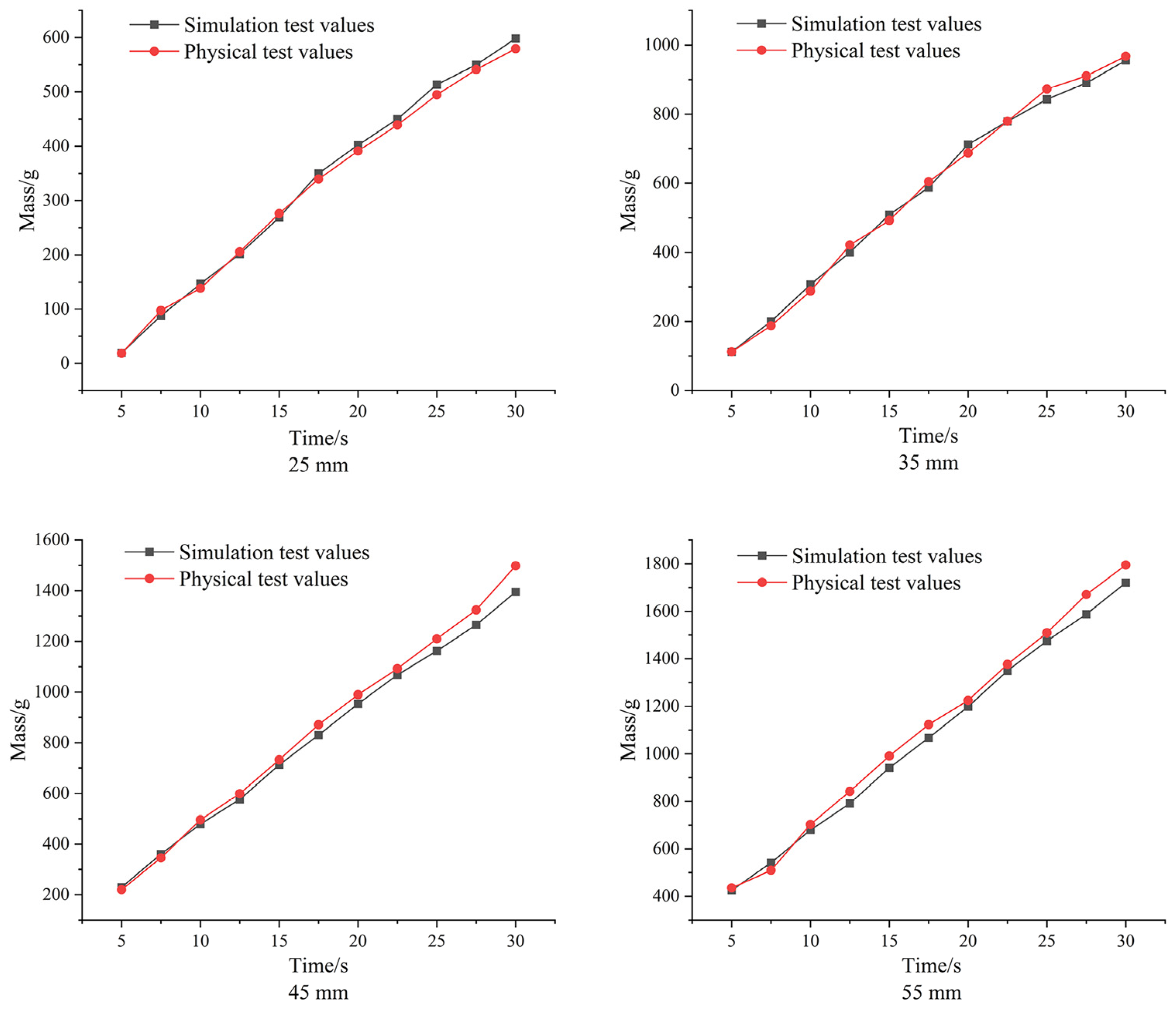
4.3.4. Test Results and Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, L.; Yan, C.; Guo, Q.; Zhang, J.; Ruiz-Menjivar, J. The impact of agricultural chemical inputs on environment: Global evidence from informetrics analysis and visualization. Int. J. Low-Carbon Technol. 2018, 13, 338–352. [Google Scholar] [CrossRef] [Green Version]
- Weeks, J.J., Jr.; Hettiarachchi, G.M. A review of the latest in phosphorus fertilizer technology: Possibilities and pragmatism. J. Environ. Qual. 2019, 48, 1300–1313. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, C.; Feng, J.; Chen, C.; Wang, J.; Liu, C.; Lin, Y.; Kang, J. Analysis of the current situation of cotton industry and mechanization development in China. China J. Agric. Chem. 2021, 42, 215–221. [Google Scholar]
- Bangura, K.; Gong, H.; Deng, R.; Tao, M.; Liu, C.; Cai, Y.; Liao, K.; Liu, J.; Qi, L. Simulation analysis of fertilizer discharge process using the Discrete Element Method (DEM). PLoS ONE 2020, 15, e0235872. [Google Scholar] [CrossRef]
- Yang, H.; Zhang, L.; Dong, W.; Fang, Y.; Hu, D.; Yang, D. Analysis and experiment of discharging device of bivariate fertilizer applicator based on discrete element method. Mech. Des. Res. 2019, 35, 179–183. [Google Scholar]
- Chen, H.; Zheng, J.; Lu, S.; Zeng, S.; Wei, S. Design and experiment of vertical pneumatic fertilization system with spiral Geneva mechanism. Int. J. Agric. Biol. Eng. 2021, 14, 135–144. [Google Scholar] [CrossRef]
- He, Y.; Li, C.; Zhao, X.; Gao, Y.; Li, S.; Wang, S. Simulation analysis of the fertilizer ejecting device of corn fertilizer applicator based on EDEM. J. Phys. Conf. Ser. 2020, 1633, 012061. [Google Scholar] [CrossRef]
- Ding, S.; Bai, L.; Yao, Y.; Yue, B.; Fu, Z.; Zheng, Z.; Huang, Y. Discrete element modelling (DEM) of fertilizer dual-banding with adjustable rates. Comput. Electron. Agric. 2018, 152, 32–39. [Google Scholar] [CrossRef]
- Dun, G.; Gao, Z.; Liu, Y.; Ji, W.; Mao, N.; Wu, X.; Liu, W. Optimization design of fertilizer apparatus owned arc gears based on discrete element method. Int. J. Agric. Biol. Eng. 2021, 14, 97–105. [Google Scholar] [CrossRef]
- Xue, Z.; Zhao, L.; Wang, F.; Wang, S.; Wang, G.; Pan, R. Performance simulation test of the spiral fertilizer distributor based on discrete element method. J. Hunan Agric. Univ. 2019, 45, 548–553. [Google Scholar]
- Sun, C.; Cao, L.; Shi, Z.; Chen, X.; Huang, S. Analysis on fertilizer performance of fertilizer machine based on discrete element method. Chin. J. Agric. Chem. 2019, 40, 44–50, 127. [Google Scholar]
- Dun, G.; Yu, C.; Yang, Y.; Chen, H.; Ji, W.; Ye, J. Discharging characteristic test of outer–groove wheel fertilizer and parameter optimization of fertilizer tongue by discrete element simulation. J. Hunan Agric. Univ. 2018, 44, 661–665. [Google Scholar]
- Cao, Y. Simulation Analysis and Experimental Research of Spiral Fertilizer Discharger Based on Discrete Element Method; Jilin Agricultural University: Changchun, China, 2017. [Google Scholar]
- Wang, B. Simulation and Parameter Optimization of the Working Process of External Slotted Wheel Fertilizer Discharger Based on Discrete Element Method; Northwest Agriculture and Forestry University: Xianyang, China, 2017. [Google Scholar]
- Zhang, H.; Li, Y.; Wang, J.; Zhang, K.; Liu, S.; Wang, Z.; Chu, G.; Quan, Z. Optimization Research of Fertilizer Guiding Mechanism Based on the Discrete Element Method. Food Nutr. Sci. Int. J. 2019, 3, 18–29. [Google Scholar]
- Xie, C.; Yang, J.; Wang, B.; Zhuo, P.; Li, C.; Wang, L. Parameter calibration for the discrete element simulation model of commercial organic fertilizer. Int. Agrophys. 2021, 35, 107–117. [Google Scholar] [CrossRef]
- Hlosta, J.; Jezerská, L.; Rozbroj, J.; Žurovec, D.; Nečas, J.; Zegzulka, J. DEM investigation of the influence of particulate properties and operating conditions on the mixing process in rotary drums: Part 1—Determination of the DEM parameters and calibration process. Processes 2020, 8, 222. [Google Scholar] [CrossRef] [Green Version]
- Blake, O.O.; Faulkner, D.R.; Tatham, D.J. The role of fractures, effective pressure and loading on the difference between the static and dynamic Poisson’s ratio and Young’s modulus of Westerly granite. Int. J. Rock Mech. Min. Sci. 2019, 116, 87–98. [Google Scholar] [CrossRef]
- American Society of Agricultural and Biological Engineers. ASAE S368.4 DEC2000 (R2017) Compression Test of Food Materials of Convex Shape; American Society of Agricultural and Biological Engineers: Michigan, MI, USA, 2017. [Google Scholar]
- Carrillo, J.; Ramirez, J.; Lizarazo-Marriaga, J. Modulus of elasticity and Poisson’s ratio of fiber-reinforced concrete in Colombia from ultrasonic pulse velocities. J. Build. Eng. 2019, 23, 18–26. [Google Scholar] [CrossRef]
- Li, T.; Chen, Y.; Hu, X.; Li, Y.; Wang, L. Exploiting negative Poisson’s ratio to design 3D-printed composites with enhanced mechanical properties. Mater. Des. 2018, 142, 247–258. [Google Scholar] [CrossRef]
- Dong, M.; Mei, Y.; Li, X.; Shang, Y.; Li, S. Experimental measurement of the normal coefficient of restitution of micro-particles impacting on plate surface in different humidity. Powder Technol. 2018, 335, 250–257. [Google Scholar] [CrossRef]
- Feng, B.; Sun, W.; Shi, L.; Sun, B.; Zhang, T.; Wu, J. Determination of restitution coefficient of potato tubers collision in harvest and analysis of its influence factors. Trans. Chin. Soc. Agric. Eng. 2017, 33, 50–57. [Google Scholar]
- Hlosta, J.; Žurovec, D.; Rozbroj, J.; Ramírez-Gómez, Á.; Nečas, J.; Zegzulka, J. Experimental determination of particle–particle restitution coefficient via double pen-dulum method. Chem. Eng. Res. Des. 2018, 135, 222–233. [Google Scholar] [CrossRef]
- Ye, Y.; Zeng, Y.; Thoeni, K.; Giacomini, A. An experimental and theoretical study of the normal coefficient of restitution for marble spheres. Rock Mech. Rock Eng. 2019, 52, 1705–1722. [Google Scholar] [CrossRef]
- Jiang, Z.; Du, J.; Rieck, C.; Bück, A.; Tsotsas, E. PTV experiments and DEM simulations of the coefficient of restitution for irregular particles impacting on horizontal substrates. Powder Technol. 2020, 360, 352–365. [Google Scholar] [CrossRef]
- Wang, L.; Li, R.; Wu, B.; Wu, Z.; Ding, Z. Determination of the coefficient of rolling friction of an irregularly shaped maize particle group using physical experiment and simulations. Particuology 2018, 38, 185–195. [Google Scholar] [CrossRef]
- Ciornei, M.C.; Alaci, S.; Ciornei, F.C.; Romanu, I.C. A method for the determination of the coefficient of rolling friction using cycloidal pendulum. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2017; Volume 227, p. 012027. [Google Scholar]
- Shi, L.; Yang, X.; Zhao, W.; Sun, W.; Wang, G.; Sun, B. Investigation of interaction effect between static and rolling friction of corn kernels on repose formation by DEM. Int. J. Agric. Biol. Eng. 2021, 14, 238–246. [Google Scholar] [CrossRef]
- Ferreira, I.S.B.; Peruchi, R.S.; Fernandes, N.J.; Junior, P.R. Measurement system analysis in angle of repose of fertilizers with distinct granulometries. Measurement 2021, 170, 108681. [Google Scholar] [CrossRef]
- Roessler, T.; Katterfeld, A. DEM parameter calibration of cohesive bulk materials using a simple angle of repose test. Particuology 2019, 45, 105–115. [Google Scholar] [CrossRef]
- Wen, X.; Jia, H.; Zhang, S.; Yuan, H.; Wang, G.; Chen, T. Test of Suspension Velocity of Granular Fertilizer Based on EDEM-Fluent Coupling. J. Agric. Mach. 2020, 51, 69–77. [Google Scholar]
- Chen, H.; Zhao, S.; Zhou, X. DEM investigation of angle of repose for super-ellipsoidal particles. Particuology 2020, 50, 53–66. [Google Scholar] [CrossRef]
- Wu, M.; Cong, J.; Yan, Q.; Zhu, T.; Peng, X.; Wang, Y. Calibration and experiments for discrete element simulation parameters of peanut seed particles. J. Agric. Eng. 2020, 36, 30–38. [Google Scholar]



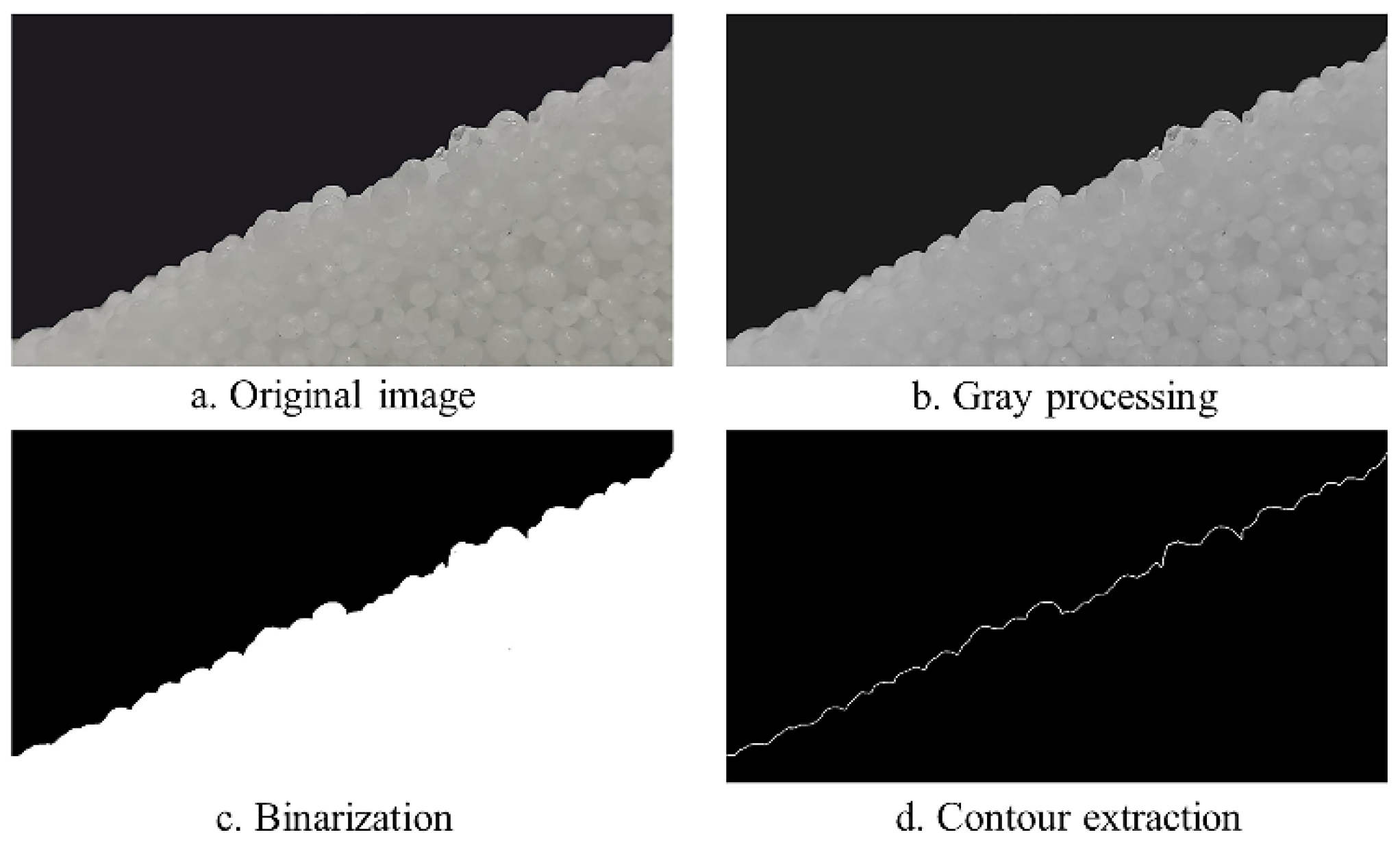



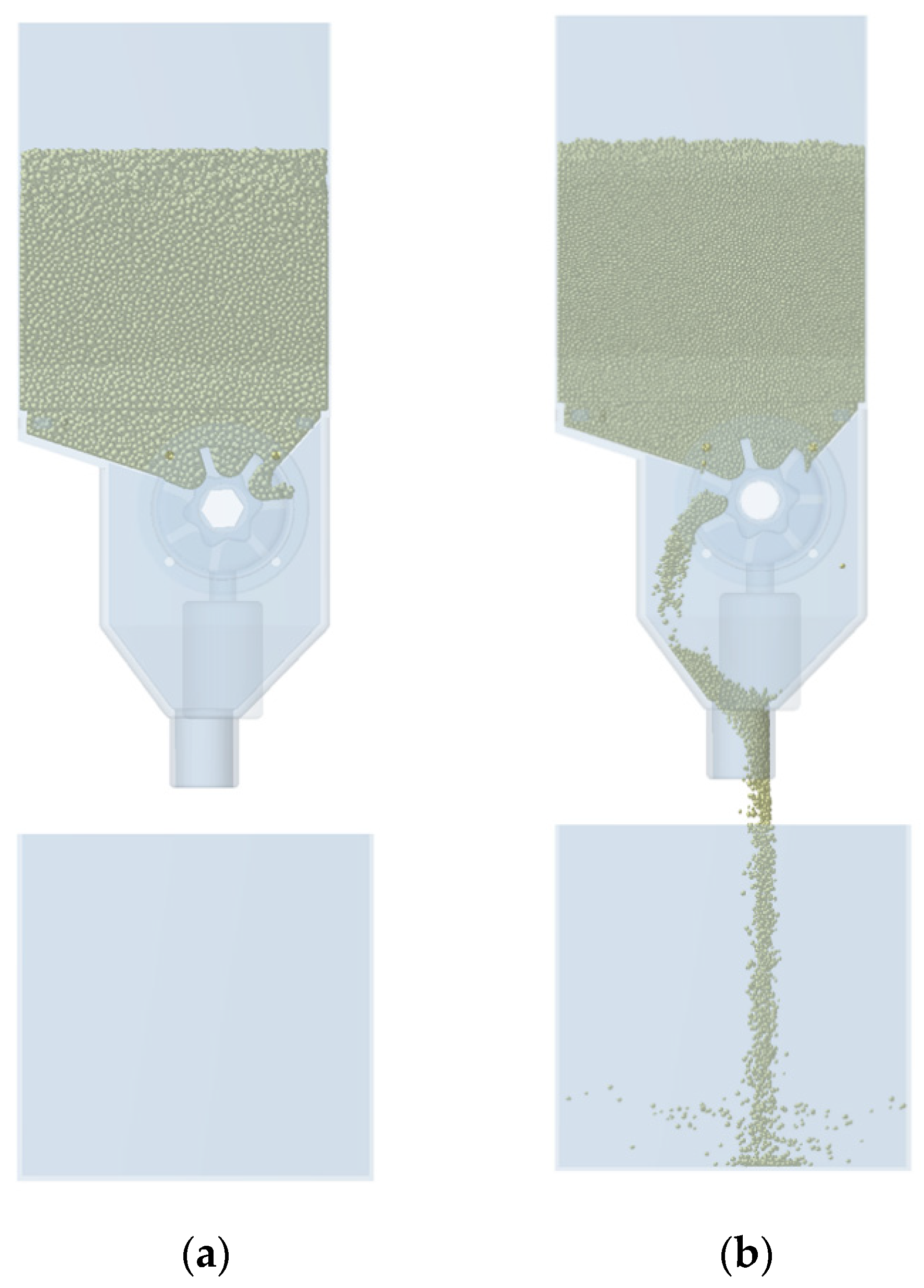

| Parameter | Value |
|---|---|
| Particle size/mm | 1.2–3.0 |
| Thousand particles quality/kg | 3.76 × 10−3 |
| Density/(kg·m−3) | 1341 |
| Moisture content/% | 0.38 |
| Bulk density/(kg·m−3) | 803.7 |
| Poisson’s ratio | 0.3 |
| Modulus of elasticity/Pa | 6.72 × 107 |
| θ | cos θ | C1 | C2 | K |
|---|---|---|---|---|
| 50 | 0.6428 | 1.754 | 0.641 | 1.198 |
| 55 | 0.5736 | 1.611 | 0.678 | 1.235 |
| 60 | 0.5000 | 1.468 | 0.717 | 1.267 |
| 65 | 0.4226 | 1.378 | 0.759 | 1.293 |
| 70 | 0.3420 | 1.284 | 0.802 | 1.314 |
| 75 | 0.2588 | 1.202 | 0.846 | 1.331 |
| 80 | 0.1736 | 1.128 | 0.893 | 1.342 |
| 85 | 0.0872 | 1.061 | 0.944 | 1.349 |
| 90 | 0.0 | 1.000 | 1.000 | 1.351 |
| Number | Interparticle Static Friction Coefficient | Interparticle Rolling Friction Coefficient |
|---|---|---|
| 1 | 0.18 | 0.04 |
| 2 | 0.26 | 0.09 |
| 3 | 0.34 | 0.14 |
| Interparticle Static Friction Coefficient | Interparticle Rolling Friction Coefficient | Simulated Repose Angle (°) |
|---|---|---|
| 0.18 | 0.04 | 15.47 |
| 0.09 | 17.79 | |
| 0.14 | 18.83 | |
| 0.26 | 0.04 | 19.04 |
| 0.09 | 21.72 | |
| 0.14 | 23.48 | |
| 0.34 | 0.04 | 19.82 |
| 0.09 | 23.46 | |
| 0.14 | 27.02 | |
| 0.04 | 19.82 |
| Parameter | Value |
|---|---|
| Poisson’s ratio of rigid PVC | 0.32 |
| Modulus of elasticity of rigid PVC (Pa) | 4.22 × 109 |
| Density of rigid PVC (kg·m−3) | 1418 |
| Poisson’s ratio of urea particles | 0.3 |
| Modulus of elasticity of urea particles (Pa) | 6.72 × 107 |
| Density of urea particles (kg·m−3) | 1341 |
| Restitution coefficient between urea particles and PVC | 0.35 |
| Static friction coefficient between urea particles and PVC | 0.32 |
| Rolling friction coefficient between urea particles and PVC | 0.04 |
| Restitution coefficient between urea particles and particles | 0.26 |
| Static friction coefficient between urea particles and particles | 0.27 |
| Rolling friction coefficient between urea particles and particles | 0.11 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bu, H.; Yu, S.; Dong, W.; Wang, Y.; Zhang, L.; Xia, Y. Calibration and Testing of Discrete Element Simulation Parameters for Urea Particles. Processes 2022, 10, 511. https://doi.org/10.3390/pr10030511
Bu H, Yu S, Dong W, Wang Y, Zhang L, Xia Y. Calibration and Testing of Discrete Element Simulation Parameters for Urea Particles. Processes. 2022; 10(3):511. https://doi.org/10.3390/pr10030511
Chicago/Turabian StyleBu, Haoran, Siyao Yu, Wancheng Dong, Yuqi Wang, Lixin Zhang, and Yuanqing Xia. 2022. "Calibration and Testing of Discrete Element Simulation Parameters for Urea Particles" Processes 10, no. 3: 511. https://doi.org/10.3390/pr10030511
APA StyleBu, H., Yu, S., Dong, W., Wang, Y., Zhang, L., & Xia, Y. (2022). Calibration and Testing of Discrete Element Simulation Parameters for Urea Particles. Processes, 10(3), 511. https://doi.org/10.3390/pr10030511






