Fast Finite-Time Stability and Its Application in Adaptive Control of High-Order Stochastic Nonlinear Systems
Abstract
:1. Introduction
- 1.
- Fast finite-time adaptive control strategy of deterministic systems is extended to stochastic cases, which provides a new idea for the FTS of HOSNSs, and expands its application scope in practical engineering successfully.
- 2.
- By choosing an appropriate Lyapunov function, an adaptive state feedback controller is constructed accordingly to ensure that equilibrium at the origin of the closed loop systems is fast finite-time stable in probability.
- 3.
- Compared with previous studies, it is shown that the fast finite-time adaptive control strategy not only improves the control speed significantly, but also reduces the settling time effectively.
2. Preliminaries
- 1.
- Finite-time attractiveness in probability: The stochastic settling time with initial value is finite almost surely; in other words, ;
- 2.
- Stability in probability: For and , which means that there exists a such that , whenever .
3. Design Procedures
4. Main Results
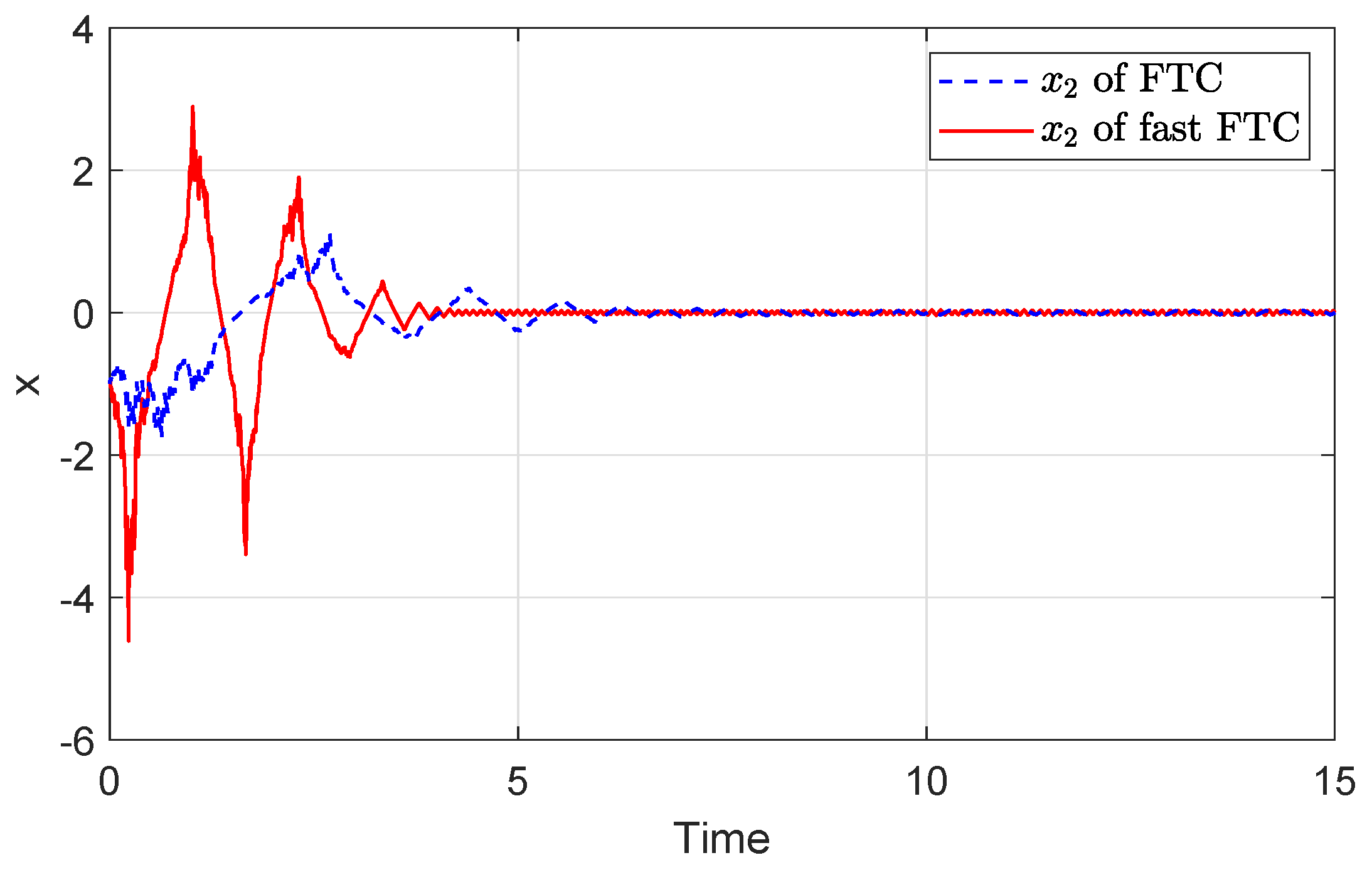
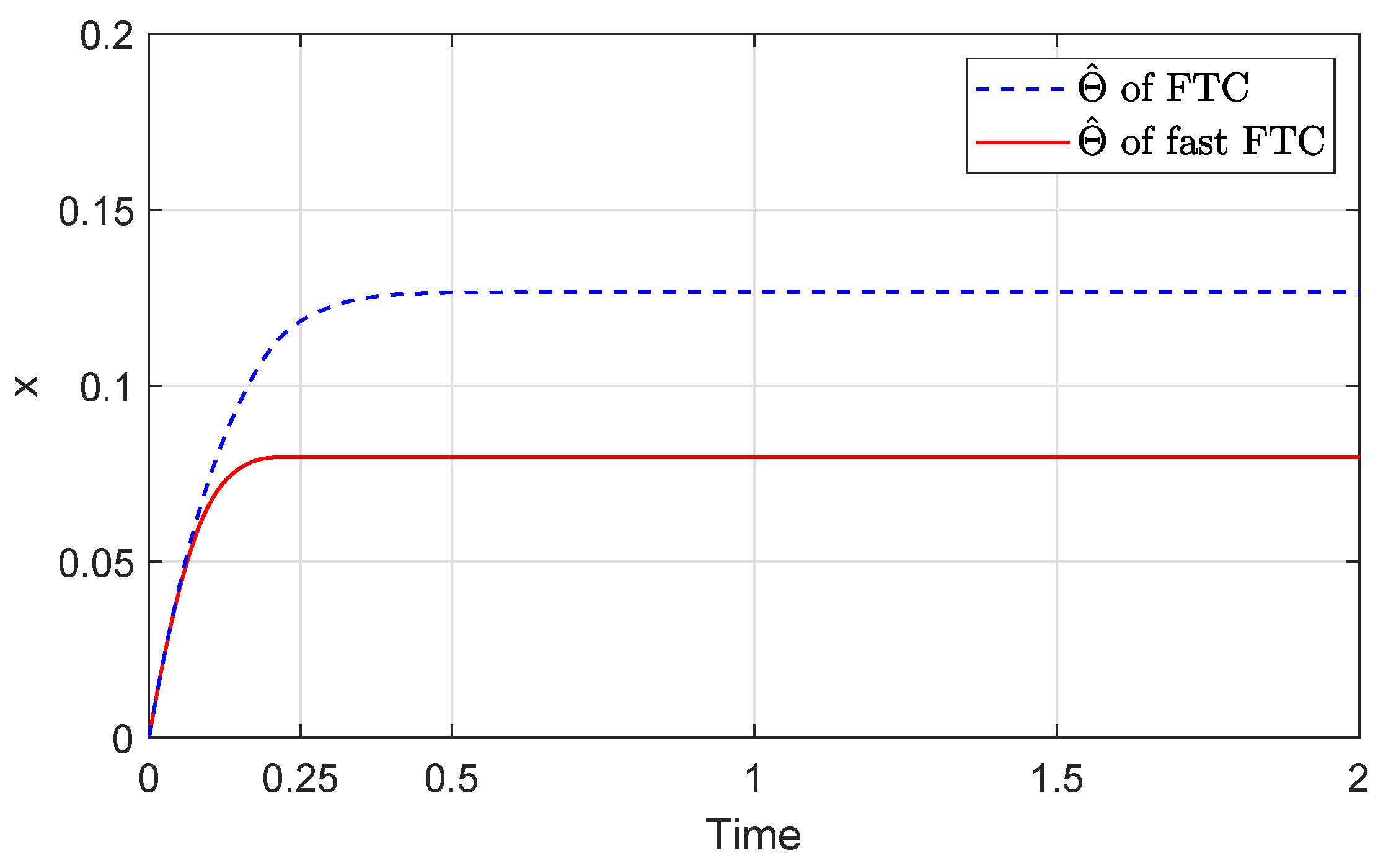
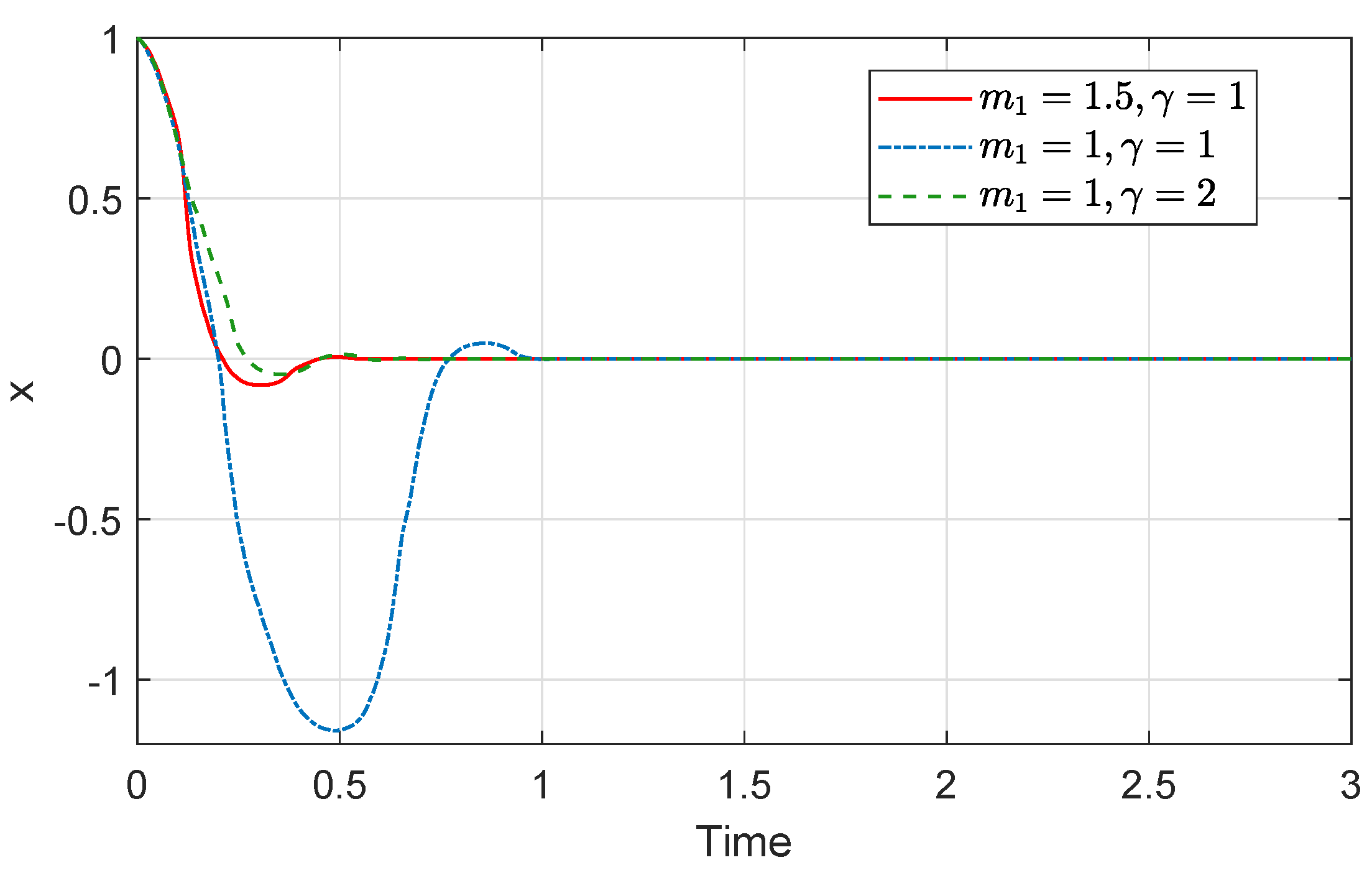
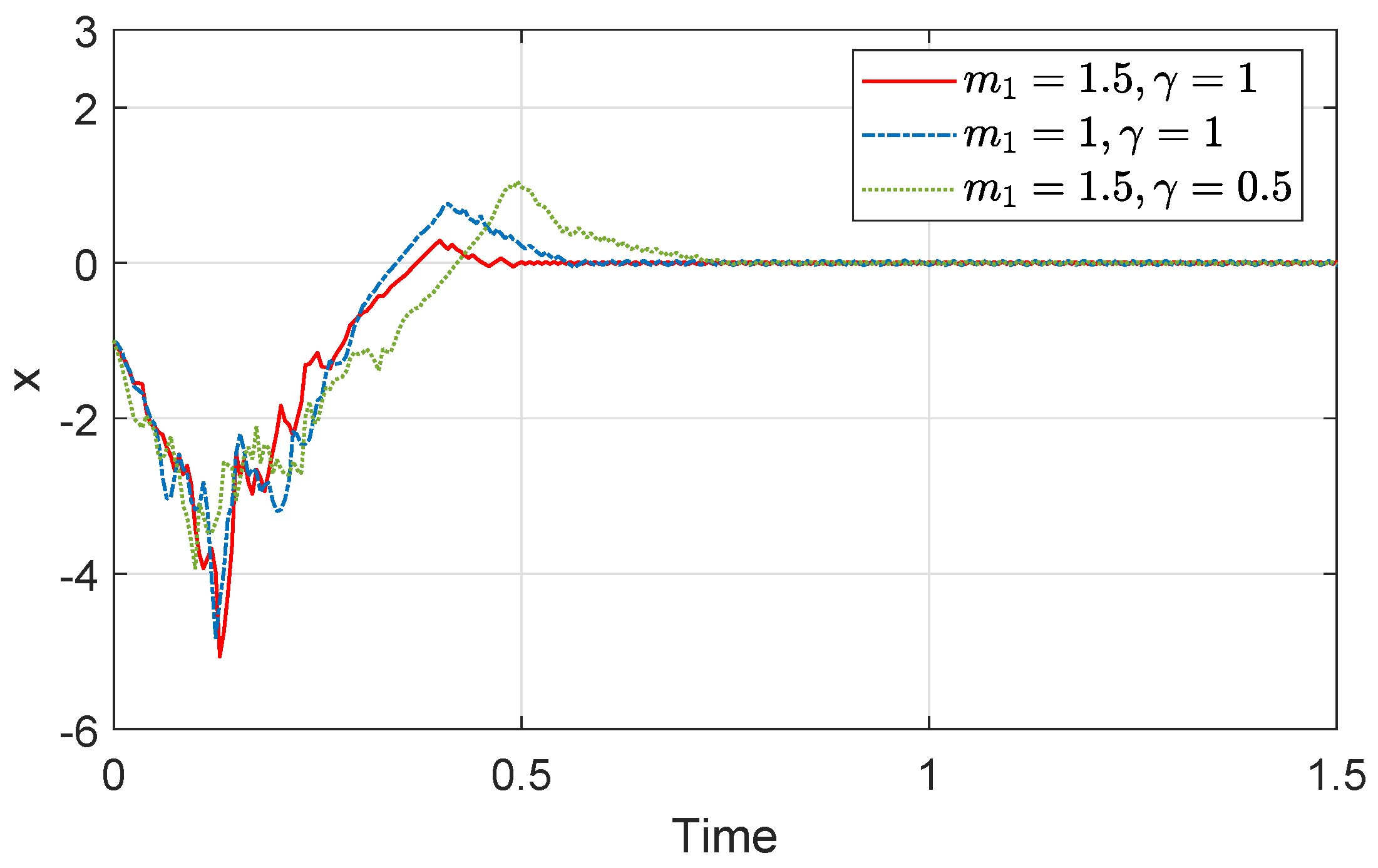
5. Simulation Example
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Qian, C.J.; Lin, W. Recursive observer design, homogeneous approximation, and nonsmooth output feedback stabilization of nonlinear systems. IEEE Trans. Autom. Control 2006, 51, 1457–1471. [Google Scholar] [CrossRef]
- Lin, W.; Qian, C.J. Adding one power integrator: A tool for global stabilization of high-order lower-triangular systems. Syst. Control Lett. 2000, 39, 339–351. [Google Scholar] [CrossRef]
- Zha, W.T.; Zhai, J.Y.; Fei, S.M. Global output feedback control for a class of high-order feedforward nonlinear systems with input delay. ISA Trans. 2013, 52, 494–500. [Google Scholar] [CrossRef] [PubMed]
- Sun, Z.Y.; Liu, C.Y.; Su, S.F.; Sun, W. Robust stabilization of high-order nonlinear systems with unknown sensitivities and applications in humanoid robot manipulation. IEEE Trans. Syst. Man Cybern. Syst. 2021, 51, 4409–4416. [Google Scholar] [CrossRef]
- Yu, D.X.; Chen, C.L.P.; Ren, C.E.; Sui, S. Swarm control for self-organized system with fixed and switching topology. IEEE Trans. Cybern. 2020, 50, 4481–4494. [Google Scholar] [CrossRef]
- Yu, D.X.; Chen, C.L.P. Automatic leader-follower persistent formation generation with minimum agent-movement in various switching topologies. IEEE Trans. Cybern. 2020, 50, 1569–1581. [Google Scholar] [CrossRef]
- Kong, M.R.; Liu, L. Exponential stabilization for a class of strict-feedback nonlinear time delay systems via state feedback control scheme. Processes 2022, 10, 1259. [Google Scholar] [CrossRef]
- Zhai, J.Y.; Zha, W.T. Global Adaptive Output Feedback Control for a Class of Nonlinear Time-Delay Systems. ISA Trans. 2014, 53, 2–9. [Google Scholar] [CrossRef]
- Jia, X.L.; Xu, S.Y.; Cui, G.Z.; Zhang, B.Y.; Ma, Q. Global adaptive regulation of feedforward nonlinear time-delay systems by output feedback. Int. J. Robust Nonlinear Control 2017, 27, 2451–2472. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Zhang, C.H.; Wang, Z. Adaptive disturbance attenuation for generalized high-order uncertain nonlinear systems. Automatica 2017, 80, 102–109. [Google Scholar] [CrossRef]
- Zhou, Q.; Li, H.Y.; Wu, C.W.; Wang, L.J.; Ahn, C.K. Adaptive fuzzy control of nonlinear systems with unmodeled dynamics and input saturation using small-gain approach. IEEE Trans. Syst. Man Cybern. Syst. 2016, 47, 1979–1989. [Google Scholar] [CrossRef]
- Li, G.J.; Xie, X.J. Adaptive state-feedback stabilization of stochastic high-order nonlinear systems with time-varying powers and stochastic inverse dynamics. IEEE Trans. Autom. Control 2020, 66, 5360–5367. [Google Scholar] [CrossRef]
- Cui, R.H.; Xie, X.J. Adaptive state-feedback stabilization of state-constrained stochastic high-order nonlinear systems. Sci. China Inform. Sci. 2021, 64, 200203. [Google Scholar] [CrossRef]
- Bhat, S.; Bernstein, D. Finite-time stability of continuous autonomous systems. SIAM J. Control Optim. 2000, 38, 751–766. [Google Scholar] [CrossRef]
- Moulay, E.; Perruquetti, W. Finite time stability conditions for non-autonomous continuous systems. Int. J. Control 2008, 81, 797–803. [Google Scholar] [CrossRef]
- Wang, F.; Zhang, X.Y. Adaptive finite time control of nonlinear systems under time-varying actuator failures. IEEE Trans. Syst. Man Cybern. Syst. 2018, 49, 1845–1852. [Google Scholar] [CrossRef]
- Li, H.Y.; Zhao, S.Y.; He, W.; Lu, R.Q. Adaptive finite-time tracking control of full state constrained nonlinear systems with dead-zone. Automatica 2019, 100, 99–107. [Google Scholar] [CrossRef]
- Yang, H.N.; Lian, Y.Z.; Zhang, X.Y.; Ye, Q.S.; Cao, X.N. Finite-time adaptive fuzzy consensus stabilization for unknown nonlinear leaderless multi-agent systems with unknown output dead-zone. IEEE Access 2019, 7, 103595–103607. [Google Scholar] [CrossRef]
- Li, K.W.; Tong, S.C. Observer-based finite-time fuzzy adaptive control for MIMO non-strict feedback nonlinear systems with errors constraint. Neurocomputing 2019, 341, 135–148. [Google Scholar] [CrossRef]
- Deng, H.; Krstic, M.; Williams, R.J. Stabilization of stochastic nonlinear systems driven by noise of unknown covariance. Int. J. Control Autom. Syst. 2001, 46, 1237–1253. [Google Scholar]
- Mao, X.R. Stochastic Differential Equations and Applications, 2nd ed.; Horwood Publishing: Chichester, UK, 2008. [Google Scholar]
- Zha, W.T.; Zhai, J.Y.; Fei, S.M. Global adaptive control for a class of uncertain stochastic nonlinear systems with unknown output gain. Int. J. Control Autom. Syst. 2017, 15, 1125–1133. [Google Scholar] [CrossRef]
- Li, H.Y.; Bai, L.; Zhou, Q.; Lu, R.Q.; Wang, L.J. Adaptive fuzzy control of stochastic nonstrict-feedback nonlinear systems with input saturation. IEEE Trans. Syst. Man Cybern. Syst. 2017, 47, 2185–2197. [Google Scholar] [CrossRef]
- Zhang, Y.; Ning, X.; Wang, Z.; Yu, D.X. High-order disturbance observer-based neural adaptive control for space unmanned systems with stochastic and high-dynamic uncertainties. IEEE Access 2021, 9, 77028–77043. [Google Scholar] [CrossRef]
- Gao, M.M.; Zhao, J.S.; Sun, W. Stochastic H2/H∞ control for discrete-time mean-field systems with Poisson jump. J. Franklin Inst. 2021, 358, 2933–2947. [Google Scholar] [CrossRef]
- Zhao, Y.X.; Wang, R.M.; Yin, C.C. Optimal dividends and capital injections for a spectrally positive Lévy process. Ind. Manag. Optim. 2017, 13, 1–21. [Google Scholar]
- Cui, R.H.; Xie, X.J. Output feedback stabilization of stochastic planar nonlinear systems with output constraint. Automatica 2022, 143, 110471. [Google Scholar] [CrossRef]
- Chen, W.S.; Jiao, L.C. Finite-time stability theorem of stochastic nonlinear systems. Automatica 2010, 46, 2105–2108. [Google Scholar] [CrossRef]
- Yin, J.L.; Khoo, S.Y.; Man, Z.H.; Yu, X.H. Finite-time stability and instability of stochastic nonlinear systems. Automatica 2011, 47, 2671–2677. [Google Scholar] [CrossRef]
- Yu, X.; Yin, J.L.; Khoo, S.Y. Generalized Lyapunov criteria on finite-time stability of stochastic nonlinear systems. Automatica 2019, 107, 183–189. [Google Scholar] [CrossRef]
- Wang, F.; Chen, B.; Sun, Y.M.; Gao, Y.L.; Lin, C. Finite-time fuzzy control of stochastic nonlinear systems. IEEE Trans. Syst. Man Cybern. 2019, 50, 2617–2626. [Google Scholar] [CrossRef]
- Li, Z.; Wang, F.; Wang, J.H. Adaptive finite-time neural control for a class of stochastic nonlinear systems with known hysteresis. IEEE Access 2020, 8, 123639–123648. [Google Scholar] [CrossRef]
- Wang, M.; Liu, Y.H.; Cao, G.; David, H.O. Energy-based finite-time stabilization and H∞ control of stochastic nonlinear systems. Int. J. Robust Nonlinear Control 2020, 30, 7169–7184. [Google Scholar] [CrossRef]
- Yu, X.; Yin, J.L.; Khoo, S.Y. “New Lyapunov conditions of stochastic finite-time stability and instability of nonlinear time-varying SDEs. Int. J. Control 2021, 94, 1674–1681. [Google Scholar] [CrossRef]
- Jiang, M.M.; Xie, X.J. Adaptive finite-time stabilization of stochastic nonlinear systems with the powers of positive odd rational numbers. Int. J. Adapt. Control Signal Process. 2019, 33, 1425–1439. [Google Scholar] [CrossRef]
- Khoo, S.Y.; Yin, J.L.; Man, Z.H.; Yu, X.H. Finite-time stabilization of stochastic nonlinear systems in strict-feedback form. Automatica 2013, 49, 1403–1410. [Google Scholar] [CrossRef]
- Cui, R.H.; Xie, X.J. Finite-time stabilization of output-constrained stochastic high-order nonlinear systems with high-order and low-order nonlinearities. Automatica 2022, 136, 110085. [Google Scholar] [CrossRef]
- Cui, R.H.; Xie, X.J. Finite-time stabilization of stochastic low-order nonlinear systems with time-varying orders and FT-SISS inverse dynamics. Automatica 2021, 125, 109418. [Google Scholar] [CrossRef]
- Yin, J.L.; Khoo, S.Y. Continuous finite-time state feedback stabilizers for some nonlinear stochastic systems. Int. J. Robust Nonlinear Control 2015, 25, 1581–1600. [Google Scholar] [CrossRef]
- Shen, Y.J.; Huang, Y.H. Global finite-time stabilisation for a class of nonlinear systems. Int. J. Syst. Sci. 2012, 43, 73–78. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Yun, M.M.; Li, T. A new approach to fast global finite-time stabilization of high-order nonlinear system. Automatica 2017, 81, 455–463. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Shao, Y.; Chen, C.C. Fast finite-time stability and its application in adaptive control of high-order nonlinear system. Automatica 2019, 106, 339–348. [Google Scholar] [CrossRef]
- Sun, W.J.; Zhao, J.S.; Sun, W.; Xia, J.W.; Sun, Z.Y. Adaptive event-triggered global fast finite-time control for a class of uncertain nonlinear systems. Int. J. Robust Nonlinear Control 2020, 30, 3773–3785. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Dong, Y.Y.; Chen, C.C. Global fast finite-time partial state feedback stabilization of high-order nonlinear systems with dynamic uncertainties. Inf. Sci. 2019, 484, 219–236. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, Y.; Zhao, J. Fast Finite-Time Stability and Its Application in Adaptive Control of High-Order Stochastic Nonlinear Systems. Processes 2022, 10, 1676. https://doi.org/10.3390/pr10091676
Yuan Y, Zhao J. Fast Finite-Time Stability and Its Application in Adaptive Control of High-Order Stochastic Nonlinear Systems. Processes. 2022; 10(9):1676. https://doi.org/10.3390/pr10091676
Chicago/Turabian StyleYuan, Yixuan, and Junsheng Zhao. 2022. "Fast Finite-Time Stability and Its Application in Adaptive Control of High-Order Stochastic Nonlinear Systems" Processes 10, no. 9: 1676. https://doi.org/10.3390/pr10091676
APA StyleYuan, Y., & Zhao, J. (2022). Fast Finite-Time Stability and Its Application in Adaptive Control of High-Order Stochastic Nonlinear Systems. Processes, 10(9), 1676. https://doi.org/10.3390/pr10091676








