High-Speed Laser Cutting Silicon-Glass Double Layer Wafer with Laser-Induced Thermal-Crack Propagation
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Procedure
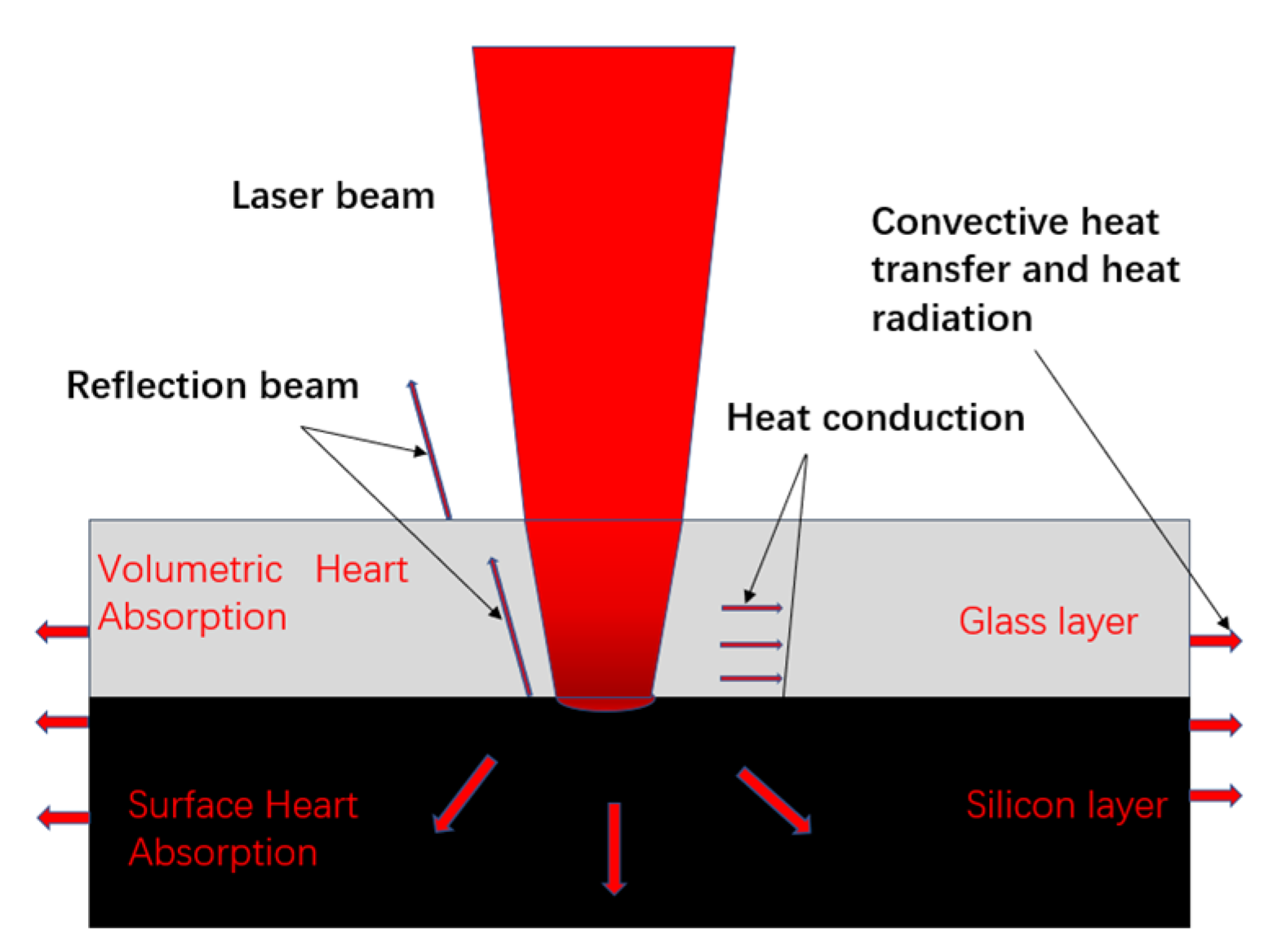
2.2. Theoretical Approach
- The material distribution of glass and silicon layers was uniform and flawless; both the glass and silicon layers were isotropic in thermal analysis, while in the stress analysis, the glass layer was isotropic and the silicon layer was anisotropic, unlike other layers.
- The glass-silicon double wafer anode bond layer was free of any defects; the friction problem and heat transfer between the sample and the fixture during the scanning process were ignored. Since the thickness of the bond layer was extremely small (2–20 nm) and can be considered zero, the gravitational and residual stresses after anode bonding could also be neglected.
- All parameters were ignored or set to zero except for the process parameters, influencing factors, and material parameters considered in the simulation such as beam quality factor, etc.
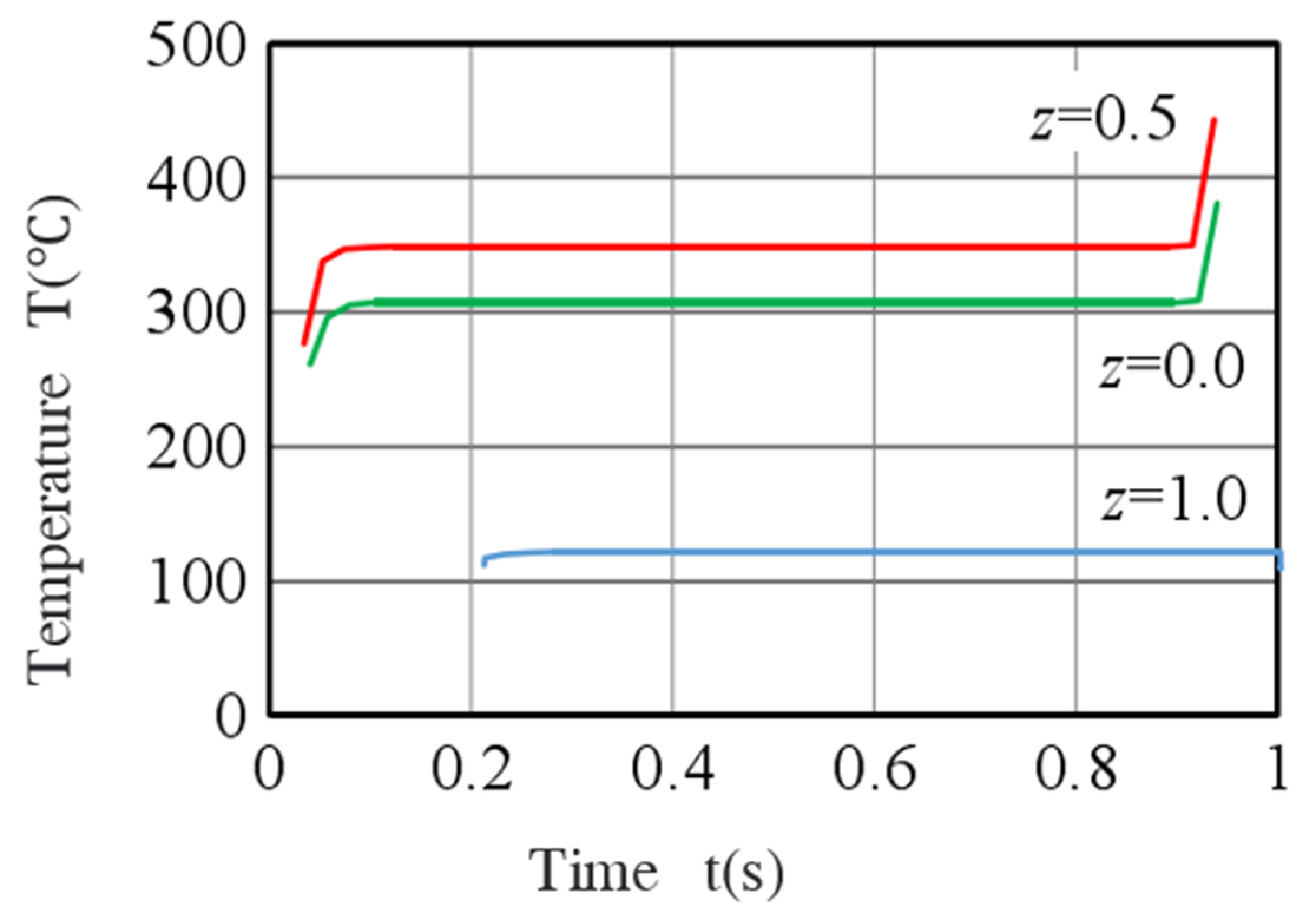
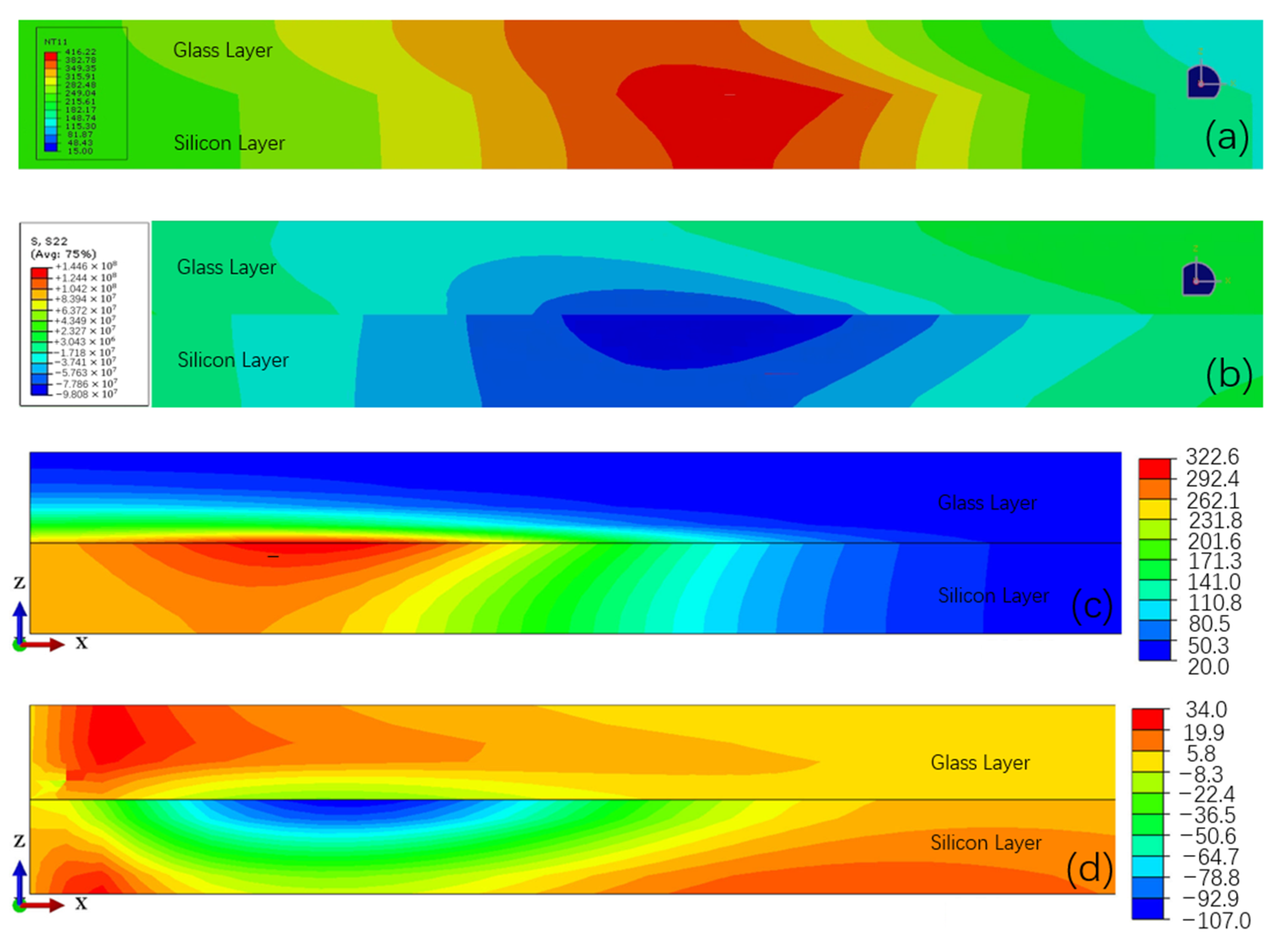
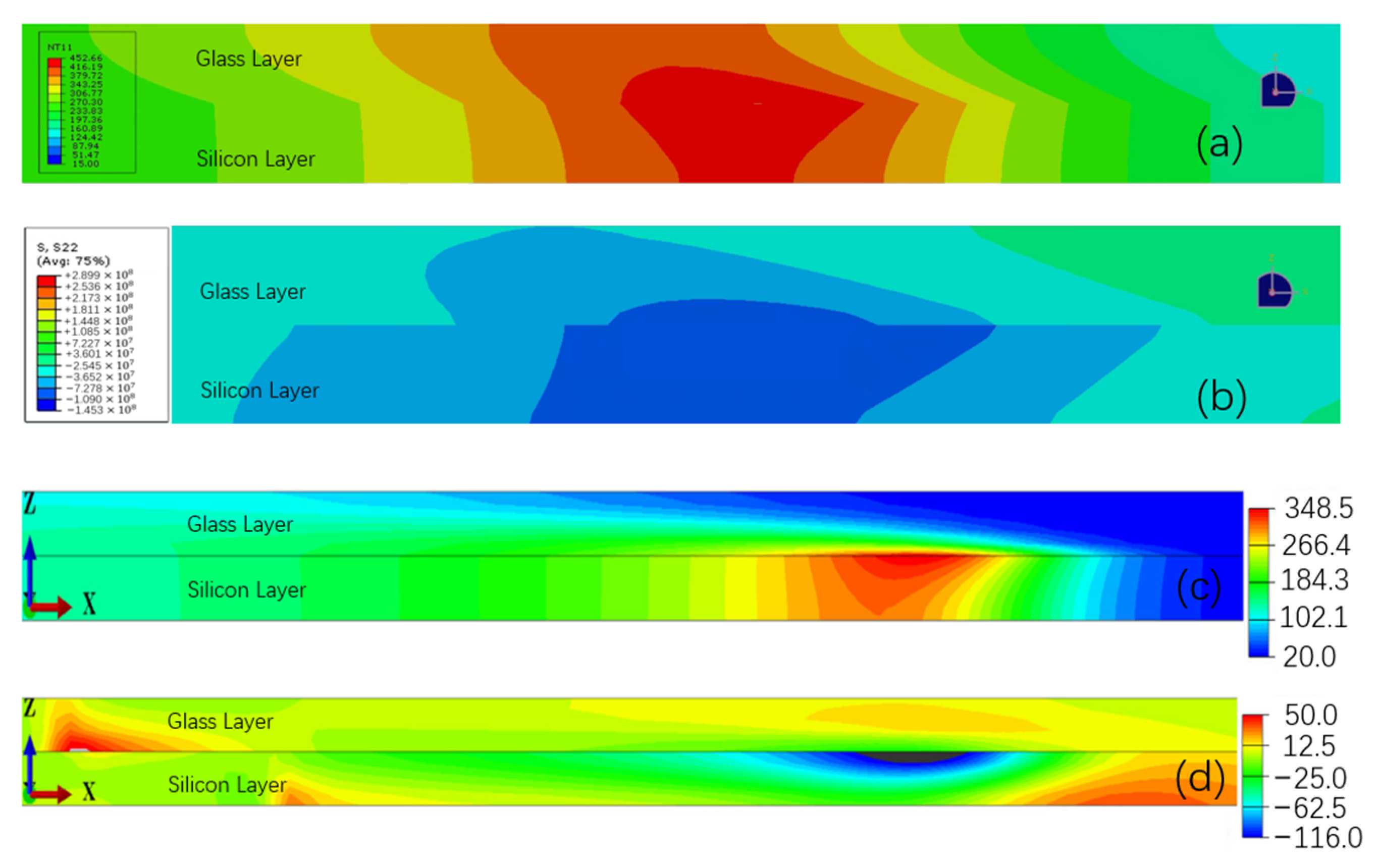
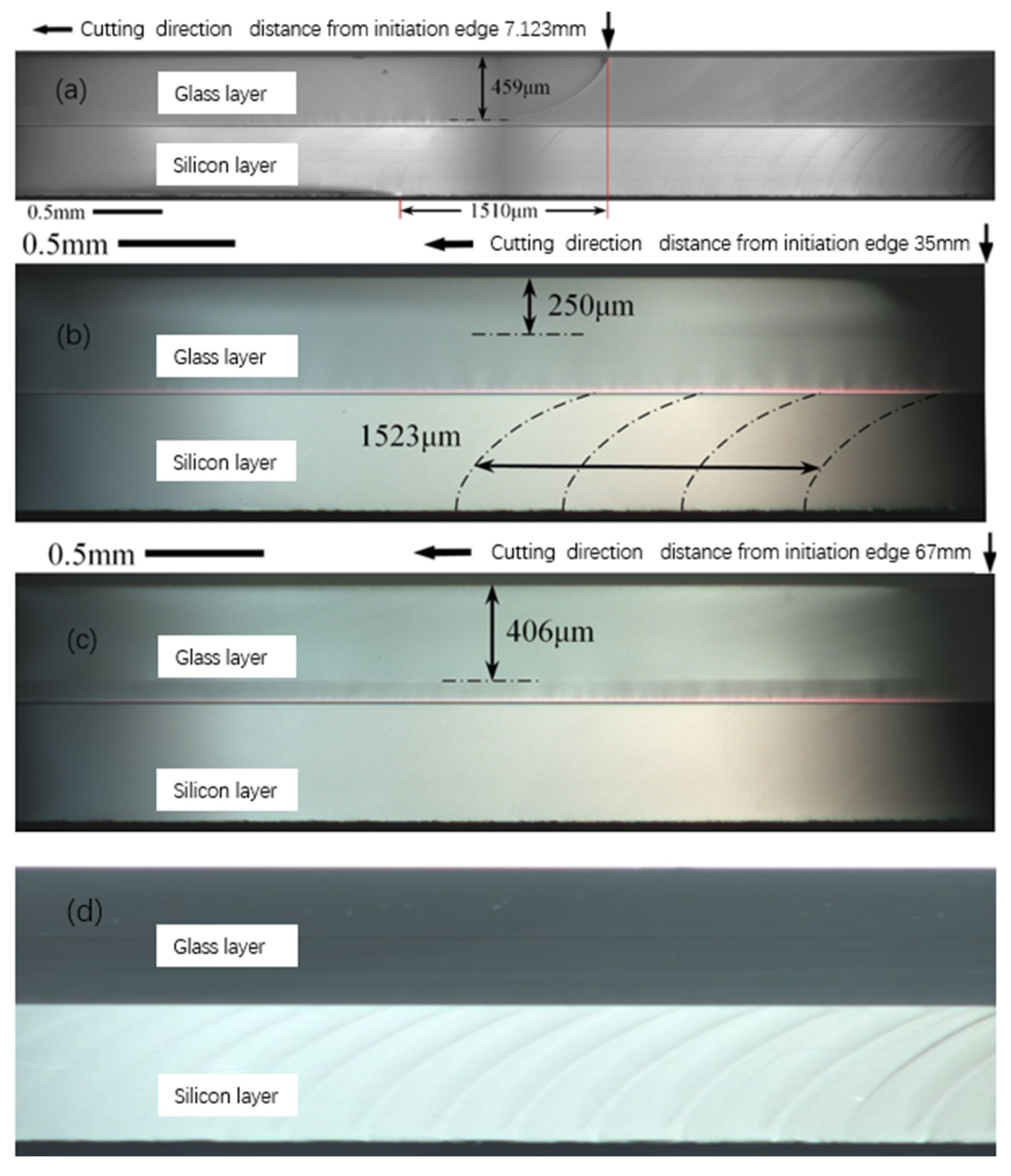
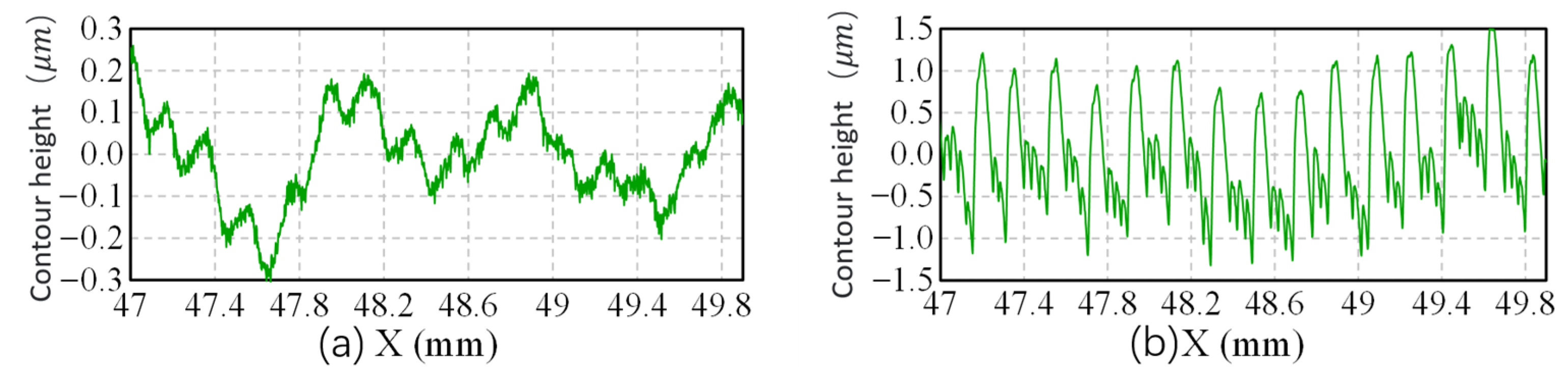
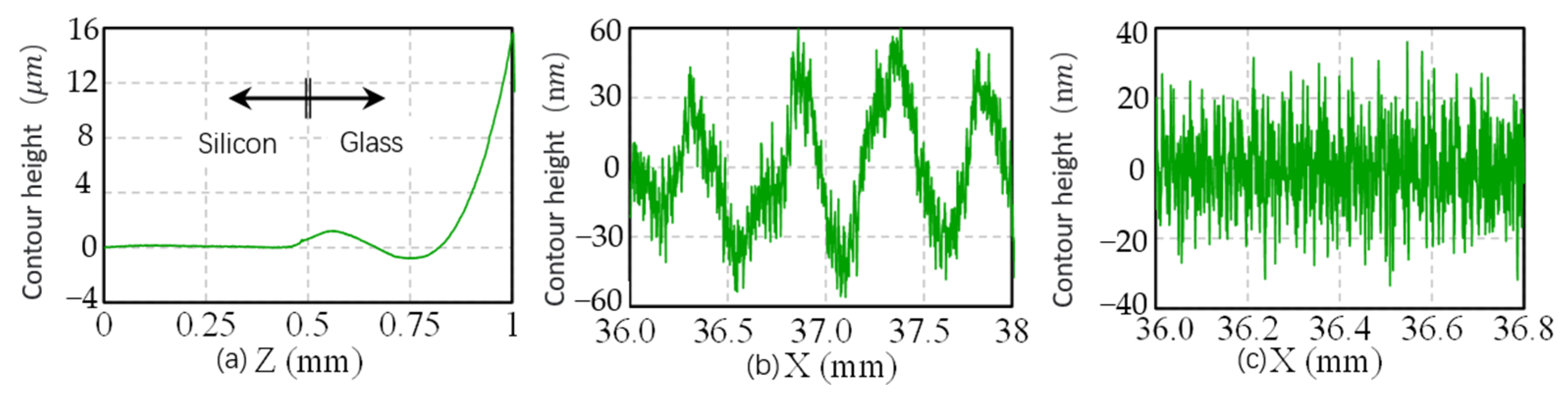
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kang, H.S.; Hong, S.K.; Oh, S.C.; Choi, J.Y.; Song, M.G. Cutting glass by laser. In Proceedings of the Second International Symposium on Laser Precision Microfabrication, Singapore, 16–18 May 2002; SPIE: Bellingham, WA, USA; Volume 4426, pp. 367–370. [Google Scholar]
- Wang, H.J.; Yang, T. A review on laser drilling and cutting of silicon. J. Eur. Ceram. Soc. 2021, 41, 4997–5015. [Google Scholar] [CrossRef]
- Knowles, K.M.; Van Helvoort, A.T.J. Anodic bonding. Int. Mater. Rev. 2006, 51, 273–311. [Google Scholar] [CrossRef]
- Esashi, M. Wafer level packaging of MEMS. J. Micromech. Microeng. 2008, 18, 073001. [Google Scholar] [CrossRef]
- Zhang, X.H.; Chen, G.Y.; An, W.K.; Deng, Z.H.; Zhou, Z.X. Experimental investigations of machining characteristics of laser-induced thermal cracking in alumina ceramic wet grinding. Int. J. Adv. Manuf. Technol. 2014, 72, 1325–1331. [Google Scholar] [CrossRef]
- Nagimova, A.; Perveen, A. A review on laser machining of hard to cut materials. Mater. Today Proc. 2019, 18, 2440–2447. [Google Scholar] [CrossRef]
- Zhimalov, A.B.; Solinov, V.F.; Kondratenko, V.S.; Kaplina, T.V. Laser cutting of float glass during production. Glass and ceramics. 2006, 63, 319–321. [Google Scholar] [CrossRef]
- Miyake, Y. Separation technology for FPD glass. J. Jpn. Soc. Abras. Technol. 2001, 47, 342–347. [Google Scholar]
- Riveiro, A.; Quintero, F.; Lusquiños, F.; Comesaña, R.; Pou, J. Study of melt flow dynamics and influence on quality for CO2 laser fusion cutting. J. Phys. D Appl. Phys. 2011, 44, 135501. [Google Scholar] [CrossRef] [Green Version]
- Puurunen, R.L.; Kattelus, H.; Suntola, T. Handbook of Silicon Based MEMs Materials and Technologies; Lindroos, V., Tilli, M., Lehto, A., Motooka, T., Eds.; Elsevier: Oxford, UK, 2010. [Google Scholar]
- Cheng, X.; Yang, L.; Wang, M.; Cai, Y.; Wang, Y.; Ren, Z. Laser beam induced thermal-crack propagation for asymmetric linear cutting of silicon wafer. Opt. Laser Technol. 2019, 120, 105765. [Google Scholar] [CrossRef]
- Lumley, R.M. Controlled separation of brittle materials using a laser. Ceram. Bull. 1969, 48, 850. [Google Scholar]
- Xu, J.; Hu, H.; Zhuang, C.; Ma, G.; Han, J.; Lei, Y. Controllable laser thermal cleavage of sapphire wafers. Opt. Lasers Eng. 2018, 102, 26–33. [Google Scholar] [CrossRef]
- Kechagias, J.D.; Fountas, N.A.; Ninikas, K.; Petousis, M.; Vidakis, N.; Vaxevanidis, N. Surface characteristics investigation of 3D-printed PET-G plates during CO2 laser cutting. Mater. Manuf. Process. 2022, 37, 1347–1357. [Google Scholar] [CrossRef]
- Fountas, N.A.; Ninikas, K.; Chaidas, D.; Kechagias, J.; Vaxevanidis, N.M. Neural networks for predicting kerf characteristics of CO2 laser-machined FFF PLA/WF plates. In Proceedings of the MATEC Web of Conferences, Rennes, France, 8–10 September 2022; EDP Sciences: Les Ulis, France; Volume 368, p. 01010. [Google Scholar]
- Samieian, M.A.; Cormie, D.; Smith, D.; Wholey, W.; Blackman, B.R.; Dear, J.P.; Hooper, P.A. Temperature effects on laminated glass at high rate. Int. J. Impact Eng. 2018, 111, 177–186. [Google Scholar] [CrossRef]
- Yahata, K.; Yamamoto, K.; Ohmura, E. Crack Propagation Analysis in Laser Scribing of Glass. J. Laser Micro/Nanoeng. 2010, 5, 109–114. [Google Scholar] [CrossRef]
- Cai, Y.; Wang, M.; Zhang, H.; Yang, L.; Fu, X.; Wang, Y. Laser cutting sandwich structure glass–silicon–glass wafer with laser induced thermal–crack propagation. Opt. Laser Technol. 2017, 93, 49–59. [Google Scholar] [CrossRef]
- Zhao, C.; Cai, Y.; Ding, Y.; Yang, L.; Wang, Z.; Wang, Y. Investigation on the crack fracture mode and edge quality in laser dicing of glass-anisotropic silicon double-layer wafer. J. Mater. Process. Technol. 2020, 275, 116356. [Google Scholar] [CrossRef]
- Zhao, C.; Zhang, H.; Wang, Y. Semiconductor laser asymmetry cutting glass with laser induced thermal-crack propagation. Opt. Lasers Eng. 2014, 63, 43–52. [Google Scholar] [CrossRef]
- Cai, Y.; Yang, L.; Zhang, H.; Wang, Y. Laser cutting silicon-glass double layer wafer with laser induced thermal-crack propagation. Opt. Lasers Eng. 2016, 82, 173–185. [Google Scholar] [CrossRef]
- Weinhold, S.; Gruner, A.; Ebert, R.; Schille, J.; Exner, H. Study of fast laser induced cutting of silicon materials. In Proceedings of the Laser Applications in Microelectronic and Optoelectronic Manufacturing (LAMOM) XIX, San Francisco, CA, USA, 1–6 February 2014; SPIE: Bellingham, WA, USA; Volume 8967, pp. 333–339. [Google Scholar]
- Haupt, O.; Schuetz, V.; Schoonderbeek, A.; Richter, L.; Kling, R. High quality laser cleaving process for mono-and polycrystalline silicon. In Proceedings of the Laser-based Micro-and Nanopackaging and Assembly III, San Jose, CA, USA, 24–29 January 2009; SPIE: Bellingham, WA, USA; Volume 7202, pp. 155–165. [Google Scholar]
- Yahata, K.; Ohmura, E.; Shimizu, S.; Murakami, M. Boundary Element Analysis of Crack Propagation in Laser Scribing of Glass. J. Laser Micro/Nanoeng. 2013, 8, 102–109. [Google Scholar] [CrossRef] [Green Version]
- Masolin, A.; Bouchard, P.-O.; Martini, R.; Bernacki, M. Thermo-mechanical and fracture properties in single-crystal silicon. J. Mater. Sci. 2013, 48, 979–988. [Google Scholar] [CrossRef] [Green Version]
- Green, M.A. Self-consistent optical parameters of intrinsic silicon at 300 K including temperature coefficients. Sol. Energy Mater. Sol. Cells 2008, 92, 1305–1310. [Google Scholar] [CrossRef]
- Watanabe, H.; Yamada, N.; Okaji, M. Linear thermal expansion coefficient of silicon from 293 to 1000 K. Int. J. Thermophys. 2004, 25, 221–236. [Google Scholar] [CrossRef]
- Zhao, C.; Zhang, H.; Yang, L.; Wang, Y.; Ding, Y. Dual laser beam revising the separation path technology of laser induced thermal-crack propagation for asymmetric linear cutting glass. Int. J. Mach. Tools Manuf. 2016, 106, 43–55. [Google Scholar] [CrossRef]

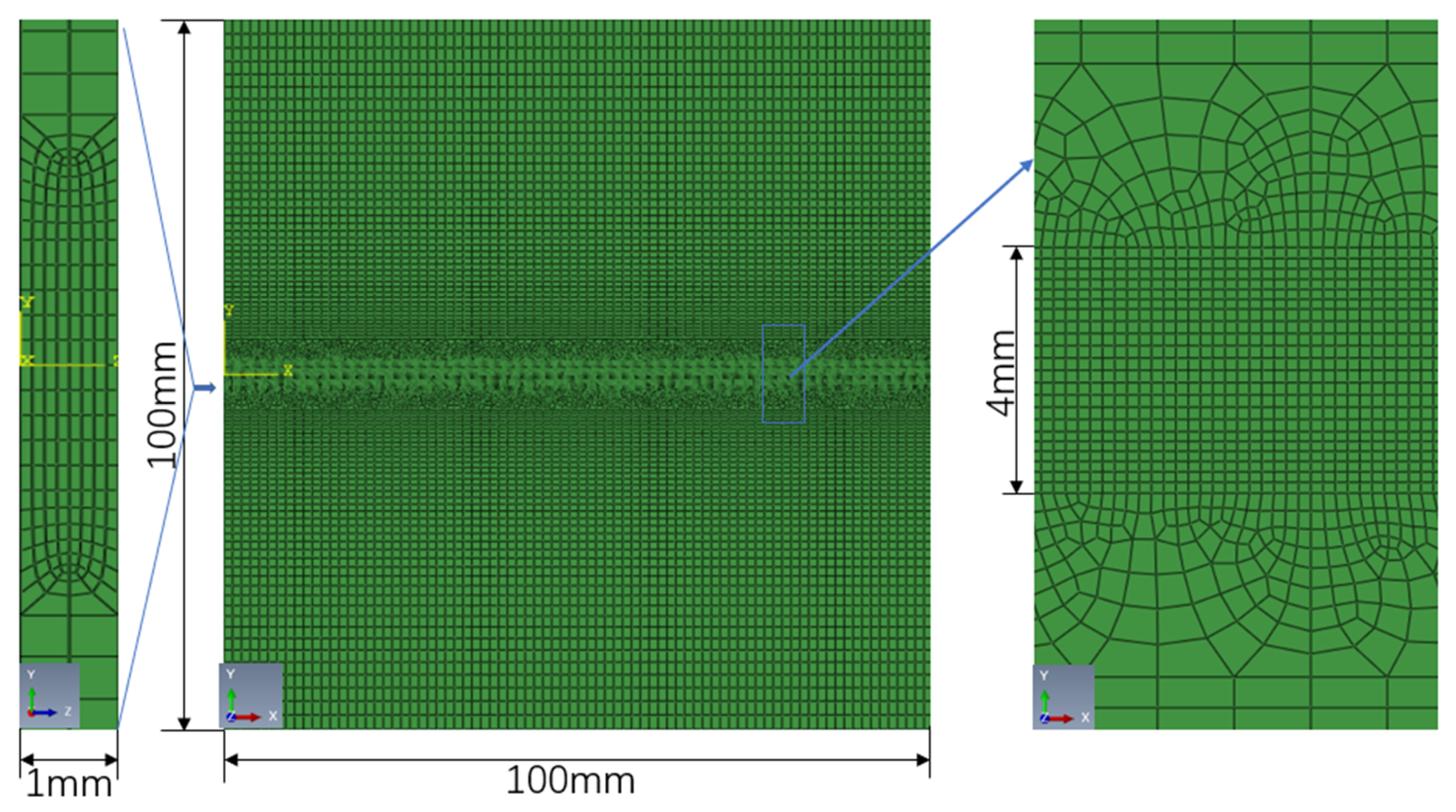


| Sample Size | Laser Power (W) | Scanning Speed (mm/s) | Laser Spot Diameter (mm) |
|---|---|---|---|
| 100 100 (0.5 + 0.5) | 195 | 110 | 4.0 |
| T | Thermal Conductivity | Specific Heat | Poisson’s Ratio | Young’s Modulus | Expansion Coefficient |
|---|---|---|---|---|---|
| 25.0 | 1.08 | 758 | 0.200 | 64.0 | 3.20 |
| 125.0 | 1.25 | 1071 | 0.202 | 65.0 | 3.20 |
| 225.0 | 1.43 | 1175 | 0.204 | 66.0 | 3.26 |
| 325.0 | 1.60 | 1244 | 0.206 | 67.0 | 3.38 |
| 425.0 | 1.76 | 1290 | 0.208 | 68.0 | 3.61 |
| 525.0 | 1.92 | 1325 | 0.210 | 69.0 | 5.70 |
| T | Specific Heat | Thermal Conductivity | Expansion Coefficient | Elastic Constant | ||
|---|---|---|---|---|---|---|
| 25.0 | 713 | 148.0 | 2.63 | 155.6 | 63.94 | 79.51 |
| 125.0 | 788 | 98.9 | 3.23 | 164.3 | 63.25 | 78.78 |
| 225.0 | 830 | 76.2 | 3.60 | 162.9 | 62.69 | 78.05 |
| 325.0 | 859 | 61.9 | 3.83 | 161.5 | 62.06 | 77.33 |
| 425.0 | 887 | 51.0 | 4.01 | 160.1 | 61.43 | 76.60 |
| 525.0 | 908 | 42.2 | 4.14 | 158.7 | 60.81 | 75.87 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, C.; Yang, Z.; Kang, S.; Qiu, X.; Xu, B. High-Speed Laser Cutting Silicon-Glass Double Layer Wafer with Laser-Induced Thermal-Crack Propagation. Processes 2023, 11, 1177. https://doi.org/10.3390/pr11041177
Zhao C, Yang Z, Kang S, Qiu X, Xu B. High-Speed Laser Cutting Silicon-Glass Double Layer Wafer with Laser-Induced Thermal-Crack Propagation. Processes. 2023; 11(4):1177. https://doi.org/10.3390/pr11041177
Chicago/Turabian StyleZhao, Chunyang, Zhihui Yang, Shuo Kang, Xiuhong Qiu, and Bin Xu. 2023. "High-Speed Laser Cutting Silicon-Glass Double Layer Wafer with Laser-Induced Thermal-Crack Propagation" Processes 11, no. 4: 1177. https://doi.org/10.3390/pr11041177
APA StyleZhao, C., Yang, Z., Kang, S., Qiu, X., & Xu, B. (2023). High-Speed Laser Cutting Silicon-Glass Double Layer Wafer with Laser-Induced Thermal-Crack Propagation. Processes, 11(4), 1177. https://doi.org/10.3390/pr11041177






