Experimental Study on Mechanical Properties of Concrete Containing Waste Glass and Its Application on Concrete-Filled Steel Tubular Columns
Abstract
1. Introduction
2. Experimental Program
2.1. Material Component and Mix Proportion of GC
2.2. Flowability and Compressive Strength Test
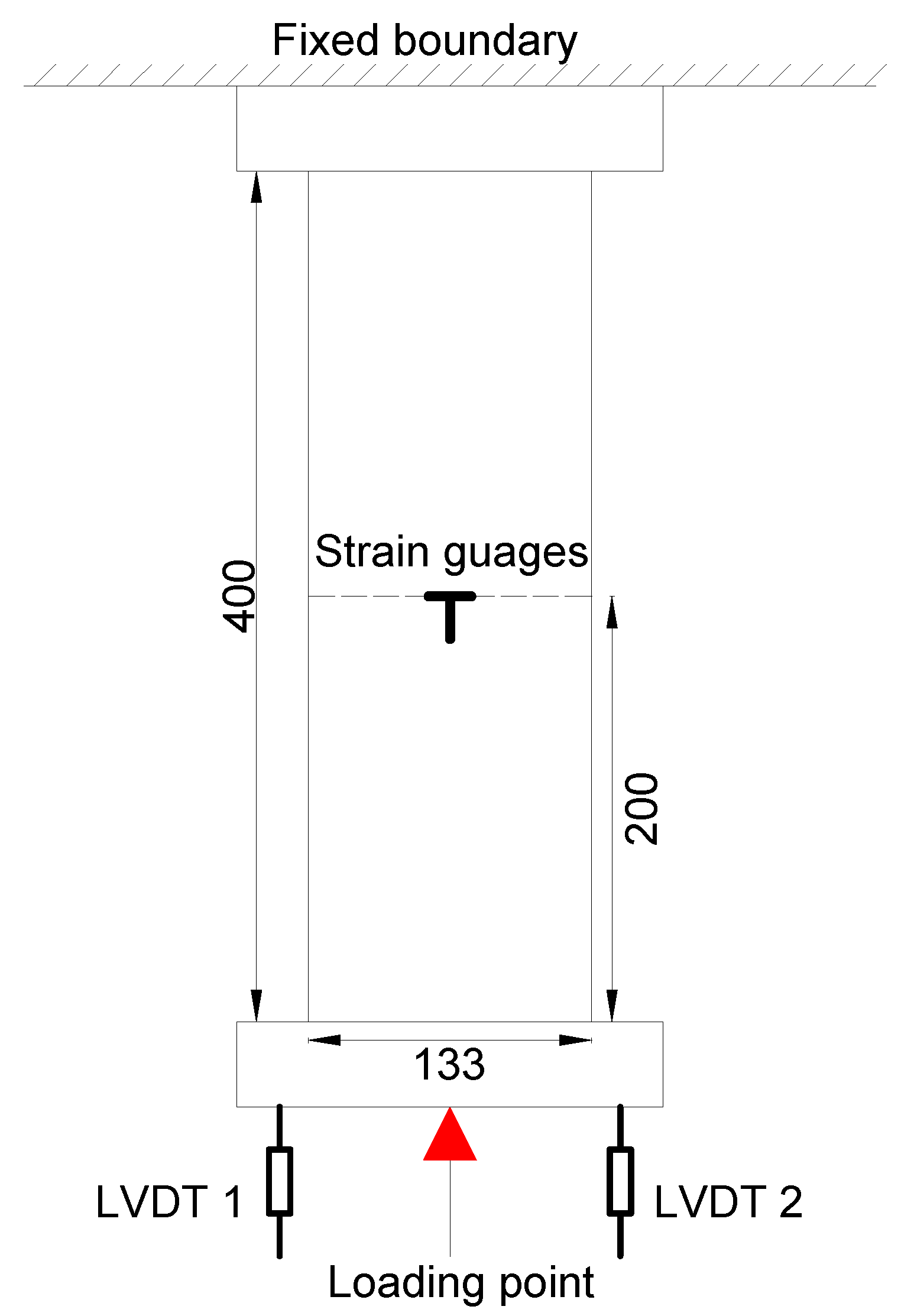
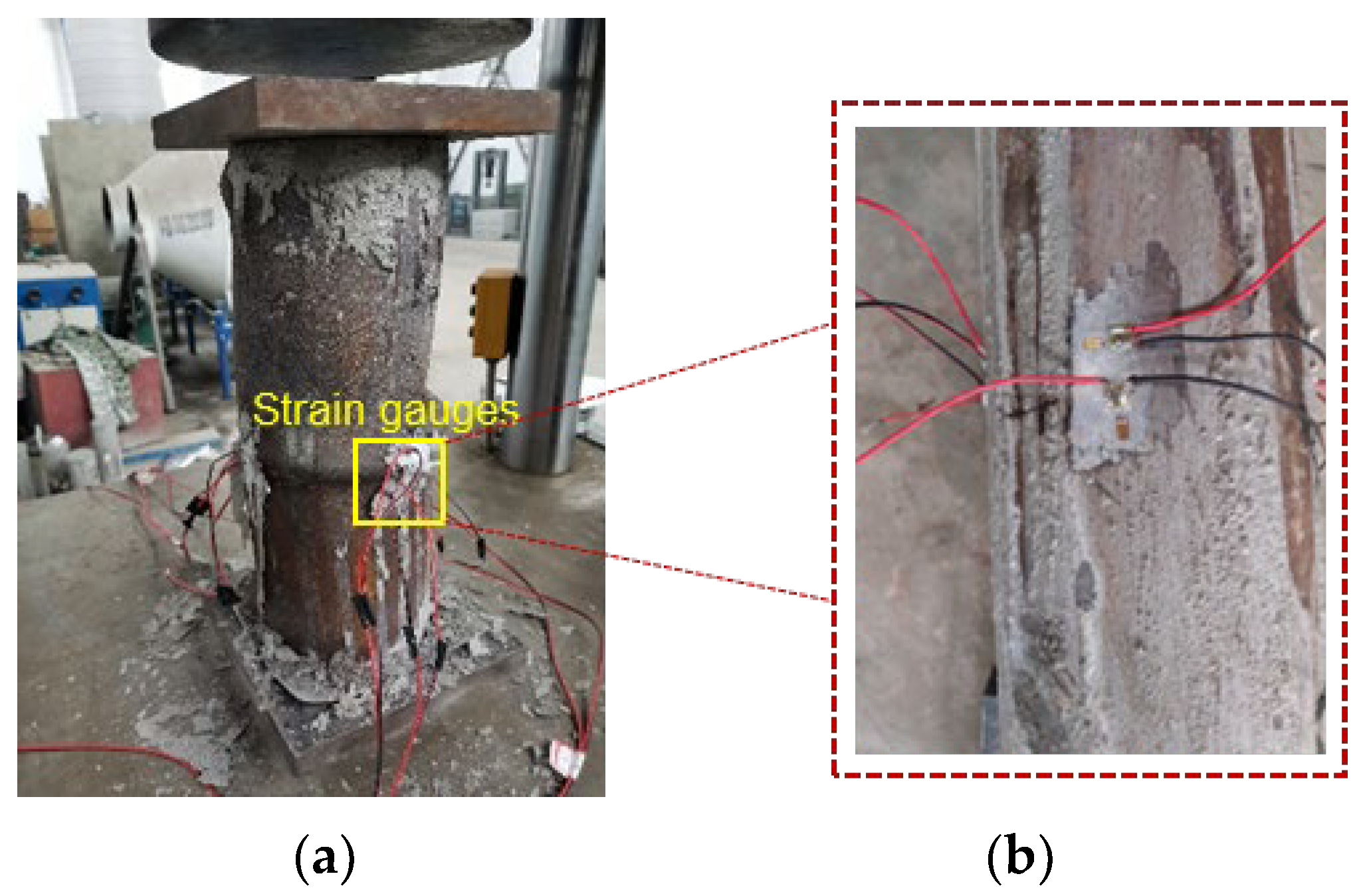
2.3. Glass Concrete-Filled Steel Tubes (GCFSTs) Test
3. Test Results and Discussion
3.1. Material Properties
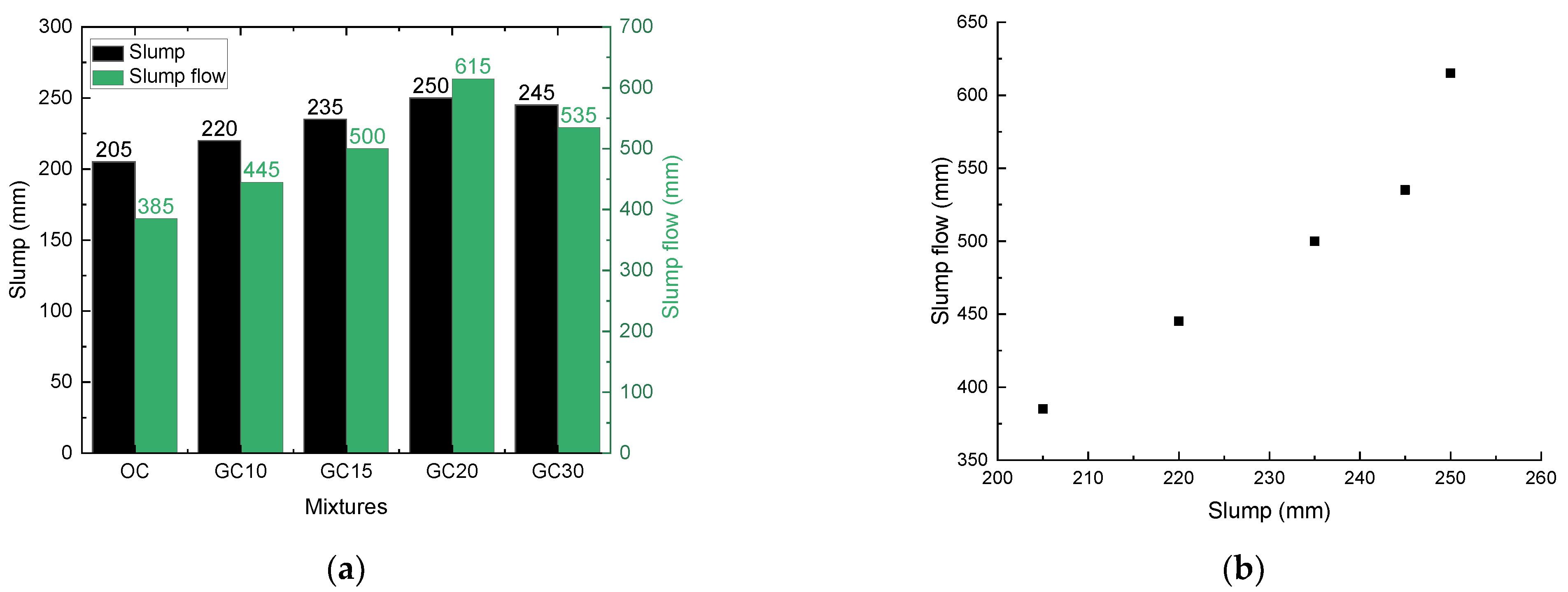
3.1.1. Slump and Slump Flow of OC and GC Mixtures
3.1.2. Compressive Strength of OC and GC Mixtures
3.2. Structural Behaviour of CFST
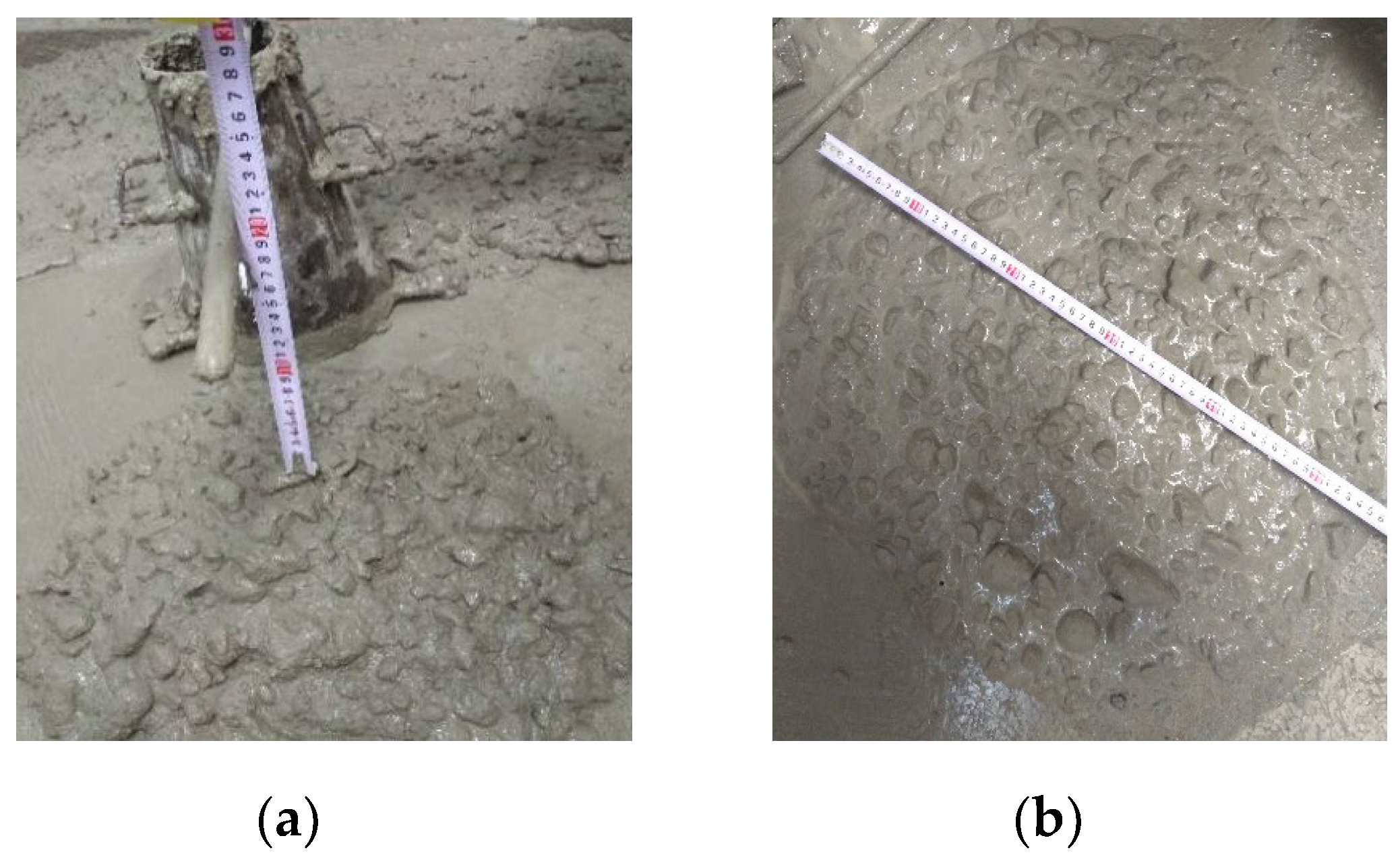
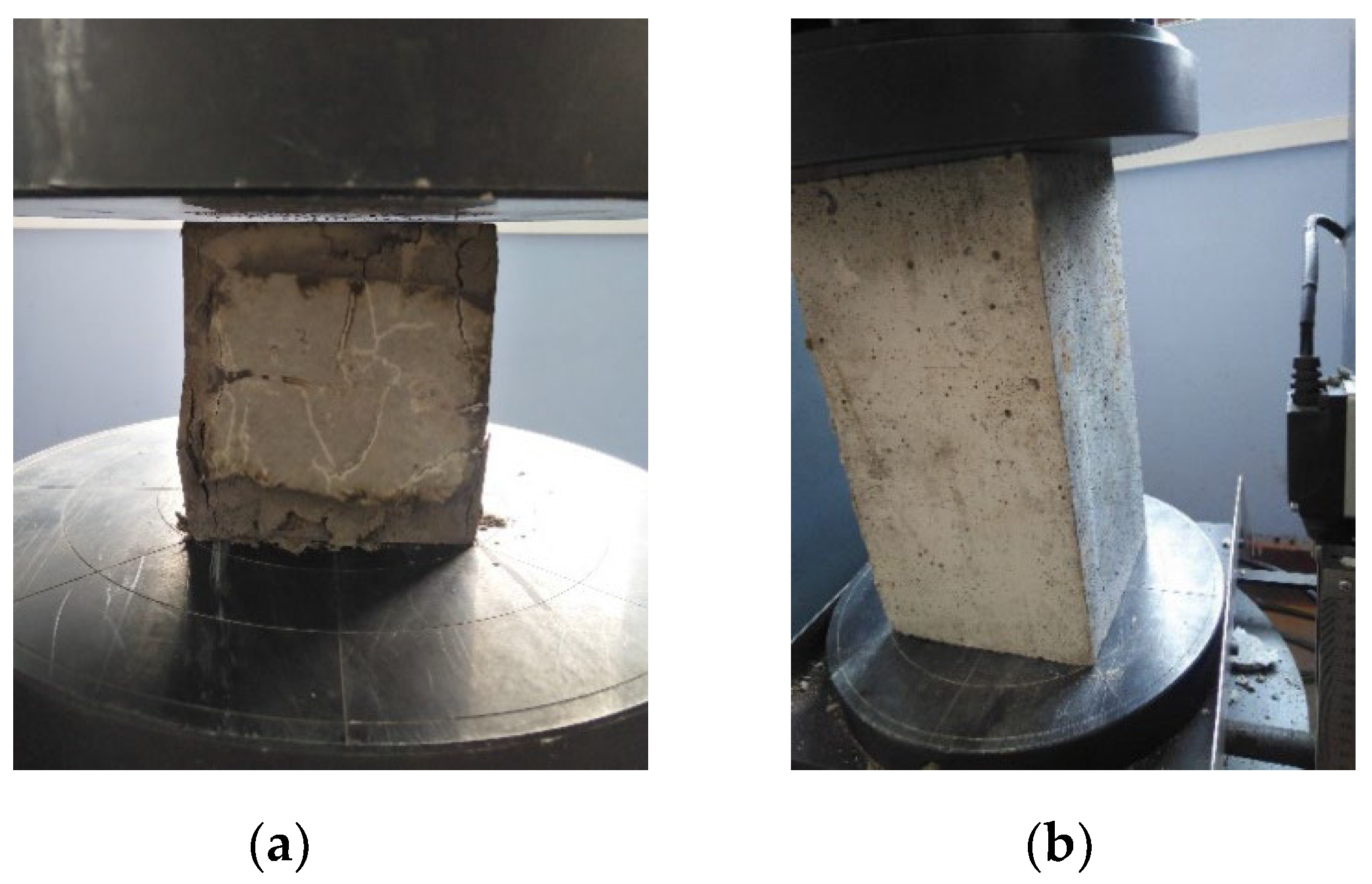
3.2.1. Failure Patterns
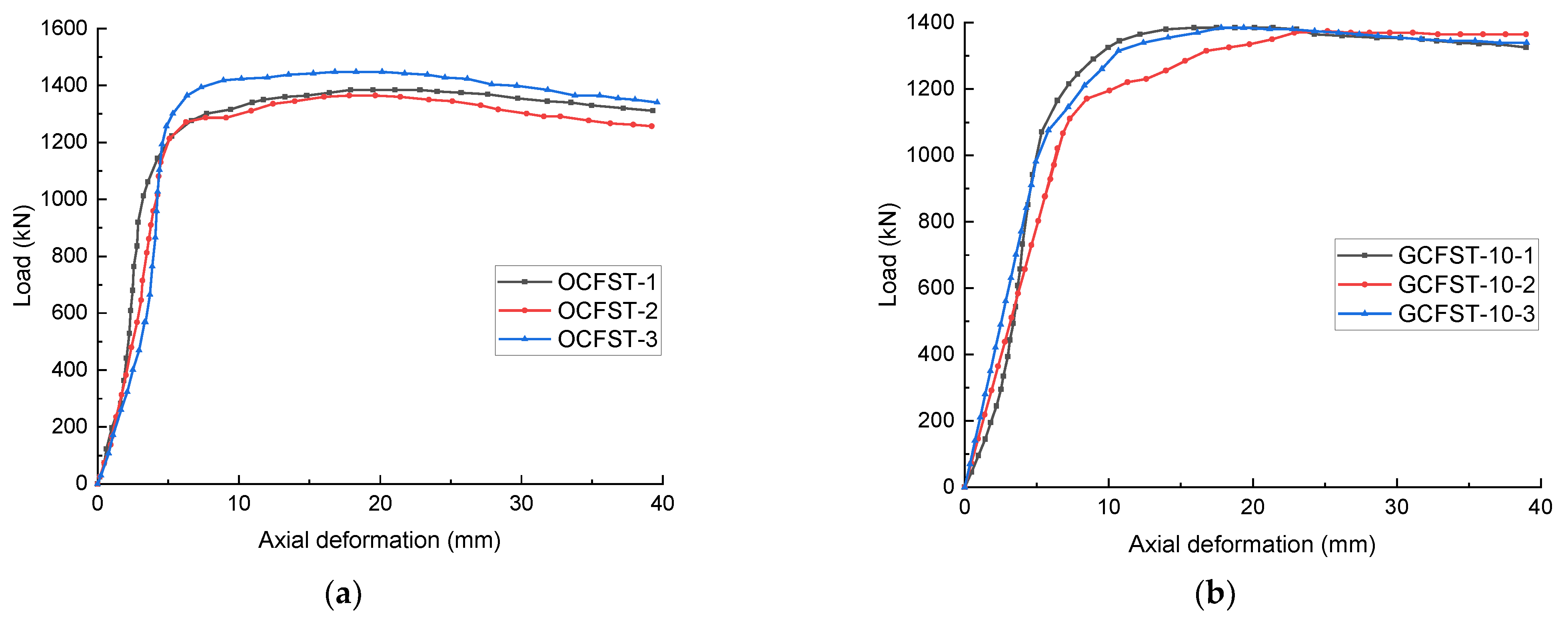
3.2.2. Load–Deformation Response
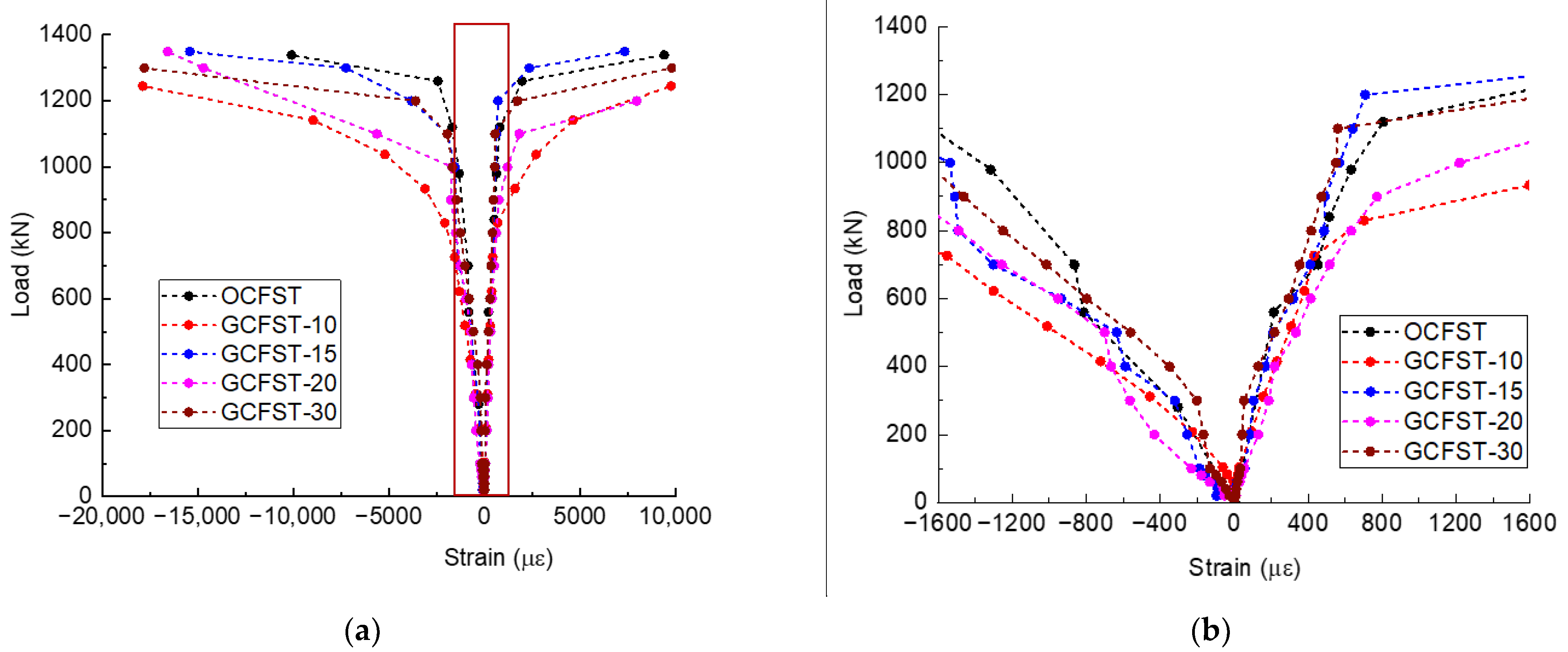
3.2.3. Load–Strain Relationship
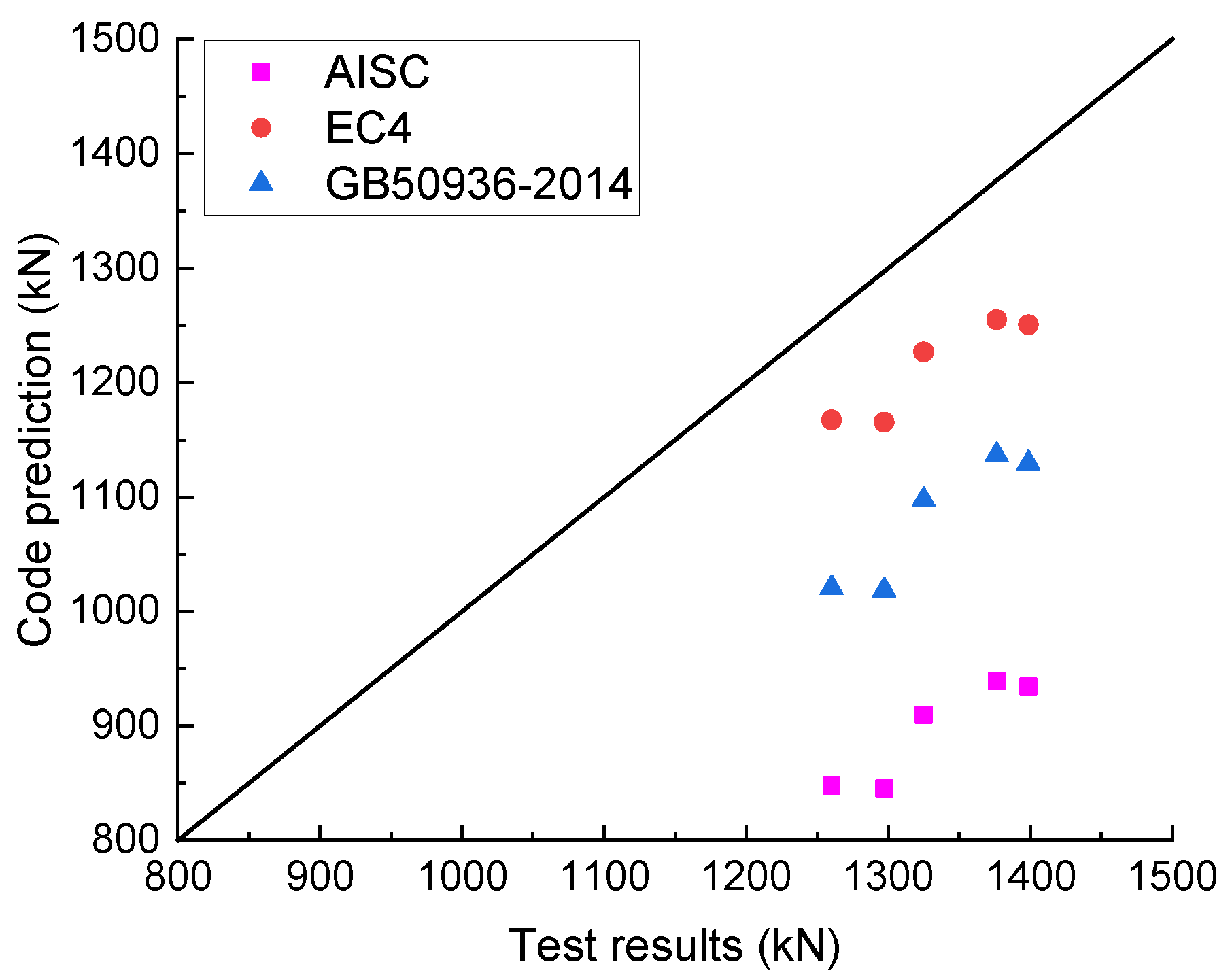
4. Evaluation of Existing Methods for Ultimate Strength Prediction
5. Conclusions
- The addition of glass particles (powders and beads) will increase the flowability of the concrete due to reduced particle cohesion resulting from the smooth surface of the glass beads, and higher w/c ratio as a consequence of cement substitution. The highest slump and slump flow are achieved at a 20% glass replacement ratio, exhibiting an increase of 20% and 60%, respectively, compared to ordinary concrete. Beyond a 20% replacement ratio, a decrease in slump and slump flow was observed due to the sharp edges and higher specific area of the glass powder.
- The compressive strength of concrete will continuously decrease as more glass particles are added to the concrete mixture, which might be owing to the decrease of cement hydration and weak bonds between the glass beads and the matrix. However, the reduction of prismatic compressive strength (within 10%) is not obvious if less than 20% glass is applied, due to the pozzolanic reaction of the glass powder. Moreover, compared to OC, more curing time is required for GC to fully develop its strength. Overall, the use of glass particles in concrete mixtures requires careful consideration of the desired properties, and the appropriate proportion of glass particles to avoid the reduction of the mechanical properties. When both workability and compressive strength are taken into consideration, it is recommended to use less than 20% glass particles for combined cement and aggregate replacement.
- Compared to OCFSTs, GCFSTs exhibit a similar damage process (i.e., linear-elastic stage, plastic deformation stage, and post-peak failure stage) and drum-type failure patterns at the mid-height of the column. Though GC has lower compressive strength compared to OCFSTs, less than a 10% decrease of the ultimate strength is observed in GCFSTs due to more significant confinement from the outer steel tube, which further restricts the expansion induced by possible adverse ASRs in GC.
- GCFSTs exhibit a higher deformational ability at peak load (1.25 times higher than OCFSTs), and comparable ultimate deformation at failure load compared to their OCFST counterparts, which is possibly due to the enhanced composite action of GC and the steel tube, which shows potential for the application of GC in CFSTs.
- Compared to OCFSTs, the addition of glass particles in GCFSTs enables it to have a higher axial strain capacity while still maintaining comparable strain limits in the lateral direction. Therefore, GCFSTs exhibit better deformational ability in the axial direction under compressive load.
- The experimental results are compared to the predicted ultimate bearing capacity based on existing design codes (AISC, EC4, and GB50936-2014). It is demonstrated that all of the design codes, in general, provide conservative and safe predictions for circular GCFSTs, and that the EC4 gives the closest prediction (predicted to experimental peak load ratio is 0.9), while the AISC underestimates the actual compressive capacity to a great extent.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nodehi, M.; Taghvaee, V.M. Sustainable Concrete for Circular Economy: A Review on Use of Waste Glass. Glas. Struct. Eng. 2021, 7, 3–22. [Google Scholar] [CrossRef]
- Dhir, R.K.; De Brito, J.; Ghataora, G.S.; Lye, C.Q. Sustainable Construction Materials: Glass Cullet; Woodhead Publishing: Sawston, UK, 2018; ISBN 9780081009840. [Google Scholar]
- Department of Distribution Industry. Development Report of China Renewable Resources Recycling Industry 2018; The Ministry of Commerce (MOFCOM): Beijing, China, 2018. [Google Scholar]
- Basaran, B.; Kalkan, I.; Aksoylu, C.; Özkılıç, Y.O.; Sabri, M.M.S. Effects of Waste Powder, Fine and Coarse Marble Aggregates on Concrete Compressive Strength. Sustainability 2022, 14, 14388. [Google Scholar] [CrossRef]
- Karalar, M.; Bilir, T.; Çavuşlu, M.; Özkiliç, Y.O.; Sabri, M.M.S. Use of Recycled Coal Bottom Ash in Reinforced Concrete Beams as Replacement for Aggregate. Front. Mater. 2022, 9, 1064604. [Google Scholar] [CrossRef]
- Karalar, M.; Özkılıç, Y.O.; Aksoylu, C.; Sabri, M.M.S.; Beskopylny, A.N.; Stel’Makh, S.A.; Shcherban, E.M. Flexural Behavior of Reinforced Concrete Beams Using Waste Marble Powder towards Application of Sustainable Concrete. Front. Mater. 2022, 9, 1068791. [Google Scholar] [CrossRef]
- Qaidi, S.; Al-Kamaki, Y.; Hakeem, I.; Dulaimi, A.F.; Özkılıç, Y.; Sabri, M.; Sergeev, V. Investigation of the Physical-Mechanical Properties and Durability of High-Strength Concrete with Recycled PET as a Partial Replacement for Fine Aggregates. Front. Mater. 2023, 10, 1101146. [Google Scholar] [CrossRef]
- Małek, M.; Jackowski, M.; Łasica, W.; Kadela, M. Influence of Polypropylene, Glass and Steel Fiber on the Thermal Properties of Concrete. Materials 2021, 14, 1888. [Google Scholar] [CrossRef]
- Małek, M.; Jackowski, M.; Łasica, W.; Kadela, M.; Wachowski, M. Mechanical and Material Properties of Mortar Reinforced with Glass Fiber: An Experimental Study. Materials 2021, 14, 698. [Google Scholar] [CrossRef]
- Mohajerani, A.; Vajna, J.; Cheung, T.H.H.; Kurmus, H.; Arulrajah, A.; Horpibulsuk, S. Practical Recycling Applications of Crushed Waste Glass in Construction Materials: A Review. Constr. Build. Mater. 2017, 156, 443–467. [Google Scholar] [CrossRef]
- Wright, J.R.; Cartwright, C.; Fura, D.; Rajabipour, F. Fresh and Hardened Properties of Concrete Incorporating Recycled Glass as 100% Sand Replacement. J. Mater. Civ. Eng. 2014, 26, 04014073. [Google Scholar] [CrossRef]
- Yousefi, A.; Tang, W.; Khavarian, M.; Fang, C.; Wang, S. Thermal and Mechanical Properties of Cement Mortar Composite Containing Recycled Expanded Glass Aggregate and Nano Titanium Dioxide. Appl. Sci. 2020, 10, 2246. [Google Scholar] [CrossRef]
- Shi, C.; Zheng, K. A Review on the Use of Waste Glasses in the Production of Cement and Concrete. Resour. Conserv. Recycl. 2007, 52, 234–247. [Google Scholar] [CrossRef]
- Elaqra, H.A.; Al-Afghany, M.J.; Abo-Hasseira, A.B.; Elmasry, I.H.; Tabasi, A.M.; Alwan, M.D. Effect of Immersion Time of Glass Powder on Mechanical Properties of Concrete Contained Glass Powder as Cement Replacement. Constr. Build. Mater. 2019, 206, 674–682. [Google Scholar] [CrossRef]
- Khan, Q.S.; Sheikh, M.N.; McCarthy, T.J.; Robati, M.; Allen, M. Experimental Investigation on Foam Concrete without and with Recycled Glass Powder: A Sustainable Solution for Future Construction. Constr. Build. Mater. 2019, 201, 369–379. [Google Scholar] [CrossRef]
- Elaqra, H.; Rustom, R. Effect of Using Glass Powder as Cement Replacement on Rheological and Mechanical Properties of Cement Paste. Constr. Build. Mater. 2018, 179, 326–335. [Google Scholar] [CrossRef]
- Omran, A.F.; Etienne, D.M.; Harbec, D.; Tagnit-Hamou, A. Long-Term Performance of Glass-Powder Concrete in Large-Scale Field Applications. Constr. Build. Mater. 2017, 135, 43–58. [Google Scholar] [CrossRef]
- Du, H.; Tan, K.H. Properties of High Volume Glass Powder Concrete. Cem. Concr. Compos. 2017, 75, 22–29. [Google Scholar] [CrossRef]
- Soliman, N.A.; Tagnit-Hamou, A. Development of Ultra-High-Performance Concrete Using Glass Powder—Towards Ecofriendly Concrete. Constr. Build. Mater. 2016, 125, 600–612. [Google Scholar] [CrossRef]
- Arabi, N.; Meftah, H.; Amara, H.; Kebaïli, O.; Berredjem, L. Valorization of Recycled Materials in Development of Self-Compacting Concrete: Mixing Recycled Concrete Aggregates—Windshield Waste Glass Aggregates. Constr. Build. Mater. 2019, 209, 364–376. [Google Scholar] [CrossRef]
- Bisht, K.; Ramana, P.V. Sustainable Production of Concrete Containing Discarded Beverage Glass as Fine Aggregate. Constr. Build. Mater. 2018, 177, 116–124. [Google Scholar] [CrossRef]
- Hendi, A.; Mostofinejad, D.; Sedaghatdoost, A.; Zohrabi, M.; Naeimi, N.; Tavakolinia, A. Mix Design of the Green Self-Consolidating Concrete: Incorporating the Waste Glass Powder. Constr. Build. Mater. 2018, 199, 369–384. [Google Scholar] [CrossRef]
- Rashid, K.; Hameed, R.; Ahmad, H.A.; Razzaq, A.; Ahmad, M.; Mahmood, A. Analytical Framework for Value Added Utilization of Glass Waste in Concrete: Mechanical and Environmental Performance. Waste Manag. 2018, 79, 312–323. [Google Scholar] [CrossRef] [PubMed]
- Fathi, H.; Lameie, T.; Maleki, M.; Yazdani, R. Simultaneous Effects of Fiber and Glass on the Mechanical Properties of Self-Compacting Concrete. Constr. Build. Mater. 2017, 133, 443–449. [Google Scholar] [CrossRef]
- Du, H.; Tan, K.H. Waste Glass Powder as Cement Replacement in Concrete. J. Adv. Concr. Technol. 2014, 12, 468–477. [Google Scholar] [CrossRef]
- Zeybek, Ö.; Özkılıç, Y.O.; Karalar, M.; Çelik, A.İ.; Qaidi, S.; Ahmad, J.; Burduhos-Nergis, D.D.; Burduhos-Nergis, D.P. Influence of Replacing Cement with Waste Glass on Mechanical Properties of Concrete. Materials 2022, 15, 7513. [Google Scholar] [CrossRef] [PubMed]
- Qaidi, S.; Najm, H.M.; Abed, S.M.; Özkılıç, Y.O.; Al Dughaishi, H.; Alosta, M.; Sabri, M.M.S.; Alkhatib, F.; Milad, A. Concrete Containing Waste Glass as an Environmentally Friendly Aggregate: A Review on Fresh and Mechanical Characteristics. Materials 2022, 15, 6222. [Google Scholar] [CrossRef] [PubMed]
- Çelik, A.İ.; Özkılıç, Y.O.; Zeybek, Ö.; Karalar, M.; Qaidi, S.; Ahmad, J.; Burduhos-Nergis, D.D.; Bejinariu, C. Mechanical Behavior of Crushed Waste Glass as Replacement of Aggregates. Materials 2022, 15, 8093. [Google Scholar] [CrossRef] [PubMed]
- Guo, S.; Dai, Q.; Sun, X.; Xiao, X. X-ray CT Characterization and Fracture Simulation of ASR Damage of Glass Particles in Alkaline Solution and Mortar. Theor. Appl. Fract. Mech. 2017, 92, 76–88. [Google Scholar] [CrossRef]
- Cota, F.P.; Melo, C.C.D.; Panzera, T.H.; Araújo, A.G.; Borges, P.H.R.; Scarpa, F. Mechanical Properties and ASR Evaluation of Concrete Tiles with Waste Glass Aggregate. Sustain. Cities Soc. 2015, 16, 49–56. [Google Scholar] [CrossRef]
- Shao, Y.; Lefort, T.; Moras, S.; Rodriguez, D. Studies on Concrete Containing Ground Waste Glass. Cem. Concr. Res. 2000, 30, 91–100. [Google Scholar] [CrossRef]
- Sanchez, L.F.M.; Fournier, B.; Jolin, M.; Mitchell, D.; Bastien, J. Overall Assessment of Alkali-Aggregate Reaction (AAR) in Concretes Presenting Different Strengths and Incorporating a Wide Range of Reactive Aggregate Types and Natures. Cem. Concr. Res. 2017, 93, 17–31. [Google Scholar] [CrossRef]
- Saccani, A.; Bignozzi, M.C. ASR Expansion Behavior of Recycled Glass Fine Aggregates in Concrete. Cem. Concr. Res. 2010, 40, 531–536. [Google Scholar] [CrossRef]
- Gorospe, K.; Booya, E.; Ghaednia, H.; Das, S. Effect of Various Glass Aggregates on the Shrinkage and Expansion of Cement Mortar. Constr. Build. Mater. 2019, 210, 301–311. [Google Scholar] [CrossRef]
- Ling, T.C.; Poon, C.S. A Comparative Study on the Feasible Use of Recycled Beverage and CRT Funnel Glass as Fine Aggregate in Cement Mortar. J. Clean. Prod. 2012, 29–30, 46–52. [Google Scholar] [CrossRef]
- Yu, X.; Tao, Z.; Song, T.Y. Effect of Different Types of Aggregates on the Performance of Concrete-Filled Steel Tubular Stub Columns. Mater. Struct. 2015, 49, 3591–3605. [Google Scholar] [CrossRef]
- Thumrongvut, J.; Seangatith, S.; Siriparinyanan, T.; Wangrakklang, S. An Experimental Behaviour of Cellular Lightweight Concrete-Filled Steel Square Tube Columns under Axial Compression. Mater. Sci. Forum 2016, 860, 121–124. [Google Scholar] [CrossRef]
- Han, L.H.; Li, W.; Bjorhovde, R. Developments and Advanced Applications of Concrete-Filled Steel Tubular (CFST) Structures: Members. J. Constr. Steel Res. 2014, 100, 211–228. [Google Scholar] [CrossRef]
- Guo, P.; Meng, W.; Nassif, H.; Gou, H.; Bao, Y. New Perspectives on Recycling Waste Glass in Manufacturing Concrete for Sustainable Civil Infrastructure. Constr. Build. Mater. 2020, 257, 119579. [Google Scholar] [CrossRef]
- GB/T 50080-2016; Standard for Test Method of Performance on Ordinary Fresh Concrete. China Architecture and Building Press: Beijing, China, 2016.
- GB/T 50081-2002; Standard for Test Method of Mechanical Properties on Ordinary Concrete. China Architecture and Building Press: Beijing, China, 2002.
- Wang, F.; Young, B.; Gardner, L. Compressive Testing and Numerical Modelling of Concrete-Filled Double Skin CHS with Austenitic Stainless Steel Outer Tubes. Thin-Walled Struct. 2019, 141, 345–359. [Google Scholar] [CrossRef]
- Taha, B.; Nounu, G. Utilizing Waste Recycled Glass as Sand/Cement Replacement in Concrete. J. Mater. Civ. Eng. 2009, 21, 709–721. [Google Scholar] [CrossRef]
- Aslam, F.; Zaid, O.; Althoey, F.; Alyami, S.H.; Qaidi, S.M.A.; de Prado Gil, J.; Martínez-García, R. Evaluating the Influence of Fly Ash and Waste Glass on the Characteristics of Coconut Fibers Reinforced Concrete. Struct. Concr. 2022. [Google Scholar] [CrossRef]
- Ali, E.E.; Al-Tersawy, S.H. Recycled Glass as a Partial Replacement for Fine Aggregate in Self Compacting Concrete. Constr. Build. Mater. 2012, 35, 785–791. [Google Scholar] [CrossRef]
- Terro, M.J. Properties of Concrete Made with Recycled Crushed Glass at Elevated Temperatures. Build. Environ. 2006, 41, 633–639. [Google Scholar] [CrossRef]
- Tan, K.H.; Du, H. Use of Waste Glass as Sand in Mortar: Part I—Fresh, Mechanical and Durability Properties. Cem. Concr. Compos. 2013, 35, 109–117. [Google Scholar] [CrossRef]
- De Castro, S.; De Brito, J. Evaluation of the Durability of Concrete Made with Crushed Glass Aggregates. J. Clean. Prod. 2012, 41, 7–14. [Google Scholar] [CrossRef]
- Shi, C.; Wu, Y.; Riefler, C.; Wang, H. Characteristics and Pozzolanic Reactivity of Glass Powders. Cem. Concr. Res. 2005, 35, 987–993. [Google Scholar] [CrossRef]
- Ma, D.Y.; Han, L.H.; Zhao, X.L. Seismic Performance of the Concrete-Encased CFST Column to RC Beam Joint: Experiment. J. Constr. Steel Res. 2018, 154, 134–148. [Google Scholar] [CrossRef]
- Xue, J.Q.; Briseghella, B.; Chen, B.C. Effects of Debonding on Circular CFST Stub Columns. J. Constr. Steel Res. 2012, 69, 64–76. [Google Scholar] [CrossRef]
- ANSI/AISC 360-16; Specification for Structural Steel Buildings. American Institute of Steel Construction: Chicago, IL, USA, 2016.
- EN 1994-1-1; Eurocode 4: Design of Composite Steel and Concrete Structures—Part 1.1: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2004.
- GB 50936-2014; Technical Code for Concrete Filled Steel Tubular Structures. China Architecture and Building Press: Beijing, China, 2014.

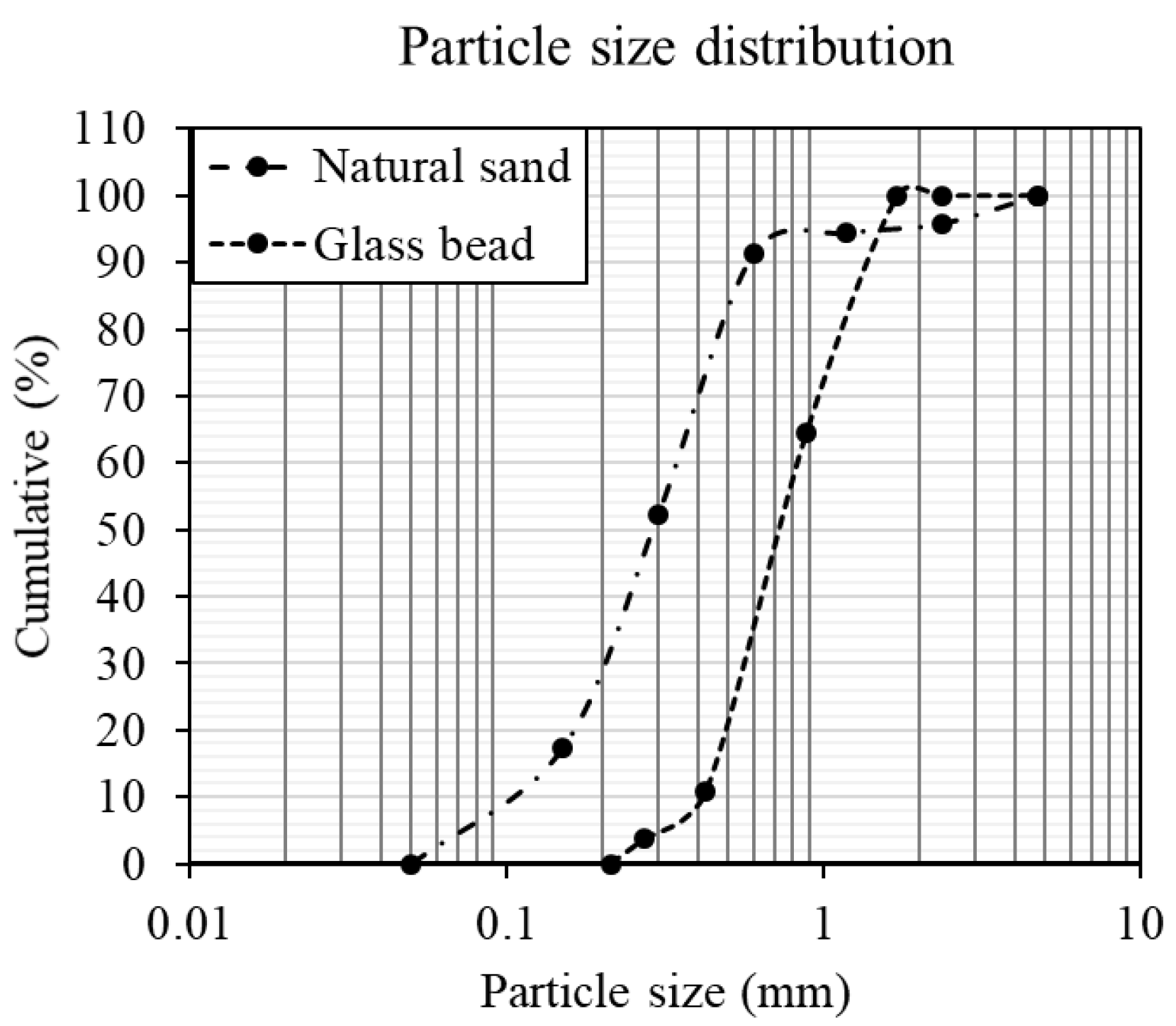



| Mix ID | Replacement Ratio | Cement | Water | Natural Sand | Aggregate | Glass Powder | Glass Beads | Superplasticizer |
|---|---|---|---|---|---|---|---|---|
| OC | 0 | 450 | 165 | 680 | 1105 | 0 | 0 | 1.29 |
| GC10 | 10 | 405 | 165 | 612 | 1105 | 45 | 68 | 1.29 |
| GC15 | 15 | 383 | 165 | 578 | 1105 | 68 | 102 | 1.29 |
| GC20 | 20 | 360 | 165 | 544 | 1105 | 90 | 136 | 1.29 |
| GC30 | 30 | 315 | 165 | 476 | 1105 | 135 | 204 | 1.29 |
| Specimen ID | Number of Specimens | Glass Replacement Ratio (%) | Dimension of Steel Tube | Dimension of Concrete Core |
|---|---|---|---|---|
| OCFST | 3 | 0 | 400 × 133 × 4.75 | 400 × 123.5 |
| GCFST-10 | 3 | 10 | 400 × 133 × 4.75 | 400 × 123.5 |
| GCFST-15 | 3 | 15 | 400 × 133 × 4.75 | 400 × 123.5 |
| GCFST-20 | 3 | 20 | 400 × 133 × 4.75 | 400 × 123.5 |
| GCFST-30 | 3 | 30 | 400 × 133 × 4.75 | 400 × 123.5 |
| Specimen ID | (kN) | Residual Bearing (kN) | Deflection at Peak Load Δp (mm) | Ultimate Deflection at the Failure of Specimen Δu (mm) | ||
|---|---|---|---|---|---|---|
| OCFST | 1398.8 | 1301.7 | 17.9 | 39.6 | 2.2 | 2.096 |
| GCFST-10 | 1376.6 | 1343.3 | 22.9 | 39.0 | 1.7 | 2.059 |
| GCFST-15 | 1325.0 | 1318.1 | 23.1 | 34.6 | 1.5 | 2.288 |
| GCFST-20 | 1260.2 | 1228.7 | 22.3 | 35.6 | 1.6 | 2.990 |
| GCFST-30 | 1297.3 | 1277.2 | 26.9 | 36.1 | 1.3 | 3.017 |
| Test | AISC | EC4 | GB50936-2014 | |
|---|---|---|---|---|
| Equation | -- | For compact circular CFST: | For circular CFST: | For circular CFST: |
| OCFST | 1398.8 | 934.3 | 1250.4 | 1129.6 |
| GCFST-10 | 1376.6 | 938.8 | 1254.7 | 1136.6 |
| GCFST-15 | 1325.0 | 909.5 | 1226.6 | 1097.7 |
| GCFST-20 | 1260.2 | 847.6 | 1167.3 | 1020.8 |
| GCFST-30 | 1297.3 | 845.3 | 1165.1 | 1018.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Diao, Y.; Chen, L.; Huang, Y. Experimental Study on Mechanical Properties of Concrete Containing Waste Glass and Its Application on Concrete-Filled Steel Tubular Columns. Processes 2023, 11, 975. https://doi.org/10.3390/pr11040975
Diao Y, Chen L, Huang Y. Experimental Study on Mechanical Properties of Concrete Containing Waste Glass and Its Application on Concrete-Filled Steel Tubular Columns. Processes. 2023; 11(4):975. https://doi.org/10.3390/pr11040975
Chicago/Turabian StyleDiao, Yan, Long Chen, and Yitao Huang. 2023. "Experimental Study on Mechanical Properties of Concrete Containing Waste Glass and Its Application on Concrete-Filled Steel Tubular Columns" Processes 11, no. 4: 975. https://doi.org/10.3390/pr11040975
APA StyleDiao, Y., Chen, L., & Huang, Y. (2023). Experimental Study on Mechanical Properties of Concrete Containing Waste Glass and Its Application on Concrete-Filled Steel Tubular Columns. Processes, 11(4), 975. https://doi.org/10.3390/pr11040975






