1. Introduction
The growing demand for energy and the problems in everyday life caused by the excessive use of traditional energy sources (fossil fuels) have recently caused researchers to look for renewable energy sources and their improvements. This may be achieved by improving the methods of using renewable energy sources and technologies that do not require fossil fuel and by trying to recover some residual energy from thermal processes from industrial plants, cars, and other mobile sources.
When an electric current flows through a thermoelectric generator (TEG), it becomes a heat pump, which transports thermal energy from one side to another: the direction and magnitude of the heat depend on the electric current strength. This phenomenon is known as the Peltier effect [
1,
2]. The efficiency of a TEG is determined by several factors, including the geometric leg shape of the TEG, the cooling used, and the materials used [
3,
4].
The leg structure is a critical factor in determining the efficiency of a TEG. Therefore, some research has been conducted on TEGs for leg structure optimization [
5,
6,
7,
8]. These studies have shown that leg structures of the thermoelectric module significantly influence the device’s behavior. Therefore, the leg structure should be designed to maximize the temperature gradient across the TEG. The following summarizes the main results in improving a TEG’s efficiency by optimizing the leg structures.
Chen et al. [
9] theoretically compared square leg structures with different leg structure dimensions of a solar thermoelectric generator and analyzed the output power and efficiency. The change in the thermal concentration ratio (cross-sectional area divided by substrate area), substrate, and temperature-dependent properties led to an improvement in the performance of the solar thermoelectric generator.
Li et al. [
10] investigated a thermoelectric generator’s performance, including thermal, mechanical, economic, and exergetic analyses. They examined how hollow cross-sectional leg structures of TEG legs can improve performance and identified the optimal conditions for each case.
Lamba and Kaushik [
11] studied a thermoelectric generator (TEG) under the Thompson effect and the leg structure configuration. Using a trapezoidal form, they performed a thermodynamic analysis to evaluate its performance. The conversion energy and exergy efficiency improved by increasing the leg structure parameter but not improving the output power.
Olivares-Robles et al. [
12] studied a segmented thermoelectric microgenerator with high-performance nanostructured materials. They considered thermoelements with symmetric and asymmetric leg structures. For a 2p-3n segmented trapezoidal leg structure, it was found that efficiency and output power were improved compared to the conventional rectangular leg structure by 6% and 7%, respectively.
Thimont and LeBlanc [
13] found a way to make singular leg structures to optimize thermoelectric device behavior through additive manufacturing in which free-form leg structures are built layer by layer. Their study included the analysis of hollow and prismatic leg structures to find the optimal leg structure design, resulting in higher temperature gradients for hollow and prismatic leg structures, which caused better heat transfer and, therefore, better efficiency.
Zhao et al. [
14] performed an energy and exergy analysis on a thermoelectric generator and examined how gas humidification can improve its performance compared to combustion gas generation.
Wiriyasart et al. [
15] found that composite fluids enhance the Peltier effect by decreasing the Fourier effect bestowal of the thermoelectric cooler. Therefore, they experimented with a compressed water sink thermoelectric cooling (TEC) module with water, and water solutions of a ferrofluid (
Fe3O4) and a nanofluid (
TiO2) with concentrations of 0.005% and 0.015%, respectively, to find that
Fe3O4 had a maximum heat transfer rate of 11.17% and 12.57% compared to
TiO2 and water, respectively.
The cooling method also affects the efficiency of a TEG. The best cooling method removes heat from the TEG as quickly as possible. The use of nanofluids is a promising method for improving the efficiency of TEGs because the nanoparticles in nanofluids increase the thermal conductivity of the base fluid, which can enhance the heat transfer rate in a TEG.
Pfeiffelman et al. [
16] studied passive and active cooling and how it affected the performance of a TEG. They concluded that water offers better conditions than air.
Yang et al. [
17] studied the different forms of cooling of an annular concentric thermoelectric generator. To improve and analyze the TEG’s performance, they used water-cooling, concurrent air-cooling, countercurrent water-cooling, and countercurrent air-cooling as refrigerants. The maximum net power
432.42 W was obtained using countercurrent water-cooling, corresponding to an optimal thermoelectric semiconductor volume of 9.06 × 10
−4.
Olivares-Robles et al. [
18] studied the cooling of graphene nanoplatelet aqueous nanofluids (GNAN) in a solar thermoelectric generator, using five different leg structures (three square and two trapezoidal) and several concentrations of the nanofluid to find the optimal leg structure design. They concluded that using GNAN improves up to 15% of the solar thermoelectric generator performance, and efficiency also depends on the leg dimensions.
Abdelkareem et al. [
19] sought to take advantage of the residual heat of a TEG; recovering this energy determines the performance of the TEG. Nanofluids were found to significantly improve heat transfer (the Seebeck effect is enhanced by reducing the contribution of the Fourier effect), allowing greater efficiency in their applications.
Ahmed et al. [
20] used
Al2O3 with a weight dispersion of 0.1 and 0.2 vol.% as a coolant on the hot side of a thermoelectric cooler (TEC), showing a 40% improvement in the coefficient of performance TEC for concentrations of 0.2 vol.%.
He Ruan et al. [
21] performed an analysis and performance comparison between a TEG and a TEC using graphene,
Al2O3, and CuO nanofluids. They concluded that using nanofluids improves the TEC’s performance coefficient more than the efficiency of the TEG.
Xing et al. [
22] showed that graphene nanoplatelets could reduce nanofluid deposition and standardization. The simulation used thermoelectric and liquid modules in an Ansys Workbench environment. GNAN was used as a coolant on the TEG’s cold side to achieve a better heat transfer. The results showed that the heat, efficiency, output power, and voltage of the hot surfaces of the TEG system were increased by the liquid nano coolant.
Chen et al. [
23] studied and compared the differences between microfluid, multifluid, and nanofluid and the applications that can be given to each; finding the different applications each can have and how they improve the performance or manufacturing of devices.
Sundar et al. [
24] studied the effective thermal conductivity and viscosity of the magnetized nanofluid Fe
3O
4/water. The thermal conductivity and viscosity of the nanofluid increased with a rise in particle concentration. However, the viscosity improvement was more significant than the improvement in thermal conductivity at the same volume concentration and temperature.
Kaushik and Manikandan [
25] investigated the exo-reversible thermodynamic model of an annular thermoelectric generator (ATEG) and considered the Thompson, Peltier, Fourier, and Joule effects using an exergy analysis. They concluded that an ATEG has a lower efficiency to that of a TEG.
Tian et al. [
26] proposed the segmented annular thermoelectric generator as an efficient structure. Although it has not been commercialized yet, they concluded that the segmented model is better than a conventional one regarding efficiency and power output.
Kedher et al. [
27] studied the combined effects of using a rotating cylinder and a shear-thinning nanofluid to improve the performance of a cabinet installed with a TEG with multiple ventilation ports. They used two nanofluids, which they called NF1 and NF2. They found that using NF1 improved behavior by up to 49%, and when using NF2, it improved by up to 61%.
Various studies on TEGs are focused on applications such as automotive, aerospace, industrial, and everyday life [
28,
29,
30].
Other studies [
31,
32,
33,
34,
35,
36] seek to improve the performance of a thermoelectric generator from different approaches, such as mechanical stress analysis, hybridization of the system, and the use of phase change materials (PCM).
The optimization of the leg structure, the use of nanofluids, and the materials used are all promising methods for improving the efficiency of TEGs. The optimization of these factors can lead to significant improvements in the efficiency of TEGs, which can make them a more viable option for renewable energy generation.
The novelty of this work lies in the comprehensive performance analysis of a TEG using ANSYS simulation. This study considers the leg structure configuration and the impact of nanofluids on the TEG to obtain the optimal leg structure. The output power, efficiency, and exergy efficiency results are presented as a function of heat flux and area ratio for water and various nanofluids. Additionally, this study compares the results with different segmentation methods. Overall, this work provides insights into the design optimization of TEGs for enhanced performance and efficiency.
In this work, in
Section 2, performance analysis of a TEG is conducted via ANSYS simulation, where the leg structures, material parameters of cooling liquids, and governing equations are the input quantities. The results for the output power, efficiency, and exergy efficiency as a function of the heat flux and area ratio for water and various nanofluids are presented in
Section 3, and the results are compared with different segmentations. Our results are obtained by considering the leg structure configuration and the influence of nanofluids on the TEG to obtain the optimal leg structure. Finally, in
Section 4, the conclusions are presented.
2. Methods and Materials
2.1. Physical Model and Assumptions
The results of our study are compared with previous results by employing computational analysis.
Parameters such as the coordinate system, material properties, and input parameters are set to compare the established leg structures and analyze their effect on TEG performance.
This study investigates the effect of various cooling nanofluids on the thermal performance of a TEG with triangular hollow/filled leg structures. The nanofluid coolants considered for improving TEG efficiency are distilled water, graphene, Al2O3, TiO2, and Fe3O4 at a weight concentration of 0.1%-wt.
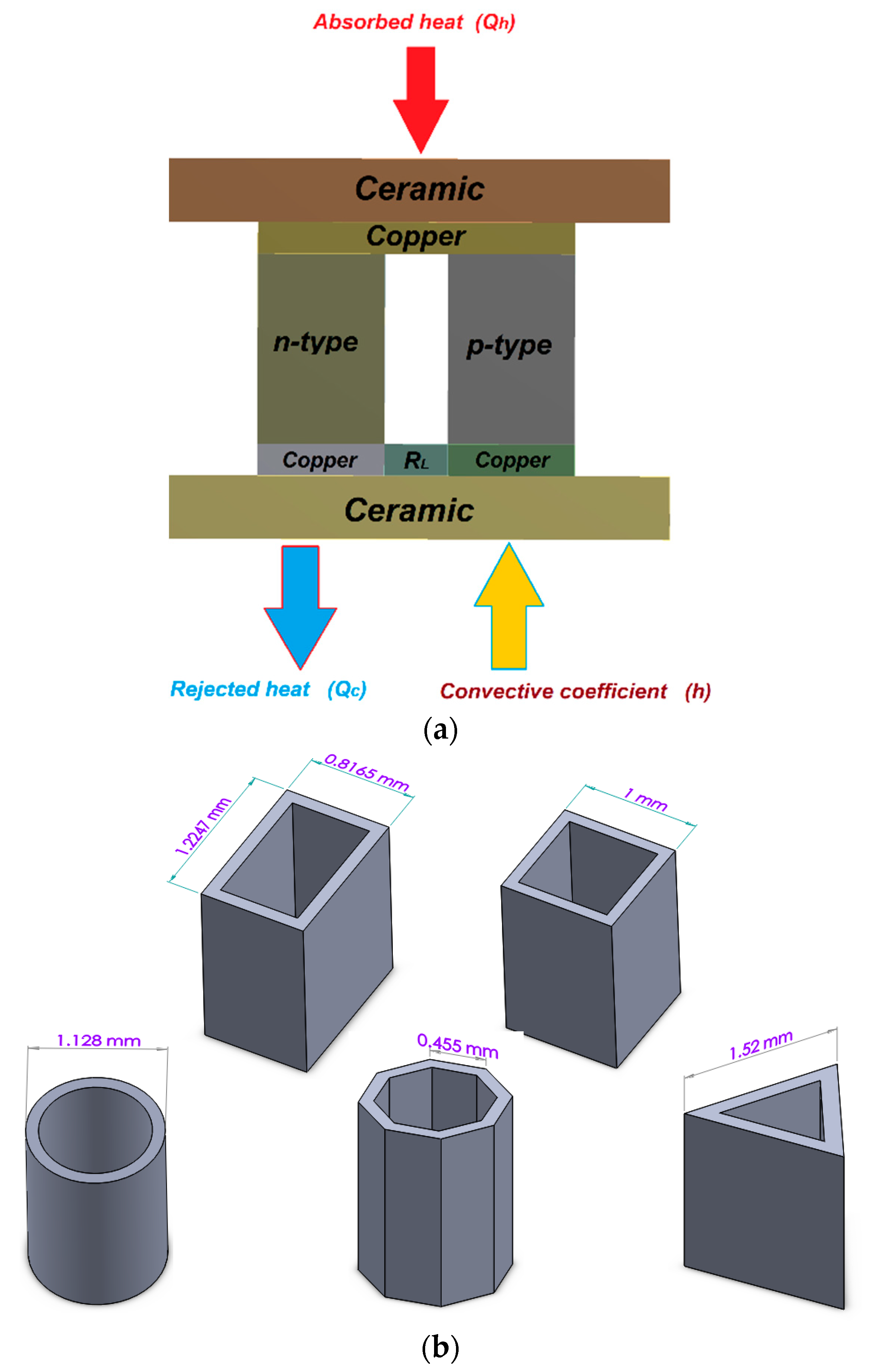
The model of a TEG is composed of two semiconductor legs (p-type and n-type) connected with copper and two layers of ceramic insulation that are bonded to the hot and cold sides of the thermoelectric generator. The scheme of the thermoelectric generator and the leg structures considered in this work are shown in
Figure 1. In addition, the dimensions of the study by Min Li et al. [
10] are considered in our study.
The model includes thin solder layers between the legs, and the thermal analysis considers the copper interconnections.
Figure 1b shows the five different hollow leg structures considered, namely, square, rectangular, octagonal, circular, and triangular, with their corresponding sizes.
A part of the cross-sectional area is removed from the semiconductors’ inside, forming ring-shaped legs. The leg structure cross-sectional area base () is between 1 and 2 mm2.
The ratio of the interior hole area to the total area (
) for all leg structure configurations is 0.5 (unless otherwise stated). Therefore, the height of all the legs used is 1.5 mm.
Bi2Te3 is selected as the material for the n-type and p-type semiconductors. A detailed scheme of thermoelectric legs in a ring-shaped structure is shown in
Figure 1b [
10]. In addition, temperature-dependent properties of
Bi2Te3 were considered to increase the accuracy of the simulations. The analysis included the elastoplastic behavior of the carrier layers and copper interconnections because it has a relaxing effect leading to reduced thermal stress on the thermoelectric legs. In addition, the analysis considers the Thompson effect, but the temperature dependence of electrical and thermal resistance is constant.
The best materials for TEGs are those with a high Seebeck coefficient and a low thermal conductivity. The most common materials used in TEGs are bismuth telluride, lead telluride, and silicon germanium. We use
PbTe and
Bi2Te3 as semiconductor materials, p-type, and n-type.
Figure 2 shows the properties of the materials dependent on temperature (Thermal conductivity,
Figure 2a; Seebeck coefficient,
Figure 2b; Electrical resistivity,
Figure 2c; and Merit figure,
Figure 2d).
PbTe and Bi2Te3 are two common materials used in the construction of segmented thermoelectric generators. The behavior of these materials in different temperature ranges is critical to their efficiency and performance.
PbTe is a narrow-bandgap semiconductor material with a high thermoelectric figure of merit (Image), in the mid-temperature range between 600 and 800 K. PbTe has a relatively high melting point and is chemically stable, making it an excellent choice for high-temperature applications. However, PbTe has low ZT values at low temperatures, and its performance decreases at temperatures above 900 K due to the formation of undesirable secondary phases. Therefore, PbTe is typically used in segmented structures, where the temperature range can be optimized to take advantage of its high ZT values.
On the other hand, Bi2Te3 is a wide-bandgap semiconductor material with a high ZT value in the low-temperature range between 300 and 400 K. Bi2Te3 is less chemically stable than PbTe, but its performance remains relatively constant over a wide temperature range. However, Bi2Te3 has a low melting point, which limits its use in high-temperature applications.
Simple hollow and layered leg geometries can result in larger temperature gradients and higher output power than the traditional cuboid structure. This dependence on thermal resistance and power output on leg geometry offers a compelling rationale for investigating additive manufacturing techniques for thermoelectric devices.
The leg structure significantly affects the temperature difference and electrical potential across the leg. Compared to their filled counterparts, the heat transfer rate is reduced in the hollow and layered geometries, resulting in higher effective thermal resistance. The increase in thermal resistance for the hollow structure is primarily due to the impact of the leg geometry on the thermal resistance. These thermal trends also extend to the open circuit voltage developed across each leg geometry, as the electrical potential is directly related to the temperature gradient.
Therefore, segmented structures made from both materials can be designed to take advantage of their specific temperature ranges, resulting in a higher overall efficiency for the thermoelectric generator.
2.2. Numerical Method and System Performance
Figure 3 shows the mesh distribution that was made for the TEG.
The performance analysis of a TEG in the stationary state is carried out with the thermoelectric module of the ANSYS Workbench. In addition, a network independence test is carried out to minimize the uncertainty of the simulations. Voltage values for various total elements tested are shown in
Table 1.
Semiconductors, interconnections, insulators, and grip layer properties are listed in
Table 2 and
Table 3. K,
, R, and
are the thermal conductivity, Seebeck coefficient, electrical resistance, and coefficient of thermal expansion, respectively.
2.3. Governing Equations
The governing equations are solved by Galerikin’s finite element method, which is a numerical approximation method [
37].
The following equations governing the system and relating the thermal and electric fields define the thermoelectric material’s behavior.
where α is the Seebeck coefficient,
T is the temperature,
is the electric field,
is the vector current density, and
Ρe and
k are the thermoelement’s resistivity and thermal conductivity [
18].
The physical scales of temperature, T, and electric scalar potential,
, over a computational element are approximated by:
where
N,
Te, and
are the element shape function, the nodal temperature vector, and the nodal electrical potentials vector, respectively.
Integrating Equations (1) and (2), the differential equations can be written algebraically:
Where
and
are the thermal load vector and the electric current load vector, respectively.
,
, and
are the thermal stiffness matrix, the Seebeck stiffness matrix, and the electric stiffness matrix, respectively, and can be defined as:
The equations are solved using the commercial software ANSYS. Then, the calculations are repeated until the convergence criteria are satisfied. Convergence is defined when the relative temperature and electric potential differences between two iterations are less than 10−6; this minimal difference is enough to ensure a stable simulation according to some numerical reference.
The heat flow equation for thermoelements is as follows:
where
is the open-circuit voltage,
is the Seebeck coefficient,
is the temperature difference between the cold side and the hot side,
RL is the load resistance, and
is the output power.
The equations used to calculate the efficiency and exergy are the following [
10]:
where
is the energy efficiency,
is the room temperature,
Th is the temperature of the hot side,
is the absorbed heat from the hot side,
is the exergy used to generate the power [
14], and
is the exergy efficiency.
2.4. Boundary Conditions
The boundary conditions established for the analysis of these models are the temperature of the cold side, which is set at 298.15 K, and the temperature of the hot side, which varies from 325.15 K to 548.15 K, having a . Voltages of 0 V and 0.08 V are established on the n-type and p-type semiconductor copper electrodes, respectively.
Equations (1) and (2) can be solved simultaneously using the three-dimensional space finite element method. With Equations (1), (2), and (9) we can find the equations for heat transfer from the hot side (Q
h) and the cold side (Q
c).
where K is the thermal conductance, R is the electrical resistance,
is the electrical current,
is the temperature of the hot side, and T
c is the temperature of the cold side.
The Fourier heat, Peltier heat, and contact Joule heat are described in Equations (15) and (16). According to basic thermoelectric theories:
The cold side is at the bottom of the TEG (25 °C) for the cooling process, while the heat is transferred from the hot side. Therefore, the ambient temperature (T0) was 20 °C.
2.5. TEG and Nanofluid System
This study analyzes the improvement of thermal cooling on the cold side of thermoelectric cooling for a segmented TEG. Water,
TiO2 nanofluid, graphene,
Al2O3, and
Fe3O4 ferrofluid with 0.1% concentration were selected because they are the refrigerants already studied in previous literature [
15,
18,
19,
20]. The properties of nanofluids (
Table 4) significantly influence the improvement of heat transfer. Viscosity is one of the most important properties to maintain optimal performance of the TEG because if the concentration of nanoparticles in the nanofluid is increased, it would be more viscous and would impair heat transfer since the refrigerant would not flow properly and would overheat.
For simulation purposes, a convective boundary condition based on the thermophysical properties of the nanofluid is established on the cold side of the TEG, as shown in
Figure 1a.
The nanofluid passes through the bottom of the cold side of the TEG. Therefore, the area where nanofluid passes is assumed to be equal to the substrate area of the TEG.
Suppose the refrigerant is detected in the laminar flow development stages, and the influence of different physical properties is negligible. In this case, the Zader–Tate equation can describe the Nusselt number with a distance of 0.052 m [
18].
where
is the Prandtl number,
is the Reynolds number, and
is the equivalent diameter. In those equations,
is the kinematic viscosity,
is the flow,
is the viscosity,
is the thermal conductivity,
is the wet perimeter, and
is the heat transfer coefficient.
Heat loss from semiconductors and thermal resistances in the cooling tube and ceramic plates are ignored. The convective values are added to the input parameters for simulation purposes, and a convective boundary condition is established based on the nanofluid’s thermophysical properties. We will use graphene,
Al2O3,
TiO2, and
Fe3O4 dispersed in a fluid. In particular, the hollow triangular leg structure was also cooled with nanofluids’ weight dispersion of 0.1%-wt.
Table 4 shows the properties of the nanofluids used. The weight concentration in nanofluids is 0.1%. The dispersion stability of nanofluids is not considered in the numerical analysis.
Nanoparticles such as TiO2, graphene, and alumina are commonly used as coolants in thermoelectric generators because they have high thermal conductivity and can enhance the heat transfer performance of the cooling fluid. In addition, these nanoparticles have unique properties that can improve the performance of the thermoelectric generator.
For example, has a high thermal conductivity and is chemically stable, making it an excellent choice for improving the heat transfer performance of the coolant. Graphene has high electrical conductivity, high mechanical strength, and a large surface area, which can enhance the material’s thermoelectric properties. Alumina has a high thermal conductivity and is also chemically stable, making it suitable for use as a coolant in harsh environments.
Overall, the choice of nanoparticle coolant depends on various factors, including the specific application, the thermoelectric materials used, and the desired performance of the thermoelectric generator.
2.6. Validation Method: Performance of TEG with Distilled Water Cooling
Using output parameters that the ANSYS Workbench gives as a result of the simulations and Equations (11) and (12), the output power and conversion efficiency are calculated as a function of the heat flow in the TEG.
Figure 4a shows the previously published results by the Min Li methodology [
10] for method validation.
Figure 4b shows the thermodynamic performance of the TEG with filled leg structures. The numerical simulation shows that hollow forms offer better performance than filled ones under the following conditions: cold side temperature is fixed at 25 °C, load resistance of 0.0212 Ω, and it is stationary for the simulation. In addition, the triangular leg structure has higher power output and energy conversion for all heat flows. Therefore, the triangle leg structure is the best choice under the given conditions.
The best leg structure is the triangular one, and the hollow leg structures present greater power output than filled leg structures.
Table 5 shows all hollow and filled leg structures’ output power, efficiency, and maximum exergy efficiency values.
In particular, the exergy efficiency of a TEG is a function of the output power and , as defined by Equations (11) and (12).
The triangular leg structure also provides a higher exergy efficiency in function of the heat flux. However, when the cold side temperature rises, all the leg structures (except the square one) give approximately the same exergy efficiency values.
All curves show a monotonic value increase except for the triangular leg, which presents a maximum of 12 kW/m2 and 74.5 kW/m2 for hollow and filled legs. This maximum value may be significant for TEG design. Remarkably, the increment rate of output power (due to the increment of heat flux) in the triangular leg structure is sharper compared to the other leg structures, which shows the suitability of the triangular leg structure for higher-range heat fluxes.
Exergy efficiency converges for high values of heat flux, which is important for design criteria and limits the operation of thermoelements in the TEG.
Regarding the improved performance of hollow leg structures over filled ones, there are several reasons:
Reduced thermal conductivity: Hollow leg structures have lower thermal conductivity compared to filled leg structures. This lower thermal conductivity reduces heat transfer through the legs, allowing for better temperature gradients and improved energy conversion.
Minimized parasitic heat losses: Hollow leg structures provide a barrier between the hot and cold sides of the thermoelectric generator, reducing parasitic heat losses. By minimizing heat transfer to the surroundings, more heat can be utilized for power generation, resulting in improved performance.
Increased surface area: The presence of the hollow cavity in the leg structure increases the effective surface area available for heat exchange. This larger surface area facilitates better heat dissipation and more efficient heat transfer, contributing to improved overall performance.
Overall, the triangular leg structure offers advantages such as enhanced heat transfer, reduced thermal resistance, improved electrical potential, while the hollow leg structures provide benefits such as reduced thermal conductivity and minimized parasitic heat losses. These factors collectively contribute to the superior power output, energy conversion, and exergy efficiency observed in the triangular leg structure and the hollow leg structures, respectively.
3. Results
For this study, what is of interest are the steady-state results, so a transient-state analysis or study was not considered.
In this section, we begin showing our results for the best hollow leg structure of the thermoelectric legs proposed by Min Li [
10] but cooled with different nanofluids.
Figure 5 shows the temperature distribution for the triangular leg structure with 16 thermoelements. Similar behavior is obtained for the other leg structures. The ambient temperature is the cold side temperature (298 K) up to 540 K for the hot side.
3.1. Performance of the Thermoelectric Generator with Hollow Triangular Legs with Different Cooling Nanofluids
The triangular shape is often used in thermoelectric generators because it allows for efficient heat transfer and reduces thermal resistance, improving performance compared to other geometries.
In a thermoelectric generator, electricity is produced by exploiting the temperature difference between the hot and cold sides of the device. The efficiency of this process depends on how effectively heat can be transferred from the hot side to the cold side.
In a triangular shape, the triangle’s base serves as the hot side, while the two sloping sides form the cold side. This design allows for a larger surface area on the cold side, increasing the heat transfer rate and reducing thermal resistance. In addition, the sloping sides of the triangle allow for more uniform temperature distribution, further improving performance.
The triangular leg structure in a thermoelectric generator has been found to achieve better power output, energy conversion, and exergy efficiency compared to other leg structures for several reasons [
10,
13,
14]:
Enhanced heat transfer: The triangular leg structure provides increased surface area for heat transfer compared to other geometries such as square, circular, octagonal, or rectangular. This larger surface area facilitates better heat dissipation, resulting in improved thermal performance and higher power output.
Reduced thermal resistance: The triangular leg structure has a lower thermal resistance compared to other leg structures. This reduced resistance allows for more efficient heat flow through the legs, minimizing heat losses and maximizing energy conversion.
Improved electrical potential: The triangular leg structure promotes a larger temperature gradient across the legs, which directly relates to the electrical potential difference. This enhanced temperature gradient leads to higher voltage generation, contributing to better power output and energy conversion.
Other geometries, such as rectangular or cylindrical shapes, may not have as large a surface area on the cold side, leading to higher thermal resistance and reduced efficiency. However, it is worth noting that the optimal geometry for a thermoelectric generator will depend on the specific application and operating conditions.
Figure 6 shows the output power as a function of heat flux. Notice that
TiO2 was the nanofluid that presented the best results, although not by a relevant difference compared to
Al2O3. The maximum power output of the TEG using
Al2O3 is 0.407 W, while
TiO2 is 0.41 W, and
Fe3O4 is 0.393 W at 17 kW/m
2. The output power increase using nanofluids compared to water is 15%, 16.53%, 17.32%, and 12.57% using graphene,
Al2O3,
TiO2, and
Fe3O4, respectively.
Figure 7 shows the efficiency versus heat flow using different nanofluids. The efficiency at 17 kW/m
2 for
Al2O3,
TiO2,
Fe3O4, and graphene is 0.123, 0.124, 0.119, and 0.121, respectively. The nanofluid that offers the best efficiency is
, and compared to Fe
3O
4, which is less efficient, results in a difference of 5.04 × 10
−4.
Increasing the temperature of the cold side increases the input exergy, but the output power decreases (Equation (11)). Due to this, the exergy efficiency is expected to decrease. Therefore, the cold side temperature increases, and the exergy efficiency of the TEG of circular and triangular leg structures is reduced by up to 27%.
Figure 8 shows the exergy efficiency of the TEG for several nanofluids with hollow triangular leg structures.
The change in efficiency using different cooling nanofluids is noticeable. The increase in efficiency is 0.013 between
TiO2 and
Fe3O4, and between
TiO2 and distilled water, there is an efficiency difference of 0.05. The exergy efficiency of
TiO2 is 0.396 at 17 kW/m
2. Remember that exergy is the most valuable work obtained from a given transfer of energy. Therefore, the difference in exergy efficiency between nanofluids is significant. Using nanofluids in a TEG can improve performance by up to 20%.
Table 6 shows the maximum output power, efficiency, and exergy values for distilled water and the nanofluids used for the triangular leg structure. Compared with the work of He Ruan [
21], who used
TiO2 at 0.1%-wt and reached a maximum TEG efficiency of 6.5%, ours reaches up to 12% TEG efficiency, using a 0.1%
TiO2-wt.
The Carnot factor and the conversion efficiency are affected by the temperature of the heat source and sink as well as the height ratio (
Section 2.2). Increasing the heat source temperature T
h enhances the conversion efficiency, as mentioned, but the Carnot factor grows as well. Taking this into account, between these two conflicting factors, the outcome is determined by the dominant factor. For a specific range of height ratios with the increase of source temperature, the increase of the Carnot factor is more than the enhancement of conversion.
The difference between distilled water and nanofluids is significant since performance can be improved by up to 20%, but the difference in nanofluids is practically negligible.
Notice that the TEG performance with
Al2O3 nanofluid as coolant outperforms the TEG performance using the other four nanofluids.
Table 6 shows the numeric values for the hollow triangular leg structure with different cooling nanofluids.
Figure 9 shows the output power and energy efficiency as a function of the area ratio. The higher the area ratio, the higher the power output and efficiency, meaning the more gap, the better performance the TEG will have. Again,
TiO2 is higher compared to the other nanofluids.
3.2. TEG: Segmentation Effect
This section studies the effect of the segmented hollow/filled leg structures on the performance of the thermoelectric generator using cooling nanofluid. The segmentation materials in each leg are
Bi2Te3 and
PbTe. Each segment has a height of 0.75 mm, and the measurements are the same as those observed in
Figure 4. As in the previous sections, we consider hollow leg structures now segmented.
Figure 10 is the meshed schematic diagram for segmented leg structures.
Figure 10c shows the measurements of the segmented models. When 2n-1p segmentation is used, the n-type semiconductor is segmented in half with a length of 0.75 mm each, while the p-type semiconductor has a length of 1.5 mm.
The segmentation is 0.75 mm for all leg structures. The external area is one mm2, and the total height is 1.5 mm. The models were in the SOLIDWORKS software and were later imported to ANSYS Workbench to carry out the pertinent analyses.
Each segment typically consists of two materials with different thermoelectric properties in a segmented thermoelectric module. When a temperature gradient is applied across the module, an electrical current is generated due to the difference in the Seebeck coefficient between the two materials.
The temperature gradient is a measure of the change in temperature over distance, and its magnitude and direction can affect the performance of the thermoelectric generator. For example, if the temperature gradient is too high, it can cause thermal stresses that can damage the material. On the other hand, if the temperature gradient is too low, it may not be sufficient to generate enough electrical power.
To optimize the performance of a segmented thermoelectric module, it is important to carefully design the module’s geometry, as well as the materials used and their doping levels, to achieve the desired temperature gradients and maximize the system’s efficiency.
The of Bi2Te3 is 250 K, while that of PbTe is 400 K. The simulations must consider this since the material can be overheated and not have optimal performance results.
Four different analyses are carried out for the case of segmented thermoelements. The first is to analyze it without segmentation. The second is to segment both thermocouples in half. The third is to segment the n-type thermocouple in half and leave the p-type normal. Finally, the fourth is to segment the p-type thermocouple in half and leave the n-type normal.
The output power, energy efficiency, and exergy efficiency are calculated for these cases regarding the heat flow.
3.2.1. Segmentation Effect with Distilled Water
Figure 11 shows differences in the segmented leg structures. The power output of the segmented square leg structure is below the non-segmented triangular leg structure, which reinforces the relevance of leg structure for TEG analysis.
For the hollow leg structures, the best structures are the segmented circular and square, with a maximum efficiency of 13.222 and 13.18%, respectively, and the maximum efficiency of the segmented triangular leg structure is 13.159%. This is due to the geometry of the segmented leg structures and the materials used in the semiconductor elements.
Figure 12 shows that the segmented circular leg structure now has the highest maximum efficiency (13.125%) for solid leg structures, while the triangular structure is 13.104%.
The exergy efficiency of segmented leg structures is lower than the structures that are not segmented; additionally, the circular leg structure is the best in segmented leg structures with a maximum exergy efficiency of 0.300.
Figure 13 shows the exergy efficiency for the segmented leg structures.
In
Appendix A, the analysis for the four different segmentations is studied, and it is shown that the 2n-1p segmentation is the optimal segmentation for the performance of the TEG. It is also shown that the circular and triangular leg structures have the best performance, so they are compared with each other using
as a refrigerant. This agrees with the conclusion of Ref. [
12].
3.2.2. Segmentation Effect with Cooling Nanofluid
In this case, since in the previous results of this study TiO2 turned out to be the optimal nanofluid for TEG’s performance compared with distilled water, the output power, energy efficiency, and exergy are analyzed for hollow leg structures.
The exergy improves with nanofluids, which allows us to choose the appropriate nanofluid depending on the application.
Figure 14a shows that segmenting leg structures improves power output compared to that obtained by Min Li [
10]. Notice that the circular structure with segmentation 2n-1p has the best output power for heat flux values less than 12 kW/m
2. While after 12 kW/m
2, the triangular leg structure with 2n-1p segmentation has better power output.
Compared with Min Li’s study with a maximum output power of 0.326 W, the output power is two times better for circular 2n-1p segmentation and three times better for triangular 2n-1p segmentation for a heat flow of 17 kW/m
2 with 0.634 W and 0.907 W, respectively, as shown in
Table 7.
Figure 14b shows the output power improvement using
TiO2 nanofluids as a cooler for the circular leg structure with 2n-1p segmentation and triangular leg structure with 1n-2p segmentation. The maximum power output of these leg structures with
TiO2 is 0.744 W and 1.064 W, respectively, at a heat flux of 17 kW/m
2.
A change in the sensitivity of the parameters is shown in
Figure 14, while the results of Min Li [
10] were performed with a
20 °C, our results are performed with a
°C, the difference of the output power is perceived due to the change in the gradient. The maximum output power of Min Li [
10] is 0.326 W, while ours is 0.223 W. In other words, varying the cold side temperature by 5 °C can alter the results by up to 32%. Moreover, the difference in efficiency is minimal for this change of gradient, as expected.
Finally, it is important to remark that the power output generated by different leg structures can be inverted; for example,
Figure 14 shows that the power output of the circular structure and the triangular structure changes for a given value of heat flux. This fact is important for the use of these structures in different operating conditions. If the heat flow is very large, the performance of a structure can improve.
The 12 kW/m2 is the critical point of heat flow, it is where the parameters begin to decrease and is due to the properties of the temperature.
For heat flux below 12 kW/m2, a circular structure is better than triangular, but if the heat flux is higher than 12 kW/m2, the triangular structure is better. The performance between both structures is reversed.
Figure 15 shows how segmenting leg structures can improve thermal efficiency compared to the efficiency obtained by Min Li [
10]. For example, at 5 kW/m
2, the efficiency of Min Li is 4.6%, that of the triangular leg structure with 2n-1p segmentation is 9.111%, and that of the circular model with 2n-1p segmentation is 9.208, improving by up to 200%. For a heat flux of 12 kW/m
2, the efficiency of Min Li is 9.1%, that of the triangular leg structure with 2n-1p segmentation is 19.57%, and that of the circular leg structure with 2n-1p segmentation is 19.786%. The maximum efficiency is 11.1%, 23.864%, and 24.213%, respectively.
The maximum exergy efficiency of Min Li is 0.338, while that of the circular and triangular leg structures with 2n-1p segmentation is 0.513 and 0.507, respectively, improving up to 150%, as shown in
Figure 16a. In
Figure 16b, before 10 kW/m
2 the exergy efficiency of the segmented models is lower than that of Min Li due to the materials used in the segmentation, but the exergy efficiency improved after 12 kW/m
2. All the results are shown in
Table 7.
The improvement over the performance of the TEG is small compared with different nanofluids, nevertheless the improvement with nanofluids is bigger compared with distilled water.
The properties of the n-type material contribute to a better performance when material segmentation is considered.
Figure A1 of
Appendix A shows that the system with segmentation 2n-1p has a better performance than segmentation 1n-2p.
4. Conclusions
The performance of a TEG was studied, performing simulations on an ANSYS Workbench with the thermal-electric module. Temperature-dependent Bi2Te3 is used as the semiconductor material of the TEG.
This study focuses on the impact of different cooling nanofluids for optimizing a thermoelectric generator, evaluating its output power, efficiency, and exergy efficiency.
The energy and exergy performance of five different leg structures (square, triangular, circular, octagonal, and rectangular) were analyzed in a stationary state condition.
This study compares the performance of hollow and filled leg structures in a thermoelectric generator using distilled water and various nanofluids as coolants. The results show that the triangular leg structure performs the best in terms of power output, energy conversion, and exergy efficiency, with a maximum efficiency of 0.111 and 0.106 for the hollow and filled leg structures, respectively.
This work demonstrates the improvement in the performance of thermoelectric generators via cooling nanofluids over traditional cooling fluids.
Using nanofluids, the power output, energy conversion, and exergy efficiency improve by 12.57%, 15%, 16.53%, and 17% using Fe3O4, graphene, TiO2, and Al2O3 respectively, compared to distilled water.
It is better to use a 2n-1p segmentation to achieve a better output power because the electron charge has a greater capacity to transit around the p-type thermocouples, reaching a maximum Pout of 1.064 W. A maximum efficiency of 24.21% was achieved using a 2n-1p segmentation.
Using nanofluids in TEG systems could be a promising way to improve their efficiency and output power. However, more research is needed to investigate the impact of different nanofluids on the performance of TEGs under other operating conditions.
For future research, we strongly suggest the following:
New materials: researchers can explore and discover new materials with higher thermoelectric performance and lower cost.
Nanostructuring: research can focus on developing new manufacturing techniques that enable the fabrication of nanostructured materials for thermoelectric generators.
System-level optimization: Research can be conducted on the system-level optimization of thermoelectric generators. The research can include developing new architectures, optimizing heat transfer and thermal management, and advanced control techniques.