Effect of Weld Shape on Temperature Field of Nozzle Flowmeter
Abstract
1. Introduction
2. Numerical Model and Method
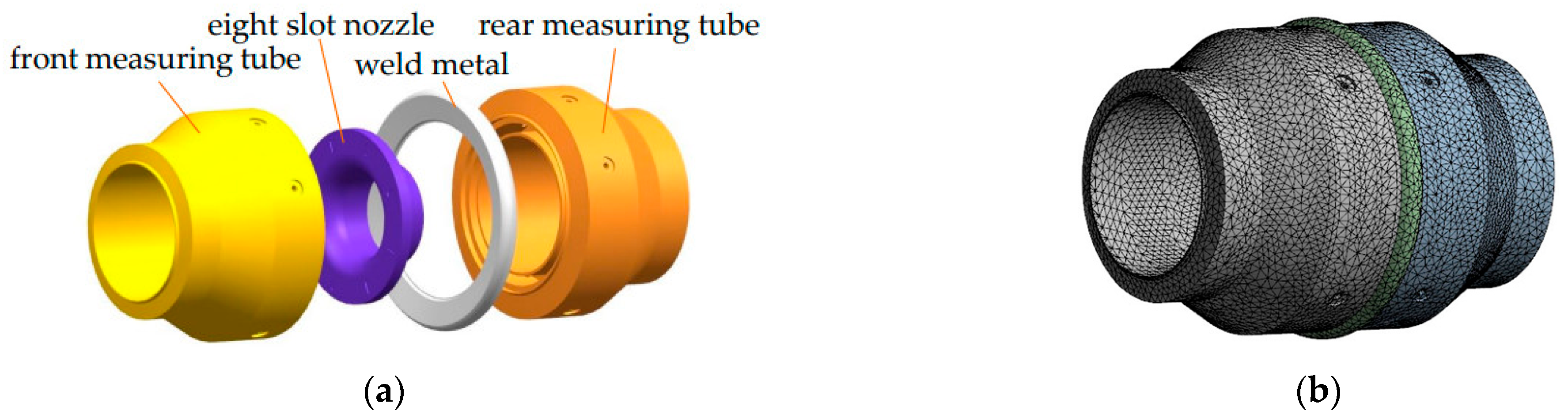
2.1. Flowmeter Model
2.2. Numerical Simulation Method
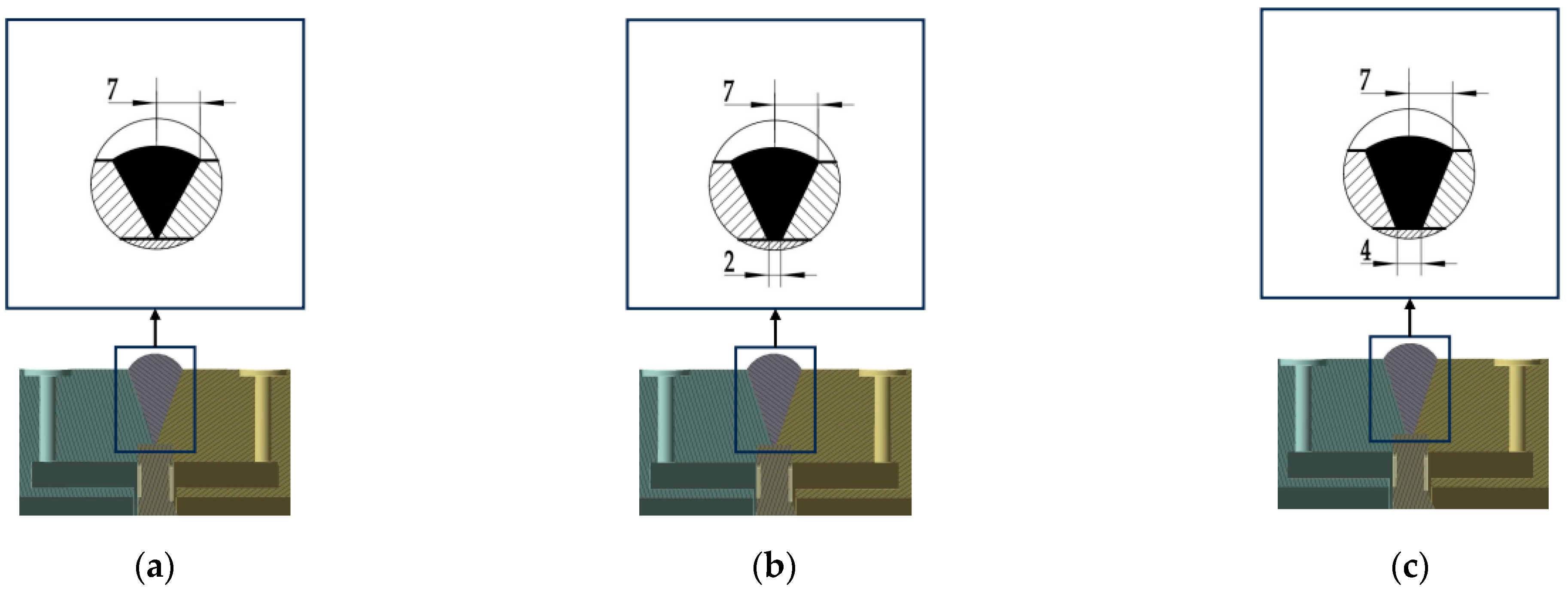
2.3. Calculation Scheme
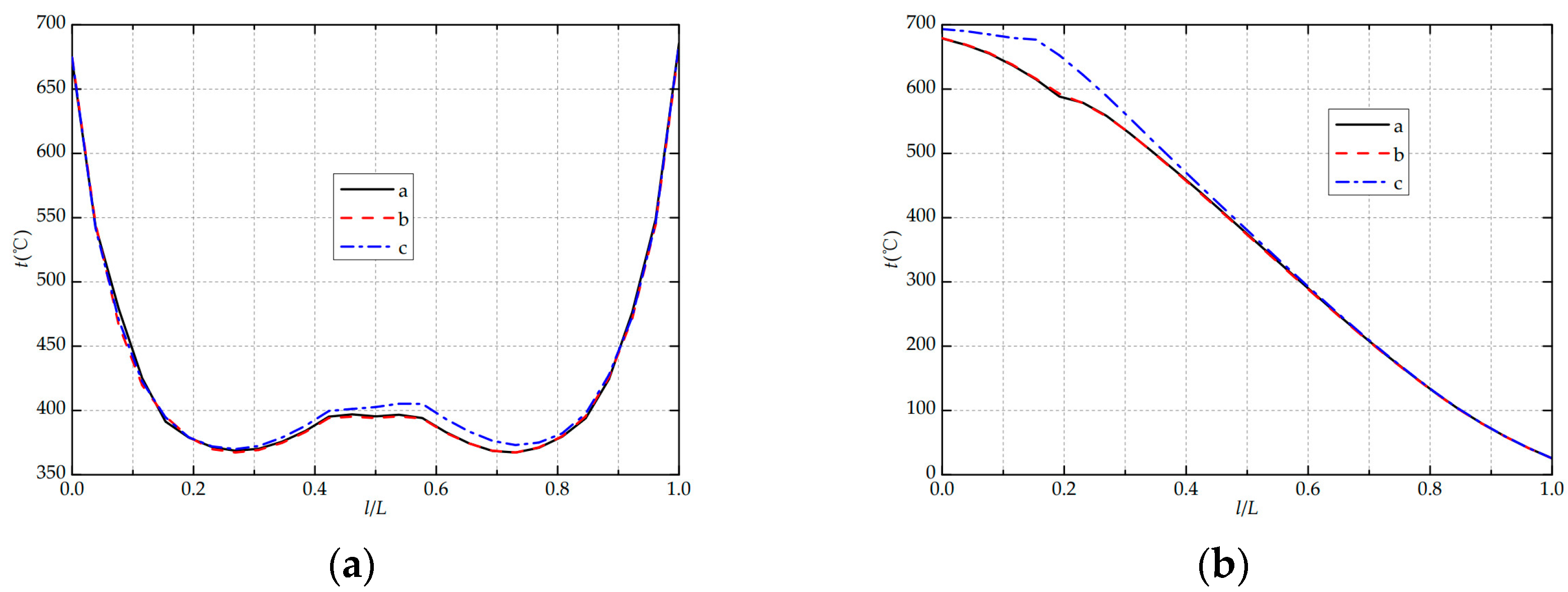
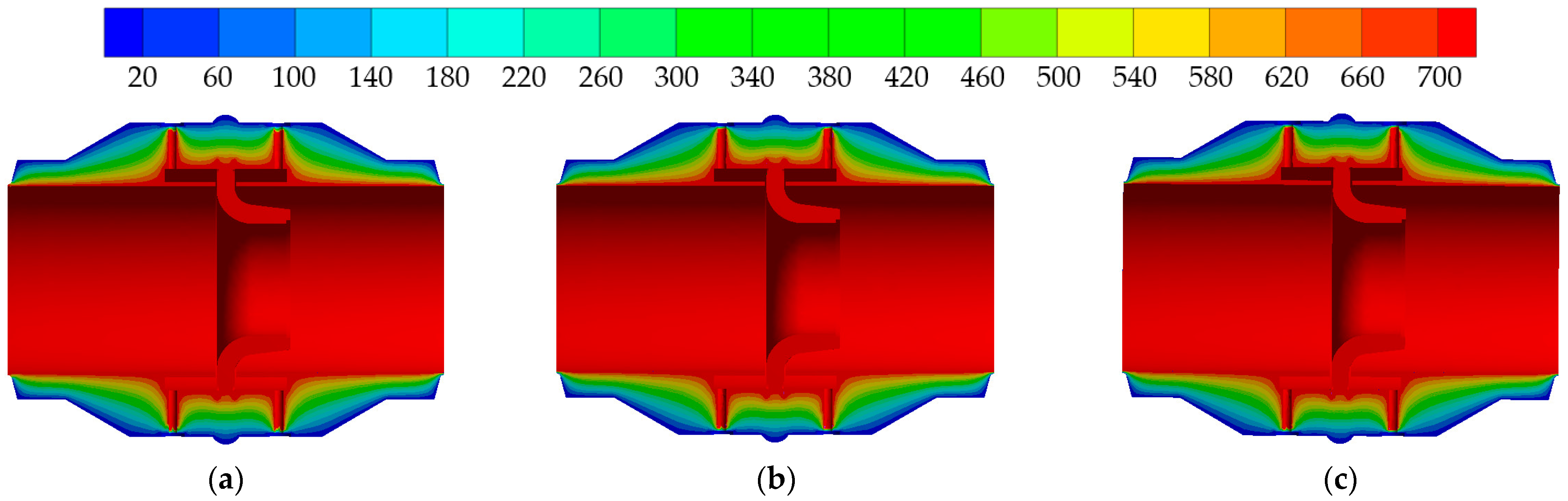
3. Analysis of Results
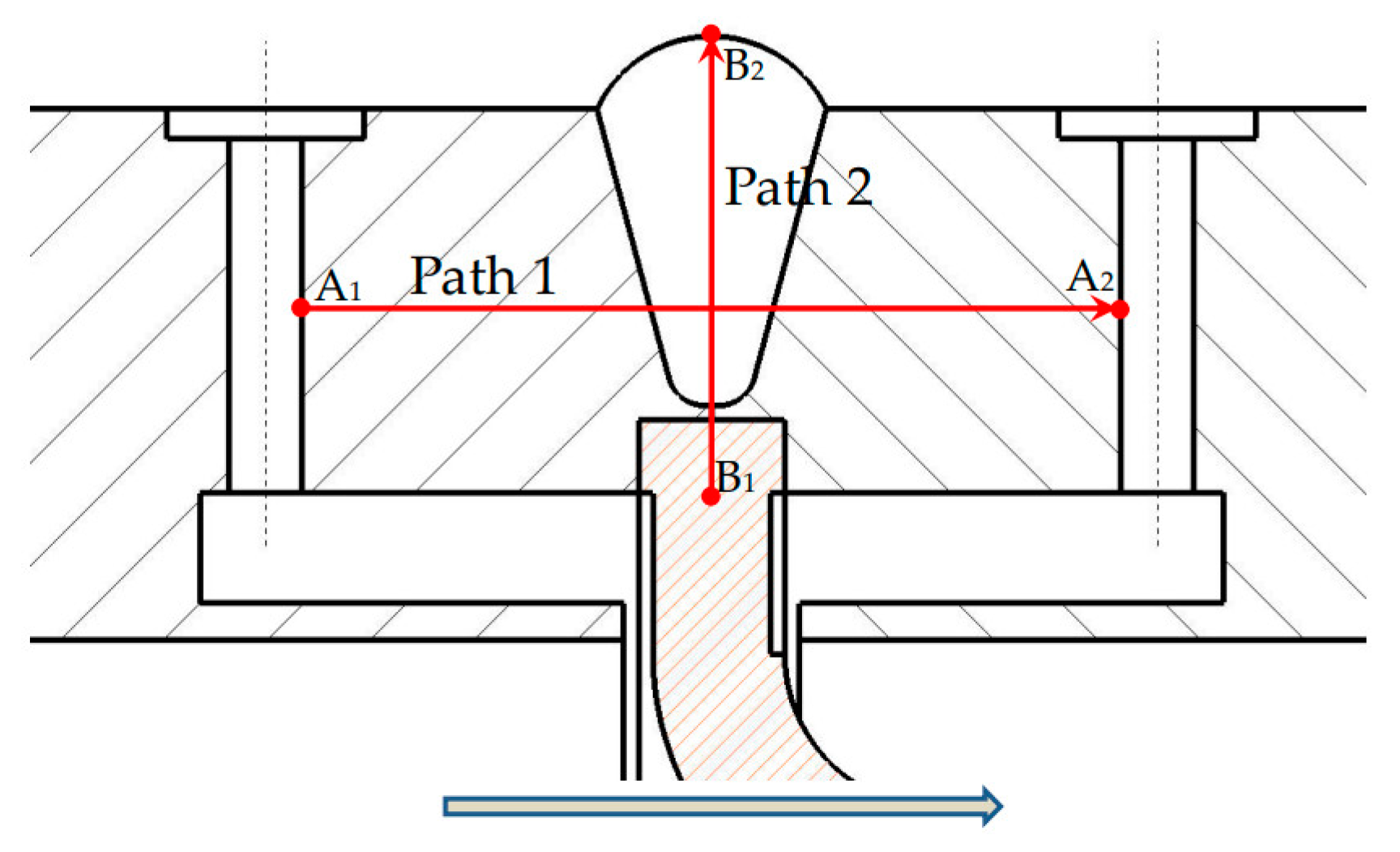
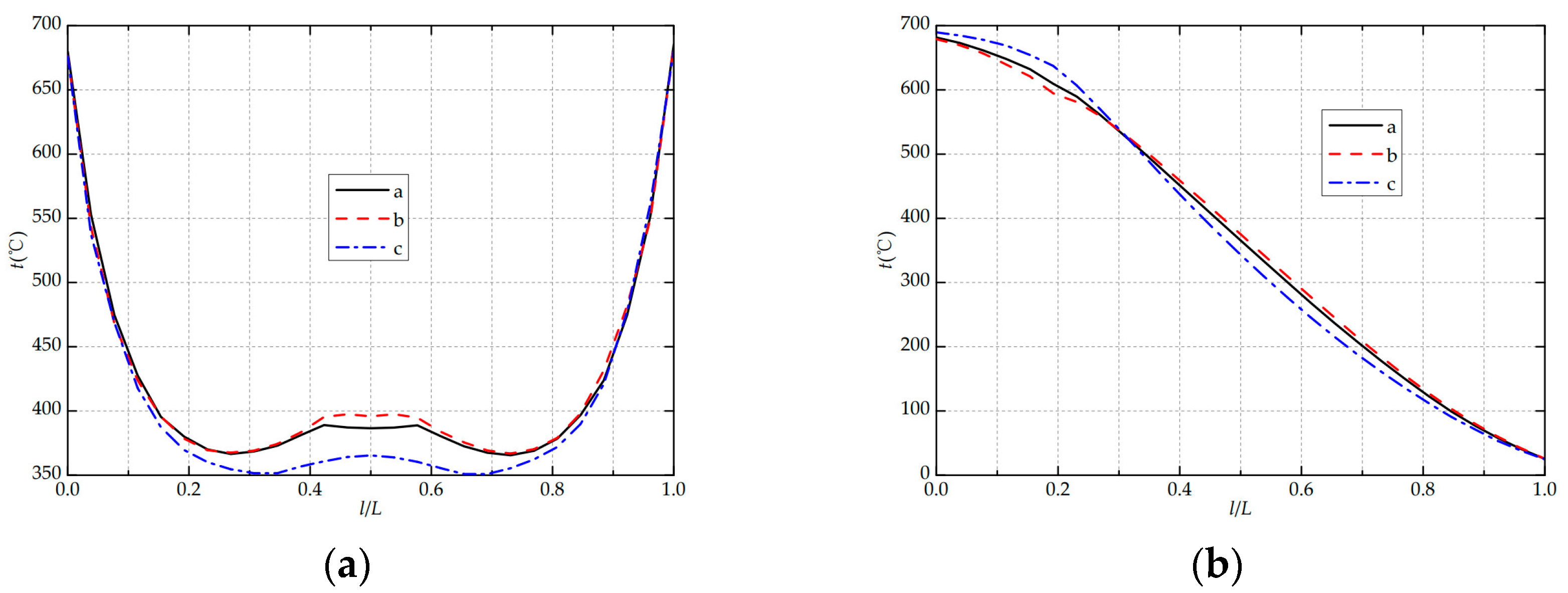
3.1. Monitoring Temperature Field
3.2. Solid Temperature Field
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Y.L.; Tong, L.H.; Xiao, J.J.; Zhang, K.Y. Influence of wall temperature on thermal effect of nozzle flowmeter. Front. Heat Mass Transf. 2022, 18, 38. [Google Scholar]
- Lua, A.C.; Zheng, Z.S. Numerical simulations and experimental studies on a target fluidic flowmeter. Flow Meas. Instrum. 2003, 14, 43–49. [Google Scholar] [CrossRef]
- Tong, L.H.; Zheng, S.L.; Zhang, Y.L.; Zhao, Y.J.; Zhang, K.Y.; Li, J.F. Study on thermal effect of nozzle flowmeter based on fluid-solid coupling method. Shock Vib. 2021, 2021, 7448439. [Google Scholar] [CrossRef]
- Tong, L.H.; Zhang, Y.L.; Yu, T.H.; Luo, B.Y.; Li, J.F. Heat flow distribution characteristics of solid wall of nozzle flowmeter. Front. Heat Mass Transf. 2023, 20, 10. [Google Scholar]
- Huang, S.F.; Ma, T.Y.; Wang, D.; Lin, Z.H. Study on discharge coefficient of perforated orifices as a new kind of flowmeter. Exp. Therm. Fluid Sci. 2013, 46, 74–83. [Google Scholar] [CrossRef]
- Kolodzie, P.A., Jr.; Van Winkle, M. Discharge coefficients through perforated plates. AIChE J. 1957, 3, 305–312. [Google Scholar] [CrossRef]
- Kim, B.C.; Pak, B.C.; Cho, N.H.; Chi, D.S.; Choi, H.M.; Choi, Y.M.; Park, K.A. Effects of cavitation and plate thickness on small diameter ratio orifice meters. Flow Meas. Instrum. 1997, 8, 85–92. [Google Scholar] [CrossRef]
- Zhang, H.; Guo, C.; Lin, J. Effects of velocity profiles on measuring accuracy of transit-time ultrasonic flowmeter. Appl. Sci. 2019, 9, 1648. [Google Scholar] [CrossRef]
- Džemić, Z.; Širok, B.; Bizjan, B. Turbine flowmeter response to transitional flow regimes. Flow Meas. Instrum. 2018, 59, 18–22. [Google Scholar] [CrossRef]
- Guo, S.N.; Sun, L.J.; Zhang, T.; Yang, W.L.; Yang, Z. Analysis of viscosity effect on turbine flowmeter performance based on experiments and CFD simulations. Flow Meas. Instrum. 2013, 34, 42–52. [Google Scholar] [CrossRef]
- Lee, W.F.Z.; Evans, H.J. Density effect and Reynolds number effect on gas turbine flowmeters. J. Basic Eng. 1965, 87, 1043–1051. [Google Scholar] [CrossRef]
- Jepson, P.; Bean, P.G. Effect of upstream velocity profiles on turbine flowmeter registration. J. Mech. Eng. Sci. 1969, 11, 503–510. [Google Scholar] [CrossRef]
- Enz, S. Effect of asymmetric actuator and detector position on Coriolis flowmeter and measured phase shift. Flow Meas. Instrum. 2010, 21, 497–503. [Google Scholar] [CrossRef]
- Shavrina, E.; Zeng, Y.; Khoo, B.C.; Nguyen, V. The Investigation of Gas Distribution Asymmetry Effect on Coriolis Flowmeter Accuracy at Multiphase Metering. Sensors 2022, 22, 7739. [Google Scholar] [CrossRef]
- Bobovnik, G.; Kutin, J.; Bajsić, I. The effect of flow conditions on the sensitivity of the Coriolis flowmeter. Flow Meas. Instrum. 2004, 15, 69–76. [Google Scholar] [CrossRef]
- Singh, S.N.; Seshadri, V.; Singh, R.K.; Singh, R. Effect of upstream flow disturbances on the performance characteristics of a V-cone flowmeter. Flow Meas. Instrum. 2006, 17, 291–297. [Google Scholar] [CrossRef]
- Ifft, S.A.; Mikkelsen, E.D. Pipe elbow effects on the V-cone flowmeter. In Proceedings of the ASME Fluid Engeering Conference, Washington, DC, USA, 26–29 October 1992. [Google Scholar]
- Singh, R.K.; Singh, S.N.; Seshadri, V. Study on the effect of vertex angle and upstream swirl on the performance characteristics of cone flowmeter using CFD. Flow Meas. Instrum. 2009, 20, 69–74. [Google Scholar] [CrossRef]
- Zheng, D.D.; Zhang, P.Y.; Xu, T.S. Study of acoustic transducer protrusion and recess effects on ultrasonic flowmeter measurement by numerical simulation. Flow Meas. Instrum. 2011, 22, 488–493. [Google Scholar] [CrossRef]
- Kang, W.; Lee, S.H.; Lee, S.J.; Ha, Y.C.; Jung, S.S. Effect of ultrasonic noise generated by pressure control valves on ultrasonic gas flowmeters. Flow Meas. Instrum. 2018, 60, 95–104. [Google Scholar] [CrossRef]
- Chen, Q.; Li, W.H.; Wu, J.T. Realization of a multipath ultrasonic gas flowmeter based on transit-time technique. Ultrasonics 2014, 54, 285–290. [Google Scholar] [CrossRef]
- Leontidis, V.; Cuvier, C.; Caignaert, G.; Dupont, P.; Roussette, O.; Fammery, S.; Nivet, P.; Dazin, A. Experimental validation of an ultrasonic flowmeter for unsteady flows. Meas. Sci. Technol. 2018, 29, 045303. [Google Scholar] [CrossRef]
- Shaaban, S. Design and optimization of a novel flowmeter for liquid hydrogen. Int. J. Hydrogen Energy 2017, 42, 14621–14632. [Google Scholar] [CrossRef]
- Oh, S.T.; Kim, Y.I. Development of a portable, small-scale multi-nozzle flowmeter with exit pressure control. ASHRAE Trans. 2017, 123, 254. [Google Scholar]
- Chen, J.F.; Zhang, K.; Wang, L.Y.; Yang, M.Y. Design of a high precision ultrasonic gas flowmeter. Sensors 2020, 20, 4804. [Google Scholar] [CrossRef] [PubMed]
- Huang, Z.X. ANSYS Workbench Handbook 16.0; People Post Press: Beijing, China, 2016. [Google Scholar]









| Materials | Density (kg/m3) | Isotropic Thermal Conductivity (W/(m·K)) | Specific Heat (J/(kg·K)) |
|---|---|---|---|
| 12Cr1MoV | 7860 | 60.5 | 434 |
| Structural Steel | 7850 | 60.5 | 434 |
| Tin | 7304 | 64 | 226.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Tong, J.; Zhu, Z. Effect of Weld Shape on Temperature Field of Nozzle Flowmeter. Processes 2023, 11, 2164. https://doi.org/10.3390/pr11072164
Zhang Y, Tong J, Zhu Z. Effect of Weld Shape on Temperature Field of Nozzle Flowmeter. Processes. 2023; 11(7):2164. https://doi.org/10.3390/pr11072164
Chicago/Turabian StyleZhang, Yuliang, Jiangbo Tong, and Zuchao Zhu. 2023. "Effect of Weld Shape on Temperature Field of Nozzle Flowmeter" Processes 11, no. 7: 2164. https://doi.org/10.3390/pr11072164
APA StyleZhang, Y., Tong, J., & Zhu, Z. (2023). Effect of Weld Shape on Temperature Field of Nozzle Flowmeter. Processes, 11(7), 2164. https://doi.org/10.3390/pr11072164






